1.
Introduction
Neural networks (NNs) serve as computational models that replicate the neural system of the human brain, and they are applied to address diverse problems in the field of machine learning. NNs have been widely used in various fields, including natural language processing, picture recognition, image encryption, wireline communication, finance, and business forecasting, because of its strong information processing capabilities (see [1,2,3,4,5,6,7,8]). Therefore, the stability analysis of NNs is a crucial matter, and has received a lot of attention in recent years (see [9,10,11]). Furthermore, the transmission of signals between neurons is subject to time-delay, which can adversely affect the performance of NNs ([12,13]). Consequently, determining the maximum allowable delay bounds (MADBs) that can ensure the stability of NNs is an important research topic that has drawn a lot of attention [14]. In the existing literature, the method of delay partitioning is commonly employed for analyzing time-delay systems. In order to obtain the MADBs, on the one hand, it is necessary to require that the constructed augmented Lyapunov-Krasovskii functional (LKF) contains more delay information. On the other hand, it is necessary to relax the requirements on the matrix variables involved. The research [15] introduced a novel asymmetric LKF, where all matrix variables involved do not need to be symmetric or positive definite. To make the augmented LKFs contain more delay information, a novel approach to delay partitioning was presented by Guo et al. [16], which involves dividing the variation interval of the delay into several subintervals. A new method for determining the negativity of a quadratic function is presented in [17], based on its geometric information. A more thorough reciprocity convex combination inequality was used by Chen et al. [18] to add quadratic terms to the time derivative of a LKF. It leads to less stringent stability conditions for delayed neural network (DNNs). A novel approach to free moving points generation was introduced in [19] based on the work of [18]. Specifically, free moving points were established for synchronous movements in each subinterval. In addition, the integral inequalities can reduce conservatism by providing tighter bounds through replacement of a function with its upper or lower limit, improving our ability to predict actual results.
As previously discussed, the majority of existing research has focused on the negative condition of LKFs. However, there is a lack of investigation into its positive condition in the literature. The main work of this paper is to construct a relaxed LFK, and study the stability properties of DNNs by using a quadratic function positive definiteness method. The main contributions are summarized as follows:
(1) Distinct from prevailing methodologies, this paper presents a novel approach for demonstrating the positive definiteness of the LKF, based on the requirement that the quadratic function satisfies the positive definite condition.
(2) By employing the asymmetric LKFs methodology, we construct a relaxed LKF that incorporates delay information. The matrix variables included in this method do not require symmetry and positive definiteness.
(3) A new delay-dependent stability criterion with reduced conservatism is derived for DNNs by extending basic inequalities and incorporating the conditions of positive definiteness for the quadratic function.
Notations: Y is an n×n real matrix; YT is transpose of Y and Y>0; (Y<0) represents the positive definite (negative definite) matrix. The ∗ is a symmetric block in a symmetric matrix, He{Y}=Y+YT. The diagonal matrix is denoted by diag{}. The n-dimensional Euclidean space is denoted by Rn and Rn×nis the set of all n×n real matrices.
2.
Preliminaries
Consider the following NNs with time-varying delay:
where
is the neuron state vector and ρ(t) is the initial condition.
denotes the activation functions.
with ai>0. B and C are the connection matrices. The hτ(t) is the time-varying delay differentiable function that satisfies 0≤hτ(t)≤h, ˙hτ(t)≤μ, where h and μ are known constants. To derive our primary outcome, we need to rely on the following assumption and lemmas.
Assumption 2.1. The Lipschitz condition that the neuron activation function satisfies is as follows:
where ι−i and ι+i are known constants. For simplicity, denote the following matrices:
Lemma 2.1. [20] Given any constant positive definite matrix K∈Rn×n, for any continuous function χ(u) and v1<v2, the following inequalities hold:
Lemma 2.2. [21] Given any constant positive definite matrix K∈Rn×n, for any continuous function χ(u) and v1<v2, the following inequalities hold:
where
Lemma 2.3. [22] Let R=RT∈Rn×n be a positive definite matrix. If there exists matrix X∈Rn×n such that
then the following inequality holds:
where
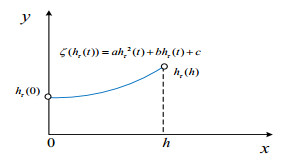
Lemma 2.4. For a quadratic function of delay,
where a,b,c∈R,hτ∈[0,h], ξ(hτ)>0 holds, if ξ(hτ) satisfies:
Proof. We will prove Lemma 2.4 by the geometry approach.
● For a>0: ξ(hτ(t)) is a convex function. When ξ(hτ(t)) increases monotonically in [0,h], ξ(0)>0 will make ξ(hτ(t))>0 (see Figure 1); when ξ(hτ(t)) is monotonically decreasing in [0,h], if ξ(h)>0, then ξ(hτ(t))>0 (see Figure 2); when ξ(hτ(t)) is not monotonically increasing or decreasing in [0,h], D is the intersection of the two tangents at ξ(0) and ξ(h); if D>0, then ξ(hτ(t))>0 (see Figure 3).
● For a<0: ξ(hτ(t)) is a concave function. ξ(hτ(t))>0 in [0,h] if ξ(0)>0 and ξ(h)>0 (see Figure 4).
□
Through the above discussion, we obtained three conditions for positive definiteness of quadratic functions. In Theorem 3.1, we constructed a quadratic function form of LKFs, and under the condition of satisfying these three conditions, we can prove that LKF is positive definite.
Remark 2.1. Lemma 2.3 is a formula derived from the Bessel-Legendre integral inequality, which provides a varying estimate based on N that can help us to evaluate the upper bound of ∫β1β3˙χT(μ)R˙χ(μ). It is apparent that Lemma 2.3 can be reduced to Lemma 2.1 when N=0 (see [23]). In [17,18,19], the negative definiteness criterion of a quadratic function is utilized to demonstrate the negativity of the derivative of the LKFs. At present, there is no research that explores the use of quadratic function methods for determining the positive-definiteness property of LKFs. In this paper, the Lemma 2.4 is a condition for a quadratic function to be positive definite. In Theorem 3.1, the h2τ(t) term is introduced in the augmented asymmetric LKFs through the integral inequalities. On the one hand, introducing h2τ(t) can include more time delay information in the LKFs and reduce conservatism. On the other hand, it can make the LKFs a quadratic function.
3.
Main results
The symbols used in the theorem are described here to help clarify its formulation.
Theorem 3.1. For given scalars μ and h>0, system (2.1) with time-varying delay is asymptotically stable if there exist positive definite symmetric matrices W1, W2; positive definite diagonal matrices Z1, Z2; positive definite matrices R1, R2, Q1, Q2; symmetric matrices P1; and any appropriate dimension matrices P2, P3, M, N, F, and P=[P1,2P2,2P3], such that the following linear matrix inequalities (LMIs) hold:
where
Proof. Consider the following candidate LKF for system (2.1):
where
By Lemmas 2.1 and 2.2, we can deduce
From the above derivation, we can conclude
The LKF (3.3) is positive definite if
Next we need to derive that the derivative of LKF is negative definite. Taking the time-derivative of LKF, we have
Applying inequalities from Lemmas 2.1–2.3, we can obtain
where
Furthermore, based on Assumption 2.1, the following condition holds for any positive definite diagonal matrices Z1 and Z2:
For any matrices M and N, from the Newton-Leibniz integral formula, we can obtain that:
then,
By adding the (3.4)–(3.7) together, we can obtain
Therefore, the proof has been completed. □
Remark 3.1. The purpose of constructing an augmented LKF is to extract more information from the system. By introducing new variables and parameters, the augmented LKF can describe the dynamic characteristics of the system in greater detail, helping us to better understand and analyze system behavior. Typically, in order to satisfy the stability conditions of an augmented LKF, the matrix variables involved need to be positive definite and symmetric. This is because in control theory, positive definite matrices and symmetric matrices have good properties that can ensure the nonnegativity and convexity of the LKF [24]. When requiring all matrix variables in the designed augmented LKFs to be positive definite and symmetric, it may lead to increased conservatism. This is because the restrictions of positive definiteness and symmetry narrow down the set of available LKFs, possibly failing to capture all system dynamics.
Remark 3.2. Inspired by [15], a relaxed and asymmetric LKF is constructed in this paper. The involved matrix variables do not require them to be all positive definite or symmetric in this LKF. By utilizing the condition that the quadratic function is positive definite, the proposed Lemma 2.4 ensures the positive definiteness of the LKF. Furthermore, when combined with certain extended fundamental inequalities, Theorem 1 is less conservative compared to some of the existing literature.
4.
Numerical example
This section uses a numerical example to demonstrate the feasibility of the proposed approach.
Example 4.1. Consider DNNs (2.1), with the following system parameters:
Solving the LMI in Theorem 3.1 yields the MADBs. Table 1 shows the MADBs of Example 1 with various μ by the obtained Theorem 3.1. Compared to some recent results in other literature both theoretically and numerically. It is undeniably established that this paper's results are significantly better than some reported. Based on the data presented in Table 1, the MADBs system (2.1) yields a value of 11.8999 for μ = 0.4.
In addition, we use different initial values (x1(0)=col[0.5,0.8],x2(0)=col[−0.2,0.8]) and
to obtain the state trajectory of the system (2.1). The graph of state trajectories show that all state trajectories ultimately converge to the equilibrium point, albeit with varying time requirements (Figures 5–8). Finally, numerical simulation results show that our proposed method is effective and the new stability criterion obtained is feasible.
5.
Conclusions
The main focus of this study is on the stability analysis of NNs with time-varying delays. To improve upon existing literature, this paper has proposed a quadratic method for proving the LKF positive definite. A relaxed LKFs has been constructed based on this method, which contains more information about the time delay and allows for more relaxed requirements on the matrix variables. Using LMIs, a new stability criterion with lower conservatism has been derived. These improvements make the stability criteria applicable in a wider range of scenarios. The numerical examples illustrate the feasibility of the proposed approach.
Use of AI tools declaration
Throughout the preparation of this work, we utilized the AI-based proofreading tool "Grammarly" to identify and correct grammatical errors. Subsequently, we thoroughly examined and made any additional edits to the content as required. We take complete responsibility for the content of this publication.
Acknowledgments
This work was supported by the National Natural Science Foundation of China under Grant (No. 12061088), the Key R & D Projects of Sichuan Provincial Department of Science and Technology (2023YFG0287 and Sichuan Natural Science Youth Fund Project (No. 24NSFSC7038).
Conflict of interest
There are no conflicts of interest regarding this work.









 DownLoad:
DownLoad: