Evans model for dynamic economics revised

1.   Introduction

In 1924, Evans ^[1] suggested a dynamical model for monopoly, and the economic dynamics was then born ^[2,3,4,5]. Evans was a mathematician, his PhD thesis was on Volterra's integral equations, which are now widely applied to various engineering problems ^[6], and he had a chance to work with the famous Italian mathematician, Vito Volterra (May 5, 1860~October 11, 1940), from 1910 to 1912. In 1920, Evans turned his interest to economics ^[3], and his theory shed a promising and challenging light in dynamic economics. Paul A. Samuelson (May 15, 1915~December 13, 2009) ^[7,8,9,10], a famous American economist, further developed Evans theory, and obtained the Nobel Prize in Economic Sciences in 1970 due to his contribution to the dynamic economics.

Evans ^[1] took for granted the following quadratic cost function

and a well-defined market demand function,

where C is the cost function, Q is the demand function, P is the price, ${a_i}$(i = 0, 1, 2) and ${b_i}$(i = 0, 1, 2) are constants.

The profit is then obtained as

Here R = PQ is the gross income function. The Evans model is to maximize the profit at a period of T ^[1]:

The Evans model is to choose an optimal path among all admissible paths from an initial time to a terminal time. A detailed introduction to mathematical economics is available in ^[11,12], where complex economic problems are explained using simple mathematical concepts.

2.   Two-scale price dynamics

In Eq (2), the demand function depends upon the price dynamics by assuming that the price changes smoothly, however, the price change is discontinuous and a fractal modification is needed ^[13]. Fractal models can describe many discontinuous problems using a two-scale fractal derivative ^[14,15,16].

2.1.   Two-scale dynamic economics

The initial concept for the two-scale economic theory was suggested in 2020 ^[17], which was to bridge the macro-economics and the micro-economics using two different scales. The two-scale theory argues that any economic phenomena should be described by the two different scales so that a more reasonable decision can be made than those by a single scale.

Beginning with the two-scale dynamic model, we introduce first a famous Chinese economist, Ma Yin-Chu (June 24, 1882~May 10, 1982), his population theory led to China's family planning in a period from 1980 to 2016, that was a couple had only one child. The family planning became a basic state policy in China for many years. Ma concluded that China's population grew exponentially, and there would be a serious population explosion if no family planning was carried out. Ma's population model can be written as

where x is the population, k is the population growth ratio, ${x_0}$ is the initial population. Eq (5) leads to the following result

Ma's assumption is correct for a large scale, but when the measured scale becomes smaller, the population grows in a zig-zag form, so the population is not a smooth function of time, $x(t)$, actually it is non-differentiable with respect to time, the change path is similar to a coastline, which can be modelled by the fractal theory ^[18,19]. When a man walks along the coastline, its motion property depends on the fractal dimension of the coastline and the man's step length. Any discontinuity within the step length is ignored. We assume that the step length is $\Delta x$, if there is a groove with a width less than $\Delta x$, then the groove is ignored. However, when the groove's width is larger than $\Delta x$, the man has to walk along the groove's boundary to the opposite point. Similar to the coastline motion, the price can be expressed as ^[20]

where $\alpha$ is the two-scale fractal dimension of time ^[20], $\Delta t$ is the minimal time scale to measure the price change.

As an illustrative example, we consider the change of a share price within one year. If we measure the change using a scale of one year, we can assume that the price changes linearly and smoothly. If we observe its change using a scale of a quarter, it is mixed, and has a simple zig-zag path. If the scale reduces further, the price change becomes totally non-differentiable. So the prediction of the share price depends upon the measured scale and the path's fractal dimension.

When the measure scale is fixed, the price can be expressed as

A modification of Eq (6) can be written as

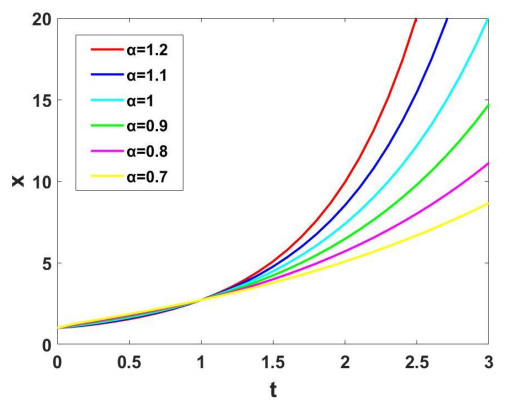
It is obvious that the fractal dimension affects greatly the population as illustrated in Figure 1.

2.2.   Two-scale price dynamics

Considering the price is non-differential with t, but it is differential with respect to ${t^\alpha }$ for given time scale, so Eq (2) can be modified as

where the fractal derivative is defined as ^[20]

The gamma function is defined as $\Gamma {\rm{(1 + }}\alpha {\rm{) = }}\int_0^\infty {{t^\alpha }} {e^{ - t}}dt$, when$\alpha$is a natural number, $\Gamma {\rm{(1 + }}\alpha {\rm{) = 1}} \times {\rm{2}} \times {\rm{3}} \times \cdots \times \alpha {\rm{ = }}\alpha {\rm{!}}$. The fractal derivative is now widely used to model various discontinuous problems ^{[21,22,23,24,25,26,27,28,29]}.

In order to maximize the profit, the fractal variational theory ^[30] has to be used. Now fractal variational principle is a hot topic in mathematics and engineering ^{[31,32,33,34]}. The fractal variational principle for the maximal profit becomes

Taking the first-order variation to Eq (12), we have

According to Eq (10), the first-order variation of Q is

Substituting Eq (14) into Eq (13) results in

We consider the following initial and terminal conditions

where P₀ and P_T are constants. So we have

Setting the first-order variation of J to zero, we have

We, therefore, obtain the following Euler-Lagrange equation

or

In view of Eq (10), we have

Simplifying Eq (23) results in

where

Eq (24) with boundary conditions of Eqs (16) and (17) can be easily solved, which reads

where m and n are constants which can be determined by the boundary conditions of Eqs (16) and (17):

3.   Quadratic-cubic cost function

Evans suggested a variational theory for dynamical economics in 1924 ^[1], which is to maximize the profit at the period of T. Using the Lagrange multiplier method ^[35], the constraints of Eqs (1) and (2) can be eliminated, the augmented variational functional is

where $\eta, \, \, \mu$ are Lagrange multipliers. The stationary conditions with respect to P, Q and C are, respectively, as follows

From Eqs (32) and (33), we have

So Eq (31) becomes

In view of Eq (1), we obtain the following differential equation for P:

This is a linear differential equation, and its solution can be easily obtained for the given boundary conditions P(0) = P₀ and P(T) = P_T.

3.1.   Quadratic-cubic cost function

The quadratic cost function leads to a linear differential equation given in Eq (36), generally the cost function can be expressed as

This will result in a complex nonlinear differential equation. Here we use a quadratic-cubic cost function to show the derivation process.

The variational principle becomes

Using the Lagrange multiplier method, we have

where $\lambda$ is the Lagrange multiplier. The stationary conditions are

From Eq (42), the multiplier can be identified as

Eq (41) becomes

Replacing Q in Eq (44) by Eq (2), we can obtain a differential equation of P.

The Lagrange multiplier method always works, but sometimes it might fail, see detailed discussion in ^[35]. We can also obtain Eq (18) without using the Lagrange multiplier. The Euler-Lagrange equation can be obtained from the following equation

From Eq (2), we have

Substituting Eq (46) into Eq (45) gives

In the above derivation, we use$\delta P(0) = \delta P(T) = 0$. From Eq (47), we obtain the following equation

which is exactly same as Eq (44).

Submitting Eq (2) into Eq (44), we obtain the following nonlinear differential equation:

Simplifying Eq (49) leads to following one

where the coefficients are given as

This equation with given initial and terminal conditions, P(0) = P₀ and P(T) = P_T, can be approximately solved by either the variational iteration method ^[36,37] or the homotopy perturbation method ^{[38,39,40,41,42,43,44,45]}.

3.2.   Nonlinear demand function

In this paper we consider the following nonlinear demand function

where ${b_{ij}}$ (i = 0, 1; j = 0, 1) are constants.

The first variation of Q is

Substituting Eq (53) into Eq (47) results in

Proceeding a similar way as above, we can obtain the following Euler-Lagrange equation

Solving the nonlinear system of Eqs (52) and (55), we can find the solution of P and Q.

4.   Discussion and conclusions

The one child policy was mathematically correct according to Ma Yinchu's population theory as discussed above, however, Ma's basic assumption used a large scale and a smooth population growth was obtained, however it becomes invalid for a smaller scale, and various debates arose ^{[46,47,48,49]}. As an illustrative example, we consider a seedling's growth. When we measure its height every year, it grows exponentially, however its prediction deviates greatly, and its error becomes infinitely large when time tends to infinity. This deviation arises in its large-scale observation. When we measure its height every hour, we can find it stops growing at the night period; if we use a scale of a month, we find it stops growing during winter. So the law for the seedling's growth is scale-dependent, any a law based on a single scale assumption will derivate remarkably when observed on a different scale.

Now we turn to Ma's population theory, it has sparked furious uproars. In view of the two-scale theory, its findings must be flawed on a different scale. The two-scale economics can avoid many unnecessary debates.

To be concluded, this paper, for the first time ever, suggests the basic concepts for a promising two-scale economic theory, all economic laws are scale-dependent. A decision based on a single-scale economic law will lead to a large derivation on a different scale, two scales are a must for analysis of many economic phenomena, and seeing with a single scale is always unbelieving ^{[50,51,52,53]}.

Generally the cost function can be written as

and the demand function can be expressed as

This paper shows that the variational principle can deal with complex economic problems with ease. An oversimplification assumption might lead to a simple equation as given in Eq (36), but it might lose some important facts for future prediction. This paper provides economists with a powerful mathematical tool to analysis of complex economic problems.

Acknowledgments

H. M. Sedighi is grateful to the Research Council of Shahid Chamran University of Ahvaz for its financial support (Grant No. SCU.EM99.98).

Conflict of interest

This work does not have any conflicts of interest.