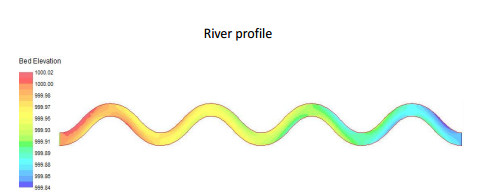
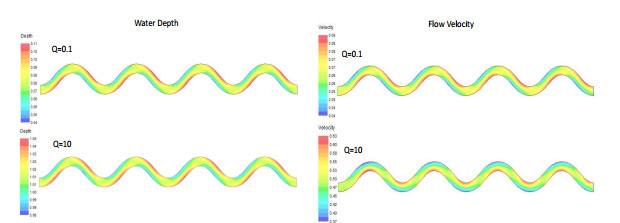
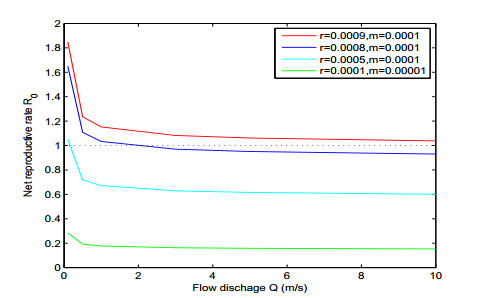
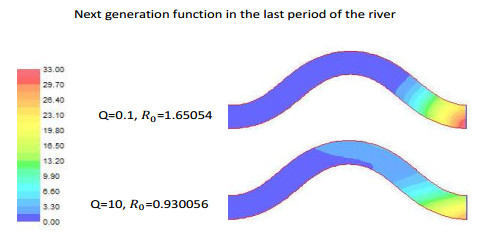
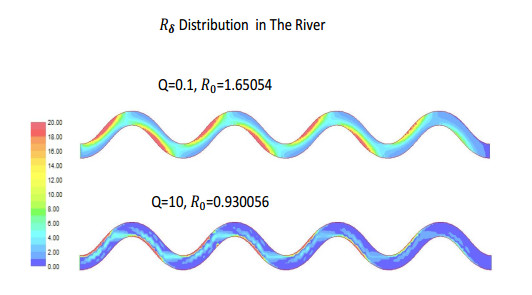
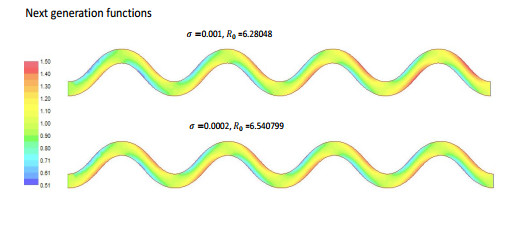
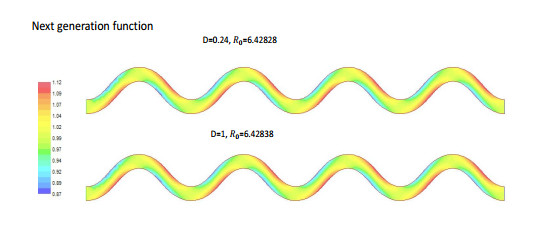
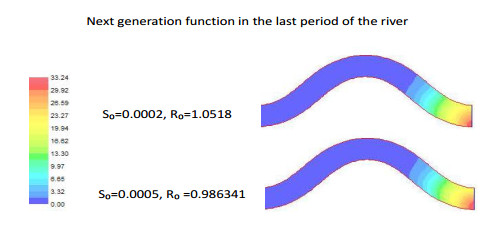
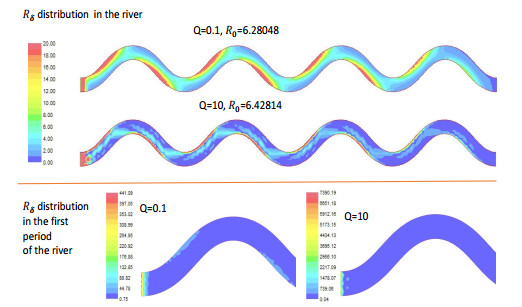
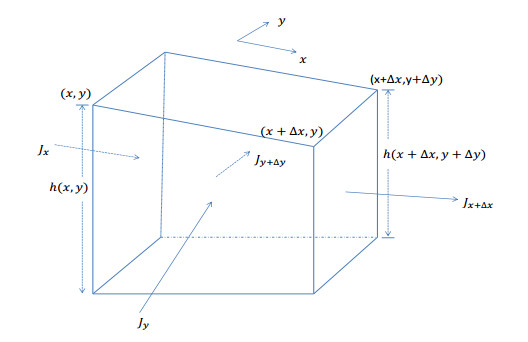
|
[1]
|
J. D. Allan, Stream Ecology: Structure and Function of Running Waters, Chapman & Hall, London, 1995.
|
|
[2]
|
K. E. Anderson, L. R. Harrisonb, R. M. Nisbet, et al. Modeling the influence of flow on invertebrate drift across spatial scales using a 2D hydraulic model and a 1D population model, Ecol. Model., 265 (2013), 207-220. doi: 10.1016/j.ecolmodel.2013.06.011

|
|
[3]
|
K. E. Anderson, A. J. Paul, E. McCauley, et al. Instream flow needs in streams and rivers: The importance of understanding ecological dynamics, Front. Ecol. Environ., 4 (2006), 309-318. doi: 10.1890/1540-9295(2006)4[309:IFNISA]2.0.CO;2

|
|
[4]
|
J. D. Armstrong, P. S. Kemp, G. J. A. Kennedy, et al. Habitat requirements of Atlantic salmon and brown trout in rivers and streams, Fish. Res., 62 (2003), 143-170. doi: 10.1016/S0165-7836(02)00160-1

|
|
[5]
|
K. E. Bencala, R. A. Walters, Simulation of solute transport in a mountain poop-and-riffle stream: A transient storage model, Water Resour. Res., 19 (1983), 718-724. doi: 10.1029/WR019i003p00718

|
|
[6]
|
D. J. Booker, Hydraulic modelling of fish habitat in urban rivers during high flows, Hydrol. Process., 17 (2003), 577-599. doi: 10.1002/hyp.1138

|
|
[7]
|
A. N. Brooks, T. J. R. Hughes, Steamline upwind/Petrov-Galerkin formulations for convection dominated flows with particular emphasis on the imcompressible Navier-Stokes equations, Comput. Method. Appl. Mech. Eng., 32 (1982), 199-259. doi: 10.1016/0045-7825(82)90071-8

|
|
[8]
|
N. J. Clifford, O. P. Harmar, G. Harvey, et al. Physical habitat, eco-hydraulics and river design: A review and re-evaluation of some popular concepts and methods, Aquat. Conserv., 16 (2006), 389-408. doi: 10.1002/aqc.736

|
|
[9]
|
J. M. Cushing, Y. Zhou, The net reproductive value and stability in matrix population models, Nat. Resour. Model., 8 (1994), 297-333. doi: 10.1111/j.1939-7445.1994.tb00188.x

|
|
[10]
|
D. L. DeAngelis, M. Loreaub, D. Neergaardc, et al. Modelling nutrient-periphyton dynamics in streams: The importance of transient storage zones, Ecol. Model., 80 (1995), 149-160. doi: 10.1016/0304-3800(94)00066-Q

|
|
[11]
|
MIKE 21 Flow Model FM: Particle Tracking Module, User Guide, Horsholm, Denmark, 2011, pp. 56.
|
|
[12]
|
MIKE 21 Flow Model FM: Particle Tracking Module, Step-by-step training guide, Horsholm, Denmark, 2011, pp. 48.
|
|
[13]
|
J. M. Elliott, Time spent in the drift by downstream-dispersing invertebrates in a Lake District stream, Freshwater Biol., 47 (2002), 97-106. doi: 10.1046/j.1365-2427.2002.00784.x

|
|
[14]
|
L. Gallien, T. Münkemüller, C. H. Albert, et al. Predicting potential distributions of invasive species: Where to go from here?, Divers. Distrib., 16 (2010), 331-342. doi: 10.1111/j.1472-4642.2010.00652.x

|
|
[15]
|
A. Ghanem, P. M. Steffler, F. E. Hicks, et al. 1995, Dry area treatment for two-dimensional finite element shallow flow modeling, Proceeding of the 12th Canadian Hydrotechnical Conference, Ottawa, Ontario, June, 1995, pp.10.
|
|
[16]
|
A. Ghanem, P. M. Steffler, F. E. Hicks, et al. Two dimensional finite element model for aquatic habitats, Water Resources Engineering Report 95-S1, Department of Civil Engineering, University of Alberta, 1995, pp. 189.
|
|
[17]
|
R. Guenther, J. Lee, Partial Differential Equations of Mathematical Physics and Integral Equations, Dover, New York, 1996.
|
|
[18]
|
J. W. Hayes, N. F. Hughes, L. H. Kelly, Process-based modelling of invertebrate drift transport, net energy intake and reach carrying capacity for drift-feeding salmonids, Ecol. Model., 207 (2007), 171-188. doi: 10.1016/j.ecolmodel.2007.04.032

|
|
[19]
|
F. M. Hilker, M. A. Lewis, Predator-prey systems in streams and rivers, Theor. Ecol., 3 (2010), 175-193. doi: 10.1007/s12080-009-0062-4

|
|
[20]
|
Q. Huang, Y. Jin, M. A. Lewis, R0 Analysis of a Benthic-Drift Model for a Stream Population, SIAM J. Appl. Dyn. Syst., 15 (2016), 287-321. doi: 10.1137/15M1014486

|
|
[21]
|
I. Ibanez, E. Gornish, L. Buckley, et al. Moving forward in global-change ecology: Capitalizing on natural variability, Ecol. Evol., 3 (2013), 170-181. doi: 10.1002/ece3.433

|
|
[22]
|
Y. Jin, F. M. Hilker, P. M. Steffler, et al. Seasonal invasion dynamics in a spatially heterogeneous river with fluctuating flows, Bull. Math. Biol., 76 (2014), 1522-1565. doi: 10.1007/s11538-014-9957-3

|
|
[23]
|
Y. Jin, M. A. Lewis, Seasonal influences on population spread and persistence in streams: Critical domain size, SIAM J. Appl. Math., 71 (2011), 1241-1262. doi: 10.1137/100788033

|
|
[24]
|
Y. Jin, M. A. Lewis, Seasonal influences on population spread and persistence in streams: Spreading speeds, J. Math. Biol., 65 (2012), 403-439. doi: 10.1007/s00285-011-0465-x

|
|
[25]
|
M. Krkosěk, M. A. Lewis, An R0 theory for source-sink dynamics with application to Dreissena competition, Theor. Ecol., 3 (2010), 25-43. doi: 10.1007/s12080-009-0051-7

|
|
[26]
|
J. Lancaster, B. J. Downes, Linking the hydraulic world of individual organisms to ecological processes: Putting ecology into ecohydraulics, River Res. Appl., 26 (2010), 385-403. doi: 10.1002/rra.1274

|
|
[27]
|
L. B. Leopold, W. B. Langbein, River Meanders, Sci. Am., 214 (1966), 60-73.
|
|
[28]
|
F. Lutscher, M. A. Lewis, E. McCauley, Effects of heterogeneity on spread and persistence in rivers, Bull. Math. Biol., 68 (2006), 2129-2160. doi: 10.1007/s11538-006-9100-1

|
|
[29]
|
F. Lutscher, R. M. Nisbet, E. Pachepsky, Population persistence in the face of advection, Theor. Ecol., 3 (2010), 271-284. doi: 10.1007/s12080-009-0068-y

|
|
[30]
|
D. A. Lytle, D. M. Merritt, Hydrologic regimes and riparian forests: A structured population model for cottonwood, Ecology, 85 (2004), 2493-2503. doi: 10.1890/04-0282

|
|
[31]
|
H. M. McKenzie, Y. Jin, J. Jacobsen, et al. R0 Analysis of a Spationtemporal Model for a Stream Population, SIAM J. Appl. Dyn. Syst., 11 (2012), 567-596. doi: 10.1137/100802189

|
|
[32]
|
R. T. Milhous, T. J. Waddle, Physical Habitat Simulation (PHABSIM) Software for Windows (v.1.5.1), Fort Collins, CO: USGS Fort Collins Science Center, 2012.
|
|
[33]
|
A. M. Mouton, M. Schneider, J. Depestele, et al. Fish habitat modelling as a tool for river management, Ecol. Eng., 29 (2007), 305-315. doi: 10.1016/j.ecoleng.2006.11.002

|
|
[34]
|
K. Müller, Investigations on the organic drift in North Swedish streams, Report of the Institute of Freshwater Research, Drottningholm, 34 (1954), 133-148.
|
|
[35]
|
K. Müller, The colonization cycle of freshwater insects, Oecologica, 53 (1982), 202-207.
|
|
[36]
|
T. Nagaya, Y. Shiraishi, K. Onitsuka, et al. Evaluation of suitable hydraulic conditions for spawning of ayu with horizontal 2D numerical simulation and PHABSIM, Ecol. Model., 215 (2008), 133-143. doi: 10.1016/j.ecolmodel.2008.02.043

|
|
[37]
|
S. A. Nazirov, A. A. Abduazizov, Approximate calculation of the multiple integrals' value by repeated application of Gauss and Simpson's quadrature formulas, Appl. Math. Sci., 7 (2013), 4223-4235.
|
|
[38]
|
E. Pachepsky, F. Lutscher, R. M. Nisbet, et al. Persistence, spread and the drift paradox, Theor. Popul. Biol., 67 (2005), 61-73. doi: 10.1016/j.tpb.2004.09.001

|
|
[39]
|
V. B. Pasour, S. P. Ellner, Computational and analytic perspectives on the drift paradox, SIAM J. Appl. Dyn. Syst., 9 (2010), 333-356. doi: 10.1137/09075500X

|
|
[40]
|
N. L. Poff, J. K. H. Zimmerman, Ecological responses to altered flow regimes: A literature review to inform the science and management of environmental flows, Freshwater. Biol., 55 (2010), 194-205. doi: 10.1111/j.1365-2427.2009.02272.x

|
|
[41]
|
D. Speirs, W. Gurney, Population persistence in rivers and estuaries, Ecology, 82 (2001), 1219-1237. doi: 10.1890/0012-9658(2001)082[1219:PPIRAE]2.0.CO;2

|
|
[42]
|
I. Stakgold, Green's Functions and Boundary Value Problems, 2 Eds., Wiley, New York, 1998.
|
|
[43]
|
P. Steffler, J. Blackburn, Two-dimensional depth averaged model of river hydrodynamics and Fish habitat, River2D User's Manual, University of Albert, Canada, 2002.
|
|
[44]
|
T. J. Stohlgren, P. Ma, S. Kumar, et al. Ensemble habitat mapping of invasive plant species, Risk Anal., 30 (2010), 224-235. doi: 10.1111/j.1539-6924.2009.01343.x

|
|
[45]
|
A. H. Stroud, Approximate Calculation of Multiple Integrals, Prentice-Hall Inc., 1971.
|
|
[46]
|
C. B. Talbert, M.K. Talbert, User Manual for SAHM package for Vis Trails, US Geological Survey, 2012.
|
|
[47]
|
H. Thieme, Spectral bound and reproductive number for infinite-dimensional population structure and time heterogeneity, SIAM J. Appl. Math., 70 (2010), 188-211.
|
|
[48]
|
F. Wang, B. Lin, Modelling habitat suitability for fish in the fluvial and lacustrine regions of a new Eco-City, SIAM J. Appl. Math., 267 (2013), 115-126.
|
|
[49]
|
W. Wang, X. Q. Zhao, Basic reproduction numbers for reaction-diffusion epidemic models, SIAM J. Appl. Dyn. Syst., 11 (2012), 1652-1673. doi: 10.1137/120872942

|
|
[50]
|
L. L. Wehmeyer, C. R. Wagner, Relation between Flows and Dissolved Oxygen in the Roanoke River between Roanoke Rapids Dam and Jamesville, North Carolina, 2005-2009, Scientific Scientific Investigations Report, Department of the Interior, U.S. Geological Survey, 2011. Available from: https://pubs.usgs.gov/sir/2011/5040/pdf/sir2011-5040.pdf.
|
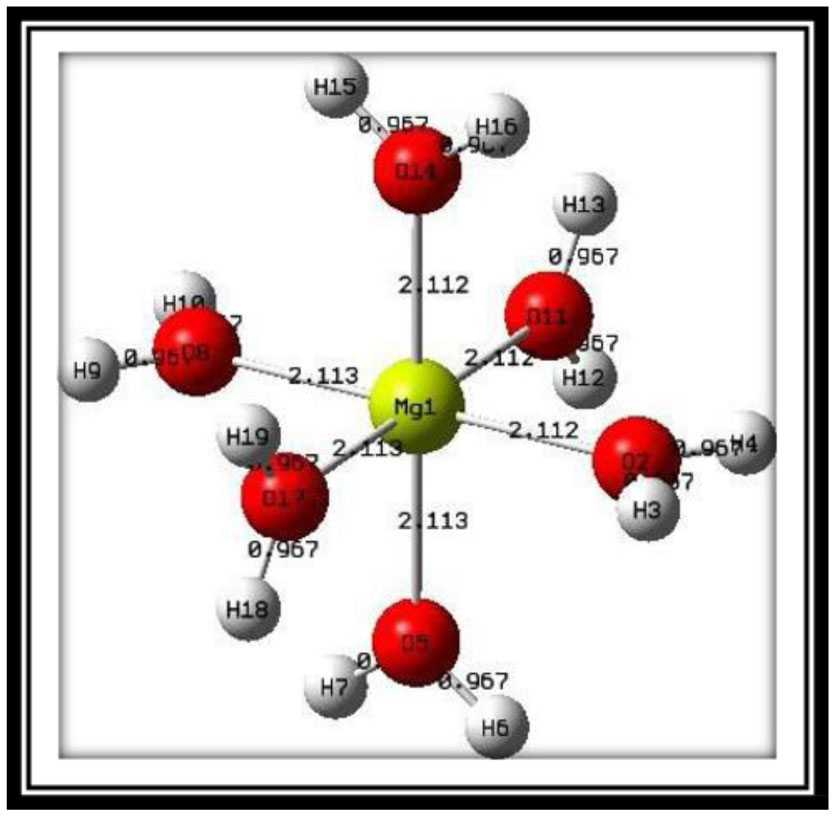









 DownLoad:
DownLoad: