Existence and uniqueness results for fractional Langevin equations on a star graph

1.   Introduction

In the last two decades, the topic in the study of fractional calculus theory has attracted significant attention from researchers. The strong interest stems not only from the important application of the theory, but also from the consideration of its mathematical nature. Indeed, many phenomena arising from scientific fields, including biology, physics, chemistry, financial economics, control theory, materials, medicine, and anomalous diffusion, are precisely described by fractional differential equations ^[1,2,3]. As an important topic for the theory of fractional differential equations, the existence results of fractional boundary value problems (BVPs) have been investigated comprehensively by scholars ^[4,5,6,7].

On the other hand, the theory of differential equations on graphs originated from Lumer's research work in the framework of ramification spaces in the 1980s ^[8]. Differential equations on graphs appear in various fields, including chemical engineering, biology, physics, and ecology ^[9,10,11,12]. For this reason, many scholars study mathematical models described by fractional BVPs on graphs.

In 2014 ^[10], Graef et al. investigated the existence of solutions for fractional BVPs on a star graph, which is composed of three nodes and two edges, that is $G{\rm{ = }}V \cup E$ with $V{\rm{ = }}\{ {\gamma _0}, {\gamma _1}, {\gamma _2}\}$ and $E = \left\{ {\overrightarrow {{\gamma _1}{\gamma _0}}, \overrightarrow {{\gamma _2}{\gamma _0}} } \right\},$ where ${\gamma _0}$ represents the junction node, $\overrightarrow {{\gamma _i}{\gamma _0}}$ is the edge connecting ${\gamma _i}$ and ${\gamma _0}$ with length ${l_i} = \left| {\overrightarrow {{\gamma _i}{\gamma _0}} } \right|, i = 1, 2.$ On each edge $\overrightarrow {{\gamma _i}{\gamma _0}}, i = 1, 2$, the authors considered the fractional BVPs in a local coordinate system with ${\gamma _i}$ as origin on $x \in (0, {l_i})$, given by

where $D_{0 + }^\alpha, D_{0 + }^\beta$ are Riemann-Liouville fractional derivative operators, $1 < \alpha \le 2, \; 0 < \beta < \alpha, \; {{\mathfrak{m}_i}} \in C[0, {l_i}], i = 1, 2$ with ${{\mathfrak{m}_i}}(x)\not \equiv 0$ on $[0, {l_i}]$ and ${{\mathfrak{f}_i}} \in C([0, {l_i}] \times \mathbb{R}, \mathbb{R}), i = 1, 2.$ By using Schauder fixed point theorem and Banach contraction mapping theorem, the existence and uniqueness of solutions of BVP (1.1) are obtained.

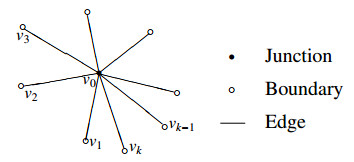
Later in 2019 ^[11], Mehandiratta et al. extended the results of Graef et al. on a general star graph (see Figure 1), which is a graph consisting of $k+1$ nodes and $k$ edges, that is, the authors considered a graph $G = V\cup E, \; V{\rm{ = }}\{ {v_0}, {v_1}, {\cdots}, {v_k}\}, \; E = \{ {e_i} = \overrightarrow {{v_i}{v_0}}, i = 1, 2, {\cdots}, k\},$ where ${v_0}$ is the junction node, $\overrightarrow {{v_i}{v_0}}$ represents the edge connecting ${v_i}$ and ${v_0}$ with length ${l_i} = \left| {\overrightarrow {{v_i}{v_0}} } \right|, i = 1, 2, {\cdots}, k.$ The author investigated the following fractional BVPs on the star graph $G$ given by

where ${}^CD_{0, x}^\alpha, {}^CD_{0, x}^\beta$ are Caputo fractional derivative, $1 < \alpha \le 2, \; 0 < \beta \le \alpha - 1, \; {{\mathfrak{f}_i}}, i = 1, 2, {\cdots}, k$ are continuous functions on $[0, l_i]\times \mathbb{R}\times \mathbb{R}.$ The existence and uniqueness results for BVP (1.2) are established using Schaefer's fixed point theorem and Banach contraction mapping theorem.

Based on the two studies mentioned above, the subject of fractional BVPs on graphs has received significant research attention, and various interesting results have been recently established ^{[12,13,14,15,16,17,18,19]}. For example, in ^[12], Zhang and Liu discussed BVPs of fractional differential equations on a star graph with $n+1$ nodes and $n$ edges. The existence and uniqueness of solutions are established using Schaefer's fixed point theorem and Banach contraction mapping principle. Etemad and Rezapour in ^[13] studied the BVPs of fractional differential equations on ethane graph. The existence results of solutions were obtained using Schaefer's fixed point theorem and Krasnoselskii's fixed point theorem. In ^[14], Baleanu et al. investigated the existence of solutions for BVPs of fractional differential equations on the glucose graphs. In ^[15], Ali et al. studied the existence of solutions of BVPs for fractional differential equations on the cyclohexane graphs using the fixed point theory. In ^[16], Mehandiratta et al. considered a nonlinear fractional BVPs on a particular metric graph. They proved the existence and uniqueness of solutions using Krasnoselskii's fixed point theorem and Banach contraction principle.

It is well known that Langevin first formulated the Langevin equation in 1908. Langevin equation is an important tool for describing the evolution of physical phenomena in fluctuating environments ^[20]. However, people have realized that the traditional integer Langevin equation cannot accurately describe dynamic systems for complex phenomena. Therefore, one way to overcome this disadvantage is to use fractional derivative instead of integer derivative ^[21]. This gives rise to the fractional Langevin equation. Studies of BVPs on fractional Langevin equations have increased in recent years, and new research is constantly emerging ^{[22,23,24,25]}. For example, in ^[22], Fazli et al. studied the anti-periodic BVPs of fractional Langevin equation and obtained the existence and uniqueness solutions using the coupled fixed point theorem for mixed monotone mappings. In ^[23], Matar et al. established the existence, uniqueness and stability of solutions for the coupled Caputo-Hadamard fractional Langevin equation with the help of the fixed point theorem. In ^[24], Salem et al. considered the fractional Langevin equation with three-point boundary value conditions and obtained the existence of solutions by using Krasnoselskii's fixed point theorem and Leray-Schauder nonlinear alternative theorem.

From the literature review, no result is concerned with fractional Langevin equations on graphs. To fill this knowledge gap, this study aims to establish the existence and uniqueness results for fractional Langevin equations on a star graph subject to mixed boundary conditions. Precisely, we investigate the following problems:

where $0 < \alpha < 1, \; 0 < \gamma < \alpha, \; {\lambda _i} \in \mathbb{R}^+, \; i = 1, 2, {\cdots}, k, \; {}^CD_{0, x}^\alpha, {}^CD_{0, x}^\gamma$ are Caputo fractional derivative, $D$ is the ordinary derivative, ${{\mathfrak{g}_i}} \in C([0, {\rho_i}] \times \mathbb{R}^2, \mathbb{R}), \; i = 1, 2, {\cdots}, k.$ The star graph has $k+1$ nodes and $k$ edges, that is $G = V\cup E, \; V{\rm{ = }}\{ {v_0}, {v_1}, {\cdots}, {v_k}\}, \; E = \{ {e_i} = \overrightarrow {{v_i}{v_0}}, \; i = 1, 2, {\cdots}, k\},$ where ${v_0}$ is the junction node, ${e_i} = \overrightarrow {{v_i}{v_0}}$ represents the edge connecting ${v_i}$ and ${v_0}$ with length ${\rho_i} = \left| {\overrightarrow {{v_i}{v_0}} } \right|, \; i = 1, 2, {\cdots}, k.$ We consider a local coordinate system with ${v_i}$ as origin and $x \in (0, {\rho_i})$ as the coordinate. The existence and uniqueness of the solution of BVP (1.3) are discussed using Schaefer's fixed point theorem and Banach contraction mapping principle.

The rest of paper is organized as follows: In Section 2, we recall some basic definitions of fractional calculus and present an auxiliary lemma (Lemma 2.6), which transforms the problem (1.3) to BVP (2.1). In Section 3, we study the existence and uniqueness results of BVP (2.1) by using Schaefer's fixed point theorem and Banach contraction principle, respectively. Finally, two illustrative examples are discussed at the end of this paper.

2.   Preliminaries

In this section, we recall some definitions of fractional calculus and provide preliminary results which we will use in the rest of the paper.

Definition 2.1 ^[1]. The Riemann-Liouville fractional integral of order $\alpha > 0$ for a function $f \in C(a, b)$ is defined by

Definition 2.2 ^[1]. The Caputo fractional derivative of order $\alpha > 0$ for a function $f \in {C^n}(a, b)$ is presented by

where $n = [\alpha] + 1.$

Lemma 2.1 ^[1]. Let $\alpha > 0.$ Suppose that $u\in AC^n[0, 1]$. Then

where ${c_i} \in \mathbb{R}, \; i = 1, 2, {\cdots}, n, \; n = [\alpha] + 1.$

Lemma 2.2 ^[26]. Let $\alpha{ > }0, \; n{\in} N,$ and $D = d/dx$. Suppose that $(D^{n}x)(t)$ and $({}^CD_{a, t }^{\alpha + n}x)(t)$ are exist. Then

Lemma 2.3 ^[1]. If $\beta > 0, \; \gamma > \beta - 1, \; t > 0,$ then

Theorem 2.4 ^[27]. (Scheafer's fixed point theorem) Let $X$ be a Banach space. Assume that $T:X \rightarrow X$ is a completely continuous operator and the set $\Omega = \{ x \in X, \; x = \mu Tx, \; \mu \in (0, 1)\}$ is bounded. Then $T$ has a fixed point in $X$.

Lemma 2.5 ^[11]. Suppose that ${{\mathfrak{y}}}$ is a function defined on $[0, \rho]$ such that ${}^CD_{0, x}^\alpha {{\mathfrak{y}}}$ exists on $[0, \rho]$ with $\alpha > 0$ and let $x\in [0, \rho], \; t = x/\rho \in [0, 1], \; y(t) = {{\mathfrak{y}}}(\rho t)$. Then

Lemma 2.6 Suppose that ${{\mathfrak{y}}}$ be a function defined on $[0, \rho]$ such that ${}^CD_{0, x}^\alpha {{\mathfrak{y}}}$ exists on $[0, \rho]$ with $\alpha \in (n-1, n)$ and let $x \in [0, \rho], \; t = x/\rho \in [0, 1], \; y(t) = {{\mathfrak{y}}}(\rho t)$. Then

Proof. By using the Definition 2.2 and Lemma 2.2, we can obtain

This completes the proof of Lemma 2.6.

By a direct calculation with help of Lemmas 2.5 and 2.6, BVP (1.3) can be transformed into a BVP defined on [0, 1] given by

where ${y_i}(t) = {{\mathfrak{y}_i}}({\rho_i}t), \; {g_i}(t, u, v) = {{\mathfrak{g}_i}}({\rho_i}t, u, v), \; i = 1, 2, {\cdots}, k.$

3.   Main results

In this section, we investigate the existence and uniqueness results of problem (2.1). To this end, we consider the space $Y{\rm{ = \{ }}y:y \in C[0, 1], \; {}^CD_{0, t}^\gamma y \in C[0, 1]\},$ endowed with the norm

where $||y|| = \mathop {\max }\limits_{t \in [0, 1]} |y(t)|, \; ||{}^CD_{0, t}^\gamma y|| = \mathop {\max }\limits_{t \in [0, 1]} |{}^CD_{0, t}^\gamma y(t)|.$ Then $(Y, || \cdot |{|_Y})$ is a Banach space, and the product space $({Y^k}, || \cdot |{|_{{Y^k}}})$ equipped with the norm

is also a Banach space, where ${Y^k} = \overbrace {Y \times Y \times{\cdots} \times Y}^k.$

Lemma 3.1 Let ${h_i} \in C[0, 1], \; i = 1, 2, {\cdots}, k.$ Then the BVP of fractional Langevin equations

is equivalent to the integral equations

where ${\ell _j}: = \frac{{\rho_j^{ - 1}}}{{\sum\nolimits_{j = 1}^k {\rho_j^{ - 1}} }}, \; i, j = 1, 2, {\cdots}, k.$

Proof. Applying the operator $I_{0{\rm{ + }}}^\alpha$ on both sides of Eq (3.1) and combining with the Lemma 2.1, we obtain

where $c_1^i \in \mathbb{R}, \; i = 1, 2, \cdots, k$. The above equation can be rewritten as

Integrating both sides of Eq (3.2) from 0 to $t,$ we get

By conditions ${y_i}(0) = 0, \; i = 1, 2, \cdots, k,$ we conclude

Applying the conditions $\sum\limits_{i = 1}^k {\rho_i^{ - 1}{{y}'_i}} (1) = 0$ and ${y_i}(1) = {y_j}(1), \; i, j = 1, 2, \cdots, k, \; i \ne j$ in Eqs (3.2) and (3.3), respectively, we find

and

Combining the above two equations, we get

This yields

from which we deduce that

Substituting $c_1^i\; (i = 1, 2, {\cdots}, k)$ into the Eq (3.3), we get the desired result. The converse of the lemma is calculated directly. The proof is completed.

In view of Lemma 3.1, we define the operator $T:{Y^k} \to {Y^k}$ by

for $t\in[0, 1]$ and ${y_i}\in Y, \; i = 1, 2, \cdots, k$, where

In the following part, for convenience of presentation, we denote the notations:

Theorem 3.1 Assume that

$(H_1)$ The functions ${g_i}:[0, 1] \times \mathbb{R}^2 \to \mathbb{R}, \; (i = 1, 2, \cdots, k)$ are continuous and there exist functions ${a_i}(t)\in C([0, 1], [0, +\infty))$, $i = 1, 2, \cdots, k$, such that

for all $t\in [0, 1]$ and $(u, v), ({u_1}, {v_1}) \in {\mathbb{R}^2}$. Then the BVP (2.1) has a unique solution on [0, 1], provided that

where

Proof. Applying the Banach contraction mapping principle, we have to prove that $T$ is a contractive mapping. To prove this, we let $y = ({y_1}, {y_2}, \cdots, {y_k}), \; \bar y = ({\bar y_1}, {\bar y_2}, \cdots, {\bar y_k}) \in {Y^k}, \; t\in [0, 1].$ By Eq (3.4), we have

By using the assumption $(H_1)$ and $t \in [0, 1], \; {\ell _j} \in (0, 1), \; j = 1, 2, \cdots, k$, we deduce

Then for any $y, \bar y \in {Y^k}$, we obtain

On the other hand, by using Lemma 2.3, we have

In a similar manner, we deduce

This implies that, for any $y, \bar y \in {Y^k}$,

By a direct calculation with help of (3.5) and (3.6), we get

From this it follows that

As a consequence, we obtain

It follows from the condition $\sum\limits_{i = 1}^k {{P_i}\Big({\sum\limits_{i = 1}^k {{A_i}} } \Big) + \sum\limits_{i = 1}^k {{Q_i}} } < 1$ that $T$ is a contractive mapping. Hence, $T$ has a unique fixed point on ${Y^k}$, that is, BVP (2.1) has a unique solution. Therefore, we obtain the conclusion of the theorem.

Theorem 3.2 Assume that

$(H_2)$ The functions ${g_i}:[0, 1] \times \mathbb{R}^2 \to \mathbb{R}, \; (i = 1, 2, \cdots, k)$ are continuous and there exist functions ${p_i}(t), {q_i}(t), {r_i}(t) \in C([0, 1], [0, +\infty)), \; (i = 1, 2, \cdots, k)$ such that

for all $t\in[0, 1], \; u, v\in \mathbb{R}$. Then the BVP (2.1) admits at least one solution in $Y$ provided that

where

and

Proof. We divide the proof into two steps.

Step 1. We need to verify that the operator $T$ is a completely continuous. In fact, since the functions ${g_i}(i = 1, 2, \cdots, k)$ are continuous, we can easily prove that the operators ${T_i}(i = 1, 2, \cdots k)$ are continuous, and thus $T$ is continuous. Next, we have to show that $T$ is compact. To see this, we define the bounded subset $\Lambda = \{ {y_i} \in Y, \; ||{y_i}|{|_Y} \le {\varepsilon _i}\}$ on $Y$, then for any $y = ({y_1}, {y_2}, \cdots, {y_k}) \in \Lambda,$ by $(H_2)$, we find that

From which we can deduce that

On the other hand, by Lemma 2.3 and $(H_2)$, we also can get the estimate

In a similar manner, we deduce

From (3.7) and (3.8), we get that

where

Form this it follows that

Hence, the operator $T$ is uniformly bounded on $\Lambda$.

Now, We will show that the operator $T$ is equicontinuous on $\Lambda$. Indeed, for $y = ({y_1}, {y_2}, \cdots, {y_k}) \in \Lambda, \; {t_1}, {t_2} \in [0, 1], \; {t_1} < {t_2},$ we have

and

Form (3.10) and (3.11), we get

which implies $||{T_i}y({t_2}) - {T_i}y({t_1})|{|_Y} \to 0$ as ${t_2} \to {t_1}$, and so $||Ty({t_2}) - Ty({t_1})|{|_{{Y^k}}} \to 0$ as ${t_2} \to {t_1}.$ Therefore, the operator $T$ is equicontinuous on $\Lambda$. According to Arzelá-Ascoli theorem that $T$ is completely continuous.

Step 2. By applying Scheafer's fixed point theorem, we now prove that $T$ has fixed point in $Y$. To this aim, we define $\Omega = \{ ({y_1}, {y_2}, \cdots, {y_k}) \in {Y^k}:({y_1}, {y_2}, \cdots, {y_k}) = \mu T({y_1}, {y_2}, \cdots, {y_k}), \mu \in (0, 1)\}$ and show that $\Omega$ is bounded. In fact, for $({y_1}, {y_2}, \cdots, {y_k}) \in \Omega,$ then $({y_1}, {y_2}, \cdots, {y_k}) = \mu T({y_1}, {y_2}, \cdots, {y_k})$, that is, for $t\in [0, 1],$ we have ${y_i}(t) = \mu {T_i}({y_1}, {y_2}, \cdots, {y_k}), \; i = 1, 2, \cdots, k.$ Similarly in the proof of (3.7), by assumption $(H_2)$, we deduce

from which we obtain

In a similar manner of deduce (3.8), by assumption $(H_2)$, we also can obtain the estimate

Then for $t\in[0, 1],$ we get

Combining this with (3.12) gives

where ${N_i}$ is defined as in (3.9), from which we deduce that

It follows from $\sum\limits_{i = 1}^k {{\theta _i}} < 1$ that $\Omega$ is bounded. By Theorem 2.4, the operator $T$ has at least one fixed point, that is, the BVP (2.1) has at least one solution.

4.   Examples

Example 4.1 Consider the BVP (1.3) with $k { = } 3, \; \alpha { = }\frac{1}{2}, \gamma { = } \frac{1}{3}, \; {\lambda _1} { = } {\lambda _2} { = } {\lambda _3} { = } {\rho_1} { = } \frac{1}{{10}}, \; {\rho_2} { = } \frac{1}{{20}}, \; {\rho_3} { = } \frac{1}{{30}},$ and

In view of Lemma 2.6, we get the equivalent system

From (4.1), for $t \in [0, 1], u, v, {u_1}, {v_1} \in \mathbb{R},$ we can conclude that

So, we get

By simple calculation, we obtain

Then

From Theorem 3.1 that the BVP (4.1) has a unique solution.

Example 4.2 Consider the BVP (1.3) with $k { = } 3, \; \alpha { = }\frac{1}{2}, \; \gamma { = } \frac{1}{3}, \; {\lambda _1} { = } {\lambda _2} { = } {\lambda _3} { = } \frac{1}{{20}}, \; {\rho_2} { = } \frac{1}{5}, \; {\rho_1} { = } {\rho_3} { = } \frac{1}{{10}},$ and

Then by Lemma 2.6, we obtain the equivalent system

Then

For $t\in [0, 1],$ we have $q_1^ * { = } \frac{1}{{54}}, \; q_2^ * { = } \frac{1}{{12}}, \; q_3^ * { = } \frac{1}{{25}}, \; r_1^ * { = } r_2^ * { = } \frac{1}{{20}}, \; r_3^ * { = } \frac{1}{{24}}.$ By calculation, we get

So,

Thus,

According to Theorem 3.2, the BVP (4.2) has at least one solution.

5.   Conclusions

This paper considers the fractional Langevin equations on a star graph of the form (1.3). By using Lemma 2.6, the problem (1.3) is transformed into an equivalent system of fractional Langevin equations supplemented with mixed boundary conditions defined on $[0, 1]$, that is, problem (2.1). Making use of the fixed point theorems (Schauder's fixed point theorem, Banach's contraction mapping principle), sufficient criteria for the existence and uniqueness results are derived. Finally, we present two examples to illustrate the validity of the obtained results. As a possible extension of this paper, we will study the higher-order fractional Langevin-type equations on star graphs in the future, such as

supplemented with the boundary conditions

and

where $0 < \alpha < 1, \; 0 < \beta < \alpha, \; {\lambda _i} \in \mathbb{R}^+, \; i = 1, 2, \cdots, k, \; {}^CD_{0, x}^\alpha, {}^CD_{0, x}^\beta$ are Caputo fractional derivative, $D^2$ is the ordinary second-order derivative, ${{\mathfrak{g}_i}} \in C([0, {l_i}] \times \mathbb{R}^2, \mathbb{R}), \; i = 1, 2, \cdots, k.$ The star graph has $k+1$ nodes and $k$ edges, that is $G = V\cup E, V{\rm{ = }}\{ {v_0}, {v_1}, \cdots, {v_k}\}, E = \{ {e_i} = \overrightarrow {{v_i}{v_0}}, i = 1, 2, \cdots, k\},$ where ${v_0}$ is the junction node, ${e_i} = \overrightarrow {{v_i}{v_0}}$ represents the edge connecting ${v_i}$ and ${v_0}$ with length ${l_i} = \left| {\overrightarrow {{v_i}{v_0}} } \right|, i = 1, 2, \cdots, k.$

Acknowledgments

The authors wish to express their sincere appreciation to the editor and the anonymous referees for their valuable comments and suggestions. This research is supported by the National Natural Science Foundation of China (11601007) and the Key Program of University Natural Science Research Fund of Anhui Province (KJ2020A0291).

Conflict of interest

The authors declare that they have no competing interests.