Optical solitons and single traveling wave solutions of Biswas-Arshed equation in birefringent fibers with the beta-time derivative

1.   Introduction

It is common knowledge that fractional partial differential equations are often used to establish mathematical models to fit experiments or practices. In order to better fit the experimental data more accurately, many scholars have studied partial differential equations (PDEs) involving a variety of fractional derivatives for decades, such as the Riemann-Liouville derivative ^[1], Caputo fractional derivative ^[2], conformable derivative ^{[3,4,5,6,7,8,9,10,11]} and beta derivative ^{[12,13,14,15,16,17,18]}. Investigating the traveling wave solutions to nonlinear PDEs plays an important role in the study of nonlinear physical phenomena ^{[19,20,21,22]}.

The Schrödinger equation is an important submicroscopic model in quantum mechanics. In recent years, researchers have established so many optical models based on the Schrödinger equation. Among these models, the Biswas-Arshed equation (BAE) is a generalization of the Schrödinger equation; it describes pulse propagation through optical fiber ^[23]. Due to the significant improvement of related technologies, the telecommunications industry has experienced great growth in the past few decades. In order to further study nonlinear optics, ignoring the self phase modulation, Anjan Biswas and Ssima Arshed established the BAE with the beta-time derivative ^[23]:

where $\psi = \psi (x, t)$ is a complex-valued function. Also, $x$ and $t$ are spatial and temporal variables, respectively. The parameters $p_{1}$ and $p_{2}$ are the coefficients for the group velocity dispersion and the spatio-temporal dispersion, respectively. The parameters $q_{1}$ and $q_{2}$ are the coefficients for the third-order dispersion and the spatio-temporal third-order dispersion, respectively. The parameter $\lambda$ is the coefficient for the self-steepening effect while $\phi$ and $\delta$ present the coefficients for nonlinear dispersions.

A large number of methods for finding the traveling wave solutions of the BAE without fractional derivatives have been proposed, such as dynamical system methods ^[24], undetermined coefficients and Kudryashov's methods ^[25], the $\Phi^6$ model expansion method ^[26], the sine-Gordon equation method ^[27], the modified simple equation method ^[28], extended sinh-Gordon equation expansion and modified $(G'/G)$-expansion schemes ^[29], the trial equation technique ^[30], Jacobi's elliptic function approach ^[31], extended simplest equation methods ^[32], improved modified extended tanh-function methods ^[33] and various other methods ^{[34,35,36,37,38,39,40,41,42]}.

However, the special methods can yield a special kind of solution. In this paper, we look for more optical solitons and single traveling wave solutions of the BAE with the beta time derivative by using the complete discrimination system method. Recently, the effectiveness of the method has been examined by some authors. For example, Xu et al. adopted the complete discrimination system method to study soliton transmission dynamics ^[24]. Tang studied the exact solutions to a conformable time-fractional Klein-Gordon equation with high-order nonlinearities ^[41].

In 2020, Biswas and Arshed first introduced the beta-time derivative into the BAE. As far as we know, there are few studies on the optical solitons traveling wave solutions of Eq (1.1). In 2020, Demiray obtained the hyperbolic function solution of the BAE with beta-time derivative ^[17]. Hosseini and his partners used Jacobi and Kudryashov methods to obtain the solutions of the BAE with the beta-time derivative, which include hyperbolic function solutions and Jacobian elliptic functions ^[18]. Using the polynomial discriminant system method, we obtain the classification of all single wave solutions of (1.1), including solutions in the forms of rational functions and trigonometric functions, in addition to the solutions in ^[17,18].

Definition 1.1. Let $f(t)$ be a function defined for all non-negative $t$. Then, the beta derivative of $f(t)$ is given by ^[18]:

where $\Gamma (\beta) = \int_0^\infty{e^{-t}{t^{\beta- 1}}{\rm{d}}t}, \beta > 0$.

Theorem 1.2. Let $f(t)$ and $g(t)$ be $\beta$-differentiable functions for all $t > 0$ and $\beta \in (0, 1]$.Then

${T^\beta }(k_1f(t) + k_2g(t)) = k_1{T^\beta }(f(t)) + k_2{T^\beta }(g(t)), \quad \forall k_1, k_2 \in R.$

${T^\beta }(f(t)g(t)) = g(t){T^\beta }(f(t)) + f(t){T^\beta }(g(t)).$

${T^\beta }\left({\frac{{f(t)}}{{g(t)}}} \right) = \frac{{g(t){T^\beta }(f(t)) - f(t){T^\beta }(g(t))}}{{{{(g(t))}^2}}}.$

${T^\beta }(f(t)) = {\left({t + \frac{1}{{\Gamma (\beta)}}} \right)^{1 - \beta }}\frac{\mathit{\rm{d}}f(t)}{\mathit{\rm{d}}t}.$

The outline of this paper is as follows. In Section 2, the reduced form of the BAE with the beta-time derivative is presented in a detailed manner, and the complete discrimination system is also reviewed. In Section 3, optical solitons and other solutions of the BAE with the beta-time derivative are derived. In Section 4, the graphical interpretation of some traveling wave solutions are given by using 2D and 3D graphics. The conclusion is given in the last section.

2.   BAE with beta time derivative and its reduction

In order to construct the optical solitons and single traveling wave solutions of Eq (1.1), we consider the following traveling wave transformation

where $\rho$ is the speed of the wave. The parameters $\gamma$, $\omega$ and $\varphi_0$ are the frequency, wave number and phase constant respectively, and $u(\xi)$ is a real function representing the amplitude portion of the traveling wave solution.

Substituting Eq (2.1) into Eq (1.1), and separating the real and imaginary parts gives

Multiplying Eq (2.2) by $\frac{{{\rm{d}}u(\xi)}}{{{\rm{d}}\xi }}$ and integrating once gives

where ${C_0}$ is the integral constant.

First, we can find the general solutions of Eq (2.4) by assuming ${C_0} = 0$:

In this case Eq (2.3) is satisfied and from Eq (2.5), we have

Integrating Eq (2.3) once, we obtain the equation

Multiplying both sides of Eq (2.7) by $\frac{{{\rm{d}}u(\xi)}}{{{\rm{d}}\xi }}$ and integrating once, we get

where $C_1$ and $C_2$ are integral constants.

Substituting Eq (2.5) into Eq (2.8), we have

where ${d_4} = \frac{{(2\gamma {q_2} + {p_2})({\gamma ^2}{q_2} + \gamma {p_2} - 1)(\delta - \phi)}}{3{({p_1}{p_2}{q_2} - {p_2}^2{q_1} - {q_1}{q_2})\gamma - {p_1}{q_2} + {p_2}{q_1}}}$, ${d_2} = \frac{{6{q_2}^2({q_1}-1){\gamma ^5} + 9{p_2}{q_2}({q_1} - 1){\gamma ^4} + ((- {p_1}{p_2} - 5{q_1} + 6){q_2} + 4{p_2}^2({q_1} - \frac{3}{4})){\gamma ^3}}}{{({p_1}{p_2}{q_2} - {p_2}^2{q_1} - {q_1}{q_2})\gamma - {p_1}{q_2} + {p_2}{q_1}}} + \frac{{(3{p_1}{q_2} + (- 6{q_1} + 3){p_2}){\gamma ^2} + 3\gamma {q_1} + {p_1}}}{{({p_1}{p_2}{q_2} - {p_2}^2{q_1} - {q_1}{q_2})\gamma - {p_1}{q_2} + {p_2}{q_1}}}$, ${d_1} = \frac{{2{C_1}(2\gamma {q_2} + {p_2})({\gamma ^2}{q_2} + \gamma {p_2} - 1)}}{{({p_1}{p_2}{q_2} - {p_2}^2{q_1} - {q_1}{q_2})\gamma - {p_1}{q_2} + {p_2}{q_1}}}$ and ${d_0} = \frac{{{C_2}(2\gamma {q_2} + {p_2})({\gamma ^2}{q_2} + \gamma {p_2} - 1)}}{{({p_1}{p_2}{q_2} - {p_2}^2{q_1} - {q_1}{q_2})\gamma - {p_1}{q_2} + {p_2}{q_1}}}$.

Making the transformation as follows

Substituting the transformation of Eq (2.10) into Eq (2.9) will change it into the following form:

where $p = \left\{ {\begin{array}{*{20}{l}} {d_2}{\left| {{d_4}} \right|^{ - \frac{1}{4}}}, & {d_4} > 0 \\ - {d_2}{\left| {{d_4}} \right|^{ - \frac{1}{4}}}, & {d_4} < 0 \end{array}} \right., q = \left\{ {\begin{array}{*{20}{l}} {d_1}{\left| {{d_4}} \right|^{ - \frac{1}{4}}}, & {d_4} > 0 \\ - {d_1}{\left| {{d_4}} \right|^{ - \frac{1}{4}}}, & {d_4} < 0 \end{array}} \right.,$ $r = \left\{ {\begin{array}{*{20}{l}} {d_0}, & {d_4} > 0 \\ - {d_0}, & {d_4} < 0 \end{array}} \right.$ and $\varepsilon = \left\{ {\begin{array}{*{20}{l}} 1, & {d_4} > 0 \\ - 1, & {d_4} < 0 \end{array}} \right..$

Denote $F\left(w \right) = {w^4} + p{w^2} +qw+ r$. Then Eq (2.11) can be written in the integral form:

Let

The system consisting of $D_1-D_4$ defined by Eq (2.13) is called the complete discriminant system of the function $F(w)$. According to the complete discriminant method, we can determine the root of the function $F(w)$ from the signs of $D_1$, $D_2$, $D_3$ and $D_4$; then we can obtain the solution of Eq (2.12).

3.   Optical solitons and traveling wave solutions of the BAE with the beta-time derivative

Case 1. ${D_1} < 0$, ${D_2} = {D_3} = 0$. $F\left(w \right)$ has a pair of conjugate complex roots. Without losing generality, let us set

When $\varepsilon = 1$, Eq (2.12) has the solution

With the help of Eq (2.1), we get the solution of Eq (1.1) as follows:

Case 2. ${D_1} = {D_2} = {D_3} = 0$. $F\left(w \right)$ has a quadruple root. So we set $F\left(w \right) = {w^4}$. When $\varepsilon = 1$, we can get the solution of Eq (2.12)

Then, the solution of Eq (1.1) is:

Case 3. ${D_1} > 0, {D_2} = {D_3} = 0, {D_4} > 0$. $F\left(w \right)$ has two different double real roots. Without losing generality, let $F\left(w \right) = {\left({w - \alpha_2 } \right)^2}{\left({w - \alpha_1 } \right)^2}$, $\alpha_2 > \alpha_1$.

When $\varepsilon = 1$, we get that:

(1) When $w > \alpha_2$ or $w < \alpha_1$, Eq (2.12) can be written in the following form:

We get the solution of Eq (1.1) as follows:

(2) When $\alpha_1 < w < \alpha_2$, we get the solution of Eq (2.12) as follows:

Then, the solution of Eq (1.1) is:

Case 4. ${D_1} > 0, {D_2} > 0, {D_3} = 0.$ $F\left(w \right)$ has a double real root and real roots with two multiplicities, namely, $F\left(w \right) = {\left({w - \alpha_2 } \right)^2}\left({w - \alpha_1 } \right)\left({w - \alpha_3 } \right)$, where $\alpha_1, \alpha_2$ and $\alpha_3$ are real numbers and $\alpha_1 > \alpha_3$.

(1) $\varepsilon = 1.$

When $\alpha_2 > \alpha_1$ and $w > \alpha_1$, or when $\alpha_2 < \alpha_3$, and $w < \alpha_3$, we get the implicit solution of Eq (2.12):

When $\alpha_2 > \alpha_1$, and $w < \alpha_3$, or when $\alpha_2 < \alpha_3$, and $w < \alpha_1$, we have the implicit solution of of Eq (2.12):

When $\alpha_1 > \alpha_2 > \alpha_3$, we have the implicit solution of of Eq (2.12):

(2) $\varepsilon = - 1$.

When $\alpha_2 > \alpha_1$, and $w > \alpha_1$, or when $\alpha_2 < \alpha_3$, and $w < \alpha_3$, the following solution of Eq (2.12) can be obtained:

When $\alpha_2 > \alpha_1$, and $w < \alpha_3$, or when $\alpha_2 < \alpha_3$, and $w < \alpha_1$, the following solution of Eq (2.12) can be obtained:

When $\alpha_1 > \alpha_2 > \alpha_3$, the following solution of Eq (2.12) can be obtained:

From Eqs (3.5), (3.6), (3.7), (3.8), (3.9) and (3.10), we get the implicit solution of Eq (2.12) which involves logarithmic and inverse trigonometric functions.

Especially, substituting $\delta = 1, \phi = 3, \gamma = 1, p_1 = 2, p_2 = 1, q_1 = 3, q_2 = 3, c_1 = 0, c_2 = 0$, $\xi_0 = 0$, $\alpha_1 = \frac{ \sqrt{6}}{\sqrt[8]{7}}, \alpha_2 = 0$ and $\alpha_3 = -\frac{ \sqrt{6}}{\sqrt[8]{7}}$ into Eq (2.12), we construct the periodic solutions involving trigonometric functions of Eq (1.1):

Case 5. ${D_1} > 0, {D_2} = {D_3} = {D_4} = 0$. $F\left(w \right)$ has real roots with three multiplicities and real roots with one multiplicity, namely,

where $\alpha_1$, and $\beta$ are real numbers.

(1) $\varepsilon = 1$. When $w > \max \left\{ {\alpha_1, \alpha_2 } \right\}$, we get the solution of Eq (1.1) as follows:

(2) $\varepsilon = - 1$. When $\min \left\{ {\alpha_1, \alpha_2 } \right\} < w < \max \left\{ {\alpha_1, \alpha_2 } \right\}$, we get the solution of Eq (1.1) as follows:

Now, we have the rational function solutions given be Eqs (3.13) and (3.14).

Case 6. ${D_3} = 0$, ${D_1}{D_2} < 0$. $F\left(w \right)$ has one double real root and a pair of conjugate complex roots, namely,

where $\alpha$, $l$ and $s$ are real numbers. Then, the solution of Eq (2.12) can be expressed as:

where $\gamma = \frac{{\alpha - 2l}}{{\sqrt {{{\left({\alpha - l} \right)}^2} + {s^2}} }}$ and $\delta = \sqrt {{{\left({\alpha - l} \right)}^2} + {s^2}} - \frac{{\alpha \left({\alpha - 2l} \right)}}{{\sqrt {{{\left({\alpha - l} \right)}^2} + {s^2}} }}$, which is a solitary wave solution.

So, we get the solution of Eq (1.1) as follows:

Case 7. ${D_1} > 0, {D_2} > 0, {D_3} > 0$. $F\left(w \right)$ has four distinct real roots, namely,

where ${\alpha _1}, {\alpha _2}, {\alpha _3}$ and ${\alpha _4}$ are real numbers, and ${\alpha _1} > {\alpha _2} > {\alpha _3} > {\alpha _4}$.

(1) $\varepsilon = 1$.

When $w > {\alpha _1}$, or when $w < {\alpha _4}$, we consider the following transformation:

From Eq (2.12), we obtain

where ${m^2} = \frac{{\left({{\alpha _1} - {\alpha _4}} \right)\left({{\alpha _2} - {\alpha _3}} \right)}}{{\left({{\alpha _1} - {\alpha _3}} \right)\left({{\alpha _2} - {\alpha _4}} \right)}}.$

According to the definition of the Jacobian elliptic sine function, we obtain that

Then, we construct the solution of Eq (1.1) to be

When ${\alpha _3} < w < {\alpha _2}$, we make the following transformation:

By using Eq (2.12), we obtain

We construct the solution of Eq (1.1) to be:

(2) $\varepsilon = - 1$.

When ${\alpha _1} > w > {\alpha _2}$, we make the following transformation:

We get the solution of Eq (2.12):

where ${n^2} = \frac{{\left({{\alpha _1} - {\alpha _2}} \right)\left({{\alpha _3} - {\alpha _4}} \right)}}{{\left({{\alpha _1} - {\alpha _3}} \right)\left({{\alpha _2} - {\alpha _4}} \right)}}.$

By the definition of the Jacobian elliptic sine function, we obtain that

Then, we construct the solution of Eq (1.1):

When ${\alpha _3} > w > {\alpha _4}$, we apply the following transformation:

Similarly, we get the solution of Eq (1.1):

From Eqs (3.16), (3.17), (3.18) and (3.16), we obtain four biperiodic single traveling wave solutions of Eq (1.1).

Case 8. ${D_1}{D_2} \geqslant 0, {D_3} < 0$. $F\left(w \right)$ has two different real roots and a pair of conjugate complex roots, namely,

where $\alpha_1$, $\alpha_2$, $l$ and $s$ are real numbers, and $\alpha_{1} > \alpha_{2}$ and $l, s > 0$.

We make the following transformation:

where $a = \frac{{\alpha_1+\alpha_2 }}{2}c - \frac{{\alpha_1-\alpha_2 }}{2}d$, $b = \frac{{\alpha_1+\alpha_2 }}{2}d - \frac{{\alpha_1-\alpha_2 }}{2}c$, $c = \alpha_1 - l - \frac{s}{{{m_1}}}$, $d = \alpha_1 - l - s{m_1}$, $E = \frac{{{s^2} + \left({\alpha_1 - l} \right)\left({\alpha_2 - l} \right)}}{{s\left({\alpha_1-\alpha_2 } \right)}}$, ${m_1} = E \pm \sqrt {{E^2} + 1}$.

From Eq (2.12), we obtain

where ${m^2} = \frac{1}{{1 + m_1^2}}.$

(1) $\varepsilon = 1$. By the definition of the Jacobian elliptic function, we obtain:

We get the solution of Eq (1.1):

(2) $\varepsilon = - 1$. The solution of Eq (1.1) is as follows:

Case 9. ${D_1}{D_2} \leqslant 0, {D_3} > 0$. $F\left(w \right)$ has two pairs of conjugate complex roots, namely,

where ${l_1}, {l_2}, {s_1}$ and ${s_2}$ are real numbers, and ${s_1} \geqslant {s_2} > 0.$

If $\varepsilon = 1$, Eq (2.12) will be meaningful. Then, we make the following transformation:

where $a = {l_1}c + {s_1}d$, $b = {l_1}d - {s_1}c$, $c = - {l_1} - \frac{{{l_2}}}{{{m_1}}}$, $d = {l_1} - {l_2}$, $E = \frac{{{{\left({{l_1} - {l_2}} \right)}^2} + {s_1}^2 + {s_2}^2}}{{2{s_1}{s_2}}}$, ${m_1} = E + \sqrt {{E^2} - 1}$. By using (2.12), we obtain

where ${m^2} = \frac{{m_1^2 - 1}}{{m_1^2}}$.

By the definition of the Jacobian elliptic function, we obtain that

Then, we get the solution of Eq (1.1):

From Eqs (3.20), (3.21) and (3.22), we have obtained three biperiodic traveling wave solutions of Eq (1.1).

4.   Numerical simulation

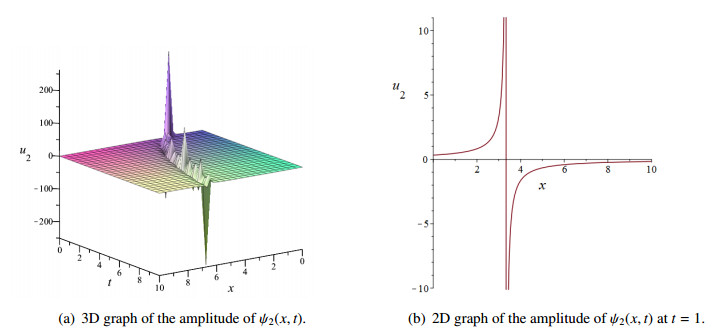
In this section, we describe the use of the mathematical software Maple to obtain the dynamical features of Eq (1.1). The results of numerical simulations are presented here to show the amplitude functions of the obtained solutions. Upon choosing suitable parameters, the main features of $\psi_2(x, t)$, $\psi_5(x, t)$, $\psi_{11}(x, t)$, $\psi_{17}(x, t)$ are shown in Figures 1, 2, 3 and 4, respectively.

Figure 1 shows the amplitude of the rational function solution. Figures 2, 3 and 4 show the amplitudes of the three kinds of biperiodic function solutions.

5.   Conclusions

In this paper, a series of new optical solitons and single traveling wave solutions of the BAE in birefringent fibers with the beta-time derivative have been successfully obtained. These solutions include hyperbolic function solutions, rational wave solutions, Jacobi elliptic solutions and triangular functions solutions. The solutions obtained in this study not only contain the conclusions of the existing solutions, but they also contain the solutions in the forms of rational functions and trigonometric functions. These obtained solutions form a complete classification of single wave solutions. Finally, with the aid of the symbolic computational software Maple, we have presented 3D and 2D visualizations of some obtained solutions.

Compared with the existing results, the polynomial complete discriminant system method is a reliable and efficient technique. The author of ^[17] obtained a series of traveling wave solutions involving hyperbolic functions, and those of ^[18] obtained a series of traveling wave solutions involving hyperbolic functions and the Jacobian elliptic function. The traveling wave solution obtained in this study contains not only hyperbolic function and Jacobian elliptic function solutions, but also rational function and trigonometric function solutions. This method allows us to solve the single wave solutions of more types of PDEs. The results of this study may help us to explore new phenomena that may appear in Eq (1.1). In further research, we will use the idea proposed in this paper to study the optical solitons and single traveling wave solutions of BAE with random terms or other fractional derivative terms.

The limitations of this study are as follows. The references cited are this papers that have been published in SCI journals in the past 10 years, and thus do not include papers from less influential journals. Therefore, we remind the readers to pay attention to the limitations of the time and journal source when reading this article.

Conflict of interest

The authors declare no conflict of interest.

Acknowledgements

The authors are grateful to the anonymous reviewers for their careful reading and useful suggestions, which have greatly improved the presentation of the paper. This work was supported by Scientific Research Funds from Chengdu University under grant no.2081920034.