| 1.
|
Usman A. Danbaba, Salisu M. Garba,
Modeling the transmission dynamics of Zika with sterile insect technique,
2018,
41,
01704214,
8871,
10.1002/mma.5336
|
|
| 2.
|
Hajar Besbassi, Khalid Hattaf, Noura Yousfi, Abdul Qadeer Khan,
Stability and Hopf Bifurcation of a Generalized Chikungunya Virus Infection Model with Two Modes of Transmission and Delays,
2020,
2020,
1607-887X,
1,
10.1155/2020/5908976
|
|
| 3.
|
Hammami Pachka, Tran Annelise, Kemp Alan, Tshikae Power, Kgori Patrick, Chevalier Véronique, Paweska Janusz, Jori Ferran,
Rift Valley fever vector diversity and impact of meteorological and environmental factors on Culex pipiens dynamics in the Okavango Delta, Botswana,
2016,
9,
1756-3305,
10.1186/s13071-016-1712-1
|
|
| 4.
|
Alessandra Lo Presti, Eleonora Cella, Silvia Angeletti, Massimo Ciccozzi,
Molecular epidemiology, evolution and phylogeny of Chikungunya virus: An updating review,
2016,
41,
15671348,
270,
10.1016/j.meegid.2016.04.006
|
|
| 5.
|
Helena Sofia Rodrigues, M. Teresa T. Monteiro, Delfim F. M. Torres, Alan Zinober,
Dengue disease, basic reproduction number and control,
2012,
89,
0020-7160,
334,
10.1080/00207160.2011.554540
|
|
| 6.
|
Salisu M. Garba, Usman A. Danbaba, Jacek Banasiak,
Modeling the effect of temperature variability on malaria control strategies,
2020,
15,
0973-5348,
65,
10.1051/mmnp/2020044
|
|
| 7.
|
Louis Clément Gouagna, Jean-Sébastien Dehecq, Didier Fontenille, Yves Dumont, Sébastien Boyer,
Seasonal variation in size estimates of Aedes albopictus population based on standard mark–release–recapture experiments in an urban area on Reunion Island,
2015,
143,
0001706X,
89,
10.1016/j.actatropica.2014.12.011
|
|
| 8.
|
Shangbing Ai, Jia Li, Junliang Lu,
Mosquito-Stage-Structured Malaria Models and Their Global Dynamics,
2012,
72,
0036-1399,
1213,
10.1137/110860318
|
|
| 9.
|
Helena Sofia Rodrigues, M. Teresa T. Monteiro, Delfim F.M. Torres,
Vaccination models and optimal control strategies to dengue,
2014,
247,
00255564,
1,
10.1016/j.mbs.2013.10.006
|
|
| 10.
|
Anne Fischer, Kurt Chudej, Hans Josef Pesch,
Optimal vaccination and control strategies against dengue,
2019,
42,
0170-4214,
3496,
10.1002/mma.5594
|
|
| 11.
|
Y. Dumont, J. Thuilliez,
Human behaviors: A threat to mosquito control?,
2016,
281,
00255564,
9,
10.1016/j.mbs.2016.08.011
|
|
| 12.
|
Xinzhi Liu, Peter Stechlinski,
2017,
Chapter 6,
978-3-319-53206-6,
179,
10.1007/978-3-319-53208-0_6
|
|
| 13.
|
Diana P. Iyaloo, Pascal Degenne, Khouaildi Bin Elahee, Danny Lo Seen, Ambicadutt Bheecarry, Annelise Tran,
ALBOMAURICE: A predictive model for mapping Aedes albopictus mosquito populations in Mauritius,
2021,
13,
23527110,
100638,
10.1016/j.softx.2020.100638
|
|
| 14.
|
Irfan A. Rather, Hilal A. Parray, Jameel B. Lone, Woon K. Paek, Jeongheui Lim, Vivek K. Bajpai, Yong-Ha Park,
Prevention and Control Strategies to Counter Dengue Virus Infection,
2017,
7,
2235-2988,
10.3389/fcimb.2017.00336
|
|
| 15.
|
Grant D. Brown, Aaron T. Porter, Jacob J. Oleson, Jessica A. Hinman,
Approximate Bayesian computation for spatial SEIR(S) epidemic models,
2018,
24,
18775845,
27,
10.1016/j.sste.2017.11.001
|
|
| 16.
|
Marguerite Robinson, Anne Conan, Veasna Duong, Sowath Ly, Chantha Ngan, Philippe Buchy, Arnaud Tarantola, Xavier Rodó, Michael J. Turell,
A Model for a Chikungunya Outbreak in a Rural Cambodian Setting: Implications for Disease Control in Uninfected Areas,
2014,
8,
1935-2735,
e3120,
10.1371/journal.pntd.0003120
|
|
| 17.
|
D. Moulay, M.A. Aziz-Alaoui, M. Cadivel,
The chikungunya disease: Modeling, vector and transmission global dynamics,
2011,
229,
00255564,
50,
10.1016/j.mbs.2010.10.008
|
|
| 18.
|
Kiran Bala Bhuyan, Arpita Arsmika Sahu, T. Sarita Achari, Tapan Kumar Barik,
2020,
Chapter 10,
978-981-15-9455-7,
209,
10.1007/978-981-15-9456-4_10
|
|
| 19.
|
Xinzhi Liu, Peter Stechlinski,
2017,
Chapter 4,
978-3-319-53206-6,
83,
10.1007/978-3-319-53208-0_4
|
|
| 20.
|
José V.J. Silva, Louisa F. Ludwig-Begall, Edmilson F. de Oliveira-Filho, Renato A.S. Oliveira, Ricardo Durães-Carvalho, Thaísa R.R. Lopes, Daisy E.A. Silva, Laura H.V.G. Gil,
A scoping review of Chikungunya virus infection: epidemiology, clinical characteristics, viral co-circulation complications, and control,
2018,
188,
0001706X,
213,
10.1016/j.actatropica.2018.09.003
|
|
| 21.
|
Shousheng Zhu, Nathalie Verdière, Lilianne Denis-Vidal, Djalil Kateb,
Identifiability analysis and parameter estimation of a chikungunya model in a spatially continuous domain,
2018,
34,
1476945X,
80,
10.1016/j.ecocom.2017.12.004
|
|
| 22.
|
UA Danbaba, SM Garba,
Stability Analysis and Optimal Control for Yellow Fever Model with Vertical Transmission,
2020,
6,
2349-5103,
10.1007/s40819-020-00860-z
|
|
| 23.
|
Xinzhi Liu, Peter Stechlinski,
Application of control strategies to a seasonal model of chikungunya disease,
2015,
39,
0307904X,
3194,
10.1016/j.apm.2014.10.035
|
|
| 24.
|
Jing Chen, John C. Beier, Robert Stephen Cantrell, Chris Cosner, Douglas O. Fuller, Yongtao Guan, Guoyan Zhang, Shigui Ruan,
Modeling the importation and local transmission of vector-borne diseases in Florida: The case of Zika outbreak in 2016,
2018,
455,
00225193,
342,
10.1016/j.jtbi.2018.07.026
|
|
| 25.
|
Gerhart Knerer, Christine S. M. Currie, Sally C. Brailsford, Donald S. Shepard,
The economic impact and cost-effectiveness of combined vector-control and dengue vaccination strategies in Thailand: results from a dynamic transmission model,
2020,
14,
1935-2735,
e0008805,
10.1371/journal.pntd.0008805
|
|
| 26.
|
Xianning Liu, Yan Wang, Xiao-Qiang Zhao,
Dynamics of a periodic Chikungunya model with temperature and rainfall effects,
2020,
90,
10075704,
105409,
10.1016/j.cnsns.2020.105409
|
|
| 27.
|
Tridip Sardar, Sourav Kumar Sasmal, Joydev Chattopadhyay,
Estimating dengue type reproduction numbers for two provinces of Sri Lanka during the period 2013–14,
2016,
7,
2150-5594,
187,
10.1080/21505594.2015.1096470
|
|
| 28.
|
Marcos Amaku, Francisco Antonio Bezerra Coutinho, Silvia Martorano Raimundo, Luis Fernandez Lopez, Marcelo Nascimento Burattini, Eduardo Massad,
A Comparative Analysis of the Relative Efficacy of Vector-Control Strategies Against Dengue Fever,
2014,
76,
0092-8240,
697,
10.1007/s11538-014-9939-5
|
|
| 29.
|
Ying Liu, Kate Lillepold, Jan C. Semenza, Yesim Tozan, Mikkel B.M. Quam, Joacim Rocklöv,
Reviewing estimates of the basic reproduction number for dengue, Zika and chikungunya across global climate zones,
2020,
182,
00139351,
109114,
10.1016/j.envres.2020.109114
|
|
| 30.
|
Taofeek O. Alade,
On the generalized Chikungunya virus dynamics model with distributed time delays,
2020,
2195-268X,
10.1007/s40435-020-00723-x
|
|
| 31.
|
Najmul Haider, Francesco Vairo, Giuseppe Ippolito, Alimuddin Zumla, Richard A. Kock,
Basic Reproduction Number of Chikungunya Virus Transmitted by Aedes Mosquitoes,
2020,
26,
1080-6040,
2429,
10.3201/eid2610.190957
|
|
| 32.
|
Martial L. Ndeffo-Mbah, David P. Durham, Laura A. Skrip, Elaine O. Nsoesie, John S. Brownstein, Durland Fish, Alison P. Galvani,
Evaluating the effectiveness of localized control strategies to curtail chikungunya,
2016,
6,
2045-2322,
10.1038/srep23997
|
|
| 33.
|
K. C. Patidar,
Nonstandard finite difference methods: recent trends and further developments,
2016,
22,
1023-6198,
817,
10.1080/10236198.2016.1144748
|
|
| 34.
|
Helena Sofia Rodrigues, M. Teresa T. Monteiro, Delfim F. M. Torres,
Sensitivity Analysis in a Dengue Epidemiological Model,
2013,
2013,
2314-4777,
1,
10.1155/2013/721406
|
|
| 35.
|
Yan Wang, Xianning Liu,
Stability and Hopf bifurcation of a within-host chikungunya virus infection model with two delays,
2017,
138,
03784754,
31,
10.1016/j.matcom.2016.12.011
|
|
| 36.
|
Ahmed M. Elaiw, Sami E. Almalki, A.D. Hobiny, Ahmed Farouk,
Stability of delayed CHIKV dynamics model with cell-to-cell transmission,
2020,
38,
10641246,
2425,
10.3233/JIFS-179531
|
|
| 37.
|
Ahmed M. Elaiw, Taofeek O. Alade, Saud M. Alsulami,
Global dynamics of delayed CHIKV infection model with multitarget cells,
2019,
60,
1598-5865,
303,
10.1007/s12190-018-1215-7
|
|
| 38.
|
Eminugroho Ratna Sari, Nur Insani, Dwi Lestari,
The Preventive Control of a Dengue Disease Using Pontryagin Minimum Principal,
2017,
855,
1742-6588,
012045,
10.1088/1742-6596/855/1/012045
|
|
| 39.
|
A. M. Elaiw, S. E. Almalki, A. D. Hobiny,
Stability of CHIKV infection models with CHIKV-monocyte and infected-monocyte saturated incidences,
2019,
9,
2158-3226,
025308,
10.1063/1.5085804
|
|
| 40.
|
Carrie A. Manore, Richard S. Ostfeld, Folashade B. Agusto, Holly Gaff, Shannon L. LaDeau, Samuel V. Scarpino,
Defining the Risk of Zika and Chikungunya Virus Transmission in Human Population Centers of the Eastern United States,
2017,
11,
1935-2735,
e0005255,
10.1371/journal.pntd.0005255
|
|
| 41.
|
Annelise Tran, Grégory L'Ambert, Guillaume Lacour, Romain Benoît, Marie Demarchi, Myriam Cros, Priscilla Cailly, Mélaine Aubry-Kientz, Thomas Balenghien, Pauline Ezanno,
A Rainfall- and Temperature-Driven Abundance Model for Aedes albopictus Populations,
2013,
10,
1660-4601,
1698,
10.3390/ijerph10051698
|
|
| 42.
|
Derdei Bichara, Carlos Castillo-Chavez,
Vector-borne diseases models with residence times – A Lagrangian perspective,
2016,
281,
00255564,
128,
10.1016/j.mbs.2016.09.006
|
|
| 43.
|
Lin Ling, Guirong Jiang, Tengfei Long,
The dynamics of an SIS epidemic model with fixed-time birth pulses and state feedback pulse treatments,
2015,
39,
0307904X,
5579,
10.1016/j.apm.2015.01.022
|
|
| 44.
|
Claire Dufourd, Yves Dumont,
Impact of environmental factors on mosquito dispersal in the prospect of sterile insect technique control,
2013,
66,
08981221,
1695,
10.1016/j.camwa.2013.03.024
|
|
| 45.
|
Xianning Liu, Yan Wang, Xiao-Qiang Zhao,
Dynamics of a climate-based periodic Chikungunya model with incubation period,
2020,
80,
0307904X,
151,
10.1016/j.apm.2019.11.038
|
|
| 46.
|
Joanna Waldock, Nastassya L. Chandra, Jos Lelieveld, Yiannis Proestos, Edwin Michael, George Christophides, Paul E. Parham,
The role of environmental variables onAedes albopictusbiology and chikungunya epidemiology,
2013,
107,
2047-7724,
224,
10.1179/2047773213Y.0000000100
|
|
| 47.
|
Folashade B. Agusto,
Optimal control and cost-effectiveness analysis of a three age-structured transmission dynamics of chikungunya virus,
2017,
22,
1553-524X,
687,
10.3934/dcdsb.2017034
|
|
| 48.
|
Helena Sofia Rodrigues, M. Teresa T. Monteiro, Delfim F. M. Torres,
Seasonality effects on dengue: basic reproduction number, sensitivity analysis and optimal control,
2016,
39,
01704214,
4671,
10.1002/mma.3319
|
|
| 49.
|
K.F. Gurski,
A simple construction of nonstandard finite-difference schemes for small nonlinear systems applied to SIR models,
2013,
66,
08981221,
2165,
10.1016/j.camwa.2013.06.034
|
|
| 50.
|
Jayanta Kumar Ghosh, Uttam Ghosh, Susmita Sarkar,
Qualitative Analysis and Optimal Control of a Two-Strain Dengue Model with its Co-infections,
2020,
6,
2349-5103,
10.1007/s40819-020-00905-3
|
|
| 51.
|
Yu-Han Kao, Marisa C. Eisenberg,
Practical unidentifiability of a simple vector-borne disease model: Implications for parameter estimation and intervention assessment,
2018,
25,
17554365,
89,
10.1016/j.epidem.2018.05.010
|
|
| 52.
|
Laith Yakob, Archie C. A. Clements, Michael George Roberts,
A Mathematical Model of Chikungunya Dynamics and Control: The Major Epidemic on Réunion Island,
2013,
8,
1932-6203,
e57448,
10.1371/journal.pone.0057448
|
|
| 53.
|
Clelia F. Oliva, Maxime Jacquet, Jeremie Gilles, Guy Lemperiere, Pierre-Olivier Maquart, Serge Quilici, François Schooneman, Marc J. B. Vreysen, Sebastien Boyer, Basil Brooke,
The Sterile Insect Technique for Controlling Populations of Aedes albopictus (Diptera: Culicidae) on Reunion Island: Mating Vigour of Sterilized Males,
2012,
7,
1932-6203,
e49414,
10.1371/journal.pone.0049414
|
|
| 54.
|
HELENA SOFIA RODRIGUES, M. TERESA T. MONTEIRO, DELFIM F. M. TORRES,
Dengue in Cape Verde: Vector Control and Vaccination,
2013,
20,
0889-8480,
208,
10.1080/08898480.2013.831711
|
|
| 55.
|
Roumen Anguelov, Yves Dumont, Ivric Valaire Yatat Djeumen,
Sustainable vector/pest control using the permanent sterile insect technique,
2020,
43,
0170-4214,
10391,
10.1002/mma.6385
|
|
| 56.
|
Rebecca C. Christofferson, Daniel M. Chisenhall, Helen J. Wearing, Christopher N. Mores, Lisa FP. Ng,
Chikungunya Viral Fitness Measures within the Vector and Subsequent Transmission Potential,
2014,
9,
1932-6203,
e110538,
10.1371/journal.pone.0110538
|
|
| 57.
|
Sylvestre Aureliano Carvalho, Stella Olivia da Silva, Iraziet da Cunha Charret,
Mathematical modeling of dengue epidemic: control methods and vaccination strategies,
2019,
138,
1431-7613,
223,
10.1007/s12064-019-00273-7
|
|
| 58.
|
Taofeek O. Alade, Ahmed M. Elaiw, Saud M. Alsulami,
Stability dynamics of a delayed generalized Chikungunya virus infection model,
2021,
65,
1598-5865,
575,
10.1007/s12190-020-01405-9
|
|
| 59.
|
A. M. Elaiw, S. E. Almalki, A. D. Hobiny,
Global properties of saturated chikungunya virus dynamics models with cellular infection and delays,
2019,
2019,
1687-1847,
10.1186/s13662-019-2409-5
|
|
| 60.
|
Folashade B. Agusto, Shamise Easley, Kenneth Freeman, Madison Thomas,
Mathematical Model of Three Age-Structured Transmission Dynamics of Chikungunya Virus,
2016,
2016,
1748-670X,
1,
10.1155/2016/4320514
|
|
| 61.
|
Annelise Tran, Morgan Mangeas, Marie Demarchi, Emmanuel Roux, Pascal Degenne, Marion Haramboure, Gilbert Le Goff, David Damiens, Louis-Clément Gouagna, Vincent Herbreteau, Jean-Sébastien Dehecq, Suzanne Touzeau,
Complementarity of empirical and process-based approaches to modelling mosquito population dynamics with Aedes albopictus as an example—Application to the development of an operational mapping tool of vector populations,
2020,
15,
1932-6203,
e0227407,
10.1371/journal.pone.0227407
|
|
| 62.
|
Djamila Moulay, Yoann Pigné,
A metapopulation model for chikungunya including populations mobility on a large-scale network,
2013,
318,
00225193,
129,
10.1016/j.jtbi.2012.11.008
|
|
| 63.
|
Optimal control of chikungunya disease: Larvae reduction, treatment and prevention,
2012,
9,
1551-0018,
369,
10.3934/mbe.2012.9.369
|
|
| 64.
|
Thomas Sochacki, Frédéric Jourdain, Yvon Perrin, Harold Noel, Marie-Claire Paty, Henriette de Valk, Alexandra Septfons, Frédéric Simard, Didier Fontenille, Benjamin Roche,
Imported chikungunya cases in an area newly colonised by Aedes albopictus: mathematical assessment of the best public health strategy,
2016,
21,
1560-7917,
10.2807/1560-7917.ES.2016.21.18.30221
|
|
| 65.
|
Hamadjam Abboubakar, Jean Claude Kamgang, Leontine Nkague Nkamba, Daniel Tieudjo,
Bifurcation thresholds and optimal control in transmission dynamics of arboviral diseases,
2018,
76,
0303-6812,
379,
10.1007/s00285-017-1146-1
|
|
| 66.
|
Yanyuan Xing, Zhiming Guo, Jian Liu,
Backward bifurcation in a malaria transmission model,
2020,
14,
1751-3758,
368,
10.1080/17513758.2020.1771443
|
|
| 67.
|
Ahmed M. Elaiw, Taofeek O. Alade, Saud M. Alsulami,
Analysis of latent CHIKV dynamics models with general incidence rate and time delays,
2018,
12,
1751-3758,
700,
10.1080/17513758.2018.1503349
|
|
| 68.
|
Ahmed M. Elaiw, Taofeek O. Alade, Saud M. Alsulami,
Analysis of within-host CHIKV dynamics models with general incidence rate,
2018,
11,
1793-5245,
1850062,
10.1142/S1793524518500626
|
|
| 69.
|
Catherine Hierlihy, Lisa Waddell, Ian Young, Judy Greig, Tricia Corrin, Mariola Mascarenhas, Abdallah M. Samy,
A systematic review of individual and community mitigation measures for prevention and control of chikungunya virus,
2019,
14,
1932-6203,
e0212054,
10.1371/journal.pone.0212054
|
|
| 70.
|
Helena Sofia Rodrigues, M. Teresa T. Monteiro, Delfim F. M. Torres, Ana Clara Silva, Carla Sousa, Cláudia Conceição,
2015,
Chapter 32,
978-3-319-16117-4,
593,
10.1007/978-3-319-16118-1_32
|
|
| 71.
|
XINZHI LIU, PETER STECHLINSKI,
SWITCHING VACCINATION SCHEMES FOR VECTOR-BORNE DISEASES WITH SEASONAL FLUCTUATIONS,
2017,
25,
0218-3390,
441,
10.1142/S0218339017500218
|
|
| 72.
|
M. Chapwanya, Y. Dumont,
On crop vector-borne diseases. Impact of virus lifespan and contact rate on the traveling-wave speed of infective fronts,
2018,
34,
1476945X,
119,
10.1016/j.ecocom.2017.08.002
|
|
| 73.
|
Hamadjam Abboubakar, Jean Claude Kamgang, Daniel Tieudjo,
Backward bifurcation and control in transmission dynamics of arboviral diseases,
2016,
278,
00255564,
100,
10.1016/j.mbs.2016.06.002
|
|
| 74.
|
Xinzhi Liu, Peter Stechlinski,
2017,
Chapter 7,
978-3-319-53206-6,
227,
10.1007/978-3-319-53208-0_7
|
|
| 75.
|
Léa Douchet, Marion Haramboure, Thierry Baldet, Gregory L’Ambert, David Damiens, Louis Clément Gouagna, Jeremy Bouyer, Pierrick Labbé, Annelise Tran,
Comparing sterile male releases and other methods for integrated control of the tiger mosquito in temperate and tropical climates,
2021,
11,
2045-2322,
10.1038/s41598-021-86798-8
|
|
| 76.
|
Harrison Watts, Arti Mishra, Dang H. Nguyen, Tran D. Tuong,
Dynamics of a vector-host model under switching environments,
2021,
0,
1553-524X,
0,
10.3934/dcdsb.2021029
|
|
| 77.
|
Guangming Qiu, Sanyi Tang, Mengqi He, Binxiang Dai,
Analysis of a High-Dimensional Mathematical Model for Plant Virus Transmission with Continuous and Impulsive Roguing Control,
2021,
2021,
1607-887X,
1,
10.1155/2021/6177132
|
|
| 78.
|
Habeeb M. Al-Solami, Alawiah M. S. Alhebshi, H. Abdo, S. R. Mahmuod, Afaf S. Alwabli, Naser Alkenani,
A bio-mathematical approach to control the Anopheles mosquito using sterile males technology,
2022,
15,
1793-5245,
10.1142/S1793524522500371
|
|
| 79.
|
S. A. Abdullahi, N. Hussaini, A. G. Habib,
Mathematical Model of In-host Dynamics of Snakebite Envenoming,
2022,
2714-4704,
193,
10.46481/jnsps.2022.548
|
|
| 80.
|
Mourad Chamekh, Mohamed Ali Latrach, Fadel Jday,
Multi-step semi-analytical solutions for a chikungunya virus system,
2023,
2731-6734,
10.1007/s43994-023-00027-8
|
|
| 81.
|
Taofeek O. Alade, Mohammad Alnegga, Samson Olaniyi, Afeez Abidemi,
Mathematical modelling of within-host Chikungunya virus dynamics with adaptive immune response,
2023,
2363-6203,
10.1007/s40808-023-01737-y
|
|
| 82.
|
Ramakant Prasad, Surendra Kumar Sagar, Shama Parveen, Ravins Dohare,
Mathematical modeling in perspective of vector-borne viral infections: a review,
2022,
11,
2314-8543,
10.1186/s43088-022-00282-4
|
|
| 83.
|
C. Gokila, M. Sambath,
The threshold for a stochastic within-host CHIKV virus model with saturated incidence rate,
2021,
14,
1793-5245,
2150042,
10.1142/S179352452150042X
|
|
| 84.
|
Ahmed Alshehri, Miled El Hajji,
Mathematical study for Zika virus transmission with general incidence rate,
2022,
7,
2473-6988,
7117,
10.3934/math.2022397
|
|
| 85.
|
Hamadjam Abboubakar, Reinhard Racke,
Mathematical modeling, forecasting, and optimal control of typhoid fever transmission dynamics,
2021,
149,
09600779,
111074,
10.1016/j.chaos.2021.111074
|
|
| 86.
|
Chatthai Thaiprayoon, Jutarat Kongson, Weerawat Sudsutad, Jehad Alzabut, Sina Etemad, Shahram Rezapour,
Analysis of a nonlinear fractional system for Zika virus dynamics with sexual transmission route under generalized Caputo-type derivative,
2022,
68,
1598-5865,
4273,
10.1007/s12190-021-01663-1
|
|
| 87.
|
Nur ’Izzati Hamdan, Adem Kilicman,
Mathematical Modelling of Dengue Transmission with Intervention Strategies Using Fractional Derivatives,
2022,
84,
0092-8240,
10.1007/s11538-022-01096-2
|
|
| 88.
|
Y. Dumont, I.V. Yatat–Djeumen,
Sterile insect technique with accidental releases of sterile females. Impact on mosquito-borne diseases control when viruses are circulating,
2022,
343,
00255564,
108724,
10.1016/j.mbs.2021.108724
|
|
| 89.
|
Yan Wang, Yazhi Li, Xinzhi Ren, Xianning Liu,
A periodic dengue model with diapause effect and control measures,
2022,
108,
0307904X,
469,
10.1016/j.apm.2022.03.043
|
|
| 90.
|
Yan Wang, Yazhi Li, Lili Liu, Xianning Liu,
A periodic Chikungunya model with virus mutation and transovarial transmission,
2022,
158,
09600779,
112002,
10.1016/j.chaos.2022.112002
|
|
| 91.
|
Mohammad Enamul Hoque Kayesh, Ibrahim Khalil, Michinori Kohara, Kyoko Tsukiyama-Kohara,
Increasing Dengue Burden and Severe Dengue Risk in Bangladesh: An Overview,
2023,
8,
2414-6366,
32,
10.3390/tropicalmed8010032
|
|
| 92.
|
Mlyashimbi Helikumi, Gideon Eustace, Steady Mushayabasa, Jan Rychtar,
Dynamics of a Fractional-Order Chikungunya Model with Asymptomatic Infectious Class,
2022,
2022,
1748-6718,
1,
10.1155/2022/5118382
|
|
| 93.
|
Jing Chen, Xi Huo, André B.B. Wilke, John C. Beier, Chalmers Vasquez, William Petrie, Robert Stephen Cantrell, Chris Cosner, Shigui Ruan,
Linking mathematical models and trap data to infer the proliferation, abundance, and control of Aedes aegypti,
2023,
239,
0001706X,
106837,
10.1016/j.actatropica.2023.106837
|
|
| 94.
|
Preety Kalra, Indu Ratti,
Mathematical Modeling on Yellow Fever with Effect of Awareness Through Media,
2022,
2267,
1742-6588,
012034,
10.1088/1742-6596/2267/1/012034
|
|
| 95.
|
Frédéric Jourdain, Henriette de Valk, Harold Noël, Marie-Claire Paty, Grégory L’Ambert, Florian Franke, Damien Mouly, Jean-Claude Desenclos, Benjamin Roche, Xavier Rodo,
Estimating chikungunya virus transmission parameters and vector control effectiveness highlights key factors to mitigate arboviral disease outbreaks,
2022,
16,
1935-2735,
e0010244,
10.1371/journal.pntd.0010244
|
|
| 96.
|
Brianne M. Hibl, Natalie J. M. Dailey Garnes, Alexander R. Kneubehl, Megan B. Vogt, Jennifer L. Spencer Clinton, Rebecca R. Rico-Hesse, Michael R. Holbrook,
Mosquito-bite infection of humanized mice with chikungunya virus produces systemic disease with long-term effects,
2021,
15,
1935-2735,
e0009427,
10.1371/journal.pntd.0009427
|
|
| 97.
|
Zhimin Li, Xiao-Qiang Zhao,
Global dynamics of a Nonlocal Periodic Reaction–Diffusion Model of Chikungunya Disease,
2023,
1040-7294,
10.1007/s10884-023-10267-1
|
|
| 98.
|
María Guadalupe Vázquez-Peña, Cruz Vargas-De-León, Jorge Fernando Camacho-Pérez, Jorge Velázquez-Castro,
Analysis and Bayesian estimation of a model for Chikungunya dynamics with relapse: An outbreak in Acapulco, Mexico,
2023,
20,
1551-0018,
18123,
10.3934/mbe.2023805
|
|
| 99.
|
Rubin Fandio, Hamadjam Abboubakar, Henri Paul Ekobena Fouda, Anoop Kumar, Kottakkaran Sooppy Nisar,
Mathematical modelling and projection of Buruli ulcer transmission dynamics using classical and fractional derivatives: A case study of Cameroon,
2023,
26668181,
100589,
10.1016/j.padiff.2023.100589
|
|
| 100.
|
Abdoulaye Kaboré, Boureima Sangaré, Bakary Traoré,
Mathematical model of mosquito population dynamics with constants and periodic releases of
Wolbachia
-infected males
,
2024,
32,
2769-0911,
10.1080/27690911.2024.2372581
|
|
| 101.
|
Jayanta Kumar Ghosh, Partha Biswas, Sudhanshu Kumar Biswas, Susmita Sarkar, Uttam Ghosh,
Qualitative and optimal control analysis of a two-serotype dengue model with saturated incidence in co-infection,
2024,
0228-6203,
1,
10.1080/02286203.2024.2414955
|
|
| 102.
|
Samia Shaikh, Padakanti Sandeep Chary, Neelesh Kumar Mehra,
Nano-interventions for dengue: a comprehensive review of control, detection and treatment strategies,
2025,
0925-4692,
10.1007/s10787-025-01655-8
|
|
| 103.
|
Gopal Chandra Sikdar, Pritam Saha, Uttam Ghosh,
Effect of cross-immunity on the transmission of a two serotypes co-infected dengue model under deterministic and stochastic environments,
2025,
0924-090X,
10.1007/s11071-025-11172-6
|
|










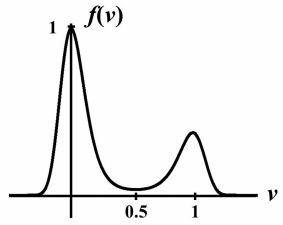
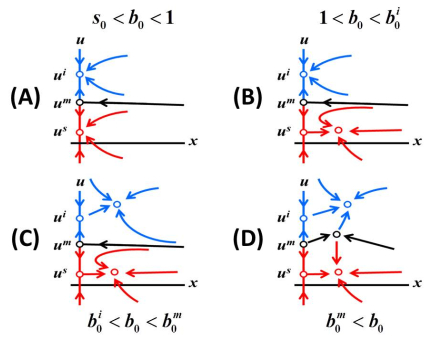

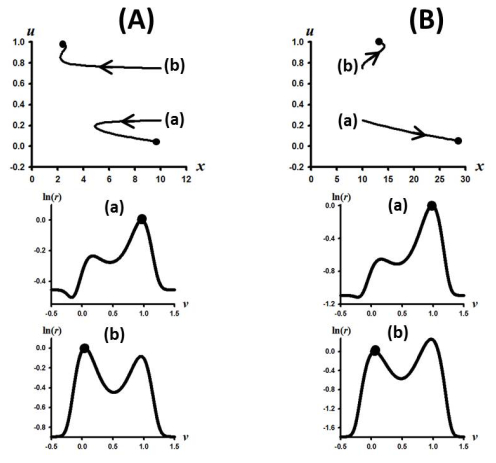


 DownLoad:
DownLoad: