Homogenization of nonlinear hyperbolic problem with a dynamical boundary condition

1.   Introduction

The study of integral-type, non-local boundary conditions are fascinating area of rapidly evolving differential equations theory. Non-local boundary conditions are useful in a variety of contexts, including wave equations, electric conduction, petroleum exploitation, heat diffusion, and the elastic behavior of perforated materials, see for instance ^[1,15,24,26]. Mathematicians, engineers and applied scientist intensively invest gated problems with non-local boundary conditions, from theoretical and computational point of views, see for instance ^{[7,10,17,18,19]}. For homogenization for boundary value problems with Dirichlet Nuemann and Robin conditions, we refer to ^[9,12,14]. The first work dealing with homogenization for non-local boundary problems we refer to ^[10], where the authors investigated the periodic homogenization using the periodic unfolding techniques for the elastic torsion problem of an infinite 3-dimensional rod along with the overall electro-conductivity problem within presence of a significant number of excellent conductors. Their results were further developed to time-dependent problems by Amar et al. in ^[2,3], where the authors obtained homogenization for classes of linear parabolic problems with non-local boundaries using the periodic unfolding techniques. The results in ^[2] are for a linear parabolic problem with special oscillations in the coefficients, whereas the results in ^[3] are for a linear parabolic problem with time space oscillations in the coefficients. To the best of the author's knowledge, there are no results for homogenization of boundary value problems with non-local boundary conditions in the hyperbolic framework. The goal of this paper is to extend the results of ^[2] by looking into homogenization results for a nonlinear hyperbolic problem with a non-local boundary condition involving the solution's time derivative. The author's forthcoming studies will concentrate on homogenization of hyperbolic problems with nonlocal boundary conditions when the coefficients oscillate in both the space and time variables at different scales, which is more technical and requires more complicated analysis. As for homogenization of deterministic linear hyperbolic problems with Dirichlet and Neumann boundary conditions, we refer to ^[16] and the references therein. For homogenization for nonlinear hyperbolic problems with Dirichlet boundary condition, see ^[25], where the authors obtained homogenization for similar model to the one in this paper, but with Dirichlet condition using multi-scale convergence in fixed domain. We also mention the work on homogenization of hyperbolic SPDEs, see ^[20,21,22]. Here, we consider the following nonlinear hyperbolic problem with non-local boundary condition.

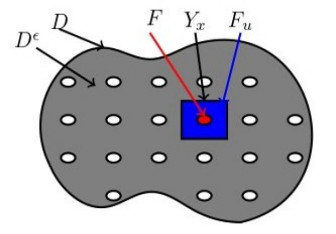
For all $(x, y, t) \in D\times Y_x \times (0, T)$. The domain in which this problem is studied is described further below.

● $F$ is an open subset of $\mathbb{R}^{n}$ such that $F+z = F$ for all $z\in \mathbb{Z}^{n}$.

● $Y_x = (0, 1)\times(0, 1)\times \cdots \times(0, 1)\subset \mathbb{R}^{n}$.

● $F_u = F\cap Y_x$, $F_s = Y_x\backslash \bar{F}$, $\Gamma = \partial F \cap Y_x$ and $\partial F_u \cap \partial Y_x = \phi$ which implies that $\partial F_u = \Gamma$.

● $D$ is an open connected and bounded subset of $\mathbb{R}^{n}$ and and $D_T = D\times (0, T)$.

● $\mathbb{X}_{\epsilon} = \{\eta \in \mathbb{Z}^{n}:\epsilon(\eta+Y_x)\subset D\}$, where $\epsilon$ represents a sequence of positive real numbers that tends to zero.

● $F^{\epsilon}_{\eta}: = \epsilon(F_u+\eta)$ and $\Gamma^{\epsilon}_{\eta} = \partial F^{\epsilon}_{\eta}$.

● $F^{\epsilon} = \bigcup_{\eta\in\mathbb{X}_{\epsilon}}F^{\epsilon}_{\eta}$ is disconnected with smooth boundary and $\Gamma^{\epsilon} = \partial F^{\epsilon}$.

● $D^{\epsilon} = D\backslash \bar{F^{\epsilon}}$ is connected.

● $\nu$ is the unit outward normal on $\Gamma$ and it is extended to $\mathbb{R}^n$ by periodicity.

● $\nu_{\epsilon} = \nu\left(\frac{x}{\epsilon}\right)$ is the unit outward normal on $\Gamma^{\epsilon}$.

Let us mention that $\frac{\partial v^\epsilon}{\partial \nu_{\epsilon A^{\epsilon}}} = \sum_{i, j = 1}^{n}a_{i, j}\left(\frac{x}{\epsilon}\right)\cdot\nu_j\left(\frac{x}{\epsilon}\right)\frac{\partial v^\epsilon}{\partial x_i}$. For a better understanding of the domain, we add Figure 1.

Let us state our data assumptions.

$({\rm{H1}})$ $B_\eta(t)$ is a constant function with respect to the spatial variable $x$ depending on $\eta$ and $t$.

$({\rm{H2}})$ $A = (a_{i, j})_{1\leq i, j \leq n}$ a symmetric matrix such that $a_{i, j}\in L^{\infty}(D; L^{\infty}_{\text{per}}(Y_x))$ where $A^{\epsilon}(x) = A\left(x, \frac{x}{\epsilon}\right)$ such that

$({\rm{H3}})$ $\beta^{\epsilon}(t, x, v^\epsilon, \nabla v^\epsilon) = \beta\left(\frac{x}{\epsilon}, v^\epsilon, \nabla v^\epsilon \right)$ is measurable with respect to $(\varphi, \psi) \in \mathbb{R}\times \mathbb{R}^n$ and $Y_x$-periodic with respect to the first arguments, such that

${\rm{(a)}}$ $|\beta(y, \varphi, \psi)|\leq c_0(1+|\varphi|^{r+1}+|\psi|)$.

${\rm{(b) }}$ $\beta(y, \varphi, \psi)\Phi \geq c_1 |\varphi|^r \varphi \Phi-c_2(1+|\Phi||\psi|)$.

${\rm{(c) }}$ $\left|\frac{\partial}{\partial \varphi}\beta(y, \varphi, \psi)\right|\leq c_0(1+|\varphi|^{r})$.

${\rm{ (d)}}$ $\left|\nabla_{\psi}\beta(y, \varphi, \psi)\right|\leq c_3$.

${\rm{(e)}}$ For all $(\varphi_1, \psi_1, \Phi_1)$, $(\varphi_2, \psi_3, \Phi_2) \in \mathbb{R}\times \mathbb{R}^n\times \mathbb{R}$, we have

where $c_0, c_1, \cdots, c_5$ are positive constants and

(H4) $\gamma > 0$.

(H5) $f\in L^2(D_T)$.

We shall refer to both the original function and its extension to the entire of $D$ as $v^\epsilon$ for the simplicity's sake. Following ^[2,10], we introduce the following spaces

where, see ^[10]

and $\left[W^{\epsilon}_{0}\right]^{'}$ is the dual space of $W^{\epsilon}_{0}$. The existence and uniqueness results for system (1.1) for fixed $\epsilon > 0$, are obtained by combining ideas from ^[1,8]. With this, we can write system (1.1) in the following weak formulation:

for all $\varphi \in \mathcal{D}(D)\times(0, T)$. For a better formulation of our system we introduce the following set of test function

Now, testing our problem by a function from the set $\mathcal{U}_\epsilon$, we have

2.   A priori estimates

Since $v^\epsilon$ is somehow taken to be constant in each $F^{\epsilon}_{\eta}$, we may take $\varphi_{\epsilon} = 2\frac{\partial v^\epsilon}{\partial t}$ in (1.9). We have

Simple calculations on the first and second terms of (2.1) give

From (H3(b)), we see that

Using (H2), (H4), (2.3) and Young's inequality, we get

This inequality and Grownall's inequality gives

From (H3(a)), one easily see that

Thanks to this and (2.5), we claim that $\beta\left(\frac{x}{\epsilon}, v^\epsilon, \nabla v^\epsilon\right)$ belongs to $L^2(D^{\epsilon}_T)$, by which and the leading equation in (1.1) we have that

3.   Time-space periodic unfolding operator

In this section, we give some definitions and properties of the time-space periodic unfolding operator, that was essentially given in ^[2], see also ^[4,5]. For this, we set

● $\hat{D}^{\epsilon} = \text{Int}\left\{\bigcup_{\eta\in \mathbb{X}_{\epsilon}}\epsilon\left(\eta+\bar{Y}_x\right)\right\}$ and $\Lambda^{\epsilon}_{x, t} = \hat{D}^{\epsilon}\times (0, T)$.

● $\mathbb{Y}^{\epsilon}_{x} = \epsilon\left([\epsilon^{-1}x]_{Y_x}+Y_x\right)\ x = \epsilon\left([x\epsilon^{-1}]_{Y_{x}}+\{x\epsilon^{-1}\}_{Y_x}\right)$, where $[a]$ is the integer part of any real number $a$.

Definition 3.1. ^[2,4] Let $u$ be a Lebesgue measurable function on the set $D\times(0, T)$, then we define the periodic unfolding operator for this function as

If $u$ is an integrable function in $D\times (0, T)$, we define the average operator as:

Note that

where $\mathcal{M}_{V}$ stands for the usual integral average on the set $V$. We also define the oscillation operator as

The following proposition gives some of the main properties for the time-space unfolding operator:

Proposition 3.1. ^[2,4,10] The operator ${\bf T}_{\epsilon}: L^2(D\times(0, T))\rightarrow L^2(D\times (0, T); L^2(\mathcal{E}))$ satisfies the following:

(1) ${\bf T}_{\epsilon}$ is linear, continuous and

for all $\varphi_1, \varphi_2 \in L^2(D\times(0, T))$.

(2) For every $\varphi \in L^2(D\times(0, T))$, we have

and

(3) For every $\varphi \in W^{1, 2}(D\times(0, T))$, the following are true.

(a) ${\bf T}_{\epsilon}(\varphi) \rightarrow \varphi$ strongly in $L^2(D\times (0, T); L^2(Y_x))$,

(b) ${\bf T}_{\epsilon}(\nabla \varphi) \rightarrow \nabla \varphi$ strongly in $L^2(D\times (0, T); L^2(Y_x))$,

(c) ${\bf T}_{\epsilon}\left(\frac{\partial \varphi}{\partial t}\right) \rightarrow \frac{\partial \varphi}{\partial t}$ strongly in $L^2(D\times (0, T); L^2(Y_x))$.

(4) If $\phi^\epsilon \in L^2(Y_x)$ is given by $\varphi^{\epsilon}(x) = \varphi\left(x\epsilon^{-1}\right)$ for all $x\in \mathbb{R}^n$, then

(a)

(b) ${\bf T}_{\epsilon}(\varphi^\epsilon) \rightarrow \varphi$ strongly in $L^2(D\times (0, T); L^2(Y_x))$.

(c) Furthermore if $\nabla_y \varphi \in L^2\left(Y_x\right)$, then

(5) Let $u^{\epsilon}\in L^2\left(D\times(0, T)\right)$ such that $u^\epsilon \rightarrow u$ strongly in $L^2\left(D\times(0, T)\right)$, then

(6) Let $\{u^\epsilon\}$ be a bounded sequence in $L^2\left(D\times(0, T)\right)$, then

(7) For $u\in L^2\left(D\times(0, T)\right)$, we have

where $y^* = \left(y_1-\frac{1}{2}, y_2-\frac{1}{2}, \cdots, y_n-\frac{1}{2}\right)$.

(8) Assume that $\{u^\epsilon\}$ be abounded sequence in $L^2(0, T; W^{1, 2}_{0}(D))$, and that

Then there exists $\hat{u} = \hat{u}(t, x, y)$ in $L^2(D_T; W^{1, 2}_{\#}(Y_x))$, where $\mathcal{M}_{Y_x}(\hat{u}) = 0$ such that up to sub-sequence

4.   Homogenization

In this section, we use the unfolding operator to pass to the limit in the weak formulation (1.9). Before that, let us introduce the following spaces, see ^[2,11]

Theorem 4.1. Suppose that (H1)–(H5) hold true and $v^\epsilon$ be the solution for system (1.1). There exists a pair $(v, \hat{v}) \in \mathcal{W}(D_T; Y_x)$ such that up to sub-sequence

Proof. Convergences (4.1) and (4.2), easily obtained using Definition 3.1, estimates (2.5) and [13, Theorem 2.19, P. 536]. Regarding convergence (4.3), we not that $v^\epsilon \in L^{2(r+1)}(0, T; W^{1, 2}_{0}(D))$, where $1 < 2(r+1) < \frac{2n}{n-2} = 2^*$. Then, Sobolev embedding theorem [11, Theorem 3.27, P.49], shows that $W^{1, 2}_{0}(D)$ is compactly embedded in $L^{2(r+1)}(D)$, which implies the strong convergence of $v^\epsilon$ to $v$ in $L^{2(r+1)}(D\times(0, T)$, this gives (4.3). Similarly, we note that $v^\epsilon \in \mathcal{H}^{\epsilon}$ and by [11, Theorem 3.59, P. 61], $\mathcal{H}^{\epsilon}$ is compactly embedded in $L^2(D_T)$, which leads to (4.4). Convergences (4.5)–(4.8) obtained as in [2, Lemma 4.1, P. 1482].

Let us state the main results of this subsection.

Theorem 4.2. Assume that (H1)–(H5) hold and

Then, the pair $(v, \hat{v})\in \mathcal{W}(D_T; Y_x)$ uniquely satisfies the following system

where $(\phi, \hat{\phi})\in \mathcal{W}(D_T; Y_x)$.

We first acquire some preliminaries before establishing this result.

Lemma 4.1. Let $\bar{\Phi} = \left(\Phi_1, \Phi_2, \cdots, \Phi_n\right)$, where $\Phi_i\in C^{\infty}_{0}\left(D_T\right)\otimes C_{\mathit{\text{per}}}(Y_x)$ and $v^{\epsilon}$ is the solution of system (1.1). Then

where $\beta^{\epsilon}\left(t, x, v^{\epsilon}, \Phi^{\epsilon}\right) = \beta\left(t, \epsilon^{-1}x, v^{\epsilon}(t, x), \Phi\left(t, x, \epsilon^{-1}x\right)\right)$.

Proof. It is easy to see from estimate (2.6) that $\beta(t, y, v, \Phi)\in L^{2}(D_T; C_{per}(Y_x)$, we also have

From (4.12) and (1.3), we obtain the following

where we have used the generalized Holder inequality in the first term on the right hand side for $\frac{r}{p}+\frac{1}{p}+\frac{1}{2} = 1$ such that $p = 2(r+1)$. But,

Since $\phi$ is an arbitrary, we have

Thus,

Remark 4.1. As in ^[25], if we let $\Psi^{\epsilon} = \varphi_0(t, x)+\varphi_1\left(t, x, \frac{x}{\epsilon}\right)$, where $\varphi_0 \in \mathcal{D}(D_T)$ and $\varphi_1 \in \mathcal{D}(D_T)\otimes\mathcal{D}_{\text{per}}(Y_x)$, we see that

Now, we state and proof the following lemma.

Lemma 4.2. The nonlinear term $\beta^{\epsilon}\left(t, x, v^\epsilon, \nabla v^{\epsilon}\right)$ satisfies the following convergence

where

Proof. First note that the sequence ${\bf T}_{\epsilon}\left(\beta^{\epsilon}\right)$ is bounded in $L^2(D_T\times Y_x)$ thus, there exists a functions $\beta^{*} \in L^2(D_T\times Y_x)$ such that up to sub-sequence

We use (1.3) to obtain

Alternatively, to be more specific

Before passing to the limit in (4.21). We first note that by (4.3) one easily obtain

Using the same steps as in [23, Theorem 2.2], we can demonstrate that

Now, we use (4.18), (4.20), (4.22) and (4.23) to pass to the limit in (4.21), we get

Take $\varphi_0 = v-\lambda u$ and $\varphi_1 = \hat{v}-\lambda \hat{u}$ where $(u, \hat{u})\in \mathcal{W}(D_T; Y_x)$, we are led to

Divide both sides of the above inequality by $\lambda$ and then let $\lambda \rightarrow 0$. We get

Because $u$ was arbitrarily chosen, this completes the proof.

Proof of Theorem 4.2. Following ^[2], we use as test function in (1.7) $\Phi^{\epsilon}(t, x) = \epsilon\Phi\left(t, x, \frac{x}{\epsilon}\right)$ such that

where $\psi \in C^{\infty}([0, T]; \mathcal{D}(D))$, $b\in \mathcal{D}(Y_x)\cap W^{\Gamma}_{\#}(Y_x), c\in C^{\infty}_{\#}(Y_x)$ and $c|_{F_{v}} \equiv 0$. We have

Unfolding (4.29) and using (4.1) to pass to the limit we obtain

All of the remaining terms on (4.29) converge to zero. Therefore we have

See ^[2], this can always be written as

Where $\Psi \in \mathcal{W}^{\Gamma}(D_T; Y_x)$. Now, we replace our test function by $\Phi^{\epsilon}(t, x) = \Phi\left(t, x, \frac{x}{\epsilon}\right)$ such that

where $\psi$ and $b$ as in (4.28) and $b|_{F_{v}} \equiv 1$. From this it is clear that

Then we have

Let us unfold (4.35) and pass to the limit as $\epsilon$ goes to zero.

where, we have used (4.1) and (4.34). For the second term on the R.H.S, we have

where we have used (3.5), (4.5) and (4.9). As for the nonlinear term, we have

where we have used (4.19) and (4.34). The limit for the boundary term is given by

We also have

Combining all the above convergences namely, (4.32), (4.36)–(4.40), we obtain the following system

This corresponds to (4.10), when putting

Part (e) of assumption (H3) and estimate (2.2), gives the uniqueness of $(v, \hat{v}) \in \mathcal{W}(D_T; Y_x)$. This gives the convergence of the whole sequence instead of sub-sequence. Following ^[2,6,10], we state that problem (4.32) has the following unique solution

such that $\chi(x, y)$, is the corrector of the first-order that uniquely solve the following:

By substituting (4.42) into (4.41), we arrive at

where $A_0$ is coercive and elliptic matrix given by

with this in hand one can easily obtain the strong formulation of (4.44) as the following nonlinear damped wave equation

where

The initial conditions $v(x, 0) = \frac{\partial v}{\partial t}(x, 0) = 0$ are obtained in the standard way.

Conclusions

Nonlinear hyperbolic problem with special oscillated coefficients and non-local boundary conditions involving the solution's time derivative in perforated domain was considered in this paper. To obtain homogenization results, we used the unfolding periodic operator. The following are the paper's main challenges:

● The non-linearity presented on the function $\beta^\epsilon(v^{\epsilon}, \nabla v^{\epsilon})$, which was introduced in ^[8] for studying existence and uniqueness for nonlinear hyperbolic problem and in ^[25] when dealing with homogenization of nonlinear hyperbolic problem with Dirichlet condition in a fixed domain. In this paper we used the concept unfolding operator to pass to the limit in this function in Lemma 4.2.

● In order to address the non-local boundary condition $\gamma\frac{\partial v^\epsilon}{\partial t} = \frac{1}{\epsilon^{n}}\int_{\Gamma^{\epsilon}_{\eta}}\frac{\partial v^\epsilon}{\partial \nu_{\epsilon A^{\epsilon}}}d\sigma_x,$ which prevents the use of unfolding techniques in the conventional sense, we followed the settings in ^[10] and ^[2].

With theses, we obtained a damped nonlinear hyperbolic problem in fixed domain.

Acknowledgments

The author would like to thank Deanship of Scientific Research at Majmaah University for supporting this work under Project Number R-2023-223.

Conflict of interest

The author declares that he has no conflict of interest.