Analysis of HIV/AIDS model with Mittag-Leffler kernel

Muhammad Mannan Akram; Muhammad Farman; Ali Akgül; Muhammad Umer Saleem; Aqeel Ahmad; Mohammad Partohaghigh; Fahd Jarad; Muhammad Mannan Akram; Muhammad Farman; Ali Akgül; Muhammad Umer Saleem; Aqeel Ahmad; Mohammad Partohaghigh; Fahd Jarad

doi:10.3934/math.2022739

AIMS Mathematics

2022, Volume 7, Issue 7: 13383-13401. doi: 10.3934/math.2022739

Previous Article Next Article

Research article

Analysis of HIV/AIDS model with Mittag-Leffler kernel

1.
Department of Mathematics and statistics, University of Lahore, Lahore-54590, Pakistan
2.
Department of Mathematics, Khawaja Fareed University of Engineering and Information Technology, Rahim Yar Khan, Pakistan
3.
Art and Science Faculty, Department of Mathematics, Siirt University, 56100 Siirt Turkey
4.
Department of Mathematics, University of Education, Lahore, Pakistan
5.
Department of Mathematics, Ghazi University, D.G. Khan, Pakistan
6.
Department of Mathematics, Clarkson University, USA
7.
Department of Mathematics, Çankaya University, 06790 Etimesgut, Ankara, Turkey
8.
Department of Medical Research, China Medical University Hospital, China Medical University, Taichung, Taiwan

Received: 20 March 2022 Revised: 20 April 2022 Accepted: 04 May 2022 Published: 17 May 2022
MSC : 37C75, 93B05, 65L07

Recently different definitions of fractional derivatives are proposed for the development of real-world systems and mathematical models. In this paper, our main concern is to develop and analyze the effective numerical method for fractional order HIV/ AIDS model which is advanced approach for such biological models. With the help of an effective techniques and Sumudu transform, some new results are developed. Fractional order HIV/AIDS model is analyzed. Analysis for proposed model is new which will be helpful to understand the outbreak of HIV/AIDS in a community and will be helpful for future analysis to overcome the effect of HIV/AIDS. Novel numerical procedures are used for graphical results and their discussion.

Keywords:

Citation: Muhammad Mannan Akram, Muhammad Farman, Ali Akgül, Muhammad Umer Saleem, Aqeel Ahmad, Mohammad Partohaghigh, Fahd Jarad. Analysis of HIV/AIDS model with Mittag-Leffler kernel[J]. AIMS Mathematics, 2022, 7(7): 13383-13401. doi: 10.3934/math.2022739

Related Papers:

[1]	Muhammad Farman, Ali Akgül, Kottakkaran Sooppy Nisar, Dilshad Ahmad, Aqeel Ahmad, Sarfaraz Kamangar, C Ahamed Saleel . Epidemiological analysis of fractional order COVID-19 model with Mittag-Leffler kernel. AIMS Mathematics, 2022, 7(1): 756-783. doi: 10.3934/math.2022046
[2]	Shao-Wen Yao, Muhammad Farman, Maryam Amin, Mustafa Inc, Ali Akgül, Aqeel Ahmad . Fractional order COVID-19 model with transmission rout infected through environment. AIMS Mathematics, 2022, 7(4): 5156-5174. doi: 10.3934/math.2022288
[3]	Mdi Begum Jeelani, Abeer S. Alnahdi, Mohammed A. Almalahi, Mohammed S. Abdo, Hanan A. Wahash, M. A. Abdelkawy . Study of the Atangana-Baleanu-Caputo type fractional system with a generalized Mittag-Leffler kernel. AIMS Mathematics, 2022, 7(2): 2001-2018. doi: 10.3934/math.2022115
[4]	Rahat Zarin, Amir Khan, Pushpendra Kumar, Usa Wannasingha Humphries . Fractional-order dynamics of Chagas-HIV epidemic model with different fractional operators. AIMS Mathematics, 2022, 7(10): 18897-18924. doi: 10.3934/math.20221041
[5]	Wei Fan, Kangqun Zhang . Local well-posedness results for the nonlinear fractional diffusion equation involving a Erdélyi-Kober operator. AIMS Mathematics, 2024, 9(9): 25494-25512. doi: 10.3934/math.20241245
[6]	Mohamed Houas, Kirti Kaushik, Anoop Kumar, Aziz Khan, Thabet Abdeljawad . Existence and stability results of pantograph equation with three sequential fractional derivatives. AIMS Mathematics, 2023, 8(3): 5216-5232. doi: 10.3934/math.2023262
[7]	Kottakkaran Sooppy Nisar, Aqeel Ahmad, Mustafa Inc, Muhammad Farman, Hadi Rezazadeh, Lanre Akinyemi, Muhammad Mannan Akram . Analysis of dengue transmission using fractional order scheme. AIMS Mathematics, 2022, 7(5): 8408-8429. doi: 10.3934/math.2022469
[8]	Muhammad Sajid Iqbal, Nauman Ahmed, Ali Akgül, Ali Raza, Muhammad Shahzad, Zafar Iqbal, Muhammad Rafiq, Fahd Jarad . Analysis of the fractional diarrhea model with Mittag-Leffler kernel. AIMS Mathematics, 2022, 7(7): 13000-13018. doi: 10.3934/math.2022720
[9]	Hasib Khan, Jehad Alzabut, Anwar Shah, Sina Etemad, Shahram Rezapour, Choonkil Park . A study on the fractal-fractional tobacco smoking model. AIMS Mathematics, 2022, 7(8): 13887-13909. doi: 10.3934/math.2022767
[10]	Jianhua Tang, Chuntao Yin . Analysis of the generalized fractional differential system. AIMS Mathematics, 2022, 7(5): 8654-8684. doi: 10.3934/math.2022484

Abstract

1. Introduction

Biomathematics is basically the theoretical analysis of mathematical models and abstraction of living organism to investigate the principle that governs the structure development and behavior of system ^[1]. HIV contaminates the enthusiastic cells and tissues of the human immune system. This infection in the absence of antiretroviral treatment (ART), medication treatment that evades or moderates the infection, develop rapidly. Generally, HIV is diffused from perinatal or blood diffusion and sexual transmission. The symptoms of HIV at initial stage may incorporate joint agony, fever, muscle throbs, chills, sore throat, broadened organs, sweats (especially during the evening), a red rash, shortcoming, tiredness and inadvertent weight reduction thrush ^[2,34]. The HIV plague is perceived as the plainest debacle in current era. Regardless of advances in the biomedical front to the mind-boggling standard of the individuals who require it the treatment remains inaccessible and the plague keeps on spreading ^[5]. NSFD techniques by Mickens ^[6] are practical for numerical mix of differential conditions logically ^[7]. Effect by changing fractional order on the disease spread is also studied in some models. HIV fractional order models have continuously been under discussion of researchers due to the dynamics of HIV epidemics ^{[8,9,10,11,12,13,14]}. The fractional order model that involves integration and transects differentiation with the help of fractional calculus can also help to understand better the explanation of real-world problems than ordinary derivatives ^[15,16]. Based on the power law, fractional derivative idea was introduced by Riemann Liouville. The new fractional derivative by utilizing the exponential kernel is proposed by Atangana ^[17,18]. Non-singular kernel fractional derivative that includes the trigonometric and exponential function related problems ^[19,20,22] shows some related approaches for the models of epidemic. Recently a numerical scheme to solve the nonlinear fractional differential equation has been presented ^[28,29]. The proposed outbreak of this virus which effectively catches the time line for the COVID-19 disease conceptual model ^[23,2425] is under discussion too nowadays.

The feasible and accurate technique for obtaining numerical solutions for a class of partial integro-differential equations of fractional order in Hilbert space within appropriate kernel functions is studied in ^[30]. The solution methodology lies in generating an infinite conformable series solution with reliable wave pattern by minimizing the residual error functions and its related PDE's are analyzed in ^[31,32,33]. The multistep generalized differential transform method is applied to solve the fractional-order multiple chaotic FitzHugh-Nagumo (FHN) neurons model ^[34]. Investigation of a novel fractional-order mathematical model that explains the behavior of COVID-19 in Ethiopia has been studied in ^[35]. The transmission of influenza has been explained by analyzing a diffusive epidemic model in ^[36]. The analysis of general fractional order system is investigated under ABC fractional order derivative ^[37].

In this paper, Section 2 consists of some basic fractional order derivative which is helpful to solve the epidemiological model. Sections 3 and 4 consist of generalized form of the model with Atangana-Baleanu in Caputo sense using Sumudu transforms, uniqueness and stability analysis of the model. A new technique with exponential decay kernel and Mittag-Leffler kernel respectively has been given in Section 5. Results and conclusion are discussed in Section 6 and Section 7 respectively.

2. Basic definitions

Definition 2.1. Atangana-Baleanu in Caputo sense (ABC) is given by ^[18]:

${}_{a}^{ABC}{D}_{\tau }^{\alpha }\left(\phi \left(\tau \right)\right) = \frac{AB\left(\alpha \right)}{n-\alpha }\int_{a}^{\tau }\frac{{d}^{n}}{d{w}^{n}}f\left(w\right){E}_{\alpha }\left\{-\alpha \frac{{\left(\tau -w\right)}^{\alpha }}{n-\alpha }\right\}dw,n-1 < \alpha < n,$

(1)

where ${E}_{\alpha }$ is Mittag-Leffler function, $AB\left(\alpha \right)$ is normalization function and $AB\left(0\right) = AB\left(1\right) = 1.$ The Laplace transform is obtained by:

$\left[{}_{a}^{ABC}{D}_{\tau }^{\alpha }\phi \left(\tau \right)\right]\left(s\right) = \frac{AB\left(\alpha \right)}{1-\alpha }\frac{{s}^{\alpha }L\left[\phi \left(\tau \right)\right]\left(s\right)-{s}^{\alpha -1}\phi \left(0\right)}{{s}^{\alpha }+\frac{a}{1-a}}.$

(2)

By using Sumudu transform (ST) for (1), we obtain

$ST\left[{}_{0}^{ABC}{D}_{\tau }^{\alpha }\phi \left(\tau \right)\right]\left(s\right) = \frac{B\left(\alpha \right)}{1-\alpha }\left\{\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)\right\}\times \left[ST\left(\phi \left(t\right)\right)-\phi \left(0\right)\right].$

(3)

Definition 2.2. Atangana-Baleanu fractional integral of a function $\phi \left(t\right)$ of order $\alpha$ is given by:

${}_{a}^{ABC}{I}_{\tau }^{\alpha }\left(\phi \left(\tau \right)\right) = \frac{1-\alpha }{B-\alpha }\phi \left(\tau \right)+\frac{\alpha }{B\left(\alpha \right){\mathrm{\Gamma }}\left(\alpha \right)}\int_{a}^{\tau }\phi \left(s\right){\left(\tau -s\right)}^{\alpha -1}ds.$

(4)

3. Fractional order HIV/AIDS model

In this section, we consider the HIV/AIDS epidemic model proposed by Huo et al. ^[26] with a treatment compartment. By transforming the model given in ^[26] into Mittag-Leffler kernel with Atangana-Baleanu Caputo derivative is given in the following equations:

${}_{0}^{ABC}{D}_{t}^{\alpha }S = {\mathrm{\Lambda }}-\beta IS-{\mu }_{1}S-dS,$

${}_{0}^{ABC}{D}_{t}^{\alpha }I = \beta IS+{\alpha }_{1}T-dI-{k}_{1}I-{k}_{2}I,$

${}_{0}^{ABC}{D}_{t}^{\alpha }A = {k}_{1}I-\left({\delta }_{1}+d\right)A+{\alpha }_{2}T,$

${}_{0}^{ABC}{D}_{t}^{\alpha }T = {k}_{2}I-{\alpha }_{1}T-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T,$

${}_{0}^{ABC}{D}_{t}^{\alpha }R = {\mu }_{1}S-dR,$

(5)

with initial conditions

$I\left(0\right) = {I}_{0},S\left(0\right) = {S}_{0},A\left(0\right) = {A}_{0},R\left(0\right) = {R}_{0},T\left(0\right) = {T}_{0} .$

(6)

Here susceptible patients is S(t), I(t) is infectious HIV-positive individuals, $A\left(t\right)$ is the number of people with full-blown AIDS, T(t) is the total number of people being treated with ARV and R(t) is recovered populations. ${\mathrm{\Lambda }}$ is the rate of recruitment of susceptible individuals into the population, $\beta$ represents the interaction rate between susceptible individuals and infectious individuals, ${\mu }_{1}$ is the rate at which susceptible individuals change their sexual behaviors per unit time, d is the natural death rate, ${\alpha }_{1}$ is the rate at which treated individuals leave T(t) compartment, ${k}_{1}$ is the rate at which people leave the infectious class and become people with full-blown AIDS, ${k}_{2}$ is the rate at which people with HIV are treated, ${\delta }_{1}$ and ${\delta }_{2}$ are the disease-induced death rates for people in A(t) and T(t) compartments, respectively. ${\alpha }_{2}$ represents the rate at which treated individuals leave the treated class and enter the AIDS compartment $A\left(t\right).$ By putting left hand side equal to zero, we get disease free and endemic equilibrium point. Disease-free equilibrium point is given as:

${E}^{*} = \left({S}^{*},{I}^{*},{A}^{*},{T}^{*},{R}^{*}\right) = \left(\frac{{\mathrm{\Lambda }}}{{\mu }_{1}+d},{\mathrm{0,0}},0,\frac{{\mathrm{\Lambda }}{{\mathrm{\mu }}}_{1}}{d\left(d+{\mu }_{1}\right)}\right)$

and EEP is given as:

${S}^{0} = \frac{{\mathrm{\Lambda }}}{\beta {I}^{0}+{\mu }_{1}+d},{I}^{0} = \frac{\left({R}_{0}-1\right)\left({\mu }_{1}+d\right)}{\beta },{A}^{0} = \frac{{k}_{1}{I}^{0}+{\alpha }_{2}{T}^{0}}{d+{\delta }_{1}},$

${T}^{0} = \frac{{k}_{2}{I}^{0}}{{\alpha }_{1}+d+{\delta }_{2}+{\alpha }_{2}},{R}^{0} = \frac{{\mu }_{1}{\mathrm{\Lambda }}}{d\left(\beta {I}^{0}+{\mu }_{1}+d\right)}.$

Reproductive number of the system ^[27] is given as:

${R}_{0} = \frac{\beta \Lambda \left(d+{\delta }_{2}+{\alpha }_{1}+{\alpha }_{2}\right)}{\left({\mu }_{1}+d\right)\left(d+{k}_{1}+{k}_{2}\right)\left(d+{\delta }_{2}+{\alpha }_{1}+{\alpha }_{2}\right)-{\alpha }_{1}{k}_{2}}.$

4. Mittag-Leffler kernel with Atangana-Baleanu Caputo derivative

Applying Mittag-Leffler kernel with Atangana-Baleanu Caputo derivative on system (5), we get

$\frac{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right)}{1-\alpha }{E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)ST\left\{S\left(t\right)-S\left(0\right)\right\} = ST\left[{\mathrm{\Lambda }}-\beta IS-{\mu }_{1}S-dS\right],$

$\frac{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right)}{1-\alpha }{E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)ST\left\{I\left(t\right)-I\left(0\right)\right\} = ST\left[\beta IS+{\alpha }_{1}T-dI-{k}_{1}I-{k}_{2}I\right],$

$\frac{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right)}{1-\alpha }{E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)ST\left\{A\left(t\right)-A\left(0\right)\right\} = ST\left[{k}_{1}I-\left({\delta }_{1}+d\right)A+{\alpha }_{2}T\right],$

(7)

$\frac{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right)}{1-\alpha }{E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)ST\left\{T\left(t\right)-T\left(0\right)\right\} = ST\left[{k}_{2}I-{\alpha }_{1}T-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\right],$

$\frac{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right)}{1-\alpha }{E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)ST\left\{R\left(t\right)-R\left(0\right)\right\} = ST\left[{\mu }_{1}S-dR\right].$

Rearranging the above equations yields:

$ST\left(S\left(t\right)\right) = S\left(0\right)+\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left[{\mathrm{\Lambda }}-\beta IS-{\mu }_{1}S-dS\right],$

$ST\left(I\left(t\right)\right) = I\left(0\right)+\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left[\beta IS+{\alpha }_{1}T-dI-{k}_{1}I-{k}_{2}I\right],$

$ST\left(A\left(t\right)\right) = A\left(0\right)+\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left[{k}_{1}I-\left({\delta }_{1}+d\right)A+{\alpha }_{2}T\right], \left(07\right)$

$ST\left(T\left(t\right)\right) = T\left(0\right)+\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left[{k}_{2}I-{\alpha }_{1}T-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\right],$

$ST\left(R\left(t\right)\right) = R\left(0\right)+\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left[{\mu }_{1}S-dR\right].$

Using inverse transform on (7) gives

$S\left(t\right) = S\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mathrm{\Lambda }}-\beta IS-{\mu }_{1}S-dS\right\}\right],$

$I\left(t\right) = I\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{\beta IS+{\alpha }_{1}T-dI-{k}_{1}I-{k}_{2}I\right\}\right],$

$A\left(t\right) = A\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{k}_{1}I-\left({\delta }_{1}+d\right)A+{\alpha }_{2}T\right\}\right],$

$T\left(t\right) = T\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{k}_{2}I-{\alpha }_{1}T-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\right\}\right],$

$R\left(t\right) = R\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mu }_{1}S-dR\right\}\right].$

We next obtain the following recursive formula:

${S}_{n+1}\left(t\right) = {S}_{n}\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mathrm{\Lambda }}-\beta {I}_{n}{S}_{n}-{\mu }_{1}{S}_{n}-d{S}_{n}\right\}\right],$

${I}_{n+1}\left(t\right) = {I}_{n}\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{\beta {I}_{n}{S}_{n}+{\alpha }_{1}{T}_{n}-d{I}_{n}-{k}_{1}{I}_{n}-{k}_{2}{I}_{n}\right\}\right],$

${A}_{n+1}\left(t\right) = {A}_{n}\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{k}_{1}{I}_{n}-\left({\delta }_{1}+d\right){A}_{n}+{\alpha }_{2}{T}_{n}\right\}\right],$

${T}_{n+1}\left(t\right) = {T}_{n}\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{k}_{2}{I}_{n}-{\alpha }_{1}{T}_{n}-\left(d+{\delta }_{2}+{\alpha }_{2}\right){T}_{n}\right\}\right],\\ {R}_{n+1}\left(t\right) = {R}_{n}\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mu }_{1}{S}_{n}-d{R}_{n}\right\}\right].$

(8)

And the solution of (8) is provided by

$S\left(t\right) = \underset{n\to \infty }{{\mathrm{lim}}}{S}_{n}\left(t\right),I\left(t\right) = \underset{n\to \infty }{{\mathrm{lim}}}{I}_{n}\left(t\right),A\left(t\right) = \underset{n\to \infty }{{\mathrm{lim}}}{A}_{n}\left(t\right),$

$T\left(t\right) = \underset{n\to \infty }{{\mathrm{lim}}}{T}_{n}\left(t\right),R\left(t\right) = \underset{n\to \infty }{{\mathrm{lim}}}{R}_{n}\left(t\right).$

Theorem 4.1. Let $\left(X,\left|.\right|\right)$ be a Banach space and H a self-map of $X$ satisfying

$‖{H}_{x}-{H}_{r}‖\le \theta ‖X-{H}_{x}‖+\theta ‖x-r‖,$

for all $x,r\in X,$ where $0\le \theta < 1.$ Suppose that H is Picard H-Stable. Let us consider Eq (8) $,$ and we get

${R}_{n+1}\left(t\right) = {R}_{n}\left(0\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mu }_{1}{S}_{n}-d{R}_{n}\right\}\right],$

where $\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}$ is the fractional Lagrange multiplier.

Theorem 4.2.

$K\left[{S}_{n+1}\left(t\right)\right] = {S}_{\left(n+1\right)}\left(t\right)$

$= {S}_{n}\left(t\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mathrm{\Lambda }}-\beta {I}_{n}{S}_{n}-{\mu }_{1}{S}_{n}-d{S}_{n}\right\}\right],$

$K\left[{I}_{n+1}\left(t\right)\right] = {I}_{\left(n+1\right)}\left(t\right)$

$= {I}_{n}\left(t\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{\beta {I}_{n}{S}_{n}+{\alpha }_{1}{T}_{n}-d{I}_{n}-{k}_{1}{I}_{n}-{k}_{2}{I}_{n}\right\}\right],$

$K\left[{A}_{n+1}\left(t\right)\right] = {A}_{\left(n+1\right)}\left(t\right)$

$= {A}_{n}\left(t\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{k}_{1}{I}_{n}-\left({\delta }_{1}+d\right){A}_{n}+{\alpha }_{2}{T}_{n}\right\}\right],$

(9)

$K\left[{T}_{n+1}\left(t\right)\right] = {T}_{\left(n+1\right)}\left(t\right)$

$= {T}_{n}\left(t\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{k}_{2}{I}_{n}-{\alpha }_{1}{T}_{n}-\left(d+{\delta }_{2}+{\alpha }_{2}\right){T}_{n}\right\}\right],$

$K\left[{R}_{n+1}\left(t\right)\right] = {R}_{\left(n+1\right)}\left(t\right)$

$= {R}_{n}\left(t\right)+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mu }_{1}{S}_{n}-d{R}_{n}\right\}\right]$

where K be a self-map.

Proof. Using triangular inequality property with norm yields:

$‖K\left[{S}_{n}\left(t\right)\right]-K\left[{S}_{m}\left(t\right)\right]‖\\\le ‖{S}_{n}\left(t\right)-{S}_{m}\left(t\right)‖+\\{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\\\times ST\left\{{\mathrm{\Lambda }}+\beta ‖\left({I}_{n}{S}_{n}-{I}_{m}{S}_{m}\right)‖+{\mu }_{1}‖\left({S}_{n}-{S}_{m}\right)‖+d‖\left({S}_{n}-{S}_{m}\right)‖\right\}\right],$

$‖K\left[{I}_{n}\left(t\right)\right]-K\left[{I}_{m}\left(t\right)\right]‖\\\le ‖{I}_{n}\left(t\right)-{I}_{m}\left(t\right)‖\\+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\\\times ST\left\{\beta ‖\left({I}_{n}{S}_{n}-{I}_{m}{S}_{m}\right)‖+{\alpha }_{1}‖\left({T}_{n}-{T}_{m}\right)‖+d‖\left({I}_{n}-{I}_{m}\right)‖+{k}_{1}‖\left({I}_{n}-{I}_{m}\right)‖+\\{k}_{2}‖\left({I}_{n}-{I}_{m}\right)‖\right\}\right],$

$‖K\left[{A}_{n}\left(t\right)\right]-K\left[{A}_{m}\left(t\right)\right]‖\\\le ‖{A}_{n}\left(t\right)-{A}_{m}\left(t\right)‖+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\\\times ST\left\{{k}_{1}‖\left({I}_{n}-{I}_{m}\right)‖+\left({\delta }_{1}+d\right)‖\left({A}_{n}-{A}_{m}\right)‖+{\alpha }_{2}‖\left({T}_{n}-{T}_{m}\right)‖\right\}\right],$

$‖K\left[{T}_{n}\left(t\right)\right]-K\left[{T}_{m}\left(t\right)\right]‖\\\le ‖{T}_{n}\left(t\right)-{T}_{m}\left(t\right)‖\\+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\\\times ST\left\{{k}_{2}‖\left({I}_{n}-{I}_{m}\right)‖+{\alpha }_{1}‖\left({T}_{n}-{T}_{m}\right)‖+\left(d+{\delta }_{2}+{\alpha }_{2}\right)‖\left({T}_{n}-{T}_{m}\right)‖\right\}\right],$

$‖K\left[{R}_{n}\left(t\right)\right]-K\left[{R}_{m}\left(t\right)\right]‖\le ‖{R}_{n}\left(t\right)-{R}_{m}\left(t\right)‖$

$+{ST}^{-1}\left[\frac{1-\alpha }{B\left(\alpha \right)\alpha {\mathrm{\Gamma }}\left(\alpha +1\right){E}_{\alpha }\left(-\frac{1}{1-\alpha }{w}^{\alpha }\right)}\times ST\left\{{\mu }_{1}‖{S}_{n}-{S}_{m}‖+d‖\left({R}_{n}-{R}_{m}\right)‖\right\}\right].$

(10)

It's satisfied the condition given in Theorem 4.1, when

$\theta = \left({\mathrm{0,0}},{\mathrm{0,0}},0\right),$

$\theta = \left\{\begin{array}{c}‖{S}_{n}\left(t\right)-{S}_{m}\left(t\right)‖\times ‖-\left({S}_{n}\left(t\right)-{S}_{m}\left(t\right)\right)‖+\Lambda -\beta ‖\left({I}_{n}{S}_{n}-{I}_{m}{S}_{m}\right)‖\\ -{\mu }_{1}‖\left({S}_{n}-{S}_{m}\right)‖-d‖\left({S}_{n}-{S}_{m}\right)‖\\ \times ‖\left({I}_{n}\left(t\right)-{I}_{m}\left(t\right)\right)‖\times ‖-\left({I}_{n}\left(t\right)-{I}_{m}\left(t\right)\right)‖+\beta ‖\left({I}_{n}{S}_{n}-{I}_{m}{S}_{m}\right)‖\\ +{\alpha }_{1}‖\left({T}_{n}-{T}_{m}\right)‖-d‖\left({I}_{n}-{I}_{m}\right)‖\\ -{k}_{1}‖\left({I}_{n}-{I}_{m}\right)‖-{k}_{2}‖\left({I}_{n}-{I}_{m}\right)‖\\ \times ‖{A}_{n}\left(t\right)-{A}_{m}\left(t\right)‖\times ‖-\left({A}_{n}\left(t\right)-{A}_{m}\left(t\right)\right)‖{+k}_{1}‖\left({I}_{n}-{I}_{m}\right)‖\\ -\left({\delta }_{1}+d\right)‖\left({A}_{n}-{A}_{m}\right)‖+{\alpha }_{2}‖\left({T}_{n}-{T}_{m}\right)‖\\ \times ‖{T}_{n}\left(t\right)-{T}_{m}\left(t\right)‖\times ‖-\left({T}_{n}\left(t\right)-{T}_{m}\left(t\right)\right)‖\\ {+k}_{2}‖\left({I}_{n}-{I}_{m}\right)‖-{\alpha }_{1}‖\left({T}_{n}-{T}_{m}\right)‖\\ -\left(d+{\delta }_{2}+{\alpha }_{2}\right)‖\left({T}_{n}-{T}_{m}\right)‖\\ \times ‖{R}_{n}\left(t\right)-{R}_{m}\left(t\right)‖\times ‖{R}_{n}\left(t\right)-{R}_{m}\left(t\right)‖\\ +{\mu }_{1}‖{S}_{n}-{S}_{m}‖-d‖\left({R}_{n}-{R}_{m}\right)‖\end{array}\right. .$

Hence, it's stable.

Theorem 4.3. The special solution of Eq (5) using the iteration method is unique singular solution.

Proof. Take into consideration the following Hilbert space $H = {L}^{2}\left(\left(p,q\right)\times \left(0,T\right)\right)$ which can be defined as

$h:\left(p,q\right)\times \left(0,T\right)\to \mathbb{R},\iint ghdgdh < \infty .$

Considering the following operator, we have

$\theta \left({\mathrm{0,0}},{\mathrm{0,0}},0\right),\theta = \left\{\begin{array}{c}\Lambda -\beta IS-{\mu }_{1}S-dS,\\ \beta IS+{\alpha }_{1}T-dI-{k}_{1}I-{k}_{2}I,\\ {k}_{1}I-\left({\delta }_{1}+d\right)A+{\alpha }_{2}T,\\ {k}_{2}I-{\alpha }_{1}T-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T,\\ {\mu }_{1}S-dR.\end{array}\right.$

By using

$P\left(\left({S}_{11}-{S}_{12},{I}_{21}-{I}_{22},{A}_{31}-{A}_{32},{T}_{41}-{T}_{42},{R}_{51}-{R}_{52}\right),\left({V}_{1},{V}_{2},{V}_{3},{V}_{4},{V}_{5}\right)\right).$

Where

$\left({S}_{11}-{S}_{12},{I}_{21}-{I}_{22},{A}_{31}-{A}_{32},{T}_{41}-{T}_{42},{R}_{51}-{R}_{52}\right) ,$

we have

$\left\{{\mathrm{\Lambda }}-\beta \left({I}_{21}-{I}_{22}\right)\left({S}_{11}-{S}_{12}\right)-{\mu }_{1}\left({S}_{11}-{S}_{12}\right)-d\left({S}_{11}-{S}_{12}\right)\right\}$

$\le {\mathrm{\Lambda }}‖{V}_{1}‖+\beta ‖{I}_{21}-{I}_{22}‖‖{S}_{11}-{S}_{12}‖‖{V}_{1}‖$

$+{\mu }_{1}‖{S}_{11}-{S}_{12}‖‖{V}_{1}‖+d‖{S}_{11}-{S}_{12}‖‖{V}_{1}‖,$

$\left\{\begin{array}{c}\beta \left({I}_{21}-{I}_{22}\right)\left({S}_{11}-{S}_{12}\right)+{\alpha }_{1}\left({T}_{41}-{T}_{42}\right)\\ -d\left({I}_{21}-{I}_{22}\right)-{k}_{1}\left({I}_{21}-{I}_{22}\right)-{k}_{2}\left({I}_{21}-{I}_{22}\right)\end{array}\right\}$

$\le \beta ‖{I}_{21}-{I}_{22}‖‖{S}_{11}-{S}_{12}‖‖{V}_{2}‖+{\alpha }_{1}‖{T}_{41}-{T}_{42}‖‖{V}_{2}‖$

$+d‖{I}_{21}-{I}_{22}‖‖{V}_{2}‖+{k}_{1}‖{I}_{21}-{I}_{22}‖‖{V}_{2}‖+{k}_{2}‖{I}_{21}-{I}_{22}‖‖{V}_{2}‖,$

$\left\{{k}_{1}\left({I}_{21}-{I}_{22}\right)-\left({\delta }_{1}+d\right)\left({A}_{31}-{A}_{32}\right)+{\alpha }_{2}\left({T}_{41}-{T}_{42}\right)\right\}$

$\le {k}_{1}‖{I}_{21}-{I}_{22}‖‖{V}_{3}‖+\left({\delta }_{1}+d\right)‖{A}_{31}-{A}_{32}‖‖{V}_{3}‖+{\alpha }_{2}‖{T}_{41}-{T}_{42}‖‖{V}_{3}‖,$

$\left\{{k}_{2}\left({I}_{21}-{I}_{22}\right)-{\alpha }_{1}\left({T}_{41}-{T}_{42}\right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)\left({T}_{41}-{T}_{42}\right)\right\}$

$\le {k}_{2}‖{I}_{21}-{I}_{22}‖‖{V}_{4}‖+{\alpha }_{1}‖{T}_{41}-{T}_{42}‖‖{V}_{4}‖+\left(d+{\delta }_{2}+{\alpha }_{2}\right)‖{T}_{41}-{T}_{42}‖‖{V}_{4}‖,$

$\left\{{\mu }_{1}\left({S}_{11}-{S}_{12}\right)-d\left({R}_{51}-{R}_{52}\right)\right\}\le {\mu }_{1}‖{S}_{11}-{S}_{12}‖‖{V}_{5}‖+d‖{R}_{51}-{R}_{52}‖‖{V}_{5}‖ .$

For convergence solution, we have

$‖S-{S}_{11}‖,‖S-{S}_{12}‖\le \frac{{\chi }_{{e}_{1}}}{\varpi },$

$‖I-{I}_{21}‖,‖I-{I}_{22}‖\le \frac{{\chi }_{{e}_{2}}}{\varsigma },$

$‖A-{A}_{31}‖,‖A-{A}_{32}‖\le \frac{{\chi }_{{e}_{3}}}{\upsilon },$

$‖T-{T}_{41}‖,‖T-{T}_{42}‖\le \frac{{\chi }_{{e}_{4}}}{\kappa },$

and

$‖R-{R}_{51}‖,‖R-{R}_{52}‖\le \frac{{\chi }_{{e}_{5}}}{\varrho }.$

Where

$\varpi = 5\left({\mathrm{\Lambda }}+\beta ‖{I}_{21}-{I}_{22}‖‖{S}_{11}-{S}_{12}‖+{\mu }_{1}‖{S}_{11}-{S}_{12}‖+d‖{S}_{11}-{S}_{12}‖\right)‖{V}_{1}‖,$

$\varsigma = \\5\left(\beta ‖{I}_{21}-{I}_{22}‖‖{S}_{11}-{S}_{12}‖+{\alpha }_{1}‖{T}_{41}-{T}_{42}‖+d‖{I}_{21}-{I}_{22}‖+{k}_{1}‖{I}_{21}-{I}_{22}‖+{k}_{2}‖{I}_{21}-{I}_{22}‖\right)‖{V}_{2}‖,$

$\upsilon = 5\left({k}_{1}‖{I}_{21}-{I}_{22}‖+\left({\delta }_{1}+d\right)‖{A}_{31}-{A}_{32}‖+{\alpha }_{2}‖{T}_{41}-{T}_{42}‖\right)‖{V}_{3}‖,$

$\kappa = 5\left({k}_{2}‖{I}_{21}-{I}_{22}‖+{\alpha }_{1}‖{T}_{41}-{T}_{42}‖+\left(d+{\delta }_{2}+{\alpha }_{2}\right)‖{T}_{41}-{T}_{42}‖\right)‖{V}_{4}‖,$

$\varrho = 5\left({\mu }_{1}‖{S}_{11}-{S}_{12}‖+d‖{R}_{51}-{R}_{52}‖\right)‖{V}_{5}‖ .$

But it is obvious that

$\left({\mathrm{\Lambda }}+\beta ‖{I}_{21}-{I}_{22}‖‖{S}_{11}-{S}_{12}‖+{\mu }_{1}‖{S}_{11}-{S}_{12}‖+d‖{S}_{11}-{S}_{12}‖\right)\ne 0,$

$\left(\beta ‖{I}_{21}-{I}_{22}‖‖{S}_{11}-{S}_{12}‖+{\alpha }_{1}‖{T}_{41}-{T}_{42}‖+d‖{I}_{21}-{I}_{22}‖+{k}_{1}‖{I}_{21}-{I}_{22}‖+{k}_{2}‖{I}_{21}-{I}_{22}‖\right)\ne 0,$

$\left({k}_{1}‖{I}_{21}-{I}_{22}‖+\left({\delta }_{1}+d\right)‖{A}_{31}-{A}_{32}‖+{\alpha }_{2}‖{T}_{41}-{T}_{42}‖\right)\ne 0,$

$\left({k}_{2}‖{I}_{21}-{I}_{22}‖+{\alpha }_{1}‖{T}_{41}-{T}_{42}‖+\left(d+{\delta }_{2}+{\alpha }_{2}\right)‖{T}_{41}-{T}_{42}‖\right)\ne 0,$

$\left({\mu }_{1}‖{S}_{11}-{S}_{12}‖+d‖{R}_{51}-{R}_{52}‖\right)\ne 0.$

Where $‖{V}_{1}‖,‖{V}_{2}‖,‖{V}_{3}‖,‖{V}_{4}‖,‖{V}_{5}‖\ne 0$ .

Therefore, we have

$‖{S}_{11}-{S}_{12}‖ = 0, ‖{I}_{21}-{I}_{22}‖ = 0, ‖{A}_{31}-{A}_{32}‖ = 0,$

$‖{T}_{41}-{T}_{42}‖ = 0,‖{R}_{51}-{R}_{52}‖ = 0.$

Which yields that

${S}_{11} = {S}_{12},{I}_{21} = {I}_{22},{A}_{31} = {A}_{32},{T}_{41} = {T}_{42},{R}_{51} = {R}_{52} .$

We get required results. Hence, it's proved.

5. Numerical scheme

We consider the following non-linear fractional ordinary equation ^[28,29].

$S\left(t\right)-S\left(0\right) = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mathrm{\Lambda }}-\beta I\left(t\right)S\left(t\right)-{\mu }_{1}S\left(t\right)-dS\left(t\right)\right\}+\\\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{t}\left\{{\mathrm{\Lambda }}-\beta I\left(\tau \right)S\left(\tau \right)-{\mu }_{1}S\left(\tau \right)-dS\left(\tau \right)\right\}{\left(t-\tau \right)}^{\alpha -1}d\tau ,$

$I\left(t\right)-I\left(0\right) = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{\beta I\left(t\right)S\left(t\right)+{\alpha }_{1}T\left(t\right)-dI\left(t\right)-{k}_{1}I\left(t\right)-{k}_{2}I\left(t\right)\right\}+\\\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{t}\left\{\beta I\left(\tau \right)S\left(\tau \right)+{\alpha }_{1}T\left(\tau \right)-dI\left(\tau \right)-{k}_{1}I\left(\tau \right)\\-{k}_{2}I\left(\tau \right)\right\}{\left(t-\tau \right)}^{\alpha -1}d\tau ,$

$A\left(t\right)-A\left(0\right) = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{1}I\left(t\right)-\left({\delta }_{1}+d\right)A\left(t\right)+{\alpha }_{2}T\left(t\right)\right\}$

$+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{t}\left\{{k}_{1}I\left(\tau \right)-\left({\delta }_{1}+d\right)A\left(\tau \right)+{\alpha }_{2}T\left(\tau \right)\right\}{\left(t-\tau \right)}^{\alpha -1}d\tau ,$

(11)

$T\left(t\right)-T\left(0\right) = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{2}I\left(t\right)-{\alpha }_{1}T\left(t\right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left(t\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{t}\left\{{k}_{2}I\left(\tau \right)-{\alpha }_{1}T\left(\tau \right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left(\tau \right)\right\}{\left(t-\tau \right)}^{\alpha -1}d\tau ,$

$R\left(t\right)-R\left(0\right) = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mu }_{1}S\left(t\right)-dR\left(t\right)\right\}+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{t}\left\{{\mu }_{1}S\left(\tau \right)-dR\left(\tau \right)\right\}{\left(t-\tau \right)}^{\alpha -1}d\tau .$

At a given point ${t}_{n+1},n = {\mathrm{0,1}},{\mathrm{2,3}},\dots ,$ the above equation is reformulated as

$S\left({t}_{n+1}\right)-S\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mathrm{\Lambda }}-\beta I\left({t}_{n}\right)S\left({t}_{n}\right)-{\mu }_{1}S\left({t}_{n}\right)-dS\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{{t}_{n+1}}\left\{{\mathrm{\Lambda }}-\beta I\left(\tau \right)S\left(\tau \right)-{\mu }_{1}S\left(\tau \right)-dS\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$I\left({t}_{n+1}\right)-I\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{\beta I\left({t}_{n}\right)S\left({t}_{n}\right)+{\alpha }_{1}T\left({t}_{n}\right)-dI\left({t}_{n}\right)-{k}_{1}I\left({t}_{n}\right)-{k}_{2}I\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{{t}_{n+1}}\left\{\beta I\left(\tau \right)S\left(\tau \right)+{\alpha }_{1}T\left(\tau \right)-dI\left(\tau \right)-{k}_{1}I\left(\tau \right)\\-{k}_{2}I\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$A\left({t}_{n+1}\right)-A\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{1}I\left({t}_{n}\right)-\left({\delta }_{1}+d\right)A\left({t}_{n}\right)+{\alpha }_{2}T\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{{t}_{n+1}}\left\{{k}_{1}I\left(\tau \right)-\left({\delta }_{1}+d\right)A\left(\tau \right)+{\alpha }_{2}T\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$T\left({t}_{n+1}\right)-T\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{2}I\left({t}_{n}\right)-{\alpha }_{1}T\left({t}_{n}\right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{{t}_{n+1}}\left\{{k}_{2}I\left(\tau \right)-{\alpha }_{1}T\left(\tau \right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$R\left({t}_{n+1}\right)-R\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mu }_{1}S\left({t}_{n}\right)-dR\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}{\int }_{0}^{{t}_{n+1}}\left\{{\mu }_{1}S\left(\tau \right)-dR\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau .$

Also, we have

$S\left({t}_{n+1}\right)-S\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mathrm{\Lambda }}-\beta I\left({t}_{n}\right)S\left({t}_{n}\right)-{\mu }_{1}S\left({t}_{n}\right)-dS\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum \limits_{j = 0}^{n}{\int }_{{t}_{j}}^{{t}_{j+1}}\left\{{\mathrm{\Lambda }}-\beta I\left(\tau \right)S\left(\tau \right)-{\mu }_{1}S\left(\tau \right)-dS\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$I\left({t}_{n+1}\right)-I\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{\beta I\left({t}_{n}\right)S\left({t}_{n}\right)+{\alpha }_{1}T\left({t}_{n}\right)-dI\left({t}_{n}\right)-{k}_{1}I\left({t}_{n}\right)-{k}_{2}I\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}{\int }_{{t}_{j}}^{{t}_{j+1}}\left\{\beta I\left(\tau \right)S\left(\tau \right)+{\alpha }_{1}T\left(\tau \right)-dI\left(\tau \right)-{k}_{1}I\left(\tau \right)\\-{k}_{2}I\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$A\left({t}_{n+1}\right)-A\left(0\right)$

$= \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{1}I\left({t}_{n}\right)-\left({\delta }_{1}+d\right)A\left({t}_{n}\right)+{\alpha }_{2}T\left({t}_{n}\right)\right\}$

$+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum _{j = 0}^{n}{\int }_{{t}_{j}}^{{t}_{j+1}}\left\{{k}_{1}I\left(\tau \right)-\left({\delta }_{1}+d\right)A\left(\tau \right)+{\alpha }_{2}T\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

(12)

$T\left({t}_{n+1}\right)-T\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{2}I\left({t}_{n}\right)-{\alpha }_{1}T\left({t}_{n}\right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}{\int }_{{t}_{j}}^{{t}_{j+1}}\left\{{k}_{2}I\left(\tau \right)-{\alpha }_{1}T\left(\tau \right)\\-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau ,$

$R\left({t}_{n+1}\right)-R\left(0\right)\\ = \frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mu }_{1}S\left({t}_{n}\right)-dR\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}{\int }_{{t}_{j}}^{{t}_{j+1}}\left\{{\mu }_{1}S\left(\tau \right)-dR\left(\tau \right)\right\}{\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau .$

By using above equation, we have generalized form as:

${S}_{n+1} = {S}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mathrm{\Lambda }}-\beta I\left({t}_{n}\right)S\left({t}_{n}\right)-{\mu }_{1}S\left({t}_{n}\right)-dS\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{\left\{{\mathrm{\Lambda }}-\beta {I}_{j}{S}_{j}-{\mu }_{1}{S}_{j}-d{S}_{j}\right\}}{h}\\\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j-1}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \\-\frac{\left\{{\mathrm{\Lambda }}-\beta {I}_{j-1}{S}_{j-1}-{\mu }_{1}{S}_{j-1}-d{S}_{j-1}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \right),$

${I}_{n+1} = {I}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{\beta I\left({t}_{n}\right)S\left({t}_{n}\right)+{\alpha }_{1}T\left({t}_{n}\right)-dI\left({t}_{n}\right)-{k}_{1}I\left({t}_{n}\right)-{k}_{2}I\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{\left\{\beta {I}_{j}{S}_{j}+{\alpha }_{1}{T}_{j}-d{I}_{j}-{k}_{1}{I}_{j}-{k}_{2}{I}_{j}\right\}}{h}\\\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j-1}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \\-\frac{\left\{\beta {I}_{j-1}{S}_{j-1}+{\alpha }_{1}{T}_{j-1}-d{I}_{j-1}-{k}_{1}{I}_{j-1}-{k}_{2}{I}_{j-1}\right\}}{h}\\\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \right),$

${A}_{n+1} = {A}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{1}I\left({t}_{n}\right)-\left({\delta }_{1}+d\right)A\left({t}_{n}\right)+{\alpha }_{2}T\left({t}_{n}\right)\right\}$

$+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum _{j = 0}^{n}\left(\frac{\left\{{k}_{1}{I}_{j}-\left({\delta }_{1}+d\right){A}_{j}+{\alpha }_{2}{T}_{j}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j-1}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \\-\frac{\left\{{k}_{1}{I}_{j-1}-\left({\delta }_{1}+d\right){A}_{j-1}+{\alpha }_{2}{T}_{j-1}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \right),$

(13)

${T}_{n+1} = {T}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{2}I\left({t}_{n}\right)-{\alpha }_{1}T\left({t}_{n}\right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{\left\{{k}_{2}{I}_{j}-{\alpha }_{1}{T}_{j}-\left(d+{\delta }_{2}+{\alpha }_{2}\right){T}_{j}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}{\left(\tau -{t}_{j-1}\right)\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \\-\frac{\left\{{k}_{2}{I}_{j-1}-{\alpha }_{1}{T}_{j-1}-\left(d+{\delta }_{2}+{\alpha }_{2}\right){T}_{j-1}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}{\left(\tau -{t}_{j}\right)\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \right),$

${R}_{n+1} = {R}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mu }_{1}S\left({t}_{n}\right)-dR\left({t}_{n}\right)\right\}\\+\frac{\alpha }{{\mathrm{\Gamma }}\left(\alpha \right)\times ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{\left\{{\mu }_{1}{S}_{j}-d{R}_{j}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j-1}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \\-\frac{\left\{{\mu }_{1}{S}_{j-1}-d{R}_{j-1}\right\}}{h}\times {\int }_{{t}_{j}}^{{t}_{j+1}}\left(\tau -{t}_{j}\right){\left({t}_{n+1}-\tau \right)}^{\alpha -1}d\tau \right).$

Thus, we get

${S}_{n+1} = {S}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mathrm{\Lambda }}-\beta I\left({t}_{n}\right)S\left({t}_{n}\right)-{\mu }_{1}S\left({t}_{n}\right)-dS\left({t}_{n}\right)\right\}\\+\frac{\alpha }{ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{{h}^{\alpha }\left\{{\mathrm{\Lambda }}-\beta {I}_{j}{S}_{j}-{\mu }_{1}{S}_{j}-d{S}_{j}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\\times \left\{{\left(n+1-j\right)}^{\alpha }\left(n-j+2+\alpha \right)-{\left(n-j\right)}^{\alpha }\left(n-j+2+2\alpha \right)\right\}\\-\frac{{h}^{\alpha }\left\{{\mathrm{\Lambda }}-\beta {I}_{j-1}{S}_{j-1}-{\mu }_{1}{S}_{j-1}-d{S}_{j-1}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\times \left\{{\left(n+1-j\right)}^{\alpha +1}-{\left(n-j\right)}^{\alpha }\left(n-j+1+\alpha \right)\right\}\right),$

${I}_{n+1} = {I}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{\beta I\left({t}_{n}\right)S\left({t}_{n}\right)+{\alpha }_{1}T\left({t}_{n}\right)-dI\left({t}_{n}\right)-{k}_{1}I\left({t}_{n}\right)-{k}_{2}I\left({t}_{n}\right)\right\}\\+\frac{\alpha }{ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{{h}^{\alpha }\left\{\beta {I}_{j}{S}_{j}+{\alpha }_{1}{T}_{j}-d{I}_{j}-{k}_{1}{I}_{j}-{k}_{2}{I}_{j}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\\times \left\{{\left(n+1-j\right)}^{\alpha }\left(n-j+2+\alpha \right)-{\left(n-j\right)}^{\alpha }\left(n-j+2+2\alpha \right)\right\}\\-\frac{{h}^{\alpha }\left\{\beta {I}_{j-1}{S}_{j-1}+{\alpha }_{1}{T}_{j-1}-d{I}_{j-1}-{k}_{1}{I}_{j-1}-{k}_{2}{I}_{j-1}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\\times \left\{{\left(n+1-j\right)}^{\alpha +1}-{\left(n-j\right)}^{\alpha }\left(n-j+1+\alpha \right)\right\}\right),$

$+\frac{\alpha }{ABC\left(\alpha \right)}\sum _{j = 0}^{n}\left(\begin{array}{c}\frac{{h}^{\alpha }\left\{{k}_{1}{I}_{j}-\left({\delta }_{1}+d\right){A}_{j}+{\alpha }_{2}{T}_{j}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\times \left\{\begin{array}{c}{\left(n+1-j\right)}^{\alpha }\left(n-j+2+\alpha \right)\\ -{\left(n-j\right)}^{\alpha }\left(n-j+2+2\alpha \right)\end{array}\right\}\\ -\frac{{h}^{\alpha }\left\{{k}_{1}{I}_{j-1}-\left({\delta }_{1}+d\right){A}_{j-1}+{\alpha }_{2}{T}_{j-1}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\ \times \left\{{\left(n+1-j\right)}^{\alpha +1}-{\left(n-j\right)}^{\alpha }\left(n-j+1+\alpha \right)\right\}\end{array}\right),$

(14)

${T}_{n+1} = {T}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{k}_{2}I\left({t}_{n}\right)-{\alpha }_{1}T\left({t}_{n}\right)-\left(d+{\delta }_{2}+{\alpha }_{2}\right)T\left({t}_{n}\right)\right\}\\+\frac{\alpha }{ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{{h}^{\alpha }\left\{{k}_{2}{I}_{j}-{\alpha }_{1}{T}_{j}-\left(d+{\delta }_{2}+{\alpha }_{2}\right){T}_{j}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\\times \left\{{\left(n+1-j\right)}^{\alpha }\left(n-j+2+\alpha \right)-{\left(n-j\right)}^{\alpha }\left(n-j+2+2\alpha \right)\right\}\\-\frac{{h}^{\alpha }\left\{{k}_{2}{I}_{j-1}-{\alpha }_{1}{T}_{j-1}-\left(d+{\delta }_{2}+{\alpha }_{2}\right){T}_{j-1}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\\times \left\{{\left(n+1-j\right)}^{\alpha +1}-{\left(n-j\right)}^{\alpha }\left(n-j+1+\alpha \right)\right\}\right),$

${R}_{n+1} = {R}_{0}+\frac{\left(1-\alpha \right)}{ABC\left(\alpha \right)}\left\{{\mu }_{1}S\left({t}_{n}\right)-dR\left({t}_{n}\right)\right\}\\+\frac{\alpha }{ABC\left(\alpha \right)}\sum\limits _{j = 0}^{n}\left(\frac{{h}^{\alpha }\left\{{\mu }_{1}{S}_{j}-d{R}_{j}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\\\times \left\{{\left(n+1-j\right)}^{\alpha }\left(n-j+2+\alpha \right)-{\left(n-j\right)}^{\alpha }\left(n-j+2+2\alpha \right)\right\}\\-\frac{{h}^{\alpha }\left\{{\mu }_{1}{S}_{j-1}-d{R}_{j-1}\right\}}{{\mathrm{\Gamma }}\left(\alpha +2\right)}\times \left\{{\left(n+1-j\right)}^{\alpha +1}-{\left(n-j\right)}^{\alpha }\left(n-j+1+\alpha \right)\right\}\right).$

6. Results and discussion

The mathematical analysis of epidemic HIV/AIDS model with non-linear occurrence is studied to notice the sound effects of the fractional parameters. Following initial conditions and parameter values ^[26] are used for simulations:

${\mathrm{\Lambda }} = 0.55,\beta = 0.03,d = 0.0196,{k}_{1} = 0.15,{k}_{2} = 0.35,{\alpha }_{1} = 0.08,$

${\alpha }_{2} = 0.03,{\delta }_{1} = 0.0909,{\delta }_{2} = 0.0667,{\mu }_{1} = 0.03,S\left(0\right) = 35,$

$I\left(0\right) = 24,A\left(0\right) = 15,T\left(0\right) = 8,R\left(0\right) = 0.$

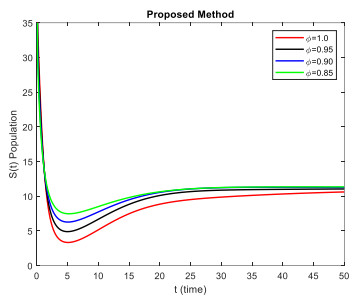
Numerical solutions are obtained for different values by using ABC derivative according to steady state. The graphs of the approximate solutions against different fractional order $\phi$ are provided in Figures 1–5. In Figures 1–5, we observe that behavior of S(t), A(t) and R(t) start increasing by decreasing the fractional values while behavior of infected I(t) and T(t) start decreasing by decreasing fractional values which approaches to our steady state. It is easily observed that susceptible individual rise after certain time while both HIV infected and AIDS infected individual start decreasing after some rise due to treatment. Also in Figure 5, the recovered individual starts increasing rapidly due to treatment for different fractional values. Observation has been made at different fractional values according to given parameters to check the effect of fractional order model. Solutions for all compartments come to our desired accuracy and more reliable by decreasing fractional values. The simulations clearly show that we can obtain better approximation to control the disease by using fractional derivative as compared to classical derivative.

Figure 1. Numerical solution of S(t) population with fractional order.

DownLoad: Full-Size Img PowerPoint

Figure 2. Numerical solution of I(t) population with fractional order.

DownLoad: Full-Size Img PowerPoint

Figure 3. Numerical solution of A(t) population with fractional order.

DownLoad: Full-Size Img PowerPoint

Figure 4. Numerical solution of T(t) population with fractional order.

DownLoad: Full-Size Img PowerPoint

Figure 5. Numerical solution of R(t) population with fractional order.

DownLoad: Full-Size Img PowerPoint

7. Conclusions

In this article, a new scheme with Mittag-Leffler law has been studied for HIV/AIDS with an antiretroviral treatment compartment. The existence and uniqueness of the solutions of the model has been proved by using iterative method and fixed-point theory. Advanced numerical approximation is used with non-singular and non-local kernel to solve for this kind of fractional order system. The advanced developed numerical technique converges to exact solution, also provides reliable and efficient results with large step size h which is mixture of the two-step Lagrange polynomial and the fundamental theorem of fractional calculus. We obtained very effective results for the proposed model. Simulation has been made to check the actual behavior of the HIV/AIDS and effect of treatment as we see rapid increase in recovered individual due to treatment. These results will be very helpful for the future study of HIV/AIDS and for control strategies with fractional operators.

Conflicts of interest

The authors declare that they have no conflicts of interest to report regarding the present study.

References

[1]	C. S. Chou, A. Friedman, Introduction, In: Introduction to mathematical biology, Springer, 2016. https://doi.org/10.1007/978-3-319-29638-8_1
[2]	F. M. Barre-Sinoussi, J. C. Chermann, R. Rey, M. T. Nugeyre, S. Chamaret, J. Gruest, et al., Isolation of a T-lymphotropic retrovirus from a patient at risk for acquired immune deficiency syndrome (AIDS), Science, 220 (1983), 868–871. https://doi.org/10.1126/science.6189183 doi: 10.1126/science.6189183
[3]	U. L. Abbas, R. M. Anderson, J. W. Mellors, Potential impact of antiretroviral chemoprophylaxis on HIV-1 transmission in resource-limited settings, PLoS ONE, 2 (2007), e875. https://doi.org/10.1371/journal.pone.0000875 doi: 10.1371/journal.pone.0000875
[4]	Z. Sadegh, N. A. Miehran, A nonstandard finite difference scheme for solving fractional-order model of HIV-1 infection of CD₄⁺ T-cells, Iran. J. Math. Chem., 6 (2015), 169–184.
[5]	J. S. Cristiana, F. M. Delfim, Global stability for a HIV/AIDS modlers, Commun. Fac. Sci. Univ. Ank. Ser., 67 (2018), 93–101.
[6]	R. E. Mickens, Advances in the applications of nonstandard finite difference schemes, Singapore: Wiley-Interscience, 2005. https://doi.org/10.1142/5884
[7]	R. E. Mickens, Calculation of denominator functions for nonstandard finite difference schemes for differential equations satisfying a positivity condition, Numer. Meth. Part. Differ. Eq., 23 (2007), 672–691. https://doi.org/10.1002/num.20198 doi: 10.1002/num.20198
[8]	Y. Ding, H. Ye, A fractional-order differential equation model of HIV infection of CD₄⁺ T-cells, Math. Comput. Model, 50 (2009), 386–392. https://doi.org/10.1016/j.mcm.2009.04.019 doi: 10.1016/j.mcm.2009.04.019
[9]	A. Gökdoǧan, A. Yildirim, M. Merdan, Solving a fractional order model of HIV infection of CD₄⁺ T cells, Math. Comput. Model, 54 (2011), 2132–2138. https://doi.org/10.1016/j.mcm.2011.05.022 doi: 10.1016/j.mcm.2011.05.022
[10]	M. M. Khader, The modeling dynamics of HIV and CD₄⁺ T-cells during primary infection in fractional order: Numerical simulation, Mediterr. J. Math., 15 (2018), 139. https://doi.org/10.1007/s00009-018-1178-9 doi: 10.1007/s00009-018-1178-9
[11]	A. Agila, D. Baleanu, R. Eid, B. Irfanoglu, Applications of the extended fractional Euler-Lagrange equations model to freely oscillating dynamical systems, Rom. J. Phys., 61 (2016), 350–359.
[12]	P. K. Gupta, Local and global stability of fractional order HIV/AIDS dynamics model, In: D. Ghosh, D. Giri, R. Mohapatra, E. Savas, K. Sakurai, L. Singh, Mathematics and computing, International Conference on Mathematics and Computing, Communications in Computer and Information Science, 834 (2018) 141–148. https://doi.org/10.1007/978-981-13-0023-3_14
[13]	N. Özalp, E. Demirci, A fractional order SEIR model with vertical transmission, Math. Comput. Model, 54 (2011), 1–6. https://doi.org/10.1016/j.mcm.2010.12.051 doi: 10.1016/j.mcm.2010.12.051
[14]	M. Javidi, N. Nyamoradi, Numerical behavior of a fractional order HIV/AIDS epidemic model, World J. Model Simul., 9 (2013), 139–149.
[15]	M. Caputo, M. Fabrizio, A new definition of fractional derivative without singular kernel, Prog. Fract. Differ. Appl., 1 (2015), 73–85. http://dx.doi.org/10.12785/pfda/010201 doi: 10.12785/pfda/010201
[16]	J. Losada, J. J. Nieto, Properties of a new fractional derivative without singular kernel, Prog. Fract. Differ. Appl., 1 (2015), 87–92. http://dx.doi.org/10.12785/pfda/010202 doi: 10.12785/pfda/010202
[17]	A. Atangana, B. S. T. Alkahtani, Analysis of the Keller-Segel model with a fractional derivative without singular kernel, Entropy, 17 (2015), 4439–4453. https://doi.org/10.3390/e17064439 doi: 10.3390/e17064439
[18]	A. Atangana, D. Baleanu, New fractional derivatives with nonlocal and non-singular kernel: Theory and application to heat transfer model, arXiv, 2016. https://doi.org/10.48550/arXiv.1602.03408
[19]	M. Farman, M. U. Saleem, M. F Tabassum, A. Ahmad, M. O. Ahmad, A linear control of composite model for glucose insulin glucagon pump, Ain Shamas Eng. J., 10 (2019), 867–872. https://doi.org/10.1016/j.asej.2019.04.001 doi: 10.1016/j.asej.2019.04.001
[20]	M. Farman, M. U. Saleem, A. Ahmad, S. Imtiaz, M. F. Tabassm, S. Akram, et al., A control of glucose level in insulin therapies for the development of artificial pancreas by Atangana Baleanu derivative, Alex. Eng. J., 59 (2020), 2639–2648. https://doi.org/10.1016/j.aej.2020.04.027 doi: 10.1016/j.aej.2020.04.027
[21]	M. Farman, A. Akgül, D. Baleanu, S. Imtiaz, A. Ahmad, Analysis of fractional order chaotic financial model with minimum interest rate impact, Fractal Fract., 4 (2020), 43. https://doi.org/10.3390/fractalfract4030043 doi: 10.3390/fractalfract4030043
[22]	M. U. Saleem, M. Farman, A. Ahmad, H. Ehsan, M. O. Ahmad, A Caputo Fabrizio fractional order model for control of glucose in insulin therapies for diabetes, Ain Shamas Eng. J., 11 (2020), 1309–1316. https://doi.org/10.1016/j.asej.2020.03.006 doi: 10.1016/j.asej.2020.03.006
[23]	A. Hussain, S. Yaqoob, On a nonlinear fractional-order model of novel coronavirus (NCOV-2019) under AB-fractional derivative, Authorea, 2020. https://doi.org/10.22541/au.158739577.76215854 doi: 10.22541/au.158739577.76215854
[24]	M. A. Khan, A. Atangana, Modeling the dynamics of novel coronavirus (2019-nCov) with fractional derivative, Alex. Eng J., 2020. https://doi.org/10.1016/j.aej.2020.02.033 doi: 10.1016/j.aej.2020.02.033
[25]	M. Amin, M. Farman, A. Akgül, R. T. Alqahtani, Effect of vaccination to control COVID-19 with Fractal-Fractional operator, Alex. Eng. J., 61 (2022), 3551–3557. https://doi.org/10.1016/j.aej.2021.09.006 doi: 10.1016/j.aej.2021.09.006
[26]	H. F. Huo, R. Chen, X. Y. Wang, Modelling and stability of HIV/AIDS epidemic model with treatment, Appl. Math. Model, 40 (2016), 6550–6559. https://doi.org/10.1016/j.apm.2016.01.054 doi: 10.1016/j.apm.2016.01.054
[27]	E. J. Moore, S. Sirisubtawee, S. Koonprasert, A Caputo-Fabrizio fractional differential equation model for HIV/AIDS with treatment compartment, Adv. Differ. Equ., 2019 (2019), 200. https://doi.org/10.1186/s13662-019-2138-9 doi: 10.1186/s13662-019-2138-9
[28]	A. Atangana, E. Bonyah, A. A. Elsadany, A fractional order optimal 4D chaotic financial model with Mittag-Leffler law, Chin. J. Phys., 65 (2020), 38–53, https://doi.org/10.1016/j.cjph.2020.02.003 doi: 10.1016/j.cjph.2020.02.003
[29]	M. Toufik, A. Atangana, New numerical approximation of fractional derivative with non-local and non-singular kernel: Application to chaotic models, Eur. Phys. J. Plus, 132 (2017), 444. https://doi.org/10.1140/epjp/i2017-11717-0 doi: 10.1140/epjp/i2017-11717-0
[30]	M. Al-Smadi, O. A. Arqub, Computational algorithm for solving fredholm time-fractional partial integrodifferential equations of dirichlet functions type with error estimates, Appl. Math. Comput., 342 (2019), 280–294. https://doi.org/10.1016/j.amc.2018.09.020 doi: 10.1016/j.amc.2018.09.020
[31]	M. Al-Smadi, O. A. Arqub, S. Momani, Numerical computations of coupled fractional resonant Schrödinger equations arising in quantum mechanics under conformable fractional derivative sense, Phys. Scr., 95 (2020), 075218. doi: 10.1088/1402-4896/ab96e0
[32]	M. Al-Smadi, O. A. Arqub, S. Hadid, An attractive analytical technique for coupled system of fractional partial differential equations in shallow water waves with conformable derivative, Commun. Theor. Phys., 72 (2020), 085001. doi: 10.1088/1572-9494/ab8a29
[33]	M. Al-Smadi, O. A. Arqub, S. Hadid, Approximate solutions of nonlinear fractional Kundu-Eckhaus and coupled fractional massive Thirring equations emerging in quantum field theory using conformable residual power series method, Phys. Scr., 95 (2020) 105205. doi: 10.1088/1402-4896/abb420
[34]	S. Momani, A. Freihat, M. Al-Smadi, Analytical study of fractional-order multiple chaotic Fitzhugh-Nagumo neurons model using multistep generalized differential transform method, Abstr. Appl. Anal., 2014 (2014), 276279. https://doi.org/10.1155/2014/276279 doi: 10.1155/2014/276279
[35]	H. Habenom, M. Aychluh, D. L. Suthar, Q. Al-Mdallal, S. D. Purohit, Modeling and analysis on the transmission of COVID-19 Pandemic in Ethiopia, Alex. Eng. J., 61 (2022), 5323–5342. https://doi.org/10.1016/j.aej.2021.10.054 doi: 10.1016/j.aej.2021.10.054
[36]	M. Asif, Z. A. Khan, N. Haider, Q. Al-Mdallal, Numerical simulation for solution of SEIR models by meshless and finite difference methods, Chaos Soliton. Fract., 141 (2020), 110340. https://doi.org/10.1016/j.chaos.2020.110340 doi: 10.1016/j.chaos.2020.110340
[37]	K. Shah, M. Arfan, A. Ullah, Q. Al-Mdallal, K. J. Ansari, T. Abdeljawad, Computational study on the dynamics of fractional order differential equations with applications, Chaos Soliton. Fract., 157 (2022), 111955. https://doi.org/10.1016/j.chaos.2022.111955 doi: 10.1016/j.chaos.2022.111955

This article has been cited by:

1.	Muhammad Farman, Ali Hasan, Muhammad Sultan, Aqeel Ahmad, Ali Akgül, Faryal Chaudhry, Mohammed Zakarya, Wedad Albalawi, Wajaree Weera, Yellow virus epidemiological analysis in red chili plants using Mittag-Leffler kernel, 2023, 66, 11100168, 811, 10.1016/j.aej.2022.10.064
2.	Muhammad Farman, Saba Jamil, Muhammad Bilal Riaz, Muhammad Azeem, Muhammad Umer Saleem, Numerical and quantitative analysis of HIV/AIDS model with modified Atangana-Baleanu in Caputo sense derivative, 2023, 66, 11100168, 31, 10.1016/j.aej.2022.11.034
3.	Dumitru Baleanu, Manijeh Hasanabadi, Asadollah Mahmoudzadeh Vaziri, Amin Jajarmi, A new intervention strategy for an HIV/AIDS transmission by a general fractional modeling and an optimal control approach, 2023, 167, 09600779, 113078, 10.1016/j.chaos.2022.113078
4.	Assad Sajjad, Muhammad Farman, Ali Hasan, Kottakkaran Sooppy Nisar, Transmission dynamics of fractional order yellow virus in red chili plants with the Caputo–Fabrizio operator, 2023, 207, 03784754, 347, 10.1016/j.matcom.2023.01.004
5.	Yanru Wu, Monireh Nosrati Sahlan, Hojjat Afshari, Maryam Atapour, Ardashir Mohammadzadeh, On the existence, uniqueness, stability, and numerical aspects for a novel mathematical model of HIV/AIDS transmission by a fractal fractional order derivative, 2024, 2024, 1029-242X, 10.1186/s13660-024-03098-1
6.	Muhammad Umer Saleem, Muhammad Farman, Kottakkaran Sooppy Nisar, Aqeel Ahmad, Zainab Munir, Evren Hincal, Muhammad Aqeel, Investigation and application of a classical piecewise hybrid with a fractional derivative for the epidemic model: Dynamical transmission and modeling, 2024, 19, 1932-6203, e0307732, 10.1371/journal.pone.0307732
7.	Muhammad Farman, Ali Akgül, Harish Garg, Dumitru Baleanu, Evren Hincal, Sundas Shahzeen, Mathematical analysis and dynamical transmission of monkeypox virus model with fractional operator, 2023, 0266-4720, 10.1111/exsy.13475
8.	Kottakkaran Sooppy Nisar, Muhammad Farman, Mahmoud Abdel-Aty, Chokalingam Ravichandran, A review of fractional order epidemic models for life sciences problems: Past, present and future, 2024, 95, 11100168, 283, 10.1016/j.aej.2024.03.059

Reader Comments

Your name:*

Email:*
© 2022 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(2176) PDF downloads(75) Cited by(8)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(5)

AIMS Mathematics

Analysis of HIV/AIDS model with Mittag-Leffler kernel

Related Papers:

Abstract

1. Introduction

2. Basic definitions

3. Fractional order HIV/AIDS model

4. Mittag-Leffler kernel with Atangana-Baleanu Caputo derivative

5. Numerical scheme

6. Results and discussion

7. Conclusions

Conflicts of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Analysis of HIV/AIDS model with Mittag-Leffler kernel

Related Papers:

Abstract

1. Introduction

2. Basic definitions

3. Fractional order HIV/AIDS model

4. Mittag-Leffler kernel with Atangana-Baleanu Caputo derivative

5. Numerical scheme

6. Results and discussion

7. Conclusions

Conflicts of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog