On a general homogeneous three-dimensional system of difference equations

1.   Introduction and preliminaries

In this paper, we study the behavior of the solutions of the following three-dimensional system of difference equations of second order

where $n\in \mathbb{N}_{0}$, the initial values $x_{-i}$, $y_{-i}$ and $z_{-i}, i = 0, 1$, are positive real numbers, the functions $f, \, g, \, h:\, \left(0, +\infty\right)^{2}\rightarrow\left(0, +\infty\right)$ are continuous and homogeneous of degree zero. We establish results on local and global stability of the unique positive equilibrium point. To do this we prove some general convergence theorems, that can be applied to generalize a lot of existing systems and to study new ones. Some results on existence of periodic and oscillatory solutions are also proved.

Now, we explain our motivation for doing this work. Clearly if we take $z_{-i} = x_{-i}, \, i = 0, 1$, and $h\equiv g$, then the system (1), will be

Noting also that if we choose $z_{-i} = y_{-i} = x_{-i}, \, i = 0, 1$, and $h\equiv g\equiv f$, then System (1) will be

In [23], the behavior of the solutions of System (2) has been investigated. System (2) is a generalization of Equation (3), studied in [17]. The present System (1) is the three-dimensional generalization of System (2).

In the literature there are many studies on difference equations defined by homogeneous functions, see for instance [1,2,5,7,11,16]. Noting that also a lot of studies are devoted to various models of difference equations and systems, not necessary defined by homogeneous functions, see for example [4,9,10,12,14,18,19,20,21,22,24,25,26,27,28,29].

Before we state our results, we recall the following definitions and results. For more details we refer to the following references [3,6,8,13].

Let $F: \left(0, +\infty\right)^{6}\rightarrow \left(0, +\infty\right)^{6}$ be a continuous function and consider the system of difference equations

where the initial value $Y_0\in \left(0, +\infty\right)^{6}$. A point $\overline{Y } \in \left(0, +\infty\right)^{6}$ is an equilibrium point of (4), if it is a solution of $\overline{Y} = F(\overline{Y})$.

Definition 1.1. Let $\overline{Y}$ be an equilibrium point of System (4), and let $\|.\|$ any convenient vector norm.

$1.$ We say that the equilibrium point $\overline{Y}$ is stable (or locally stable) if for every $\epsilon > 0$ there exists $\delta > 0$ such that for every initial condition $Y_0$: $\|Y_0-\overline{Y}\|<\delta$ implies $\|Y_n-\overline{Y}\| <\epsilon$. Otherwise, the equilibrium point $\overline{Y}$ is unstable.

$2.$ We say that the equilibrium point $\overline{Y}$ is asymptotically stable (or locally asymptotically stable) if it is stable and there exists $\gamma>0$ such that $\|Y_0-\overline{Y}\|<\gamma$ implies

$3.$ We say that the equilibrium point $\overline{Y}$ is a global attractor if for every $Y_0$,

$4.$ We say that the equilibrium point $\overline{Y}$ is globally (asymptotically) stable if it is stable and a global attractor.

Assume that $F$ is $C^{1}$ on $\left(0, +\infty\right)^{6}$. To System (4), we associate a linear system, about the equilibrium point $\overline{Y}$, given by

where $F_J$ is the Jacobian matrix of the function $F$ evaluated at the equilibrium point $\overline{Y}$.

To study the stability of the equilibrium point $\overline{Y}$, we need the following theorem.

Theorem 1.2. Let $\overline{Y}$ be an equilibrium point of System $(4)$. Then, the following statements are true:

(i) If all the eigenvalues of the Jacobian matrix $F_J$ lie in the open unit disk $|\lambda| < 1$, then the equilibrium $\overline{Y}$ is asymptotically stable.

(ii) If at least one eigenvalue of $F_J$ has absolute value greater than one, then the equilibrium $\overline{Y}$ is unstable.

Definition 1.3. A solution $\left(x_{n}, y_{n}, z_{n}\right)_{n\geq-1}$ of System (1) is said to be periodic of period $p\in \mathbb{N}$ if

The solution $\left(x_{n}, y_{n}, z_{n}\right)_{n\geq-1}$ is said to be periodic with prime period $p\in \mathbb{N}$, if it is periodic with period $p$ and $p$ is the least positive integer for which (1.3) holds.

Definition 1.4. Let $(x_n, y_n, z_n)_{n\geq-1}$ be a solution of System (1). We say that the sequence $(x_n)_{n\geq-1}$ (resp. $(y_n)_{n\geq-1}$, $(z_n)_{n\geq-1}$) oscillates about $\overline{x}$ (resp. $\overline{y}$, $\overline{z}$) with a semi-cycle of length one if: $(x_n-\overline{x})(x_{n+1}-\overline{x})<0, n\geq-1$ (resp. $(y_n-\overline{y })(y_{n+1}-\overline{y})<0, n\geq-1$, $(z_n-\overline{z})(z_{n+1}-\overline{z} )<0, n\geq-1$).

Remark 1. For every term $x_{n_0}$ of the sequence $(x_n)_{n\geq-1}$, the notation "$+$ " means $x_{n_0}-\overline{x}>0$ and the notation "$-$" means $x_{n_0}- \overline{x}<0$. The same notations will be used for the terms of the sequences $(y_n)_{n\geq-1}$ and $(z_n)_{n\geq-1}$.

Definition 1.5. A function $\Phi:\, \left(0, +\infty\right)^{2}\rightarrow\left(0, +\infty \right)$ is said to be homogeneous of degree $m\in \mathbb{R}$ if we have

for all $(u, v)\in \left(0, +\infty\right)^{2}$ and for all $\lambda>0$.

Theorem 1.6. Let $\Phi:\, \left(0, +\infty\right)^{2}\rightarrow\left(0, + \infty\right)$ be a $C^{1}$ function on $\left(0, +\infty\right)^{2}$.

1. Then, $\Phi$ is homogeneous of degree $m$ if and only if

(This statement is usually called Euler's Theorem).

2. If $\Phi$ is homogeneous of degree $m$ on $\left(0, +\infty\right)^{2}$, then $\frac{\partial \Phi}{\partial u}$ and $\frac{\partial \Phi}{\partial v}$ are homogenous of degree $m-1$ on $\left(0, +\infty\right)^{2}$.

2.   Stability of the equilibrium points

A point $(\overline{x}, \overline{y}, \overline{z})\in \left(0, +\infty\right)^{3}$ is an equilibrium point of System (1) if it is a solution of the following system

Using the fact that $f$, $g$ and $h$ are homogeneous of degree zero, we get that

is the unique equilibrium point of System (1).

Let $F: \left(0, +\infty\right)^{6}\rightarrow \left(0, +\infty\right)^{6}$ be the function defined by

with

Then, System (1) can be written as follows

So, $(\overline{x}, \overline{y}, \overline{z}) = (f(1, 1), g(1, 1), h(1, 1))$ is an equilibrium point of system (1) if and only if

is an equilibrium point of $W_{n+1} = F(W_{n})$.

Assume that the functions $f$, $g$ and $h$ are $C^{1}$ on $\left(0, +\infty\right)^{2}$. To System (1), we associate about the equilibrium point $\overline{W}$ the following linear system

where $J_{F}$ is the Jacobian matrix associated to the function $F$ evaluated at

We have

As $f$, $g$ and $h$ are homogeneous of degree $0$, then using Part 1. of Theorem 1.6, we get

which implies

Similarly we get

It follows that $J_{F}$ takes the form:

The characteristic polynomial of the matrix $J_F$ is given by

Now assume that

and consider the two functions

We have

So, by Rouché's Theorem it follows that all roots of $P(\lambda)$ lie inside the unit disk.

Hence, by Theorem 1.2, we deduce from the above consideration that the equilibrium point $(\overline{x}, \overline{y}, \overline{z} ) = (f(1, 1), g(1, 1), h(1, 1))$ is locally asymptotically stable.

Using Part 2. of Theorem 1.6 and the fact that the function $f$, $g$, $h$ are homogeneous of degree zero, we get that $\frac{\partial f}{\partial w }$, $\frac{\partial g}{\partial r}$ and $\frac{\partial h}{\partial u}$ are homogeneous of degree $-1$. So, it follows that

In summary, we have proved the following result.

Theorem 2.1. Assume that $f(u, v)$, $g(u, v)$ and $h(u, v)$ are $C^{1}$ on $\left(0, +\infty\right)^{2}$. The equilibrium point

of System $(1)$ is locally asymptotically stable if

Now, we will prove some general convergence results. The obtained results allow us to deal with the global attractivity of the equilibrium point $( \overline{x}, \overline{y}, \overline{z}) = (f(1, 1), g(1, 1), h(1, 1))$ and so the global stability.

Theorem 2.2. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v), \, g(u, v), \, h(u, v)$ are increasing in $u$ for all $v$ and decreasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$, $(m_3^{i})_{i\in\mathbb{N}_{0}}$ (resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$ and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \cdots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.3. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v), \, g(u, v)$ are increasing in $u$ for all $v$ and decreasing in $v$ for all $u$ and $h(u, v)$ is decreasing in $u$ for all $v$ and increasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$, $(m_3^{i})_{i\in\mathbb{N}_{0}}$ (resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$ and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \cdots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.4. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v)$ is increasing in $u$ for all $v$ and decreasing in $v$ for all $u$ and $g(u, v)$, $h(u, v)$ are decreasing in $u$ for all $v$ and increasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$, $(m_3^{i})_{i\in\mathbb{N}_{0}}$ (resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$ and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \cdots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.5. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v)$, $h(u, v)$ are increasing in $u$ for all $v$ and decreasing in $v$ for all $u$ and $g(u, v)$ is decreasing in $u$ for all $v$ and increasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$, $(m_3^{i})_{i\in\mathbb{N}_{0}}$ (resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$ and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \cdots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.6. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v), \, g(u, v), \, h(u, v)$ are decreasing in $u$ for all $v$ and increasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

Hence,

and

Now, we have

and it follows that

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$ $(m_3^{i})_{i\in\mathbb{N}_{0}}$(resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$, and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \cdots$, we get

For $n = 2, 3, ...,$ we have

that is

Now, for $n = 4, 5, ...,$ we have

that is

Similarly, for $n = 6, 7, ...,$ we have

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.7. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v), \, g(u, v)$ are decreasing in $u$ for all $v$ and increasing in $v$ for all $u$, however $h(u, v)$ is increasing in $u$ for all $v$ and decreasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$ $(m_3^{i})_{i\in\mathbb{N}_{0}}$(resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$, and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $, n = 1, 2, \ldots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.8. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v)$ is decreasing in $u$ for all $v$ and increasing in $v$ for all $u$, however $g(u, v), \, h(u, v)$ are increasing in $u$ for all $v$ and decreasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$, $(m_3^{i})_{i\in\mathbb{N}_{0}}$ (resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$ and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \ldots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

Theorem 2.9. Consider System $(1)$. Assume that the following statements are true:

1. $H_1$: There exist $a, \, b, \, \alpha, \, \beta, \lambda, \, \gamma \in\left(0, +\infty\right)$ such that

2. $H_2$: $f(u, v), \, h(u, v)$ are decreasing in $u$ for all $v$ and increasing in $v$ for all $u$, however $g(u, v)$ is increasing in $u$ for all $v$ and decreasing in $v$ for all $u$.

3. $H_{3}$: If $(m_{1}, M_{1}, m_{2}, M_{2}, m_{3}, M_{3})\in \left[ a, b\right] ^{2}\times \left[ \alpha , \beta \right] ^{2}\times \left[ \lambda , \gamma \right] ^{2}$ is a solution of the system

then

Then every solution of System $(1)$ converges to the unique equilibrium point

Proof. Let

and for each $i = 0, 1, ...,$

We have

and so,

and

Hence,

and

Now, we have

and it follows that

and

By induction, we get for $i = 0, 1, ...,$ that

and

It follows that the sequences $(m_1^{i})_{i\in\mathbb{N}_{0}}$, $(m_2^{i})_{i\in\mathbb{N}_{0}}$, $(m_3^{i})_{i\in\mathbb{N}_{0}}$ (resp. $(M_1^{i})_{i\in\mathbb{N}_{0}}$, $(M_2^{i})_{i\in\mathbb{N}_{0}}$, $(M_3^{i})_{i\in\mathbb{N}_{0}}$) are increasing (resp. decreasing) and also bounded, so convergent. Let

Then

By taking limits in the following equalities

and using the continuity of $f$, $g$ and $h$ we obtain

so it follows from $H_3$ that

From $H_1$, for $n = 1, 2, \ldots$, we get

For $n = 2, 3, ...,$ we have

and

that is

Now, for $n = 4, 5, ...,$ we have

and

that is

Similarly, for $n = 6, 7, ...,$ we have

and

that is

It follows by induction that for $i = 0, 1, ...$ we get

Using the fact that $i\rightarrow+\infty$ implies $n\rightarrow+\infty$ and $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$, we obtain that

From (1) and using the fact that $f$, $g$ and $h$ are continuous and homogeneous of degree zero, we get

The following theorem is devoted to global stability of the equilibrium point $(\overline{x}, \overline{y}, \overline{z}) = (f(1, 1), g(1, 1), h(1, 1))$.

Theorem 2.10. Under the hypotheses of Theorem 2.1 and one of Theorems 2.2– 2.9, the equilibrium point $(\overline{x}, \overline{y}, \overline{z} ) = (f(1, 1), g(1, 1), h(1, 1))$ is globally stable.

Now as an application of the previous results, we give an example.

Example 1. Consider the following system of difference equations

where $x_{-1}$, $x_{0}$, $y_{-1}$, $y_{0}$, $z_{-1}$, $z_{0}$ $\in\left(0, +\infty\right)$ and

Assume that $r_i: = a_i\beta_i-\alpha_ib_i, \, i = 1, 2, 3$ are positive. For all $(u, v)\in\left(0, +\infty\right)$, we have

It follows from Theorem 2.1 that the unique equilibrium point

of System (6) will be locally stable if

which is equivalent to

Also, we have conditions $H_1$ and $H_2$ of Theorem 2.2 are satisfied. So, to prove the global stability of the equilibrium point $(f(1, 1), g(1, 1), h(1, 1))$ it suffices to check condition $H_3$ of Theorem 2.2.

For this purpose, let $(m_1, M_1, m_2, M_2, m_3, M_3)\in[a, b]\times[\alpha, \beta] \times[\gamma, \lambda]$ such that

From (7)-(9), we get

Now, from (10)-(12), we get

where $\Theta(m_1, M_1, m_2, M_2, m_3, M_3)$ is equal to

It is not hard to see that

Using the fact

we get

If we choose the parameters $a_i, \, b_i, \, \alpha_i, \, \beta_i, \, i = 1, 2, 3$ such that

then we get that

and so it follows from (13) that

Using this and (10)-(12), we obtain $m_1 = M_1, \, m_2 = M_2, \, m_3 = M_3$. That is the condition $H_3$ is satisfied.

In summary we have the following result.

Theorem 2.11. Assume that that parameters $a_i, \, b_i, \, \alpha_i, \, \beta_i, \, i = 1, 2, 3$ are such that

● $C_1$: $a_i\beta_i>\alpha_ib_i, \, i = 1, 2, 3$.

● $C_2$:

● $C_3$:

Then the equilibrium point $\left(f(1, 1), g(1, 1), h(1, 1)\right) = \left(\frac{ a_1+b_1}{\alpha_1+\beta_1}, \frac{a_2+b_2}{\alpha_2+\beta_2}, \frac{a_3+b_3}{ \alpha_3+\beta_3}\right)$ is globally stable.

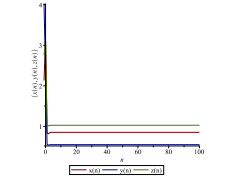
We visualize the solutions of System (6) in Figures 1-3. In Figure 1 and Figure 2, we give the solution and corresponding global attractor of System (6) for $x_{-1} = 2.13, x_{0} = 3.1, y_{-1} = 4.03, y_{0} = 2.21, z_{-1} = 2.76, z_{0} = 3.12$ and $a_1 = 3, \alpha_1 = 3.2, b_{1} = 4, \beta_{1} = 5, a_2 = 1.2, \alpha_2 = 2, b_{2} = 3.2, \beta_{2} = 6, a_3 = 2.4, \alpha_3 = 2.3, b_{3} = 1.3, \beta_{3} = 1.3$, respectively. Note that the conditions $C_1, C_2, C_3$ is satisfied for these values.

However Figure 3 shows the unstable solution corresponding to the values $x_{-1} = 2.13, x_{0} = 3.1, y_{-1} = 4.03, y_{0} = 2.21, z_{-1} = 2.76, z_{0} = 3.12$ and $a_1 = 0.3, \alpha_1 = 4, b_{1} = 3, \beta_{1} = 1.1, a_2 = 1.2, \alpha_2 = 4, b_{2} = 3.7, \beta_{2} = 1, a_3 = 2.7, \alpha_3 = 0.2, b_{3} = 1.3, \beta_{3} = 3$, which do not satisfy the conditions $C_1, C_2, C_3$.

3.   Existence of periodic solutions

Here, we are interested in existence of periodic solutions for System (1). In the following result we will established a necessary and sufficient condition for which there exist prime period two solutions of System (1).

Theorem 3.1. Assume that $\alpha$, $\beta$ and $\gamma$ are positive real numbers such that $(\alpha-1)(\beta-1)(\gamma-1)\neq0$. Then, System $(1)$ has a prime period two solution in the form of

if and only if

Proof. Let $\alpha$, $\beta$, $\gamma$ be positive real numbers such that $(\alpha-1)(\beta-1)(\gamma-1)\neq0$ and assume that

is a solution for System (1). Then, we have

From (14)-(19), it follows that

Now, assume that

and let

We have

By induction we get

In the following, we apply our result in finding prime period two solutions of two special Systems.

Consider the three dimensional system of difference equations

where $n\in \mathbb{N}_{0}$, the initial values $x_{-i}, \, y_{-i}, \, z_{-i}, \, i = 0, 1$ and the $a_{i}, b_i, \, c_i, \, i = 1, 2, 3$ are positive real numbers.

System (20) can be seen as a generalization of the system

studied in [23]. This last one is also a generalization of the equation

studied in [7] and [15].

Corollary 1. Assume that $(\alpha-1)(\beta-1)(\gamma-1)\neq0$, then System $(20)$ has prime period two solution of the form

if and only if

Proof. System (20) can be written as

where

So, from Theorem 3.1,

will be a period prime two solution of System (20) if and only if

Clearly this condition is equivalent to (21).

Example 2. If we choose $\alpha = 2, \, \beta = 3, \, \gamma = \frac{1}{2}$ then, condition (21) will be

The last condition is satisfied for the choice

of the parameters. The corresponding prime period two solution will be

and

that is

Now, consider the following system of difference equations

where the initial values $x_{-i}, \, y_{-i}, \, z_{-i}, \, i = 0, 1$ and $a_{i}, b_i, \, c_i, \, i = 1, 2, 3$ are positive real numbers.

Corollary 2. Assume that $(\alpha-1)(\beta-1)(\gamma-1)\neq0$, then System $(22)$ has prime period two solution of the form

if and only if

Proof. System (22) can be written as

where

So, from Theorem 3.1,

will be a period prime two solution of System (20) if and only if

Clearly this condition is equivalent to

Example 3. For $\alpha = 3, \, \beta = 2, \, \gamma = \frac{1}{3}$ then, condition (23) will be

The last condition is satisfied for the choice

of the parameters. The corresponding prime period two solution will be

and

that is

4.   Existence of oscillatory solutions

Here, we are interested in the oscillation of the solutions of System (1) about the equilibrium point $(\overline{x}, \overline{y}, \overline{z }) = (f(1, 1), g(1, 1),$ $h(1, 1))$.

Theorem 4.1. Let $\left(x_n, y_n, z_n\right)_{n\geq-1}$ be a solution of System $(1)$ and assume that $f(x, y)$, $g(x, y)$ $h(x, y)$ are decreasing in $x$ for all $y$ and are increasing in $y$ for all $x$.

1. If

then we get

That is for the sequences $(x_n)_{n\geq-1}$, $(y_n)_{n\geq-1}$ and $(z_n)_{n\geq-1}$ we have semi-cycles of length one of the form

2. If

then we get

That is for the sequences $(x_n)_{n\geq-1}$, $(y_n)_{n\geq-1}$ and $(z_n)_{n\geq-1}$ we have semi-cycles of length one of the form

Proof. 1. Assume that

We have

By induction, we get

2. Assume that

We have

By induction, we get

So, the proof is completed. Now, we will apply the results of this section on the following particular system.

Example 4. Consider the system of difference equations

where $p, \, q, \, r\in \mathbb{N}$, the initial values $x_{-i}, \, y_{-i}, \, z_{-i}, \, i = 0, 1$ and the parameters $a_i, \, b_i, \, i = 1, 2, 3$ are positive real numbers.

Let $f$, $g$ and $h$ be the functions defined by

It is not hard to see that

System (24) has the unique equilibrium point $(\overline{x}, \overline{y }, \overline{z}) = (a_1+b_1, a_2+b_2, a_3+b_3)$.

Corollary 3. Let $\left(x_n, y_n, z_n\right)_{n\geq-1}$ be a solution of System $(24)$. The following statements holds true:

1. Let

Then the sequences $(x_n)_n$ (resp. $(y_n)_n$, $(z_n)_n$) oscillates about $\overline{x}$ (resp. about $\overline{y}$, $\overline{z}$) with semi-cycle of length one and every semi-cycle is in the form

2. Let

Then the sequences $(x_n)_n$ (resp. $(y_n)_n$, $(z_n)_n$) oscillates about $\overline{x}$ (resp. about $\overline{y}$, $\overline{z}$) with semi-cycle of length one and every semi-cycle is in the form

Proof. 1. Let

We have

which implies that

Using the fact that

we get

Also as,

we get

Now, as

we obtain

Similarly,

and

and by induction we get that

for $n\in \mathbb{N}_{0}$. That is, the sequences $(x_n)_n$ (resp. $(y_n)_n$, $(z_n)_n$) oscillates about $\overline{x}$ (resp. about $\overline{y}$, $\overline{z}$) with semi-cycle of length one and every semi-cycle is in the form

and this is the statement of Part 1. of Theorem 4.1.

$2.$Let

We have

which implies that

Using the fact that

we get

Also as,

we get

Now, as

we obtain

Similarly,

and

Thus, by induction we get that

for $n\in \mathbb{N}_{0}$. That is the sequences $(x_n)_n$ (resp. $(y_n)_n$, $(z_n)_n$) oscillates about $\overline{x}$ (resp. about $\overline{y}$, $\overline{z}$) with semi-cycle of length one and every semi-cycle is in the form

and this is the statement of Part 2. of Theorem 4.1.

5.   Conclusion

In this study, the global stability of the unique positive equilibrium point of a three-dimensional general system of difference equations defined by positive and homogeneous functions of degree zero was studied. For this, general convergence theorems were given considering all possible monotonicity cases in arguments of functions $f$, $g$ and $h$. In addition, the periodic nature and oscillation of the general system considered was also discussed and successful results were obtained. It is noteworthy that the results obtained on our general three-dimensional system have high applicability.

Acknowledgments

The authors thanks the two referees for their comments and suggestions. The work of N. Touafek and Y. Akrour was supported by DGRSDT (MESRS-DZ).