Abbreviations: OC: Ovarian cancer; ncRNA: Non-coding RNA; lncRNAs: Long non-coding RNAs; GC: Gastric cancer; GO: Gene ontology; KEGG: Kyoto Encyclopedia of Genes and Genomes
1.
Introduction
Ovarian cancer (OC) is one of the most common gynecological malignant tumors and has been one of the leading causes of cancer-related death in western countries [1,2,3,4,5]. Of note, the incidence of OC is also increasing in China. Despite surgery and chemotherapy have been widely used in the treatment of OC, the prognosis of OC remained to be unsatisfied. Therefore, further understanding of the molecular mechanisms involved in the development of OC is still an urgent need for the diagnosis and treatment of this disease.
Non-coding RNA (ncRNA) is a class of RNA modules which have no protein-coding function. Recently, ncRNAs had been revealed to play a crucial role in human diseases. Emerging evidence has shown that long non-coding RNAs (lncRNAs) played important roles in both physiological and pathological processes of cancers [6,7,8]. LncRNA could affect a variety of biological processes, such as autophagy, proliferation, apoptosis, differentiation, and cell cycle, in cells. For example, lncRNA Fendrr played an important role in the development of heart and body wall in the mouse, and the lack of Fendrr lead to abnormal mouse embryonic development [9]. Thomas et al. found hundreds of TNFα-induced inflammation-related lncRNAs in mice, in which Lethe was involved in the negative feedback of inflammatory signals [10]. H19 is overexpressed in gastric cancer (GC) tissues and promotes GC proliferation, migration, invasion, and metastasis [11]. Recent studies also demonstrated that lncRNA HOST2 [12], LSINCT5 [13], HOTAIR [14], NEAT1 [15] were dysregulated in OC and associated with the occurrence and progression of OC.
LncRNA XIST is the first lncRNA reported to be involved in the regulation of X chromosome inactivation. Recent studies have shown that XIST is dysregulated in multiple cancer types, including gastric, liver, nasopharyngeal carcinoma, cervical, non-small cell lung, glioma, pancreatic, osteosarcoma, colorectal, and breast cancer. In cervical cancer, XIST competitively binds to miR-200a to promote tumor progression [16]. In non-small cell lung cancer, XIST inhibits tumor cell proliferation and EMT progression by modulating miR-137 to regulateNotch-1 signaling pathway [17]. However, the functional roles and expression pattern of XIST in OC remain unclear. The present study aimed to explore whether XIST could serve as a new diagnostic target in OC.
2.
Materials and methods
2.1. Database analysis
The present study used TCGA (The cancer genome atlas) to analysis the XIST expression levels. The TCGA database, including a total of 305 OC samples (23 Stage Ⅱ OC samples, 244 Stage Ⅲ OC samples, and 38 Stage Ⅳ OC samples). The Kaplan-Meier plotter database was used to analyze the correlation between XIST and survival time in patients.
2.2. Bioinformatics analysis
The TCGA database was used to analyze XIST co-expressed genes. Gene Ontology (GO) analysis and Kyoto Encyclopedia of Genes and Genomes (KEGG) Pathway analysis were performed on the top 1000 co-expressed genes by using the DAVID database [18,19] (https://David.ncifcrf.gov/). The interaction network between proteins was performed using the STRING (https://string-db.org/) database. The Starbase database was used to predict the regulatory cascade of XIST-miRNAs. Pearson’s correlation coefficient ≥ 0.4 is selected as a candidate, and the network was presented by Cytoscape.
2.3. Statistical analysis
The statistical analysis was performed using SPSS version 17.0 software. T-test and Mann-Whitney U-test statistics were used to perform the statistical significance of differences between two groups; One-way ANOVA was used to perform the statistical significance of differences between multiple groups. The survival curve was performed by the Kaplan-Meier method. P < 0.05 was selected as significant.
3.
Results
3.1. LncRNA XIST was highly expressed in ovarian tissues
To explore the expression levels of XIST in OC, we first used the GTEx database to determine the expression of XIST in multiple human tissues, including ovary, cervix, uterus, testes, brains, and kidney. The results showed that the expression level of XIST in the human ovary, cervix, uterus was significantly higher than that in other tissues (Figure 1A).
3.2. XIST was downregulated in OC compared to normal tissues.
Next, we analyzed the expression of XIST in OC tissues and normal ovarian tissues by using the GEPIA database, which contained RNA sequencing data of 88 normal ovarian tissues and 426 OC samples by integrating TCGA and GTEx databases. As shown in Figure 1B, our results showed that the XIST was significantly downregulated in OC compared to normal tissues (Figure 1B, p < 0.05).
Furthermore, we analyze the correlation between its expression and stage. Totally, there are 305 OC samples that were with well-defined pathological stages in the TCGA dataset was used to conduct the analysis. The results showed that XIST was significantly downregulated in Stage Ⅲ OC compared that in Stage Ⅱ OC (Figure 1C, p < 0.05) and significantly downregulated in Stage Ⅳ OC compared that in Stage Ⅱ OC (Figure 1C, p < 0.05). However, no significant difference of XIST levels was observed between Stage Ⅲ OC and Stage Ⅳ OC samples.
3.3. Downregulated XIST is correlated with worse prognosis in OC
To further explore the role of XIST in OC, the present study first analyzed the relationship between XIST and survival time in OC by using the TCGA database. The results showed that higher XIST expression levels were associated with longer overall survival time (Figure 1D, p < 0.05).
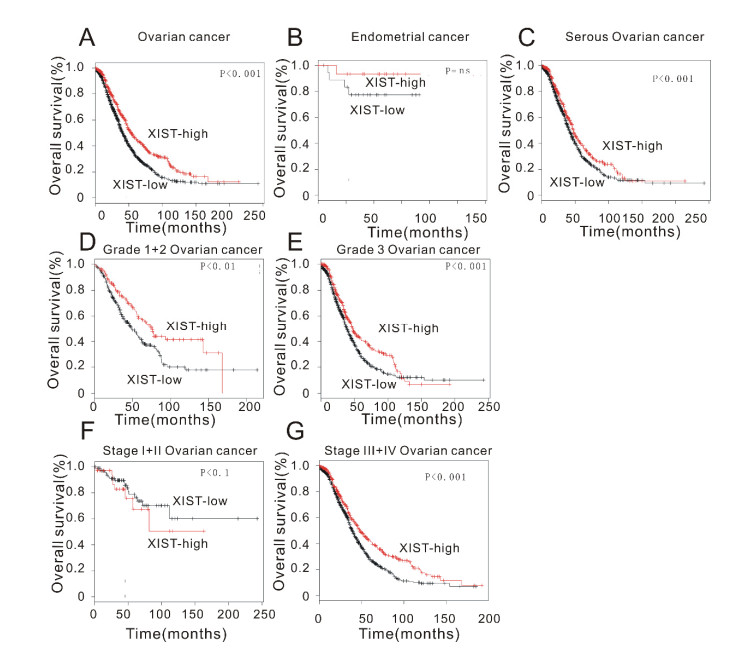
The present study also used the Kaplan-Meier plotter datasets to further explore the relationship between XIST and survival time in different types of OC. The present study found that upregulated XIST was positively correlated with longer survival time in OC (Figure 2A, p < 0.001), Serous OC (Figure 2C, p < 0.001), Grade 1 + 2 OC (Figure 2D, p < 0.01), Grade 3 OC (Figure 2E, p < 0.001), Stage Ⅲ + Ⅳ OC (Figure 2G, p < 0.001). However, the present study did not find the correlation between XIST and survival time in endometrial OC (Figure 2B) and Stage Ⅰ + Ⅱ OC (Figure 2F).
3.4. Bioinformatics analysis of XIST.
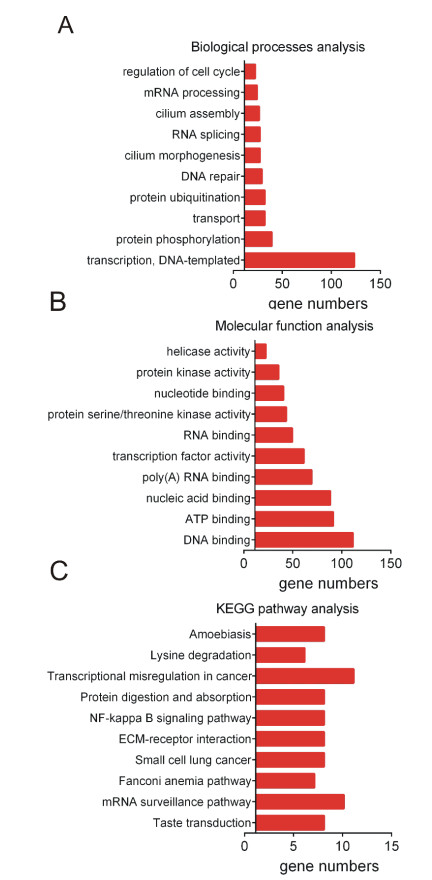
The present study first analyzed the co-expressed gene of XIST by using TCGA database, and the most significant 1000 co-expressed genes were selected. Gene Ontology analysis (GO analysis) was further used to predict the potential roles of XIST in OC. The results revealed that XIST is involved in a series of biological processes including transcription, protein phosphorylation, transport, protein ubiquitination, DNA repair, ciliary morphogenesis, RNA splicing, cilia assembly, mRNA processing, and cell cycle regulation (Figure 3A). Molecular Function analysis showed that XIST could participate in many functions, including DNA binding, ATP binding, nucleic acid binding, poly (A) RNA binding, transcription factor activity, RNA binding, protein serine/threonine kinase activity, nucleoside Acid binding, protein kinase activity (Figure 3B). KEGG analysis showed that XIST affected many signaling pathways, including taste, mRNA monitoring, Fanconi anemia, small cell lung cancer, ECM-receptor interaction, NF-κB, protein digestion and absorption, transcriptional dysregulation in cancer, lysine degradation, amebic disease (Figure 3C).
3.5. Construction of XIST mediated protein-protein interaction network
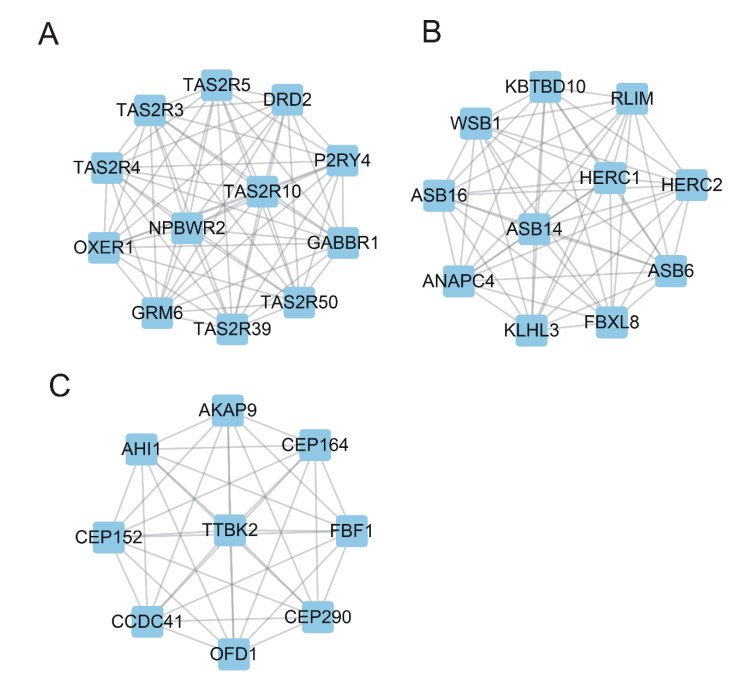
The present study constructed XIST‑mediated PPI networks by using the top 1000 co-expressing genes of XIST via the STRING database. The results identified three core networks. Among them, Module 1 included 12 core proteins (P2RY4, DRD2, TAS2R4, TAS2R5, TAS2R10, AS2R3, NPBWR2, GRM6, TAS2R39, OXER1, GABBR1, TAS2R50, Figure 4A), Module 2 included 11 core proteins (KBTBD10, KLHL3, HERC2, WSB1, ASB14, FBXL8, ANAPC4, ASB16, RLIM, ASB6, HERC1, Figure 4B) and Module 3 included 9 core proteins (CCDC41, CEP152, CEP164, FBF1, OFD1, CEP290, AHI1) TTBK2, AKAP9, Figure 4C).
3.6. Construction of XIST-mediated endogenous competitive RNA interaction network
Recent studies have found that XIST could participate in the regulation of tumor progression as a natural miRNA sponge. However, the mechanisms of XIST in OC remained unclear. Therefore, the present study systematically and comprehensively constructed an XIST-mediated endogenous competitive RNA interaction network. This study predicted the XIST-miRNAs regulatory cascade by using the Starbase database and then used the same method to predict the XIST-miRNAs-mRNAs regulatory cascade. Only XIST-mRNAs with positive correlation expression were selected for the construction of ceRNAs networks based on co-expression analysis results.
The network included 202 miRNAs and 462 mRNAs as shown in Figure 5. Ten of these miRNAs were identified as the core regulator of the network, including hsa-miR-590-3p, hsa-miR-340-5p, hsa-miR-181b-5p, hsa-miR-424-5p, hsa-miR-15b-5p, hsa-miR-495-3p, hsa-miR-15a-5p, hsa-miR-181a-5p, hsa-miR-181d-5p, hsa-miR-30c-5p, hsa-miR-497-5p, hsa-miR-16- 5p, hsa-miR-30e-5p, hsa-miR-181c-5p, involved in the regulation of up to 100 different mRNAs. The present study also found that DDX3X, CHD9, POU2F1, YOD1, MAT2A, BRWD1, INO80D, GPCPD1, and MDM4 were regulated by more than 100 different miRNAs, suggesting that they acted as key XIST regulatory genes.
4.
Discussions
Emerging studies demonstrated lncRNAs play important roles in regulating cell proliferation, apoptosis, and differentiation. For example, lncRNA loc285194 played as a tumor suppressor function by competitively binding to miR-211 in colorectal cancer [20]. UCA1 is overexpressed in liver cancer and sponged miR-216b to inhibit tumor growth [21]. MALAT1 could cause microvascular dysfunction in diabetic patients [22]. Exploring the roles of lncRNAs in human diseases could provide novel biomarkers for prognosis and treatment.
OC is one of the most common gynecological malignant tumors. Previous studies had reported that lncRNA played an important role in the development and progression of OC. A series of lncRNAs are abnormally expressed in OC, such as GAS5 [23], TP73-AS1 [24], CCAT1, and MALAT1 [25]. LncRNA could regulate a series of OC related biological processes such as proliferation, cycle, and apoptosis. For instance, Lnc-OC1 promotes proliferation and migration of OC cells by competitively binding to miR-34a and miR-34c [26]. LncRNA CTD-2020K17.1 has been reported as an oncogene to promote OC metastasis, invasion, and proliferation [27]. However, it should be noted that the functions of most lncRNAs in OC are still unclear. Several previous studies indicated XIST might be associated with the development of OC. However, there is still a lack of systematic analysis to support XIST as a target for clinical diagnosis in OC. Then in the silico analysis based on multiple platforms in this study showed that the expression level of XIST was significantly increased in normal ovary, uterus, and cervix tissues, while the expression level was significantly decreased in OC tissues.
Further analysis showed that XIST expression levels in advanced-grade OC were significantly lower than in low-grade OC. Moreover, the overexpression of XIST was positively correlated with the longer survival time of patients with OC, Serous OC, Grade 1 + 2 OC, Grade 3 OC, and Stage Ⅲ + Ⅳ OC. These analyses showed that XIST could serve as a potential diagnostic marker for OC.
Emerging studies have shown that in addition to routine functional assays in vitro and in vivo, the widespread use of bioinformatics tools such as protein-protein interaction networks and endogenous competitive RNA networks plays an important role in uncovering the underlying pathogenesis of the human disease. To explore the potential functions and mechanisms of XIST in OC, the present study first performed co-expression analysis and bioinformatic analysis and identified three core interaction networks included 12 core proteins, 11 core proteins, and 9 core proteins respectively. The results revealed that XIST was involved in the biological processes of transcription, protein phosphorylation, transport, protein ubiquitination, DNA repair, ciliary morphogenesis, RNA splicing, DNA binding, ATP binding. KEGG signaling pathway analysis showed that XIST affected many signaling pathways, including taste, mRNA monitoring, Fanconi anemia, small cell lung cancer, ECM-receptor interaction, NF-κB, protein digestion and absorption, transcriptional dysregulation in cancer, lysine degradation, and amebic disease.
Previous studies show XIST plays an important regulatory role in the development of multiple tumors, including gastric cancer, hepatocellular carcinoma, and breast cancer. XIST, as one of the longest lncRNAs in mammals, previous reports have shown that XIST plays a wide range of endogenous competitive RNA in cells, affecting multiple miRNA activity included miR-137, miR-200c, miR-155, miR-320 and miR-34a [28,29,30]. For example, XIST can inhibit the proliferation and metastasis of breast cancer cells by affecting the miR-155/CDX1 cascade control axis. The present study first systematically constructed OC-specific XIST-mediated endogenous competitive RNA network. Several studies in OC also demonstrated that XIST had a regulatory role in cancer progression. However, these reports are often contradictory. For example, Zuo et al. found XIST promoted malignant behavior of epithelial OC, while Wang et al. demonstrated that XIST has anticancer effects on epithelial OC cells through inverse downregulation of hsa-miR-214-3p. Of note, with the development of bioinformatics analysis, emerging studies revealed that bioinformatics analysis could provide more clues to understand the potential roles of genes in human diseases. The network included 202 miRNAs and 462 mRNAs, of which 10 miRNAs were identified as the core regulatory factors of this network, including hsa-miR-590-3p, hsa-miR-340-5p, hsa-miR-181b-5p, which regulated more than 100 different mRNAs in the network. These miRNAs played an important regulatory role in OC. For example, miR-590-3p could promote the proliferation and metastasis of OC by regulating FOXA2. miR-424 could inhibit the metastasis, invasion, and epithelial-mesenchymal transition of OC.
In this study, there also exist some limitations. Firstly, the expression levels of XIST in OC and normal tissues should be detected to validate the bioinformatics analysis in future study. Secondly, the specific functions of XIST had not been further excavated in this study. Further experimental validation would be required for future verification.
In summary, the present study showed lncRNA XIST was significantly down-regulated in OC and was associated with the development of OC. Overexpression of XIST was significantly associated with longer survival time in patients with OC. Also, our analysis also showed that lncRNA XIST was closely related to biological processes such as transcription, protein phosphorylation, transport, protein ubiquitination, and DNA repair, which provided a theoretical basis for the diagnosis and treatment of OC.
Conflict of interest
All authors declare no conflicts of interest in this paper










 DownLoad:
DownLoad: