This paper proposes a LoRa-based wireless communication system for data transfer in microgrids. The proposed system allows connection of multiple sensors to the LoRa transceivers, and enables data collection from various units within a microgrid. The proposed system focuses on communications at the secondary communication level of the microgrid between local controllers of each distributed generation (DG) unit and the microgrid central controller due to the possibility of applying low-bandwidth communication systems at this level. In a proof of concept test bed setup, the data collected by the sensors are sent to the LoRa gateway, which serves as the central monitoring system from which control messages are sent to various microgrid components through their local controllers such as DG units, storage systems and load. In this work, to improve communication security, a private server has been developed using Node-Red instead of cloud servers that are currently used in most Internet-of-Things (IoT) monitoring systems. A range test of the proposed system is carried out to observe the rate of data delivery. It demonstrated over 90% data delivery at 4 km. Finally, a test bed experiment is conducted to validate key features of the proposed system by achieving one-directional data transfer in a grid monitoring system.
1.
Introduction
Renewable energy-based Microgrids with distributed energy resources (DERs), such as wind and solar generation, can operate at either grid-connected or islanded mode [1,2]. In the grid-connected mode, the grid defines the microgrid’s voltage and frequency, hence the DERs are controlled to produce the desired amount of power [2]. In the islanded mode, the DERs are not only required to supply the microgrid’s load demand, but also to regulate the distribution feeder’s voltage and frequency within acceptable limits [3,4]. Isolated microgrids without grid connection in remote communities permanently operate in islanded mode.
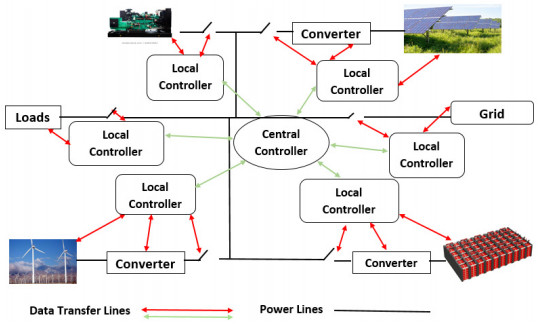
To achieve proper control and operation of a microgrid, each DG unit should be updated with information pertaining to the microgrid’s operating mode [5]. This entails real-time power measurement of the grid, loads, and DGs as well as the state of charge (SoC) of battery storage systems [6]. The RMS value, phase angle, and frequency of the voltage, active and reactive power at specific points in the microgrid can also serve as monitoring signals for the microgrid control systems [7]. Furthermore, instantaneous values of voltages at the DG terminals and the feeder are needed for synchronizing DG units within the microgrid [8]. It is expected that in the near future automated operation of microgrids can be achieved [9,10] by monitoring the system through various sensors, analyzing the collected sensor data and integrating the results into an advanced control scheme, and passing the control commands back to the nodes [11]. To achieve automation, a fast and reliable communication system for data and command transfer within the microgrid is a fundamental requirement [12]. Figure 1 shows a typical microgrid with advanced communication flow.
Communication technologies for microgrids can be categorized into wired and wireless: 1) the wired communication for data transfer within microgrids include ModBus, ProfiBus, power line communication and the Ethernet; 2) the major wireless communication technologies currently in use include Zig-Bee, Wi-Fi, WiMax and cellular. Table 1 shows the wireless communication systems currently employed for microgrid communication.
LoRa, also known as ‘long-range’ is an emerging communication technology, with applications in Internet of Things (IoT). Several examples of LoRa’s application in various technical sectors are summarized as follows. In [13], a new architecture deploying LoRa was proposed for a large system monitoring, where the proposed system surpasses the need of existing gateways to enable much longer range of communication. The application of LoRa in farming and agriculture was presented by researchers in [14], where LoRa devices were employed to transfer data from sensors used for monitoring cows to achieve better animal health and wellbeing. A LoRa-based sensor system is proposed in [15] for monitoring personnel in unfriendly work environments, and the sensor data are sent to the cloud through LoRa. This communication medium facilitates analysis and observation of the data and sends alerts when a problem is detected. In [16], a car diagnostic system is proposed using LoRa to communicate sensor data to a cloud for vehicle fault diagnosis. Ref. [17] presents LoRa’s use in monitoring patients with mental health issues.
The works reviewed above demonstrate various systems where LoRa technology has been applied for communication and data transfer. However, to date, to the best of the authors’ knowledge, LoRa communication has not been applied for data transfer within microgrids. This paper is the first to apply LoRa for microgrid communication.
The motivations for proposing the LoRa-based communication system include: 1) low cost, 2) acceptable communication range, 3) low energy consumption, and 4) high possibility of using in remote areas where other forms of communication, such as the internet and cellular networks, do not exist.
The main objective of the proposed LoRa-based communication system in this paper is to achieve data transfer from the nodes to the private server through the gateway, which is a one-directional data transfer for the grid monitoring function.
The main contributions of this paper include:
1) Proposal, design and development of a novel LoRa-based data communication system for microgrids,
2) Range testing of the proposed communication system,
3) Hardware test bed implementation of the proposed communication system.
This paper is organized as follows: Section 2 presents a literature review; Section 3 reviews LoRa technology for use in microgrids; Section 4 presents the proposed LoRa based microgrid communication system; Section 5 describes the system implementation; Section 6 presents the experimental set-up and range test; Section 7 presents the results. Key features of the proposed system and discussions on the achieved results are presented in Section 8. Main conclusions and directions for future work are presented in Section 9.
2.
Literature review
2.1. Microgrid communication schemes and layers
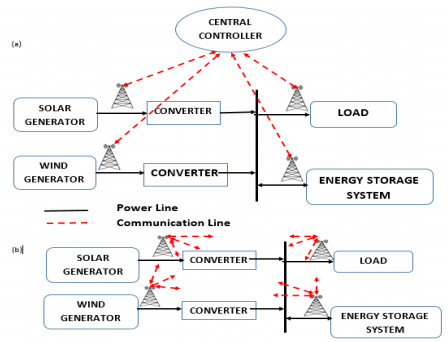
Predominantly, two types of data communication schemes are presently applied in microgrids: (a) centralized (also known as hierarchical), and (b) distributed. In the centralized scheme, all data from DGs, energy storage systems (ESSs), and loads are transmitted to the microgrid central controller, where the data are processed, and control commands are then transmitted back to the DGs and ESSs. In a distributed scheme, the data from DGs, ESSs, and loads are transmitted to other DGs and ESSs, where the data are received and processed [18,19]. The centralized scheme is easy to implement and maintain [20,21]. However, it has drawback in scalability, requires complex communication networks, and is more prone to communication failure [21]. The distributed scheme offers more flexibility and is more robust from communication failure [22]. On the other hand, it requires a complex algorithm and a longer processing time, has less precision and a higher possibility of data collision [23]. Most communication technologies (wired or wireless) can be employed in centralized or distributed data schemes [24]. Simplified illustrations of centralized and distributed data communication schemes are shown in Figure 2.
With full automation of microgrids in view, which would involve large number of sensors and actuators applied for monitoring and control, the microgrid communication can be classified into three levels [25] as shown in Figure 3.
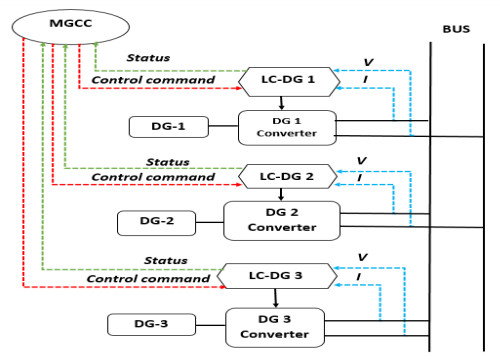
At the first (primary) level, the sensors are connected to a DG unit to be monitored, and are also connected to the local controller for data collection through serial connection. This is feasible due to the proximity of the sensors, the local controller and the monitored DG units, ESSs and interfacing converters [25]. The second (secondary) level involves the communication between various local controllers situated in the DG units and the microgrid central controller. The secondary communication level is the main communication level of a microgrid [25]. At the secondary communication level, the communication requirement is primarily to send the sensor data (voltage and frequency), which are obtained from the sensors by local DG controllers, to the microgrid central controller. Control signals are sent from the microgrid central controller back to the DG unit’s local controller for voltage and frequency control and stabilization. The microgrid circuit breaker status is also sent to the microgrid central controller during grid connection and isolation processes. Data to be transferred at the secondary communication layer can be formatted into packets that are generally in the bits size, and thus, the use of a reliable low-bandwidth communication system can handle the communication needs at this level. A bandwidth value of 1–100 kbps would be ideal for data transfer at the secondary communication level [44]. A detailed description of data transfer is shown in Figure 4, where the green and red dotted lines depict the status and control command communications, respectively, between the microgrid central controller and DG unit’s local controllers. The third (tertiary) level for communication between networked microgrids requires higher data rates.
In this study, the proposed LoRa-based communication system is designed for the second control/communication level of the microgrids, transferring data between the local controllers on each DG unit and the central controller of microgrids, which enables parameter updates and control command signal transfer.
2.2. LoRaWAN and LoRa technology
The LoRaWAN protocol is a low power wide area network (LPWAN) designed for wireless connection of equipment to the internet [26] to achieve bi-directional communication between nodes and servers. A typical LoRaWAN architecture consists of various end-nodes connected to sensors, one or more gateways, and a minimum of one network server as shown in Figure 5. LoRaWAN is deployed in a star of stars topology where various nodes are connected to the gateway via LoRa links, and the gateway is connected to the network servers by IP networks [43].
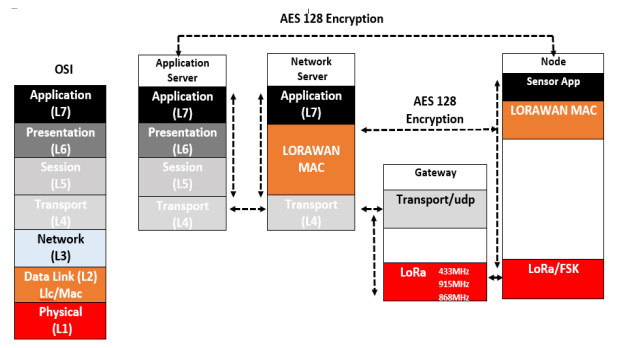
The communication between nodes and the gateway is achieved by the Physical Layer LoRa communication system. The gateway functions more like a router that relays the data received from various nodes to the Server (Figure 5). A comparison of the LoRaWAN protocol to the Open System Interconnection (OSI) reference as shown in Figure 6 can serve as a better explanation of the LoRaWAN protocol.
LoRaWAN networks are differentiated from the classical TCP/IP communication through gateways and end-nodes [43]. The network server operates over the Transport Layer and controls the Media Access Control layer functions of the network [43]. LoRa, a physical layer technology, operates on the “Physical” layer L1 in Figure 6 with its main function as the “Application” layer L7 data transmission to the medium [43]. The OSI model’s “Data link” layer L2 matches the LoRaWAN protocol, which defines secure medium access and end-node management strategies [43]. The end-nodes also have a function of energy conservation, which is at the “Application” layer L7 in the OSI model. Therefore, the end-nodes conduct functions at L1, L2, and L7 of the OSI model [43]. More details on LoRaWAN can be found in [26].
2.2.1. LoRa physical layer communication
LoRa is the physical layer or the wireless modulation used in creating a long-range communication link [26]. Most of the known wireless communication technologies use frequency shift keying (FSK) modulation at the physical layer [26] to achieve low power communication. LoRa, on the other hand, makes use of the Chirp Spread Spectrum Modulation (CSS) technique, which can achieve both low power and long-range communication. The chirp spread spectrum for message modulation works with chirps whose frequencies increase or decrease linearly over a certain amount of time [27]. LoRa operates in the free spectrum band (ISM), for example, at 868 MHz and 915 MHz band for Europe and USA, respectively [28]. It has a low data rate of up to 50 kbps [28], and bandwidth capabilities of 125 KHz, 250 KHz, and 500 KHz [29]. Conventionally, it uses a sweep tone, which increases (up chirp) or decreases (down chirp) in frequency over time for message encoding instead of the pseudorandom binary sequence used by the well-known direct-sequence spread spectrum (DSSS) [30]. The modulation technique employed in LoRa spreads the message (signal) over a wide bandwidth, which makes it less affected by noise and interference [30]. Other low power communication systems do not have this feature by default. A LoRa transceiver can decode transmission 20 dB below the noise floor, making very long-distance communication at low power consumption possible.
Four important parameters control LoRa communication, which need to be well adjustable to achieve peak communication. These parameters are: 1) Transmission Power: is typically between 2 dBm and 14 dBm although it can be adjusted between -4 dBm and 20 dBm. This parameter has a direct impact on the system energy consumption and the range of the signal coverage [30]. 2) Spreading Factor (SF): is concerned with how many bits are encoded in each symbol, which can be set from 6 to 12. The range of signal and signal-to-noise-ratio are both increased by an increased spreading factor. However, it has a reducing effect on the transmission rate when the energy consumption is increased. 3) Bandwidth (BW): LoRa operates on the bandwidths of 125 KHz, 250 KHz and 500 KHz. 4) Coding Rate (CR): is the amount of forward error correction (FEC) that is applied to the message for protection against interference [30]. Therefore, an equation that states the transmission rate of the LoRa system is stated as follows:
where, TR is Transmission Rate, SF is Spreading Factor, BW is Bandwidth, and CR is Coding Rate.
Ref [31] is the only literature that has looked into the enhancement of the Physical Layer scheme to solve the LoRa collision problems. Here an interleaved chirp spreading LoRa was devised to address LoRa’s capacity limitations. In [32], a decoding algorithm to alleviate the impact of collision on the LoRa communication system is developed by employing a slight de-synchronization of the superimposed signals and specific features of the LoRa network. A full Media Access Control (MAC) protocol for collision resolution was also designed. The performance of LoRa was improved, which indicates that LoRa can be applied to even higher collision scenarios without any complications. The operation of the LoRa communication system was observed under Doppler effects in an experiment carried out in [33]. It showed that the LoRa modulation had high immunity to Doppler effects, and can be used in satellite communication systems in orbits above 550 km with little or no restrictions. Ref. [34] proposes that LoRa’s performance can be enhanced by applying message replication and using LoRa gateways with more diversified antennas. Furthermore, [35] states that the mass produced LoRa kits have also reduced the communication strength of the LoRa system. Therefore, a customized LoRa transceiver should be designed in order to achieve improved characteristics, such as increased sensitivity and improved immunity to interference. In the recent past the improvement in LoRa’s scalability has been closely dependent on the variation of the Spreading Factor (SF) as mentioned in [36,37,38,39].
2.2.2. LoRa software implementation
LoRa communication is implemented on the software platform using open source codes available on GitHub. Various forms of software codes are made available in GitHub for easy design of automation systems. This alone contributes greatly to the low cost feature of the LoRa technology. The nodes are configured with various programming languages, such as C, C++ and Python. The nodes send processed sensor data to the gateway, through which the data can be forwarded to a network server.
3.
LoRa communication in microgrids
LoRa is a fast-rising wireless communication technology. Its advantages over the existing wireless technologies make LoRa a suitable choice for communication applications in microgrids. Table 2 shows a comparison of the LoRa technology with other technologies already being used in microgrids. LoRa can be the most attractive technology for microgrid communication applications particularly in remote areas. For a complete LoRa communication system design, the three components (node, gateway and server) are important, which are briefly explained in the following subsections.
3.1. LoRa node
LoRa nodes are basically Semtech SX1276 transceivers that operate at the ISM frequency bands (868,915,433 MHz) of various regions of the globe. The nodes can send and receive data over the frequencies with capabilities to directly connect with sensors through microcontrollers, which makes them very reliable for communication. LoRa nodes can connect to a large number of sensors, which are employed to measure various physical data such as voltage, current, temperature and pressure. There is one limitation that the LoRa node can only communicate with a node that is set to the same frequency. In microgrids, the LoRa node will basically fit into the end-nodes that are connected to various DG units for data acquisition through sensors and microcontrollers. The acquired data is then sent out at a fixed frequency. A LoRa node has the capability to operate with open source microcontrollers such as the Arduino Uno, Arduino Mega or ESP 32 to process the acquired data. There are three classes of LoRa nodes (Class A, B, and C) based on their capability of enabling bi-directional communication. In particular, Class C allows for a seamless bi-directional communication [26]. In this paper, this class of LoRa node is employed. The whole process of data acquisition and transmitting can be programmed on the end-node on the Arduino Integrated Development Environment (IDE) by coding.
3.2. LoRa gateway
A LoRa gateway comprises of a concentrator board with the capability to receive and forward LoRa packets (data received from the nodes) to the server. It also allows reverse communication from the server to the nodes for control purposes. A LoRa gateway can be described as a bridge between the sensors and the server. There are two types of LoRa gateways: single-channel and multi-channel. The multi-channel gateway has the ability to receive data at different frequencies. It is applied mostly to systems that require high amount of nodes operating at different frequencies. In microgrids, the LoRa gateway can be located at the microgrid central controller, where it can obtain the data sent in by various nodes connected to different DG units. Although the gateway has no data processing capabilities, it can collect all the data received from the nodes, and then relay to the server for processing.
3.3. LoRa server
A server is a database with a user interface where the acquired data can be stored, processed and displayed to the user. In microgrids, the server serves as the central controller where the received data is processed, and control messages are sent back to the DG units.
4.
The proposed LoRa communication system
In this paper, the proposed LoRa-based communication system consists of an IoT approach for transfer data. This involves the interaction between the LoRa communication devices and the sensors that are connected to various microgrid components for monitoring and transferring data. The sensors are programmed to obtain data from the monitored components. The data are processed and then sent by the LoRa nodes to the gateway. The gateway forwards these data to the server for processing. As stated earlier, the proposed system belongs to the second communication level. Figure 7 shows the full configuration of the proposed LoRa-based communication system for microgrid monitoring.
In a practical microgrid, LoRa nodes can be connected to various local controllers. The system operation data for DG units, circuit breakers and battery storage are available at the local controllers. Such data can be transmitted by LoRa to the central controller of the microgrid. Command signals can also be sent back from the central controller to the local controllers using the proposed LoRa-based communication system.
5.
Proposed system implementation methodology
To implement the proposed LoRa-based Microgrid communication system, the sensors are connected to the Arduino Uno. The Arduino Uno unit is connected to the LoRa shield for data transmission. On initialization, the sensors read the monitored data, and the data are accessed by the Arduino through the connection pins, which are processed using modified open-source Arduino codes from the open-source coding platform (GitHub). The processed data is displayed on the serial monitor of the Arduino IDE. At this point, the values of the sensor readings are shown to confirm if the sensors had measured the correct values from the DG units. After the display and confirmation, the data is converted to LoRa packets for transmission using codes that are also integrated into the main processing program and uploaded to the board. The illustration of how a measured data is converted into LoRa packet format for transmission is shown in Section V. The format for transmitting the data over LoRa is in Base64 format. Once in this format, the data can be transmitted to the gateway at the fixed allotted frequency for the sub-unit. This process is repeated continuously as long as the system is connected to the DG unit and is powered ‘ON’. The flowchart for the operations for the node connected to the DG unit is shown in Figure 8.
The gateway consists of a RAK831 concentrator module with multi-channel (8 channels) capabilities connected by the General Purpose Input/Output (GPIO) pins to the Raspberry Pi that houses the local server. Once data arrives at the concentrator, it is forwarded to the server for processing. As stated earlier, the concentrator has no processing abilities but has the ability to pick up data that are sent by only designated nodes through a method “frequency selection”. The RAK 831 concentrator is programmed to accept data packets arriving from devices that have been locked to its range of frequencies. The flow chart of the operation of the LoRa concentrator is shown in Figure 9.
The accepted data is then forwarded to the server through a designated port on the Raspberry Pi. Conventionally, in most IoT applications, web-based clouds, such as the Things Network, Thinger IO, Adafruit and LoRa Server, are used for monitoring. This requires monitoring from internet-enabled devices. This means that the data cannot be monitored without internet. Furthermore the data is at a lower security level. In this work, the server was developed using Node-Red that was installed on the Raspberry Pi. This can be managed and viewed locally by authorized users. The main difference between the private server proposed in the paper and the cloud-based server is the absence of internet for data access in the private server. The advantage of the proposed private server is the data security. The private server is hosted on a hardware, which allows verification before someone can have access to the data received at the server. This makes the private server applicable to any system that requires a high level of data privacy.
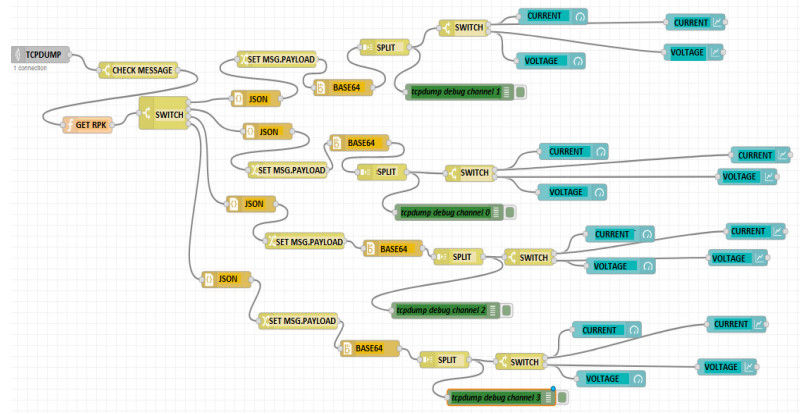
At the server, the data is accessed at a designated port using the TCDUMP protocol. A java function code is written in the function node to extract the data, which contains the Base64 data. This node is also followed by a frequency dissection node (switching node) where the data is classified according to their frequencies. The data in each frequency is passed through a JSON block, where the data is changed to Base64 object. Then through a Base64 node, the data is converted back to human readable values. These values are then displayed on the server using the debug tool, and can be further displayed on gauges and charts as shown in Figure 10. The Node-Red flows to attain this function is shown in Figure 11.
5.1. LoRa data processing algorithm
The sensor data are transmitted using LoRa communication to the gateway terminal. The data being transmitted must be processed into the LoRa acceptable format before transmission. This format must conform to the required length and data size for appropriate transmission. LoRa uses the chirp spread spectrum as its mode of data modulation [26]. LoRa transfers the data in the Base64 format and the structure of a basic LoRa data frame is shown in Figure 12.
5.1.1. Base 64 encoding of a measured sensor value
Prior to transmission, the measured data are formatted into a LoRa transmittable data packet as illustrated using two examples. To encode the data in Base64, the following steps are followed:
a) The data is prepared in ASCII text format
b) The data is converted to ASCII binary format for each letter of the ASCII text
c) The binary codes obtained from Step 2) with 8-bits are joined together
d) The data is split into groups of 6-bit binary codes
e) The groups of six bits are converted back to decimal
f) Each obtained decimal value is matched with a Base64 symbol on the Base64 table where each decimal value between 0-63 has a matching symbol.
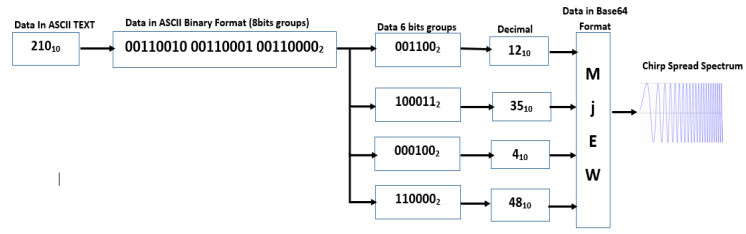
As an example, the steps of data conversion for a voltage sensor value of 210 V (as measured and converted for transmission), are shown in Figure 13.
As shown in Figure 13, the voltage value of 210 V is transmitted over LoRa as a Base64 Symbol ‘MjEw’. Correspondingly, if the data is to be sent as a value specifically from a device on a particular DG unit, with specific details such as a voltage sensor connected to DG unit 2 on Channel 2 with device number 2, and measured voltage value of 210 V, the data to be transmitted is shown in Figure 14.
The length of the data in Base64 format can be obtained by a relationship from the character length of the ASCII format. This is given as:
where, ML is the Base64 message length, N is the length of ASCII text message. For instance, our data above in ASCII TEXT [DG02V210DEV2Ch2] has a length of 15 bytes.
Therefore, the Base64 encoded message will have 20 characters using the equation above.
6.
Experimental test bed setup
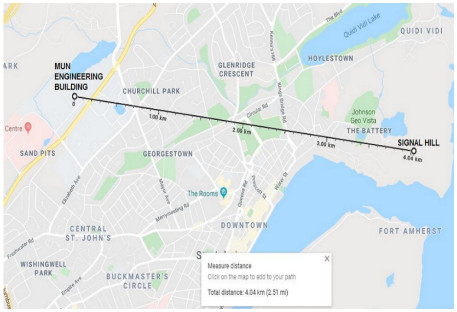
The proposed communication system was validated with a range test to confirm its maximum distance and accuracy of data transmission and reception. In this experiment, the LoRa gateway was placed at the top-most window on the Memorial University of Newfoundland (MUN) engineering building, and kept stationary with power ‘ON’. The node on the other hand was moved around in a car. Packet delivery was monitored at various distances as shown on the map in Figure 15.
Also, a test bed was set up in the Electrical Engineering Laboratory of MUN to observe the physical implementation of the proposed system. The laboratory houses a photovoltaic (PV) semi-microgrid consisting of 12 solar panels, which produces 130 W with 7.6 A at each panel. Two PV modules are connected in parallel to obtain 6 sets of PV systems with 260 W at about 14 A with a storage system. The system has a Maximum Power Point Tracking (MPPT) controller, which ensure maximum power production even during periods of unfavorable weather conditions.
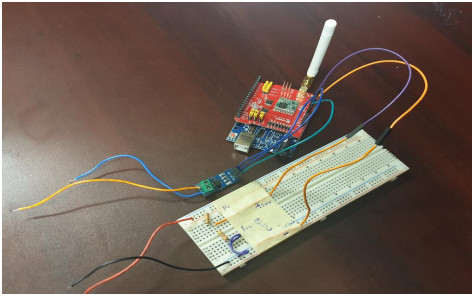
During experiments, the voltage and current values of four sets of PV systems were monitored using four LoRa nodes. This setup was developed as a proof of concept, which can be scaled up to a larger number of nodes as required. Each node set-up is made up of an Arduino Uno microcontroller [48], a Dragino LoRa shield [49], AC712 Hall effect current sensor [50], and a voltage sensor as shown in Figure 16. Each PV unit’s maximum voltage output is 16 V and the maximum current extraction is 30 A. This governs the choice of voltage and current sensors employed in the experiment. The wiring was sized to meet the voltage and current ratings. The voltage sensor was built using a resistive voltage divider so as to not overload on the arduino microcontroller. This was scaled back up after data processing using the original voltage division ratio. These values were processed by the nodes and were sent to the gateway located in another part of the laboratory about 10 meters apart. At this location, their values were received, processed and displayed on the gauges and chats for observation. The gateway is comprised of a RAK831 LoRa concentrator [51], a Raspberry Pi 3 B+ model [52] and a monitor connected using HDMI. The experimental test bed setup is shown in Figure 17 with the LoRa nodes circled in green. These nodes were connected to various PV systems for data acquisition and transmission. The features and the mode of operation of the hardware components in the experiments are explained in references [48,49,50,51,52].
7.
Test results
7.1. Range test results
The results obtained from the LoRa range test are shown in Table 3. Table 3 shows the packet delivery ratio (PDR) of the LoRa node at various distances from the gateway. The nodes sent data at the spreading factor of 7, coding rate of 4/5, and bandwidth of 125 kHz.
Table 3 shows up to a distance of 4 km where a PDR of up to 90% was obtained. A PDR lower than 90% can reduce the fidelity of the data being transferred due to packet losses. Although not shown in Table III, the experiment was performed up to 7 km. However, after 4 km, the rate of packet delivery reduced drastically. This is caused by various forms of obstructions in the urban test area. LoRa can achieve data delivery to almost 10 km in rural areas that have very low amount of obstructions. A data transfer range of 5 km maximum can be achieved in urban areas due to the presence of buildings and other structures [40,41,42].
The results from the range test show the packet delivery ratios for data transfer distances at a fixed Spreading Factor of 7. In reality, LoRa devices have the capability to automatically switch between Spreading Factors 7 and 12 to achieve better system performance in terms of transmission distance and data rate.
7.2. Experimental test bed results
The proposed communication system was set up within the electrical laboratory about 10 meters apart from the sensors node and the gateway. Data transfer between the DG units and the central control system was achieved using LoRa communication technology to attain 100% data delivery and accuracy. As presented in Table 3, a range test was carried out to confirm the maximum distance at which the nodes (end devices) could send data to the gateway without packet losses. The sensors measured voltages and currents, which are displayed on the charts at the server end. This confirmed that the data were acquired, processed and transmitted using the LoRa communication technology without any disruption.
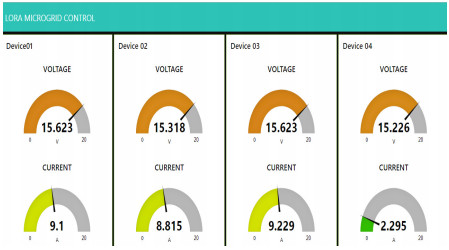
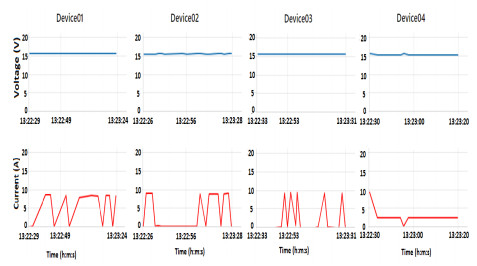
Figure 18 shows the measured data arriving at the RAK 831 LoRa gateway in the Base64 format. The data packet circled in yellow are the actual data to be monitored. The data is comprised of the DG unit device number, monitored value and channel number, which are decoded before they are displayed. The data obtained at the gateway is forwarded to the local node-Red server through the TCPDump port for decoding, separation and display as shown in Figure 11. Figure 19 shows the measured voltage and current values from each node displayed on the Node-Red gauges as devices 1 to 4. The values in Figure 19 are instantaneous screenshots of the voltage and current values obtained from each unit of the PV system. Figure 20 shows the measured voltage and current over a period of time, which demonstrates their real-time fluctuations. The stochastic nature of the current in Figure 20 is due to the intermittent nature of the renewable energy source (PV) as the measurements were taken before the MPPT. The measured values conformed to the values displayed on the PV system boards. This verifies that the data are transferred and displayed in real-time.
8.
Discussions
This paper presents the design and development of a LoRa-based communication system for data transfer in microgrids for remote area applications. The features of the proposed system are summarized as follows:
● Long Range Communication: A data transmission range of 4 km was achieved. Table I shows the distances of data coverage of the existing wireless communication systems. Although LoRa cannot achieve data coverage distance compared with Cellular and WiMAX, LoRa is more advantageous from other perspective such as low cost and low power consumption, which are critical factors for remote application. Research activities are on-going to determine approaches through which range of data transfer can be extended in LoRa.
● Low Cost: The components for the proposed systems are readily available and inexpensive. Table 4 shows a bill of materials of the components used in the prototype.
LoRa is an open source system with no continual charges as compared with WIMAX and Cellular communication.
● Low Power Consumption: Table 5 shows the power requirements of the various existing wireless communication systems currently being used in microgrids. The study was carried out in real-time where the actual power requirements of the routers were used. The power requirement values were obtained from the datasheets of the devices for each communication system [45,46,47].
The results in Table 5 was obtained for data transfer between two devices of each wireless communication system. This approach reduced the complexity of the study since expanding the network would subsequently increase the power consumption. LoRa communications system still shows one of the lowest power consumption.
● Multi-sensor Connection Capabilities: The proposed system allows the connection of a high number of sensors, which is a highly desirable feature for IoT application in microgrids. The system has the capability to monitor multiple parameters simultaneously. This advantage is demonstrated in our experiment where two sensors (voltage and current) have been connected to each node. Although only two sensors were connected in the setup, a node is able to accommodate multiple sensors if required.
9.
Conclusion and future work
A LoRa-based communication system for data transfer within microgrids is proposed in this paper. The scheme can be employed to transfer measured data such as voltage, current, frequency and other parameters between the local controllers of DG units and the central controller of microgrids at the secondary level of control and communication. It features long-range data transfer up to 4 km, high interference immunity, low cost and low power consumption. The system can be deployed in areas where no internet or other form of communication exists, ideally for remote areas in developing countries. The proposed LoRa-based communication system was tested for one-directional communication where data was transferred from a DG unit’s local controller represented by the LoRa nodes to the microgrid central controller housed by the LoRa gateway connected to the Raspberry Pi.
To achieve bi-directional communication, a reverse communication for command transmission is required. This will be explored in our future work. In this phase, the communication system will be set up to observe if DG units can be controlled from the server using LoRa. In addition, the delays caused by LoRa communication will be studied in future.
Acknowledgement
The authors would like to appreciate the School of Graduate Studies and the Faculty of Engineering and Applied Science at Memorial University of Newfoundland, Canada for the conducive environment and the facilities to carry out this research. Our profound appreciation also goes to our families and friends for their support.
Conflict of interest
The authors declare that there is no conflict of interest regarding the publication of this paper.










 DownLoad:
DownLoad: