A mathematical model of the Warburg Effect: Effects of cell size, shape and substrate availability on growth and metabolism in bacteria

Anshuman Swain; William F Fagan; Anshuman Swain; William F Fagan

doi:10.3934/mbe.2019009

Mathematical Biosciences and Engineering

2019, Volume 16, Issue 1: 168-186. doi: 10.3934/mbe.2019009

Previous Article Next Article

Research article Special Issues

A mathematical model of the Warburg Effect: Effects of cell size, shape and substrate availability on growth and metabolism in bacteria

Anshuman Swain ^,,
William F Fagan

Department of Biology, University of Maryland, College Park, MD 20742, USA

Received: 14 March 2018 Accepted: 05 October 2018 Published: 11 December 2018

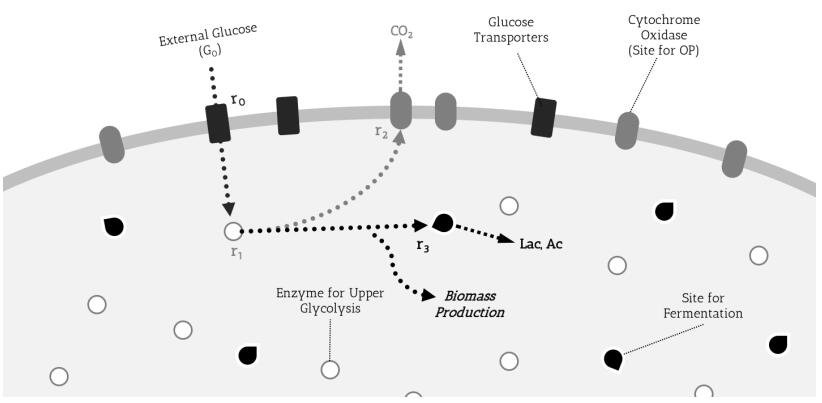
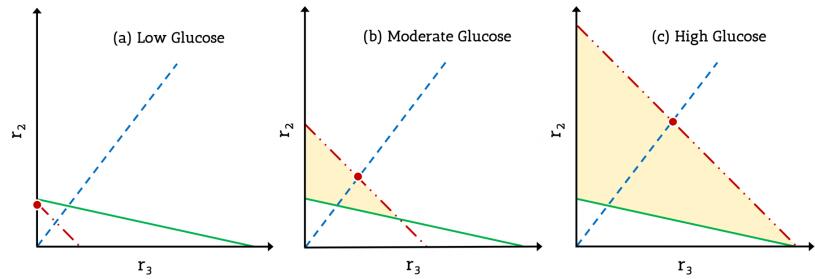
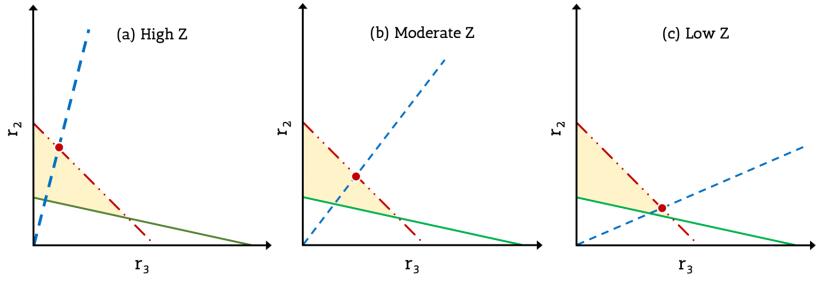
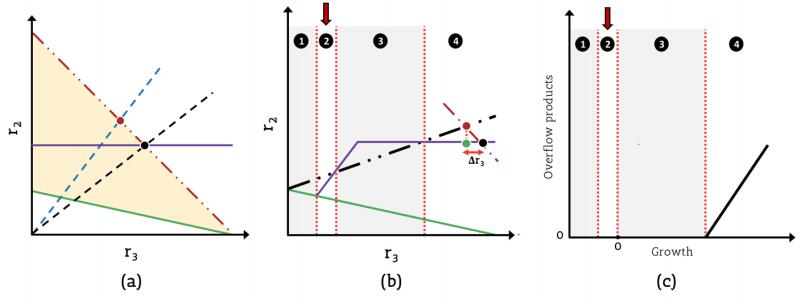
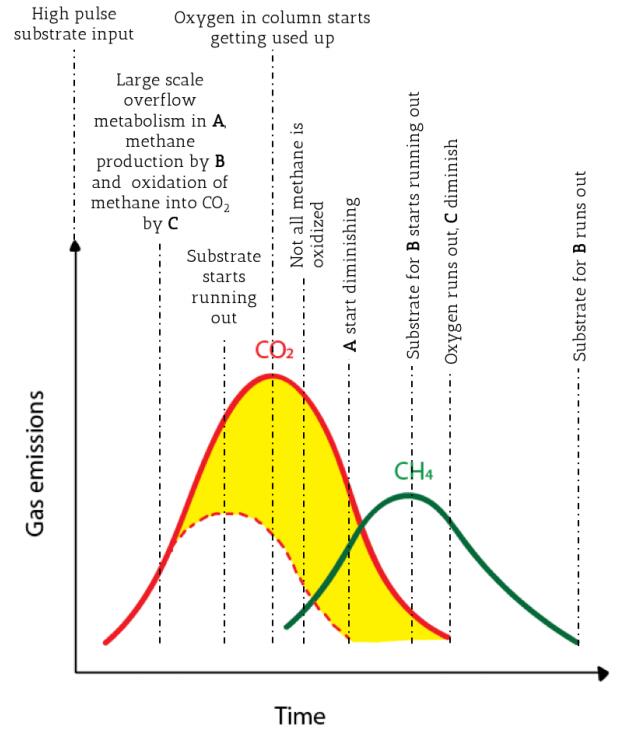
The Warburg effect refers to a curious behavior observed in many organisms and cell types including cancer cells, yeast and bacteria, wherein both the efficient aerobic pathway and the inefficient fermentation pathway are utilized for respiration, despite the presence of ample oxygen. Also termed as overflow metabolism in bacteria, this phenomena has remained an enigmatic and poorly understood phenomenon despite years of experimental work. Here, we focus on bacterial cells and build a model of three trade offs involved in the utilization of aerobic and anaerobic respiration pathways (rate versus yield, surface area versus volume, and fast versus slow biomass production) to explain the observed behavior in cellular systems. The model so constructed also predicts changes in the relative usage of both pathways in terms of size and shape constraints of the cell, and identifies how substrate availability influences growth rate. Additionally, we use the model to explain certain complex phenomena in modern- and paleo-ecosystems, via the concept of overflow metabolism.

Keywords:

Citation: Anshuman Swain, William F Fagan. A mathematical model of the Warburg Effect: Effects of cell size, shape and substrate availability on growth and metabolism in bacteria[J]. Mathematical Biosciences and Engineering, 2019, 16(1): 168-186. doi: 10.3934/mbe.2019009

Related Papers:

[1]	Evi Mintowati Kuntorini, Laurentius Hartanto Nugroho, Maryani, Tri Rini Nuringtyas . Maturity effect on the antioxidant activity of leaves and fruits of Rhodomyrtus tomentosa (Aiton.) Hassk.. AIMS Agriculture and Food, 2022, 7(2): 282-296. doi: 10.3934/agrfood.2022018
[2]	Francyelli Regina Costa-Becheleni, Enrique Troyo-Diéguez, Alan Amado Ruiz-Hernández, Fernando Ayala-Niño, Luis Alejandro Bustamante-Salazar, Alfonso Medel-Narváez, Raúl Octavio Martínez-Rincón, Rosario Maribel Robles-Sánchez . Determination of bioactive compounds and antioxidant capacity of the halophytes Suaeda edulis and Suaeda esteroa (Chenopodiaceae): An option as novel healthy agro-foods. AIMS Agriculture and Food, 2024, 9(3): 716-742. doi: 10.3934/agrfood.2024039
[3]	Pham Thi Thu Ha, Nguyen Thi Bao Tran, Nguyen Thi Ngoc Tram, Vo Hoang Kha . Total phenolic, total flavonoid contents and antioxidant potential of Common Bean (Phaseolus vulgaris L.) in Vietnam. AIMS Agriculture and Food, 2020, 5(4): 635-648. doi: 10.3934/agrfood.2020.4.635
[4]	Nicolas Nagahama, Bruno Gastaldi, Michael N. Clifford, María M. Manifesto, Renée H. Fortunato . The influence of environmental variations on the phenolic compound profiles and antioxidant activity of two medicinal Patagonian valerians (Valeriana carnosa Sm. and V. clarionifolia Phil.). AIMS Agriculture and Food, 2021, 6(1): 106-124. doi: 10.3934/agrfood.2021007
[5]	Ebrahim Falahi, Zohre Delshadian, Hassan Ahmadvand, Samira Shokri Jokar . Head space volatile constituents and antioxidant properties of five traditional Iranian wild edible plants grown in west of Iran. AIMS Agriculture and Food, 2019, 4(4): 1034-1053. doi: 10.3934/agrfood.2019.4.1034
[6]	Chiao Ying Huang, Gerald L. Riskowski, Jennifer Chang, Ching Hsuan Lin, Jinn Tsyy Lai, Audrey Chingzu Chang . Pecan shell by-products—phenolic compound contents and antimicrobial properties. AIMS Agriculture and Food, 2020, 5(2): 218-232. doi: 10.3934/agrfood.2020.2.218
[7]	Olukemi Osukoya, Adewale Fadaka, Olusola Adewale, Oluwatobi Oluloye, Oluwafemi Ojo, Basiru Ajiboye, Deborah Adewumi, Adenike Kuku . In vitro anthelmintic and antioxidant activities of the leaf extracts of Theobroma cacao L.. AIMS Agriculture and Food, 2019, 4(3): 568-577. doi: 10.3934/agrfood.2019.3.568
[8]	Albert Nugraha, Asadin Briliantama, M Umar Harun, Li Sing-Chung, Chin Xuan Tan, Vuanghao Lim, Amir Husni, Widiastuti Setyaningsih . Ultrasound-assisted extraction of phenolic compounds from ear mushrooms (Auricularia auricula-judae): Assessing composition and antioxidant activity during fruiting body development. AIMS Agriculture and Food, 2024, 9(4): 1134-1150. doi: 10.3934/agrfood.2024059
[9]	Thi Thuy Le, Trung Kien Nguyen, Nu Minh Nguyet Ton, Thi Thu Tra Tran, Van Viet Man Le . Quality of cookies supplemented with various levels of turmeric by-product powder. AIMS Agriculture and Food, 2024, 9(1): 209-219. doi: 10.3934/agrfood.2024012
[10]	Rossaporn Jiamjariyatam, Orachorn Mekkerdchoo, Pakkapong Phucharoenrak, Lu Zheng . Effects of freeze-drying and vacuum-drying on the quality, total phenolic contents, and antioxidant activities of bee honey in northern Thailand. AIMS Agriculture and Food, 2024, 9(2): 430-444. doi: 10.3934/agrfood.2024025

Abstract

1. Introduction

Eleutherine palmifolia (L.) Merr., in Indonesia called "bawang dayak", is a medicinal plant that is grown in Borneo Island, Kalimantan, Indonesia. E. palmifolia is one of the species of Iridaceae family and is considered a synonym of E. americana, E. plicata and E. bulbosa ^[1,2]. This species is widely cultivated in regions of Africa, South America, and Asia for its beneficial chemical compounds as traditional medicine ^[2,3]. E. palmifolia is traditionally used to treat diabetes, stroke, hypertension, breast cancer, fertility, and hypertensive diseases ^[4]. Numerous studies reported the beneficial effects of E. palmifolia on human health, including its anti-cancer ^[5], anti-fungal ^[6], anti-malarial ^[7], antioxidant ^[8] and anti-melanogenesis ^[9] activities. Bioactive chemicals of E. palmifolia have a large variety of pharmacological targets. For example, the antidiabetic activity of E. palmifolia is strongly connected with its eleutherinoside A content ^[4], whereas its antioxidant activity is associated with polyphenols ^[8]. Polyketide compounds in E. palmifolia have been linked to its antimicrobial activity ^[10]. With regard to investigations on E. palmifolia as a medicinal plant, it is very important to associate the number of active compounds in the plant extracts with its pharmacological activity. Therefore, in this study, total phenolic and flavonoid content (TPC and TFC) and antioxidant activity were assessed as parameters to evaluate the effect of nitrogen (N) and potassium (K) fertilizers on E. palmifolia.

Plant nutrition is a critical aspect of increasing plant productivity. An important factor in plant growth and development is N ^[11]. N fertilizers have an impact on the yield and productivity of many plants, such as onions ^[12,13], rice ^[14] and milk thistle ^[15]. On the other hand, K is one of the most critical elements in physiological functions, such as photosynthetic capacity, ionic homeostasis, carbohydrate metabolism ^[16], enzymatic activation ^[17], water relations ^[18], osmotic adjustment ^[19] or resistance against abiotic stress ^[20,21] of plants. Secondary metabolites and their biological activity in plants may be influenced by N and K levels as reported by Barzegar et al ^[22], who stated that combination of N and K increased the polyphenol and vitamin C content and antioxidant activity of sweet fennel. Another study found that fertilizing N and K combination on Berberis microphylla increased the polyphenol secondary metabolite contents and antioxidant activities ^[23]. Typically, E. palmifolia is planted as a side plant by farmers and is not cultivated under optimal conditions. Numerous fertilizers can influence the flavonoid content, growth, and yield of E. palmifolia. Application of NPK 16:16:16 fertilizer at 2.5 g/L increased the production of E. palmifolia, and a concentration of 1.25 g/L increased its flavonoid content ^[24], chicken manure ^[25], and vinasse liquid organic ^[26]; however, our knowledge is limited about the effect of the N and K on the growth, polyphenol content, and antioxidant activity of E. palmifolia. In this study, we investigated the effect of N and K combination on morphological characters, polyphenol content and antioxidant activity of different extracts of E. palmifolia. The application of nitrogen and potassium fertilizers with optimum concentrations may have a positive impact on increasing the growth and yield of E. palmifolia, as well as improving the quality of its important chemical compounds.

2. Materials and method

2.1. Experimental treatments

Eleutherine palmifolia seedlings were obtained from the Faculty of Agriculture of Bengkulu University. The research was conducted from February to June 2020 in Bengkulu province, Indonesia (3°45′44″ S; 102°16′45″ E; altitude of 15 m). Bengkulu University station is located at 15 m above sea level with range of humidity of 78.6% to 85.9%, temperature of 25.5 ℃ to 28.5 ℃, and 4.5 mm/year of rainfall. Urea (46% N) and KCl (50% K₂O) were used as the N and K source fertilizer, respectively. The treatments of N and K combinations are presented in Table 1. The experiment was organized in a completely randomized design with 3 replications. K fertilizer was applied at the time of planting, while N fertilizer was given in two times, once at the time of planting (0.5 dose) and again 21 days after planting (0.5 dose). The fertilizers were given according to the treatments as presented in Table 1.

The sixth-month bulbs of E. palmifolia were used as planting material. The bulbs were selected with relatively uniform shape and size. The top 1/4 of bulbs were cut horizontally using a cutter to enhance shoot growth. The bulbs were immersed in a PGPR (plant growth promotion rhizobacteria) solution with a concentration of 10 ml/L for 15 min. Bulbs were planted in polybags with a diameter of 45 cm containing 10 kg of growing medium (mixed of soil, cow manure, and rice husk in a ratio of 2:1:1 (v/v/v)). The chemicals properties of growing medium were consisted of pH 4.43, C 3.99%, N 0.31%, P 6.10 ppm, and K 0.20 meq of K/100 g of soil). Each polybag was planted with three E. palmifolia bulbs. The irrigation, fertilization, weed, and diseases control were manually performed for maintenance of plants. Plant height, number of tillers, number of leaves, chlorophyll reading value (SPAD), total plant weight, number of bulbs, the diameter of the bulb, and fresh bulb weight were observed as morphological characteristics. Randomly selected ten plants from each unit were used for data collection. Four months after planting, the bulb of E. palmifolia (n = 10 plants) randomly selected plant was harvested and further analyses were carried out.

Table 1. Treatment combination of N and K fertilizers.

Treatment combination	kg of N per ha	kg of K₂O per ha
N₀K₂₅	0	12.5
N₁₀₀K₂₅	46	12.5
N₂₀₀K₂₅	92	12.5
N₁₀₀K₀	46	0
N₁₀₀K₅₀	46	25
N₁₀₀K₁₀₀	46	50

| Show Table

DownLoad: CSV

2.2. Sample preparation and extraction

The bulbs collected of randomly selected ten plants of each treatment were cleaned, cut, dried, and then reduced into powdered form. Samples were extracted using aqueous, ethanol, and n-hexane as solvent extraction using the procedure by Nurcholis et al ^[27] with modifications. Briefly, sample (2 g) was extracted twice with solvent (10 mL) in the dark room and then sonicated and stirred for 30 min. The mixtures were centrifugated at 10, 000 × g at 4 ℃ (Kitman-T24, Tomy Tech USA Inc.) for 15 min, and the supernatants were concentrated to the volume of 10 mL using a rotary vacuum evaporator (HAHNVAPOR, Korea). The final extract concentration of E. palmifolia that used for further analysis was 2 g/10 mL or 0.2 g/mL. The final extract of E. palmifolia was obtained and then used for further analysis total phenolic, flavonoid contents, and antioxidant activity.

2.3. Total phenolic content (TPC) and total flavonoid content (TFC)

In accordance with previously published assays, TPC and TFC were determined using spectrophotometric techniques ^[28]. TPC was measured in the extract obtained from each sample with Folin-Ciocalteu reagent and expressed as mg gallic acid equivalents per g of dry weight (mg GAE/g DW). Meanwhile, TFC was measured using an aluminium chloride reagent and expressed as mg quercetin equivalents per g of dry weight (mg QE/g DW).

2.4. Antioxidant analysis

Antioxidant activity was determined using two in-vitro techniques. The free radical scavenging and reducing power antioxidant activities were determined using the 2, 2-diphenyl picrylhydrazyl (DPPH) and ferric reducing antioxidant power (FRAP) assays ^[29]. Results of antioxidant activity expressed in μmol Trolox equivalent per g of dry weight (μmol TE/g DW).

2.5. Antioxidant analysis

Three replicates were used to get mean data ± SD. ANOVA was determined using the ExpDes packages in R, and then a Scott-Knott test was performed ^[30]. When p < 0.05 is used, there are significant differences.

3. Results and discussion

3.1. Growth and yield of E. palmifolia

Nitrogen is a macroelement that all plants, including E. palmifolia, require in substantial amounts to enhance their growth and productivity ^[13]. The results of the study showed that the combination of N and K fertilizer significantly (p < 0.05) affected chlorophyll reading value and total plant weight, which all attained maximum values at a combination of 46 kg/ha N and 12.5 kg/ha K (Table 2). The results showed that the combination treatment of N and K fertilizers has no significant effect on the growth parameters of plant height, number of tillers, and leaves of E. palmifolia. An increase in chlorophyll reading value with growth parameters was also found in strawberries treated with fresh chicken manure ^[31]. The role of nitrogen during plant growth can be observed through the chlorophyll reading value in plants to increase the efficiency of N and K use according to plant needs ^[32].

The results recorded that treatment of N (0–92 kg/ha) combined with K (12.5 kg/ha) increased the chlorophyll reading value of E. palmifolia compared to other combination treatments (Table 2). This data indicated that the K (12.5 kg/ha) produced the highest chlorophyll reading value. The obtained data of this work were in line with the previous study on cotton photosynthetic capacity ^[33]. As reported by Naciri et al. ^[34], potassium significantly affects the chlorophyll content of tomato. The result indicated the importance of the presence of K for plant growth of E. palmifolia, especially in the chlorophyll formation.

A higher total plant weight parameter was shown in the combination treatment of 46 kg/ha N with 12.5 kg/ha K, which was not significantly different from the combination treatment of 46 kg/ha N with 0–50 kg/ha K (Table 2). These results indicate that the application of nitrogen fertilizer combination of N and K was required for E. palmifolia to increase growth and development, which was shown by higher plant weights. In different plants, N and K enhances the growth performance in rice ^[35], strawberry ^[36] and rainfed wheat ^[37] plants.

Table 2. The growth characters of E. plamifolia as a response to the application of different fertilizers.

Treatment combination	Plant height (cm)	Number of tillers	Number of leaves	Chlorophyll reading value (SPAD)	Total plant weight (g)
N₀K₂₅	36.32 ± 2.14^a	8.00 ± 5.29^a	27.00 ± 5.29^a	46.03 ± 6.56^a	78.00 ± 6.55^b
N₁₀₀K₂₅	40.58 ± 3.64^a	13.67 ±7.37^a	40.00 ± 9.98^a	47.65 ± 1.43^a	95.33 ± 7.50^a
N₂₀₀K₂₅	41.95 ± 1.72^a	8.00 ± 2.64^a	25.00 ± 2.64^a	47.80 ± 11.60^a	78.67 ± 8.08^b
N₁₀₀K₀	38.92 ± 1.59^a	10.17 ± 10.15^a	31.67 ± 10.15^a	37.33 ± 4.73^b	94.83 ± 14.25^a
N₁₀₀K₅₀	39.90 ± 2.03^a	10.83 ± 6.33^a	36.83 ± 6.33^a	37.00 ± 4.73^b	106.33 ± 12.66^a
N₁₀₀K₁₀₀	40.62 ± 2.27^a	10.67 ± 9.98^a	39.33 ± 7.37^a	35.33 ± 1.53^b	102.83 ± 10.34^a
Note: Each value is expressed as the mean plus standard deviation; The Scott-Knott test indicates that the mean values in each column denoted by different letters differ significantly at p < 0.05; For the treatment combination, see Table 1.

| Show Table

DownLoad: CSV

The effect of N and K fertilizers on the yield parameters of E. palmifolia is presented in Table 3. The results showed that the combination of N and K fertilizers has no significantly different effect on the number and diameter of a bulb of E. palmifolia. However, the combination of N and K fertilizers significantly impacted fresh bulb weight. The highest fresh bulb weight (66.01–71.14 g/clump) was obtained in the combination treatment of N fertilizer (46–92 kg/ha) with K fertilizer (0–50 kg/ha). Similar results were previously reported by Gebretsadik and Dechassa ^[38] that increasing the rate of nitrogen from 50 to 100 kg/ha increased the onion marketable fresh bulb yield by a different percentage of 30%. The result showed that the treatment of K fertilizer (12.5 kg/ha) without N fertilizer produced the lowest tuber weight (58.68 g/clump). The result indicates the importance of nitrogen nutrients in increasing the yield of E. palmifolia. Similar results were reported by Uher et al. ^[39] that the yield depended on the increase in nitrogen dose, and the highest nitrogen dose obtained the highest broccoli yield. The deficiency of nitrogen may decrease chlorophyll biosynthesis, which leads to yield reduction.

The results showed that without the addition of K fertilizer, E. palmifolia produced good yields (Table 3). Under conditions of low K availability, plants have a strategy to maintain K needs. For example, the plant increased K uptake from the soil redistributes K ions between cytosolic and vacuolar pools, cytosolic homeostasis, and modifications in root system development and architecture ^[40]. Supplying optimum N and K levels was proved to be essential for plant growth and production of high yield and improving the quality of E. palmifolia.

Table 3. The yield components of E. plamifollia as response to fertilizer application.

Treatments	Number of bulbs	Diameter of bulb (cm)	Fresh bulb weight (g)
N₀K₂₅	14.67 ± 1.3^a	1.75 ± 0.06^a	58.68 ± 6.11^b
N₁₀₀K₂₅	16.17 ± 2.4^a	1.76 ± 0.08^a	71.14 ± 5.29^a
N₂₀₀K₂₅	13.83 ± 1.5^a	1.75 ± 0.02^a	67.06 ± 2.64^a
N₁₀₀K₀	11.50 ± 2.6^a	1.81 ± 0.06^a	65.55 ± 8.75^a
N₁₀₀K₅₀	11.50 ± 0.9^a	1.86 ± 0.09^a	66.70 ± 5.76^a
N₁₀₀K₁₀₀	14.67 ± 4.5a	1.75 ± 0.11^a	66.01 ± 7.28^a
Note: Each value is expressed as the mean plus standard deviation; The Scott-Knott test indicates that the mean values in each column denoted by different letters differ significantly at p < 0.05; For the treatment combination, see Table 1.

| Show Table

DownLoad: CSV

3.2. Growth and yield of E. palmifolia

TPC in the E. palmifolia extracts is strongly influenced by the combination of N and K fertilizer. TPC ranged from 2.192–3.688 GAE /g DW in aqueous extract, 1.474–2.815 GAE/g DW in ethanol extract, and 0.219–0.304 GAE/g DW in n-hexane extract (Table 4). The phenolic content of several plants has been shown to be affected by environmental factors and crop management ^[41,42]. Phenolics are antioxidant compounds in stressed plant tissues ^[43], especially those with nutritional deficiencies ^[44]. Previous research revealed that the concentration of phenolics varies in response to N and K fertilization. Nitrogen is the substrate for the biosynthesis of phenylalanine, whereas phenylalanine is the substrate for polyphenol chemicals ^[45]. In most cases, increasing the application of nitrogen reduces the polyphenol content of plants ^[46]. It is possible, due to conflict between the utilization of nitrogen for growth and the formation of secondary metabolites; if growth increases, polyphenolic compound production will decrease ^[42]. In general, the administration of N and K fertilizers has been regarded as a technique for enhancing the phenolic content of plant tissue ^[47]. Among the combination treatments of N and K fertilizers observed, the combination treatment of 46 kg/ha N and 12.5 kg/ha K fertilizers produced the highest TPC (aqueous extract, 3.688 mg GAE/g DW) compared to other treatments. These results indicated that N and K fertilizers strongly affected the growth and polyphenol metabolism of E. palmifolia. Similar results were recorded that boosting N and K fertilization increased the total phenolic content in sweet fennel ^[22] and colored potato ^[48]. Meanwhile, the TPC content obtained in E. palmifolia with aqueous extract was higher than using ethanol and n-hexane extracts. Our finding is in contras with Febrinda et al. ^[49], who discovered that TPC concentrations in ethanolic extract from traditional market at East Kalimantan, Indonesia was higher than those in aqueous extract. The results of this study are intriguing since the combination of N and K fertilizers can increase the phenolic content that is soluble in aqueous solvents of E. palmifolia. As a result, herbal medicine will be safer than utilizing ethanol or hexane extracts.

Table 4. Total phenolic content in aqueous, ethanol and n-hexane extract of E. palmifolia.

Treatments	Total phenolic content (mg GAE/g DW)
Treatments	Aqueous	Ethanol	n-Hexane
N₀K₂₅	2.694 ± 0.00^dA	2.740 ± 0.01^aA	0.253 ± 0.01^cB
N₁₀₀K₂₅	3.688 ± 0.00^aA	2.815 ± 0.00^aB	0.304 ± 0.01^aB
N₂₀₀K₂₅	2.192 ± 0.01^eA	2.192 ± 0.01^cA	0.288 ± 0.00^bB
N₁₀₀K₀	3.032 ± 0.00^cA	1.474 ± 0.00^dB	0.251 ± 0.01^cC
N₁₀₀K₅₀	2.624 ± 0.01^dA	2.381 ± 0.00^bB	0.286 ± 0.01^bC
N₁₀₀K₁₀₀	3.150 ± 0.01^bA	1.940 ± 0.00^bB	0.219 ± 0.00^dC
Note: Each value is expressed as the mean plus standard deviation; a–d, the Scott-Knott test indicates that the mean values in each column denoted by different letters differ significantly at p < 0.05; A–C, the Scott-Knott test indicates that the mean values in each row denoted by different letters differ significantly at p < 0.05; For the treatment combination, see Table 1.

| Show Table

DownLoad: CSV

TFC of E. palmifolia was measured using different extraction solvents, namely aqueous, ethanol, and n-hexane, as shown in Table 5. TFC in the E. palmifolia extracts depended on the combination of N and K fertilizer and the extraction solvents. Among the treatment of N and K fertilizer combinations investigated, TFC ranged from 0.398 to 0.529 QE/g DW in aqueous extract, 0.616 to 0.861 QE/g DW in ethanol extract, and 0.573 to 0.676 QE/g DW in n-hexane extract. These results indicated that the TFC in the combination of 46 kg/ha N and 12.5 kg/ha K has the highest TFC content. Lower content of TFC with aqueous extracts as a solvent was obtained after the application of the nitrogen-free with K of 25 kg/ha. The results showed that plants produced low amounts of flavonoid compounds without nitrogen fertilizer. Results indicate that nitrogen is an essential element in increasing plant metabolism. In solvent extraction, ethanol extract was presented higher TFC than aqueous and n-hexane extracts. Febrinda et al. ^[49] also found that TFC in the ethanolic extract were higher than aqueous extract. This study shows that the combination of 46 kg/ha N and 12.5 kg/ha K fertilizers has increased TFC in ethanol extract of E. palmifolia, which is likely to be a dose target for the production of flavonoid compounds.

Table 5. Total flavonoid content in aqueous, ethanol and n-hexane extract of E. palmifolia.

Treatments	Total flavonoid content (mg QE/g DW)
Treatments	Aqueous	Ethanol	n-Hexane
N₀K₂₅	0.398 ± 0.00^cC	0.779 ± 0.00^bA	0.651 ± 0.01^bB
N₁₀₀K₂₅	0.529 ± 0.01^aC	0.861 ± 0.02^aA	0.676 ± 0.01^aB
N₂₀₀K₂₅	0.409 ± 0.00^cC	0.842 ± 0.02^aA	0.573 ± 0.01^eB
N₁₀₀K₀	0.464 ± 0.01^bB	0.616 ± 0.01^dA	0.617 ± 0.00^dA
N₁₀₀K₅₀	0.448 ± 0.01^bC	0.711 ± 0.01^cA	0.629 ± 0.00^cB
N₁₀₀K₁₀₀	0.399 ± 0.01^cC	0.722 ± 0.00^cA	0.572 ± 0.01^eB
Note: Each value is expressed as the mean plus standard deviation; a–e, the Scott-Knott test indicates that the mean values in each column denoted by different letters differ significantly at p < 0.05; A–C, the Scott-Knott test indicates that the mean values in each row denoted by different letters differ significantly at p < 0.05; For the treatment combination, see Table 1.

| Show Table

DownLoad: CSV

3.3. Growth and yield of E. palmifolia

This study examined the antioxidant capacity of different extracts of E. palmifolia using DPPH and FRAP assays. Based on the antioxidant activities determined using the DPPH (Table 6) and FRAP (Table 7) methods, we concluded that FRAP method was better than DPPH in analyzing the anti-oxidant activities. This effect is caused by distinct reaction processes of antioxidant activity, including power reduction for FRAP and free radical scavenging for DPPH ^[29]. Antioxidant activity differed amongst a sample of E. palmifolia extracts, which can be explained by the varying forms or concentrations of antioxidant compounds contained inside ^[27,50]. The ability to absorb and neutralize free radicals, to quench single and triple oxidants, or to degrade peroxides is referred to as antioxidant activity ^[51]. Antioxidant-rich plants may be beneficial as possible therapeutic herbs. The plant's antioxidant properties can help scavenge free radicals. Polyphenol (flavonoid and phenolic) chemicals found in plants act as natural antioxidants, scavenging free radicals—the higher the flavonoids or total phenols content, the better the antioxidant ability. The antioxidant activity of E. bulbosa extracts demonstrated its unique nutritional value, indicating that it has the potential to serve as a natural source of antioxidants ^[8].

Table 6. DPPH radical scavenging activity in aqueous, ethanol, and n-hexane extracts of E. palmifolia.

Treatments	DPPH scavenging activity (µmol TE/g DW)
Treatments	Aqueous	Ethanol	n-Hexane
N₀K₂₅	0.232 ± 0.01^bA	0.221 ± 0.00^cC	0.143 ± 0.01^bA
N₁₀₀K₂₅	0.318 ± 0.01^aA	0.305 ± 0.01^aB	0.153 ± 0.01^aB
N₂₀₀K₂₅	0.339 ± 0.00^aA	0.253 ± 0.00^bC	0.105 ± 0.01^cB
N₁₀₀K₀	0.361 ± 0.01^aA	0.208 ± 0.00^dB	0.073 ± 0.01^dC
N₁₀₀K₅₀	0.215 ± 0.00^bA	0.219 ± 0.00^cA	0.097 ± 0.01^cB
N₁₀₀K₁₀₀	0.198 ± 0.01^bA	0.204 ± 0.00^dB	0.143 ± 0.01^bA
Note: Each value is expressed as the mean plus standard deviation; a–e, the Scott-Knott test indicates that the mean values in each column denoted by different letters differ significantly at p < 0.05; A–C, the Scott-Knott test indicates that the mean values in each row denoted by different letters differ significantly at p < 0.05; For the treatment combination, see Table 1.

| Show Table

DownLoad: CSV

DPPH radical scavenging activity of E. palmifolia extracts was affected by N and K fertilizer and solvent extraction, as presented in Table 6. Among the treatment of N and K fertilizer investigated, DPPH scavenging activity ranged from 0.097 to 0.361 µmol TE/g DW. The highest antioxidant scavenging activity (0.361 µmol TE/g DW) was recorded at 46 kg/ha N followed by 92 kg/ha N and 12.5 kg/ha K combined (0.339 µmol TE/g DW) and 46 kg/ha N and 12.5 kg/ha K combined (0.318 µmol TE/g DW). These results are in agreement with Barzegar et al. ^[22], who reported that applying N and K fertilizer affected increasing of DPPH scavenging activity in sweet fennel extract. Ma et al. ^[52] found that the N management increased antioxidant activity in wheat grain. Meanwhile, DPPH scavenging activity in different solvents extracted ranged from 0.198 to 0.361 µmol TE/g DW in aqueous extract, 0.204 to 0.305 µmol TE/g DW in ethanol extract, and 0.097 to 0.153 µmol TE/g DW in n-hexane extract. Results indicated that aqueous extracts of E. palmifolia were higher radical scavenging activity than ethanol and n-hexane extract. Attractive, these results are in line with the data of total phenolic content (Table 4). Antioxidant properties caused by phenolic compounds have been proven in a number of research in the last several decades ^[53,54,55].

Ferric reducing antioxidant power (FRAP) value of E. palmifolia extracts is showed in Table 7. FRAP value varied from 61.598 to 123.810 µmol TE/g DW among the treatments of N and K fertilizer studied. The highest reducing antioxidant power value was identified at 46 kg/ha N and 12.5 kg/ha K. These findings are in line with those of Michalska et al. ^[48], who found that the FRAP value of colored potato increased when N and K fertilizer was applied. Besides fertilization treatments, the solvent used to extract antioxidants can also have an effect on how effective they are. The FRAP values varied from 87.545 to 104.757 µmol TE/g DW in aqueous extract, 103.393 to 123.810 µmol TE/g DW in ethanol extract, and 61.598 to 72.151 µmol TE/g DW in n-hexane extract. Results indicated that ethanol extracts of E. palmifolia were higher in reducing antioxidant power activity than aqueous and n-hexane extracts. According to the total flavonoid content as presented in Table 5, these results are in line with them. These results indicated that flavonoid compounds are responsible for the antioxidant activity of E. palmifolia, especially through the mechanism of reducing antioxidant power. There has been a lot of research in the last few decades that shows that flavonoid compounds have antioxidant properties ^[56,57,58].

Table 7. Ferric reducing antioxidant power (FRAP) in aqueous, ethanol, and n-hexane extracts of E. palmifolia.

Treatments	Ferric reducing antioxidant power (µmol TE/g DW)
Treatments	Aqueous	Ethanol	n-Hexane
N₀K₂₅	91.030 ± 0.00^cB	122.219 ± 0.00^bA	64.242 ± 0.00^cC
N₁₀₀K₂₅	104.757 ± 0.00^aB	123.810 ± 0.01^aA	65.477 ± 0.00^bC
N₂₀₀K₂₅	101.992 ± 0.00^bB	114.621 ± 0.00^cA	72.151 ± 0.00^aC
N₁₀₀K₀	90.712 ± 0.00^cB	103.393 ± 0.00^fA	61.856 ± 0.00^dC
N₁₀₀K₅₀	88.401 ± 0.01^dB	106.166 ± 0.00^eA	65.174 ± 0.00^bC
N₁₀₀K₁₀₀	87.545 ± 0.00^dB	109.681 ± 0.00^dA	61.598 ± 0.00^dC
Note: Each value is expressed as the mean plus standard deviation; a–d, the Scott-Knott test indicates that the mean values in each column denoted by different letters differ significantly at p < 0.05; A–C, the Scott-Knott test indicates that the mean values in each row denoted by different letters differ significantly at p < 0.05; For the treatment combination, see Table 1.

| Show Table

DownLoad: CSV

4. Conclusions

The results of our study indicated that fertilization application of N and K improved chlorophyll reading value, total plant weight, and fresh bulb weight of E. palmifolia. In addition, the E. palmifolia treated with N and K exhibited higher total phenolic content, total flavonoid content, and antioxidant activity in aqueous and ethanol extracts. These results suggest that commercial application of N 46 kg/ha and K 12.5 kg/ha can be proposed to improve E. palmifolia growth, polyphenol content, and antioxidant activities.

Acknowledgments

This research was supported by the Faculty of Agriculture, the University of Bengkulu for research stations and facilities, the Faculty of Mathematics and Natural Sciences for Agricultural Biochemistry Division of Department Biochemistry, IPB University, for polyphenol and antioxidant determination.

Conflict of interest

The authors declare no conflict of interest. The funders had no role in the design of the study; in the collection, analyses, or interpretation of data; in the writing of the manuscript, or in the decision to publish the results.

References

[1]	M. Basan, S. Hui, H. Okano, Z. Zhang, Y. Shen, J. R. Williamson and T. Hwa, Overflow metabolism in Escherichia coli results from e_cient proteome allocation, Nature, 528 (2015) 99–104.
[2]	M. Bekker, S. de Vries, A. Ter Beek, K. J. Hellingwerf and M. J. T. de Mattos, Respiration of Escherichia coli Can Be Fully Uncoupled via the Nonelectrogenic Terminal Cytochrome bd-II Oxidase, J. Bacteriol., 191 (2009), 5510–5517.
[3]	Q. K. Beg, A. Vazquez, J. Ernst, M. A. de Menezes, Z. Bar-Joseph, A. L. Barabasi, and Z. N. Oltvai Intracellular crowding defines the mode and sequence of substrate uptake by Escherichia coli and constrains its metabolic activity, Proc. Natl. Acad. Sci. U S A, 104 (2007), 12663–12668.
[4]	K. J. Begg and W. D. Donachie, Cell shape and division in Escherichia coli: experiments with shape and division mutants, J. Bacteriol., 163 (1985), 615–622.
[5]	E. M. Corteselli, J. C. Burtis, A. K. Heinz and J. B. Yavitt, Leaf Litter Fuels Methanogenesis Throughout Decomposition in a Forested Peatland, Ecosystems, 20 (2017), 1217–1232.
[6]	J. P. DeLong, J. G. Okie, M. E. Moses, R. M. Sibly and J. H. Brown, Shifts in metabolic scaling, production, and e_ciency across major evolutionary transitions of life, Proc. Natl. Acad. Sci. U.S.A, 107 (2010), 12941–12945.
[7]	R. H. de Deken, The Crabtree E_ect: A Regulatory System in Yeast, Microbiology, 44 (1966), 149–156.
[8]	C. E. Deutch and G. S. Perera, Myceloid cell formation in Arthrobacter globiformis during osmotic stress, J. Appl. Bacteriol., 72 (1992), 493–499.
[9]	A. Esteve-Nunez, M. Rothermich, M. Sharma and D. Lovley, Growth of Geobacter sulfurreducens under nutrient-limiting conditions in continuous culture, Environ. Microbiol., 7 (2005), 641–648.
[10]	I. Famili, J. Forster, J. Nielson and B. O. Palsson, Saccharomyces cerevisiae phenotypes can be predicted by using constraint-based analysis of a genome-scale reconstructed metabolic network, Proc. Natl. Acad. Sci. U.S.A, 100 (2003), 13134–13139.
[11]	A. Flamholz, E. Noor, A. Bar-Even,W. Liebermeister and R. Milo, Tradeo_s in glycolytic strategy, Proc. Natl. Acad. Sci. U.S.A, 110 (2013), 10039–10044.
[12]	A. Frenkel and W. Hirsch, Spontaneous development of L forms of streptococci requiring secretions of other bacteria or sulphydryl compounds for normal growth, Nature, 191 (1961), 728–730.
[13]	T. Frick and S. Schuster, An example of the prisoner's dilemma in biochemistry, Naturwis-senschaften, 90 (2003), 327–331.
[14]	J. J. Germida and L. E. Casida Jr, Myceloid growth of Arthrobacter globiformis and other Arthrobacter species, J. Bacteriol., 144 (1980), 1152–1158.
[15]	G. Jayachandran, H. Gorisch and L. Adrian, Studies on hydrogenase activity and chlorobenzene respiration in Dehalococcoides sp. strain CBDB1, Arch. Microbiol., 182 (2004), 498–504.
[16]	I. Kareva, Prisoner's dilemma in cancer metabolism, PLoS One, 6 (2011), e28576.
[17]	C. P. Kempes, P. M. van Bodegom, D. Wolpert, E. Libby, J. Amend, and T. Hoehler, Drivers of Bacterial Maintenance and Minimal Energy Requirements, Front. Microbiol., 8 (2017), 31.
[18]	R. Lange and R. Hengge-Aronis, Growth phase-regulated expression of bolA and morphology of stationary-phase Escherichia coli cells are controlled by the novel sigma factor sigma S, J. Bacteriol., 173 (1991), 4474–4481.
[19]	R. E. Lenski and M. Travisano, Dynamics of adaptation and diversification: a 10,000-generation experiment with bacterial populations, Proc. Natl. Acad. Sci. U.S.A, 91 (1994), 6808–6814.
[20]	S. Y. Lunt and M. G. Vander Heiden, Aerobic Glycolysis: Meeting the Metabolic Requirements of Cell Proliferation, Annu. Rev. Cell Dev. Biol., 27 (2011), 441–464.
[21]	M. J. McInerney and M. P. Bryant, Anaerobic Degradation of Lactate by Syntrophic Associations of Methanosarcina barkeri and Desulfovibrio Species and Effect of H2 on Acetate Degradation, Appl. Environ. Microbiol., 41 (1981), 346–354.
[22]	D. Molenaar, R. van Berlo, D. de Ridder and B. Teusink, Shifts in growth strategies reflect tradeoffs in cellular economics, Mol. Syst. Biol., 5 (2009), 323.
[23]	P. M¨oller, X. Liu, S. Schuster and D. Boley, Linear programming model can explain respiration of fermentation products, PLoS ONE 13 (2018), e0191803.
[24]	N. Nanninga, Growth and form in microorganisms: morphogenesis of Escherichia coli, Can. J. Microbiol., 34 (1988), 381–389.
[25]	E. A. Newsholme, B. Crabtree and M. S. Ardawi The role of high rates of glycolysis and glutamine utilization in rapidly dividing cells, Biosc. Rep., 5 (1985), 393–400.
[26]	T. Pfei_er, S. Schuster and S. Bonhoe_er, Cooperation and competition in the evolution of ATPproducing pathways, Science, 292 (2001), 504–507.
[27]	L. Pine and C. J. Boone, Comparative cell wall analyses of morphological forms within the genus Actinomyces, J. Bacteriol., 94 (1967), 875–883.
[28]	N. D. Price, J. L. Reed and B. O. Palsson, Genome-scale models of microbial cells: evaluating the consequences of constraints, Nat. Rev. Microbiol., 2 (2004), 886–897.
[29]	C. Risso, J. Sun, K. Zhuang, R. Mahadevan, R. DeBoy, W. Ismail, S. Shrivastava, H. Huot, S. Kothari, S. Daugherty, O. Bui, C. H. Schilling, D. R. Lovley and B. A. Methe, Genome-scale comparison and constraint-based metabolic reconstruction of the facultative anaerobic Fe(III)-reducer Rhodoferax ferrireducens, BMC Genomics, 10 (2009), 447.
[30]	D. H. Rothman, G. P. Fournier, K. L. French, E. J. Alm, E. A. Boyle, C. Cao and R. E. Summons, Methanogenic burst in the end-Permian carbon cycle, Proc. Natl. Acad. Sci. U.S.A, 111 (2014), 5462–5467.
[31]	K. L. Ruoff, Nutritionally variant streptococci, Clin. Microbiol. Rev., 4 (1991), 184–190.
[32]	E. Ruppin, J. A. Papin, L. F. de Figueiredo and S. Schuster, Metabolic reconstruction, constraintbased analysis and game theory to probe genome-scale metabolic networks, Curr. Opin. Biotech., 21 (2010), 502–510.
[33]	M. Schaechter, O. Maaloe and N. O. Kjeldgaard, Dependency on medium and temperature of cell size and chemical composition during balanced growth of Salmonella typhimurium, J. Gen. Microbiol., 19 (1958), 592–606.
[34]	S. Schuster, D. Boley, P. Moller, H. Stark and C. Kaleta, Mathematical models for explaining the Warburg e_ect: a review focussed on ATP and biomass production, Biochem. Soc. Trans., 43 (2015), 1187–1194.
[35]	T. E. Shehata and A. G Marr, E_ect of Nutrient Concentration on the Growth of Escherichia coli, J. Bacteriol., 107 (1971), 210–216.
[36]	H. Shim and S. T. Yang, Biodegradation of benzene, toluene, ethylbenzene, and o-xylene by a coculture of Pseudomonas putida and Pseudomonas fluorescens immobilized in a fibrous-bed bioreactor, J. Biotechnol., 67 (1999), 99–112.
[37]	T. Shlomi, T. Benyamini, E. Gottlieb, R. Sharan and E. Ruppin, Genome-scale metabolic modeling elucidates the role of proliferative adaptation in causing the Warburg effect, PLoS Comput. Biol., 7 (2011), e1002018.
[38]	R. E. Steinberger, A. R. Allen, H. G. Hansma and P. A. Holden, Elongation correlates with nutrient deprivation in Pseudomonas aeruginosa unsaturated biofilms, Microb. Ecol., 43 (2002), 416–423.
[39]	A. Swain and S. Chatterjee, A new formulation for determination of the competition coeffcient in multispecies interaction for Lotka-Volterra type competition models, Curr. Sci., 112 (2017), 1920–1926.
[40]	C. P. Tseng, J. Albrecht and R. P. Gunsalus, Effect of microaerophilic cell growth conditions on expression of the aerobic (cyoABCDE and cydAB) and anaerobic (narGHJI, frdABCD, and dmsABC) respiratory pathway genes in Escherichia coli, J. Bacteriol., 178 (1996), 1094–1098.
[41]	M. G. Vander Heiden, L. C. Cantley and C. B. Thompson, Understanding the Warburg effect: the metabolic requirements of cell proliferation, Science, 324 (2009), 1029–1033.
[42]	A. Vazquez, J. Liu, Y. Zhou and Z. N. Oltvai Catabolic effciency of aerobic glycolysis: The Warburg effect revisited, BMC Syst. Biol., 4 (2010), 58.
[43]	G. N. Vemuri, E. Altman, D. P. Sangurdekar, A. B. Khodursky and M. A. Eiteman, Overflow metabolism in Escherichia coli during steady-state growth: transcriptional regulation and effect of the redox ratio, Appl. Environ. Microbiol., 72 (2006), 3653–3661.
[44]	D. Voet and J. G. Voet, Biochemistry, 3^rd edition, Wiley and Sons, Hoboken, 2004.
[45]	O. Warburg, Origin of cancer cells, Science, 123 (1956), 309–314.
[46]	M. Webb, The influence of magnesium on cell division. I. The growth of Clostridium welchii in complex media deficient in magnesium, J. Gen. Microbiol., 2 (1948), 275–287.
[47]	M. Webb, E_ects of magnesium on cellular division in bacteria, Science, 118 (1953), 607–611.
[48]	K. D. Young, The selective value of bacterial shape, Microbiol. Mol. Biol. Rev., 70 (2006), 660–703.
[49]	K. Zhuang, G. N. Vemuri and R. Mahadevan, Economics of membrane occupancy and respirofermentation, Mol. Syst. Biol., 7 (2011), 500.

This article has been cited by:

1.	E. Bimenyindavyi, L. Z. Khusnetdinova, O. A. Timofeeva, Phenolic Compounds of Plants Bidens tripartita (L.) and Bidens pilosa (L.) from Different Locations, 2023, 70, 1021-4437, 10.1134/S1021443723700243
2.	E. Bimenyindavyi, L. Z. Khusnetdinova, O. A. Timofeeva, Phenolic Compounds of Plants Bidens tripartita (L.) and Bidens pilosa (L.) from Different Locations, 2023, 70, 0015-3303, 765, 10.31857/S001533032360081X
3.	Nevena Opačić, Sanja Radman, Mia Dujmović, Sanja Fabek Uher, Božidar Benko, Nina Toth, Marko Petek, Lepomir Čoga, Sandra Voća, Jana Šic Žlabur, Boosting nutritional quality of Urtica dioica L. to resist climate change, 2024, 15, 1664-462X, 10.3389/fpls.2024.1331327

Reader Comments

Your name:*

Email:*
© 2019 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)