The Ecology and Evolutionary Biology of Cancer: A Review of Mathematical Models of Necrosis and Tumor Cell Diversity

1.   Introduction

Pavement infrastructure today is more vulnerable to the failure. Several types of failures occurred in the asphalt pavement among rutting is the most common. The rate of rutting occurrence in the asphalt pavement depends on external and internal factors. External factors include load and volume of the traffic, tire pressure, temperature and construction practices. Internal factors include thickness of pavement, bitumen, agammaegate and mixture properties ^[1]. In the recent decades countries more vulnerable to the climate change facing primary challenge in the road failure is the rutting. Rutting is the most common type of asphalt distress observed in china and Japan ^[2]. Performance of 42 highways in the USA were observed in 14 states the result shows rutting occurred in the top layers of the asphalt pavement of depth 7.6 to 10.2 cm ^[3]. Rutting causing factors are categorized into three groups: Asphaltic material properties; quality of the construction work, climate and traffic volume ^[2]. Study of Golalipour et al. ^[4] claimed that agammaegate characteristics are the most common parameter caused rutting followed by the bitumen. Study postulated nine possible factors of the rutting where primary reason is the materials followed by construction quality and temperature susceptibility.

Material mixing and gradation is found the most common type of reason caused rutting or in other words rutting highly depended on the materials and quality ^[5]. Mostly rutting occurred on the top layers where materials of the layers has either to be modified up to the standards or reduce the traffic volume or control the traffic volume or some suggested to increase the depth of the super pavement layers in the result cost of the project will increased. Thus asphaltic materials modification is usually practiced to analyze the properties and observed the improvement in the road pavement. Locally available cost effective additives or modifiers such as polymers, Bakelite, fibers and rubber have proved to the most significant additives. The asphalt pavement modification over polymer has potential to reduce the rutting effect as well increase the stability ^[6,7]. The polymer addition usually results in higher degree of stiffness in asphalt accompanied with enhancement in temperature and moisture susceptibility which results in increased rutting resistance. Polymers are also used as a coating material for agammaegates where they increase surface roughness and also make agammaegates moisture resistant. Polymer classified into elastomer and plastomer where Bakelite is classified as plastomer. Plastomers decrease the elasticity of bitumen and low temperature flexibility is decreased but strength is increased at higher temperatures due to increase in stiffness and decrease in penetration ^[8]. Likewise any other polymers rubbers are also used as modifiers such as SBS (Styrene Butadiene Styrene), SBR (Styrene Butadiene Rubber) and CRM (Crumb Rubber Modifier). Utilization of crumb rubber for modification of bitumen binder is considered to be the smart solution for sustainable and economic development.

Crumb rubber modifier (CRM) is believed to be a good alternative polymer for improving binder performance properties in HMA ^[7]. Physical and rheological properties of CRM modified bitumen was studied by Mashan et al. ^[9] and found that the addition of crumb rubber has an effect on the physical properties of rubberized bitumen binders, by increasing its elastic recovery and decreasing its penetration and ductility. The rubberized bitumen binder with higher crumb rubber content has an obvious effect on the rheological properties (increase in complex shear modulus G, storage modulus G', loss modulus G'' and decrease in phase angle I).

The results indicated that the rubber content has the potential to resist rutting deformation that occurs in road pavement as result of increased traffic loading. According to the Mashaan et al. ^[10], shin et al. ^[11], CRM modified bitumen has more resistance against rutting. Crumb rubber modified asphalt has analyzed by Palit et el. ^[12] for the permanent deformation in the pavement, temperature and permeability where study found improved result for the fatigue and higher temperature susceptibility and less permeability compare to unmodified asphalt. Compaction temperature of hot asphalt mix has been reduced by 15 ℃ using crumb rubber in the dry process ^[13]. Considerable studies have conducted on the crumb rubber modified asphalt the basic gap is the bitumen grading and agammaegate material which paly major role in the asphalt mix. Review studies shows mostly granite agammaegate is used and bitumen grade 80/100. In the Pakistan content where lime stone is commonly use in the flexible pavement and grade 60/70 bitumen so this study is trying to bridge the gap. Thus concluded modification of bitumen with crumb rubber improves the physical, mechanical and thermal properties of the asphalt.

Irrespective to the crumb rubber another modifier is Bakelite chemically known (poly-oxy-benzyl-ethylene-glycol-anhydride) is a thermosetting resin of phenol formaldehyde due to non-conductivity and good heat resistant characteristics i.e., thermosetting property, it is widely used in manufacturing cases of electrical appliances such as radio, irons, switches etc. ^[14]. Bakelite waste products in the powder form can be alternatively used in the asphalt pavement. Bitumen modified with 2% Bakelite found increased complex modulus, decreased phase angle, and improved rutting resistance and Marshall Stability has significantly increased ^[15]. Study of Cubuk et al. ^[16] shows addition of 2% Bakelite with bitumen enhance the Marshall stability, reduce rutting, bleeding, stripping and cracking effect in the asphalt pavement. Based on the past studies utilization of crumb rubber and Bakelite has potential to improve the asphalt physical and mechanical properties and helpful in reducing the rutting failure of the asphalt pavement in the result this study has set following objectives. To perform laboratory test to evaluate the compatibility of Bakelite and Crumb Rubber as an additive in the asphalt pavement; to investigate the performance comparison of control and modified HMA mixes for mechanical Properties and to compare the mechanical properties of Bakelite and crumb rubber.

2.   Materials and methods

In this study asphalt mixture of AC-20 extensively used in Pakistan National highway authority in the major highways and road construction were selected. Bitumen penetration grade 60/70 were used in this study; penetration, softening and flash fire test were conducted. Course Agammaegate most commonly Lime stone were collected from the Margalla (Pakistan, Islamabad) Query. Agammaegate were tested in the national logistic cooperation (NLC) concrete laboratory and Table 1 shows the agammaegate properties. Crumb Rubber (Waste crumb rubber shredded was collected from the local waste tyre industry at Taxila, Pakistan as shown in the Figure 1. Bakelite Powder Bakelite was collected from the waste industry in Taxila, Pakistan to use in this study as shown in the Figure 2.

Table 1.  Asphalt mixture composition and gradation.

Gradation sieve size (mm)	Sieve size (inches)	Lab trial	NHA Class A specification
25	1	100	100
19	3/4	95	90–100
12.5	1/2	67	-
9.5	3/8	60	56–70
4.75	#4	44	35–50
2.36	#8	30	23–35
0.30	#50	9	5–12
0.075	#200	5.6	03–08

---

 | Show Table

DownLoad: CSV

Figure 1.  Waste crumb rubber.

DownLoad: Full-Size Img PowerPoint

Figure 2.  Powder Bakelite.

DownLoad: Full-Size Img PowerPoint

In the pavement failures agammaegate plays a major role where it is essential to carried out necessary agammaegate test before preparing Marshall Mixture. Agammaegate properties test were conducted and outcome is shown in Table 2. All tests conducting using international standards and findings result meet the range of international standards recommended practices in engineering.

Table 2.  Properties of aggregate.

Properties	Test standards	Result
Bulk specific gravity of course aggregates	AASHTO T 85–91 ASTM C 127–84	2.62
Water absorption of coarse aggregates	AASHTO T 85–91 ASTM C 127–84	0.27 %
Bulk specific gravity of Fine aggregates	AASHTO T 84–93 ASTM C 128–84	2.64
Water absorption of fine aggregates	AASHTO T 84–93 ASTM C 128–84	2.49 %
Loss angele abrasion test method A, B, C	AASHTO T 96–92 ASTM C 131–81	22.6 % 22.3 % 21.01 %
Aggregate impact value test (30%max)	BS 812	15.80 %

---

 | Show Table

DownLoad: CSV

Bitumen properties were studied and finding is listed in the Table 3; Bitumen penetration grade 60/70 was used in this study. Dry process of mixing were used to perform Marshall stability

Table 3.  Bitumen properties.

Test type	Sample	Test standard	Test results	Specifications
Penetration test	1	AASHTO T49–93 ASTM D5	64	60–70
	2		67
Softening test	1	AASHTO T53–92 ASTM 36–89	49.7	46–56
	2		53
Flash & fire	1	AASHTO T48 ASTM D92	228 and 262	> 232 ℃ and > 246
	2			> 246

---

 | Show Table

DownLoad: CSV

3.   Result and analysis

3.1.   Optimum bitumen content (OBC)

For AC-20 optimum bitumen content (OBC) was determined using trial method of bitumen contents of 4.5%, 5.0%, 5.5% and 6.0 % accordingly in the Marshall mix design. Finding indicated that at 5% of bitumen content has the highest Marshall stability result which meet with the standard of National highway and international standards. In order to find optimum bitumen content (OBC), density, Marshall stability, air voids, flow and stiffness of the Marshall mix AC-20 were determined as shown in the Table 4.

Table 4.  Optimum Bitumen content.

Bitumen contents (%)	Density (kg/cm3)	Stability (N)	Air voids (%)	Flow (mm)	Stiffness (N/mm)
4.5	2.24	7227.6	9.39	2.540	2845.51
5.0	2.35	9554.0	5.24	2.89	3306.61
5.5	2.39	15530.0	3.51	3.56	4362.35
6.0	2.37	12491.5	2.76	4.07	3069.16

---

 | Show Table

DownLoad: CSV

The study has carried into two stages firstly crumb rubber percentage of 4%, 8%, 12%, 16% and 20% were added in the Marshall mix. In the second stage, Bakelite powder proportion of 4%, 8%, 12%, 16% and 20% were added to study the performance of Marshall mixed.

3.2.   Mechanical properties of crumb rubber modified asphalt

It is observed that by addition of crumb rubber the Marshall stability increased, the optimum crumb rubber is 12% shows the highest Marshall stability of 22516 N followed by the highest stiffness of 5248 N/mm. The density of crumb rubber modified asphalt increased as shown in the Table 5 similar result were claimed by addition of polymers or crumb rubber increased the density of asphalt in the past studies. Air voids contents decrease by the addition of crumb rubber at 4% addition shows the highest air voids of 5.60% compare to 20% addition of crumb rubber the air voids contents recorded 1.90% which indicated significant reduced of the air voids contents. Marshall Flow's values indicated the flexibility of the Marshall sample higher flow indicated more flexible pavement as the result documented shows flow values increase by the addition of crumb rubber.

Table 5.  Mechanical properties of crumb rubber modified asphalt.

Crumb rubber (%)	Density (kg/cm3)	Stability (N)	Air voids (%)	Flow (mm)	Stiffness (N/mm)
4.0%	2.19	15137.1	5.60	3.70	4124.55
8.0%	2.21	16599.7	4.20	3.80	4345.24
12.0%	2.24	22516.4	3.12	4.40	4648.5
16.0%	2.26	21889.4	2.53	5.10	3907.54
20.0%	2.25	19340.7	1.90	5.90	3582.1

---

 | Show Table

DownLoad: CSV

3.3.   Mechanical properties of bakelite modified asphalt

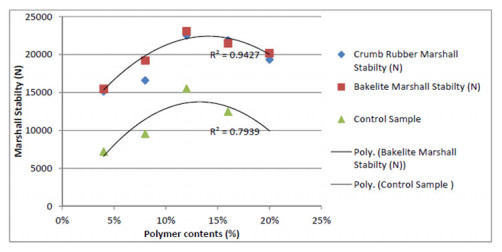
Contrary to the crumb rubber addition of the waste Bakelite polymer was added with 4-8-12-16 and 20% in the asphalt mixture. Table 6 shows the mechanical properties of Marshall Mix of the Bakelite polymer powder. The trend of density is increased by increasing the contents in the asphalt mixture up to 16% addition and further addition reduces the density. Maximum density 2.42 kg/cm³ of the asphalt mixture achieved at ratio of 16% addition. The Marshall stability which is one of the main parameter of the flexible asphalt shows compare to control sample the stability increased by almost double as graphically represented in Figure 3 showing the comparison of Marshall Stability between modifiers and control sample. Likewise the stability of the asphalt concrete increased the air voids decrease by addition of modifiers decreased lesser the air voids higher is the performance of the asphalt concrete. Flow indicated that flexibility of the asphaltic concrete higher flow indicated more flexible asphalt pavement. Addition of Bakelite polymers shows the higher flow in the asphalt concrete. Against the rutting affect the stiffness properties of Marshall Show's higher stiffness by the addition of Bakelite

Table 6.  Mechanical properties of bakelite polymer modified asphalt.

Bakelite powder (%)	Density (kg/cm3)	Stability (N)	Air voids (%)	Flow (mm)	Stiffness (N/mm)
4.0%	2.19	15483.3	5.10	3.8	3656.8
8.0%	2.21	19215.5	4.75	4.60	4160.03
12.0%	2.24	23083.4	3.60	5.10	4895.2
16.0%	2.27	21468.7	2.43	5.40	3997.8
20.0%	2.23	20182.5	1.46	5.95	3287.1

---

 | Show Table

DownLoad: CSV

Figure 3.  Marshall stability (N) comparison of modifier (crumb rubber and Bakelite) and control sample.

DownLoad: Full-Size Img PowerPoint

4.   Comparison

Addition of waste polymer modifier of wasted crumb rubber and Bakelite by percentage of 4%, 8%, 12%, 16% and 20% in the asphalt concrete separately tested for the Marshall Properties and comparison result has made. Coefficient of determination "R²" value has determined with trend line to understand the strength of two variable strength verse polymer addition the average R² for Bakelite and crumb rubber R² = 0.9427 highly significant. Where R² = 0.7939 for the control sample shows less significant compere to polymer. Marshall Stability compares to control showing almost double and optimum achieved by addition of 12% as shown in the Figure 3.

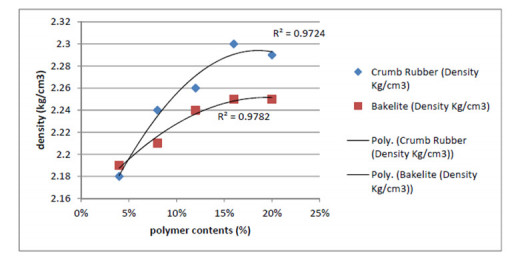
Density: Figure 4 indicated crumb rubber shows higher density of the asphalt mixture and the optimum density of the asphalt concrete observed at 16% addition of crumb rubber. Past studies trend of density by addition of modifier in the asphalt shows likewise result addition of modifier or additives polymer to the asphalt mixture will increase the density which is positive sign of improvement in the asphalt concrete. Addition of crumb rubber shows highly significant as the coefficient of determination R² = 0.9724 followed by R² = 0.9529. In the both cases it found density significantly increased.

Figure 4.  Crumb rubber and Bakelite modified asphalt density.

DownLoad: Full-Size Img PowerPoint

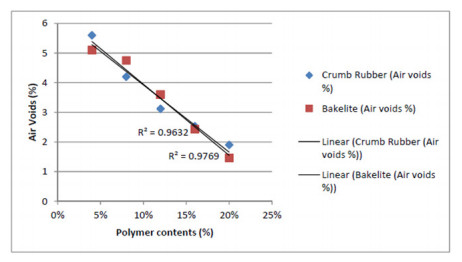
Air voids contents: Generally, air voids total mix decrease by increasing bitumen contents in the mix. International recommended standard of air voids content is 3–6%. Air voids contents trend followed literature shows decreasing by addition of the modifier contents in the asphalt mixture as shown in the Figure 5. Bakelite which is used in the powder form shows lower air voids content in the asphalt mixture. The lesser is the air voids content in the asphalt mixture has more durability and longer life against the water. Optimum air voids content has achieved by addition by 16%. Coefficient of determination R² = 0.9769 Bakelite and R² = 0.9632 crumb rubber shows strongly significant.

Figure 5.  Air voids contents in the modified asphalt mixture.

DownLoad: Full-Size Img PowerPoint

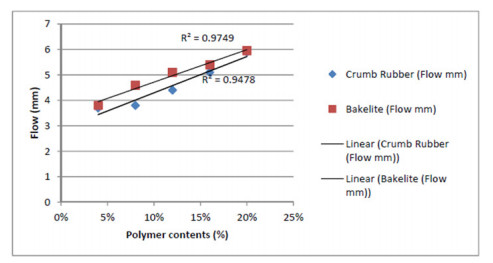
Flow: The property of the Marshall flow indicated the flexibility of the asphalt mixture. The minimum 2 mm and maximum 5 mm is allowable flow in the asphalt mixture according to the international standards. Findings shown in the Figure 6 indicated the flow values are in the standard range. Modifier comparison shows Bakelite has higher flow compare to the crumb rubber but generally result is in the range values. Coefficient of determination which indicate the strength of variables indicated that R² = 0.9749 Bakelite and R² = 0.9448 crumb rubber shows strongly significant relations.

Figure 6.  Flow of the Modified asphalt mixture.

DownLoad: Full-Size Img PowerPoint

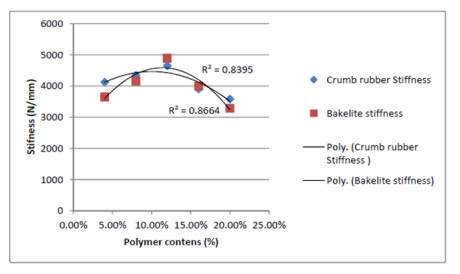
Stiffness: Asphalt mixture stiffness indicated the resistance against the permanent deformation and rutting failure. Higher stiffness of the asphalt mixture shows the asphalt mixture can resist against the heavy traffic load. Figure 7 shows the addition of modifier crumb rubber and Bakelite enhance stiffness of the asphalt mixture both modifier shows positive result. Optimum 4648 N/mm stiffness recorded by addition of 12% crumb rubber and 4895 N/mm stiffness by addition of 12 % Bakelite. Bakelite and crumb rubber enhance the stiffness of the asphalt mixture and improve the flexibility as well increase the resistance against permanent deformation failure. Coefficient of determination R² = 0.8664 Bakelite and R² = 0.8395 crumb rubber shows highly significant relations of polymer addition and stiffness strength enhancement.

Figure 7.  Stiffness of the modified asphalt mixture.

DownLoad: Full-Size Img PowerPoint

5.   Discussion

Mechanical properties of hot mixed asphalt modified over crumb rubber and Bakelite has been studies in this research paper. Experimental study has conducted to modify the asphalt mix by using 5-10-15 and 20% of crumb rubber and Bakelite waste polymers and the result has compared. Bitumen grade 60/70 and course agammaegate Lime stone ACW-20 were used. Material tests for bitumen, penetration, softening, flash and fire and for agammaegates specific gravity, water absorption, loss Angele abrasion, agammaegated impact value and fine agammaegate has been tested according to the engineering standards and practices. Optimum binder/bitumen content (OBC) has determined by using trial method 4.5%, 5.0%, 5.5% and 6.0% bitumen were added and maximum mechanical properties and Marshall Stability has determined using 5.5%. Further using OBC (5.5% bitumen contents) waste polymer of crumb rubber 4-8-12-16 and 20% were added in the Marshall mix to observe the mechanical properties.

Likewise method has adopted for the Bakelite addition of 4-8-12-16 and 20% addition to the Marshall mixture. The findings has tabulated as shown in the Tables 5 and 6. Each mechanical property has discussed and graphically represented in the comparison Figures 3–7. Figure 3 shows the Marshall stability comparison among control sample and modified hot mix asphalt using crumb rubber and Bakelite. Addition of polymer shows significant improvement where R² = 0.9427 compare to control sample R² = 0.7939. Maximum Marshall stability has achieved by the addition of polymer of 12%. The findings indicated waste polymer of crumb rubber and Bakelite has potential to increase the Marshall stability. Density has notably increased by the addition of crumb rubber and Bakelite R² = 0.9724 followed by R² = 0.9529. Past studies on the polymer addition in the hot asphalt mix shows the similar trend of higher density.

The voids in the asphalt mixture will allow the water flow and indicated high permeability where high permeability for the asphalt mixtures is consider dangerous and leads to the various failure causes. The findings indicated air voids has significantly reduced by the addition of polymer, Bakelite which is used in the powder form shows lower air voids content in the asphalt mixture. The lesser is the air voids content in the asphalt mixture has more durability and longer life against the water. Optimum air voids content has achieved by addition by 16%. The property of the Marshall flow indicated the flexibility of the asphalt mixture. The minimum 2 mm and maximum 5 mm is allowable flow in the asphalt mixture according to the international standards. Findings shown in the Figure 6 indicated the flow values are in the standard range. Modifier comparison shows Bakelite has higher flow compare to the crumb rubber but generally result is in the range values. Asphalt mixture stiffness indicated the resistance against the permanent deformation and rutting failure. Higher stiffness of the asphalt mixture shows the asphalt mixture can resist against the heavy traffic load. Figure 7 shows the addition of modifier crumb rubber and Bakelite enhance stiffness of the asphalt mixture both modifier shows positive result. Optimum 4648 N/mm stiffness recorded by addition of 12% crumb rubber and 4895 N/mm stiffness by addition of 12% Bakelite. Bakelite and crumb rubber enhance the stiffness of the asphalt mixture and improve the flexibility as well increase the resistance against permanent deformation failure.

6.   Conclusion

Waste crumb rubber and Bakelite (phenol formaldehyde) polymer utilization in the asphalt pavement has the potential to enhance the mechanical properties of the hot mixed asphalt. Crumb rubber and Bakelite waste materials were added into the asphalt with the ratio of 4.0%, 8.0%, 12.0%, 16.0% and 20.0%. Optimum bitumen content 5.5% with penetration grade 60/70 and AC-20 agammaegate was selected to study the mechanical properties of hot mix asphalt. Chemical composition of crumb rubber and Bakelite is complex and different so the finding shows the result of addition in the asphalt has comparative different result. Bakelite compare to crumb rubber shows more better enhancement in term of higher Marshall stability and stiffness by addition of 12.0% further addition tends to reduce the Marshall stability and stiffness.

-Marshall stability of hot asphalt mixture significantly increased by the addition of 12% waste crumb rubber and Bakelite;

-Density of the asphalt mixture increased;

-Air voids content decreased will leads significantly improve the permeability of hot mixed asphalt to reduce the cause of failure;

-Flow of the hot mixed asphalt increased;

-Stiffness of the hot mixed asphalt increased.

Higher strength of Marshall and stiffness shows better resistance against permanent deformation especially rutting. This study also focuses on the air voids content trend shows addition of crumb rubber and Bakelite reduce the air voids content. Density of the asphalt mixture shows increased. Flow of the asphalt mixture by addition of crumb rubber and Bakelite is in the control range of 2–6 mm. Crumb rubber and Bakelite has potential to enhance the mechanical properties of the asphalt mixture and can use by 12% addition to the asphalt mixture will have better result. Bakelite shows better result compare to the crumb rubber so future studies is recommended to further enlighten with replacement of bitumen or fine agammaegate material and more studies required for the wheel tracking test, viscosity, temperature susceptibility with different penetration grade bitumen as well different ratios of mixing waste materials.

Acknowledgements

The authors extend gratitude and gratefully acknowledge the financial support of Yayasan Chanselor Uniten (YCU) project code 201901001YUC/11.

Conflict of interests

No conflict of interest has been identified.