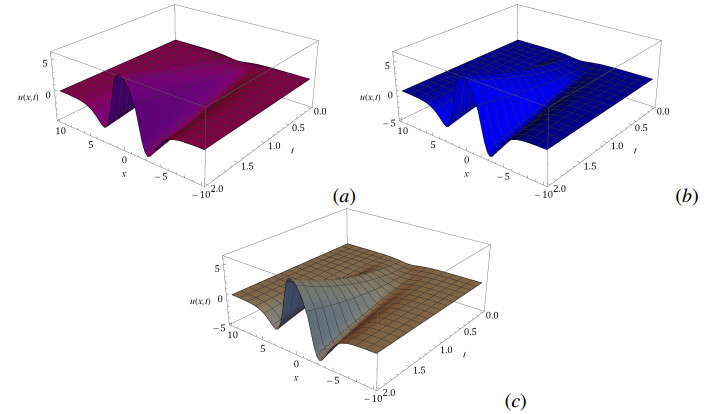
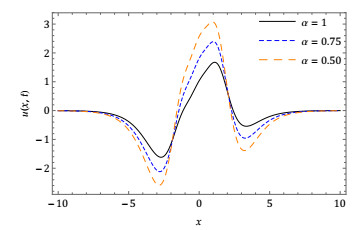
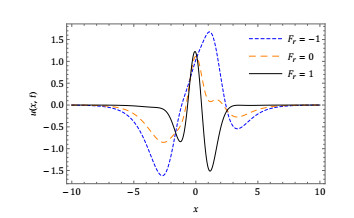
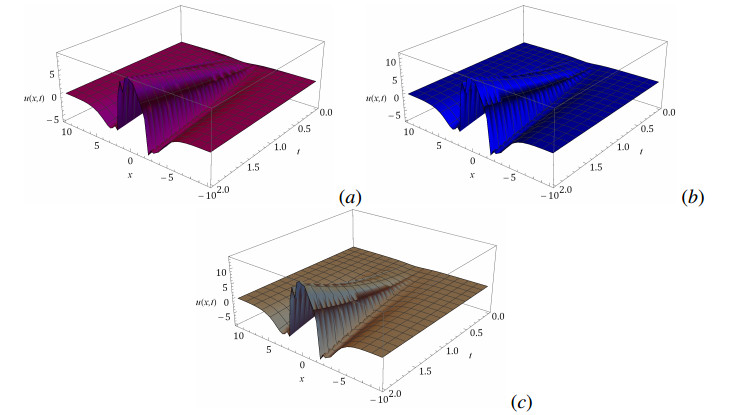
The fractional natural decomposition method (FNDM) is employed in the present investigation to find the solution for fractional forced Korteweg-de Vries (FF-KdV) equation. Three distinct cases are chosen for each equation to validate and illustrate the effectiveness of the future technique. The behaviour for different values of Froude number (Fr) has been presented to assure the proficiency and reliability and of the considered method. Moreover, we captured the behaviour of the FNDM solution for distinct arbitrary order. The obtained results elucidate that, the considered method is very effective and easy to employ while analyse the behaviour of nonlinear fractional differential equations arising in connected areas of science and technology.
1.
Introduction and main results
Let T be a linear operator. Given a function a, the commutator [T,a] is defined by
There is an increasing interest to the study of T being a pseudo-differential operator because of its theory plays an important role in many aspects of harmonic analysis and it has had quite a success in linear setting. As one of the most meaningful branches, the study of bilinear pseudo-differential operators was motivated not only as generalizations of the theory of linear ones but also its natural appearance and important applications. This topic is continuously attracting many researchers.
Let a be a Lipschitz function and 1<p<∞. The estimates of the form
have been studied extensively. In particular, Calderón proved that (1.1) holds when T is a pseudo-differential operator whose kernel is homogeneous of degree of −n−1 in [7]. Coifman and Meyer showed (1.1) when T=Tσ and σ is a symbol in the Hörmander class S11,0 go back to [10,11], this result was later extended by Auscher and Taylor in [4] to σ∈BS11,1, where the class BS11,1, which contains S11,0 modulo symbols associated to smoothing operators, consists of symbols whose Fourier transforms in the first n-dimensional variable are appropriately compactly supported. The method in the proofs of [10,11] was mainly showed that, for each Lipschitz continuous functions a on Rn, [T,a] is a Calderón-Zygmund singular integral whose kernel constants are controlled by ‖a‖Lip1. For another thing, Auscher and Taylor proved (1.1) in two different ways: one method is based on the paraproducts while the other is based on the Calderón-Zygmund singular integral operator approach that relies on the T(1) theorem. Fore a more systematic study of these (and even more general) spaces, we refer the readers to see [38,39].
Given a bilinear operator T and a function a, the following two kinds commutators are respectively defined by
and
In 2014, Bényi and Oh proved that (1.1) is also valid to this bilinear setting in [6]. More precisely, given a bilinear pseudo-differential operator Tσ with σ in the bilinear Hörmander class BS11,0 and a Lipschitz function a on Rn, it was proved in [6] that [T,a]1 and [T,a]2 are bilinear Calderón-Zygmund operators. The main aim of this paper is to study (1.1) of [Tσ,a]j(j=1,2) on the products of weighted Lebesgue spaces and variable exponent Lebesgue spaces with σ∈BBS11,1. Before stating our main results, we need to recall some definitions and notations. We say that a function a defined on Rn is Lipschitz continuous if
Let δ≥0, ρ>0 and m∈R. An infinitely differentiable function σ:Rn×Rn×Rn→C belongs to the bilinear Hörmander class BSmρ,δ if for all multi-indices α,β,γ∈Nn0 there exists a positive constant Cα,β,γ such that
Given a σ(x,ξ,η)∈BSmρ,δ, the bilinear pseudo-differential operator associated to σ is defined by
In 1980, Meyer [34] firstly introduced the linear BSm1,1, and corresponding boundedness of [Tσ,a]j(j=1,2) is obtained by Bényi-Oh in [6], that is, given m∈R and r>0, an infinitely differentiable function σ:Rn×Rn×Rn→C belongs to BrBSm1.1 if
where ˆσ1 denotes the Fourier transform of σ with respect to its first variable in Rn, that is, ˆσ1(τ,ξ,η)=^σ(⋅,ξ,η)(τ), for all τ,ξ,η∈Rn. The class BBSm1,1 is defined as
Recently, many authors are interested in bilinear operators, which is a natural generalization of linear case. With the further research, Árpád Bényi and Virginia Naibo proved that boundedness for the commutators of bilinear pseudo-differential operators and Lipschitz functions with σ∈BBS11,1 on the Lebesgue spaces in [5]. In 2018, Tao and Li proved that the boundedness of the commutators of bilinear pseudo-differential operators was also true on the classical and generalized Morrey spaces in [40]. Motivated by the results mentioned above, a natural and interesting problem is to consider whether or not (1.1) is true on the weighted Lebesgue spaces and variable exponent Lebesgue spaces with σ∈BBS11,1. The purpose of this paper is to give an surely answer. And also, the endpoint estimate is obtained on L∞×L∞. Our proofs are based on the pointwise estimates of the sharp maximal function proved in the next section.
Many results involving bilinear pseudo-differential operators theory have been obtained in parallel with the linear ones but some new interesting phenomena have also been observed. One aspect developed rapidly is the one related to the compactness of the bilinear pseudo-differential operators, especially, the properties of compactness for the commutators of bilinear pseudo-differential operators and Lipschitz functions. As the commutators [Tσ,a]j (j=1,2) are bilinear Calderón-Zygmund operators if σ∈BBS11,1, similar to the proof of [15] (Theorem A and Theorem 2.12), we can obtain easily that [Tσ,a]j and [[Tσ,a]j,b]i (i,j=1,2) are compact operators on the Lebesgue spaces and the Morrey spaces. For the sake of convenience, there are no further details below.
Suppose that σ∈BBS11,1. Let K and Kj denote the kernel of Tσ and [Tσ,a]j(j=1,2), respectively. We have
Then the following consequences are true.
Theorem A. [6] If x≠y or x≠z, then we have
(1) ∣∂αx∂βy∂γzK(x,y,z)∣≲(|x−y|+|x−z|)−2n−1−|α|−|β|−|γ|;
(2) |Kj(x,y,z)|≲‖a‖Lip1(|x−y|+|x−z|+|y−z|)−2n.
The statement of our main theorems will be presented in follows.
Theroem 1.1. Let q′>1, σ∈BBS11,1 and a be a Lipschitz function on Rn. Suppose for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1. If 0<δ<1/2, then
for all f,g of bounded measurable functions with compact support.
Theorem 1.2. Let q′>1, σ∈BBS11,1 and a be a Lipschitz function on Rn. Suppose for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1. If b∈BMO, 0<δ<1/2, δ<ε<∞, q′<s<∞, then
where i,j=1,2 and above inequality is valid for all f,g of bounded measurable functions with compact support.
Theorem 1.3. Let q′>1, σ∈BBS11,1 and a be a Lipschitz function on Rn. Suppose for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1. If (ω1,ω2)∈(Ap1/q′,Ap2/q′) and ω=ωpp11ωpp22, then for q′<p1,p2<∞ with 1/p=1/p1+1/p2, [Tσ,a]j(j=1,2) is bounded from Lp1(ω)×Lp2(ω) into Lp(ω).
Theorem 1.4. Let q′>1, σ∈BBS11,1 and a be a Lipschitz function on Rn. Suppose for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1. If b∈BMO, (ω1,ω2)∈(Ap1/q′,Ap2/q′) and ω=ωpp11ωpp22, then for q′<p1,p2<∞ with 1/p=1/p1+1/p2, [[Tσ,a]j,b]i(i,j=1,2) is bounded from Lp1(ω)×Lp2(ω) into Lp(ω).
Theorem 1.5. Let p(⋅),p1(⋅),p2(⋅)∈B(Rn) with 1/p(⋅)=1/p1(⋅)+1/p2(⋅), and qj0 be given as in Lemma 4.4 for pj(⋅), j = 1, 2. Suppose that σ∈BBS11,1, a is a Lipschitz function on Rn and 1<q′≤min{q10,q20}. If for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1, then [Tσ,a]j(j=1,2) is bounded from Lp1(⋅)(Rn)×Lp2(⋅)(Rn) into Lp(⋅)(Rn).
Theorem 1.6. Let p(⋅),p1(⋅),p2(⋅)∈B(Rn) with 1/p(⋅)=1/p1(⋅)+1/p2(⋅), and qj0 be given as in Lemma 4.4 for pj(⋅), j = 1, 2. Suppose that σ∈BBS11,1, a is a Lipschitz function on Rn and 1<q′≤min{q10,q20}. If for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1, and b∈BMO, then [[Tσ,a]j,b]i(i,j=1,2) is bounded from Lp1(⋅)(Rn)×Lp2(⋅)(Rn) into Lp(⋅)(Rn).
Theorem 1.7. Let σ∈BBS11,1 and a be a Lipschitz function. Suppose for fixed 1≤r1,r2≤q′ with 1/r=1/r1+1/r2, [Tσ,a]j(j=1,2) is bounded from Lr1×Lr2 into Lr,∞ with norm controlled by ‖a‖Lip1. Then [Tσ,a]j(j=1,2) is bounded from L∞×L∞ into BMO.
We use the following notation: For 1≤p≤∞, p′ is the conjugate index of p, that is, 1/p+1/p′=1. B(x,R) denotes the ball centered at x with radius R>0 and fB=1|B(x,R)|∫B(x,R)f(y)dy. The paper is organized as follows. The pointwise estimates of the sharp maximal functions are presented in Section 2. The weighted boundedness is given in Section 3. The proofs of the boundedness on the product of variable exponent Lebesgue spaces are showed in Section 4. The endpoint estimate is proved in Section 5.
2.
Pointwise estimates for the sharp maximal functions
In this section, we shall prove Theorems 1.1 and 1.2. In order to do this, let's recall some definitions.
Given a function f∈Lloc(Rn), the sharp maximal function is defined by
where the supremum is taken over all balls B containing x. Let 0<δ<∞. We denote by M♯δ the operator
Similarly, we use Mδ to denote the operator Mδ(f)=[M(|f|δ)]1/δ, where M is the Hardy-Littlewood maximal function defined by
The operator M♯δ was appeared implicitly in a paper by John [20] and was introduced by Strömberg [37]. The sharp maximal function M♯ and M♯δ not only have close relation to BMO, but also are important tools to obtain pointwise inequalities regarding many operators in harmonic analysis (see [3,12,21,25,26,36]).
To prove the Theorems 1.1 and 1.2, we need the following Kolmogorov's inequality and the inequality regarding the BMO functions.
Lemma 2.1. [19,28] Let 0<p<q<∞. Then there is a constant C=Cp,q>0, such that
for all measurable functions f.
Lemma 2.2. [27] Let f∈BMO(Rn). Suppose 1≤p<∞, r1>0, r2>0 and x∈Rn. Then
where C is a positive constant independent of f, x, r1 and r2.
Lemma 2.3. [5] If σ∈BBS11,1 and a is a Lipschitz function on Rn, then the commutators [Tσ,a]j,j=1,2 are bilinear Calderón-Zygmund operators. In particular, [Tσ,a]j,j=1,2 are bounded from Lp1×Lp2 into Lp for 1p=1p1+1p2 and 1<p1,p2<∞ and verify appropriate end-point boundedness properties. Moreover, the corresponding norms of the operators are controlled by ‖a‖Lip1.
Proof of Theorem 1.1. Let f,g be bounded measurable functions with compact support. Then for any ball B=B(x0,rB) containing x, we decompose f and g as follows:
Choose a z0∈3B∖2B. Then
For any 0<δ<r<∞, it follows from Lemma 2.1 that
If z∈B,y1∈(16B)c,y2∈16B, noticing that |z−y1|+|z−y2|+|y1−y2|∼|z−y1|+|z−y2|≥|z−y1|, then we have by Theorem A,
By the similar way, we can get that
As z∈B and y1,y2∈(16B)c, then |y1−z0|≥2|z−z0|, |y2−z0|≥2|z−z0| and rB≤|z−z0|≤4rB. It follows from Hölder's inequality that
where we use the fact of a weaker size condition of standard m-linear Calderón-Zygmund kernel than its classical size condition given in [31], that is: For any k1,⋯,km∈N+, there are positive constant Cki, i=1,⋯,m, such that
where ∞∑ki=1Cki<∞,i=1,2, 1<q<∞. Together with the commutators [Tσ,a]j,j=1,2 are bilinear Calderón-Zygmund operators and Theorem A, then we obtain the fact that
Thus, we have
Thus we finish the proof of Theorem 1.1.
Proof of Theorem 1.2. Without loss of generality, we consider the case i=1, the proof of the case i=2 is similar. Let f1, f2 be bounded measurable functions with compact support. As in the proof of Theorem 1.1, we write f and g as
Then
where b16B=1|16B|∫16Bb(z)dz. Therefore, for any fixed z0∈3B∖2B, we have
Since 0<δ<1/2 and δ<ε<∞, there exists an l such that 1<l<min{εδ,11−δ}. Then δl<ε and δl′>1. By Hölder's inequality, we have
Since q′<s<∞, denoting t=s/q′, then 1<t<∞. Noticing that 0<δ<r<∞, it follows from Lemmas 2.1 and 2.3 that
By Theorem A, as z∈B,y1∈(16B),y2∈16Bc, noticing that |z−y1|+|z−y2|+|y1−y2|∼|z−y1|+|z−y2|≥|z−y2|, then we have
Similar to estimate II3, by Lemma 2.2, we can get that
As z∈B and y1,y2∈(16B)c, then |y1−z0|≥2|z−z0|, |y2−z0|≥2|z−z0| and rB≤|z−z0|≤4rB. Noticing that 1q+1tq′+1t′q′=1. It follows from Hölder's inequality, Theorem A and the fact (2.3) that
Combining the estimate of II_{j}, j = 1, 2, 3, 4, 5 , we get
Similarly, for the case i = 2 , we can obtain that
Thus,
This finishes the proof of Theorem 1.2.
3.
Boundedness on product of weighted Lebesgue spaces
The theory of weighted estimates has played very important roles in modern harmonic analysis with lots of extensive applications in the others fields of mathematics, which has been extensively studied (see [35,29,30,33], for instance). In this section, for the commutators of bilinear pseudo-differential operators and Lipschitz functions, we will establish its boundedness of product of weighted Lebesgue spaces owning to the pointwise estimate of its sharp maximal function, that is, Theorem 1.1. The boundedness of the corresponding bilinear commutators with \mathrm{BMO} function on the product of weighted Lebesgue spaces is also obtained by using Theorem 1.1 and Theorem 1.2.
Let us recall the definition of the class of Muckenhoupt weights A_{p} before proving Theorems 1.3 and 1.4. Let 1 < p < \infty and \omega be a non-negative measurable function. We say \omega\in A_{p} if for every cube Q in \mathbb{R}^{n} , there exists a positive C independent of Q such that
Denote by A_{\infty} = \bigcup_{p\geq 1}A_{p} . It is well known that if \omega\in A_{p} with 1 < p < \infty , then \omega\in A_{r} for all r > p , and \omega\in A_{p} for some q , 1 < q < p .
To prove Theorems 1.3 and 1.4, we need the following inequality regarding maximal functions which is a version of the classical ones due to Fefferman and Stein in (see [17]), and a property of A_{p} .
Lemma 3.1. [17] Let 0 < p, \delta < \infty , and \omega\in A_{\infty} . Then there exists a positive constant C depending on the A_{\infty} constant of \omega such that
for every function f such that the left-hand side is finite.
Lemma 3.2 [18] For (\omega_{1}, \cdots, \omega_{m})\in (A_{p_{1}}, \cdots, A_{p_{m}}) with 1\leq p_{1}, \cdots, p_{m} < \infty , and for 0 < \theta_{1}, \cdots, \theta_{m} < 1 such that \theta_{1}+\cdots+\theta_{m} = 1 , we have \omega_{1}^{\theta_{1}}\cdots\omega_{m}^{\theta_{m}}\in A_{\max\{p_{1}, \cdots, p_{m}\}} .
Proof of Theorem 1.3. It follows from Lemma 3.2 that \omega\in A_{\max\{p_{1}/q^{\prime}, p_{2}/q^{\prime}\}}\subset A_{\infty} . Take a \delta such that 0 < \delta < 1/2 . Then by Lemma 3.1 and Theorem 1.1, we get
We complete the proof of the Theorem 1.3.
Proof of Theorem 1.4. It follows from Lemma 3.2 that \omega\in A_{\infty} . Take \delta and \varepsilon such that 0 < \delta < \varepsilon < 1/2 . Then by Lemma 3.1 and Theorem 1.1, let \vec{f} = (f_{1}, f_{2}) , we get
Since \omega_{t}\in A_{p_{t}/q^{\prime}} , t = 1, 2 , there exists an l_{t} such that 1 < l_{t} < p_{t}/q^{\prime} and \omega_{t}\in A_{l_{t}} . It follows from q^{\prime} < p_{t}/l_{t} that there is an s_{t} such that q^{\prime} < s_{t} < p_{t}/l_{t} < p_{t} . Let s = \min\{s_{1}, s_{2}\} . Then s > q^{\prime} and s < p_{t} .
Since l_{t} < p_{t}/s_{t}\leq p_{t}/s , then \omega_{i}\in A_{l_{t}}\subset A_{p_{t}/s} , t = 1, 2 . It follows from Lemma 3.1 and Theorem 1.2 that
We complete the proof of the Theorem 1.4.
4.
Boundedness on product of variable exponent Lebesgue spaces
The spaces with variable exponent have been widely studied in recent ten years. The results show that they are not only the generalized forms of the classical function spaces with invariable exponent, but also there are some new breakthroughs in the research techniques. These new real variable methods help people further understand the function spaces. Due to the fundamental paper [24] by Kovóčik and Rákosník, Lebesgue spaces with variable exponent L^{p(\cdot)}(\mathbb{R}^{n}) becomes one of the important class function spaces. The theory of the variable exponent function spaces have been applied in fluid dynamics, elastlcity dynamics, calculus of variations and differential equations with non-standard growth conditions (for example, see [1,2,16]). In [8], authors proved the extrapolation theorem which leads the boundedness of some classical operators including the commutators on L^{p(\cdot)}(\mathbb{R}^{n}) . Karlovich and Lerner also obtained the bundedness of the singular integral commutators in [23]. The boundedness of some typical operators is being studied with keen interest on spaces with variable exponent (see [9,22,41,42,43]).
In this section, we will establish the boundedness of [T_{\sigma}, a]_{j} and [[T_{\sigma}, a]_{j}, b]_{i}(i, j = 1, 2) on the product of variable exponent Lebesgue spaces, that is, we shall prove Theorems 1.5 and 1.6.
Denote \mathcal{P}(\mathbb{R}^{n}) to be the set of all measurable functions p(\cdot):\mathbb{R}^{n}\rightarrow [1, \infty) with
and \mathcal{B}(\mathbb{R}^{n}) to be the set of all functions p(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) satisfying the condition that the Hardy-littlewood maximal operator M is bounded on L^{p(\cdot)}(\mathbb{R}^{n}) .
Definition 4.1. [23] Let p(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) . The variable exponent Lebesgue space is defined by
As p(\cdot) = p is a constant, then L^{p(\cdot)}(\mathbb{R}^{n}) = L^p(\mathbb{R}^{n}) coincides with the usual Lebesgue space. It is pointed out in [23] that L^{p(\cdot)}(\mathbb{R}^{n}) becomes a Banach space with respect to the norm
Lemma 4.2. [13] Let p(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) . Then M is bounded on L^{p(\cdot)}(\mathbb{R}^{n}) if and only if M_{q_{0}} is bounded on L^{p(\cdot)}(\mathbb{R}^{n}) for some 1 < q_{0} < \infty , where M_{q_{0}}(f) = [M(|f|^{q_{0}})]^{1/q_{0}} .
Lemma 4.3. [32] Let p(\cdot), p_{1}(\cdot), \cdots, p_{m}(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) so that 1/p(x) = 1/p_{1}(x)+\cdots+1/p_{m}(x) . Then for any f_{j}\in L^{p_{j}}(\mathbb{R}^{n}) , j = 1, 2, \cdots, m , there has
Lemma 4.4. [14] Given a family \mathcal{F} of ordered pairs of measurable functions, suppose for some fixed 0 < p_{0} < \infty , every (f, g)\in \mathcal{F} and every \omega\in A_{1} ,
Let p(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) with p_{0}\leq p_{-} . If (\frac{p(\cdot)}{p_{0}})^{\prime}\in \mathcal{B}(\mathbb{R}^{n}) , then there exists a constant C > 0 such that for all (f, g)\in \mathcal{F} , \|f\|_{L^{p(\cdot)}(\mathbb{R}^{n})}\leq C\|g\|_{L^{p(\cdot)}(\mathbb{R}^{n})} .
Lemma 4.5. [14] If p(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) , then C_{0}^{\infty} is dense in L^{p(\cdot)}(\mathbb{R}^{n}) .
Lemma 4.6. [13] Let p(\cdot)\in \mathcal{P}(\mathbb{R}^{n}) . Then the following conditions are equivalent.
(1) p(\cdot)\in \mathcal{B}(\mathbb{R}^{n}) ;
(2) p^{\prime}(\cdot)\in \mathcal{B}(\mathbb{R}^{n}) ;
(3) p(\cdot)/p_{0}\in \mathcal{B}(\mathbb{R}^{n}) for some 1 < p_{0} < p_{-} ;
(4) (p(\cdot)/p_{0})^{\prime}\in \mathcal{B}(\mathbb{R}^{n}) for some 1 < p_{0} < p_{-} .
Proof of Theorem 1.5. Here we note \vec{f} = (f_{1}, f_{2}) , where f_{1} and f_{2} are bounded measurable functions with compact support. Since p(\cdot)\in \mathcal{B}(\mathbb{R}^{n}) , then by Lemma 4.6, there exists a p_{0} such that 1 < p_{0} < p_{-} and (p(\cdot)/p_{0})^{\prime}\in \mathcal{B}(\mathbb{R}^{n}) . Take a \delta such that 0 < \delta < 1/2 . For any \omega\in A_{1} , it follows from Lemma 3.1 and Theorem 1.1 that
Applying Lemma 4.4 to the pair ([T_{\sigma}, a]_{j}(\vec{f}), \prod_{t = 1}^{2}M_{q_{0}^{t}}(f_{t})) , we can get
Then by Lemmas 4.2 and 4.3, we have
This completes the proof of the Theorem 1.5.
Proof of Theorem 1.6. Denote q_{0} = \min \{q_{0}^{1}, q_{0}^{2}\} , then q^{\prime} < q_{0} < \infty . Let \vec{f} = (f_{1}, f_{2}) , where f_{1} and f_{2} are bounded measurable functions with compact support. Since p(\cdot)\in \mathcal{B}(\mathbb{R}^{n}) , then by Lemma 4.6, there exists a p_{0} such that 1 < p_{0} < p_{-} and (p(\cdot)/p_{0})^{\prime}\in \mathcal{B}(\mathbb{R}^{n}) . Take \delta and \varepsilon such that 0 < \delta < \varepsilon < 1/2 . For any \omega\in A_{1} , it follows from Lemma 3.1, Theorem 1.1 and Theorem 1.2 that
Applying Lemma 4.4 to the pair ([[T_{\sigma}, a]_{j}, b]_{i}(\vec{f}), \prod_{t = 1}^{2}M_{q_{0}^{t}}(f_{t})) , we can get
Then by Lemmas 4.2 and 4.3, we have
We complete the proof of Theorem 1.6.
5.
Endpoint estimate
In this section, we will show the endpoint estimate for the [T_{\sigma}, a]_{j} \; {\rm{with} }\; j = 1, 2 , that is, we will give the proof of Theorem 1.7.
Proof of Theorem 1.7. Take p_{1}, p_{2} such that \max\{q^{\prime}, 2\} < p_{1}, p_{2} < \infty . Let 1/p = 1/p_{1}+1/p_{2} . Then 1 < p < \infty . It follows from Lemma 2.3 that [T_{\sigma}, a]_{j}(j = 1, 2) is bounded from L^{p_{1}}\times L^{p_{2}} into L^{p} .
Let f, g\in L^{\infty} . Then for any ball B = B(x_{0}, r_{B}) with r_{B} > 0 , we decompose f and g as follows
Then
It follows from the Hölder's inequality and Lemma 2.3 that
By the size conditions in Theorem A of the kernel, we have
Similarly, we can obtain that
Noting that as z\in B , and y_{1}, y_{2}\in (2B)^{c} , then |y_{1}-x_{0}|\geq 2|z-x_{0}| and |y_{2}-x_{0}|\geq 2|z-x_{0}| . It follows from the Hölder's inequality and (2.3) that
Thus,
Which completes the proof of the Theorem 1.7.
6.
Conclusions
In this paper, we consider the commutators of bilinear pseudo-differential operators and the operation of multiplication by a Lipschitz function. By establishing the pointwise estimates of the corresponding sharp maximal function, the boundedness of the commutators is obtained respectively on the products of weighted Lebesgue spaces and variable exponent Lebesgue spaces with \sigma \in\mathcal{B}BS_{1, 1}^{1} . Moreover, the endpoint estimate of the commutators is also established on L^{\infty}\times L^{\infty} .
Acknowledgments
This work is supported by the Doctoral Scientific Research Foundation of Northwest Normal University (202003101203), Young Teachers ^{'} Scientific Research Ability Promotion Project of Northwest Normal University (NWNU-LKQN2021-03) and National Natural Science Foundation of China (11561062).
Conflict of interest
The authors declare that they have no conflict of interest.













 DownLoad:
DownLoad: