Linear superposition and interaction of Wronskian solutions to an extended (2+1)-dimensional KdV equation

1.   Introduction

The Euler numbers $E_n$ are defined by the exponential generating function

This is the sequence A000111 in [20]. In 1877 Seidel [19] defined the triangular array $(E_{n,k})$ by the recurrence

with $E_{1,1} = 1$, $E_{n,1} = 0$ ($n\ge2$), and proved that $E_n = \sum_k E_{n,k}$, i.e., the Euler number $E_n$ is the sum of the entries of the $n$-th row of the following triangle:

The first few values of ${E}_{n,k}$ are given in Table 1.

André [1] showed in 1879 that the Euler number $E_n$ enumerates the alternating permutations of $[n]: = \left\{{1,2,\dots,n}\right\}$, i.e., the permutations $\sigma_1\sigma_2\dots\sigma_n$ of $12\ldots n$ such that $\sigma_1 > \sigma_2 < \sigma_3 > \sigma_4 < \cdots.$ Let ${{\mathcal{DU} }_n}$ be the set of (down-up) alternating permutations of $[n]$. For example,

In 1933 Kempener [14] used the boustrophedon algorithm (2) to enumerate alternating permutations without refering to Euler numbers. Since Entringer [7] first found the combinatorial interpretation of Kempener's table $(E_{n,k})$ in terms of André's model for Euler numbers, the numbers $E_{n,k}$ are then called Entringer numbers.

Theorem 1 (Entringer). The number of the (down-up) alternating permutations of $[n]$ with first entry $k$ is $E_{n,k}$, i.e., $E_{n,k} = \# {{\mathcal{DU} }_{n,k}}$, where

According to Foata-Schützenberger [9] a sequence of sets $(X_{n})$ is called an André complex if the cardinality of $X_{n}$ is equal to $E_{n}$ for $n\geq 1$. Several other André complexs were introduced also in [9] such as André permutations of first and second kinds, André trees or increasing 1-2 trees and Rodica Simion and Sheila Sundaram [23] discovered the Simsun permutations; see Section 2. A sequence of sets $(X_{n,k})$ is called an Entringer family if the cardinality of $X_{n,k}$ is equal to $E_{n,k}$ for $1 \le k \le n$. During the last two decades of the twentieth century, Poupard worked out several Entringer families in a series of papers [15,16,17]. Stanley [22,Conjecture 3.1] and Hetyei [12] introduced more Entringer families by refining of Purtill's result [18,Theorem 6.1] about the $cd$-index of André and Simsun permutations with fixed last letter.

The Springer numbers $S_n$ are defined by the exponential generating function [21]

Arnold [2,p.11] showed in 1992 that $S_n$ enumerates a signed-permutation analogue of the alternating permutations. Recall that a signed permutation of $[n]$ is a sequence $\pi = (\pi_1, \ldots, \pi_n)$ of elements of $[\pm n]: = \{-1,\dots, -n\}\cup \{1,\dots,n\}$ such that $|\pi| = (|\pi_1|, \ldots, |\pi_n|)$ is a permutation of $[n]$. We write $\mathcal{B}_n$ for the set of all signed permutations of $[n]$. Clearly the cardinality of $\mathcal{B}_n$ is $2^n n!$. An (down-up) alternating permutation of type $B_n$ is a signed permutation $\pi\in \mathcal{B}_n$ such that $\pi_1>\pi_2<\pi_3>\pi_4\cdots$ and a snake of type $B_n$ is an alternating permutation of type $B_n$ starting with a positive entry. Let ${{\mathcal{DU} }_n}^{(B)}$ be the set of (down-up) alternating permutations of type $B_n$ and ${{\mathcal{S}}_n}$ the set of snakes of type $B_n$. Clearly the cardinality of ${{\mathcal{DU} }_n}^{(B)}$ is $2^n E_n$. Arnold [2] showed that the Springer number $S_n$ enumerates the snakes of type $B_n$. For example, the $11$ snakes of ${\mathcal{S}}_3$ are as follows:

where we write $\bar{k}$ for $-k$. Arnold [2] introduced the following pair of triangles to compute the Springer numbers:

where $S_{n,k}$ is defined by $S_{1,1} = S_{1,-1} = 1$, $S_{n,-n} = 0$ ($n \ge 2$), and the recurrence

Theorem 2 (Arnold). For all integers $1\le k\le n$, the number of the snakes of type $B_n$ starting with $k$ is $S_{n,k}$, i.e., $S_{n,k} = \# {{\mathcal{S}}_{n,k}}$ with $S_n = \sum_{k>0} \#{{\mathcal{S}}_{n,k}}$, where

Moreover, for all integers $-n \le k \le -1$, it holds that

Similarly, the numbers $S_{n,k}$ are called Arnold numbers and a sequence of sets $(X_{n,k})$ is called an Arnold family if the cardinality of $X_{n,k}$ is equal to $S_{n,k}$ for $1 \le \left|{k}\right| \le n$. The first values of Arnold and Springer numbers are given in Table 2. The aim of this paper is to provide some new Entringer families and new Arnold families by refining known combinatorial models for Euler and Springer numbers. To this end we shall build bijections between these new Entringer (resp. Arnold) families with the known ones. We refer the reader to the more recent papers [23,11,4,13,8] related to the combinatorics of Euler numbers and Springer numbers.

This paper is organized as follows. In Section 2, we shall give the necessary definitions and present our main results. The proof of our theorems will be given in Sections 3-4. In Section 5, we shall give more insightful description of two important bijections. More precisely, Chuang et al.'s constructed a $\phi: {\mathcal{T}}_{n+1} \to {\mathcal{RS}}_{n}$ in [4], we show that $\phi$ can be factorized as the compositions of two of our simpler bijections, and then give a direct description of Gelineau et al.'s bijection $\psi: {{\mathcal{DU} }_n} \to {{\mathcal{T}}_{n}}$ in [11].

2.   Definitions and main results

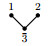
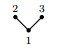
Let $V$ be a finite subset of ${\mathbb{N}}$. An increasing 1-2 tree on $V$ is a vertex labeled rooted tree with at most two (upward) branchings at any vertex and vertex labels in increasing order on any (upward) path from the root, see Figure 2. In what follows, when one draws an increasing 1-2 tree, let's designate the left child if its parent has a unique child or it is smaller than the other sibling and the others, except of the root, are designate the right child.

For each vertex $v$ of a binary tree, by exchanging the left and right subtrees of $v$, we obtain another binary tree. This operation is called a flip. If two binary trees can be connected by a sequence of flips, we say that these two trees are flip equivalent. Since flip equivalence is obviously an equivalence relation, we are able to define the equivalence classes of binary trees, which are corresponding to increasing 1-2 trees; see [23,Section 3.2] in detail.

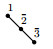
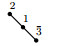
Definition 3. Given an increasing 1-2 tree $T$, the minimal path of $T$ is the unique sequence $(v_1, \cdots, v_{\ell})$ of vertices where $v_1$ is the root, $v_{k+1}$ is the left child of $v_k$ ($1\le k<\ell$), and $v_\ell$ is a leaf. The vertex $v_\ell$ is called the minimal leaf of $T$ and denoted by ${\rm{Leaf}}(T)$. Similarly, the unique path $(v_1, \cdots, v_{\ell})$ from $v_1 = v$ to a leaf $v_{\ell}$ of $T$ is called the maximal path from $v$ if $v_{k+1}$ is the right child of $v_k$ for $1\le k<\ell$.

Let ${\mathcal{T}}_V$ be the set of increasing 1-2 trees on $V$ with ${\mathcal{T}}_{n}: = {\mathcal{T}}_{[n]}$ and

Donaghey [5] (see also [3]) proved bijectively that the Euler number $E_n$ enumerates the binary increasing trees in ${\mathcal{T}}_n$ and Poupard [15] showed that the sequence $({\mathcal{T}}_{n,k})$ is an Entringer family. In a previous work Gelineau et al. [11] proved bijectively Poupard's result by establishing a bijection between ${{\mathcal{DU} }_n}$ and ${{\mathcal{T}}_{n}}$.

Theorem 4 (Gelineau-Shin-Zeng). There is an explicit bijection $\psi: {{\mathcal{DU} }_n} \to {{\mathcal{T}}_{n}}$ such that

for all $\sigma\in {{\mathcal{DU} }_n}$, where ${\rm{First}}(\sigma)$ is the first entry of $\sigma$.

Let ${\mathfrak{S}}_n$ be the group of permutations on $[n]$. For a permutation $\sigma = \sigma_1 \dots \sigma_n \in {\mathfrak{S}}_n$, a descent (resp. ascent) of $\sigma$ is a pair $(\sigma_i, \sigma_{i+1})$ with $\sigma_i > \sigma_{i+1}$ (resp. $\sigma_i < \sigma_{i+1}$) and $1 \le i \le n-1$, a double descent of $\sigma$ is a triple $(\sigma_i, \sigma_{i+1}, \sigma_{i+2})$ with $\sigma_i > \sigma_{i+1} > \sigma_{i+2}$ and $1 \le i \le n-2$, and a valley of $\sigma$ is a triple $(\sigma_i, \sigma_{i+1}, \sigma_{i+2})$ with $\sigma_i > \sigma_{i+1} < \sigma_{i+2}$ and $1 \le i \le n-2$.

Hetyei [12,Definition 4] defined recursively André permutation of second kind if it is empty or satisfies the following:

(ⅰ) $\sigma$ has no double descents.

(ⅱ) $(\sigma_{n-1}, \sigma_n)$ is not descent, i.e., $\sigma_{n-1} < \sigma_n$.

(ⅲ) For all $2\le i \le n-1$, if $(\sigma_{i-1}, \sigma_{i}, \sigma_{i+1})$ is a valley of $\sigma$, then the minimum letter of $w_2$ is larger than the minimum letter of $w_4$ for the $\sigma_i$-factorization $(w_1, w_2, \sigma_i, w_4, w_5)$ of $\sigma$, where the word $w_1 w_2 \sigma_i w_4 w_5$ is equal to $\sigma$ and $w_2$ and $w_4$ are maximal consecutive subwords of $\sigma$ satisfies its all letters are greater than $\sigma_i$.

It is known that the above definition for André permutation of second kind is simply equivalent to the following definition. Let $\sigma_{[k]}$ denote the subword of $\sigma$ consisting of $1,\dots, k$ in the order they appear in $\sigma$.

Definition 5. A permutation $\sigma \in {\mathfrak{S}}_n$ is called an André permutation if $\sigma_{[k]}$ has no double descents and ends with an ascent for all $1\leq k\leq n$.

For example, the permutation $\sigma = 43512$ is not André since the subword $\sigma_{[4]} = 4312$ contains a double descent $(4,3,1)$, while the permutation $\tau = 31245$ is André since there is no double descent in the subwords:

Foata and Schützenberger [10] proved that the Euler number $E_n$ enumerates the André permutations in ${\mathfrak{S}}_n$. Let ${{\mathcal{A}}_n}$ be the set of André permutations in ${\mathfrak{S}}_n$. For example,

Remark. Foata and Schützenberger in [10] introduced augmented André permutation is a permutation $\sigma \in {\mathfrak{S}}_n$, if $\sigma$ has no double descents, $\sigma_n = n$, and, for $1<j<k\le n$ satisfying

there exists $\ell$ such that $j<\ell <k$ and $\sigma_\ell < \sigma_k$.

Definition 6. A permutation $\sigma \in {\mathfrak{S}}_n$ is called a Simsun permutation if $\sigma_{[k]}$ has no double descents for all $1\leq k\leq n$.

By definition, an André permutations is always a Simsun permutation, but the reverse is not true. For example, the permutation $\sigma = 25134$ is Simsun but not André, because $\tau_{[2]} = 21$ ends with an descent:

Let ${{\mathcal{RS}}_n}$ be the set of Simsun permutations in ${\mathfrak{S}}_n$. For example,

As for ${{{\mathcal{DU} }}_{n,k}}$, we define two similar refinements of André permutations and Simsun permutations as

Some examples are shown in Table 3.

Foata and Han [8,Theorem 1 (ⅲ)] proved that ${{\mathcal{A}}_{n,k}}$ is an Entringer family by constructing a bijection between ${{\mathcal{DU} }_{n,k}}$ and ${{\mathcal{A}}_{n,k}}$. We shall give an easier proof of their result by constructing a simpler bijection $\omega$ between ${{\mathcal{T}}_{n,k}}$ and ${{\mathcal{A}}_{n,k}}$. Of course, combining $\psi$ (cf. Theorem 4) and $\omega$ we obtain another bijection between ${{\mathcal{DU} }_{n,k}}$ and ${{\mathcal{A}}_{n,k}}$.

Theorem 7. For positive integer $n\ge 1$, there is a bijection $\omega: {{\mathcal{T}}_{n}} \to {{\mathcal{A}}_n}$ such that

for all $T \in {{\mathcal{T}}_{n}}$, where ${\rm{Last}}(\sigma)$ is the last entry of $\sigma$. In words, for all $1\le k \le n$, the mapping $\omega$ is a bijection from ${{\mathcal{T}}_{n,k}}$ onto ${{\mathcal{A}}_{n,k}}$.

Whereas one can easily show that the cardinality $\#{{\mathcal{DU} }}_{n,k}$ of (down-up) alternating permutations of length $n$ with first entry $k$ satisfies (1), it seems hard to show that the cardinality $\#{{\mathcal{A}}_{n,k}}$ of André permutations of length $n$ with last entry $k$ or the cardinality $\#{{\mathcal{RS}}_{n-1,k-1}}$ of Simsun permutations of length $n-1$ with last entry $k-1$ does. Thus, in order to show (4) and (6), we shall construct a bijection between ${{\mathcal{DU} }_{n,k}}$, ${{\mathcal{A}}_{n,k}}$, ${{\mathcal{RS}}_{n-1,k-1}}$, and other known Entringer families in [11].

Stanley [22,Conjecture 3.1] conjectured a refinement of Purtill's result [18,Theorem 6.1] about the $cd$-index of André and Simsun permutations with fixed last letter. In this conjecture, he mentioned three kinds of André permutations: (ⅰ) Augmented André permutations in Remark 2, (ⅱ) André permutations in Definition 5, and (ⅲ) augmented Sundaram permutations, where the third corresponds to Simsun permutations in Definition 6 by removing last letter. Hetyei [12] proved the conjecture for the second and the third by verifying that both sides satisfy the same recurrence. In particular, he proves the following result.

Theorem 8 (Hetyei). For all $1\le k \le n$, we have that two cardinalities of ${{\mathcal{A}}_{n,k}}$ and ${{\mathcal{RS}}_{n-1,k-1}}$ are same, that is,

In the next theorem, we give a bijective proof of the conjecture of Stanley by constructing an explicit bijection.

Theorem 9. For positive integer $n\ge 1$, there is a bijection $\varphi: {{\mathcal{A}}_n}\to {{\mathcal{RS}}_{n-1}}$ such that

for all $\sigma \in {{\mathcal{A}}_n}$. In words, the mapping $\varphi$ is a bijection from ${{\mathcal{A}}_{n,k}}$ onto ${{\mathcal{RS}}_{n-1,k-1}}$. Moveover, the bijection $\varphi$ preserves the $cd$-index of André and Simsun permutation.

Given a permutation $\sigma \in B_n$, denote $\sigma_{[k]}$ the subword of $\sigma$ consisting of $k$ smallest entries in the order they appear in $\sigma$. A signed André permutation of $[n]$ is a permutation $\sigma \in B_n$ such that $\sigma_{[k]}$ has no double descents and ends with an ascent for all $1\leq k\leq n$. Let ${{{\mathcal{A}}^{(B)}}_n}$ be the set of signed André permutations of $[n]$ and ${{{\mathcal{A}}^{(B)}}_{n,k}}$ be the set of signed André permutations $\sigma$ in ${{{\mathcal{A}}^{(B)}}_n}$ ending with entry $k$. For example, the permutation $\sigma = 2\bar{4}\bar{1}35$ is Andŕe due to

Some examples of ${{\mathcal{A}}^{(B)}}_{3,k}$ are shown in Table 4.

Definition 10. A type $B$ increasing 1-2 tree on $[n]$ is a binary tree with $n$ signed labels in $\{\pm1,\pm2,\dots,\pm n\}$ such that the absolute values of signed labels are distinct and any vertex is greater than its children.

For example, all type $B$ increasing 1-2 trees on $[3]$ are given in Figure 2. Let ${{{\mathcal{T}}^{(B)}}_{n}}$ be the set of type $B$ increasing 1-2 trees on $n$ vertices and ${{{\mathcal{T}}^{(B)}}_{n,k}}$ be the set of trees $T$ in ${{{\mathcal{T}}^{(B)}}_{n}}$ with leaf $k$ as the end of minimal path. Clearly we have ${{{\mathcal{T}}^{(B)}}_{n}} = \bigcup_{\left|{k}\right|>0} {{{\mathcal{T}}^{(B)}}_{n,k}}$.

Our second aim is to show that these two refinements are new Arnold families. Recall that the sequence ${{\mathcal{S}}_{n,k}}$ is an Arnold family for $1\leq \left|{k}\right| \leq n$ as

Theorem 11. For all $1\le \left|{k}\right| \le n$, there are two bijections

Thus, for all $1\le \left|{k}\right| \le n$,

In particular, the two sequences ${{{\mathcal{A}}^{(B)}}_{n,k}}$ and ${{{\mathcal{T}}^{(B)}}_{n,k}}$ are Arnold families for $1\leq \left|{k}\right| \leq n$.

Hetyei[12,Definition 8] defined another class of signed André permutations.

Definition 12 (Hetyei). A signed André permutation is a pair $(\varepsilon, \pi)$, where $\pi$ is an André permutation such that $\varepsilon(i) = 1$ if $\pi_i = \min \left\{{\pi_i, \pi_{i+1}, \dots, \pi_{n}}\right\}$.

We write ${{{\mathcal{A}}^{(H)}}_n}$ (resp. ${{{\mathcal{A}}^{(H)}}_{n,k}}$) for the set of the signed André permutations (resp. ending with entry $k$) in $\mathcal{B}_n$. Some examples of ${{\mathcal{A}}^{(H)}}_{4,k}$ are shown in Table 4. We have the following conjecture.

Conjecture 13. For all $1 \le k \le n$, we have

Since the last entry of any permutation in the family ${{{\mathcal{A}}^{(H)}}_n}$ is always positive, even if Conjecture 13 is true, it covers only the half of Table 2. Now we define signed Simsun permutations corresponding to Heytei's signed André permutations.

Definition 14. A permutation $\pi$ in $\mathcal{B}_n$ is a signed Simsun permutation if $\left|{\pi_1}\right| \left|{\pi_2}\right| \dots \left|{\pi_{n}}\right|$ is a Simsun permutation and $\pi_{i}>0$ for all $\left|{\pi_i}\right| = \min \left\{{\left|{\pi_i}\right|, \left|{\pi_{i+1}}\right|, \dots, \left|{\pi_{n}}\right|}\right\}$.

Let ${{{\mathcal{RS}}^{(B)}}_n}$ be the set of signed Simsun permutations in $\mathcal{B}_n$ and ${{{\mathcal{RS}}^{(B)}}_{n,k}}$ the set of signed Simsun permutations in ${{{\mathcal{RS}}^{(B)}}_n}$ with last entry $k$. Some examples of ${{\mathcal{RS}}^{(B)}}_{3,k}$ are shown in Table 4.

Theorem 15. For positive integer $n\ge 1$, there is a bijection $\varphi^{(B)}: {{{\mathcal{A}}^{(H)}}_n} \to {{{\mathcal{RS}}^{(B)}}_{n-1}}$ such that

for all $\sigma \in {{{\mathcal{A}}^{(H)}}_n}$. In words, the mapping $\varphi^{(B)}$ is a bijection from ${{{\mathcal{A}}^{(H)}}_{n,k}}$ onto ${{{\mathcal{RS}}^{(B)}}_{n-1,k-1}}$.

Remark. Ehrenborg and Readdy [6,Section 7] gave a different definition of signed Simsun permutation as follows: A signed permutation $\sigma$ of length $n$ is a Simsun permutation if $\sigma_{[k]}$ have no double descents for all $1\le k\le n$, where $\sigma_{[k]}$ is obtained by removing the $(n-k)$ entries $\pm (k+1), \dots, \pm n$ from $\sigma$. Beacuse all eight signed permutations of length $2$

are Simsun permutations, we note that it is not an Arnold family.

3.   Proof of Theorems 7, 9, and 15

First of all, we prove Theorem 7, in order to show that $({{\mathcal{A}}_{n,k}})_{1\le k \le n}$ is a Entringer family, that is, $E_{n,k} = \# {{\mathcal{A}}_{n,k}}$. We construct a bijection $\omega$ between ${{\mathcal{T}}_{n,k}}$ and ${{\mathcal{A}}_{n,k}}$ in Section 3.1. Hence the map $\omega \circ \psi$ is a bijection from the set ${{\mathcal{DU} }_{n,k}}$ of (down-up) alternating permutations with first entry $k$ to the set ${{\mathcal{A}}_{n,k}}$ of André permutations with last entry $k$. Also, in order to show that $({{\mathcal{RS}}_{n-1,k-1}})_{1\le k \le n}$ is a Entringer family, that is $E_{n,k} = \# {{\mathcal{RS}}_{n-1,k-1}}$, we construct a bijection between ${{\mathcal{A}}_{n,k}}$ and ${{\mathcal{RS}}_{n-1,k-1}}$ in Section 3.2 and then two sets ${{\mathcal{A}}_{n,k}}$ and ${{\mathcal{RS}}_{n-1,k-1}}$ have the same cardinality.

3.1.   Proof of Theorem 7: Bijection $\omega : {{\mathcal{T}}_{n,k}} \to {{\mathcal{A}}_{n,k}}$.

Given $T \in {{\mathcal{T}}_{n,k}}$, write down the word $\sigma$ of the vertices of the tree $T$ in inorder, namely, for any vertex $v$ in $T$, the left child of $v$ and its descendants precede the vertex $v$ and the vertex $v$ precede the right child of $v$ and its descendants. Since $T$ is an increasing tree, we can recover $T$ from $\sigma$ by finding minimum in subwords in $\sigma$ successively. The word $\sigma$ has no double ascents because no vertex in $T$ has only a right child. The leaf $p(T)$ of the minimal path of $T$ is $\sigma_1$ and the parent of $\sigma_1$ is $\sigma_2$, so $\sigma$ starts with a decent, that is, $\sigma_1 > \sigma_2$. Similarly, since the subgraph of $T$ consisting of $1, \dots, k$, for any $k$, is also well-defined an increasing 1-2 subtree, the subwords of $\sigma$ consisting of $1, \dots, k$ has also no double ascents and starts with a decent. Thus the word $\sigma^R$, which is the reverse word of $\sigma$, is an André permutation of $n$ and let $\omega(T) = \sigma^R$.

For example, if the tree $T$ is given by the following figure with a corresponding word $\sigma$,

then $\omega(T) = \sigma^R = 684512937$ as reading reversely vertices of $T$ in inorder.

3.2.   Proof of Theorem 9: Bijection $\varphi : {{\mathcal{A}}_{n,k}} \to {\mathcal{RS}}_{n-1,k-1}$.

Given $\sigma: = \sigma_1\ldots \sigma_n \in {{\mathcal{A}}_{n,k}}$, let $i_1<\ldots< i_\ell$ be the positions of the right-to-left minima of $\sigma$. Clearly $\sigma_{i_1} = 1$, $i_{\ell-1} = n-1$ and $i_\ell = n$ with $\sigma_{n} = k$. Let $\varphi(\sigma) = \pi$, where $\pi = \pi_1 \pi_2 \dots \pi_{n-1}$ is defined by

We show that $\pi \in {\mathcal{RS}}_{n-1,k-1}$. Suppose $\pi_{[i]}$ has a double descent for some $1\le i \le n$. There exists a triple $(a,b,c)$ such that $1\le a < b < c \le n$, $i \ge \pi_a > \pi_b > \pi_c$, and $\pi_{a+1}, \ldots, \pi_{b-1}$, $\pi_{b+1}, \ldots, \pi_{c-1}$ are greater than $i$, which yields $\pi_c = \min\left\{{\pi_j : a\le j \le c}\right\}$. Then $\pi_{c}$ could be a right-to-left minimum in $\pi$ and the others $\pi_a, \pi_{a+1}, \dots, \pi_{c-1}$ are not in $\pi$. As $\varphi(\sigma) = \pi$, we have

Hence a triple $(\sigma_{a}, \sigma_{b}, \sigma_{c})$ is a double descent in $\sigma_{[i]}$, which contradicts that $\sigma$ is an André permutation. As this procedure is clearly reversible, the mapping $\varphi$ is a bijection.

Consider the running example $\sigma = 684512937$. The right-to-left minimums of $\sigma$ are $1$, $2$, $3$, $7$. So after removing $1$ from $\sigma$, the entries $2$, $3$, $7$ are moved to the positions of $1$, $2$, $3$, respectively, and we get the permutation $\hat\pi = 68452397$, and then $\varphi(\sigma) = \pi = 57341286$, which is a Simsun permutation of length $8$ with last entry $6$.

Remark. Considering the bijection $\psi$ in Theorem 4, the map $\varphi \circ \omega \circ \psi$ is a bijection from the set of (down-up) alternating permutations of length $n$ with first entry $k$ to the set of Simsun permutations of length $n-1$ with last entry $k-1$. Namely we have the diagram in Figure 3. For example, if $\tau = 739154826 \in {\mathcal{DU} }_{9,7}$, then

where $T$ is the increasing 1-2 tree given in Section 3.1.

3.3.   Proof of Theorem 15

One can extend the above mapping $\varphi$ defined on ${{\mathcal{A}}_n}$ to a mapping $\varphi^{(B)}$ on ${{{\mathcal{A}}^{(H)}}_n}$. It is also bijective between ${{{\mathcal{A}}^{(H)}}_{n,k}}$ and ${{{\mathcal{RS}}^{(B)}}_{n-1,k-1}}$, but the description of $\varphi^{(B)}$ and a proof of bijectivity are omitted, because it is very similar in Section 3.2.

Remark. This bijection preserves the $cd$-indices between André permutations and Simsun permutations. The variation of a permutation $\pi = \pi_1 \dots \pi_n$ is given by ${\mathtt{a}}{\mathtt{b}}$-monomial $u_1\dots u_{n-1}$ such that $u_i = {\mathtt{a}}$ if $\pi_i < \pi_{i+1}$ and $u_i = {\mathtt{b}}$ if $\pi_i > \pi_{i+1}$. The reduced variation of André permuation is defined by replacing each ${\mathtt{b}}{\mathtt{a}}$ with ${\mathtt{d}}$ and then replacing each remaining ${\mathtt{a}}$ by ${\mathtt{c}}$. For example, the variation and reduced variation of André permutation $\sigma = 684512937$ is

For the cd-index of a Simsun permutation $\sigma$, we consider augmented Simsun permutation by adding $\sigma(0) = 0$. Here, the reduced variation of augmented Simsun permuations is defined by replacing each ${\mathtt{a}}{\mathtt{b}}$ with ${\mathtt{d}}$ and then replacing each remaining ${\mathtt{a}}$ by ${\mathtt{c}}$. So the variation and reduced variation of augmented Simsun permutation $057341286$ by adding $0$ to $\varphi(\sigma) = 57341286$ is

4.   Proof of Theorem 11

Given a $\sigma \in {{\mathcal{S}}_{n,k}}$, there is a unique order-preserving map $\pi_\sigma$, say just $\pi$, from $\left\{{\sigma_1, \dots, \sigma_n}\right\}$ to $[n]$. In other words, $\pi$ replaces the $i$-th smallest entry in $\sigma$ by $i$. The permutation $\tau = \pi \sigma$ belongs to ${{{\mathcal{DU} }}_{n,k}}$ and $\psi(\tau) = \psi(\pi \sigma)$ in ${{\mathcal{T}}_{n,k}}$. Then $\pi^{-1}(\psi(\tau))$ means the tree with vertex labelings $\left\{{\sigma_1, \dots, \sigma_n}\right\}$ instead of $[n]$ and it should belong to ${{{\mathcal{T}}^{(B)}}_{n,k}}$. So we construct the bijection $\psi^{B}$ from ${{\mathcal{S}}_{n,k}}$ to ${{{\mathcal{T}}^{(B)}}_{n,k}}$ by

through the unique order-preserving map $\pi$. Hence, it yields (7).

For example, in the case of $\sigma = 6\bar{3}9\bar{8}2\bar{1}7\bar{4}5$, the order-preserving map $\pi_\sigma$ is

So we have $\tau = \pi \sigma = 739154826$ and $\psi(\tau) = \psi(\pi \sigma)$ and $\psi^{B}(\sigma) = \pi^{-1}(\psi(\pi \sigma))$ are illustrated as

In Subsection 3.1, we define the bijection $\omega$ from ${{\mathcal{T}}_{n,k}}$ to ${{\mathcal{A}}_{n,k}}$. Given a tree $T$ in ${{{\mathcal{T}}^{(B)}}_{n,k}}$, there is a unique order-preserving map $\pi_T$, say just $\pi$, from $V(T)$ to $[n]$. In other words, $\pi$ replaces the $i$-th smallest $V(T)$ by $i$. After relabeling on vertices of $T$ by $\pi$, we obtain the tree $\pi(T)$ which belongs to ${{\mathcal{T}}_{n,k}}$ and $\omega(\pi(T))$ is in ${{\mathcal{A}}_{n,k}}$. Then $\pi^{-1}(\omega(\pi(T)))$ should belong to ${{{\mathcal{A}}^{(B)}}_{n,k}}$. So we construct the bijection $\omega^{B}$ from ${{{\mathcal{T}}^{(B)}}_{n,k}}$ to ${{{\mathcal{A}}^{(B)}}_{n,k}}$ by

through the unique order-preserving map $\pi$. Such the map $\omega^{B}$ can be described simply, as same as $\omega$, by reading reversely vertices of $T$ in inorder. Hence, it yields (8).

For example, in the case of $T$ illustrated as

we obtain $\omega^B(T) = \sigma^R = 57\bar{1}2\bar{8}\bar{4}9\bar{3}6$ by reading reversely vertices of $T$ in inorder. So bijections for type A and type B commute in the diagram of Figure 3.

We summarize four interpretations for Entringer numbers $E_{4,k}$, $k \in \left\{{2,3,4}\right\}$ in Table 5 and left three interpretations for Arnold number $S_{3,k}$, $k \in \left\{{1,2,3}\right\}$ in Table 6. In every column, the corresponding elements are described via the different bijections mentioned in the paper.

5.   New description of two known bijections

5.1.   A decomposition of Chuang et al.'s bijection $\phi: {\mathcal{T}}_{n+1} \to {\mathcal{RS}}_{n}$

In 2012, Chuang et al. [4] construct a bijection $\phi: {\mathcal{T}}_{n+1} \to {\mathcal{RS}}_{n}$. If $x$ has only one child then it is the right child of $x$. They described the bijection $\phi$ between increasing 1-2 trees and Simsun permutations using the following algorithm.

Algorithm A.

(A1) If $T$ consists of the root vertex then $T$ is associated with an empty word.

(A2) Otherwise, the word $\rho(T)$ is defined inductively by the factorization

where the subword $\omega$ and the subtree $T'$ are determined as follows.

(a) If the root of $T$ has only one child $x$ then let $\omega = x$ (consisting of a single letter $x$), let $T' = \tau(x)$ (i.e, obtained from $T$ by deleting the root of $T$), and relabel the vertex $x$ by $1$.

(b) If the root of $T$ has two children $u$, $v$ with $u>v$ then traverse the right subtree $\tau(u)$ reversely in inorder, put down the word $\omega$ of the vertices of $\tau(u)$ and let $T' = T-\tau(u)$.

As deleted only $1$ in $\rho(T)$, every permutation $\rho(T) = a_1 a_2\cdots a_n$ is a Simsun permutation on $\left\{{2, 3, \dots, n+1}\right\}$. Thus we get a Simsun permutation $\phi(T) = b_1 b_2\cdots b_n$ on $[n]$ with $b_i = a_i - 1$ for all $1\le i \le n$.

Remark. Originally, in [4], the increasing 1-2 trees on $n$ vertices are labeled with $0,1, \ldots, n-1$ instead of $1,2, \ldots, n$ and was drawn in a canonical form such that if a vertex $x$ has two children $u$, $v$ with $u > v$ then $u$ is the left child, $v$ is the right child.

Theorem 16. The bijection $\phi : {{\mathcal{T}}_{n,k}} \to {{\mathcal{RS}}_{n-1,k-1}}$ is the composition of $\varphi$ and $\omega$; i.e., $\phi = \varphi \circ \omega$.

Proof. Suppose that we let $\omega$ be the root of $T$ without relabeling the vertex $x$ in (A2a), it is obvious that Algorithm A follow the bijeciton $\omega$, i.e., reading the vertex of $T$ reversely in inorder. So it is enough to show that the change in two rules of (A2a) follows $\varphi$.

The root of $T'$ becomes to the left-child of the root of $T$ after (A2a) and the root of $T'$ is same with the root of $T$ in (A2b). So Algorihm A executes the step (A2a) only when a vertex on the minimal path of an original tree becomes the root of its subtree.

To record the left-child $x$ instead of the root $1$ with relabeling the vertex $x$ by $1$ in each of (A2a)'s means to exchange $x$ and $1$ sequentially according to the minimal path from the root $1$ of the original tree $T$.

It is clear that all vertices in the minimal path in a tree become the right-to-left minimums in a permutation under $\omega$. So each right-to-left minimums is recorded in the position of the previous right-to-left minimums in a permutation obtained from a tree by $\omega$. Since all elements are decreased by 1 in the last step, it satisfies (11), then Algorithm A follows $\varphi \circ \omega$.

5.2.   A new description of Gelineau et al.'s bijection $\psi: {{{\mathcal{DU} }}_{n,k}}\to {{\mathcal{T}}_{n,k}}$

The bijection $\psi$ between ${{{\mathcal{DU} }}_{n,k}}$ and ${{\mathcal{T}}_{n,k}}$ was constructed as a composition of two bijections via the set $\mathcal{ES}_{n,k}$ of encoding sequences in [11]. In this section, we just give directly another description of this bijection $\psi$ from ${{{\mathcal{DU} }}_{n,k}}$ to ${{\mathcal{T}}_{n,k}}$, which does not use encoding sequences.

Given an increasing 1-2 tree $T \in T_n$, by convention, if a vertex $x$ of $T$ has two children $u$, $v$ with $u<v$ then $u$ is the left child and $v$ is the right child. By convention, if $x$ has only one child then it is the left child of $x$.

Algorithm B. Gelineau et al. described the bijection $\psi$ between alternating permutations and increasing 1-2 trees using the following algorithm. Due to, for $n = 1$ or $2$,

we can define trivially $\psi$. For $n\ge 3$, given $\pi \in {{{\mathcal{DU} }}_{n,k}}$ ($k = \pi_1$), we define the mapping $\psi:{{{\mathcal{DU} }}_{n,k}} \to {{\mathcal{T}}_{n,k}}$ recursively as follows:

(B1) If $\pi_2 = k-1$, then define $\pi' = \pi'_1\pi'_2 \dots \pi'_{n-2} \in {{\mathcal{DU} }}_{n-2,i-2}$ by deleting $k-1$ and $k$ from $\pi$ and relabeling by $[n-2]$ where $i> k$, that is, for all $1\le j \le n-2$

We get $T' = \psi(\pi') \in {\mathcal{T}}_{n-2,i-2}$. Relabel $T'$ by $\left\{{1,\dots,k-2,k+1,\dots,n}\right\}$ keeping in the order of labels, denoted by $T''$. Let $m$ be the smallest vertex greater than $k$ in the minimal path of $T''$ and $\ell$ the parent of $m$ in $T''$. Then insert a vertex $k-1$ in the middle of the edge $(\ell,m)$ and graft $k$ as the left-child of $k-1$.

We get the tree $T = \psi(\pi) \in {{\mathcal{T}}_{n,k}}$.

(B2) If $\pi_2<k-1$, then define $\pi' = (k-1\; k)\pi \in {{\mathcal{DU} }}_{n,k-1}$ by exchanging $k-1$ and $k$ in $\pi$. We get $T' = \psi(\pi') \in {\mathcal{T}}_{n,k-1}$.

(a) If $k$ is a not sibling of $k-1$ in $T'$, then we get the tree $T = \psi(\pi) \in {{\mathcal{T}}_{n,k}}$ exchanging the labels $k-1$ and $k$ in $T'$.

(b) If $k$ is a sibling of $k-1$ in $T'$, then we get the tree $T = \psi(\pi) \in {{\mathcal{T}}_{n,k}}$ modifying as follows:

Algorithm C. We define another bijection $\psi' : {{{\mathcal{DU} }}_{n,k}} \to {{\mathcal{T}}_{n,k}}$ by the following algorithm. Given $\sigma = \sigma_1 \dots \sigma_n \in {{{\mathcal{DU} }}_{n,k}}$, denote by $d_i(\sigma) = (\sigma_{2i-1}, \sigma_{2i})$ with $\sigma_{2i-1} > \sigma_{2i}$ for $1 \le i \le m$, where $m = \lfloor (n+1)/2\rfloor$, but $d_{m}(\sigma) = (\sigma_{n})$ if $n$ is odd. We shall construct a series of trees $T^{(m)}, \ldots, T^{(1)}$ by reading the pairs $d_m(\sigma)$, $\ldots, d_1(\sigma)$ in this order.

If $n$ is odd, so $d_{m} (\sigma) = (\sigma_n)$, define $T^{(m)}$ to be the tree with only root $\sigma_n$. If $n$ is even, so $d_{m}(\sigma) = (\sigma_{n-1}, \sigma_{n})$ with $\sigma_{n-1} > \sigma_{n}$, define $T^{(m)}$ to be the tree with the root $\sigma_{n}$ and its left child $\sigma_{n-1}$. We note that the vertex $\sigma_{2m-1}$ is the minimal leaf of $T^{(m)}$.

For $1 \le i \le m-1$, assuming that we have a tree $T^{(i+1)}$ of which the minimal leaf is $\sigma_{2i+1}$, read $d_i(\sigma) = (\sigma_{2i-1}, \sigma_{2i})$. As $\sigma_{2i} < \sigma_{2i+1}$, the smallest vertex, say $a^{(i)}$, greater than $\sigma_{2i}$ in the minimal path of $T^{(i+1)}$ is well-defined. By removing all left edges from the increasing 1-2 tree $T^{(i+1)}$, we have several paths, each connected component of which is called a maximal path consisting only right edges.

(C1) If $a^{(i)} < \sigma_{2i-1}$, the largest vertex, say $b^{(i)}$, less than $\sigma_{2i-1}$ in the maximal path from $a^{(i)}$ of $T^{(i+1)}$ is well-defined. For some $j\ge 1$, let $(v_1, v_1, \ldots, v_j)$ be the path from $a^{(i)}$ to $b^{(i)}$ with $v_1 = a^{(i)}$ and $v_j = b^{(i)}$. The vertices $v_1, \dots, v_{j-1}$ should have left child $u_1, \dots, u_{j-1}$, but $v_j$ may not, with

Decomposing by the maximal path from $a^{(i)}$ to $b^{(i)}$, we write $S_1, \ldots, S_{j}$ the left-subtrees of the vertices $v_1, \ldots, v_{j}$ and $S_{j+1}$ the right-subtree of $b^{(i)}$. Since each of $u_1, \dots, u_{j-1}$ lies on each of $S_1, \dots, S_{j-1}$, $S_1, \dots, S_{j-1}$ should not be empty, but two trees $S_{j}$ and $S_{j+1}$ may be empty. Then, the tree $T^{(i)}$ is obtained from $T^{(i+1)}$ by the following operations:

● Graft $\sigma_{2i}$ so that $a^{(i)}$ is its left-child;

● Flip the tree at vertex $a^{(i)}$;

● Transplant the trees $S_1, \ldots, S_{j+1}$ as right-subtrees of the vertices $\sigma_{2i}$, $a^{(i)}$, $v_2, \ldots, v_{j-1}$, $b^{(i)}$;

● Graft $\sigma_{2i-1}$ as the left-child of $b^{(i)}$.

We can illustrate the above transformation by

(C2) If $\sigma_{2i-1}<a^{(i)}$, then $b^{(i)}$ does not exist. Let $S$ be the subtree with root $a^{(i)}$ of $T^{(i+1)}$, then the tree $T^{(i)}$ is defined as follows.

● Graft $\sigma_{2i}$ so that $a^{(i)}$ is its right-child;

● Transplant the trees $S$ as the right-subtree of the vertex $\sigma_{2i}$;

● Graft $\sigma_{2i-1}$ as the left-child of $\sigma_{2i}$.

We can illustrate this transformation by the following

We note that the vertex $\sigma_{2i-1}$ is the minimal leaf of $T^{(i)}$ for $1 \le i \le m-1$. And then, we define $\psi'(\sigma) = T^{(1)}$.

Example. We run the new algorithm to the examples $\sigma = 748591623$ in Example 3.2 and Fig. 2 in [11]. As $n = 9$, we have five pairs

By Algorithm C, we get five trees sequentially

with

Thus, the increasing 1-2 tree $T^{(1)}$ obtained from $\sigma$ under $\psi'$ is the same with the tree obtained from $\sigma$ under $\psi$ in Example 3.2 and Fig. 2 in [11].

Theorem 17. The two bijections $\psi$ and $\psi'$ from ${{{\mathcal{DU} }}_{n,k}}$ to ${{\mathcal{T}}_{n,k}}$ are equal.

Proof. It is clear that (C2) is equivalent to (B1). Since the rule (B2a) just exchange two labels, but does not change the tree-structure, it is enough to show that (C1) is produced recursively from (B1) and (B2b).

Assume that $\sigma_{2i-1} > a^{(i)} > \sigma_{2i}$ for some $1 \le i \le m-1$. We obtain the right tree in the following by applying (B1) to the tree $T^{(i+1)}$.

Due to $\sigma_{2i-1} > v_1$ $( = a^{(i)})$, it is not an increasing 1-2 tree and we apply (B2b) to the above tree as follows.

Since $\sigma_{2i-1} > v_j > \dots > v_2$, until we have an increasing 1-2 tree, repeat to apply (B2b) as follows.

Since (C2a) is produced from the rule (B1) and (B2b), then Algorithm C follows Algorithm B.

Acknowledgments

The first author's work was supported by the National Research Foundation of Korea (NRF) grant funded by the Korea government (MSIP) (No. 2017R1C1B2008269).