1.
Introduction
Nowadays, one of the most widely known fruit plants and favorite among people is strawberry (Fragaria × ananassa Duch.). Strawberry becomes a favorite fruit because of its color, the shape and the taste of the fruit on top of its healthy nutritional content (antioxidant and phenolic compounds) which have effects on human body such as prevention of various infections [1,2]. Strawberry is a and because of its short production cycle, it has become the fastest-growing crop in Asian tropical and subtropical regions. Accordingly, many people develop this crop as one of the farm products. Strawberries are grown on 244 thousand hectares in 73 countries and are among the highest yielding fruit crops. In Indonesia, strawberry distribution is found in the high areas of Java, Sumatra, Bali, Sulawesi and East Nusa Tenggara. It is estimated that there are more than 30 varieties of strawberries cultivated in Indonesia such as Camarosa, Sweet Charlie, Rosalinda, and Earlibrite [3,4]. However, planting strawberries in Indonesia has constraints due to climate change factors, including drought.
Strawberries are crops which require extreme water and are vulnerable to drought. Drought has an impact on the plant growth and development [5,6,7,8]. Drought had significantly impacted changes in plant morphology, physiology and biochemistry. Under this condition, stomata will be closed hence decreasing the leaf conductance (gs). Furthermore, this will reduce the CO2 availability in plants and decrease in net photosynthesis (A) under mild and moderate water stress conditions. Besides, the reduced leaf gas exchange properties under this condition decline in carbon assimilation in plants, ultimately influencing the photoassimilates supply in the plants. If the carbon assimilation yield declines, the development of the vegetative phase and the reproductive organs are also inhibited. Previous studies have also showed that the water deficit will limit plant growth, particularly during the vegetative and reproductive stages [9,10,11,12,13]. Water deficit is also a major factor limiting growth, stomata conductance and net photosynthesis of strawberry plants [14]. The results of research conducted by [15] reported that anthocyanin content was enhanced in strawberry under water stress conditions. Also, in the Barbados cherry genotypes, the free proline (genotype 13-CPA) in the roots was also increased by 36, 4% under 75% water field capacity.
Although several research have reported about drought stress on strawberries, still there is not enough information about the influence of drought stress on strawberries cultivars in Indonesia. Therefore, in overcoming the impact of drought stress in strawberry plants, research is needed to investigate thoroughly the physiological and the biochemical damage of drought stress on strawberries cultivars in Indonesia. The research would provide deeper and more detailed understanding of the plant reaction to overcome the drought stress problem through understanding the optimum water usage for this plant that will not affect the vegetative and the reproductive stages. With that, the study aims to determine the growth, the biochemical changes and the leaf gas exchange of strawberries cultivars (Earlibrite, California, and Sweet Charlie) exposed to drought stress and also to discover the best strawberry cultivar that thrives under drought stress conditions.
2.
Materials and methods
2.1. Planting and treatments of plants
The experiment was handed out in Indonesian Citrus and Subtropical Fruits Research Institute (ICSFRI), Batu, East Java, Indonesia (950 m above sea level, Latitude 7" 54' 41.4" (7.9115"5 south, Longitude 112" 32' 20" (112.5389") east) from July-November 2018. Strawberry runner (one plant per pot) was grown in plastic pots containing 1.5 kg of soil, cocopeat, charcoal husk and goat manure (3:2:2:1, v/v/v/v) mixture. The pots were held under a screen house, watered every day in the morning. After two weeks, a factorial experiment with RCBD (Randomized Completely Block Design, three replications) was conducted with four water deficit levels [W1 = 100% field capacity (FC)/well-watered), W2 = 75% of FC (mild WD), W3 = 50% of FC (moderate WD), and W4 = 25% of FC (severe WD)] for three strawberry cultivars (C1 = Earlibrite, C2 = California and C3 = Sweet Charlie).
2.2. Plant growth characteristics
Measurements of plant growth such as plant height, leaves number, crown diameter, canopy length, and leaf area [16,17]. The height of plant was determined by cupping all the leaves each other, then measured from the bottom of the crown to the highest end of the leaf using a ruler. The number of leaves was calculated by counting total leaves per plant from the appearance of the first leaf until the end of the research. The crown diameter was obtained by measuring the main stem of strawberry plants using digital caliper. The canopy length was recorded for the east-west and north-south in centimeters with a ruler. The leaf area was obtained with portable leaf area meter (Model MLA-A). Ten leaves per treatment per replication were collected randomly. The average number of leaves was calculated for each plant severally. Measurements plant growth were done biweekly.
2.3. Leaf gas exchange measurement
The measurements of leaf gas exchange were taken two months following the water deficit treatments. The gas exchange parameters containing the transpiration rate, the photosynthetic rate, and the stomatal conductance of strawberry plant were recorded on fully expanded leaves by a portable photosynthetic system (ADC BioScientific LCpro-SD System Serial No.34205) between 09:00–12:00 h. Under these measurements, the transpiration rate, the net photosynthesis and the stomatal conductance were obtained [18].
2.4. Relative water content of leaf (LRWC)
The LRCW was recorded using the leaves from each water deficit treatment. The leaves were cut into discs of uniform sizes and weighed immediately to determine fresh weight (FW). The entire leaves were floated at 25 ℃ distilled water in the dark for 12 hours, and the surface was left to dry before weighing again to determine the turgid weight (TW). The dry weight (DW) of the leaves was measured 48 hours after they were oven at 80 ℃. The LRCW was calculated using the formula below [19].
2.5. Number of flowers and fruits
The number of flowers and fruits from the same plant during the strawberry season was collected from randomly chosen plants. The results were reported as the total average total amount of flowers and fruits per plant [17].
2.6. Length, diameter, weight and total soluble solid (TSS) of fruit
Determinations of length, diameter and fruit weight were done on average throughout the planting strawberry season. Length and diameter of fruits were presented in centimeter (cm) and the fruit weight was expressed in grams (g). TSS were calculated with a hand refractometer. The strawberry juice was taken and then dropped into a prism refractometer to get the results. The TSS is stated in oBrix [20].
2.7. Destructive chlorophyll content measurements
Fresh leaves (one gram) were chopped into pieces. The extracted pigment was done by grinding the cut tissue in a mortar with glass powder. The homogenate was transferred quantitatively to a Buchner filter with Whatman No.1 filter paper. The filtrate was placed in a 100 mL volumetric flask and filled to a total volume of 100 mL with 96 percent alcohol. A spectrophotometer with two wavelengths (649 and 665 nm) was used to determine the plant extract optical density. The chlorophyll content was determined using the [21] equation, where OD = Optical Density:
2.8. Anthocyanin content determination
Anthocyanin measurements were performed on ripe strawberries. About two grams of strawberries were homogenized with 10 mL ethanol. The solution was put into test-tube then warmed in a water bath at 80 ℃ for 5 minutes. The supernatant was diluted 10× with ethanol. Quantitative analysis of total anthocyanin was performed using the spectrophotometer Shimadzu (UV-1601PC, Japan), based on the technique used by [22]. The supernatants were measured using spectrophotometer at 535 nm, and anthocyanin content was received using the following formula:
where A (absorbance) = 535 nm; MW (molecular weight) = 449.2 g.mol−1; DF (dilution factor) established in D; l = path length (cm); ℇ = 26900 (L × mol−1 × cm−1), and 1000 = variable for converting grams to milligrams.
2.9. Malondialdehyde (MDA) and Proline content determination
Lipid peroxidation was estimated by measuring the malondialdehyde (MDA) level in the leaves described by [23]. 0.5 g of fresh leaf samples was homogenized in 5 mL of 0.1% trichloroacetic acid (TCA). The homogenate was centrifuged at 10,000 rpm for 5 min at 4 ℃. The supernatant (0.3 mL) was mixed with 0.5% thiobarbituric acid (TBA, 1.2) prepared in TCA 20%, and incubated at 95 ℃ for 30 min. After stopping the reaction in an ice bath for 5 min, samples were centrifuged at 10,000 rpm for 10 min at 25 ℃. The supernatant absorbance at 532 nm was then measured using spectrophotometer Shimadzu (UV-1601PC, Japan).
The method used to determine proline content was using bates Leaf sample 0.5 g was extracted in sulfosalicylic acid 3% 10 mL. Then the sample was filtered with filter paper (Whatman). The filtrate was taken as much as 2 mL, added with ninhydrin and 100% glacial acetic acid 2 mL. The samples were heated in water bath 100 ℃ for 1 hour. The process was halted by soaking the samples in ice liquid for 15–20 minutes. Added with toluene 4 mL and stirred with the test-tube for 15–20 seconds. Let stand the samples until the toluene phase was separated from the sample solution phase. The toluene phase was determined by using spectrophotometer Shimadzu (UV-1601PC, Japan) at absorbance 520 nm. Proline levels are expressed in µmol.g−1 [24].
2.10. Statistical and correlation analysis
Data were examined using SPSS (statistical software ver. 24) and were performed with analysis of variance (two-way ANOVA). The Duncan Multiple Range Test was used to determine the mean separation between treatment groups (DMRT). Pearson's correlation analysis was used to define the results of the correlation analysis. Differences at p ≤ 0.05 were determined significant.
3.
Results and discussion
3.1. Plant height, leaves numbers, crown diameter and canopy length of strawberry
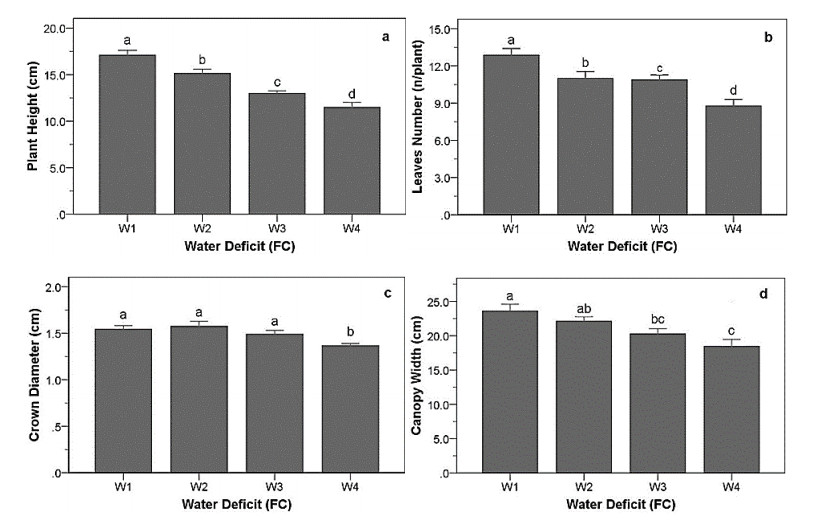
Water deficit treatments were found to have significant effects (p ≤ 0.05) in plant height, number of leaves, canopy width, and, crown diameter of strawberry but there was no significant difference among cultivars and interaction effects between cultivars and water deficit treatment observed on plant height, leaves numbers, crown diameter and canopy width. Generally, the results showed that as the water field capacity reduced from 100% to 25%, parameters including plant height, leaf number, crown diameter and canopy width were reduced (Figure 1.). The obtained results indicate that water deficit is capable of limiting the growth of the strawberry plant. Plant growth inhibition is one of the first reactions to water deficit. In limited irrigation, the plant cell's turgor and water potential decrease, which can enhance the solute's concentration in cytosol and situated outside cell matrices. Finally, cell magnification diminishes relieved to development restraint [25,26,27]. Water deficit could limit the growth of the plant, which can be seen by reduced plant height and leaf number. Another sign of water deficit is reduced development of canopy. It happens before the closure of stomatal and photosynthesis decrement [28]. The findings of this research were in accordance with the study [11,12] which stated that under water deficit conditions, plant height and leaf number were lower than plants cultivated under normal water conditions. This study proved that increasing volume of drought deficit decreased the plant height. The reduced plant height may be due to water stress alleviating the plant cells' water capacity and the turgor that enhances the solutes' concentration in cytosols and extracellular matrices. As a result, cell enlargement declines and this leads to the inhibition of growth [27].
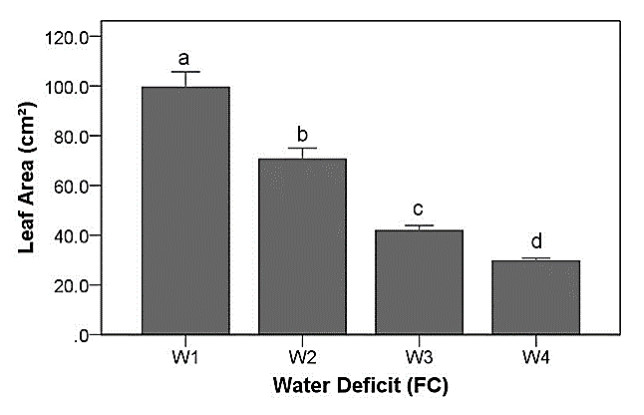
3.2. Leaf area of strawberry
The water deficit diminished the leaf area of strawberry (Figure 2). The result illustrated there was a significant difference (p ≤ 0.05) in leaf area as influenced by water deficit treatments. As the water deficit is enhanced, the leaf area of strawberries tends to decrease. In the present evaluation, the widest leaf was found in the treatment of 100% FC and the smallest leaf was observed in the 25% FC treatment. From Table 1, it was studied that leaf area has a strong positive correlation with length of fruits (r = 0.843; p ≤ 0.05), diameter of fruits (r = 0.839; p ≤ 0.05) and weight of fruits (r = 0.882; p ≤ 0.05), which indicated that enhanced leaf area increases the length, the diameter and the weight of fruits. Therefore, the decline of leaf area in the current study might reduce the fruit qualities under high water deficit treatments.
The reduction in the total leaf area in the present research might reduce division and enlargement of plant cells under water stress conditions. The present study was in agreement with [29,30,31,32], that stated the process of cell growth in plants is very sensitive to water shortages. Deficit water will inhibit the growth process. The limited growth process is one of the reasons for the reduced leaf area in plants. The results of the research by [33] also found that drought stress reduces leaf area in some strawberries cultivars (Elsanta, Elkat, and Salut). [34] informed that adjusting leaf size in plants is the plant's reaction in adapting to environmental stress. The declined of leaf area under water stress was perchance because of the decreased turgor pressure caused the plant cell enlargement to be reduced [35].
3.3. Leaf gas exchange
3.3.1. Photosynthesis rate (A)
Water deficit treatments also had significant effect on photosynthesis (p ≤ 0.05; Figure 3). There was no statistical difference observed on cultivars and interaction between treatments. Irrigation with well-watered (100% FC) had higher photosynthesis than other water deficit treatments but there was no significant difference observed with moderate water deficit treatments (75% FC). Watering at 25% of the field capacity can cause plants to experience water shortages. The photosynthesis rate of strawberry was almost around fourfold less under severe WD compared to well-watered treatment. The current result indicated that photosynthesis had positive significant correlation with plant height (r = 0.808, p ≤ 0.05), leaf area (r = 0.777; p ≤ 0.05), fruit length (r = 0.906; p ≤ 0.05), fruit diameter (r = 0.889; p ≤ 0.05) and fruit weight (r = 0.891; p ≤ 0.05) as shown in Table 1.
In this study, we found that water deficit decreased photosynthesis rate. As we know, photosynthesis has a vital role in plant productivity, for example, in the vegetative growth of strawberry plants. This was proven by [36] who confirmed that water stress inhibited the photosynthesis process in maize. The photosynthesis process inhibition was improved by the disruption of drought stress by the support of the stress. If the process of photosynthesis is inhibited, it can also inhibit plant growth. According to previous studies, if there is lack of water, the turgidity of the guard's cell will decrease [7,37,38,39]. It causes the stomata to close. The closure of the stomata will inhibit the uptake of CO2 needed for photosynthesis. It leads the photosynthesis process to be inhibited. So, water stress can restrict the net of photosynthesis. The findings revealed that there were positive correlations existed between photosynthesis and plant height (Table 1). It means that high net photosynthesis could enhance plant growth and fruits size in the strawberry plant. The results were supported by [40] that stated acceleration of photosynthesis supported the growth of the plant in dry weight of leaf and leaf area, leading to enhanced weight, number of fruits and yield of strawberry.
3.3.2. Transpiration rate (E)
The rate of transpiration was significantly affected by water deficit. (p ≤ 0.05). There was no significant difference observed on cultivars and interaction between treatments. The transpiration rate results on irrigation with well-watered (100% FC) had no significant difference observed with moderate water deficit treatments (75% FC) but had significant difference with severe water deficit (25% FC). As shown in Figure 4, the transpiration rate decreased with enhancing water deficit level. The transpiration rate for 25% FC resided lower for this experiment.
The transpiration rate is reduced with the rising water deficit level. This result is in line with the research conducted by [41]. The result of the study found that the irrigation given to apple plants influences the rate of transpiration. Water deficit will limit the transpiration rate to about 66% compared to plants grown at 80–100% FC. According to [42], plants adjust the interaction between water and transpiration as an adaptation to drought stress via stomatal modifications in order to increase CO2 uptake. These systems also limit water loss by lowering the transpiration rate.
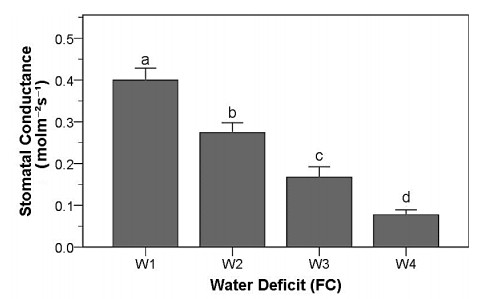
3.3.3. Stomatal conductance (gs)
Figure 5. shows the impact of water deficit treatments on stomatal conductance. There was significant difference (p ≤ 0.05) in the values of gs between water-deficit treatments. Generally, as water deficit increases, the stomatal conductance tends to decrease. The stomatal conductance result from 25% of FC treatments was the lowest among other treatments. The reduction in stomatal conductance is an indication of plant acclimatization to adapt to extreme environments such as water deficit conditions. In the research study, it was reported that stomatal conductance had significant positive correlation with net photosynthesis (r = 0.857; p ≤ 0.05), number of flowers (r = 0.841; p ≤ 0.05), number of fruits (r = 0.822; p ≤ 0.05), length of fruit (r = 0.841; p ≤ 0.05), diameter of fruit (r = 0.804; p ≤ 0.05), weight of fruit (r = 0.826; p ≤ 0.05) as shown in Table 1.
Plants usually manage their stomatal conductance under drought conditions to reduce or even avoid the negative effects of water deficit. Drought increases stomatal constraints as the first action to manage water stress. This constraint leads to the reduction of photosynthetic parameters. The minimal availability of groundwater will cause stomatal conductance to decrease and disrupt gas exchange. Specifically, in leaves, stomata serve as a place for exchanging CO2 and O2 gas. So, closing the stomata limits the concentration of CO2 and O2 in photosynthesis [6,43,44,45] In this research, it was observed that stomatal conductance had significant positive correlation with net photosynthesis. So, it can be presumed that increasing in gs might enhance the quality of strawberries. These results were in line with research by [46] that stated stomatal conductance (gs) correlates intimately and positively with yield in Iriticum aestivum L. (r = 0.94, p < 0.01).
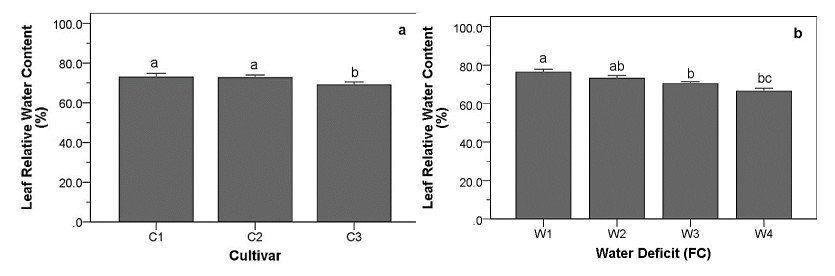
3.4. Leaf relative water content (LRCW)
The result of LRCW observed in this research showed a significant difference (p ≤ 0.05) between cultivars and water deficit treatments. Still, there was no interaction between cultivar and water deficit treatment in LRCW of strawberry. In Figure 6a, we can find that Earlibrite had higher LRCW than other treatments but no difference with LRCW of California. Sweet Charlie had the lowest LRCW. The water deficit treatment also reduced LRCW (p ≤ 0.05). The LRCW of strawberry plants significantly decreased with increasing water deficit. In field capacity conditions, 100% FC had LRCW higher than other treatments. The smallest LRCW was observed in 25% FC treatment (Figure 6b).
The LRCW of strawberry plants reduced significantly as the water deficit enhanced. The outcome of the study by [47] indicated that LRWC is an important element in plants that has physiological function and also as an indicator of drought conditions in plants. As the level of water deficiency rises, the RCW decreases significantly. The LRCW observation was also reported by [48] in ndhB-inactivated tobacco (cv. Xanthi) mutants. [34] found a comparable drop in leaf RWC in Asteriscus maritimus cultivated in a water-stressed environment. One of the first steps toward drought resistance in plants is osmotic adjustment, which necessitates a reduction in leaf RWC [49].
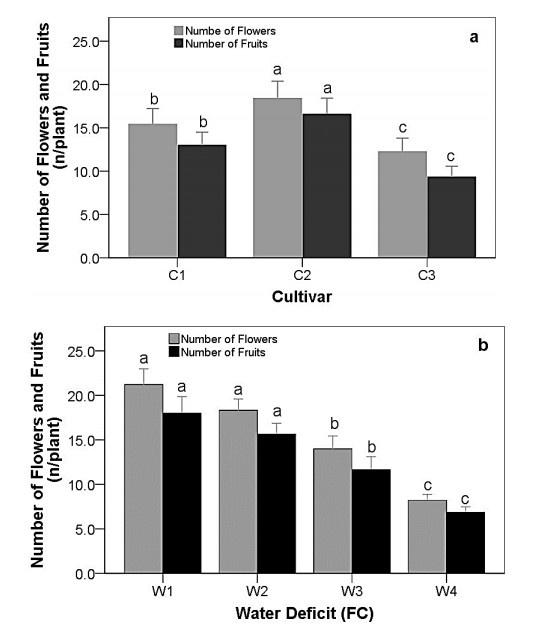
3.5. Number of flowers and fruits
Cultivars and water deficit influenced the number of flowers and fruits. However, there was no interaction (p ≤ 0.05) between treatments in number of flowers and fruits of strawberry. The total flowers and fruits in California strawberry were higher than other cultivars. Sweet Charlie had the lowest number of flowers and fruits plant−1. The numbers of flowers were found 16.2% (Earlibrite) and 33.3% (Sweet Charlie) which lower than California. Total fruits were reduced by 21.5% and 43.5% for Earlibrite and Sweet Charlie, respectively (Figure 7). Hence, California might have better tolerance in higher water deficit environments compared to other cultivars studied. Field capacity conditions can produce the optimal number of flowers and fruits. In the 75% FC condition, the number of flowers and fruits decreased but not significantly different from the results of field capacity. However, with decrease in water by 50% FC and 75% FC, the flowers and fruits number had greatly reduced. The result showed that the number of flowers and fruits under water deficit conditions were significantly reduced. The number of fruits produced in this study was related to the number of flowers. This is indicated by significant positive correlation between the numbers of flowers and fruits in this study (r = 0.974; p ≤ 0.05; Table 1.).
The study evidenced that the number of flowers and the number of fruits under water deficit conditions was significantly declined. Water stress negatively impacts the vegetative and flowering stages because of the reduced chlorophyll a, chlorophyll b, and total chlorophyll [50,51]. According to [52,53], water deficit had significantly influenced the number of fruits. The number of fruits in strawberry plants from water deficit treatment was statistically reduced by 29.23% contrasted to the control (no water deficit). There was still strong correlation between the numbers of flowers and fruits in this study. This study has shown the number of fruits increased when the number of flowers enhanced. This result is supported by the opinion of [54] which stated that the number of flowers was the variable that determined the number of fruits.
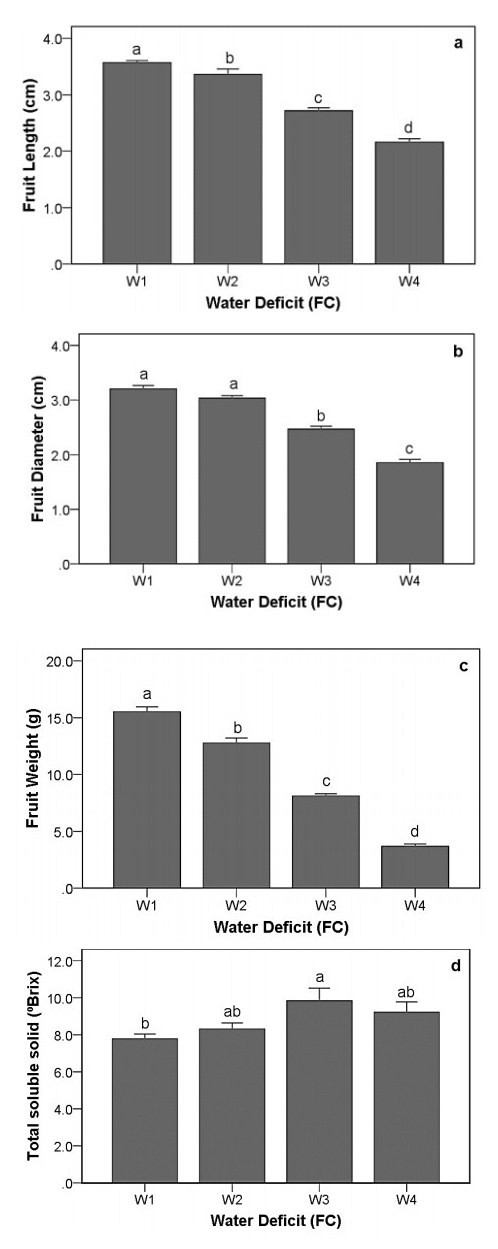
3.6. Length, diameter, weight, and total soluble solid of fruit (TSS)
Figure 8. presents the effect of water treatments on length, diameter, weight and total soluble solid of strawberry fruits. The results obtained confirmed that there was no statistically significant difference (p ≤ 0.05) in length, diameter, weight and total soluble solid of fruit among cultivars of strawberry fruits. However, under water deficit treatment, there was significant difference (p ≤ 0.05) among water deficit treatments but no interaction between cultivar and water deficit treatment in length, diameter, weight and TSS of fruit among cultivars of strawberry fruits. The current study observed water deficit significantly reduced the length, the diameter and the weight of fresh strawberry fruit but significantly increased the TSS. Length, diameter and weight of fresh fruit were decreased by 75%, 50%, and 25% of FC treatments as compared to field water capacity treatment. The highest TSS was in the treatment of 50% FC. The lowest TSS was in the well-watered treatment. The TSS of 100% FC was 6.2%, 24.1% and 18.2% higher respectively compared to 75%, 50% and 25% FC.
The outcomes of this experiment revealed water deficit decreased the length, diameter and weight of fresh strawberry fruit but increased the TSS significantly. The result is in line with research from [15] that showed the yield and the fruit weight in cultivars of strawberry observed under water stress conditions were significantly decreased. The mean weight of fruit under stress was smaller than that under control conditions. Yield per unit showed a decrease area under water stress conditions as compared to the control. [55] also reported the same result in kiwi fruit, also the TSS content was increased under stress full conditions. Water deficit has a substantial impact on fruit quality features such as total soluble solid. The effect of water stress treatment on TSS in citrus orchards [56] and in tomatoes [57] lead to increase in TSS. The increase in soluble solids is due to an increase in phloem sat and a decrease in its flow. [58] discovered that rising soluble solid concentration was mostly related to decreases in fruit water content and small increases in soluble sugar accumulation.
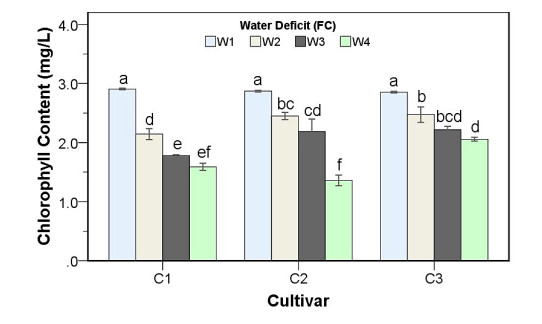
3.7. Total Chlorophyll Content (TCC)
The interaction among varieties and water deficit significantly influenced the TCC of strawberries (Figure 9; p ≤ 0.05). It was observed that all three varieties obtained the highest TCC under 100% FC. However, as the water field capacity reduced to 25%, Sweet Charlie strawberry retained the highest TCC (2.06 mg. L−1). Under 25% FC California strawberry recorded the lowest TCC (1.36 mg. L−1). The TCC tends to decrease under lower field water capacity. Table 1. demonstrates a strong positive correlation between chlorophyll content and length of fruits (r = 0.765; p ≤ 0.05), diameter of fruits (r = 0.836; p ≤ 0.05) and weight of fruits (r = 0.851; p ≤ 0.05). This indicates that TCC also influenced the quality of fruit in the present study. In lower water field capacity, the TCC tends to decrease. This result was similar to the report by [59] that observed water deficit significantly decrease total chlorophyll in the leaf of Matricaria chamomilla L. Studies by [60] mentioned that TCC declined significantly under severe water stress due to destruction of chlorophyll content under water deficit. Water deficit also dropped the chlorophyll content in peanut leaves (Arachis hypogaea) accession number ICGV 91114 [61]. Water stress repressed chlorophyll synthesis and decreased the element of chlorophyll-binding proteins, leading to a decline in the light-harvesting pigment-protein complex related to photosystem Ⅱ. The reduction in chlorophyll is primarily the effect of destruction to chloroplasts caused by the reactive oxygen species (ROS) affected by drought stress [62,63,64].
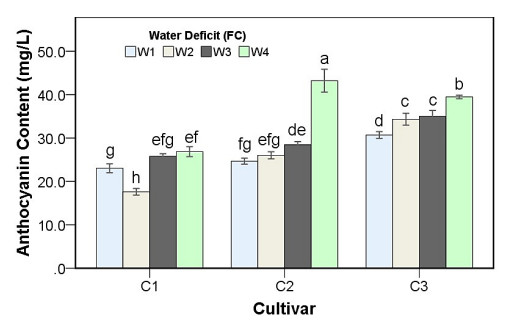
3.8. Anthocyanin content
In strawberry fruit, there was significant interaction (p≤0.05) between cultivar and water deficit treatment on anthocyanin content (Figure 10). Generally, in the three cultivars, as field capacity conditions reduced, the anthocyanin content seems to be increased. The anthocyanin content was the highest in C2W4 (43.2 mg. L−1) and the lowest was in C2W2 (17.6 mg. L−1). Anthocyanin are the so plentiful flavonoid compound in fruit of strawberry and also mainly play a part into the bright red color of strawberry fruit [65,66,67]. The results in this study are in accordance with research from [68] which shows that the anthocyanin content in water shortage (WD) condition in the samples of strawberries were higher than water supply (WS) condition. In WD condition, anthocyanin contents were higher than that of WS by 66.8%. The same findings were also observed by our findings which in concordat with those notified by [69] that observed total anthocyanin in inner part of strawberry fruit was increased by 35.1% under water stress condition. Drought shows to be supported to promote the flavonoid production pathway. [70]. This is most likely due to the increase of flavanone 3 hydroxylase and chalcone synthase activity (key enzymes in the pathway of flavonoid), resulting in greater concentrations of total anthocyanin content in water-stressed conditions [71].
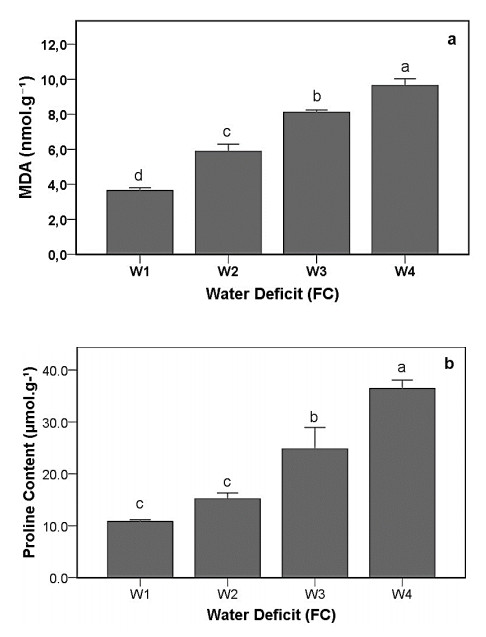
3.9. Malondialdehyde (MDA) and Proline content
There was a significant difference (Figure 11; p ≤ 0.05) between the mean MDA of water deficit treatment. There was no significant difference observed on cultivars and interaction between treatments. The MDA of strawberry leaves progressively enhanced as the water deficit levels raised, with 25% WFC having the highest MDA, followed by 50% WFC and 75% WFC, respectively. The lowest MDA value was in 100% WFC. Furthermore, it found that water stress also had significantly effect on proline content of strawberry (Figure 11; p ≤ 0.05). However, no significant difference in cultivar treatments and also there was no interaction among cultivars and water deficit treatments. In this study, proline content increased in the leaves of strawberry plants under water deficit conditions. Proline content was increased by 40.2%, 128.7% and 236.1% in 75% FC, 50% FC and 25% FC, respectively. Proline content increased under water deficit conditions.
The formation of MDA was considered as a measure of lipid peroxidation that was induced by a high water stress level [72]. Water stress-induced severe lipid peroxidation because ROS removed hydrogen from unsaturated fatty acids, resulting in lipid radical formation, which runs to the formation of alkenes, lipids, and aldehydes, all of which demolish the structure of lipid [73]. The results in this study are in line with research from [74] and [75] which shows that The number of MDA in the leaves of pea (Pisum sativum L.) and genus Avena was raised due to water stress. When plants are exposed to water stress conditions, ROS production will overwhelm the scavenging action of the antioxidant system, ensuing in cell damage and death [76]. MDA is produced in drought-exposed plants in response to ROS, to evaluate the damage of plasma membrane and tolerance to drought stress [77]. Separately from MDA, Enhanced proline content in strawberry plants under severe drought conditions is a common physiological to various stress disorders. Under water deficit conditions, the proline accumulation was enhanced compared to the control plant in the Frankenia [78]. Proline accumulation suggests that there may be a tolerance mechanism to prevent dehydration by adjusting the osmotic pressure resulting in water retention. The solubility of protein molecules can be related to the presence of proline, which keeps them from denaturation under drought stress. As a result, proline production was regulated under water [79,80,81,82,83].
4.
Conclusions
The current study showed that water deficit had significant influences on growth, biochemical changes, and leaf gas exchange of strawberry plants, by 25% FC treatment (severe water deficit) generating a reduced in plant growth, leaf gas exchange, leaf relative water content, number of flowers and fruits, length, diameter, and weight of fruits. Among the cultivars, it was found that California strawberry is more adaptable to water deficit compared to other cultivars by producing more flowers and fruits compared other cultivars. TSS, anthocyanin, MDA, and proline contents were increased in strawberries under water deficit conditions but the treatment of 100% FC (well-watered) had the lowest effect on TSS, anthocyanin and proline contents. Among all water stress treatments, 75% field capacity had the best results to optimize water utilization on the strawberry plants.
Acknowledgements
This research was funded by the (Sustainable Management of Agricultural Research and Technology Dissemination (SMARTD) Program of Indonesian Agency for Agricultural Research and Development no. B-2816/KP.320/H.1/10/2016.
Conflict of interest
The authors declare no conflict of interest in this paper.









 DownLoad:
DownLoad: