1.
Introduction
Calcaneal fractures account for approximately 60% of tarsal bones fractures [1,2] The traditional extended lateral calcaneal approach can be used to clearly expose the posterior articular surface fracture and the lateral wall of the calcaneus for reduction under direct vision. However, the complication rate is as high as 37%, and the infection rate is approximately 20% [1,3]. To reduce the incision complications and the infection rate of calcaneal fractures, the new minimally invasive treatment techniques have emerged successively [4,5,6,7]. However, the minimally invasive techniques have limited exposure and are unable to be used for articular surface reduction under direct vision [8,9]. Potential complications, such as poor articular surface reduction, malunion, and loss of reduction may occur [10,11].
To date, the challenges of minimally invasive calcaneal fracture treatment lie in the following: ① how to improve the reduction rate of the posterior articular surface and improve the shape of the calcaneus; and ② how to improve the accuracy in the placement of the SS, the LAS, and the PASS. The sinus tarsi approach can be used to expose the operative field and to protect the soft tissue. This approach is the current popularized surgical method for the minimally invasive treatment of calcaneal fractures [11,12,13]. During the reduction and internal fixation via the sinus tarsi approach, the sustentaculum tali is an important reference point for the reduction of intra-articular fractures of the calcaneus [14,15]. However, the SS can only be placed indirectly from the lateral side to the medial side [16], the screw trajectory is narrow, and the success rate of SS placement is not high [16,17,18,19].
To improve the reduction rate of the posterior articular surface and the shape of the calcaneus fracture, and the SS, LAS and PASS placement success rate, we proposed a minimally invasive treatment of calcaneus fractures via the sinus tarsi approach based on a 3D printing technique. Currently, the application of 3D printing technology is rarely reported in the field of ankle surgery, especially in the case of the minimally invasive treatment of calcaneal fractures [20,21,22,23].
The aims of the current work were: ① The 3D reconstruction calcaneal model will be obtained, and the optimal screw trajectory will be designed on the calcaneus model to determine the individualized parameters of SP. ② The model of the calcaneus will be printed using 3D printing, and the minimally invasive steel plate will be pre-shaped to fit the lateral wall of the model. ③ According to the determined parameters for SP, minimally invasive surgery of the calcaneal fracture will be performed including fabrication of the steel plate and SP. Finally, postoperative evaluations will be performed to verify the accuracy of the SP.
2.
Materials and methods
The current work was reviewed and approved by the Ethics Committee of the Ningbo No. 6 Hospital. The patients reviewed the written surgical plan and alternative regimen before the procedure and signed the informed consent form.
2.1. Minimally invasive treatment of calcaneal fracture via sinus tarsi approach
The minimally invasive treatment via the sinus tarsi approach is divided into two sections: the reduction of the posterior articular calcaneus surface, and the precise placement of the SS, LAS and PASS (Figure 1).
First, the sinus tarsi approach was used to expose the posterior calcaneus articular surface and to protect the superficial peroneal nerve and sural nerve. Then, the displacement of the calcaneus was reduced by manipulation. Intraoperative fluoroscopy was performed to verify the fitness of the pre-shaped minimally invasive steel plate to the lateral wall of the calcaneus. If the plate fitted, the reduction of calcaneus posterior articular surface and shape was considered to be satisfactory. If it did not fit, the further reduction was required.
Second, the SS, LAS, and PASS were placed precisely. First, the screw entry points and exit points of the SS, LAS, and PASS at the lateral wall of the calcaneus and the calcaneal tuberosity were determined. Next, the angle of the screw insertion and the length of the screw trajectory were determined. Finally, the screws were placed one by one to complete the internal fixation of the calcaneus fracture.
2.2. 3D modeling of the calcaneus and 3D printing
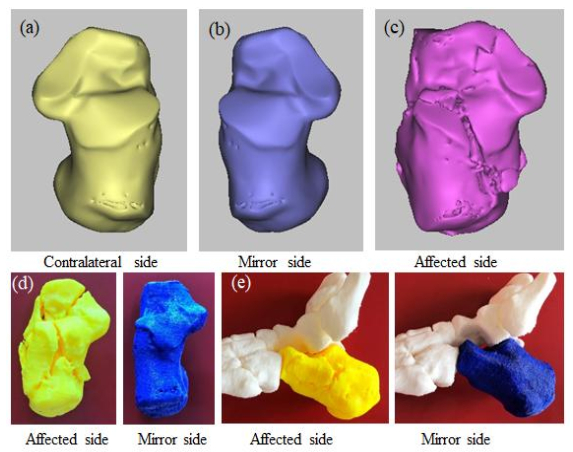
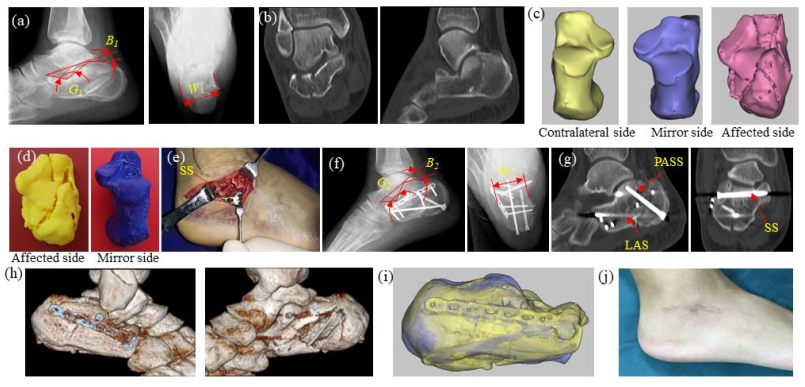
The patient's bilateral calcanei was scanned using CT (Philips Brilliance 64 CT, Philips Medical Systems, Netherlands) with a slice thickness of 1 mm and a slice interval of 0.5 mm. The CT data were imported into MIMICS 15.0 (Meterialise, Leuven, Belgium) for 3D reconstruction. The 3D reconstruction model of the calcaneus is shown in Figures 2a–c.
The STL formatted data of the 3D reconstruction model of the affected calcaneus and the mirror model of the contralateral calcaneus were imported into the Makerbot replicator 3D printing device (Makerbot, USA) to obtain a printed 3D model (Figures 2d, e).
2.3. Surgical design
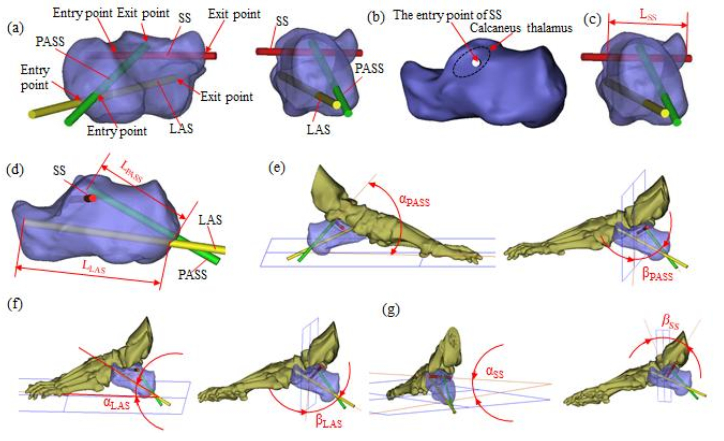
MIMICS software was used to simulate SP on the 3D model of the mirror-image calcaneus.
First, the SS, PASS and LAS entry and exit points were determined. Then, the maximum trajectory lengths, LSS,
LPASS, and LLAS, were determined. The trajectories of SS, PASS, and LAS placement were adjusted individually to ensure no involvement of the subtalar articular surface during SS placement, no penetration of the medial wall and posterior articular surfaces of the calcaneus during PASS placement, and no penetration of the lateral wall and the calcaneocuboid joint during LAS placement. Next, the interference of the three screw trajectories was assessed. If interference was identified, the screw trajectories were adjusted until there was no sign of interference. Finally, the actual SP angles were determined for application during the procedure. The angles between the PASS, LAS, and SS and the horizontal plane and the angles between the PASS, LAS, and SS and the coronal plane were obtained and expressed, respectively, as follows: αPASS and βPASS; αLAS and βLAS;
αSS and βSS (Figures 3a–g).
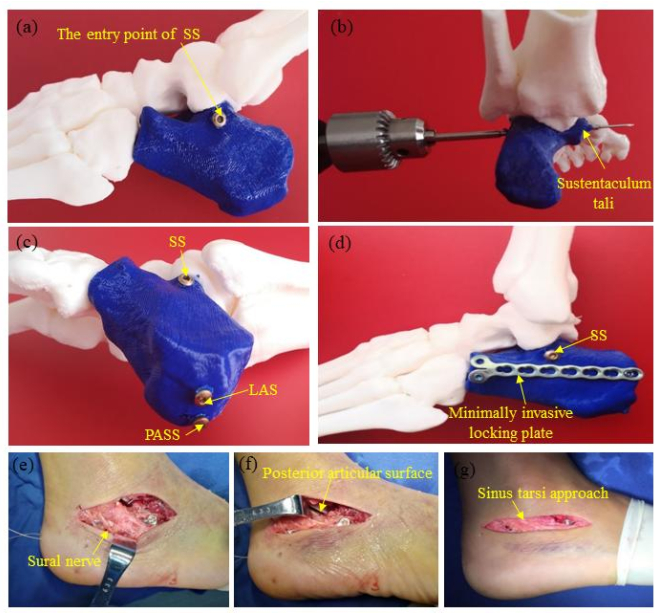
In the 3D printed model, the SS, LAS, and PASS entry points were designed. The simulated procedure was performed according to the depth and angle of the screw insertion determined by the simulated SP. Finally, the minimally invasive steel plate was pre-shaped to fit the lateral wall of the calcaneus and fixed to the model (Figures 4a–d).
2.4. Intraoperative procedure
First, a periosteal stripper was used to manipulate the medial fracture fragment of the calcaneus at the fracture line for reduction. Then, a Kirschner wire was used to transversely cross through the calcaneus tuberosity for lateral traction to correct the calcaneal varus and restore the length of the calcaneus. Finally, the fracture fragment of the lateral wall of the calcaneus was reduced under direct vision and temporarily fixed with the Kirschner wire (Figures 4e, f). The pre-shaped minimally invasive steel plate was placed on the lateral wall of the calcaneus. If it fitted, the reduction of the posterior articular surface and the shape restoration of the calcaneus were considered satisfactory.
The SS, PASS, and LAS were placed in sequence. The pre-shaped minimally invasive steel plate was then fixed to the lateral wall of the calcaneus. Fluoroscopy was used to confirm that the reduction of the posterior articular surface and the shape restoration of the calcaneus were satisfactory and that the force line was restored (Figure 4g). A drainage tube was placed in the incision site. The incision was closed in layers and dressed with a sterile compression bandage.
2.5. Postoperative evaluation
The Bohler's angle (B2), the Gissane's angle (G2), and the calcaneus width (W2) were measured in the lateral and axial view of the heel after surgery. Postoperative CT scans with 3D reconstruction were performed to compare the preoperative and postoperative data to determine the accuracy of the SS, LAS, and PASS placements. Furthermore, the Kurozumi [24] grading system was used to evaluate the articular surface reduction. The patient's incision complications were recorded after surgery, and the patient's the American Orthopedic Foot and Ankle Society (AOFAS) [25] score and visual analog scale (VAS) [26] score were evaluated at the final follow-up visit.
2.6. Application
From June 2016 to February 2017, 25 patients underwent the proposed procedure. The patients were 20 to 59 years of age, with an average age of 43 years (Table 1). Patients with Sanders type-Ⅱ and simple Sanders type-Ⅲ fractures of the calcaneus were included.
The follow-up measurement showed that the Bohler's angle (B2), the Gissane's angle (G2), and the calcaneus width (W2) of the calcaneus in the lateral and axial view of the heel returned to normal after surgery suggesting that the posterior articular surface and shape of the calcaneus returned to normal.
After surgery, the average AOFAS score was 91.5 points (range: 85–100). The average postoperative average VAS score was 1.7 points (range: 0–4 points). Only 2/25 (8%) of the patients had superficial incision infections and recovered after changing the incision dressing, and 2/25 (8%) patients had numbness and discomfort in the lateral margin of the foot and recovered spontaneously approximately 3 months after surgery.
3.
Results
3.1. Typical case of Sanders type-Ⅱ calcaneal fracture
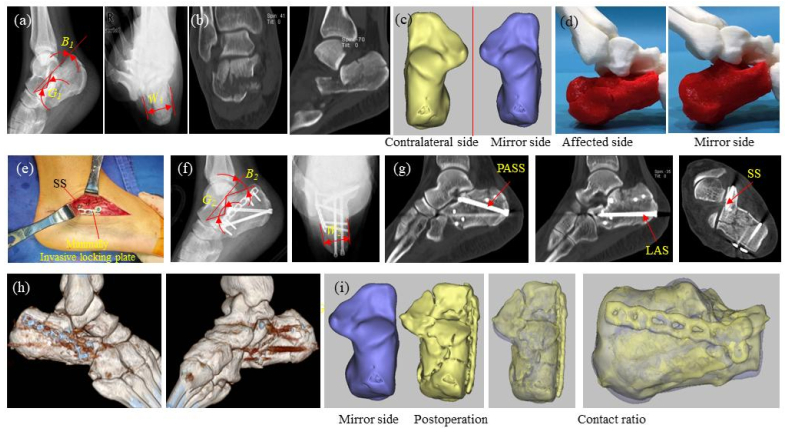
A 46-year-old female presented with a Sander Ⅱ comminuted fracture of the right calcaneus caused by a fall from a height. Simulated SP: (1) the maximum length of each screw trajectory was listed as follows: LPASS = 46 mm, LLAS = 70 mm, and LSS = 46 mm. (2) the angles of the screw insertion were shown as follows: αPASS = 54°, βPASS = 36°, αLAS = 29°, βLAS = 61°, αSS = 8°, and βSS = 16°.
Postoperative fluoroscopy indicated that the size and shape of the calcaneus returned to normal. Postoperative CT showed a good reduction of the subtalar articular surface and accurate placement of each screw. No complications occurred during follow-ups. In the final follow-up visit, the AOFAS score was 94 points, and the VAS score was 2 points. Six months after surgery, the patient had returned to his daily work with well-recovered function (Figure 5).
3.2. Typical case of Sanders type-Ⅲ calcaneal fractures
A 55-year-old female presented with Sanders type-Ⅱ comminuted fracture of the right calcaneus due to fall from a tree. Fluoroscopy showed the collapsed calcaneal articular surface and the widened calcaneus. The simulated surgical plan was given as follows: (1) the maximum length of each screw trajectory was shown the following: LPASS = 48 mm,
LLAS = 75 mm, and LSS = 46 mm. (3) the angles of the screw insertion were listed as follows: αPASS = 48°, βPASS = 33°,
αLAS = 25°, βLAS = 64°,
αSS = 11°, and βSS = 20°.
Postoperative fluoroscopy indicated that the shape of the calcaneus returned to normal. Postoperative CT showed a good reduction of each articular surface and accurate placement of each screw. The patient's incision healed well, and there was no complaint of discomfort after surgery. Comparing the contralateral mirror-image calcaneal model with the postoperative calcaneus model, the axial and lateral contact ratio was high. It was indicated that the shape of the affected calcaneus returned to normal. In the final follow-up, the AOFAS score was 92 points, and the VAS score was 2 points. Five months after surgery, the patient walked freely with well-recovered function (Figure 6).
3.3. CT evaluation
The evaluation of the articular surface reduction showed excellent recovery in 11 patients, good recovery in 9 patients, fair recovery in 5 patients, and poor recovery in no patients. The evaluation of the reduction of the articular surface of the calcaneocuboid joint showed good recovery in 14 patients, fair recovery in 11 patients, and poor recovery in no patients. Only 1 patient (4%) had a loss of reduction of the posterior calcaneus articular surface. The reduction rate of the posterior calcaneus surface was 96%. In the past, 2D conventional radiography was used to evaluate calcaneal fracture. However, CT is the main and effective tool to evaluate calcaneal fracture classification systems. Because CT can demonstrate better characterization and visualization of fragment displacement and fracture lines.
4.
Discussion
Minimally invasive treatment via the sinus tarsi approach is a difficult operation, and the sural nerve is easily damaged during surgery due to the limited exposure in the sinus tarsi incision [20]. The difficulties of the procedure included the restoration of the calcaneus posterior articular surface and the precise orientation of the SS, LAS, and PASS. Here, we proposed a personalized minimally invasive treatment of calcaneal fractures via the sinus tarsi approach using 3D printing technology. Preoperatively, the 3D model of the calcaneus was printed for each individual and used for pre-shaping the minimally invasive calcaneus steel plate and for the surgery simulation. During surgery, the pre-shaped steel plate was used to evaluate the reduction of the calcaneal fracture by fitting the plate to the calcaneus. Here, we improved the SP accuracy, improved the reduction rate of the posterior articular surface, restored the shape of the calcaneus, and increased the precision of the minimally invasive treatment of calcaneal fractures via the sinus tarsi approach.
The screw trajectory was designed on the reconstructed 3D model, and the simulated procedure was performed on the 3D printed model. These steps improved the success rate of SS, LAS, and PASS placement and reduced the iatrogenic injury caused by the failure of SP [27,28]. Accurate insertion of the screw restores and maintains the height, width, articular surface, and force line of the calcaneus to achieve a strong internal fixation [15]. The calcaneus thalamus portion and the calcaneal tuberosity are the structures that include cross-linked trabecular bones, which are hard and can remain intact in calcaneal fractures. Therefore, we chose the posterior-inferior site of the calcaneus thalamus portion as the SS entry point and had the PASS and LAS entry points at the medial and lateral sides of the calcaneus tuberosity, respectively. The SP parameters obtained using the 3D reconstructed model were used to guide the SP during surgery and can greatly improve the accuracy of the SP. At the same time, the length of the guide wire and the maximum length of the screw trajectory were compared during the operation to assess the initial accuracy of the SP. In summary, these preoperative simulations and intraoperative operations guarantee improved success rates in terms of SS, PASS, and LAS placement.
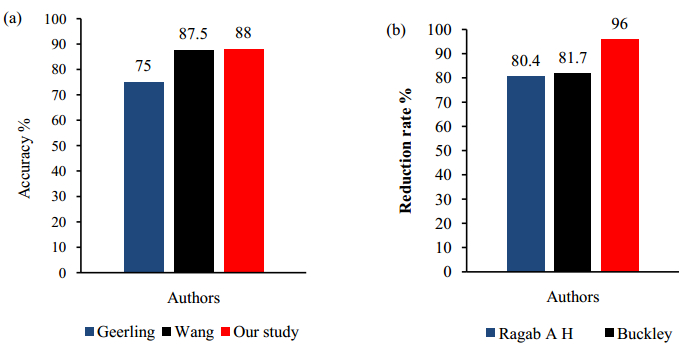
The success rate of SS placement in this study is significantly greater than that in traditional SS placement, as shown in Figure 7. Geerling et al. [29] used 3D image navigation technology to guide SP, and the success rate of SS placement was 24/32 (75%). Wang et al. [18] designed a SS-guiding device to assist SS placement, and the SS placement success rate in 10 cadaver specimens was 35/40 (87.5%). Here, postoperative CT findings showed that in 22 cases, SS were accurately placed in the sustentaculum tali, and in 3 cases, SS were placed in the bone of the base of the sustentaculum tali. No medial vessel, nerve, or tendon injury occurred. In 24 patients, the PASS trajectory was parallel with the lateral wall of the calcaneus and perpendicular to the posterior calcaneus articular surface but did not penetrate the posterior calcaneus articular surface or the medial wall of the calcaneus. In one patient (Case 3), PASS went through the edge of the posterior articular surface of the calcaneus. No treatment was required because the patient had no symptoms. In 23 patients, the LAS were accurately placed in the calcaneus protrusion, and in two patients (Cases 8 and 20), the LAS were placed in the bone below the anterior process of the calcaneus. There was no case of involvement of the lateral bone cortex or the calcaneocuboid joint.
In this study, the reduction of the posterior calcaneal articular surface after calcaneus fracture was satisfactory with a greater posterior calcaneus articular surface reduction rate than when using the traditional method (Figure 7). Zhang et al. [10] compared the sinus tarsi incision with the longitudinal incision and revealed that the postoperative intra-articular steps after treatment via the sinus tarsi incision in patients with Sanders type-Ⅱ and Sanders type-Ⅲ fractures were 1.0 mm and 1.2 mm, respectively. In the study by Buckley et al. [30], postoperative CT was used to measure the posterior articular step and showed the step was no larger than 2 mm in 81.7% of patients. The reduction rate of the posterior calcaneus articular surface reported by Ragab et al. [31] was 37/46 (80.4%). In our work, only 4% of patients had loss of posterior calcaneus articular surface reduction, and there was no loss of reduction in the articular surface of the calcaneocuboid joint. The reduction rate of the posterior articular surface was 96%. Obviously, the reduction rate of the posterior calcaneus articular surface was greater in this study than in previous studies. These results suggest that the pre-shaped minimally invasive steel plate on the personalized 3D-printed model can improve the reduction rate of calcaneal fracture.
Acknowledgements
This work was supported by Australian National Health and Medical Research Council (NHMRC) Fellowship (1158402) and National Natural Science Foundation of China (NSFC) project (81671928), Ningbo Science & Technology Program on Social Development (2017C50048, 2016A22). LPW is supported by NHMRC Fellowship (1158402) and NSFC (81671928).
Conflicts of interest
The authors declare no conflict of interest.










 DownLoad:
DownLoad: