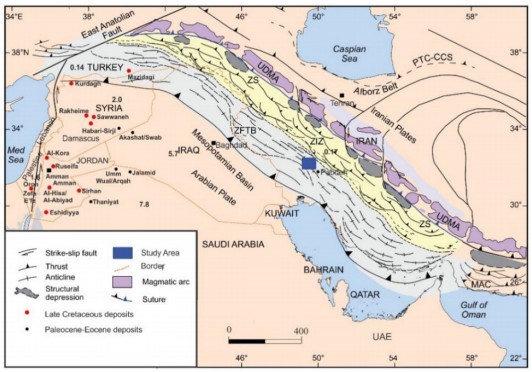
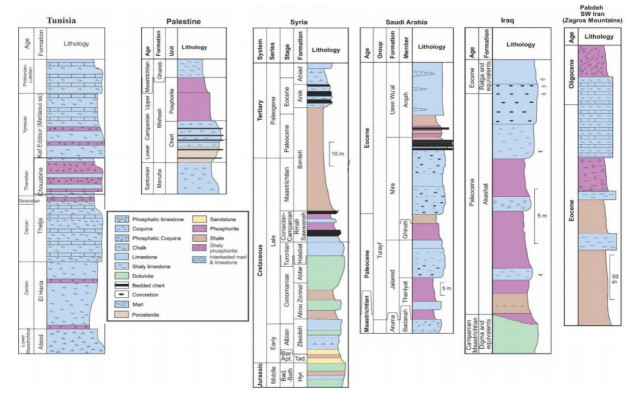
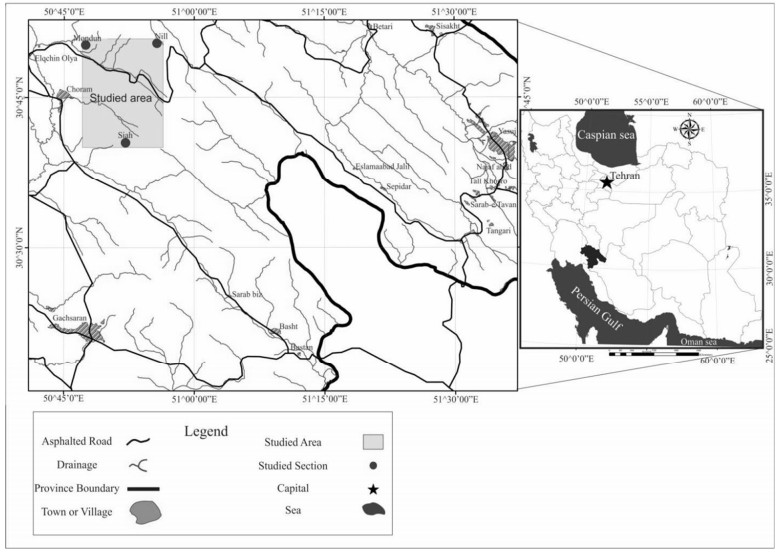
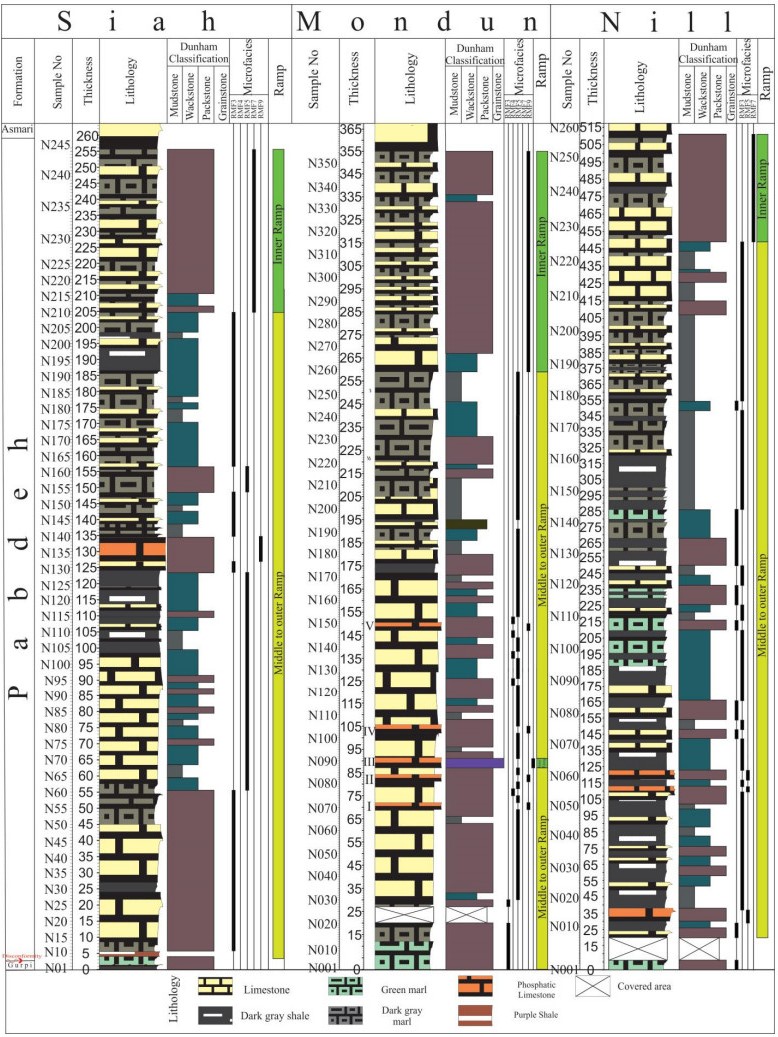
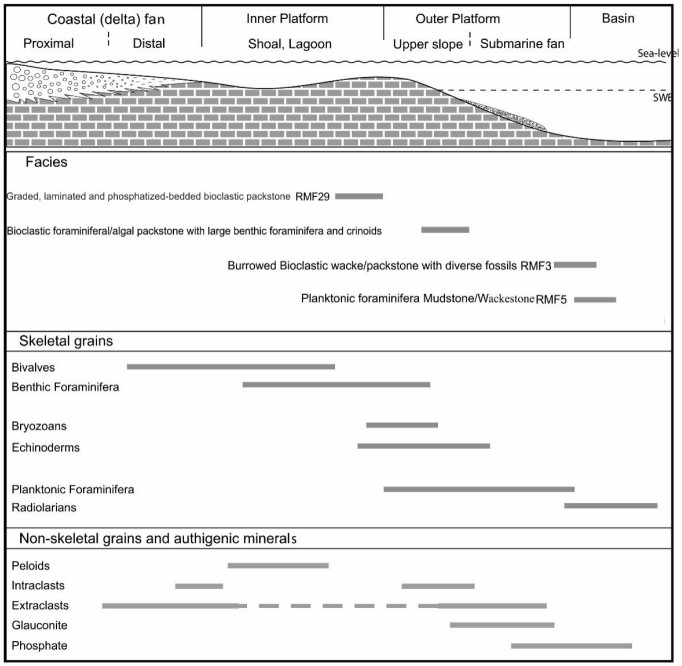
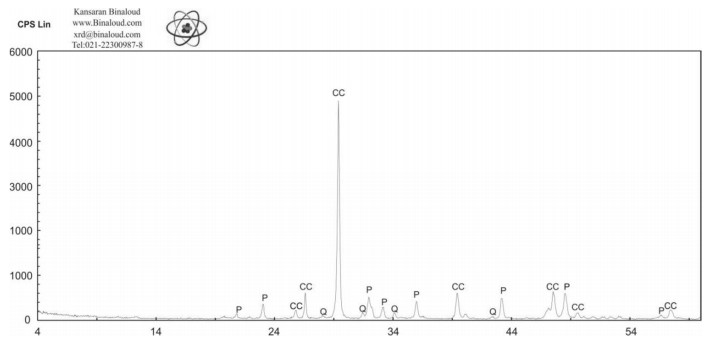
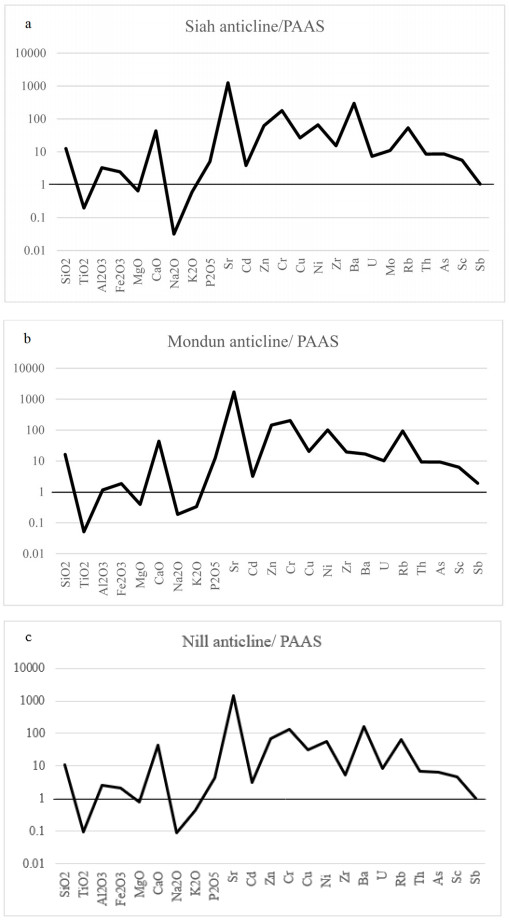
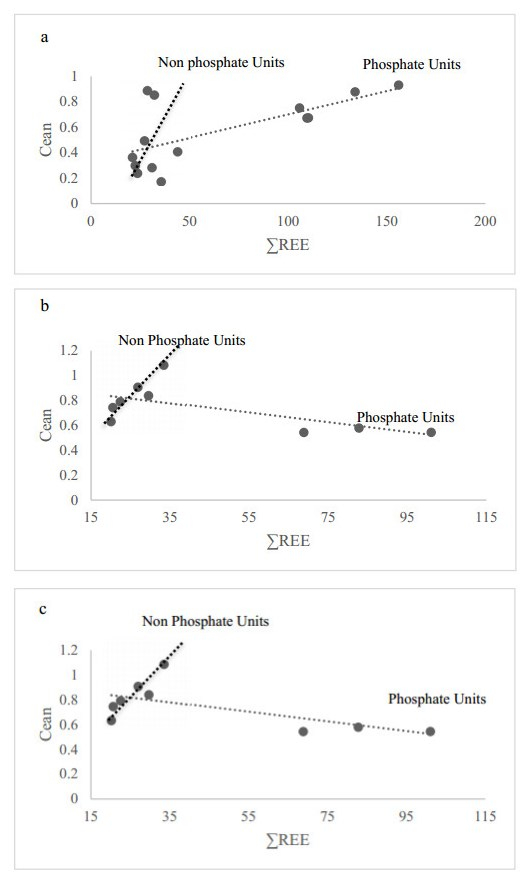
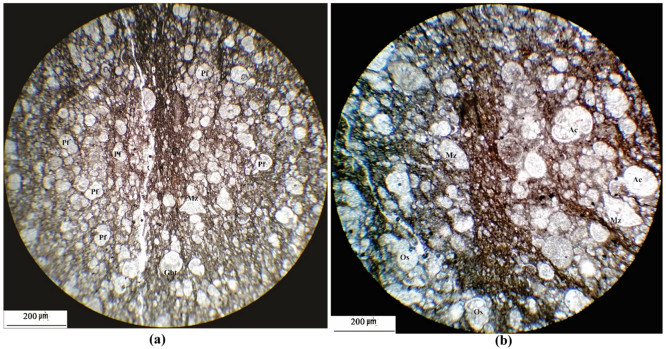
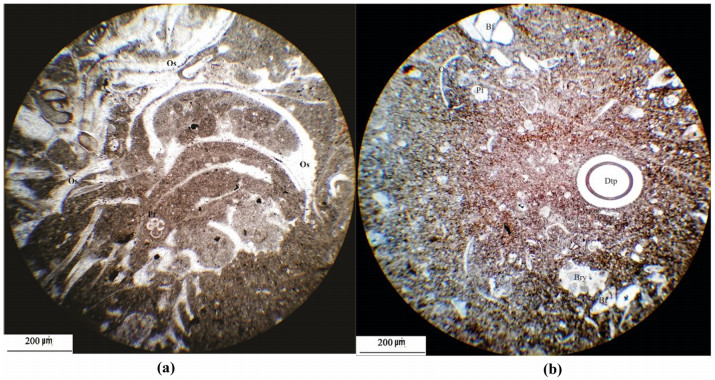
Phosphate deposits in south-western Iran are part of the South Tethyan Phosphogenic Province, a huge carbonate-dominated strata that extends to the Middle East. The Tethyan phosphorites of Iran are dated Eocene-Oligocene (Pabdeh Formation) and categorized as low-grade ore deposits on a global scale. Depositional conditions of the facies indicate that the Pabdeh Formation was deposited on a carbonate ramp setting as a distally steepened ramp. Under such an environment, turbidity currents transported phosphate particles from the back-shoal setting to the deeper middle and outer ramp of the ocean where they were suspended and deposited as shell-lag and phosphate lamination. Microfacies studies demonstrate that all the phosphatic ooids and phosphatized foraminifera, fish scales, bones and phosphatic intraclasts reworked from shallow parts of the Tethyan Ocean to deeper parts with the help of turbidity currents. Analysis and interpretation of the data reveal positive correlation between. REE+Y and P2O5 in all studied sections which attests to their strong coherence as a geochemical group. The shale normalized REE patterns of Mondun phosphorites are characterized by negative Ce anomalies. This anomaly indicate that the depositional environment was oxic and highly reworked, bioturbated with higher energy realm during phosphate deposition, conversely Nill section with Ce enrichment reflect conditions of relatively deeper water sedimentation. These geochemical findings are in accordance with microfacies studies which indicate shallow and high energy condition for Mondun section with negative cerium anomalies and a deep ramp setting for Nill and Siah sections which denote a positive cerium anomalies in REE patterns.
1.
Introduction
Since the Nineties, the political leaders of the South-eastern countries of the Mediterranean Sea1 (MEDA) have been committed to removing economic and institutional obstacles to accelerate development and enhance their attractiveness (Hoekman and Messerlin, 2002; Kamrava, 2004; Halliday, 2005; Hoekman and Sekkat, 2010). However, these economies are transitioning towards reforms, having distinct models of socioeconomic and political development (Ali, 1999; Hakimian and Nugent, 2003; Najjar, 2005; Çizakça, 2011; Bianchi, 2013).
1 The main countries for size and economic importance sorted by per-capita GDP in US$ thousands (World Development Indicators-WDIs-WB, 2019) are: Israel (44.5), Libya (10.5), Turkey (9.2), Lebanon (8.9), Jordan (4.2), Algeria (4.0), Tunisia (3.5), Morocco (3.5), Egypt (3), and Syria (1.1).
Egypt initiated restructuring programs in 1991; Algeria in 1994; Tunisia in 1995; and Israel and Morocco began earlier, in the mid-Eighties. Libya, Syria, and Jordan, on the other hand, have faced significant challenges in implementing structural reform programs. Reforms in Jordan have been the most significant; those in Syria, however, have been slower and much less impactful. Finally, Turkey initiated structural reform programs starting in 1995 to pursue closer ties with the European Union (EU), which were later interrupted due to political misunderstandings and cultural differences not yet fully resolved (IMF, 1996, 2013; Vandewalle, 2006; El-Said and Harrigan, 2014; Masoud, 2015; Moran, 2016).
In summary, MEDA economies have had to face numerous challenges and unforeseen events in achieving development, with significant macroeconomic repercussions and considerable political implications. Nevertheless, the challenges they face include:
1. Employment, particularly youth and female employment,
2. Preventing the generalized increase in food prices to combat poverty, and
3. Improving governance in various aspects, such as managing limited water resources, combating climate change, and achieving sustainable development goals.
In other words, MEDA economies experience a plurality of transnational challenges and tensions that affect the legitimacy of states, their governance structures, and ultimately alter macro-regional balances. The MEDA region includes countries seeking international economic and socio-political consolidation – sometimes difficult to implement – with significant internal imbalances. As a result, a virtuous model of Euro-Mediterranean cooperation and growth could be particularly relevant, especially considering that, in the current geopolitically evolving context, global actors like China and Russia are extending their economic influence in the region.
Thus, our purpose is to present a quantitative and qualitative macroeconomic and sociopolitical outlook of the MEDA transition economies, representative of a region of the world with significant development and growth potential, despite the many regional difficulties and concerns persist (EURO-MED AGENDA, 1995; Portelli, 2004; Amendola and Ferragina, 2012; Tankosić et al., 2013; Horst et al., 2016; Saidi and Hammami, 2016; Salamon, 2016; Scalamonti, 2021; EURO-MED AGENDA, 2021).
As far as we know, at the current state-of-the-art, no researchers have recently explored or extended the countries' macroeconomic outlook, especially for MEDA transitional economies identified (Summo and Pepe, 2008; Daniele and Malanima, 2008; D'Aponte, 2014; Pierangeli et al., 2015). To address this purpose, we have used various analytical techniques integrated in this study, including macroeconomic indicators, SWOT analysis, clustering, Markovian chains, investment development-path, and sociocultural factors.
MEDA transitional economies can have sectors involved to not negligible manner within global value chains, for instance: (ⅰ) In agricultural and food productions, (ⅱ) in chemical and petrochemical, (ⅲ) in equipment and others light productions, (ⅳ) in financial services and logistics, and (ⅳ) in some productions to high-tech. However, attractiveness of countries is low to cause relevant foreign investments flows, and international aid has likely been insufficient to face adequately public debt crisis that stifles some economies a long time.
These inflows have been hindered by unsound governance, flawed regulatory frameworks, arbitrary tax regimes, and excessive public interventionism has discouraged private entrepreneurship. Nevertheless, MEDA transitional economies have become more integrated into global markets and portfolio investment has almost doubled in the last decade, but foreign direct ones have halved (UNCTAD, 2020).
This means that there are some fundamental weaknesses relative to other emerging economies in the world that instead have improvement in their country-systems (Khandelwal and Roitman, 2013; IMF, 2020). For instance, Morocco, Syria, Egypt, and Jordan have a very low per-capita GDP, which makes riots and civil uprisings more likely. Turkey and Israel have relatively high per-capita GDP, meaning these countries are less vulnerable to upheaval. Instead, Libyan per-capita GDP is an exception and seems good, but this is due to a combination by high natural resources rent and low population density.
To highlight strengths and weaknesses at the country level, the SWOT analysis can be a powerful tool to identify and analyze the internal and external factors impacting MEDA transitional economies (IEMED, 2012; IMF, 2014).
Strengths: (ⅰ) The presence of natural resources is a significant strength, particularly in several MEDA economies, where oil, gas, and other natural resources are abundant, however, the countries' reliance on these resources can lead to economic imbalances and strategic vulnerabilities, especially if there is a lack of export diversification; (ⅱ) the presence of a low-cost labor force is advantageous for attracting investment in labor-intensive industries, however, this should be coupled with efforts to improve skills and productivity to avoid a placing firms along global value chains in low value-added activities, where wages remain low and there are no improvements in living standards; (ⅲ) starting of democratic processes can stabilize several MEDA economies and create a more predictable business environment, however, these processes can also be fraught with challenges and may lead to temporary instability, such as the "Arab Springs" unrest; and (ⅳ) external aid support can facilitate development in transitional economies, but it can also create dependency, therefore, it is important how this external aid is used to ensure it fosters sustainable development rather than short-term solutions.
Weaknesses: (ⅰ) The high unemployment, especially among youth, can lead to social unrest, if it not adequately addressed with effective education and labor policies by countries' governance improving labor market efficiency; (ⅱ) the informal sector often dominates these economies, which undermines tax revenues, social security systems, and the rule of law, complicating the countries' development; (ⅲ) conversely, small private sector limits innovation, competition, and economic diversification; (ⅳ) low education levels hinder the ability of the workforce to adapt to new technologies and contribute to economic growth and firms' productivity; (ⅴ) imperfections of business environment, such as hight bribery, wide bureaucracy, and weak regulations can deter investment and stifle economic growth; and (ⅵ) the macroeconomic imbalances may include high inflation, large deficits, or currency instability undermining investor confidence and growth prospects.
Opportunities: (ⅰ) Leveraging technology and fostering innovation could help MEDA transitional economies towards sustainable development, especially in basic sectors like agriculture, renewable energy, and financial; (ⅱ) improved governance climate, infrastructure, and a skilled workforce can attract more foreign direct investments, vital for economic growth and job creation; (ⅲ) there is potential to expand the private sector, particularly in developing small and medium-sized enterprises, which are important for economic resilience and growth; (ⅳ) structural reforms, jointed with the above opportunities, can set the stage for extensive economic growth, including reforms in the labor market, tax policies, and trade agreements; and (ⅴ) as economies grow and stabilize, opportunities of improving living standards increase, through better health care, education, and social services.
Threats: (ⅰ) In resource-dependent MEDA countries, rising commodity prices, while potentially increasing revenues, also make their economies more vulnerable to global price shocks; (ⅱ) in many MEDA economies, ethnic tensions can lead to social unrest and even conflict undermining their economic stability; (ⅲ) the presence of ongoing or potential conflicts is a serious threat to the stability of whole North Africa and the Middle East, and which can disrupt economic activities in the region, or disintegrate populations and destroy infrastructural capital of countries; and (ⅳ) slackness of governance climate and a slowdown in democratization process, or, if corruption and informality remain unchecked issues, these can lead MEDA economies to structural crises, thus making it difficult to implement necessary reforms and maintain investor confidence.
This SWOT analysis can provide valuable insights, but it should be complemented with other analytical frameworks to better understand the complexity and dynamism involving MEDA countries' institutional and business environments. This is exactly what our study aims to do. In fact, SWOT analysis can be an interesting starting point for the analysis. Table 1 summarizes the highlights of the comprehensive SWOT analysis for MEDA transitional economies.
The rest of the paper is structured as follows: (ⅰ) Quantitative macroeconomic outlook, (ⅱ) qualitative macroeconomic outlook, and (ⅲ) conclusions.
2.
The quantitative macroeconomic outlook
The macroeconomic indicators provide insights into economic trends and are critical for policymakers, businesses, and investors to make better choices, understanding the institutional and business environment, and identifying opportunities and risks (Marelli and Signorelli, 2010).
However, many indicators could be released with a delay by main international organizations, which means they reflect past rather than current conditions. The data release is often subject to revision, which can alter the economic outlook over time. Countries' economies are influenced by numerous interconnected factors, making it difficult to isolate the impact of individual indicators. Finally, external factors, such as global events, natural disasters, and geopolitical tensions, can affect economic indicators and complicate their interpretation.
Macroeconomic indicators are vital tools for understanding the economic landscape, despite their limitations. By carefully analyzing these indicators, stakeholders can make more informed decisions, anticipate economic shifts, and develop strategies to navigate economic challenges in target countries.
Table 2 shows a quantitative macroeconomic overview for the MEDA economies using time series from the WDIs-WB from 1990 to 2019. In providing this overview, significant social and macroeconomic indicators are shown: (ⅰ) The GDP per capita growth rate, the urban population growth rate, and the unemployment rate, such as indicators for economic development, and (ⅱ) the GDP deflator, the exchange rate, and the stock market capitalization index, such as indicators for financial development. Finally, to complete this outlook, other relevant macroeconomic indicators are shown, such as: (ⅲ) Natural resource exploitation, gross fixed capital formation, manufacturing and services value-added growth rates, and countries' involvement along the global value chains measured by the merchandise trade, medium and high-tech exports, and foreign direct investments.
The emerging outlook shows a fairly diverse cluster of countries. In some cases, the indicators have shown a high variability in time series and numerous missing values.
Last, in Table 3, (ⅳ) the governance dimension is shown in a compact form through a composite indicator of our own creation and articulated in its sub-dimensions, represented by the partial indicators provided by the World Governance Indicators (WGIs).
Kaufmann et al. (2011) defined overall governance as the traditions and institutions by which authority in a country is exercised. Governance encompasses three dimensions and is measured through six intertwined indicators: (ⅰ) Political governance, the process by which governance is selected, monitored, and replaced by the social base – political stability index, voice and accountability index, (ⅱ) economic governance, the capability of governance to formulate and effectively implement policies – government effectiveness index, regulatory quality index, and (ⅲ) institutional governance, the respect that both the people and policymakers have for the institutions governing social and economic interactions – rule of law index, control of corruption index. A positive governance climate fosters the necessary conditions for growth, facilitating human development, infrastructural capital, as well as a more effective interception of foreign investments; therefore, governance matters (Kaufmann et al., 1999, 2002). An effective governance climate index (GC) should efficiently synthesize these three governance dimensions2. As a result, governance climate is an efficient aggregate indicator, computed for each country and year as the arithmetic mean of the geometric means for pairs of WGIs in each of the three governance dimensions and with the scale normalized. It respects the mathematical properties of consistency, monotonicity, and compact synthesis of the average values. Furthermore, it provides each dimension with parsimonious and equal consideration through the two different averages, efficiently synthesizing a set of otherwise non-interchangeable indicators, and considering this interdependence is important for accurately measuring the countries' governance climate.
2 This computation has respected the mathematical properties of consistency, monotonicity, and compact synthesis for the average values. Its formulation has been the following (1):
Moreover, it provides each dimension with parsimonious and equal consideration through the two different averages. This index efficiently synthesizes a set of otherwise non-interchangeable indicators – the geometric mean in fact ensures that an index cannot compensate the other – adequately considering the interdependence across governance dimensions. Therefore, considering this interdependence is important for accurately measuring the countries' governance climate.
Therefore, the governance dimension can reflect the citizens' perception of the countries' government, allowing qualitative evaluations. In summary, the MEDA cluster is characterized by an overall unsatisfactory governance, except for Israel. However, Jordan and Turkey exhibit some barely satisfactory governance indicators.
The years 2011–2012 were those of the "Arab Springs". The most significant socio-political turmoil occurred in Egypt and Tunisia, but with effects across the entire region. All countries felt the impact of intensified internal crisis mitigation policies. While Morocco – thanks to a more stable macroeconomic outlook – managed to better counteract the effects of the crisis, Egypt, Algeria, Syria, and Libya experienced particularly slow, ineffective, and repeatedly interrupted political and social reforms due to the collapse of oil prices – vital for their economies. In Jordan and Lebanon, on the other hand, measures had to be introduced to counteract the inflationary spiral that affected them.
Therefore, comparing the current status quo with the period before the unrest due to the "Arab Springs", the state of instability characterizes a large part of the MEDA region. The promised and desired reforms in the countries' governance architecture have been blocked; therefore, states are looking for a sound path to reorganize themselves. The "Arab Springs" riots have left MEDA transitional economies in a transition stage that has not yet concluded, where resilient regimes and revolts remain.
MEDA transitional economies can be grouped based on the presence of natural resources and labor-cost, as follows (Diop et al., 2012; Woertz, 2014; Cammett, 2018): resource-rich/low labor-cost (Algeria, Tunisia, Libya, Egypt, Syria); resource-poor/low labor-cost (Morocco, Jordan, Lebanon, Turkey); resource-rich/high labor-cost (Israel); resource-poor/high labor-cost.
A cluster analysis is a statistical technique used to group similar objects or data points into clusters based on their characteristics. Its purpose is to ensure that objects within a cluster are more similar to each other than to those in other clusters. This technique is widely used in various fields of social sciences for exploratory data analysis, pattern recognition, and their classification. It helps identify inherent groupings and patterns within the data, making it easier to interpret and visualize. However, the results may be sensitive to the choice of parameters or distance metrics used. Overall, clustering is a powerful method for visualizing undiscovered structures in data.
In Figure 1 we report the Hudson's clustering matrix where the two clusters of MEDA transitional economies are located – 1996 in grey and 2019 in black. All countries are within the second and third quadrant that identify the situations of political fragility and/or instability; the only exception is Tunisia, being in 1996 the most stable of the MEDA transition economies.
In fact, most MEDA transitional economies suffer from a crisis of governance. Political systems are stagnant and oppressive, populations suffer widespread human rights violations, the rule of law is arbitrary, corruption is endemic, and demographic and economic imbalances are rising. Therefore, countries that suffer from an internal fragmentation due to, for instance, political sectarianism, relgions, and ethnicities, and that are characterized by weak and non-functioning institutions are at risk of being of failed states.
In these states, the weakness of institutions reinforces political instability which, in turn, further weakens institutions and undermines their legitimacy; nevertheless, it could also to contribute to strengthen the same regimes. According to Hudson (1977), it is possible to classify the countries on basis of political stability and degree of government effectiveness. In failed states, social dimensions and governments are intertwined.
People no longer receive basic services, and the regime loses its legitimacy; therefore, institutions are no longer effective. These states are characterized by growing internal social, economic, and political inequalities, slow human development, and conflicts that deteriorate the governance climate (Gisselquist, 2015).
The failed state presents at least three characteristics: (ⅰ) Weak legitimacy and governance, (ⅱ) internal inequalities, and (ⅲ) prolonged state of internal or external conflicts. For instance, Syria, Libya, and Lebanon are MEDA states where governance is not any more able to provide security and basic services or to impose itself effectively.
2.1. The development paths of countries
Foreign investment flows are important in economies during transitions since they can promote spillovers of know-how to contribute to socio-economic processes of restructuring to accelerate development (Scalamonti, 2024a). Therefore, they are an indicator of sentiment over the country by investors (Sawada, 2010; Liargovas and Skandalis, 2011; Lautier and Morebaub, 2012; Rogmans and Ebbers, 2013; Silajdzic and Mehic, 2015; Huidrom et al., 2017; Castellani et al., 2018; Peng and Lin, 2019; Scalamonti, 2020). However, foreign firms might not use the factors of production from the domestic market, or they might introduce more negative externalities in country-systems than positive ones.
Foreign investment inflow in MEDA transitional economies has not been adequately valorized (Ferragina, 2011, 2014, 2015; Scalamonti, 2021). For instance, they could improve financial institutions and stock-market capitalization that remain rather limited. Otherwise, foreign investments are often concentrated in real estate and tourism sectors with few spill-overs on productivity and know-how for the host country.
Usually, foreign and portfolio investments can be distinguished (Uctum and Uctum, 2011; Harms and Méon, 2017; Krugman et al., 2018). However, they could also be considered together as a single flow of foreign capital, although with different theoretical justifications. In fact, Scalamonti (2021) has properly found for MEDA transitional economies that foreign capital inflows are justified by the greatest international openness, per-capita GDP growth, presence of public debt, growth of manufacturing industry, capitalization of firms in stock-market exchange, unemployment reduction, finally, by adoption of international accounting standards such as proxy of transparency in financial reporting, while improvement in governance climate, "Arab Springs" effects and economic global crisis have had negative impacts.
According to development-path theory, development reached by MEDA transitional economies can be grasped for the period 1990–2019. According to this theory, the development path consists of five stages. The first two stages relate to developing economies, the third one involves economies in transition, and the last ones refer to developed economies (Dunning and Narula, 1996; Durán and Úbeda, 2001).
In stage (ⅰ), outward investments are negligible or zero due to firms' insufficient ownership advantages. Inward investment levels also remain low because of restricted markets, low incomes, inadequate government policies, human capital scarcity, insufficient infrastructure, or political and social instability. Firms prefer accessing foreign markets through commercial relationships or spot contracts. Despite being in a pre-industrial stage, countries with sufficient attractiveness and resources can attract investors. The governance should focus on improving basic infrastructure and human capital formation.
In stage (ⅱ), outward investments remain negligible, but inward ones increase due to firms' localization advantages, active governance policies, higher market openness, and overall improvements in the country's conditions. Knowledge spillovers and human capital formation become possible.
In stage (ⅲ), outward investments begin to increase while inward ones decrease, resulting in a net positive position. Governance remains crucial in this intermediate stage, where per-capita income rises, and the manufacturing industry boosts product supply through R & D activities, increasing market competition.
In stage (ⅳ), outward investments equal or exceed inward ones. Governance must regulate competition and support business development with focused, active policies. Firms seek a low-cost labor force in developing economies and internalize strategic activities with greater added value. They also become more active in the foreign markets of developed countries through strategic alliances, cooperative agreements, and M & A. The country's localization advantage, initially associated with a low-cost labor force or resources, gradually diminishes as manufacturing processes become more labor-saving due to technical progress.
In stage (ⅴ), the investment net position fluctuates around zero, with both outward and inward investments continuing to increase. This indicates certain maturity achieved by the country in its development path. Its economic system enters global value chains, and it begins to resemble other advanced economies structurally. In this last stage, governance continues to play an important strategic role. In fact, investment flows depend on firms' localization strategies and their ability to obtain, expand, and coordinate strategic assets effectively at a geographical level. The country's attractiveness depends on the resource endowment and the technological-gap and organizational capabilities held by firms.
MEDA economies in Figure 2 that show a tendency to stay above the threshold level for per-capita net-outward foreign direct investments (y-axis) are usually those with more advanced markets and higher per-capita GDP (x-axis), where outward foreign investment exceeds that inward. On the other hand, economies below this threshold level are in the developing stage, and they show more low outflow investments compared to inflow ones. Therefore, for investors, these countries might have economic structures advantageous to them, for instance, due to the presence of cheap labor force or commodities.
The scatter plots support the development-path theory in relation to investment flows in the first two phases of the development path for MEDA transitional economies, including Morocco, Algeria, Tunisia, Egypt, Jordan, Lebanon, and Turkey. Instead, Libya and Syria cannot be placed, and their development path does not allow us to make precise assessments of the phase in which they are located since they show twisted tendencies.
In other words, investors reduce investments in countries where they perceive greater risk, or where governance conditions could deteriorate (Noutary and Luçon, 2013; Hanieh, 2014; Karam and Zaki, 2015; Ferragina, 2016; Forte et al., 2018; Giovannetti and Marvasi, 2019). Firms by countries that are in the first two stages of development, according to the investment flows theory, could have some ownership advantage, but they also might not be able to approach foreign markets; therefore, outward investments remain modest. On the other hand, the only market that has entered the most advanced stages of the model is Israel. This is coherent with its better macroeconomic outlook and its greatest integration in global economy even if it presents some socio-political imbalances due to internal and foreign conflicts, especially with neighboring countries.
However, the development path of countries could be interpreted based on their macroeconomic and governance conditions or based on their economy positioning along the global value chains.
These factors will inevitably influence the development path of countries. The international fragmentation of production along the global value chains – which regards both trade in product/semifinished product and foreign direct investment flows – impacts on within-country incomes through several ways: (ⅰ) The offshoring of low-value added tasks towards underdeveloped countries could entail a higher (or lower) remuneration of high-skilled workers in developed (or developing and emerging) countries, thus increasing income inequalities in advanced economies while reducing it in less developed ones (Van Bergeijk, 2018; Irwin, 2020); (ⅱ) this same offshoring of low-value added tasks from capital-abundant economies to labor abundant ones entails a higher capital-output ratio in the former and reduction wages in developed countries, exactly to the extent that capital acts as a substitute for labor (Helpman, 2017), though to the extent that undeveloped countries are marked by a lower level of education and knowledge capital than developed ones: The value chain tasks offshored by the latter may result in high-skilled and capital-intensive activities for developing and emerging economies, finally increasing wage inequalities in both developed and underdeveloped countries (Jaumotte et al., 2013; Sheng and Yang, 2017; Dao et al., 2019); (ⅲ) the production along the global value chains is always more skill-based and capital-intensive than traditional trade (Antràs, 2020), due to the higher level of capabilities required to perform given tasks with strong complementarities with other geographically fragmented value-adding activities (Antràs, 2021), and also due to the more skill- and capital-intensive production techniques used by firms operating in global value chains than domestic firms (Bernard et al., 2018); (ⅳ) trade and capital liberalization fosters the most mobile production factor, thus capital; and (ⅴ) finally, the fragmented nature of international production can be a threat for workers, weakening the bargaining power, reducing wages, and increasing inequalities in both developed and underdeveloped countries (Stansbury and Summers, 2020; Coveri and Pianta, 2022; Hartmann et al., 2017). In summary, the fragmented production along the global value chains has prompted a hyper-specialization of world economies and trade towards specified value chain activities and tasks over others (Feenstra, 1998; Lall et al., 2009; Dedrick et al., 2010; Timmer et al., 2014; Timmer et al., 2019; Coveri et al., 2020; Paglialunga et al., 2022; Coveri and Zanfei, 2023; Ayadi et al., 2024).
Finally, the theory of development-path, according to investment flow, is not without criticism. An increase of investment net positions, which can usually be interpreted with more country-system competitiveness, could instead be due to a disinvestment process of firms in response to a rapidly deteriorating business environment and governance climate. However, its exemplary value remains a valid interpretive instrument for economic growth, even to MEDA transitional economies (Scalamonti, 2021). In other words, development is a specific path that each economy follows in its own direction, often based on the speed of changes taking place.
3.
The qualitative macroeconomic outlook
From a structural standpoint, the MEDA economies share the same shortcomings (Wolde and Bhattacharya, 2010): (ⅰ) Low productive diversification with production concentrated in few sectors – primary, extractive, and low value-added manufacturing, (ⅱ) labor market challenges – although some measures have been introduced to combat child labor and unemployment, and (ⅲ) markets are perceived by foreign investors as burdened by political-institutional difficulties and often insurmountable cultural differences; although some trade agreements have been signed, their implementation has been made difficult (ILO, 2012; Noutary and Luçon, 2013; El-Said and Harrigan, 2014; Anderson and Anderson, 2014; McKee et al., 2017).
Overall, the MEDA economies represent a rapidly growing potential market, with strong urbanization, and it is estimated that the population will reach one hundred million inhabitants by the middle of the decade (UNHSP, 2012, 2020). Therefore, many cities are candidates to become rapidly emerging global entities with a driving role in the economic and social progress of the region (Mulligan et al., 2017). These demographic processes are pushing the MEDA economies towards premature industrialization without a real network for providing advanced services to businesses.
Below and summarized in Table 4 are synthetic and detailed descriptions of the economic and social fabric of the MEDA countries, excluding Syria, which is engaged in post-war reconstruction and known for being a rentier state and for the predominance of agricultural exports (NATO, 2016).
Morocco. In 2016, Algeria was the leading North African destination for foreign investment, second only to South Africa on the continent (UNCTAD, 2020). Therefore, since the Nineties, the country has implemented a series of macroeconomic reforms aimed at gaining a comparative advantage in terms of the country. Some of these reforms have focused on policies to attract foreign investment, the massive privatization of state-owned enterprises, and the signing of free trade agreements for international trade. The increasing number of foreign investments suggests that investors have responded positively to improvements in competitiveness, and the concentration of foreign investments from EU countries also underscores the importance of trade relations between Morocco and its Mediterranean trading partners. Previously, the Moroccan economy was highly protectionist and based its industrialization on import substitution and forms of agricultural self-sufficiency (Currie and Harrison, 1997). As a result, the taxation system has also been simplified, and a five-year value-added tax exemption has been introduced for investors presenting new projects. This has put Morocco's comparative advantage over its North African neighbor in a better perspective. Therefore, the Moroccan economy is somewhat more diversified than others, although there is a structural dependence on certain natural resources, agricultural revenues are volatile, and remittances from migrant workers play an important role. Today, Morocco is seeking to establish itself as a hub between Africa, Europe, and Sub-Saharan Africa. Since 2005, there has been rapid expansion - due to the downsizing of French banks in the western region - which has led to increased trade flows from Morocco to reposition surplus production due to the limited domestic market and establish itself in many strategic sectors such as telecommunications, pharmaceuticals, textile industries, and agri-food industries (Moran, 2016; Mathieu et al., 2019).
Algeria. Algeria has been experiencing a serious governance crisis for decades and can no longer afford to postpone necessary structural reforms (Talahite and Beji, 2013). Despite unprecedented government spending, the country's economic performance remains rather uncertain (Mebtoul, 2017). Algeria's dependence on hydrocarbons is strong, and the agricultural and manufacturing sectors are not in good health. There are three key sectors of the economy, but they have very weak links with each other. The public sector, which concentrates on oil and natural gas exports, absorbs almost all foreign revenues but also contributes to the system's liquidity, which is necessary to cope with investments in the petrochemical industry and to support the banking system, especially during periods of rising oil prices. On the other hand, the economy's excessive dependence on the export of natural resources and volatile revenues also leads to periods in which excess liquidity results in further contraction of overall revenues, followed by credit tightening necessary for the normal restoration of economic activity, but ultimately transferring liquidity problems to the banking system. Sectors affected by imports are food, electric, electronic, and textile, which have a significant involvement along the global value chains. Finally, it should be noted that in Algeria, economic activities in the formal and informal spheres are closely related, mostly involving service sectors, retail trade, construction, and otherwise non-tradable goods.
Tunisia. This economy also benefits from natural resource rents, although to a lesser extent than neighboring countries (Talahite and Beji, 2013). Its economy focuses on a few sectors affected by the outsourcing of assembly activities by European SMEs (Calza et al., 2010; Kahia, 2017). These sectors include textiles, clothing, and business support services, where cost advantages sought by subcontracting companies have led to the employment of overly professionalized labor and overqualified graduates (Ghazali, 2009). Furthermore, foreign direct investment in the agricultural sector and non-negotiable service sectors due to a limited market is virtually absent. Instead, it is the coastal tourism sector that absorbs most of the Algerian workers and contributes the most to value-added formation.
Egypt and Libya. The Egyptian economy is mainly centered on the activities of small and medium-sized enterprises operating in the agriculture and tourism sectors. However, tourism is the primary activity generating foreign currency inflows, followed by migrant workers' remittances. Important sectors are also connected to crude oil extraction, quarries, and mines. Therefore, like the other neighboring economies, this economy has been subject to political shocks and socio-economic uncertainties that have made these revenues extremely uncertain (Hassine, 2012). The Suez Canal is one of the main sources of foreign currency inflows and a catalyst for foreign investment in sectors and economic zones that develop around it, as well as for food and oil trade. To increase the economic impact of the canal, Egyptian authorities have established Special Economic Zones (SEZs)3, factories, and logistic facilities around it (Kenawy, 2016; Kahia, 2017). The Libyan economy is very similar to the Algerian one in the sense that oil revenues represent almost all foreign currency inflows and more than half of the wealth produced in the country, but population levels are among the lowest in the region, and this wealth is concentrated only among oligarchs (Collombier, 2018).
3 The Special Economic Zones (SEZs) are geographically defined areas, managed and administered as separate socio-economic entities, with the aim of promoting foreign investment and increasing employment levels in the manufacturing industry. Finally, they promote the positioning of the industrial system along global value chains, enhancing the attractiveness and competitiveness of the country's system. Firms located within them can benefit from a duty-free environment that facilitates knowledge spillovers and technology transfers (Zeng, 2015).
Israel, Jordan, Lebanon, and Syria. Israel is the country with the highest innovation capacity in the region and where the most important sector is high-tech. Taking off in the 1990s and supported by the growing importance of software production for computers, the development of this sector has also meant flows of highly qualified human capital and greater public support for research and development. The sector is closely connected to the military, which acts as an incubator for private sector start-ups (Senor and Singer, 2009). In this sense, military productions, as for Turkey and Egypt, also have a significant weight in their economies (Biscop and Sassel, 2017). While Jordan's ability to increase public spending on investments is limited, considering the high public debt it has accumulated, resulting in reduced foreign direct investment and international aid (Yitzhak, 2018; Abu-Murad and Alshyab, 2019), in Lebanon, migrant workers' remittances and inflows of foreign capital fund the enormous public debt accumulated with current account deficits, and in Syria numerous internal conflicts contributed to its decline and led to the intervention of international organizations to restore its socioeconomic and political environment (World Bank, 2013).
Turkey. The emergence of a new dynamic and conservative middle class, which finds in the ethics of work and wealth distribution the means to be devout believers, has influenced the development of the more traditional sectors of the economy, such as agriculture, food, the automotive industry, and textiles. This social phenomenon has also been compared to a sort of Islamist-derived Calvinism (ESI, 2005). For example, Turkey is among the world's most important agricultural economies and has made its textile industry a strength, implementing a strategy to diversify its portfolio of exports and products towards neighboring countries in the Middle East and Central Eastern Europe (Kahia, 2017). Therefore, the ruling class places great importance on good relations with geographically and culturally close economies and has organized its interests into a series of dialogue and cooperation-inspired bodies over time, thus making Turkey a hub for the development of the neighboring Middle East (Altunisik, 2009; Albarracín, 2011). This is extremely true in the case of energy supplies due to the presence of numerous pipelines, and Turkey also controls access to the Black Sea, allowing it to impose tariffs on commercial traffic.
3.1. The upheaval of "Arab Springs"
We mentioned the existence of association among development and governance, which varies for each country, and can be traced back to combining macroeconomic and socio-cultural factors. Regimes historically fear political and social turbulences that can derive from not granting reforms, even if they might decide to grant basic socio-economic ones and postpone the others more complex as political ones (Kuran, 2011; Asongu and Nwachukwu, 2015). Under this point, MEDA transitional economies have been disappointing expectations about socio-economic and political reforms although in some countries governance has improved respect to Nineties; in none it has been such to reduce unemployment, to increase wages, therefore, such to improve well-being of populations (Freedom House, 2011; Hafez, 2016; Çakmak, 2017).
What happened in "Arab Springs" upheaval? In summary, there were protest movements by young people – otherwise said "youth bulges" – that begin from global economic crisis and been combined with other factors as a diffusion of new media, and more information circulation triggers a state of crisis. Therefore, causes of "Arab Springs" are attributable to (Dupont and Passy, 2011; Joffé, 2011; Joya, 2011; Aarts et al., 2012; Campante and Chor, 2012; Dabashi, 2012; Manhire, 2012; Noueihed and Warren, 2012; Volpi, 2013; Kaboub, 2013; Gerges, 2013): (ⅰ) Stagnant authoritarian regimes, (ⅱ) lack of the civil right and liberties, (ⅲ) income inequality between elites and populations, (ⅳ) endemic corruption, (ⅴ) weakness of economic policies and ineffective growth models, (ⅵ) low development of private sector, (ⅶ) excessive dependence on foreign aids, (ⅷ) increase in food and commodities prices, (ⅸ) trade openness and growth of processes connected to globalization, and (ⅹ) a new public space generates from internet and from the use of social media has led to freer political communications and expressions, it was doing thus as "sounding board" at riots (Howard, 2010; Khondker, 2011; Chadwick, 2013; Driouchi, 2014).
Therefore, digital technologies and web communications have increased the participation of people in public life and allowed for a more rapid and cheap circulation of information, which otherwise has only been in newspapers and is subjected to censure by regimes (Van Eeten and Mueller, 2012).
Until 2011–2012, stability of many MEDA transitional economies was in the hands of autocrats, whose regimes were seen as one exception to democratization, likely due to incompatibility of Islamism with democracy (Diamond, 2010; Elbadawi and Makdisi, 2017). In other words, for some political international actor, the regimes were the best way to keep the order and secure in the region, as well as to likely protect own economic interests (Cordesman et al., 2011; Del Sarto et al., 2019). Therefore, regimes for their nature are unstable, and they have more probably to experiment conflicts than democracies.
For instance, looking at Syria and Libya, these countries have had personalistic regimes where the power was concentrated in the hands of a single man and the protests have been repressed with use of violence (Beinin and Vairel, 2013). After the war in Syria finished, long negotiations led by the United Nations in Libya have failed and have been not able to resolve internal civil conflicts to start a democratic governance. Tunisia or Egypt, on the other hand, have experimented a form of regime where their leaders have been shared a part of power with political parties and military forces. Algeria, Morocco, Israel, Jordan, Lebanon, and Turkey are vulnerable at state of crisis since they can suffer from ethnic or religious conflicts within them.
For instance, in Algeria, there have been popular uprisings to obtain more political and voting rights. Governance by Jordan and Morocco have responded to protests, granting some reforms. Israel has been busy for many years with difficult issues of Palestinian State. In Lebanon, the political landscape remains fragmented, and it is divided along sectarian fault lines. Turkey has seen violent political clashes, then coercively repressed, and it has been one of the greatest receiver countries of international aid in contrast to extremist and sectarian groups.
Therefore, sectarianism is another aspect that distinguishes MEDA societies, but it is also used by elites to promote their interests and to intensify internal divisions in the countries with a purpose to narrow down forms of opposition and reinforce their legitimacy (Barany, 2011). For instance, Cavatorta (2010) has paid attention to political and socio-economic impacts of war conditions. Indeed, in the Middle East among the most dangerous armed groups are those extremists of Islamic origin such as Hamas, Hezbollah, or jihadists, which in 2014 took control of a large portion of Syrian territory (Marsili, 2016).
The Markov chains analysis is a statistical method used to model the probability of various outcomes in a system that transitions from one state to another. It is based on the concept of Markovian processes, characterized by the "memoryless" property: The probability of transitioning to the next state depends only on the current state and not on the sequence of events that preceded it. This basic assumption simplifies the modeling process, as future states depend only on the current state.
The analysis can be useful for predicting long-term behavior of systems, such as steady-state distributions, or in many cases, it admits for analytical solutions and insights into system dynamics.
However, the assumption that future states depend only on the current state and not on the past may not hold true for all systems, limiting the model's applicability. Additionally, for more complex systems and with many states, the transition matrix can become very large in the size, making computations and analyzes very difficult. An accurate estimation of the transition odds requires sufficient data – which may not always be available – and assumes that these remain constant over time – which may not be realistic in dynamic contexts. Finally, this technique requires defining discrete states – which may oversimplify complex systems or phenomena. In conclusion, the analysis of Markov chains for systems with stochastic transitions is another method included in this study.
Therefore, we show Markov chains (Belokurov et al., 2020) on time series of governance climate by MEDA transitional economies from 1996 to 2019, having divided them among before and after the uprisings of the "Arab Springs" (Figure 3). We show the odds of transition to increment and decrement, or to remain in the steady state. The odds of having an increase of governance climate after this upheaval is improved in all almost countries, and less than in Libya, Lebanon, Syria, and Turkey, which may have been affected by negative effects triggered by state of transition of governance climate. On the other hand, probability of remaining in the given condition has also decreased for some MEDA transitional economies, while for others it has increased – it has not changed only for Morocco, as evidence of the different impacts the "Arab Springs" have had on governance climate.
3.2. The sociocultural factors
The effects of culture underlying a population have received more attention in the economic literature in the last decade and have been considered in different ways in international business studies (Hutzschenreuter et al., 2011; Gooris and Peeters, 2014; Martín-Martín and Drogendijk, 2014; Beugelsdijk et al., 2015; Williams and Gregoire, 2015; De Santis et al., 2016; Spolaore and Wacziarg, 2016; Gorodnichenko and Roland, 2017; Spolaore and Wacziarg, 2018; Bailey et al., 2018; Liu et al., 2020; Demir and Im, 2020; Stor, 2021; Obradovich et al., 2022).
De Benedictis et al. (2023) have defined culture as the collection of norms, customs, attitudes, and habits, constituting a value system that can be divided into various interrelated subsets. In this definition, emphasis is placed on the existence of an interdependence between the underlying cultural dimensions and the value system. This definition is particularly relevant because it makes culture a concept that can be divided and encompassed by diverse dimensions. The definition of culture provided by Fernández (2008) is also valuable, as it emphasizes the presence of cultural distinctions in the beliefs and preferences of a specific group of people across different times and locations.
However, Shenkar (2001, 2012) raises critical concerns about how cultural distance is calculated, as some methodological issues may not receive sufficient attention. In other words, a composite indicator that does not adequately consider the heterogeneity of the partial indicators that compose it may suffer from compensatory effects. Therefore, for identifying culture and the differences in dimensions that compose it, we referred to these authors.
Cultural dimensions proposed by Hofstede et al. (2010) are valuable for facilitating qualitative comparisons among MEDA societies, as culture is considered from multiple perspectives contributing to its definition (Figure 4). It is noteworthy that MEDA societies are quite similar, with only slight variations observed in each indicator, except for Egypt and Israel. Below, we provide the definitions of six sociocultural factors and their comparison across MEDA societies.
1. Power distance is high in societies dominated by hierarchy and where power is concentrated in a few individuals, or in a single individual. Conversely, it is low in societies where hierarchy holds little importance, and power is distributed in more egalitarian forms. Among MEDA societies, it is generally high, with the only exception being Israeli society, although the difference is slight.
2. Individualism refers to individual or collective decisions and choices. In individualistic societies, people are independent and have individual freedom, while collective societies are based on the understanding of a certain degree of reciprocity between people. MEDA societies tend to be more collective, with Israel having the highest score just above the benchmark, and the Egyptian society having the lowest score.
3. Masculinity measures competitiveness within a society and is high in more competitive societies that prioritize success. It is low in societies where people care more about others and the quality of life. MEDA societies have moderate scores, with Lebanese society scoring the highest and Algerian society scoring the lowest.
4. Uncertainty avoidance indicates how comfortable people feel when confronted with ambiguity and the unknown. High scores are associated with societies that may exhibit fundamentalism or intolerance towards new ideas and behaviors. They adhere to strict codes of conduct and are firmly attached to their beliefs. Low scores distinguish societies where people are more open to novelty and pragmatism is more important than principles. MEDA societies generally have relatively high scores, particularly Turkish and Egyptian societies, along with Israeli society.
5. Long-term orientation is high in societies where people are better prepared for potential future changes, while it is low in societies with a short-term orientation, where people are more resistant to changes and tend to adhere to norms and traditions. MEDA societies show a short-term orientation.
6. Indulgence – as a measurement of hedonism – is the final dimension to consider. This score is high in societies where people embrace the pursuit of pleasure throughout life, potentially leading to excesses. Conversely, it is low in societies where people suppress their instincts, emphasizing self-control or deprivation. MEDA societies generally exhibit relatively low scores for this cultural dimension, which aligns with the significance of religious components within these socio-economic systems.
The cultural distance can be considered an expression of similarity among people belonging to different countries. By starting from the seminal work of Cavalli-Sforza and Feldman (1981), this concept can be traced in numerous studies (Felbermayr and Toubal, 2010; Egger and Lassmann, 2012; Maystre et al., 2014; Melitz and Toubal, 2014; Ahern et al., 2015; Hutzschenreuter et al., 2016; Egger and Touba, 2016; Hellmanzik and Schmitz, 2017; Carrère and Masood, 2018; Cuomo et al., 2023). These studies have provided evidence about the need for a multidimensional interpretation of the culture concept. The cross-cultural distance is reflected in the differences existing in the countries' institutional and business environments and, thus, in their development paths.
In Table 5, we present the cultural distances for MEDA societies. We aggregated the differences computed on each of the six indicators of cultural dimensions by Hofstede et al. (2010) using the method proposed by Mazziotta and Pareto (2020) – to which we refer. Specifically, we employ the non-compensatory methodology to construct a composite indicator. This procedure effectively allows the synthesis of a set of diverse indicators that cannot be interchangeably substituted. It employs the arithmetic mean and standard deviation, and it incorporates a correction term based on the coefficient of variation to appropriately account for all components. Therefore, this procedure has the non-negligible advantage of adequately considering the interdependence and heterogeneity existing between all cultural dimensions. This is crucial for the accurate measurement and interpretation of cultural distance between countries.
A smaller cultural distance can signify the presence of some similarities between cultures. As expected, the result is a heterogeneous group of countries, with Israel showing more differences than the others.
In conclusion, cultural distance can represent a problem for foreign investors, as it impacts both intra- and inter-organizational relationships. Therefore, cultural bridges can be a useful tool to overcome the effects of cultural distance (Calza et al., 2013). The involvement of the population and adaptation to local customs are therefore crucial. In other words, foreign investors should be aware of local values, especially religious and family values, which have a profound impact on business and social relations in Islamic societies.
4.
Conclusions
4.1. Concluding remarks and contribution
Economic policies in MEDA countries have been effectively implemented only after the oil shocks of the Seventies, in front of increased inflows of money and migrant workers (Ayadi et al., 2015; Haliti and Merovci, 2020). Countries that invest little in education and human capital development structurally often exhibit reduced long-term growth. Moreover, the abundance of natural resources could represent a "curse" for countries (Corden and Neary, 1982; Wijnbergen, 1986; Torvik, 2002; Mehlum et al., 2006; Matsen et al., 2016; Bjornland et al., 2019).
In other words, in MEDA economies, growth has not been homogeneous enough to absorb shocks from population and workforce increases. Various reasons may underlie this situation: (ⅰ) Volatility of the GDP growth rate, (ⅱ) supplies dominated by the public sector, (ⅲ) dependence on natural resource rents—particularly oil, (ⅳ) reliance on low value-added productions, and (ⅴ) poor integration of manufacturing systems along global value chains. Therefore, MEDA countries have suffered and continue to suffer from profound and widespread social instability and internal political conflicts to the extent that Algeria, Libya, Israel, Lebanon, and Syria, albeit for different reasons, have gone through and continue to undergo periods of violence and social tensions (Ragionieri and Schmidt di Friedberg, 2003; HEIDELBERG INSTITUTE, 2018).
In light of this, hawse have presented a quantitative and qualitative macroeconomic and sociopolitical outlook of the South-East Mediterranean transitional economies (MEDA), representative of a region of the world with significant development and growth potential, despite the many regional difficulties and concerns persist (EURO-MED AGENDA, 1995; Portelli, 2004; Amendola and Ferragina, 2012; Tankosić et al., 2013; Horst et al., 2016; Saidi and Hammami, 2016; Salamon, 2016; Scalamonti, 2021; EURO-MED AGENDA, 2021). To investigate their potential, we have used various analytical techniques integrated in this study, including macroeconomic indicators, SWOT analysis, clustering, Markovian chains, investment development-path, and sociocultural factors.
Restructuring programs initiated at various times have only partially, slowly, and disjointly achieved the macroeconomic stability objectives set (World Bank, 2009). Government expenditure on armaments has increased over the years, and the outbreak of armed conflicts is a serious threat to internal stability (Del Sarto et al., 2019). Although disarmament agreements have been signed, it is evident that they have not produced the desired effects, especially in Israel, Lebanon, and Syria.
Furthermore, Libya continues to be the site of numerous armed conflicts and the center of political instability in North Africa. The enormous earnings from the sale of oil and natural gas have only been reinvested in a small way to foster the emergence of an industrial system and promote it. The deterioration of political relations between autocratic Libyan governance and the system of Western institutions has caused various embargoes on crude oil exports, ultimately affecting the Libyan economy since the mid-Nineties.
The impulse for reforms in MEDA economies stemmed from at least three geopolitical shocks in the late Eighties (Brzezinski, 1997; Hobson, 2005; Makarychev, 2009; Tzannatos, 2010; Obiols, 2010; Kowalewski and Rybinski, 2011; Cordesman et al., 2011; Moratinos, 2012; Dabrowski, 2012, 2019; Fukuyama, 2020): (ⅰ) The collapse of the communist economy and the Soviet bloc, which ended the bipolar world, followed by (ⅱ) the emergence of the United States as the hegemonic nation, the only interlocutor in the Middle East, and (ⅲ) the drastic drop in oil revenues in the years immediately following the fall of the Berlin Wall.
In this scenario, Euro-Mediterranean agreements could contribute – and can contribute today – to a reduction in tariff restrictions, and a significant intensification of trade between MEDA economies and European countries (EURO-MED AGENDA, 1995, 2021). In particular, the agreements have provided significant amounts of financial assistance from the European countries to MEDA-partner countries (Montalbano and Nenci, 2014; Ayadi et al., 2015; Schumacher et al., 2017).
In other words, the signatory countries agreed to work together to improve the integration of manufacturing systems along global value chains to harmonize trade practices, to promote sustainable and shared development, to improve the living conditions, to decrease unemployment, and to improve the socioeconomic integration (Schlumberger, 2011; Boserup and Tassinari, 2012; Tömmel, 2013; Pace 2014; Dandashly, 2015; Abbott and Teti, 2017; Guecioueur, 2019). As a result, MEDA-transitional economies have several industries internationally involved, such as: (ⅰ) Agricultural and food, (ⅱ) chemical, petrochemical, and mining, (ⅲ) machinery and equipment, (ⅳ) technology, and (ⅴ) service. However, the attractiveness of these countries remains inferior due to some weaknesses in the governance climate and business environment (IMF, 2019, 2022).
MEDA countries have unique socioeconomic features (Saraceno, 1978), with each having shown its own path of development (Acemoglu et al., 2001, 2019). As a result, this diverse set of countries differs in socioeconomic development, cultural background, and diversity of political relationships and trade with Italy, the EU, and the rest of the world (Calza et al., 2010; Giovannetti et al., 2015; Ferragina, 2016; Radl, 2017; Ayadi et al., 2024).
Although with somewhat heterogeneous traits, transitioning MEDA economies are characterized by: (ⅰ) Varying degrees of dependence on oil, (ⅱ) sometimes underdeveloped or poorly diversified production structures, (ⅲ) positive population growth rates, (ⅳ) high unemployment rates, (ⅴ) strong state interventionism or interference by the elite in the countries' economic life, (ⅵ) lower levels of integration of productive systems into global value chains, (ⅶ) undersized financial markets, (ⅷ) institutional weaknesses, finally, (ⅸ) often unsatisfactory foreign investment returns, and (ⅹ) the perceived country risk is often high.
MEDA economies have made significant progress in macroeconomic conditions. However, these remain somewhat unsatisfactory. Institutional or governance deficiencies persist, undermining their attractiveness, which nonetheless remains lower than in other areas of the world (IMF, 2019, 2022).
In conclusion, this study contributes to the understanding of MEDA transitional economies as representative of an area with significant development and growth potential, but in need of support from the international community for more rigorous and fruitful cooperation among neighboring countries. Additionally, it aims to serve as a supporting paper for the research endeavours of analysts, researchers, and academics interested in the macroeconomic and sociopolitical dynamics of the area.
As a result, future developments could delve into the countries' governance climate and human development in relation to economic growth and sociocultural factors, as well as trade, foreign investments, and firms' internationalization.
4.2. Policy implications
The MEDA emerging economies can represent a significant opportunity to develop international business, especially for Italian and European firms (Scalamonti, 2024b). This implies that Italian and European policymakers cannot afford to ignore or underestimate the evolving dynamics of the geoeconomic and geopolitical landscape affecting the South-eastern countries of the Mediterranean Sea (Ehteshami et al., 2017; Gillespie and Volpi, 2018; Gani and Hinnebusch, 2019).
For instance, Italy could be not only an important trade hub for the merchandise to and from MEDA countries, but through targeted governance actions aimed at the integration between neighboring countries, it could also play a pivotal role in promoting various Mediterranean cultures. Its natural geographic position in the center of the Mediterranean region can make it an ideal meeting point for these cultures. However, there are lingering challenges that must be addressed before a satisfactory level of political and economic integration across Euro-Mediterranean countries can be achieved. To establish a stable international order, maintaining sound relations with the South-eastern neighboring countries of the Mediterranean Sea should be among the EU's main priorities to strengthen the economic and political order in the Euro-Med region (Guecioueur, 2019). This advancement would help improve the MEDA countries' institutional and business environment, establishing industrial networks and clusters, and ultimately enhancing countries' attractiveness. Therefore, the EU should play a leading role in the structural upgrading of MEDA transitional economies by acting at the community level and implementing shared economic policies.
Despite this, China and Russia are pursuing both economic and political interests in the MEDA region, leading to increased international pressure in the area. As a result, European policymakers should not overlook the reasons behind this renewed interest in the Mediterranean region, as it reflects a redefinition of global balances and interests. The EU should continue to provide political and socio-economic support to MEDA transitional economies and be pivotal for demands related to sound governance and human development, positioning itself as a credible and preferable alternative to autocratic regimes.
In other words, Euro-Mediterranean cooperation should play an important role in aiding MEDA transitional economies in their pursuit of improved sustainable development, employment opportunities, and stable governance. To align the interests of EU member countries, it could be necessary to reconsider the EU approach in the following issues: (ⅰ) Maintaining peace and countering terrorism; (ⅱ) promoting sound governance, sustainable development, and renewable energy resources; (ⅲ) managing migratory flows; (ⅳ) protecting human rights; and (ⅴ) enhancing free trade and tourism.
4.3. Suggestions for future research
Future research could delve deeper into the relationship between governance, trade, firms' internationalization, and cultural distance for the MEDA country cluster. For instance, gravity models for trade flow between countries employing a SAR-AR specification through a feasible estimation in two steps could also be estimated by combining the Spatial Auto Regressive (SAR) model and the Spatial Error-Mechanism (SEM), inserting the cultural distance across cluster's countries. Two spatial components referring to the cultural affinity in models could capture effects depending precisely on the culture and that are not easily observable, but that could influence the intra-industry trade flow. In this way, we can treat culture exactly for what it is – a fixed value, by resulting in a long-term space-time equilibrium value.
On the other hand, to study the relationship between countries' governance and the effects of time-invariant sociocultural factors, random-effect models may be estimated for panel data. We could also consider time variant explanatory variables as a proxy for countries' development-paths (HDI or GDP), as well as the same lagged dependent variable on the right-side of the equation to consider the persistent effect of policies over time. In other words, the initial level of the variables could be important in econometric analysis because economic actors do not necessarily have sufficient information at time t about the policies implemented within countries. This means that there is always a time gap between the policy implementation and the effect manifestation in socioeconomic systems.
However, incorporating the lagged dependent variable on the right-side of the model equation can lead to endogeneity issues caused by the simultaneity across variables, and the inclusion of lagged explanatory variables can mitigate this bias. Additionally, logarithmic transformations and robust standard errors can help address the heteroscedasticity issue and serial correlation bias. Therefore, with random-effect models, we could efficiently account for cross-sectional unobserved heterogeneity and treat time-invariant variables, such as sociocultural factors. In random-effect models, the cross-sectional component of the error term is considered a random variable unrelated to regressors. Panel data analysis is then advantageous as it combines cross-sectional and time-series data providing a more comprehensive understanding of the phenomena under investigation. A probit model with sample selection may also be suitable for a robustness check. Finally, WBESs data on the firms' internationalization could be used.
Use of AI tools declaration
The author declares he has not used Artificial Intelligence (AI) tools in the creation of this article.
Conflict of interest
The author declares no conflict of interest in this paper.









 DownLoad:
DownLoad: