Abbreviations: VOC: volatile organic compound; SVOC: semi-volatile organic compound; VVOC: very volatile organic compound; CLP: Classification, Labelling and Packaging; IARC: International Agency for Research on Cancer; DHI/BKH: Consulting Engineers; US EPA: United States Environmental Protection Agency; NA: Not Available; SIN: Substitute It Now! TEDX: The Endocrine Disruption Exchange
1. Introduction
The French Indoor Air Quality Observatory has planned a national field campaign in 100 age care and social facilities across France for 2019 to characterize the indoor air quality (IAQ). To date, these facilities have been rarely studied, though vulnerable populations spend most of their time there. To identify which volatile organic compounds (VOCs) to look for in the indoor air, a survey was performed targeting all 18,432 French age care and social facilities to describe the buildings, activities, and cleaning tasks and cleaning products used, with the objective of identifying the VOC emission sources. A focus was put on cleaning products since they may be widely used in these buildings and emit VOCs, which have an impact on the IAQ [1].
Only six studies worldwide were identified to have investigated VOCs in the indoor air of age care facilities, while none were found for social facilities. In South Korea, Hwang et al. investigated the total volatile organic compounds (TVOCs) and formaldehyde in 62 facilities [2], and Lee et al. characterized the indoor and outdoor VOC and formaldehyde concentrations in 30 elderly care centers [3]. In Portugal, Almeida-Silva et al. measured the TVOCs in 10 elderly care centers [4]. Also in Portugal, under the framework of the Geriatric Study in Portugal on Health Effects of Air Quality in Elderly Care Centers (GERIA) project, the TVOC and formaldehyde concentrations were measured in 22 facilities [5]. Walgraeve et al. measured 25 VOCs in 6 elderly homes in Belgium [6]. Finally, within the European Geriatric study on health effects of air quality in nursing homes in Europe (GERIE) project, formaldehyde was measured in eight facilities in 7 countries [7]. In most of these studies, though, the individual VOCs were not measured.
An increasing number of studies have shown potential health effects due to chemical substances used in cleaning activities. Svanes et al. investigated the long-term effects of cleaning product exposure on lung function decline and airway obstruction in a cohort including occupational cleaners [8]. This study highlighted that cleaning sprays and other cleaning agents accelerate lung function decline in women cleaning at home or working as occupational cleaners. A study in California on 183 cleaning workers in an academic medical center investigated the association between acute symptoms and chemical exposure [9]. The study showed that symptoms of irritation due to chemical products are frequent for cleaning workers. Su et al. looked for the determinants of VOC exposures in healthcare settings and showed that exposure to various VOCs was associated with mixed cleaning tasks and the chemical ingredients of the products used [10]. Moreover, a recent review of epidemiological studies highlighted that the use of cleaning and disinfecting products is harmful to respiratory health and increases the risk of asthma [11]. Spray products were specifically targeted [12,13].
Meanwhile, the specific VOCs emitted by cleaning products remain poorly described. Gerster et al. identified the most used cleaning products by a survey of Swiss professional cleaning companies and, through a review of safety data sheets (SDSs), listed the hazardous substances, including VOCs, present in these products [14]. In five hospitals in the United States, Saito et al. assessed the exposure to cleaning and disinfecting chemicals among many occupations [15]. On the basis of the SDSs of the cleaning and disinfecting products, they concluded that different occupations in hospitals involved irritants or sensitizers. On the basis of the SDSs, Wei et al. identified the hazardous substances, including VOCs, present in cleaning products used in 310 nurseries and schools in France [16].
In this context, the aims of this study were to (1) describe the cleaning frequencies, techniques and products employed in French age care and social facilities, including the use of spray products and air fresheners; (2) identify the VOCs that may be emitted from these products in indoor air; and (3) compile a list of VOCs of concern based on their hazard classifications.
2. Materials and methods
2.1. Questionnaire
An online questionnaire was developed that included questions describing the frequency of floor and surface cleaning (several times a day, once a day, several times a week, or once a week or less), cleaning practices (i.e., without any product, with water, with liquid cleaning product and a mop, with pre-impregnated wet wipes, or with sprays), and storage areas (i.e., in shared living rooms or bedrooms or in a ventilated or unventilated adjacent or non-adjacent specific room). The use of air fresheners was also investigated. For each facility, a maximum of two buildings could be described, and for each building, the three most used cleaning products, along with their respective brand and commercial names, could be listed. The quantity of product used was also investigated. However, the low number of data points and disparities in the answers for this question required us to ignore this item.
2.2. Selection of facilities and data collection
The survey was performed in facilities with a susceptible population, namely, dependent elderly people and disabled people. The target facilities were nursing homes and long-term care units for the elderly and specific facilities for disabled children or adults. The contact details (i.e., email, postal address, phone number) of the target facilities were identified from the National File of Sanitary and Social Institutions (FINESS) [17] managed by the French Ministry of Health, which included 18,432 facilities. The link to the online questionnaire was sent to all facilities by email on June 6, 2017. Three reminders were sent by email in June and July to non-responders. The web interface was closed on July 31, 2017.
2.3. Data analysis
The first step was to clean up the database to group duplicate data and remove unusable data (i.e., dashes, question marks, only the brand or an incomplete name). Then, the SDSs of the reported cleaning products were collected from the internet, mostly from supplier websites. Some SDSs were obtained by emailing the supplier via an online contact form. When the SDS could not be found for a specific product, the product was excluded from further analysis.
SDSs provide information about the health risks associated with the exposure to or use of hazardous products. Section 3 gives the names of the chemical substances contained in each product, along with their Chemical Abstracts Service (CAS) numbers, if available. Moreover, some substances, such as fragrance allergens, that can be found under section 15 "Regulatory Information" were collected. For each cleaning product, the names and CAS numbers of the chemical substances mentioned in the SDS were retrieved.
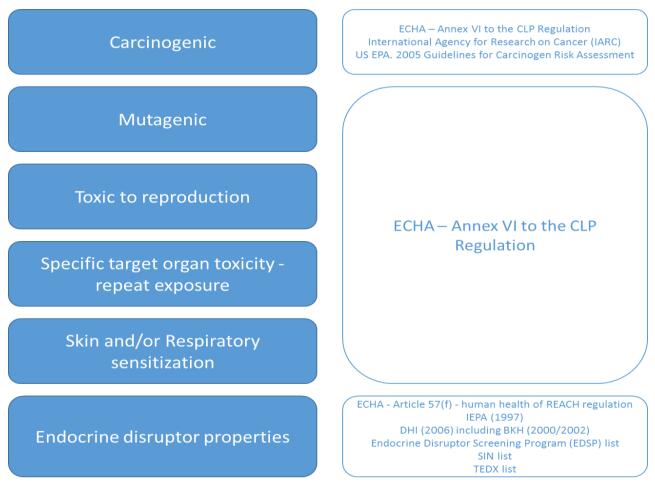
Six categories of health effects were investigated for each substance identified from the SDSs: carcinogenic, mutagenic, toxic to reproduction, specific target organ toxicity - repeat exposure (STOT-RE), endocrine-disrupting properties, and skin or respiratory sensitizing. When possible, different sources were checked to determine the health effects, and the most severe ranking was used.
Substances that are potentially carcinogenic, mutagenic, toxic to reproduction, STOT-RE, or skin or respiratory sensitizing were identified according to the European Chemicals Agency (ECHA) [18]. For those with carcinogenic potential, classifications by the US Environmental Protection Agency (US EPA) [19] and the International Agency for Research on Cancer (IARC) [20] were also considered. Similarly, multiple sources were consulted to evaluate the possible endocrine-disrupting properties of each substance. Using the candidate list of substances of very high concern for authorization available on the ECHA website and published in accordance with the Registration, Evaluation, Authorization and Restriction of Chemicals (REACh) Regulation, those classified to have endocrine-disrupting properties [21] were identified. Other projects, which were carried out for the European Commission DG ENV, the Illinois EPA (1997), and the US EPA were also taken into account [22,23,24,25,26,27]. Other non-regulatory lists were collected. First, the SIN list is a comprehensive database maintained by the non-governmental organization Chemsec, which identifies chemicals likely to be restricted or banned in the EU [28]. In this case, the list was restricted to substances included because of their potential endocrine-disrupting properties. Second, the Endocrine Disruption Exchange (TEDX) list of potential endocrine disruptors identifies chemicals that have demonstrated evidence of endocrine-disrupting properties in at least one study [29].
The health effects considered along with the different sources investigated are summarized in Figure 1.
Finally, the boiling points of the chemical substances were retrieved to classify them according to their volatility and to identify the VOCs. Four sources were considered as follows in order of priority: (1) the ECHA website [30], (2) the Hazardous Substances Data Bank (HSDB) [31], (3) the Chemspider website [32] and (4) the CompTox Dashboard by the US EPA [33]. Based on the definition provided by ISO 16000-6 [34], organic substances with a boiling point between 0 ℃ and 50–100 ℃, are considered to be very volatile organic compounds (VVOCs). When the boiling point is between 50–100 ℃ and 240–260 ℃, the organic substance belongs to the family of VOCs. Finally, between 240–260 ℃ and 380–400 ℃, the substance belongs to the family of semi-volatile organic compounds (SVOCs). For practical reasons, the limits between VVOCs and VOCs, between VOCs and SVOCs, and between SVOCs and non-volatile compounds were set at 50 ℃, 260 ℃ and 400 ℃, respectively.
3. Results and discussion
Among the 18,432 questionnaires sent to the age care and social facilities, 4041 web questionnaires and 137 paper questionnaires were collected. After discarding incomplete or duplicate questionnaires, 2140 complete questionnaires were obtained, representing a response rate of 12% with good representativeness against the national base: among the final selected facilities, 58% are nursing homes, 25% are specific facilities for disabled adults, 14% are specific facilities for disabled young people, and 3% are long-term care units for elderly people. Because each questionnaire contained space for information regarding one or two buildings, the final Excel database contained 2739 recordings.
3.1. Cleaning product storage
In more than half of the facilities, cleaning products are stored in a specific room that is non-adjacent to shared living rooms (53%) or to bedrooms (52%). The storage room is adjacent to the shared living rooms in 40% of facilities and adjacent to the bedrooms in about one-third of the facilities (29%). In both situations, the room is ventilated in most cases (72% and 79% for shared living rooms and bedrooms, respectively). In only 4% of the facilities, the cleaning products are stored in shared living rooms or in bedrooms. Information was not provided for 3% and 15% of the shared living rooms and bedrooms, respectively.
3.2. Cleaning frequencies and cleaning practices
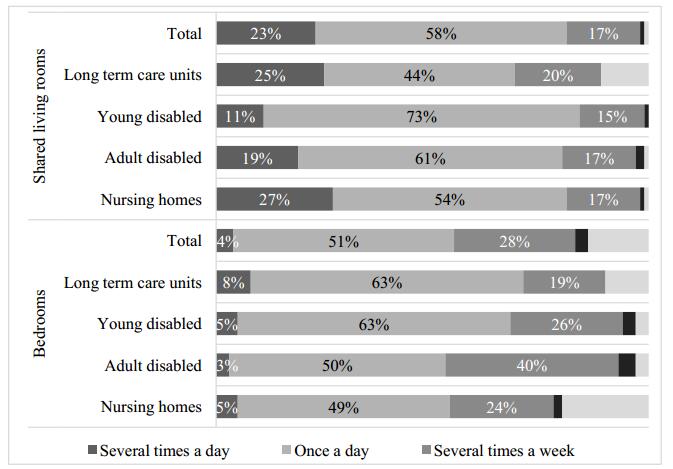
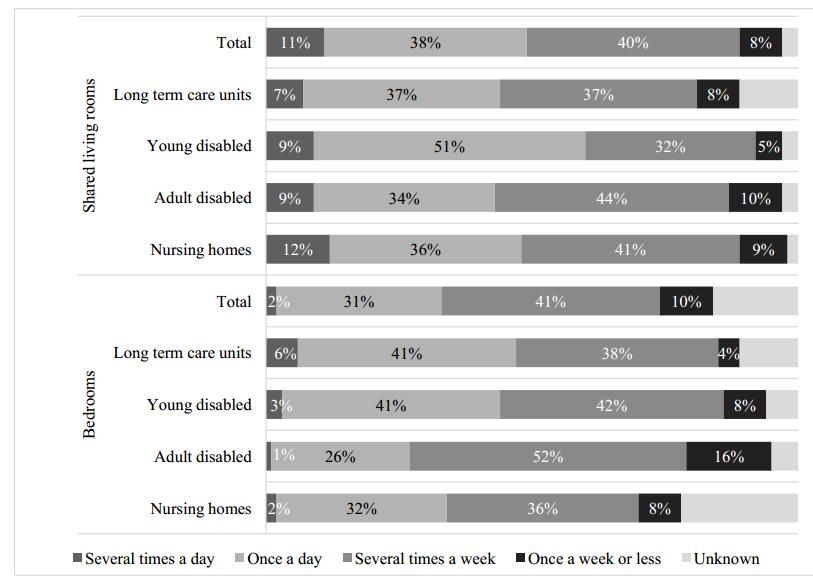
The cleaning frequencies vary according to the type of room. The floors of shared living rooms are cleaned once per day (58%) or several times per day (23%). In bedrooms, the floors are less frequently cleaned: once per day in 51% or several times per day in 4%. The surface cleaning frequencies are lower than the floor cleaning frequencies. Surface cleaning is mostly performed several times a week but not daily in both shared living rooms (40%) and bedrooms (41%). In approximately one-third of facilities, surfaces are cleaned several times per day (11%) or once per day (38%) in shared living rooms and several times per day (2%) or once per day (31%) in bedrooms. The cleaning frequencies are detailed according to the type of facility for floors in Figure 2 and for surfaces in Figure 3.
Two mains techniques of floor cleaning are highlighted: liquid cleaning products are used in 61% of shared living rooms and 50% of bedrooms, and wet wipes in 19% of shared living rooms and 23% of bedrooms. For surfaces, sprays are the most used cleaning technique in shared living rooms (36%) and in bedrooms (31%). In shared living rooms, liquid products are used in 29% of the facilities, and wet wipes in 22%. In bedrooms, the percentages of use are 22% for both liquid cleaning products and for wet wipes.
The resuspension of settled dust may have a detrimental impact on IAQ [1,35]. Cleaning with a wet mop and wipes avoids the resuspension of settled dust from floors or surfaces. However, VOCs can be emitted from the cleaning products on the wipes or used with the mop.
3.3. Use of air fresheners
Air fresheners are commonly used (66%) and can be a major source of indoor air pollution. Air fresheners are more frequently used in nursing or elderly homes (75%), long-term care units (71%) and specific facilities for disabled adults (57%) than in specific facilities for young disabled people (41%). This frequent use of air fresheners may be related to the frequent bad smells in these types of facilities, as they are employed to mask these odors. Air fresheners emit many chemical substances and contribute to the production of secondary pollutants [36].
3.4. Chemical substances in the reported cleaning products
Overall, 2739 buildings were described, and for each, up to three cleaning products could be reported. In the end, 2348 answers were registered corresponding to 1403 different wordings. After sorting these wordings, 1109 answers containing the brand and name of the product were usable, corresponding to 341 distinct cleaning products. Among these 341 products, 285 SDSs were available on the internet, 14 were received from the supplier by email, and 38 could not be found or were not received. Four cleaning products without SDSs were retained for analysis because of their simple formulations, i.e., methylated spirit, rubbing alcohol, white vinegar and bleach.
The analysis of the 299 SDSs and the four additional cleaning products (n = 303 products) allowed us to list 228 chemical substances. Among them, twelve refer to mixtures and could not be associated with a CAS number. Therefore, these twelve were not included in the final list. In the end, 216 chemical substances were considered. Among them, the 22 chemicals (10%) that were the most frequently present in the 303 products are listed in Table 1.
Table 1. Top 10% most frequent chemical substances in the reported cleaning products.
| CAS number |
Substance name |
Class |
Use |
Number of cleaning products |
Occurrence across the 303 cleaning products (%) |
| 67-63-0 |
Isopropanol |
VOC |
Solvent |
80 |
26.4% |
| 7173-51-5 |
Didecyldimethylammonium chloride |
Salt |
Disinfectant |
72 |
23.8% |
| 64-17-5 |
Ethanol |
VOC |
Solvent |
58 |
19.1% |
| 5989-27-5 |
D-Limonene |
VOC |
Scenting agent |
56 |
18.5% |
| 68424-85-1 |
C12-16-Alkyldimethylbenzylammonium chlorides |
Salt |
Detergent and surfactant |
41 |
13.5% |
| 78-70-6 |
Linalool |
VOC |
Scenting agent |
37 |
12.2% |
| 69011-36-5 |
Poly(oxy-1, 2-ethanediyl), alpha-tridecyl-gamma-hydroxy-, branched |
SVOC |
Solvent |
34 |
11.2% |
| 64-02-8 |
Ethylenediaminetetraacetic acid tetrasodium salt |
Salt |
Chelating agent |
30 |
9.9% |
| 2372-82-9 |
1, 3-Propanediamine, N-(3-aminopropyl)-N-dodecyl- |
SVOC |
Disinfectant |
29 |
9.6% |
| 68439-46-3 |
Alcohols, C9-11, ethoxylated |
SVOC |
Detergent and surfactant |
27 |
8.9% |
| 68891-38-3 |
Poly(oxy-1, 2-ethanediyl), alpha-sulfo-omega-hydroxy-, C12-14-alkyl ethers, sodium salts |
Salt |
Detergent and surfactant |
26 |
8.6% |
| 101-86-0 |
Hexyl cinnamic aldehyde |
SVOC |
Scenting agent |
25 |
8.3% |
| 1310-73-2 |
Sodium hydroxide |
Salt |
Detergent and surfactant |
23 |
7.6% |
| 106-22-9 |
Citronellol |
VOC |
Scenting agent |
21 |
6.9% |
| 5949-29-1 |
1, 2, 3-Propanetricarboxylic acid, 2-hydroxy-, hydrate (1:1) |
SVOC |
Disinfectant |
20 |
6.6% |
| 80-54-6 |
Lilial |
SVOC |
Scenting agent |
18 |
5.9% |
| 7664-38-2 |
Phosphoric acid |
Inorganic |
Detergent and surfactant |
18 |
5.9% |
| 68515-73-1 |
D-Glucopyranose, oligomeric, decyl octyl glycosides |
Non-volatile |
Detergent and surfactant |
18 |
5.9% |
| 79-33-4 |
L-Lactic acid |
VOC |
Detergent and surfactant |
17 |
5.6% |
| 5392-40-5 |
3, 7-Dimethyl-2, 6-octadienal (Citral) |
VOC |
Scenting agent |
17 |
5.6% |
| 2634-33-5 |
1, 2-Benzisothiazolin-3-one |
SVOC |
Disinfectant |
16 |
5.3% |
| 308062-28-4 |
Amines, C12-14 (even numbered)-alkyldimethyl, N-oxides |
Non-volatile |
Surfactant |
16 |
5.3% |
In comparison to previous studies, 14 substances (63%) of our top 10% most frequent chemical substances in the used cleaning products are also described in Gerster et al. (2014) [14], and 17 substances (77%) of our top 10% most frequent chemical substances in the used cleaning products were also reported by Wei et al. (2015) [16].
3.5. Hazard classification of the chemical substances in the reported cleaning products
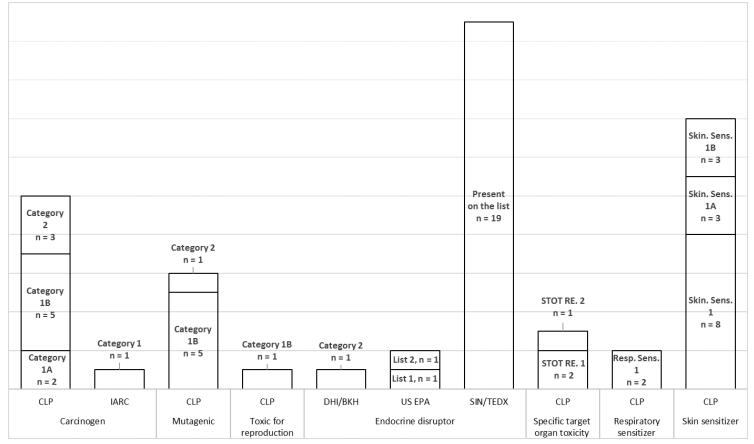
Among the 216 substances, 41 are classified for their health effects. Ten substances are registered as carcinogenic by at least one of the sources: 9 by the ECHA and 1 by both the IARC and the ECHA. Six substances are classified as mutagenic by the ECHA. One substance is toxic to reproduction. Nineteen substances are considered to have endocrine-disrupting properties by at least one of the sources: the 19 substances are present on the TEDX list, 2 on the SIN list, 2 on the US EPA lists, and 1 in the second category of the DHI/BKH classification. Three substances are registered as STOT-RE by the ECHA: 2 in the first category and 1 in the second category. Finally, the ECHA classifies 2 substances as respiratory sensitizers and 14 as skin sensitizers. The detailed results are presented in Table 2 and Figure 4.
Table 2. Hazard classifications of the 41 chemical substances in cleaning products used in French age care and social facilities.
| Substance name |
CAS number |
Class |
Occurrence (%) (1) |
Carcinogenicity |
Mutagenicity |
Reproductive toxicity |
Endocrine disruption |
STOT-RE |
Sensitization |
| Skin |
Respiratory |
| Didecyldimethylammonium chloride |
7173-51-5 |
Salt |
23.8 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| D-Limonene |
5989-27-5 |
VOC |
18.5 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| Ethanol |
64-17-5 |
VOC |
19.1 |
* |
NA |
NA |
TEDX |
NA |
NA |
NA |
| Linalool |
78-70-6 |
VOC |
12.2 |
NA |
NA |
NA |
NA |
NA |
CLP 1B |
NA |
| Lilial |
80-54-6 |
SVOC |
5.9 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| 2-Methyl-3(2H)-isothiazolinone |
2682-20-4 |
VOC |
4, 6 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| Benzyl salicylate |
118-58-1 |
SVOC |
4.3 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| 1, 6-Hexanediamine, polymer with N, N'''-1, 6-hexanediylbis [N'-cyanoguanidine], hydrochloride |
27083-27-8 |
Non-volatile |
2.0 |
CLP 2 |
NA |
NA |
NA |
CLP 1 |
CLP 1B |
NA |
| 1, 2-Benzisothiazolin-3-one |
2634-33-5 |
SVOC |
5.3 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| 3, 7-Dimethyl-2, 6-octadienal |
5392-40-5 |
VOC |
5.6 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| Ethanolamine |
141-43-5 |
VOC |
4.0 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| 2-(2-Butoxyethoxy)ethanol |
112-34-5 |
VOC |
3.0 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| 2-Phenylphenol |
90-43-7 |
SVOC |
0.3 |
NA |
NA |
NA |
DHI/BKH 2 |
US EPA 1 |
TEDX |
NA |
NA |
NA |
| Bio-Perge |
55965-84-9 |
VOC |
2.6 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| Bronopol |
52-51-7 |
SVOC |
2.3 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| Glutaraldehyde |
111-30-8 |
VOC |
1.0 |
NA |
NA |
NA |
TEDX |
NA |
CLP 1A |
CLP 1 |
| Limonene |
138-86-3 |
VOC |
1.0 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| 1-Methoxy-2-propanol |
107-98-2 |
VOC |
1.3 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| 2-Butoxyethanol |
111-76-2 |
VOC |
1.3 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| Octhilinone |
26530-20-1 |
VOC |
1.3 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| Methyl ethyl ketone |
78-93-3 |
VOC |
0.7 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| Ethylene glycol |
107-21-1 |
VOC |
0.7 |
NA |
NA |
NA |
US EPA 2 |
TEDX |
NA |
NA |
NA |
| Methanol |
67-56-1 |
VOC |
0.7 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| (Z)-9-Octadecenylamine |
112-90-3 |
SVOC |
0.7 |
NA |
NA |
NA |
NA |
CLP 2 |
NA |
NA |
| Butane |
106-97-8 |
VVOC |
0.7 |
CLP 1A |
CLP 1B |
NA |
NA |
NA |
NA |
NA |
| Poly (hexamethylene biguanide) hydrochloride |
32289-58-0 |
SVOC |
0.3 |
CLP 2 |
NA |
NA |
NA |
CLP 1 |
CLP 1B |
NA |
| Isobutane |
75-28-5 |
VVOC |
0.7 |
CLP 1A |
CLP 1B |
NA |
NA |
NA |
NA |
NA |
| Glycine, N, N-bis(carboxymethyl)-, trisodium salt |
5064-31-3 |
SVOC |
0.3 |
CLP 2 |
NA |
NA |
NA |
NA |
NA |
NA |
| Cyclopenta[g]-2-benzopyran, 1, 3, 4, 6, 7, 8-hexahydro-4, 6, 6, 7, 8, 8-hexamethyl- |
1222-05-5 |
SVOC |
0.3 |
NA |
NA |
NA |
SIN |
TEDX |
NA |
NA |
NA |
| Distillates, petroleum, hydrotreated light paraffinic |
64742-55-8 |
VOC |
0.3 |
CLP 1B |
NA |
NA |
NA |
NA |
NA |
NA |
| dl-Carvone |
99-49-0 |
VOC |
0.3 |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
NA |
| Formaldehyde |
50-00-0 |
VVOC |
0.3 |
CLP 1B |
IARC 1 |
CLP 2 |
NA |
TEDX |
NA |
CLP 1 |
NA |
| Naphtha, petroleum, hydrotreated light |
64742-49-0 |
VOC |
0.3 |
CLP 1B |
CLP 1B |
NA |
NA |
NA |
NA |
NA |
| Naphtha, (petroleum) hydrodesulfurized light, dearomatized |
92045-53-9 |
VOC |
0.3 |
CLP 1B |
CLP 1B |
NA |
NA |
NA |
NA |
NA |
| Heptane |
142-82-5 |
VOC |
0.3 |
NA |
NA |
NA |
TEDX |
NA |
NA |
NA |
| 4-(4-Hydroxy-4-methylpentyl) cyclohex-3-ene-1-carbaldehyde |
31906-04-4 |
SVOC |
0.3 |
NA |
NA |
NA |
NA |
NA |
CLP 1A |
NA |
| 3-(4-Hydroxy-4-methylpentyl) cyclohex-3-ene-1-carbaldehyde |
51414-25-6 |
SVOC |
0.3 |
NA |
NA |
NA |
NA |
NA |
CLP 1A |
NA |
| Naphtha, petroleum, hydrotreated heavy |
64742-48-9 |
VVOC |
0.3 |
CLP 1B |
CLP 1B |
NA |
NA |
NA |
NA |
NA |
| Octoxynol-9 |
9036-19-5 |
SVOC |
0.3 |
NA |
NA |
NA |
SIN |
TEDX |
NA |
NA |
NA |
| Sodium perborate tetrahydrate |
10486-00-7 |
Inorganic salt |
0.3 |
NA |
NA |
CLP 1B |
NA |
NA |
NA |
NA |
| Subtilisin |
9014-01-1 |
Non-volatile |
0.3 |
NA |
NA |
NA |
NA |
NA |
NA |
CLP 1 |
(1): occurrence across the 303 different cleaning products.
*Specific classification: IARC Category 1 in alcoholic beverages only |
3.6. Limitations of the study
Among all the questionnaires registered (N = 2348), 1239 responses were not usable because of the lack of information provided by the interviewees. Most of the time, only the brand names were given, and some product names were often wrong or incomplete. The part of the questionnaire regarding the identification of the cleaning products used should have been more detailed to ensure a complete answer. Moreover, 38 SDSs were missing or were not received from the supplier after request.
The reported substances were determined from the SDSs, but the chemical compositions of the cleaning products are not fully listed in SDSs. The manufacturers must declare the compositions of hazardous substances only when their concentration is higher than 0.1% in the formulated products. Several substances with low concentrations in cleaning products were thus outside of the scope of this study. Moreover, the SDSs are declarative, and the reported information must be taken with caution (e.g., the wrong CAS number for eucalyptol was found in an SDS).
Despite these limitations, this work allowed us to list numerous substances in cleaning products with health effects and improved the knowledge of VOCs likely to be present in the indoor air of age care and social facilities.
4. Conclusion
This study showed that (1) the cleaning frequency is rather high in health care and social facilities, with floors being cleaned once or several times per day in most cases and (2) liquid cleaning products, wet wipes, and sprays are commonly used in all types of facilities and all types of rooms. The use of air fresheners is also very common. Among the cleaning products, 341 different commercial references were identified, and information about the compositions of 303 could be retrieved. Among the 216 different chemical substances listed, 41 were classified for their potential health effects: 10 as carcinogenic, 6 as mutagenic, 1 as toxic for reproduction, 19 having possible endocrine-disrupting properties, 3 as toxic for a specific target organ under repeated exposure, 2 as respiratory sensitizers, and 14 as skin sensitizers. Of these 41 chemicals, 10% (n = 4) are VVOCs, 51% (n = 21) are VOCs, 29% (n = 12) are SVOCs, 5% (n = 2) are not volatile, 5% (n = 2) are salts. The VOCs emitted from the cleaning products used in these facilities will be considered for measurement during the upcoming nationwide monitoring campaign.
Acknowledgements
We thank the French Ministries of Health (Grant 2016) and Environment (Grant 2201026654) for supporting this study.
We thank People Vox for managing the online questionnaire and all the French age care and social facilities who responded and contributed to the survey.
Conflicts of interest
The authors declare that they have no conflict of interest.









 DownLoad:
DownLoad: