1.
Introduction
Computer architecture and computing paradigms play a vital role in fast, reliable, and power-efficient computing applications. The traditional Von Neumann architecture-based computations have evolved into very sophisticated and reliable computing sources but the present-day requirements of big data applications, central processing units' (CPUs') performance, and memory latency gaps have an ever-growing impact on the overall performance of such systems. Apart from the memory wall problem, the scaling of transistors such that the number of transistors on a chip doubles every 18 months, known as Moore's law, has already undergone saturation.
Thus, a growing field of research work is directed towards finding new computing paradigms [1]. Implementing computing systems that mimic the human brain and biological systems have already been an inspiration for researchers in the area but the interest in such endeavors has increased greatly in recent years. Neuromorphic computing [2], that is, mimicking the human brain on computing chips, has therefore received great attention. However, the level of abstraction used to model the human nervous system remains an area of exploration for researchers in this field.
The human brain can be modeled on a neuromorphic chip by simulating the neo-cortex with electrical synapses and their encodings or at a deeper level by including both electrical and chemical synapses, along with the biochemical pathways within neuronal cells [3]. For the latter approach, another domain of research called cytomorphic computing, where electronic modeling of different biocellular pathways is done, comes into play, as many such pathways commonly exist even within neuronal cells [4]. Besides providing a means to accurately simulate and/or emulate a biological process on a semiconductor substrate for computing benefits, such implementations give a profound insight into biological and neurological processes that benefit not only pharmacologists for drug testing but also researchers working in the field of computational biology, synthetic biology, neuroscience, and biomedicine [5].
This paper explores the modeling of biocellular processes and neural pathways on analog, mixed-signal, and digital hardware. Extensive research has been carried out in the field of cytomorphic and neuromorphic computing over the past few decades. Cytomorphic computing [6] is understood as a biocellular process modeled on an electronic platform, and the term neuromorphic computing [2],[7],[8] refers to the modeling of biological neural pathways on an electronic substrate. The modeling and simulations of these neural and biological processes can be carried out at various levels of abstraction, as well as by using various electronic platforms [3].
In this survey, we intend to explore bottom-up implementations of these processes on electronic substrates at various levels of abstraction. Section 2 explores the analog domain implementations of these cytomorphic and neuromorphic chips. Section 3 discusses mixed-signal integrated circuit (IC) fabricated and the related work for simulating biocellular and neural pathways. Section 4 focuses on digital implementations for the biological modeling of cellular and neural networks including field-programmable gate array (FPGA)-based implementations that support reconfigurable computing. We have explored more than 100 papers in the three domains of analog, mixed-signal, and digital implementations and have extracted 68 highly relevant research endeavors in the past 16 years from 2008 to 2023 with respect to bioplausibility, abstraction level, and the chosen electronic platform. We have considered the timeframe of 16 years, since in this era, we have witnessed an ever-increasing trend of researchers in the field exploring new paradigms of computing. This is primarily due to performance saturation in the traditional methods of computing and the ever-increasing energy efficiency requirements due to the Internet of Things and the enormous amounts of data available for processing. The increasing trend of cytomorphic research alongside the already pervasive field of neuromorphic computing has been listed and analyzed. This survey paper is thus an attempt to bridge the gap between the fields of cytomorphic and neuromorphic computing in order to assist active researchers increase their biorealistic realizations. This kind of survey is apparently not present in the existing literature so far. Section 5 discusses these trends and lists the most important work in analog, mixed-signal, and digital platforms. Section 6 concludes the survey.
2.
Analog design for biological and neural pathways
Implementing biocellular processes and neural pathways on an analog substrate can range from a low-level approach, where complementary metal-oxide semiconductor (CMOS) transistors operate in the sub-threshold region, to a higher-level approach, where transistors function above the threshold and their tunable parameters are mapped to mathematical models of the biocellular process [3].
The analog design in the sub-threshold region emulates a biocellular process with a real time constant similar to the biological time constant, whereas in the above-threshold region, as the device operates with a drift current instead of a diffusion current, we get an accelerated time constant of 103 to 105 times more than the biological clock and thus the simulation would give much faster results compared with biophysical modeling in the sub-threshold operation [9],[10].
In this section, we analyze different techniques used for analog emulations/simulations in sub-threshold and above-threshold modes of operation.
2.1. Analog CMOS implementations in the sub-threshold region of operation
Some of the pioneering work in the field of cytomorphic and neuromorphic analog designs using the below-threshold region of CMOS can be seen in [11] by Maher et al. Here, the researchers have used analog CMOS very large scale integration (VLSI) technology to develop visual and motor sub-systems with basic components so that they can be combined in a hierarchical manner to create larger biological systems. Metal-oxide semiconductor (MOS) transistors in a weak inversion layer regime are used to build analog circuits such that the transistors conduct using diffusion currents and the drain current is exponential to the gate voltage. Since the currents in such circuits are in the range of 10−12 to 10−7A, power consumption is quite low. Hence, such transistor models are used to compute the exponential functions quite often required in the implementations. Using this transistor model, a nine transistor-based transconductance amplifier is used for various functions in the design. Utilizing basic square root and logarithmic properties of transistors and making many circuits using the transconductance amplifier, like the unity-gain follower, the half-wave rectifier, the Gilbert transconductance multiplier, and other trans-linear circuits, analog circuits are implemented to serve the purpose in the neural sub-systems designed.
VLSI chips are also fabricated to use the output of the stimulus in an image to generate frequency encoding for driving a motor system. This chip works by computing a useful function of an image, namely its center of intensity, and utilizes it with a servo motor system to perform the ocular movement. Some other work related to this work, as reported by Maher et al., includes the uniform motion achieved [12], depth from stereopsis [13], center of intensity, and edge orientation [14]. Hutchinson et al., in [15], used resistive networks to interpolate, smooth, and enhance the edges in noisy samples. Lyon et al., in [16], discussed an analog cochlea from the owl that is used with binaural auditory localization in an auditory localization system [17]. Similarly, in [18], Sarpeskar et al. proposed a 45-stage VLSI cochlea with a transconductance amplifier with a wide linear range in the sub-threshold range, which exhibits low noise. It also incorporates nonlinear positive feedback amplification for second-order filtering in the cochlea. This fuse filter models the function of hairs in the ears, serving as gain control.
Andreou et al. [19] proposed a device-level approach for neural circuits using sub-threshold CMOS VLSI with current-controlled current conveyors and the translinear principle, eliminating the need for the transconductance amplifiers or differential voltages used in [11]. Demonstrated with associative memory circuits and a silicon retina system, this method optimizes power and area efficiency, minimizes global communication, and directly maps voltage-gated ionic channels to MOS transistors while reducing device mismatch concerns. As the current-controlled current conveyor relies on negative feedback, and the excitatory and inhibitory channels on the receptors also form a negative feedback loop, there is strong correlation between the modeling technique and the biological counterpart. In [20], Moore et al. used an improved version of Land's Retinex theory-based algorithm in an implementation of CMOS VLSI at the sub-threshold level to solve the problem of color constancy in video images. Neurobiological understanding, computational biology standards, and electronic substrate parameters at the device level are taken into account such that not only are tools to simulate the latest algorithms developed but simulations are also done using a resistive grid instead of convolution. As a result, a simulation that had taken about an hour to take place could be done in a minute. A sample-and-hold technique is used in the design. The circuit details are same as those given in [2] by Mead. In [21],[22], Boahen and Andreou established yet again a current-mode-based CMOS circuit to implement the outer plexiform layer of the vertebrate retina, which is contrast-sensitive.
Chemical synapses are modeled using CMOS transistors in saturation mode, with the input currents representing synaptic signals. In this state, the MOS transistor acts as a current source, driving the post-synapse through a voltage-controlled presynapse. Similarly, the cone outer segment is modeled by a bipolar phototransistor in saturation mode, generating a current proportional to the light intensity, forming a one-dimensional current-mode silicon retina circuit. Asai et al. [23] implemented an analog circuit using sub-threshold MOS transistors to realize a Lotka–Volterra competitive neural network, which selects among the external inputs. In biological neural networks, mutual inhibition ensures that only certain neurons remain active while others are suppressed, depending on the received stimuli, forming the foundation for neuronal decision-making and motor command selection.
Mandal et al. discussed the implementation of active radio frequency in a silicon cochlea and presented the mechanism through which the biological cochlea performs spectral analysis. The radio spectrum from 600 MHz to 8 GHz can be handled by the chip, which is implemented using 0.13 micron CMOS technology [24],[25], is shown in the work of Hossein et al., where nonlinear Bernoulli cell formalism is used to mimic the model of the biochemical network of cyclin-dependent kinases (CDKs) that drives the cell cycle. Sub-threshold metal-oxide semiconductor field effect transistors (MOSFETs) are used to emulate the ordinary differential equation (ODE)-based CKD model. In [26], Yang and his colleagues give a complete neural network implementation with spiking time-dependent plasticity (STDP) and leaky integrate-and-fire (LIF) features using sub-threshold CMOS VLSI. Sharma and Dhanoa in [27] discussed the 14 analog CMOS transistor-based implementations of a cortical neuron. These neurons can give different patterns of spikes like regular, fast, and burst-mode spikes besides being power-efficient and taking up very less space on the chip compared with a previous implementation comprising 20 MOSFETs. In their work in [28], Ronchini et al. designed an analog CMOS-based neuron that was not only more energy-efficient but also area-efficient than the rest of the contemporary designs. Izhikevich's neuron model was used. Spiking time constants are biological rather than accelerated in this work. Aghar et al., in [29], designed an analog CMOS implementation for the “integrate and fire” model of neuron using a current multiplier charge-injector-based synapse. Oren et al. [30] employed a biomorphic chip design approach, using ultra-low-power circuits inspired by gene networks to create a biomolecular neuron. This neuron, designed with cytomorphic principles, functions like a perceptron in artificial intelligence (AI). By utilizing sub-threshold MOSFETs, the resulting artificial neural networks achieve high energy efficiency. In [31],[32], Beahm et al. suggests the use of cytomorphic chips to be used for drug cocktail formulation for various diseases like COVID-19.
2.2. Analog CMOS implementations in saturation or triode regions of operation
In [5], Waqas et al. used a velocity saturated short-channel MOS transistor to model very frequently seen biocellular reactions, namely receptor–ligand binding and Michaelis–Menten reactions. Using the mathematical models established as ODEs for these reactions, a set of analogies were created for the electronic attributes of MOS transistors, and thus, these were successfully modeled on silicon substrates using very few transistors. On the basis of this information, a receptor–ligand binding circuit was designed by utilizing adder/subtractors, multipliers, and integrator blocks. Waqas et al. also incorporated another commonly found biochemical reaction in biological pathways called the Hill process and gave a comparison of two such circuits to model cooperativity in the process [33]. In [34], Waqas et al. further extended her work in [5],[33] to propose an analog MOS-based design of a complete biochemical pathway called the cyclic adenosine monophosphate (cAMP)-dependent pathway. An analog circuit at a pathway level using analog MOS transistors in a modular fashion was proposed for the first time.
In [35], the p53 protein led pathway responsible for cell death called apoptosis was examined by Patra et al. Using ODEs of the system model, CMOS circuits of the entire pathway were implemented. Mathematical models were derived on the basis of the law of mass action and Michaelis–Menten kinetics. Each block of the system was converted to a CMOS circuit and evaluated for its correctness against MATLAB-based simulations and the results of biological experiments conducted previously. This design allows for the study of p53 pathways dynamics without the need for biological cells in a dry lab and would benefit cancer treatment and drug control for such diseases.
3.
Mixed-signal design for biological and neural pathways
By mixed-signal IC design, we refer to circuits where the basic implementation of biocellular processes and cellular structures like neurons and synapses are done using analog circuit elements but the on-chip communication, memory storage to handle weights of the synapses, and real-world interfacing are done through digital means. Moreover, for scalability purposes and parallelism in the larger structures, just like in their biological counterparts, a multicore architecture or network of chips (NoC) techniques may be used in the digital domain alongside analog implementations [1]. We also see some switched-capacitor-based designs implemented as mixed-signal fabrications, where, for scalability purposes, digital control and digital memory are incorporated along with biophysical implementation of synapses through switched capacitor-based circuits [3]. In [9], Schemmel et al. modeled analog-based synaptic plasticity while choosing a leaky integrate and fire neuron that produced digital spikes as action potentials. Two plasticity mechanisms (short-term and long-term) were incorporated in the design. Short-term variables in the design are represented by capacitive storage, and digital memory is used to handle synaptic weights. Schemmel et al., in [10], presented BrainScaleS-2, a mixed-signal inter-wafer-scale neuromorphic system. The LIF neuron model with programmable ion channel emulation and inter-compartmental conductance allowed the modeling of nonlinear dendrites, back-propagating action potentials, and N-methyl-D-aspartate (NMDA) and calcium plateau potentials.
There has been integration of a digital plasticity processing unit, a highly parallel microprocessor specially built for the computational needs of learning in an accelerated analog neuromorphic system. Hasan, in [36],[37], developed a CMOS circuit to model signal reception and transduction within a biological cell. The logical faults in the circuit mimic a cellular malfunction (i.e., a disease). The states of D flip-flops can be taken as the intermediate steps in the signal transduction pathway, which maintain control over the molecular interactions such as phosphorylation and binding. In [38], Hasan came up with a mixed-signal IC model to represent DNA–protein regulatory genetic circuits and state machines. He proposed a repressible transcription circuit using a p-channel metal-oxide semiconductor (pMOS) pass transistor and a positive gene regulation through an n-channel metal-oxide semiconductor (nMOS) pass transistor. He created a map of the genetic circuit variables to the electronic circuit's attributes. The transcription rate for a particular concentration of RNA polymerase was determined by a current mirror. In [39], Schemmel et al. discussed a European research project called fast analog computing with emerging transient states (FACETS), in which various neuromorphic systems are considered, with the emphasis on being helpful to neuroscientists. For this, a software/hardware platform was devised that uses a standard neural system description language known as PyNN. Using PyNN, a neuroscientist can easily describe a neural model and simulate it either on neuromorphic hardware or on a computational simulator. Hence, to achieve this goal, an analog neuromorphic system at a very large scale was developed under FACETS. It can model 10,000 synapses to one neuron. In this implementation, the standard integrate and fire model is modified to the exponential integrate and fire model called AdExp by making new additions to it. This model works at accelerated biological time, thus reducing internal capacitances, increasing leakage currents, and reducing the circuit sizes. Each synapse is organized to incorporate a four-bit address decoder interface with any one of four enable signals. As a result, the synapses in a column may receive inputs from 64 presynaptic points. Two juxtaposed columns share the same inputs. A four-bit multiplying digital-to-analog converter produces current to represent the synapses' weight. A four-bit static random access memory holds each synapse's weight values. Folowosele et al., in [40]–[42], discussed the simulation and silicon substrate modeling of the Mihalas–Niebur neuron model, which exhibits almost all types of spiking behaviors that can be found in biological neurons. The study in [42] explores the first implementation of a chip for the model and it encompasses 10 of the spiking behaviors. This model not only depicts variable thresholds but it also has biologically conforming parameters while using spike-inducing currents. The main advantage of this model, however, is the presence of linear equations for spike-induced currents and threshold variation to include many spiking patterns.
A switched capacitor implementation is attained by modifying the equations of the model to be linear. In [43], Mandal et al. proposed a stochastic genetic circuit based on prior work, where they used log-domain transistor circuits for modeling chemical reactions. The researchers mapped biochemical reactions using a sub-threshold regime of transistors and implemented fast and scalable genetic pathways through a digitally programmable connection matrix. Bamford et al., in [44], presented a mixed-signal VLSI circuit based on the spike timing-dependent plasticity (STDP) rule, and the design includes parallel interfacing so as to incorporate a large number of synapses. The main emphasis of this work is to establish many variations of weight dependence of STDP. Leakage of capacitance for the transistor that holds the weight is addressed by incorporating reverse-biased transistors. Alam and Hasan, in [45], created a mixed-signal VLSI implementation for post-transcriptional stages in the gene expression of biological systems and presented an electronic substrate mechanism of how a protein decays in the pathway. The design gives insight into how microRNA and the proteasome regulate gene expression. mRNA degraded by microRNA is modeled by the emitter's degeneration, and protein is decayed by the proteasome designed by a mixed-signal CMOS circuit. Benjamin et al., in [46], discussed a large-scale mixed-signal neuromorphic system called NeuroGrid, where all the neural elements except the soma were created using shared electronic circuits to maximize synaptic connections. All neural elements except axonal parts were designed in the analog domain for energy efficiency requirements, and a tree network was used as the topology for synaptic connections. In [6], Woo et al. presented a cytomorphic chip using 0.35-µm bipolar complementary metal-oxide semiconductor (BiCMOS) technology to model basic biochemical entities using log-domain transistors that represent cascade and fan-out topologies, as in the Hill coefficient operation, or in DNA binding of gene transcription and other such cellular aspects. The various chips interact and communicate through analog-to-digital converters and digital-to-analog converters, thus allowing scalable biochemical pathways using the fundamental circuits. Woo et al. extended his work in [6] by incorporating digital programmable inter-chip communication among the fundamental entities of the biocellular processes using FPGAs [47]. By amplifying the inherent thermal noise in circuits depicted in [6],[47], the complex simulation of noise through Poisson process generation can be avoided. This idea has been demonstrated in [48] by Kim et al.
In [49], Mayr et al. proposed a switched capacitor-based neuromorphic system that can interface with in vitro biological systems and can benefit from digital interfacing. It is an extremely low-power 28-nm CMOS technology-based implementation that uses a 1 V power supply. Based on the work of Rolls and Mayr [50], Noack et al. [51] implemented memory and decision-making mechanisms. The model for short-term dynamics, as given in [52], is used to implement transistor-level switched capacitor circuits that are induced from the high-level blocks. The basic synapse structure is deduced from the work of Noack et al. [53]. Since this design is intended to be interfaced primarily with biological interfaces, longer time constants are required but, due to leakage in the switching transistors, it cannot be achieved; hence, the circuit designs [54],[55] by Elguth and Ishida are utilized. The system can be configured digitally and can easily be interfaced with low-power digital systems. This design incorporated more of the neurons than in the prior implementations and is configurable. Aamir et al., in [56], presented a biologically plausible extension to the adaptive exponential IF neuron model for the BrainScaleS hardware for its second-generation implementation. This design facilitates large-scale implementation of neuromorphic hardware and is designed on the basis of an existing AdEx model [57]–[59]. The design integrates exponential and adaptive circuits into a modular LIF neuron, enabling diverse spike patterns, including exponential bursts. It incorporates multicompartment emulation with passive and active dendrites, as well as sodium and potassium spikes with distinct refractory periods. This physiologically plausible, reconfigurable design operates 1000 times faster than biological time constants. In [60], Aamir et al. demonstrated an array of LIF neurons along with thorough measurements and meticulous calibration results. A transconductor mechanism was used that nullified the residual offsets from the synapses' outputs. Digital spikes and refractory periods in spiking are included in the design. Neckar et al. [61] developed Braindrop, a mixed-signal neuromorphic system that abstracts hardware synthesis from user-defined nonlinear differential equations. Each equation is represented by a neuron pool, leveraging the neural engineering framework (NEF) to accommodate and utilize mismatches. The design combines quasi-delay asynchronous digital logic with sub-threshold current-mode analog techniques. The work of Teo et al., in [62] presented an artificial tissue homeostasis implementation in analog circuits using feedback system analysis to control stem cells' proliferation and differentiation. A comprehensive review of cytomorphic systems was provided in [63] by Beahm et al. Rubina et al. [64] presented a mixed-signal power-efficient implementation of neurons and synapses that uses the features of advanced fully depleted silicon on insulator (FDSOI) integration processes. Using this technology, Rubino et al. tried to mitigate the analog design issues and designed the adaptive LIF neuron and synaptic circuits in an optimized fashion. The designed circuit incorporates bioplausibility and is optimized for the large-scale spiking neural networks to be used in edge devices. Jo et al. [65] came up with a Poisson process generator using Verilog-A by incorporating multiple thermal noise amplifiers as a source of randomness to simulate stochastic biochemical reactions. The fundamental block of biochemical reactions comprises continuous-time multiplication and addition using an AND gate and a 1-bit current-steering digital-to-analog converter respectively. Zhao et al. [66] presented a compact and power-efficient amplified bipolar junction transistor (BJT) white noise and adaptive low-pass filtering to be used in stochastic simulations where the emphasis is on biochemical processes.
4.
Digital design for biological and neural pathways
In the implementation of biocellular pathways, an analog design is preferred, as it is more aligned with the already existing analog world. The neural systems and biochemical processes within cells are inherently fuzzy and noisy, but the design process in the analog domain is tedious and very costly, and hence, many researchers in the domain prefer to keep their design in either the digital or mixed-signal domain.
Many different digital platforms can be used to emulate and/or simulate neural and biological systems. The most common methodology used to simulate biophysical processes is to use an in silico implementation called executable biology, but such simulations require a lot of power and computational resources and have speed limitations. Apart from the Von Neumann architecture, graphical processing units (GPUs) are often used for such implementations. A more popular way of designing such systems is through full-custom digital designs or to make the design reconfigurable. Field-programmable gate array (FPGA)-based designs are often taken into account. Digital designs have an edge over analog counterparts in the fact that they are less prone to noise, -Process–voltage–temperature (PVT) variations, and device mismatch issues. Furthermore, if the design is meant to be interfaced with digital devices, this domain is a better choice.
4.1. GPU-based implementations
Wang et al. [67] implemented a GPU-based Hodgkin and Huxley (HH) neuronal model to reduce the high computational cost of in silico simulations. The HH model was chosen over simpler spike-based models due to its biological and physiological accuracy. The design utilizes a look-up table approach with high arithmetic intensity and minimal thread divergence. An improved mathematical integration mechanism was developed to capture the model's dynamic characteristics. This implementation successfully simulated a network of one million neurons and 200 million synapses on a GPU with 240 streaming processors, achieving a 600-fold increase in speed over in silico simulations, 28 times higher than CPU-based models, and only 2–3 times slower performance than GPU implementations of more abstract neuronal models. Igarashi et al. [68] presented a biologically plausible real-time implementation of basal ganglia circuits using general purpose graphical processing units (GPGPUs). The model incorporates neurophysiological and structural aspects, consisting of 370 spiking conductance-based neurons and 11,002 synapses. Leveraging the parallel processing capabilities of GPUs, the design achieved time-scaled simulations while utilizing only one-third of the available resources. Three instances of the basal ganglia circuits were implemented using the full GPU capacity, with visualizations rendered on custom simulator software. However, the design lacks scalability.
4.2. Performance analysis using sequence formulation techniques
When it comes to digital implementation, one of the two most important approaches is to have an application-specific integrated circuit (ASIC) design, but this renders the design rigid, and the fabrication process incurs a huge cost. Another approach is to use reconfigurable platforms like FPGAs. Neuromorphic designs or cytomorphic designs at a higher level of abstraction are much easier to carry out using FPGAs, but using the bottom-up approach, where physical dynamics of the cellular process are taken into account, poses a huge challenge. Nonetheless, we can still appreciate the researchers in the field who have come forth with tremendous expertise that have paved new ways of modeling such systems. Our focus while discussing such endeavors is the implementation at lower levels of abstraction. Yamaguchi et al. [69] discussed the implementation of the spatiotemporal dynamics involved in the modeling of a whole cell in a biorealistic manner. The Brownian motion that the protein molecules undergo within a cell were taken care of through a Monte Carlo simulation methodology. An FPGA-based system was designed so as to enable the whole cell pathway to be modeled. The mitogen-activated protein kinase (MAPK) cascade, which is very commonly found in cellular pathways, was modeled using the technique described. Then a cell division cycle was modeled. In [70], Yamaguchi et al. further extended their work by modeling at a mesoscopic level with a two-phase processing (migration and reaction) pipelined circuits. Mak et al. provided a framework to model neuronal ion channels in [71] with real-time dynamics involved so that neuroprosthetic devices may benefit. Usually, while modeling ion channels, functions such as exponentiation and division are carried out using look-up tables [72]–[75], but in this component-based framework, mathematical algorithms for these operations are first implemented in the logic, so it is a memoryless implementation. Hasan [76] proposed a CMOS-based sequential circuit as a biomimetic chip to implement phagocytosis. Phagocytes produce antigens in some micro-organisms. Phagocytes are essentially white cells or their derivatives. The process through which phagocytes are activated is called phagocytosis. Hasan's paper discusses the modeling of phagocytosis with digital CMOS technology. Binding, hydrolysis, and breakdown of the biochemical pathway are modeled using delay flip-flops that are registers to indicate the state of these molecular interactions and are a major part of the process. Weinstein et al., in [77], suggested the use of hardware–software co-simulation to produce FPGA-based implementation of biologically plausible neural network. Weinstein et al. proposes a methodology based on Xilinx System Generator to ease the process of designing such systems on FPGA. The design comprises 40 neurons based pre-Bötzinger complex population model with HH style conductances in a fully connected synapse mesh. Blocks like adders, subtractors, multipliers, ROM, register, delay and counter were used for the Piecewise Linear Approximation (PLA)-based implementation. In [78], Soleimani et al. furnishes his digital FPGA-based implementation of the spiking neural network (SNN) and used a piecewise linear model which is a modified version of Izhikevich's model. Multiplication operations are replaced by add or shift logic so that more neurons can be packed on the FPGA, allowing larger systems.
Cassidy et al. [79] presented a neuromorphic design framework that mirrors the layered parallel architecture of biological brains. The framework implements spiking neurons and STDP learning, with neurons abstracted as arithmetic logic units and digital processors for communication. The design efficiently integrates millions of neurons on a single FPGA. To validate the approach, the authors incorporated neurons, STDP synapses, and a parallel communication architecture into a cortical development circuit. The framework also supported multiple abstraction levels, allowing both LIF and Izhikevich neuron models to coexist within the same design. Nazari et al. [80] discussed the modeling of one of the most important types of cell in neuronal networks, namely astrocytes. These are a type of glial cell, and they play an essential role in processing in the neural network and also in synaptic transmission aspects. The intracellular Ca2+ oscillations produced by astrocytes are modeled by considering the neuron–astrocyte interactions found in the neural pathways of a biological brain. Linear approximation method is used in the design. Another very interesting model of a very important structure of the brain known as inferior olivocerebellar neurons was studied in [81] by Smaragdos et al. These neurons are responsible for sensorimotor learning skills and processing. Directly modeling this population of neurons on an electronic substrate is intricate and complex, so high-level synthesis of Xilinx Vivado was deployed. A very detailed biophysical model in MATLAB is ported to C code and then the high level synthesis (HLS) tool is used to synthesize the model to be mapped onto an FPGA. Some optimizations are also performed alongside. A 45-fold increase in speed was achieved against the original MATLAB code and was 12.5 times higher than the C code running on a Von Neumann-based CPU. In [82], Moctezuma et al. provided a methodology to implement biologically plausible neural networks on an FPGA so that the model is simpler to implement as compared with the HH model yet does not forgo any biological information in the implementation. The simplified model described in [83] by Pinsky et al. is deployed for the conductance-based neuron model. A soma-like compartment is where the Na1+- and K1+-activated currents reside, and the second compartment handles Ca2+-activated and K1+-dependent currents corresponding to dendritic structures. In [84], Yang et al. puts forth a four daisy-chained FPGA-based design of a modular small world model for a spiking neural network. The LIF neuron model with electrical and chemical synapses was used.
Deng et al. [85] implemented 13 kinds of motifs with pipelined structures and the synchronization properties of motif-based small world networks that were inspected from the perspective of network size, rewiring probability, and coupling strength. The Hindmarsh–Rose neuron model was deployed, which is based on the FitzHigh–Nagumo model. Rahimian et al. [86] presented another FPGA-based implementation of simplified P-R neuron model called the Kepecs–Wang neuron model, which was implemented on FPGA. Piecewise linear approximation was used to simplify nonlinear differential equations. Yang et al. [87] presented an FPGA-based implementation of the basal ganglia to model both normal and Parkinsonian states in a biologically plausible manner. The design uses piecewise linear approximation (PLA) for cost efficiency while maintaining the dynamic behavior of the biological basal ganglia. The model includes four basal ganglia nuclei, each with 64 neurons, and employs time-multiplexing to implement 256 neurons on a single core, effectively simulating both healthy and diseased states. Luo et al. [88] presented a real-time FPGA-based implementation of passage-of-time (POT) encoding in the cerebellum, which is crucial for sensorimotor skills and learning. This design aims to support neuroprosthetics for patients with cerebellar impairments. It models 100,000 biologically plausible neurons and is implemented as a frame-based network-on-chip (NoC) on an FPGA. Additionally, TrueNorth [89], a 65-mW fully digital neuromorphic processor, connects 1 million neurons across 4096 cores. A novel design methodology integrates mixed asynchronous–synchronous circuits with a complete tool flow for building an event-driven, low-power neurosynaptic chip, offering configurable connectivity and neural parameters.
Soleimani and Drakakis, in [90], came up with an FPGA-based implementation of the calcium cellular model. Here, 10 K to 40K cellular calcium units are pipelined and are compared with a CPU-based simulation, and the results show significant speedup. Davies et al. [91] presented an Intel-based fully digital application specific integrated circuit (ASIC) design, Loihi, with Intel's 14-nm process. It uses a modified current based (CUBA) leaky integrate and fire neuronal model. Hierarchical connectivity, dendritic compartments, and programming synaptic learning rules are its some prominent features. Intel also came up with a second-generation Loihi 2 chip [92] which supports a wide range of stateful spiking neuron models with fully programmable dynamics. In [93], Soleimani and Drakakis presented an ASIC design for calcium oscillations. Real-time dynamics are taken into consideration, and the design takes less area and power consumption than analog chips when real-time large-scale slow biological systems are taken into account. Faramarzi et al. [94] carried out yet another work on astrocytic calcium oscillations and their relations in neural processing and information encoding using the amplitude and frequency, and variation in both amplitude and frequency of Ca2+ ions. This circuit comprising astrocytes is implemented on FPGA and can be used in self-healing systems. Yang et al. [95] presented a large-scale implementation of a biologically plausible cortico-basal ganglia–thalamocortical loop using a scalable three-dimensional (3D)-network-on-chip (NoC) architecture on six Altera Stratix III FPGAs, incorporating one million neurons. This design introduces a router enabling multiple data flows to the multinucleus neural network. It features conductance-based, biophysically realistic neurons with a multiplierless layout, reducing memory and digital signal processor (DSP) resource requirements. Named the large-scale conductance-based spiking neural network (LaCSNN), it surpasses existing neuromorphic implementations in biophysical accuracy, reconfigurability, and runtime plasticity. The system enables the study of diseased basal ganglia and potential neuroprosthetic biosensing applications. In [96], Yang et al. came up with a dopamine neuron model implemented on FPGA using piecewise linear optimization and a multiplierless pipeline-based structure. Error analysis and resource utilization were used to make the neuronal model similar to biological neurons. Hao et al. [97] is another contribution from the same group of researchers, where the Purkinje cell found in the cerebellar structure of the nervous system, which has a profound impact on cerebellar processing, was implemented using the coordinate rotation digital computer (CORDIC) algorithm. This was implemented on the LaCSNN network as discussed in [95] by Yang et al. The Purkinje model thus designed, when evaluated on the basis of biological activities and dynamic mechanisms, tends to produce nearly the same results as biological models. Since this model uses only logical resources on FPGA, it supports large-scale implementations.
In [98], Hao et al. came up with the efficient implementation of a spiking neural network of hippocampus. The model is based on a designed task where a rat finds a pot and gets a reward. Software realization in MATLAB and FPGA-based implementation was carried out. The LIF neuron model was used. In [99], Yang et al. discussed the implementation of a retinal spiking neural network that deploys Hodgkin and Huxley's neuronal model. The study in [100] by George et al. is an excellent survey examining biohybrid systems. With the immense advancements that are being carried out in neuromorphic computing, the possibility of brain–machine interfaces (BMIs) and signal processing through such systems are being explored for neurophysiologists as well as for engineers working in the domain. Yang et al. [101] proposed a scalable implementation of a biologically realistic neural network using a multicompartment neuronal model. This large-scale design incorporates one million neurons, offering detailed physiological and morphological insights. By eliminating memory and multiplication overhead at the cellular level, the computational speed improved by 56.59% compared with contemporary designs. A novel NoC routing algorithm enhances scalability, performance, and throughput. In addition to speed, the design excels in area efficiency and biological plausibility, making it superior to similar implementations.
A biologically inspired SNN with three layers was realized on FPGA in [102] by Kuang et al. for solving pattern recognition and cognitive tasks. The LIF neuron model with conductance-based synapses was utilized in this work. In [103] by Seyedbarhagh et al., we see a digital hardware implementation of an astrocyte model that incorporates Glu Receptor 5 (mGlu5R)-induced Ca2+ oscillations, which has not been modeled in any prior work. Piecewise linear approximation was used. mGlu5R plays an essential role in the working memory and hence its inclusion in the models is of paramount importance. The MATLAB simulation results and the simulation results from hardware description language implementations are very coherent.
Despite the fact that many neuromorphic implementations are on the rise and an increasing interest in making the system biologically realistic is also underway, very few implementations take fault tolerance and online learning into account in their design. Previous work by Yang et al., where they developed LaCSNNs [95], contributes towards a neuromorphic system named fault-tolerant context-dependent learning (FCL) which provides the facility of online learning and fault tolerance. This model not only gives a better understanding of the brain but can also be useful for neurobiotics and BMIs in a very efficient way [104]. Deng et al. [105] demonstrated an FPGA-based auditory system with anti-noise capability. An SNN was realized with a modified LIF neuronal model. In [106], Yang et al. came up with visual SNN where LIF neurons and event-driven third-order STDP were used. In [107], Yang et al. presented a biologically inspired cognitive supercomputing system (BiCoSS), which is a large-scale SNN neuromorphic platform implemented on FPGA chips with four million bioplausible neurons. Different levels of granules and bioplausibility can be achieved, and many cognitive activities can be used. The design is scalable too. Yang et al. presented a large-scale bioplausible realization of the cerebellum in [108]. In [109], Acharya et al. proposed an FPGA-based implementation for basic biochemical reactions to be used as a subset library for designing any cellular activity. The system generation toolbox of Xilinx was used to implement a Simulink model on FPGA. This work is based on the prior work in [110] by Soma et al.
5.
Discussion and analysis
This survey explores the active research carried out in the neuromorphic and cytomorphic domains with an emphasis on biorealistic implementations. In Tables 1, 2, and 3, the trends of neuromorphic and cytomorphic research in the past 16 years are reported, focusing on the analog, mixed-signal, and digital platforms, respectively. Moreover, the potential applications and future research areas with respect to the analysis are described in this section. Some analysis on the trend of the research in the past 16 years based on these tables is carried out. Percentagewise summary statistics are also given in Tables 4 and 5.
5.1. Classification based on implementation platforms
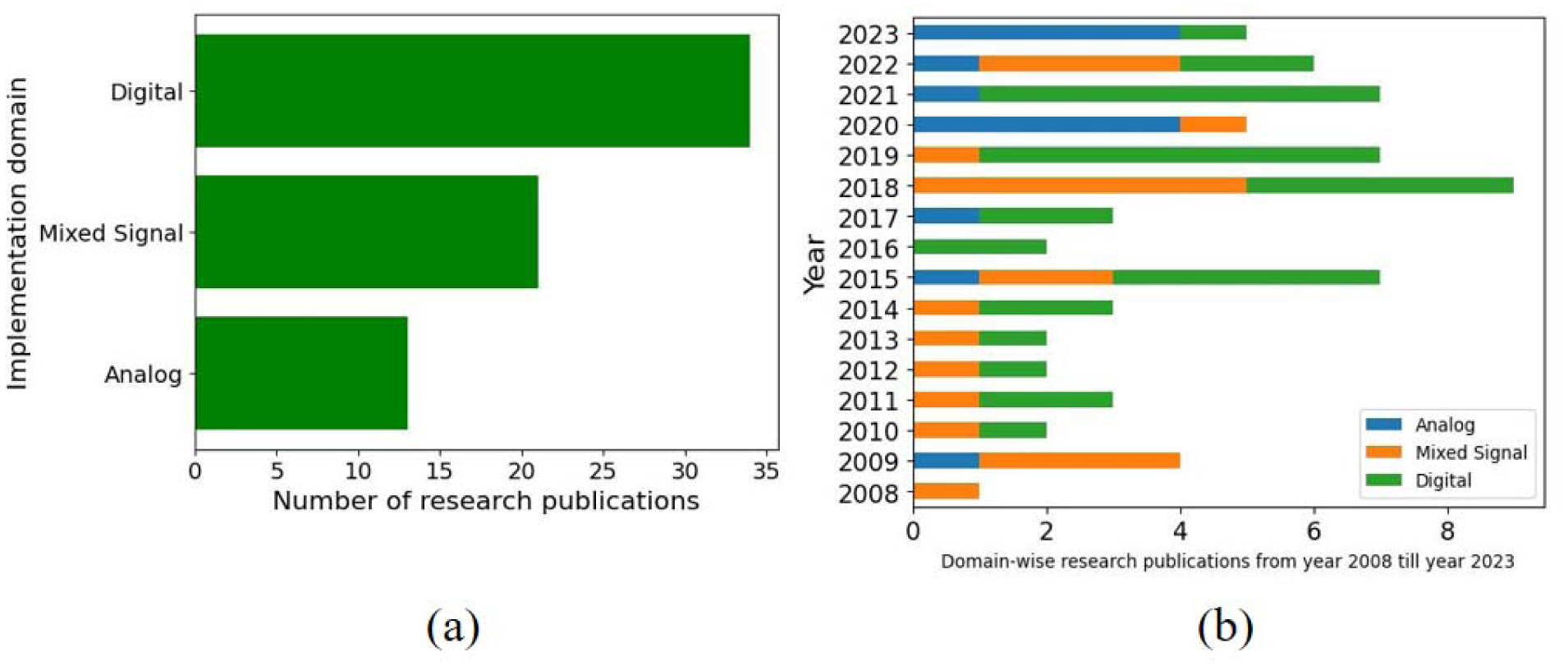
The number of research publications based on various hardware platforms over the past 16 years (2008–2023) is illustrated in Figure 1(a). We can infer that most of the work has been carried out in the digital domain, accounting for 50% of the total research, while the mixed-signal and analog domains account for 31% and 19%, respectively. Despite the dominance of digital implementations, their lack of biological plausibility limits their ability to accurately replicate bioprocesses, even though they offer scalability, precision, and reconfigurability, especially in FPGA-based designs. Conversely, analog circuits allow for compact and biorealistic designs that closely mimic biological functions but suffer from scalability issues, noise susceptibility, and design complexity. Mixed-signal approaches attempt to bridge this gap by leveraging the strengths of both domains; however, their integration introduces synchronization challenges and increases the design complexity. Figure 1(b) shows the year-wise trend of research using various hardware platforms. While digital and mixed-signal implementations dominate due to their flexibility and ease of prototyping, analog realizations have not been entirely disregarded, as they continue to offer advantages in biomimicry. However, the limitations of all current approaches—whether in terms of biological realism, scalability, or design complexity—suggest that further research is needed to optimize hardware platforms for bioprocess modeling.
5.2. Classification based on bioplausibility
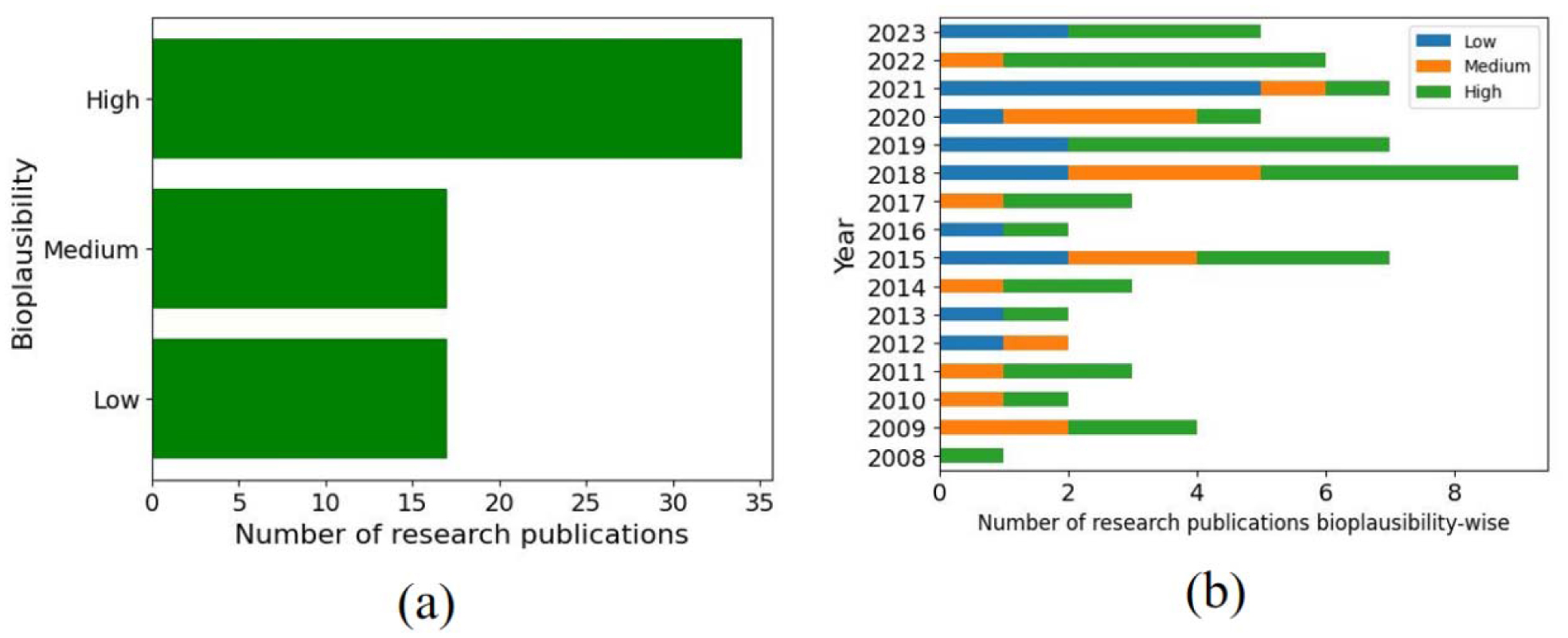
Figure 2(a) examines the number of research publications based on the level of bioplausibility of designs over the past 16 years. This survey reveals a growing trend of publications where bioplausibility is a major concern among researchers, with 24% classified as high, 26% as medium, and 50% as low. Many researchers are striving to make their designs biologically realistic, which not only benefits basic medical science research but also enhances the accuracy of the results. Figure 2(b) further illustrates a year-wise breakdown of research based on bioplausibility, highlighting the increasing inclination of researchers toward biorealistic designs. Additionally, many research groups that initially adopted higher levels of abstraction in their designs are now shifting their focus toward achieving greater biorealism in future implementations.
5.3. Classification based on type of biological circuits
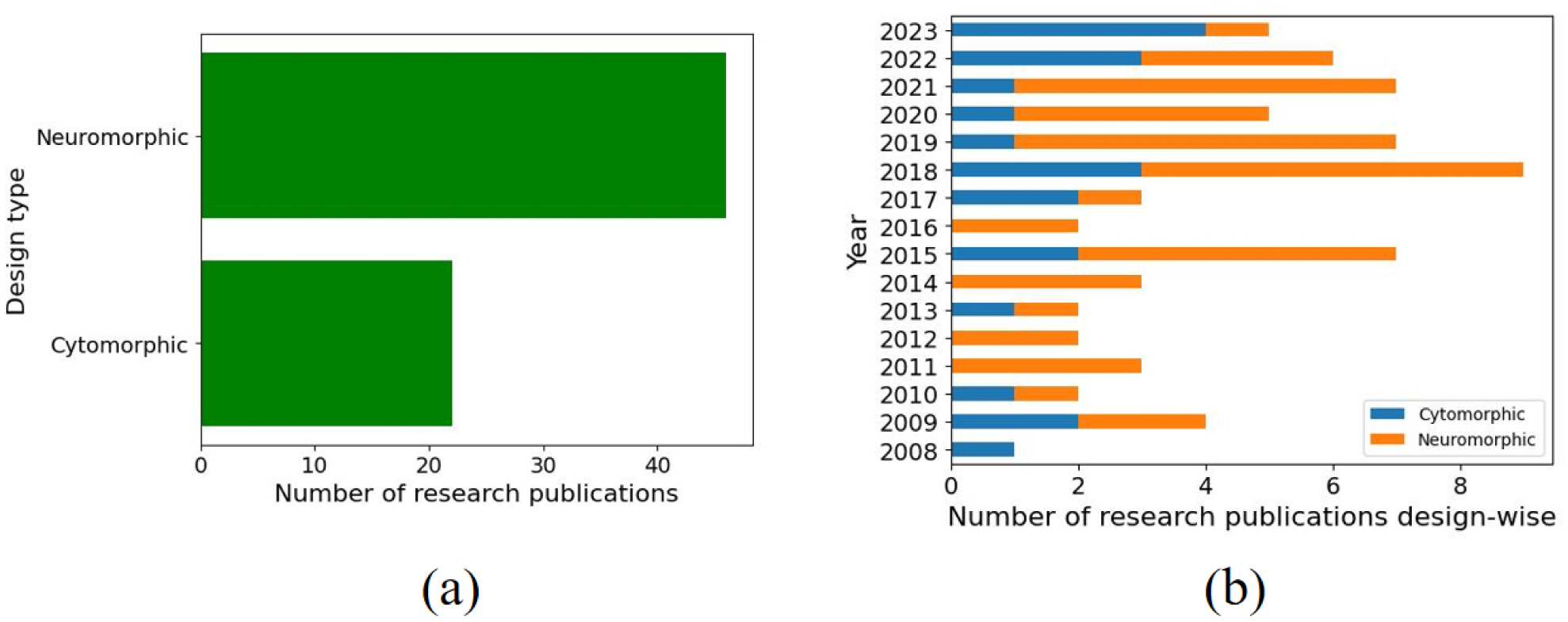
Over the past 16 years, research into biological pathways has followed two primary directions: neuromorphic, accounting for 68%, and cytomorphic, accounting for 32%. Figure 3a,b suggests a growing number of active researchers contributing to both fields by developing various types of biological circuits. Neuromorphic designs, inspired by the brain's structure and function, excel in parallel processing and energy efficiency, making them highly suitable for AI and computational neuroscience applications. However, their reliance on simplified neuron models often limits biological realism. In contrast, cytomorphic research, which models intracellular processes and signaling pathways, provides a more biorealistic approach. While less prevalent, it has significant advantages in medicine, pharmacology, and synthetic biology, where accurate biological replication is crucial. Despite its potential, cytomorphic implementations face challenges in scalability and computational efficiency, hindering their broader adoption. Figure 3(b) illustrates a noticeable increase in cytomorphic research since 2017, suggesting a growing interest in bridging the gap between abstract computational models and biologically faithful designs. The trade-off between efficiency and biological realism remains a key challenge, emphasizing the need for hybrid approaches that integrate the strengths of both domains.
Table 4 presents the percentage-wise statistics of biological circuit types across implementation platforms. The table indicates that neuromorphic implementations are predominantly based on digital platforms, whereas cytomorphic implementations primarily utilize analog platforms. Table 5, on the other hand, presents percentage-wise summary statistics of bioplausibility across different implementation platforms. It shows that bioplausibility is favored the most in the analog domain and the least in the digital domain. Based on the data presented in Tables 4 and 5, several conclusions can be drawn. First, neuromorphic circuits predominantly rely on digital platforms, likely due to their scalability, ease of implementation, and compatibility with existing computing architectures. In contrast, cytomorphic circuits favor analog platforms, as analog implementations more closely mimic continuous biological processes, making them more suitable for biochemical and cellular-level modeling. Additionally, the correlation between bioplausibility and the implementation platform highlights a key trade-off: While digital platforms offer flexibility and computational efficiency, they tend to sacrifice biorealism. Conversely, analog implementations, despite their challenges in design complexity and scalability, are better suited for achieving high bioplausibility. The preference for analog platforms in cytomorphic research further supports this notion, as cytomorphic circuits often require a high degree of biological realism. These findings suggest that future research may benefit from exploring mixed-signal approaches that balance bioplausibility with computational efficiency, leveraging the strengths of both digital and analog domains.
5.4. Potential applications of cytomorphic and neuromorphic research
By the current research in the domain of cytomorphic and neuromorphic chips, it is imperative that bioplausibility is a major concern. Regardless of the hardware platform incorporated in the design, all research work seems to actively include many biochemical reactions that are at the core of all functions of living cells and neurons. The major challenge in this regard is the analog nature of such reactions and the large-scale biochemical and neural networks that must be furnished in the design. Thus, future work requires means and techniques to be explored and formulated that allow for future cytomorphic and neuromorphic chips to be not only biologically realistic but also as small and power-efficient as possible, along with the accuracy trade-off. Analog designs, in the sub-threshold and above-threshold regions, are quite promising in this connection, as designs with fewer number of transistors can help in achieving the said metrics; however, researchers are also exploring new techniques and algorithms to incorporate accurate and biologically realistic designs using reconfigurable digital setups. The possibility of future neuromorphic and cytomorphic design endeavors with compactness, accuracy, and bioplausibility will benefit many fields. Some of the emerging fields in this connection are listed here.
5.4.1. Neuroprosthetics and brain–computer interfaces (BCIs)
Current research suggests that incorporating neuromorphic computing in neuroprosthetic devices offer an advantage over the currently used software-based sensory-somatic interfaces as neuromorphic devices provide better compatibility with biological interfacing. Designing neuromorphic hardware with biorealistic implementations of neurons and synapses and taking into account that the devices thus designed should be energy efficient as well as portable and embeddable is of profound importance in future research to be carried out in neuroprosthetics. BCIs (brain–computer interfaces), also called BMIs, are a major area where active researchers are still working to include neuromorphic architectures for information processing instead of software-based machine learning and AI-based approaches.
5.4.2. Bioelectronic medicine and molecular medicine
Bioelectronic medicine involves electrical stimulation of the autonomic nervous system. A growing body of research is again directed towards the profound impact that the neuromorphic approach can bring in this regard compared with traditional software-based approaches. Molecular medicine, which deals with medical approaches applied to cellular structures and gene expression rather than the organs of the human body, is also reliant on advancements in the fields of cytomorphic and neuromorphic engineering.
5.4.3. Synthetic biology
Synthetic biology can be defined as engineering biological substrates. It is about customizing biocellular entities, their behavior, and functions by designing and creating new cellular entities like enzymes and genetic circuits. The ongoing research in the field of synthetic biology may benefit from these electronic models [111]–[113].
5.4.4. Neuroscience and medical science research
The bottom-up approach of neuromorphic and cytomorphic computing already plays a pivotal role in research-by-synthesis aspects; however, while the active research groups are working to bridge the gap between the bottom-up and top-down approaches, the basic medical science and neuroscience knowledge can be studied at various levels of abstraction as well as from the application perspective of the designed neural or cellular pathways.
5.4.5. DNA-based memory and computing devices
An emerging field of biotechnology that may benefit from the research in neuromorphic as well as cytomorphic chips with biorealistic parameters is the ongoing research into DNA-based storage media instead of contemporary CMOS-based chips. DNA-based computing devices are envisioned to be incorporated in future computing machines.
5.4.6. Biosensors
The cytomorphic and neuromorphic chips at the bottom-up level allow semiconductor material to be used in biosensor systems. Noninvasive electrical biosensors are a preferred choice over optical biosensors, and thus research in the area is of paramount importance.
5.4.7. Robotics and biomorphic solutions
Some more recent research groups are working on bioinspired neuronal models and neural structures based on molecular and genetic circuits [30]. For instance, Biomorphic Intelligence Lab is working on bioinspired solutions for aerial robotics.
5.4.8. Pharmacology, drug discovery, and drug testing
Pharmacological research, drug testing, and drug formulation are among the major beneficiaries of research in the neuromorphic and cytomorphic realms. Traditionally, software simulation-based approaches have been used but these solutions are computationally expensive and a lot of abstraction is required. Electronic platforms are very well suited for pharmaceutical applications. The work in [31],[32] and many others are already coming up with revolutionary drug testing and disease control strategies.
5.4.9. Biomorphic AI
The current trend in neuromorphic and cytomorphic research also supports a growing trend of research into biomorphic engineering and biomorphic AI. In this regard, Biomorphic Research Lab has been established and is working on biologically inspired solutions for aerial robots. They are considering the aspects of how biological systems sense, think, and act and incorporate them in the design of drones.
6.
Conclusion
In this survey paper, we have reviewed the techniques used in bioplausible neuromorphic and cytomorphic circuits on different electronic platforms and with different levels of abstraction since the conception of the fields. On the basis of the review, we have analyzed the emerging trends in the ongoing research in the fields of interest. We have reviewed over 100 papers and have listed the most relevant ones in the past 16 years from 2008 to 2023. We have observed that though research in the cytomorphic domain is relatively new as compared with the neuromorphic domain, there has been an increase in the interest shown by recent active researchers towards cytomorphic chips.
In the early years, researchers focused on analog platforms in the sub-threshold domain but for various reasons like speed and accuracy, many researchers took above-threshold analog platforms to design neuromorphic and cytomorphic hardware. However, analog designs show promising results with respect to resource utilization and energy efficiency, and thus many researchers are inclined towards sub-threshold and above-threshold regimes of analog design. To gain the advantages of speed, accuracy, and scalability, mixed-signal design is also preferred by many stalwarts of the field. On the other hand, digital design allows for greater flexibility, speedup, low cost, rapid development, and scalability, particularly, by incorporating reconfigurable platforms. Hence, a large body of designers has opted for FPGA platforms but at the expense of biorealistic realizations. Since bioplausibility is the main focus of researchers in the neuromorphic domain, cytomorphic research certainly adds strength to neuromorphic research in this regard. It can be seen that all major research groups are trying to mitigate the gap between the higher abstraction mechanisms and lower abstraction aspirations so that many emerging fields may benefit in a profound way. In the days to come, such knowledge may lead the researchers to come up with more advanced hybrid designs that include digital and analog interfaces that can be more readily utilized with real-world biological systems and other electronic environments. Moreover, we expect to see a surge in the already existing computing paradigms with bioplausible mechanisms incorporated in them.
Use of AI tools declaration
The authors declare they have not used AI tools in the creation of this article.









 DownLoad:
DownLoad: