Remarks on the decay/growth rate of solutions to elliptic free boundary problems of obstacle type

1.   Introduction

In this paper, we consider the non-isentropic compressible Euler equations with a source term in the following Euler coordinate system:

where$\; \rho, u, S$ and$\; p(\rho, S)$ are the density, velocity, entropy and pressure of the considered gas, respectively. $x\in[0, L]$ is the spatial variable, and $L > 0$ is a constant denoting the duct's length. $p(\rho, S) = a e^{S} \rho ^{\gamma }$, with constants $a > 0$ and $\gamma > 1$. And, the term $\beta \rho |u|^{\alpha} u$ represents the source term with $\alpha, \; \beta \in R$. Especially, the source term denotes friction when $\beta < 0$.

System (1.1) is equipped with initial data:

and boundary conditions:

If $S = Const.$, the system (1.1) is the isentropic Euler equations with a source term. In the past few decades, the problems related to the isentropic compressible Euler equations with different kinds of source terms have been studied intensively. We refer the reader to ^{[1,2,3,4,5,6,7,8,9,10]} to find the existence and decay rates of small smooth (or large weak) solutions to Euler equations with damping. The global stability of steady supersonic solutions of 1-D compressible Euler equations with friction $\beta\rho|u|u$ was studied in ^[11]. For the singularity formation of smooth solutions, we can see ^{[12,13,14,15]} and the references therein. Moreover, the authors in ^[16] established the finite-time blow-up results for compressible Euler system with space-dependent damping in 1-D. Recently, time-periodic solutions have attracted much attention. However, most of these temporal periodic solutions are driven by the time-periodic external force; see ^[17,18] for examples. The first result on the existence and stability of time-periodic supersonic solutions triggered by boundary conditions was considered in ^[19]. Then, the authors of ^[20] studied the global existence and stability of the time-periodic solution of the isentropic compressible Euler equations with source term $\beta \rho |u|^{\alpha}u$.

If $S\neq Const.$, much less is known. In ^{[21,22,23,24,25,26]}, the authors used characteristics analysis and energy estimate methods to study 1-D non-isentropic p-systems with damping in Lagrangian coordinates. Specifically, the global existence of smooth solutions for the Cauchy problem with small initial data has been investigated in ^[21,22]. The influence of the damping mechanism on the large time behavior of solutions was considered in ^[23,24]. For the results of the initial-boundary value problem, see ^[25,26]. The stability of combination of rarefaction waves with viscous contact wave for compressible Navier-Stokes equations with temperature-dependent transport coefficients and large data was obtained in ^[27]. As for the problems about non-isentropic compressible Euler equations with a vacuum boundary, we refer the reader to ^[28,29]. In ^[30,31,32], the relaxation limit problems for non-isentropic compressible Euler equations with source terms in multiple space dimensions were discussed.

In this paper, we are interested in the dynamics of non-isentropic Euler equations with friction. Exactly speaking, we want to prove the global existence and stability of temporal periodic solutions around the supersonic steady state to non-isentropic compressible Euler equations with the general friction term $\beta \rho |u|^{\alpha} u$ for any $\alpha, \; \beta\in R$. It is worth pointing out that the temporal periodic non-isentropic supersonic solution considered in this paper is driven by periodic boundary conditions.

We choose the steady solution $\tilde{W}(x) = (\tilde{\rho}(x), \tilde{u}(x), \tilde{S}(x))^{\top}$ (with $\tilde{u}(x) > 0$) as a background solution, which satisfies

The equation $(1.4)_{3}$ indicates that the static entropy in the duct must be a constant. That is, $\tilde{S}(x) = S_{0}$. Moreover, when $(\alpha, \beta)$ lies in different regions of $R^{2}$, the source term $\beta \tilde{\rho} \tilde{u}^{\alpha+1}$ affects the movement of flow dramatically. We analyze the influence meticulously and gain the allowable maximal duct length for subsonic or supersonic inflow.

Based on the steady solution, we are interested in two problems. The first one is, if $\rho_{l}(t)-\rho_{-}$, $u_{l}(t)-u_{-}$, $S_{l}(t)-S_{0}$ and $\rho_{0}(x)- \tilde{\rho}(x)$, $u_{0}(x)-\tilde{u}(x)$, $S_{0}(x)-S_{0}$ are small in some norm sense, can we obtain a classical solution of the problem described by (1.1)–(1.3) for $[0, \infty) \times [0, L]$ while this classical solution remains close to the background solution? If the first question holds, our second one is whether the small classical solution is temporal-periodic as long as the inflow is time-periodic at the entrance of ducts?

We use $\bar{W}(t, x) = (\bar{\rho}(t, x), \bar{u}(t, x), \bar{S}(t, x))^\top = (\rho(t, x)-\tilde{\rho}(x), u(t, x)-\tilde{u}(x), S(t, x)-S_{0})^\top$ to denote the perturbation around the background solution, and, correspondingly,

that is,

and

The main conclusions of this article are as follows:

Theorem 1.1. For any fixed non-sonic upstream state $(\rho_{-}, u_{-}, S_{0})$ with $\rho_{-} \neq \rho^{*} = \left[\frac{(\rho_{-}u_{-})^2}{a \gamma e^{S_{0}} }\right]^ {\frac{1}{\gamma +1}} > 0$ and $u_{-} > 0$, the following holds:

1) There exists a maximal duct length $L_{m}$, which only depends on $\alpha, \beta, \gamma$ and $(\rho_{-}, u_{-}, S_{0})$, such that the steady solution $\tilde{W}(x) = (\tilde{\rho}(x), \tilde{u}(x), S_{0})^{\top}$ of the problem (1.1) exists in $[0, L]$ for any $L < L_{m}$;

2) The steady solution $(\tilde{\rho}(x), \tilde{u}(x), S_{0})^{\top}$ keeps the upstream supersonic/subsonic state and $\tilde{\rho} \tilde{u} = \rho_{-}u_{-} > 0$;

3) $\|(\tilde{\rho}(x), \tilde{u}(x), S_{0})\|_{C^{2}([0, L])} < M_{0}$, where $M_{0}$ is a constant only depending on $\alpha$, $\beta$, $\gamma$, $\rho_{-}$, $u_{-}$, $S_{0}$ and $L$;

4) If $\beta > 0$, $\alpha \leq 1$ and the upstream is supersonic, the maximal duct length $L_{m}$ can be infinite and a vacuum cannot appear in any finite place of ducts;

5) When $\beta > 0$, $\alpha\geq-\gamma$ and the upstream is subsonic, the maximal duct length $L_{m}$ can also be infinite, and the flow cannot stop in any place of ducts.

Theorem 1.2. Assume that the length of duct $L < L_{m}$ and the steady flow is supersonic at the entrance of a duct, i.e., $\rho_{-} < \rho^{*} = \left[\frac{(\rho_{-} u_{-})^2}{a \gamma e^{S_{0}} }\right]^ {\frac{1}{\gamma +1}}$. Then, there are constants $\varepsilon_{0}$ and $K_{0}$ such that, if

and the $C^{0}, \; C^{1}$ compatibility conditions are satisfied at point $(0, 0)$, there is a unique $C^{1}$ solution $W(t, x) = (\rho(t, x), u(t, x), S(t, x))^{\top}$ for the mixed initial-boundary value problems (1.1)–(1.3) in the domain $G = \{(t, x)|t\geq0, \; x\in[0, L]\}$, satisfying

Remark 1.1. Since the flows at $\{x = L\}$ are entirely determined by the initial data on $x\in[0, L]$ and the boundary conditions on $\{x = 0\}$ under the supersonic conditions, we only need to present the boundary conditions on $\{x = 0\}$ in Theorem 1.2.

If we further assume that the boundaries $\rho_{l}(t), u_{l}(t), S_{l}(t)$ are periodic, then the $C^{1}$ solution obtained in Theorem 1.2 is a temporal periodic solution:

Theorem 1.3. Suppose that the assumptions of Theorem 1.2 are fulfilled and the flow at the entrance $x = 0$ is temporal-periodic, i.e., $W_{l}(t+P) = W_{l}(t)$; then, the $C^{1}$ solution $W(t, x) = (\rho(t, x), u(t, x), S(t, x))^{\top}$ of the problem described by $(1.1)$–$(1.3)$ is also temporal-periodic, namely,

for any $t > T_{1}$ and $x \in [0, L]$, where $T_{1}$ is a constant defined in (4.3).

The organization of this article is as follows. In the next section, we study the steady-state supersonic and subsonic flow. The wave decomposition for non-isentropic Euler equations is introduced in Section 3. In Section 4, based on wave decomposition, we prove the global existence and stability of smooth solutions under small perturbations around the steady-state supersonic flow. And, in Section 5, with the help of Gronwall's inequality, we obtain that the smooth supersonic solution is a temporal periodic solution, after a certain start-up time, with the same period as the boundary conditions.

2.   Steady-state supersonic and subsonic flow

In this section, the steady-state flow is considered for some positive constants upstream $(\rho_{-}, u_{-}, S_{0})$ on the left side. In ^[11], the authors considered the differential equation in which the Mach number varies with the length of the duct. In ^[20], the authors investigated the steady-state equation with sound speed and flow velocity. Different from the methods used in ^[11] and ^[20], and motivated by ^[33], we rewrite (1.4) as the equations related to momentum and density in this paper, namely,

where $\tilde{m} = \tilde{\rho} \tilde{u}$ represents momentum. The advantage of this method is that the vacuum and stagnant states can be considered. Now, we analyze this problem in three cases:

Case 1: $\alpha \neq 1$ and $\alpha \neq -\gamma$.

In this case, (2.1) becomes

where

Then, we get

Let $G(\tilde{\rho}, \tilde{m}) = \tilde{\rho}^2 p_{\tilde{\rho}} -\tilde{m}^{2}$. For any fixed $\tilde{m} > 0$, we have that $\underset{ \tilde{\rho} \rightarrow 0}{\lim} G(\tilde{\rho}, \tilde{m}) = -\tilde{m}^{2} < 0$. From the definition of $p(\tilde{\rho}, S_{0})$, we obtain

Thus, when $\tilde{\rho}\rightarrow +\infty$, $G(\tilde{\rho}, \tilde{m})\rightarrow +\infty$. Then, there exists $\rho^{*} = \left[\frac{(\rho_{-} u_{-})^2}{a \gamma e^{S_{0}} }\right]^ {\frac{1}{\gamma +1}} > 0$ such that $G\left(\rho^{*}, \tilde{m}\right) = 0$ (i.e., $\left(\rho^*\right)^2 p_{\tilde{\rho}} \left(\rho^*\right) = \tilde{m}^{2}$). That is, when $\tilde{\rho} = \rho^{*}$, the fluid velocity is equal to the sound speed (i.e., $\tilde{u} = \tilde{c} = \sqrt{\frac {\partial p}{\partial \tilde{\rho}}} = \sqrt{a\gamma} e^{\frac{S_{0}}{2}} \tilde{\rho}^{\frac{\gamma -1}{2}}$). Therefore, we have

and

We conclude that $\frac{\partial F_1(\tilde{\rho}, \tilde{m})}{\partial \tilde{\rho}} < 0$ for $\tilde{\rho} < \rho^{*}$ and $\frac{\partial F_1(\tilde{\rho}, \tilde{m})}{\partial \tilde{\rho}} > 0$ for $\tilde{\rho} > \rho^{*}$. Furthermore, we have

and

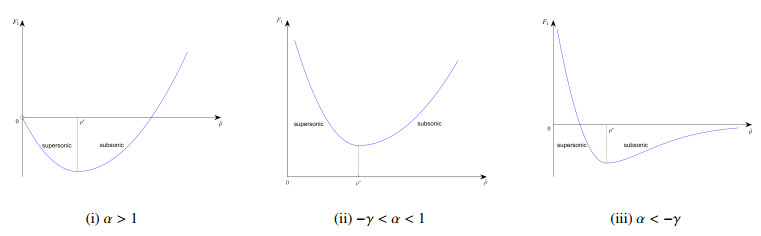
Then, for any fixed $\tilde{m} = \rho_{-}u_{-} > 0$, according to different regions of $\alpha \in R$, we draw the graphs of $F_1(\tilde{\rho}, \tilde{m})$. See Figure 1 below.

Integrating $(2.2)_{2}$ over $(0, x)$, we obtain

If $\beta < 0$, by $(2.10)$, $F_{1}(\tilde{\rho}, \tilde{m})$ will decrease as the length of ducts increases, until it arrives at the minimum $F_{1}(\rho^{*}, \tilde{m})$, no matter whether the upstream is supersonic (i.e., $\rho_{-} < \rho^{*}$) or subsonic (i.e., $\rho_{-} > \rho^{*}$). Therefore, we get the maximal length of ducts

for a supersonic or subsonic flow before it gets choked, which is the state where the flow speed is equal to the sonic speed.

However, if $\beta > 0$, $\alpha > 1$ and the upstream is supersonic (i.e., $\rho_{-} < \rho^{*}$), by (2.7), (2.10) and Figure 1 (i), we know that $\tilde{\rho}$ is decreasing as duct length $x$ increases. Then, we get the maximal length of ducts

for a supersonic flow before it reaches the vacuum state. If $-\gamma < \alpha < 1$ or $\alpha < -\gamma$, by (2.8)–(2.10) and Figure 1(ii) and (iii), $\tilde{\rho}$ is decreasing as the duct length $x$ increases for supersonic upstream, too. But, the vacuum will never occur for any duct length $L$.

Moreover, if $\beta > 0$, $\alpha < -\gamma$ and the upstream is subsonic (i.e., $\rho_{-} > \rho^{*}$), combining (2.9), (2.10) with Figure 1(iii), $\tilde{\rho}$ is increasing as the duct length $x$ increases. At the same time, $F_{1}(\tilde{\rho}, \tilde{m})$ is increasing and approaching its supremum 0. Then, we get the maximal length of the duct $L_{m}$, which is still as shown in (2.12). When $L > L_{m}$, the fluid velocity is zero, that is, the fluid stagnates in a finite place. While, if $-\gamma < \alpha < 1$ or $\alpha > 1$, again, by (2.7), (2.8), (2.10) and Figure 1(i) and (ii), $\tilde{\rho}$ is also increasing as the duct length $x$ increases, but $F_{1}(\tilde{\rho}, \tilde{m})$ goes to infinity as $\tilde{\rho}$ grows. In this case, although the fluid is slowing down, it does not stagnate at any finite place.

Case 2: $\alpha = 1$.

Now, (2.2) turns into

where

And, we get

and

Similarly, the function $F_{2}\left(\tilde{\rho}(x), \tilde{m}\right)$ gets its minimum at point $\tilde{\rho} = \rho^{*}$. If $\beta < 0$, combining (2.14) with (2.16), we get the maximal length of ducts

for a supersonic or subsonic flow before it gets choked. While, if $\beta > 0$, the flow remains in its entrance state for any duct length $L > 0$, no matter whether it is supersonic or subsonic.

Case 3: $\alpha = -\gamma$.

In this case, $(2.1)$ changes into

where

Then, we have

and

Similar to the other two cases, the function $F_{3}\left(\tilde{\rho}(x), \tilde{m}\right)$ gets its minimum at point $\tilde{\rho} = \rho^{*}$. If $\beta < 0$, by (2.19) and (2.21), we obtain the maximal length of ducts

for a supersonic or subsonic flow before it gets choked. While, if $\beta > 0$, again, by (2.19) and (2.21), the flow also keeps the upstream supersonic or subsonic state for any duct length $L > 0$.

To sum up, we draw the following conclusion from the above analysis:

Lemma 2.1. If $\rho_{-} \neq \rho^{*} > 0, \; u_{-} > 0, \; c^{*} = (a \gamma e^{S_{0}})^{\frac{1}{\gamma+1}}(\rho_{-} u_{-})^{\frac{\gamma-1}{\gamma+1}} > 0$ and the duct length $L < L_{m}$, where $L_{m}$ is the maximal allowable duct length given in (2.11), (2.12), (2.17) and (2.22), then the Cauchy problem (1.4) admits a unique smooth positive solution $(\tilde{\rho}(x), \tilde{u}(x), S_{0}) ^{\top}$ which satisfies the following properties:

1) $0 < \rho_{-} < \tilde{\rho}(x) < \rho^{*}$ and $c^{*} < \tilde{u}(x) < u_{-}$, \quad if $\beta < 0$ and $\rho_{-} < \rho^{*}$;

2) $0 < \rho^{*} < \tilde{\rho}(x) < \rho_{-}$ and $u_{-} < \tilde{u}(x) < c^{*}$, \quad if $\beta < 0$ and $\rho_{-} > \rho^{*}$;

3) $0 < \tilde{\rho}(x) < \rho_{-}$ and $c^{*} < u_{-} < \tilde{u}(x) < +\infty$, \quad if $\beta > 0$ and $\rho_{-} < \rho^{*}$;

4) $0 < \rho_{-} < \tilde{\rho}(x) < +\infty$ and $0 < \tilde{u}(x) < u_{-} < c^{*}$, \quad if $\beta > 0$ and $\rho_{-} > \rho^{*}$;

5) $\tilde{\rho} \tilde{u} = \rho_{-}u_{-}$;

6) $\|(\tilde{\rho}(x), \tilde{u}(x), S_{0})\|_{C^{2}([0, L])} < M_{0},$ where $M_{0}$ is a constant only depending on $\alpha$, $\beta$, $\gamma$, $\rho_{-}$, $u_{-}$, $S_{0}$ and $L$.

Remark 2.1. The following is worth pointing out:

1) When $\beta > 0$ and the upstream is supersonic, a vacuum can occur at the finite place for $\alpha > 1$, while a vacuum will never happen in any finite ducts for $\alpha\leq 1$;

2) When $\beta > 0$ and the upstream is subsonic, fluid velocity can be zero at the finite place for $\alpha < -\gamma$, while the movement of fluid will never stop in the duct for $\alpha \geq -\gamma$;

3) For the case of $\beta = 0$, we refer the reader to ^[19] for details.

Thus, from Lemma 2.1 and Remark 2.1, we can directly get Theorem 1.1.

3.   Wave decomposition

In order to answer the two problems proposed in the introduction, we introduce a wave decomposition for system (1.1) in this section. Here, we choose the steady supersonic solution $\tilde{W}(x) = (\tilde{\rho}(x), \tilde{u}(x), \tilde{S}(x))^{\top}$ (with $\tilde{u}(x) > 0$) as the background solution, which satisfies (1.4). For system (1.1), the corresponding simplification system has the form

Let us denote $W(t, x) = \bar{W}(t, x)+\tilde{W}(x)$, where $\bar{W} = (\bar{\rho}, \bar{u}, \bar{S})^\top$ is the perturbation around the background solution. Substituting

into (3.1) yields

Combining this with (1.4), system (3.3) can be simplified as

where $\Theta(\rho, \tilde{\rho}, S, S_{0}) e^{\bar{S}} \bar{\rho} = a \gamma (e^{S} \rho ^{\gamma-2}-e^{S_{0}} \tilde{\rho}^{\gamma-2})$ and $g(u, \tilde{u}) \bar{u} = -\beta [(\bar{u}+\tilde{u})^{\alpha+1}-\tilde{u}^{\alpha+1}]$. $g(u, \tilde{u})$ can be represented as follows:

Obviously, system (3.4) can be expressed as the following quasi-linear equations:

where

Through simple calculations, the three eigenvalues of system (3.5) are

where $c = \sqrt{a\gamma} e^{\frac{S}{2}} \rho^{\frac{\gamma -1}{2}}$. The three right eigenvectors $r_{i}(W)\; (i = 1, 2, 3)$ corresponding to $\lambda_{i}\; (i = 1, 2, 3)$ are

The left eigenvectors $l_{i}(W)\; (i = 1, 2, 3)$ satisfy

where $\delta_{i j}$ represents the Kroneckeros symbol. It is easy to get the expression for $l_{i}(W)$ as follows:

Besides, $l_{i}(W)$ and $r_{i}(W)$ have the same regularity.

Let

then,

Noticing (3.5) and (3.12), we have

where

By using (3.5) and (3.13), we get

Thus, noting $\nabla(l_{i}(W) r_{j}(W)) = 0$ and $\nabla l_{i}(W) r_{j}(W) = -l_{i}(W) \nabla r_{j}(W)$, we get

where

and

Similarly, we have from (3.10) and (3.13) that

and

where $C_{ksi} (W) = l_{i} (W) \frac{\partial \left(r_{k} (W) \right)}{\partial W_{s}}$. It is concluded from (3.16) that

Therefore,

Then,

where we used (3.5). By differentiating

with respect to $W_{s}$ and multiplying the result by $l_{i}(W)$, we get

Thus,

where

In view of Lemma 2.1, it is clear that the term $H(\tilde{W})_{x}$ in (3.26) is meaningful.

For the convenience of the later proof, we can rewrite system (3.5) as

by swapping the variables $t$ and $x$. Here, we represent the eigenvalues, left eigenvectors and right eigenvectors of the matrix $A^{-1}(W)$ as $\hat{\lambda}_{i}$, $\hat{l}_{i}(W)$ and $\hat{r}_{i}(W), i = 1, 2, 3$, respectively.

Let

Similar to the above arguments, we can get similar results by combining (3.27) and (3.28):

with

and

where

The wave decomposition for the initial data

and boundary conditions

have the following form:

with

where

4.   Existence and stability of global solutions

In this section, based on wave decomposition, we prove the global existence and stability of smooth solutions under small perturbations around the steady-state supersonic flow in region $G = \{(t, x)|t\geq0, \; x\in[0, L]\}$. The initial data and boundary conditions satisfy the compatibility conditions at point (0, 0) (see ^[11]).

In order to verify Theorem 1.2, we first establish a uniform prior estimate of the supersonic classical solution. That is, we assume that

when

where $\varepsilon$ is a suitably small positive constant. Here and hereafter, $K$, $K_{i}$ and $K_{i}^{*}$ are constants that depend only on $L$, $\varepsilon$, $\left\| (\tilde{\rho}, \tilde{u}, S_{0})) \right\|_{C^{2}([0, L])}$ and $T_{1}$, defined by

Here, $\lambda_{1}$, $\lambda_{2}$ and $\lambda_{3}$ are the three eigenvalues of system (3.5). Combining (3.9) and (3.13), (4.1) means

In what follows, we will show the validity of the hypothesis given by (4.1).

Let $x = x^{*}_{j}(t)\; (j = 1, 2, 3)$ be the characteristic curve of $\lambda_{j}$ that passes through (0, 0):

Since $\lambda_{3}(W) > \lambda_{2}(W) > \lambda_{1}(W)$, we have that $x = x^{*}_{3}(t)$ lies below $x = x^{*}_{2}(t)$ and $x = x^{*}_{2}(t)$ lies below $x = x^{*}_{1}(t)$. In what follows, we divide domain $G = \{(t, x)|t\geq0, \; x\in[0, L]\}$ into several different regions.

Region 1: The region $G_{1} = \left\{(t, x) \mid 0 \leq t \leq T_{1}, \; 0 \leq x \leq L, \; x \geq x_{3}^{*}(t)\right\}$.

For any point $(t, x) \in G_{1}$, integrating the $i$-th equation in (3.17) along the $i$-th characteristic curve about $t$ from 0 to $t$, we have

where we have used (4.3) and (4.4) and assumed that the line intersects the $x$ axis at $(0, b_{i})$. Similarly, integrating the $i$-th equation in (3.26) along the $i$-th characteristic curve and assuming that the line intersects the $x$ axis at $(0, b_{i})$ again, we get

and

where $\Theta = \Theta(\rho, \tilde{\rho}, S, S_{0})$. Adding (4.6)–(4.8) together, for any $i = 1, 2, 3$, and using Gronwall's inequality, one gets

Due to the boundedness of $T_{1}$, the arbitrariness of $(t, x)\in G_{1}$ and (4.9), it holds that

Region 2: The region $G_{2} = \left\{(t, x) \mid t \geq 0, \; 0 \leq x \leq L, \; 0 \leq x \leq x_{1}^{*}(t)\right\}$.

We make the change of variables $t$ and $x$. For any point $(t, x) \in G_{2}$, integrating (3.29) along the $i$-th characteristic curve about $x$, it follows that

where we assumed that the line intersects the $t$ axis at the point $(t_{i}, 0)$. Similarly, repeating the above procedure for (3.30), we get

Summing up (4.11) and (4.12) for $i$ = 1, 2, 3 and applying Gronwall's inequality, we obtain

where we exploit the arbitrariness of $(t, x)\in G_{2}$.

Region 3: The region $G_{3} = \left\{(t, x) \mid 0 \leq t \leq T_{1}, \; 0 \leq x \leq L, \; x_{2}^{*}(t) \leq x \leq x_{3}^{*}(t)\right\}$.

For any point $(t, x) \in G_{3}$, integrating the 1st and 2nd equations in (3.17) and (3.26) along the 1st and 2nd characteristic curve, we get

and

where we assumed that the line intersects the $x$ axis at points $(0, x^{\prime}_{1})$ and $(0, x^{\prime}_{2})$, respectively. Similarly, integrating the 3rd equations in (3.17) and (3.26) along the 3rd characteristic curve, one has

and

where the point $(t^{\prime}_{3}, 0)$ is the intersection of the line and the $t$ axis.

Since the boundary data are small enough, we sum up $(4.14)-(4.19)$ and apply Gronwall's inequality to obtain the following:

where we exploit the arbitrariness of $(t, x)\in G_{3}$.

Region 4: The region $G_{4} = \left\{(t, x) \mid 0 \leq t \leq T_{1}, \; 0 \leq x \leq L, \; x_{1}^{*}(t) \leq x \leq x_{2}^{*}(t)\right\}$.

For any point $(t, x) \in G_{4}$, integrating the 1st equations in (3.17) and (3.26) along the 1st characteristic curve, we get

and

where we assumed that the line intersects the $x$ axis at $(0, x^{\prime \prime}_{1})$. Similarly, integrating the 2nd and 3rd equations in (3.17) and (3.26) along the 2nd and 3rd characteristic curve, one has

and

where the line intersects the $t$ axis at points $(t^{\prime \prime}_{2}, 0)$ and $(t^{\prime \prime}_{3}, 0)$, respectively.

Noticing that the boundary data are small enough, we sum $(4.21)$–$(4.26)$ and then apply Gronwall's inequality to obtain

where we exploit the arbitrariness of $(t, x)\in G_{4}$.

From (4.10), (4.13), (4.20) and (4.27), we have proved that the assumption of (4.1) is reasonable. Therefore, we have obtained a uniform $C^{1}$ a priori estimate for the classical solution. Thanks to the classical theory in ^[34], we further obtain the global existence and uniqueness of $C^{1}$ solutions (see ^{[11,35,36,37,38,39]}) for problems (1.1)–(1.3). This proves Theorem 1.2.

5.   Temporal-periodic solution

In this section, we show that the smooth supersonic solution $W(t, x) = (\rho(t, x), u(t, x), S(t, x))^\top$ is temporal-periodic with a period $P > 0$, after a certain start-up time $T_{1}$, under the temporal periodic boundary conditions. Here, we have assumed that $W_{l}(t+P) = W_{l}(t)$ with $P > 0$.

For system (1.1), Riemann invariants $\xi$, $\eta$ and $\zeta$ are introduced as follows:

Then, system (1.1) can be transformed into the following form:

where

are three eigenvalues of system (1.1). For supersonic flow (i.e., $u > c$), we know that $\lambda_{3} > \lambda_{2} > \lambda_{1} > 0$. Obviously, (1.2)–(1.3) can be written as

where $\xi_{l} (t+P) = \xi_{l} (t), \; \eta_{l} (t+P) = \eta_{l} (t)$ and $\zeta_{l} (t+P) = \zeta_{l} (t)$ with $P > 0$.

We swap $t$ and $x$ so that the problem described by (5.2)–(5.4) takes the following form:

where $t > 0$ and $x\in[0, L]$. Next, we set

then, the Cauchy problem (5.5) can be simplified as follows:

where

According to

and (5.1), we can easily obtain

where the constant $J_{1}(>0)$ depends solely on $\; \tilde{\rho}, \tilde{u}, \gamma$ and $L$.

In order to prove that $W(t+P, x) = W(t, x)$, for any $t > T_{1}$ and $x \in [0, L]$, we first prove that the following conclusions hold:

where $T_{1}$ is the start-up time, which is defined in (4.3).

Let

then, according to (5.7), we obtain

where

Using the continuity of $\lambda_{i}\; (i = 1, 2, 3)$ and (5.8), after some calculations, we obtain the following estimates:

and

where the constants $J_{i}\; (i = 2, \cdots, 10)$ depend only on $\tilde{\rho}, \tilde{u}, \gamma$ and $L$.

In the above calculation, we have used

Now, fix a point $(t^{*}, x^{*})$ with $t^{*} > T_{1}$ and $0 < x^{*} < L$. Let $\Gamma_{1}: t = \check{t}_{1}(x)$ and $\Gamma_{3}: t = \check{t}_{3}(x)$ be two characteristic curves passing through point $(t^{*}, x^{*})$, that is,

and

where $x \in [0, x^{*}]$. Since $\lambda_{3}(W) > \lambda_{1}(W)$, $\Gamma_{1}$ lies below $\Gamma_{3}$. Set

where $0 \leq x < x^{*}$. According to the definition of $T_{1}$, and combining $t^{*} > T_{1}$ and $0 \leq x^{*} \leq L$, we obtain that $(\check{t}_{1}(0), \check{t}_{3}(0))\subset (0, +\infty)$. Then, it follows from (5.10) that $N(t, 0) \equiv 0$. Thus, $\Psi(0) = 0$.

Taking the derivative of $\Psi(x)$ with regard to $x$ gives

where we used (5.16) and (5.17).

Therefore, using Gronwall's inequality, we obtain that $\Psi(x) \equiv 0$. In addition, according to the continuity of $\Psi(x)$, we obtain that $\Psi(x^{*}) = 0$; then, $N(t^{*}, x^{*}) = 0$. Using the arbitrariness of $(t^{*}, x^{*})$, we get

Thus, (5.9) holds. Then, from $(5.1)$ and $c = \sqrt{a\gamma} e^{\frac{S}{2}} \rho^{\frac{\gamma -1}{2}}$, it follows that

for any $t > T_{1}$ and $x \in [0, L]$, where $T_{1}$ is the start-up time defined in (4.3). This proves Theorem 1.3.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was supported in part by the Natural Science Foundation of China Grant No. 12101372, No. 12271310, and the Natural Science Foundation of Shandong Province Grant No. ZR2022MA088.

Conflict of interest

The authors declare there is no conflict of interest.