Spatial propagation for a reaction-diffusion SI epidemic model with vertical transmission

1.   Introduction

The work is devoted to the study of spreading speed of the following reaction-diffusion SI epidemic model with vertical transmission

where $S(t, x)$ and $I(t, x)$ represent the densities of the susceptible individuals and the infective individuals, respectively, $0 < \theta < 1$ denotes the proportion of offspring born from an infective individual that is susceptible at birth, $\beta$ represents the incidence rate, $b$ and $b_I$ stand for the birth rate of the susceptible individuals and the infective individuals, respectively, $m$ defines the mortality rate of the individuals, $\frac{1}{\alpha}$ is the average infection cycle. Without loss of generality, we can assume that the birth rate of the susceptible individuals is not less than the infective individuals, and the death rate of the infective individuals is less than the birth rate of the infective individuals, that is, $0 < m + \alpha < b_I \leqslant b$. In addition, we consider system (1.1) associated with logistic growth, precisely speaking, $\frac{1}{k}$ expresses the carrying capacity of the country. Consequently, the following assumptions are made.

(A) We assume that $\alpha$, $\beta$, $b$, $b_I$, $\theta$, $m$ and $k$ are nonnegative constants. In addition, let $0 < m+\alpha < b_I\leqslant b$, $0 < \theta < 1$, $\beta - k > 0$, $(\beta - k)(b - m) \leqslant \theta k b_I$, $\hat{K} = \frac{b-m}{k}$, the two critical speeds $c^* = 2 \sqrt{b-m}$ and $c^{**} = 2 \sqrt{b_I - m -\alpha}$ and $S_0(x)$ and $I_0(x)$ be non-trivial, non-negative, uniformly continuous and bounded functions on $\Bbb{R}^N$.

In the paper, with regard to the basic reproduction number $R_0 = \frac{\beta \hat{K}}{b + \alpha - (1 - \theta) b_I}$ and the minimal wave speed $c^\diamond = 2 \sqrt{(\beta-k) \hat{K} +(1- \theta) b_I-m-\alpha}$, which have been defined in ^[1], we investigate the spreading properties of the corresponding solution of system (1.1). More precisely, we firstly prove some properties of the solution of system (1.1), which can be used to complete the proof of asymptotic behaviour of the solution for system (1.1). Secondly, we show that the solution of the system converges to the disease-free equilibrium as $t \rightarrow \infty$ if $R_{0} \leqslant 1$. Thirdly, if $R_0 > 1$, there exists the minimal speed $c^\diamond$ such that if $\|x\| = ct$ with $c \in (0, c^\diamond)$, the disease is persistent and if $\|x\| \geqslant ct$ with $c > c^\diamond$, the infection dies out is established. Finally, we illustrate the asymptotic behaviour of the solution of system (1.1) via numerical simulations.

In fact, the definition of spreading speed was firstly introduced by Aronson and Weinberger ^[2,3] for scalar reaction-diffusion equations and then applied by Aronson ^[4] to an integro-differential equation, that is, Aronson ^[4] established that there exist two speed $c_1$ and $c_2$ with $c_1 < \underline{c} < c_2$ satisfying if $\|x\| \geqslant c_2 t$, then $I(t, x)$ in the system can convergence to zero and $I(t, x)$ in the system can be more than zero if $\|x\| \leqslant c_1 t$, where $\underline{c}$ is asymptotic speed of spread of the model and $I(t, x)$ represents density of the infective individuals. After that, Weinberger ^[5,6] established asymptotic behavior of the solution of a discrete-time population model by using translation-invariant order-preserving operator. In addition, there have been other literatures studying the asymptotic speed of spread of the monotone reaction-diffusion equations or systems, see ^[2,7,8,9,10] and the cited reference therein.

Let us mention that a major difficulty encountered when studying (1.1) is the lack of comparison principle. Recently, there have been some results on spreading speed of some non-monotone reaction-diffusion systems, which lack the comparison principle. Ducrot et al. ^[11] investigated spreading speed of a large class of two-component reaction-diffusion systems, including prey-predator systems as a special case. Their conclusions includes the two cases, that is, the prey invades the environment faster than the predator and the predators population grows fast enough to always catch up with the prey. After that, Ducrot et al. ^[34] established spreading speed and the minimal wave speed of a predator-prey system with nonlocal dispersal. Furthermore, Ducrot et al. ^[12] took into account the large time behaviour of solutions of a three species reaction-diffusion system, modelling the spatial invasion of two predators feeding on a single prey species. For prey-predator systems, we refer to Ducrot ^[13], Lin ^[14] and Pan ^[15] and so on. Liu et al. ^[16] analyzed spreading speed of a competition-diffusion model with three species by using the Hamilton-Jacobi approach. In addition, Ducrot ^[17] established some conclusions for spreading speed of the SIR epidemic model with external supplies on the whole space $\Bbb{R}^n$.

In system (1.1), we consider the vertically transmitted infection. That is, it can be transmitted directly from the mother to an embryo, fetus, or baby during pregnancy or childbirth, such as rubella virus, cytomegalovirous, hepatitis B, HIV etc. (^[18,19]). In fact, it is obvious that the infectious disease can be transmitted through not only contact between the susceptible individuals and the infective individuals, but also vertical transmission in model (1.1). In other word, even in the absence of infected hosts, the disease can be also transmitted by vertical transmission. In addition, the minimal speed $c^\diamond = 2 \sqrt{(\beta-k) \hat{K} +(1- \theta) b_I-m-\alpha}$ of model (1.1) without vertical transmission $($namely, $\theta = 1)$ is less than that with vertical transmission $($that is, $\theta \in (0, 1))$, indicating that compared with infectious diseases without vertical transmission, infectious diseases with vertical transmission spread faster. Up to now, investigation of spreading speed of a reaction-diffusion epidemic model with vertical transmission is seldom. However, the existence of traveling waves for system (1.1) has been established (^[1]). They firstly summarized the dynamics of the corresponding kinetic system for (1.1) and then analyzed the threshold dynamics of system (1.1) on the bounded domain $\Omega \subset \Bbb{R}^n$. In particular, they investigated the existence of traveling waves of system (1.1) which connects the disease-free equilibrium with the endemic equilibrium. After that, Ducrot et al. ^[20] also analyzed the existence of traveling waves of system (1.1) under the situation of $d_S = 1$ and $\alpha = 0$ connecting the trivial equilibrium and the interior equilibrium by using center-unstable manifold around the interior equilibrium. Let us also mention that the existence of traveling wave solutions of other reaction-diffusion epidemic models have been extensively studied, see Anderson and May ^[21], Aronson ^[4], Brown and Carr ^[22], Diekmann ^[23], Hosono and Ilyas ^[24], Kenndy and Aris ^[25], Murray ^[26], Rass and Radcliffe ^[27], Ruan and Wu ^[28], Wang et al. ^[29,30,31] and the cited references therein.

The rest of the paper is organized as follows. In section 2, we state the main results of this work, namely, the solution of the system converges to the disease-free equilibrium as $t \rightarrow \infty$ if $R_{0} \leqslant 1$ and if $R_0 > 1$, there exists the minimal speed $c^\diamond > 0$ such that if $\|x\| = ct$ with $c \in (0, c^\diamond)$, the disease is persistent and if $\|x\| \geqslant ct$ with $c > c^\diamond$, the infection dies out. Section 3 deals with uniform boundedness of the solution of system (1.1) dedicated to the proof of the main theorems in the paper. Section 4 is concerned with the proof of the main results in section 2 describing the spatial spread of the infectious disease. In section 5, we illustrate the asymptotic behaviour of the solution of system (1.1) via numerical simulations.

2.   Main results

In this section, the main conclusions which shall be proved and discussed in the paper can be stated. Before stating our conclusions, let us introduce that $\Bbb{X} = BUC(\Bbb{R}^N, \Bbb{R}^2)$ be the Banach space of bounded and uniformly continuous functions from $\Bbb{R}^N$ to $\Bbb{R}^2$, which is endowed with the usual supremum norm. Its positive cone $\Bbb{X}^+$ consists of all functions in $\Bbb{X}$ with both nonnegative components.

Firstly, asymptotic behavior of the solution of system (1.1) if $R_0 \leqslant 1$ is investigated.

Theorem 1. Let $($A$)$ be satisfied and $N(0, x) = S(0, x) + I(0, x)$ on $\Bbb{R}^N$. If $R_0 \leqslant 1$, then the corresponding solution $(S, I)(t, x)$ of system $(1.1)$ satisfies the following properties:

$(1)$

uniformly with $x \in \Bbb{R}^N$.

$(2)$ If $N(0, x) \geqslant \delta, \; \forall x \in \Bbb{R}^N$, then

$(3)$ Assume that $S_0(x)$ and $I_0(x)$ are compactly supported on $\Bbb{R}^N$. Then

and

Theorem 1 shows that the solution $I$ of the system converges to zero as $t \rightarrow \infty$ if $R_0 \leqslant 1$, implying that the infection tends to dying out if $R_0 \leqslant 1$.

Next, we take into account asymptotic behavior of the solution of system (1.1) if $R_0 > 1$. The situation with $R_0 > 1$ is much more delicate. As mentioned above, the epidemic is sustained and persistent case $R_0 > 1$ under the semiflow associated to the corresponding kinetic system of system (1.1) ^[1]. In the spatially structured situation, we aim at describing the spatial spread of the epidemic. Now, let us turn to asymptotic behavior of the solution of system (1.1) case $R_0 > 1$ and $c \in (0, c^\diamond)$, where $c^\diamond = 2 \sqrt{(\beta - k)\hat{K} +(1- \theta) b_I-m-\alpha}$. Due to $(\beta - k)(b - m) \leqslant \theta k b_I$ and $\alpha \geqslant 0$ of $($A$)$, it has $c^\diamond \leqslant c^{**}\leqslant c^{*}$. Note that system (1.1) does not satisfy the parabolic comparison principle, that turns out to be one of the major difficulty to overcome.

Theorem 2. Assume that $($A$)$ is satisfied, $R_0 > 1$ and $w$ is a positive constant. Let $c \in (- c^\diamond, c^\diamond)$, $e \in \Bbb{S}^{N-1}$, $S_0(x)$ be compactly supported such that $0 \leqslant S_0(x) \leqslant w$, $0 \leqslant I_0(x) \leqslant w$ and $U_0 = (S_0, I_0)$. Let $\{t_n\}_{n \geqslant 0}$ be a given sequence such that $t_n \rightarrow \infty$ as $n \rightarrow \infty$. Then one has

locally uniformly for $(t, x) \in \Bbb{R} \times \Bbb{R}^N$, where $(S^\infty, I^\infty)$ is a bounded entire solution of (1.1) such that $\inf_{(t, x) \in \Bbb{R} \times \Bbb{R}^N}I^\infty(t, x) > 0$.

Remark 1. A bounded entire solution $(S^{\infty}, I^{\infty})$ of system (1.1) is said to be uniformly persistence if

In order to obtain a rather complete picture of the solution, Theorem 2 provides information on the long term asymptotic of uniformly persistent entire solutions $(S^\infty, I^\infty)$ of the Cauchy problem $(1.1)$ and more particularly on the quantity $(S, I)(t, cet)$ for $|c| < c^\diamond$, $e \in \Bbb{S}^{N-1}$ and large time, implying that when $R_0 > 1$ and $\|x\| = c t$ with $c \in [0, c^\diamond)$, the disease is persistent.

Based on the above arguments, we get the following proposition.

proposition 1. Assume that $($A$)$ is satisfied and $R_0 > 1$. Let $(S^\infty, I^\infty)$ be a uniformly persistent entire solution of system $(1.1)$. Then one has $S^\infty \equiv S^*$ and $I^\infty \equiv I^*$, where $S^*$ and $I^*$ have been defined in ^[1].

As discussed above the properties of uniformly persistent entire solutions provides that when $R_0 > 1$ then the set of uniformly persistent entire solutions only consists in the unique endemic equilibrium point $(S^\infty, I^\infty)$.

Next, we analyze asymptotic behavior of the solution of system (1.1) case $R_0 > 1$ and $c > c^\diamond$. The results are as follows.

Theorem 3. Assume that $($A$)$ holds and $R_0 > 1$. Let both $S_0(x)$ and $I_0(x)$ be compactly supported on $\Bbb{R}^N$. Then for each $c_2 > c_1 > c_0 > c^\diamond$, one has

and

Theorem 4. Let $($A$)$ be satisfied and $R_0 > 1$. In addition, suppose that there exists a positive constant $\eta$ such that $S_0(x) \geqslant \eta, \; \forall x \in \Bbb{R}^N$ and $I_0(x)$ is compactly supported on $\Bbb{R}^N$. Then the following result holds true for each $c > c_0 > c^\diamond$,

Theorems 3 and 4 can express that the critical minimal speed $c^\diamond$ becomes sharp in the sense that ahead the front, namely if $R_0 > 1$ and $|x|\geqslant ct$ for any $c \geqslant c^\diamond$, the infection dies out.

3.   Preliminary

In the section, we state some conclusions on the uniformly boundedness for the solution of the Cauchy problem (1.1) as $t \rightarrow \infty$, which can be used to prove Theorem 1-4. To solve it, we use parabolic estimates and the profile of propagation of the solution of Fisher-KPP reaction-diffusion equation, precisely speaking, consider the following Fisher-KPP equation

where the initial datum $u_0$ is assumed to satisfy non-trivial, continuous and compactly supported and $f: [0, 1] \rightarrow \Bbb{R}$ is of the class $C^1$ and $f$ satisfies $f(0) = f(1) = 0, f'(0) > 0 > f'(1), f(u) > 0$ for all $u \in (0, 1)$, together with the so-called KPP assumption

The problem has a long history, which was introduced in the pioneer works of Fisher ^[32] and Kolmogorov, Petrovskii and Piskunov ^[33] to model some problems in population dynamics. Aronson and Weinberger in 1970s proved that the solution $u = u(t, x)$ of system (3.1) together with $f(u) = u(1-u)$ owns the so-called asymptotic speed of spread property:

where the speed $c^\nu = 2 \sqrt{f'(0)}$, $\|\cdot \|$ denotes the Euclidean norm on $R^n$ and 0 and 1 are two equilibria of the system.

lemma 1. Let $($A$)$ be satisfied. For each initial data $U_0 = (S_0, I_0) \in X^+$, the solution $(S, I)(t, x; U_0) = (S, I)(t, x)$ of system $(1.1)$ satisfies the following properties:

(i) $(S, I) \in C([0, \infty); \Bbb{X}^+)$;

(ii) Let $N(t, x) = S(t, x) + I(t, x)$. Then we get the following conclusions:

$(1)$

$(2)$ Let both $S_0(x)$ and $I_0(x)$ be compactly supported on $\Bbb{R}^N$. Then

and

$(3)$ For each a positive constant $\delta$, one has

Proof. It is easy to see that conclusion (i) holds and we only prove (ii). Obviously, according to $b_I \leqslant b$ in $($A$)$, $N(t, x)$ satisfies

Consequently, $N(t, x)$ can be dominated by

By a directly computation, one gets

It follows from the parabolic maximum principle that

Secondly, $N(t, x)$ also satisfies

Consequently, the parabolic maximum principle implies that

which $\underline{N}(t, x)$ and $\tilde{N}(t, x)$ are solutions of the following systems

and

respectively. It is easy to see that system (3.4) and (3.5) are of the usual Fisher-KPP form. Similar to the conclusion (3.2) of system (3.1) together with $f(u) = u(1-u)$, one has

for (3.4) and

and

for (3.5), which indicates that conclusion (2) of (ii) holds true.

At last, we consider the following system

A straightforward computation yields

Using the parabolic maximum principle associated with (3.3), we obtain

It completes the proof.

4.   Proof of the main results

4.1.   Proof of Theorem 1

Now, using Lemma 1 together with usual limiting arguments and parabolic estimates, we are able to complete the proof of Theorem 1.

Proof of Theorem 1 Let $N(t, x) = S(t, x) + I(t, x)$ for every $(t, x) \in \Bbb{R}^+ \times \Bbb{R}^N$. Due to

in Lemma 1, it has that for every $\epsilon > 0$, there exists a positive constant $T$ large enough such that $N(t, x) \leqslant \hat{K} + \epsilon, \; \forall t > T, \; x \in \Bbb{R}^N$, which means that for each $(t, x) \in (T, +\infty) \times \Bbb{R}^N$, we can obtain

by using $\beta - k > 0$ of $($A$)$.

Furthermore, consider the following equation

By the straightforward computation and $R_0 \leqslant 1$, we can get

In addition, the parabolic maximum principle implies that $I(t, x) \leqslant u (t; \eta_1), \; \forall t > T, \; x \in \Bbb{R}^N$. Thus, it has

It follows from the arbitrariness of $\epsilon > 0$ that

In addition, (1) of (ii) in Lemma 1 implies that

In view of (4.1), we have

Furthermore, according to (3) of (ii) in Lemma 1, that is, if $N(0, x) \geqslant \delta, \; \forall x \in \Bbb{R}^N$, then one has

According to (4.1) and (4.2), one has

Similarly, (2) of (ii) in Lemma 1 can deduce conclusion (3).

This completes the proof.

4.2.   Proof of Theorem 2

The aim of this section is to prove Theorem 2. The proof of Theorem 2 will depend on uniform persistence like arguments. The section is divided into the following three subsections: (1) we devote to the proof of weak uniform persistence property, (2) we focus on the proof of uniform persistence property, (3) Theorem 2 is proved.

4.2.1.   Weak uniform persistence

Before proving a weak uniform persistence property of solution of system (1.1), let us first state the following results that will be used in the proof of a weak uniform persistence property.

lemma 2. Let $a \in \Bbb{R}$ be given and $e \in \Bbb{S}^{N - 1}$. For each $L > 0$, $c \in \Bbb{R}$ and $B(0, L)$ be a sphere with radius $L$, consider the principle elliptic eigenvalue problem[17, Lemma 5.2]

Then $\lambda_{L}[c, e]$ does not depend upon $e \in \Bbb{S}^{N - 1}$, it is denoted by $\lambda_{L}[c]$ and one has

locally uniformly for $c \in \Bbb{R}$.

lemma 3. Fix $c_0 \in [0, c^\diamond)$. Let $S_0(x)$ be compactly supported on $\Bbb{R}^N$ such that $0 \leqslant S_0(x) \leqslant w$ and $0 \leqslant I_0(x) \leqslant w$($w$ is a positive constant). Assume that for each $n \geqslant 0$, there exist some initial values $U_0^n = (S_0^n, I_0^n)$, $x_n \in \Bbb{R}^N$, $c_n \in [-c_0, c_0]$ and $e_n \in \Bbb{S}^{N-1}$ so that the solution of system $(1.1)$, defined by $(S^n, I^n)$, satisfies

Let $\{t_n\}_{n \geqslant 0}$ be a given sequence such that $t_n \rightarrow \infty$ as $n \rightarrow \infty$. Then one has

uniformly for $t \geqslant 0$ and $x$ in a bounded sets.

Proof. Obviously, it has

Consider the sequence of functions $u_n$ and $v_n$ defined by

In view of (4.3), one has

Due to the uniformly boundedess of the solution of system (1.1) provided by Lemma 1 and parabolic estimates, possibly up to subsequence, one may assume that $(u_n, v_n) \rightarrow (u_\infty, v_\infty)$ locally uniformly for $(t, x) \in \Bbb{R} \times \Bbb{R}^N$. According to $\{ c_n\}_{n \geqslant 0} \in [-c_0, c_0]$, one may also assume that $c_n \rightarrow c \in [-c_0, c_0]$ as $n \rightarrow \infty$. Next, the function $(u_\infty, v_{\infty})$ satisfies

Furthermore, (4.5) leads to $v_\infty(t, 0) = 0$ for any $t \geqslant 0$, which implies that $v_\infty(t, x) \equiv 0$ by the parabolic maximum principle.

Let $L > 0$ be given. Let us assume by contradiction that $v_n \rightarrow 0$ as $n \rightarrow \infty$ but not uniformly on $[0, \infty) \times \bar{B}(0, L)$, which means that there exists a sequence $(t_n, x_n) \in [0, \infty) \times \bar{B}(0, L)$ such that $v_n(t_n, x_n) \geqslant \epsilon, \; \forall t \geqslant 0$ for some $\epsilon > 0$. Without loss of generality, we assume that $x_n \rightarrow x_\infty \in \bar{B}(0, L)$ and $t_n \rightarrow \infty$ as $n \rightarrow \infty$. Consider the function sequence $w_n(t, x) = v_{n}(t + t_n, x)$. According to the parabolic estimates, one has $w_n \rightarrow w_\infty$ as $n \rightarrow \infty$ locally uniformly for $(t, x) \in \Bbb{R} \times \Bbb{R}^N$. In particular, $w_\infty(0, x_\infty) \geqslant \epsilon$. Moreover, using (4.4) and (4.5), one has that $w_\infty$ satisfies

where $a \equiv a(t, x)$ is some given bounded function. Here again, it follows from the parabolic maximum principle that $w_\infty(t, x) \equiv 0$. There is a contradiction with $w_\infty(0, x_\infty) \geqslant \epsilon$. Consequently, one obtains

Now, we show that $u_n \rightarrow \hat{K}$ uniformly for $t \geqslant 0$ and locally in $x \in \Bbb{R}^N$ by using the contradiction way. Let $L > 0$ be given and assume that there exist a $\epsilon > 0$ and a sequence $(t_n, x_n) \in (0, \infty)\times B(0, L)$ such that

Let $x_n \rightarrow x_\infty \in \overline{B(0, L)}$, then one has $u_n(t_n + \cdot, \cdot) \rightarrow u^\infty$ as $n \rightarrow \infty$ locally uniformly by using the parabolic estimates. Thus, (4.8) implies that

where $u^\infty$ is a bounded entire solution of

Obviously, the right side of the above equation $u^\infty$ satisfies Fisher-KPP hypothesis. Consider the following equations

From conclusion (3.2) of system (3.1) together with $f(u) = u(1-u)$, it follows that

Due to $c^\diamond \leqslant c^*$, one has $u^\infty(t, x) \equiv \hat{K}$, which causes to a contradiction with the inequality (4.9). This completes the proof.

Now, we discuss the proof of a weak uniform persistence property.

Theorem 5. Assume that $($A$)$ is satisfied, $R_0 > 1$ and $w$ is a positive constant. Fix $c_0 \in [0, c^\diamond)$. Let $S_0(x)$ be compactly supported on $\Bbb{R}^N$ such that $0 \leqslant S_0(x) \leqslant w$ and $0 \leqslant I_0(x) \leqslant w$. Then there exists $\epsilon = \epsilon(w, c_0) > 0$ such that for each $x \in \Bbb{R}^N$, for each $e \in \Bbb{S}^{N-1}$, for each $c \in [-c_0, c_0]$ and any $U_0 = (S_0, I_0)$, it holds that

where $(S(t, x; U_0), I(t, x; U_0))$ denotes the solution of system $(1.1)$ with initial value $U_0$.

Proof. We prove the result by contradiction. Assume that for each $n \geqslant 0$, there exist some initial values $U_0^n = (S_0^n, I_0^n)$, $x_n \in \Bbb{R}^N$, $c_n \in [-c_0, c_0]$ and $e_n \in \Bbb{S}^{N-1}$ so that the solution of system (1.1), defined by $(S^n, I^n)$, satisfies

Let the sequence of functions $u_n$ and $v_n$ be defined by

Due to Lemma 3, it has

uniformly on $t \geqslant 0$ and $x \in B(0, L)$, where $B(0, L)$ is a sphere with radius $L$ and $L > 0$ is a constant. Therefore, there exist $\eta > 0$(it will be determined later) and $n_\eta > 0$ such that

Let $N_n(t, x) = u_n(t, x) + v_n(t, x)$. Then the function $v_n$ satisfies for each $n > n_\eta$, $t \geqslant 0$ and $x \in B(0, L)$,

which implies that

where $a_\eta : = - [(\beta - k) \hat{K} + (1 - \theta) b_I - \alpha - m - (\beta + k) \eta]$.

Based on $R_0 > 1$ and $c_0 < c^\diamond$, let $\eta > 0$ be given small enough such that

Consider the principle eigenvalue of the following problem

Furthermore, the principle eigenvalue of (4.12) is denoted by $\lambda_{L}$. According to Lemma 2, it is obvious that $\lambda_{L}$ satisfies

It further follows from (4.11) that

indicating that there exists a sufficiently large constant $L_{0} > 0$ such that

Let $n \geqslant n_\eta$ be given and the function $\Theta_0 : B(0, L) \rightarrow [0, \infty)$ be defined as a principle eigenfunction of (4.12). Consider $\delta > 0$ small enough such that $v_n(0, x) \geqslant \delta \Theta_0(x), \; \forall x \in B(0, L)$. In addition, it is clear that the function $\underline{v}(t, x) = \delta e^{- \lambda_L t}\Theta_0(x)$ satisfies

Since there are

we infer from the parabolic maximum principle that

Due to $\lambda_L < 0$, we obtain $v_n(t, x) \rightarrow \infty$ as $t \rightarrow \infty$, which leads to a contradiction with (4.5). This completes the proof of the result.

4.2.2.   Uniform persistence

Secondly, we show the uniform persistence of the solution of model (1.1) by using dynamical system arguments, namely, parabolic regularity and weak dissipativity.

proposition 2. Assume that $($A$)$ is satisfied, $R_0 > 1$ and $w$ is a positive constant. Fix $c_0 \in [0, c^\diamond)$. Let $S_0(x)$ be compactly supported on $\Bbb{R}^N$ such that $0 \leqslant S_0(x)\leqslant w$ and $0 \leqslant I_0(x) \leqslant w$. Then there exists $\epsilon = \epsilon(w, c_0) > 0$ such that the initial value $U_0 = (S_0, I_0)$, each $c \in [-c_0, c_0]$, each $x \in \Bbb{R}^N$ and $e \in S^{N-1}$, then it has

Proof. Let us argue by contradiction. Assume that there exists a sequence of initial data $\{ U_0^m = (S_0^m, I_0^m)\}_{m \geqslant 0}$, $\{x_m\}_{m \geqslant 0} \in \Bbb{R}^N$ and $\{e_m\}_{m \geqslant 0} \in \Bbb{S}^{N-1}$ such that the sequence of solution of system (1.1) defined by $(S^m, I^m)$ satisfies

Let $\epsilon = \epsilon(w, c_0) > 0$ be the constant provided by Theorem 5. Then one has

for each $U_0$, $x \in \Bbb{R}^N$ and $e \in \Bbb{S}^{N-1}$.

Set $U^m = S^m(t, x_m + x + c t e_m; U_0^m)$ and $V^m(t, x) = I^m(t, x_m + x + c t e_m; U_0^m)$ for $t \geqslant 0$ and $x \in \Bbb{R}^N$. Then there exist a sequence $\{t_m\}_{m \geqslant 0}$ tending to $\infty$ and a sequence $\{a_m\}_{m \geqslant 0} \in (0, \infty)$ such that for each $m \geqslant 0$, it holds that

Up to a subsequence, one may assume that $V^m(t + t_m, x) \rightarrow V^\infty(t, x)$ and $U^m(t + t_m, x) \rightarrow U^\infty(t, x)$ locally uniformly for $(t, x) \in \Bbb{R}\times \Bbb{R}^N$, and $\tilde{L} = \liminf_{m \rightarrow \infty} a_m$ and $\liminf_{m \rightarrow \infty} e_m = e$. Then, the function $V^\infty$ satisfies

In addition, $(U^\infty, V^\infty)$ satisfies the following system

If $\tilde{L} < \infty$, one obtains $V^\infty(\tilde{L}, 0) = 0$, indicating that $V^\infty(t, x) \equiv 0$ for $(t, x) \in \Bbb{R} \times \Bbb{R}^N$ by the parabolic maximum principle. Consequently, it contradicts the fact $V^\infty(0, 0) = \frac{\epsilon}{2}$. If $\tilde{L} = \infty$ which means that $a_m \rightarrow \infty$ as $m \rightarrow \infty$, one has

Now recall that the function $(\hat{S}^\infty, \hat{I}^\infty)$ defined by

satisfies the system

It further follows from Theorem 5 that

implying that $\limsup_{t \rightarrow \infty}V^\infty(t, 0) \geqslant \epsilon$. As a consequence, it contradicts the fact (4.13). This completes the proof.

Proof of Theorem 2 Let $\{t_n\}_{n \geqslant 0}$ be a given sequence such that $t_n \rightarrow \infty$ as $n \rightarrow \infty$ and

Using the standard parabolic estimates, one may assume that $\{(U_n, V_n)\}$ converges towards some function pair $\{ (U, V)\}$ which is an entire solution of the following system

locally uniformly for $(t, x) \in \Bbb{R} \times \Bbb{R}^N$. In view of Proposition 2, one obtains that there exists a $\epsilon > 0$ satisfying

Note that $(S^\infty, I^\infty)(t, x) \equiv (U, V)(t, x+cet)$ is an entire solution of (1.1). It completes the proof.

4.2.3.   Proof of Theorem 1

We now consider the uniformly persistent entire solutions of system (1.1). The following classification holds true.

lemma 4. Let $($A$)$ be satisfied and $R_0 > 1$. Let $(S^\infty, I^\infty)$ be a given uniformly persistence entire solution of system $(1.1)$. Then there exists a $\epsilon \in (0, 1)$ such that

Proof. By Lemma 1, there exists a $\epsilon_0 \in (0, 1)$ such that $S^\infty(t, x) \leqslant \epsilon_0^{-1}$ and $I^\infty(t, x) \leqslant \epsilon_0^{-1}$ for any $(t, x) \in \Bbb{R} \times \Bbb{R}^N$. Let

for some $\epsilon_1 > 0$. Next, it is sufficient to show

for some $\epsilon_2 > 0$ by using a contradiction way. Assume that there exists $(t_n, x_n) \in \Bbb{R} \times \Bbb{R}^N$ such that $S^\infty(t_n, x_n) \rightarrow 0$ as $n \rightarrow \infty$. Let

Then $S^\infty_n \rightarrow \bar{S}$ and $I^\infty_n \rightarrow \bar{I}$ in $C^{1, 2}_{loc}(\Bbb{R} \times \Bbb{R}^N)$. $(\bar{S}, \bar{I})$ satisfies $0 \leqslant \bar{S}$, $\epsilon_1 \leqslant \bar{I} \leqslant \epsilon_0^{-1}$, $\bar{S}(0, 0) = 0$ and

Plugging the point $(0, 0)$ into the above equation, we can obtain $\theta b_I \bar{I}(0, 0) \leqslant 0$, which leads to a contradiction. As a consequence, there exists a $\epsilon_2 > 0$ such that $\inf_{(t, x) \in \Bbb{R} \times \Bbb{R}^N}S^\infty(t, x) > \epsilon_2$. The proof is completed.

Based on the above arguments, Theorem 1 can be proved.

Proof of Theorem 1 Consider the positive maps $g: (0, \infty) \rightarrow \Bbb{R}$ defined by $g(x) = x - 1 - \ln x$ and let us define the function $W: \Bbb{R} \times \Bbb{R}^N \rightarrow [0, \infty)$ by

where $V_S$ and $V_I$ are two constants and satisfy

By a straightforward computation together with $($A$)$, for $(t, x) \in \Bbb{R} \times \Bbb{R}^N$, one has

Due to Lemma 4, $W$ is uniformly bounded. Let $(t_n, x_n) \in \Bbb{R} \times \Bbb{R}^N$ be a given sequence satisfying

Consider the sequences $u_n(t, x) = S^\infty(t + t_n, x + x_n)$, $v_n(t, x) = I^\infty(t + t_n, x + x_n)$ and $W_n(t, x) = W(t + t_n, x + x_n)$. Up to a subsequence, assume that $u_n \rightarrow u$ and $v_n \rightarrow v$ locally uniformly on $\Bbb{R} \times \Bbb{R}^N$. Consequently, one gets that

where $\hat{W}(t, x)$ satisfies

The parabolic maximum principle implies that $\hat{W}(t, x) \equiv \sup_{\Bbb{R} \times \Bbb{R}^N} \hat{W}(t, x) \equiv \hat{W}(0, 0)$. From system (4.14), one obtains

which implies that $v(t, x) \equiv I^*$ and thus $\hat{W} \equiv 0$. It further follows that $W(t, x) \equiv 0$, expressing that $S^\infty(t, x) \equiv S^*$ and $I^\infty(t, x) \equiv I^*$. It completes the proof.

4.3.   Proof of Theorems 3 and 4

In this section, the outer spreading property stated in Theorems 3 and 4 can be proved.

Proof of Theorem 3 By Lemma 1 and $($A$)$, there exists a $T_\delta > 0$ such that

for any $t \geqslant T_\delta$ and $x \in \Bbb{R}^N$. It further follows from Lemma 4.5 in ^[1] that the map $\bar{I}(\xi) = e^{- \lambda (|x| - c_0t)}, \; \forall \xi : = |x| - c_0t \in \Bbb{R}$ satisfies the following equation

Let $u(t, x) = \delta e^{- \lambda (|x| - c_0t)}, \; \forall (t, x) \in \Bbb{R}^+ \times \Bbb{R}^N$, where $\delta$ satisfies $u(T_\delta, x) \geqslant I(T_\delta, x), \; \forall x \in \Bbb{R}^+.$ Using the parabolic maximum principle, we have

Due to $|x|\geqslant c_1 t$, $c_1 > c_0$ and $\lambda > 0$ (Lemma 4.5 in ^[1]), we get

At last, by using the similar arguments to conclusion (3) of Theorem 1 associated with the second conclusion in Lemma 1, we obtain Eqs (2.1), (2.2) and (2.3). The proof is completed.

Proof of Theorem 3 Similar to Theorem 3, we can get

In addition, based on (3) of Lemma 1, it has

This completes the proof.

5.   Numerical simulations and discussion

In this section, we provide some numerical simulations to confirm the long-term temporal dynamics of system (1.1).

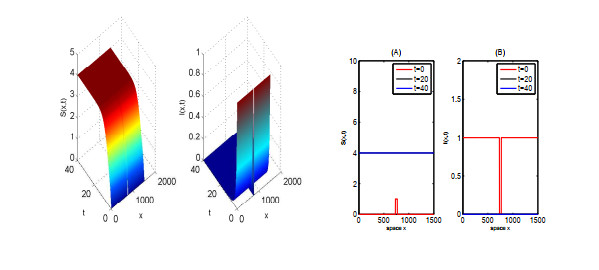
Firstly, the case when $R_0 \leqslant 1$ is described in Theorem 1. Our results are divided into three parts: (1) solution $I$ of the system converges to zero as $t \rightarrow \infty$, implying that the infection tends to dying out if $R_0 \leqslant 1$. In addition, solution $S$ of the system is not larger than $\hat{K}$ as $t \rightarrow \infty$. (2) if the initial value of the individuals is greater than 0, then the susceptible individuals are not tending to 0 at larger time. (3) if the initial value of the individuals is compactly supported, then there is a single propagating front with a critical speed $c^*$ defined in $($A$)$, ahead of which the solution $S$ of the system converges to zero, and behind the front it perhaps does not converges to 0. To illustrate conclusion (3), we take some parameters of the model as below:

which satisfies $($A$)$. Using these parameters, we obtain the basic reproduction number $R_0 = \frac{\beta \hat{K}}{b + \alpha -(1- \theta)b_I} \approx 0.93 < 1$ and a critical speed $c^* = 2\sqrt{b-m} \approx 0.6$. Furthermore, we truncate the spatial domain $\Bbb{R}$ by $[0, 1500]$ and the time domain $\Bbb{R}^+$ by $[0, 40]$ and use the following piecewise functions as initial conditions:

and

In addition, we take Neumann boundary condition for system (1.1). Consequently, Figure 1 illustrates the simulation result on the solution of (1.1) with the given parameters, which shows the above conclusions (1) and (3) with Theorem 1.

Now, we discuss conclusion (2), namely, if the initial value of the individuals is greater than 0, then the susceptible individuals are not tending to 0 at larger time. To the aim, we choose the same parameters as (5.1) and the following piecewise functions as initial conditions:

and

In addition, we also take Neumann boundary condition for system (1.1). Figure 2 shows that the above conclusion (2), implying that the infection tends to dying out if $R_0 \leqslant 1$. It is worth noting that the solution $S$ of the system converges to $\hat{K} = \frac{b-m}{k} = 4$ in Figure 2.

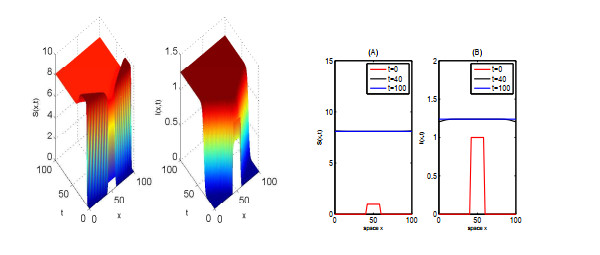
Secondly, we focus on the case when $R_0 > 1$ and $\|x\| = ct$ with $c \in (0, c^\diamond)$, which can be described in Theorems 2.2 and 1. In order to simulate it, the following parameters are taken:

which also satisfies $($A$)$. Based on these parameters, we obtain the basic reproduction number $R_0 \approx 1.16 > 1$, a critical speed $c^\diamond = 2 \sqrt{(\beta -k)\hat{K} + (1- \theta)b_I-m-\alpha} \approx 1.36$ and the disease equilibrium $(S^*, I^*) = (8.0926, 1.2385)$. In addition, we choose the following conditions for the initial value problem:

and

We further truncate the spatial domain $\Bbb{R}$ by $[0, 100]$ and the time domain $\Bbb{R}^+$ by $[0, 100]$. Figure 3 expresses that if $R_0 > 1$ and $\|x\| = ct$ with $c \in (0, c^\diamond)$, the solution of system (1.1) tends to $(S^*, I^*)$ as $t \rightarrow \infty$.

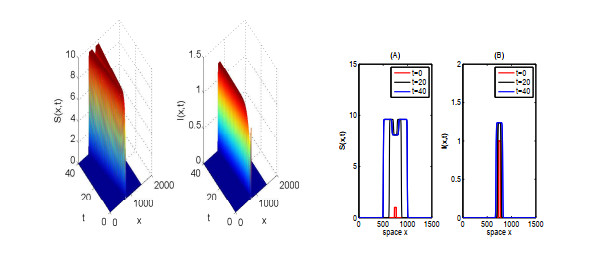
At last, the case with if $R_0 > 1$ and $\|x\|\geqslant ct$ with $c > c^\diamond$ is stated in Theorems 3 and 4. In order to showing Theorem 3, we take the same parameters and initial conditions as (5.4), (5.2) and (5.3), respectively. We also use the spatial domain $\Bbb{R}$ by $[0, 1500]$ and the time domain $\Bbb{R}^+$ by $[0, 40]$. Figure 4 indicates Theorem 3, precisely speaking, there are the following three zones: (1) If $\|x\|\leqslant ct$ with $c \in (0, c^\diamond)$, then the solution of system (1.1) tends to $(S^*, I^*) = (8.0926, 1.2385)$; (2) The zones roughly between $c^\diamond t$ and $c^*t$, where the solution of the system converges to $(\frac{b-m}{k}, 0) = (9.6, 0)$; (3) Ahead of the moving frame with speed $c^*$, where both the susceptible and infective individuals "die out".

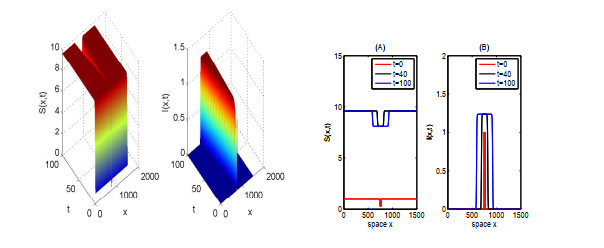
Next, we choose the same parameters as (5.4) and the following conditions for the initial value problem£º

and

In addition, we truncate the spatial domain $\Bbb{R}$ by $[0, 1500]$ and the time domain $\Bbb{R}^+$ by $[0, 100]$. As a consequence, Figure 5 illustrates Theorem 4, namely, there is a single propagating front with a critical speed $c^\diamond \approx 1.36$, ahead of which the solution of the system converges to $(\frac{b-m}{k}, 0) = (9.6, 0)$, and behind the front the solution of the system tends to $(S^*, I^*) = (8.0926, 1.2385)$.

Acknowledgments

Researcher was supported by National Natural Science Foundation of China(11801244), the HongLiu first class disciplines development program of Lanzhou University of Technology.

Conflict of interest

The authors declare no conflict of interest in this paper.