A new multi-group alcoholism model with public health education and alcoholism age is considered. The basic reproduction number R0 is defined and mathematical analyses show that dynamics of model are determined by the basic reproduction number. The alcohol-free equilibrium P0 of the model is globally asymptotically stable if R0≤1 while the alcohol-present equilibrium P∗ of the model exists uniquely and is globally asymptotically stable if R0>1. The Lyapunov functionals for the globally asymptotically stable of the multi-group model are constructed by using the theory of non-negative matrices and a graph-theoretic approach. Meanwhile, the combined effects of the public health education and the alcoholism age on alcoholism dynamics are displayed. Our main results show that strengthening public health education and decreasing the age of the alcoholism are very helpful for the control of alcoholism.
1.
Introduction
A small intake of alcohol may be beneficial to health, but alcoholism, also known as alcohol dependence or alcohol abuse, is among the main healthy risky behavior due to the high relevance of negative health and social effect. Alcohol consumption has been identified as a major contributor to the global burden of chronic disease, injury and economic cost [1,2,3]. The World Health Organization reports the harmful use of alcohol causes approximately 3.3 million deaths every year (or 5.9% of all the global deaths), and 5.1% of the global burden of disease is attributable to alcohol consumption [4]. Furthermore, alcoholism is reported to be among the major concerns to public health in many countries, like UK [5,6]. Recently, the study of alcoholism model has become an important aspect of social epidemic. A great number of literatures can be found where healthy risky behavior, including drinking, smoking, drug use and obesity, are viewed as a treatable contagious disease, see [7,8,9,10] and the reference contained therein. In particular, several alcohol models described by ordinary differential equations or delay differential equations have been investigated extensively (see [11,12,13,14,15,16,17,18,19,20,21,22]). In [11], the authors modelled alcoholism as a contagious disease and studied how "infected" drinking buddies spread problem drinking. Manthey et al. [12] studied campus drinking and suggested that the basic reproductive numbers are not sufficient to predict whether drinking behavior will persist on campus and that the pattern of recruiting new members play a significant role in the reduction of campus alcohol problems. The impact of environmental factors and peer influences on the distribution of heavy drinking was studied in [13,16]. In addition, the two-stage models: one stage where people who admit to having a alcohol problem and other stage where people who do not admit to having a alcohol problem have been developed in [17,18]. Bhunu [19] studied the co-interaction of alcoholism and smoking in a community. Walters et al. [20] also discussed alcohol problems, and their results showed that an increase in the recovery rate decreased the proportion of binge drinkers in the population. Xiang et al. [21] considered the effect of constant immigration on drinking behavior. Wang et al. [22] presented a deterministic mathematical model for the spread of alcoholism with two control strategies to gain insights into this increasingly concerned about health and social phenomenon. The optimal control strategies are derived by proposing an objective functional and using Pontryagin's Maximum Principle. Zhu et al. [23] formulated a alcohol model with the impact of tax and investigated their dynamical behaviors. Other related drinking epidemic or population models, we refer to see [24,25,26,27,28,29,30,31,32,33].
Public health education (e.g. Radio, Newspapers, Billboards, TV, and Internet, etc.) has been used to control the alcohol problems, which can not only influence the individuals' behavior but also increase the governmental health care involvement to control the spread of heavy drinking. These behavioral responses can change the transmission patterns and decline to drink. In recent years, many mathematical models [34,35,36,37] have been used for studying the impact of awareness programs by media on drinking problem. In [35], the authors studied drinking dynamics and focused on awareness programs and treatment in the modelling process. They extended the model in [34] via including a treatment class and established some sufficient conditions for the stability of the alcohol-free and alcohol-present equilibria. Xiang et al. [36] also studied a drinking model with public health education campaigns. Their results showed that awareness programs is an effective measure in reducing alcohol problems. These studies suggested that public health education and media had huge impact in controlling the spread of alcoholism. Recently, Ma et al. [37] proposed an alcohol consumption model with awareness programs and time delay described by including media function SMk0+M, where M was the cumulative density of awareness programs driven by media. The results showed that the time delay in alcohol consumption habit which developed in susceptible population might result in a Hopf bifurcation.
Actually, it has been found that heterogeneity (e.g., age, sex, space and so on) exists in many aspects of social epidemic transmission processes. Since multi-group models play important roles in considering the heterogeneity of host population, the study of the multi-group models can contribute to clarify the transmission pattern of infectious diseases in more realistic situations. There are lots of studies of the global stability of multi-group epidemic models, in which a general approach is used (see [38,39,40,41,42,43,44,45,46]). This graph-theoretic approach is sufficiently general to be applicable to a variety of coupled systems. On the other hand, since the pioneering work of Hoppensteadt established an age-dependent epidemic model in 1974 [47], the effects of the age factor on the multi-group epidemic models have already been studied by many researchers (see [48,49,50,51]). Dynamical behaviors of these multi-group epidemic models with age structure have been studied, respectively. However, to our knowledge, there are few studies on the alcoholism model in heterogeneous populations. In particular, we should notice that the impact of the age of alcoholism on other people is different. Thus, the study of the multi-group alcoholism model with public health education and age of alcoholism can contribute to the control of alcohol problems in more realistic situations.
In this paper, motivated by the above works, we formulate a novel and more reasonable multi-group alcoholism model with public health education and alcoholism age to describe alcoholism spread in a heterogeneous host population. Inspired by the method developed in [38,39,40], we construct Lyapunov functionals and obtain the global stability of the alcohol-free equilibrium and alcohol-present equilibrium. Our results demonstrate that, for age structured multi-group alcoholism model, this graph-theoretic approach can be successfully applied by choosing an appropriate weighted matrix as well. At the same time, our main results indicate that public health education is beneficial for alcoholism control, and alcoholism age structure does not alter the dynamical behaviors.
This paper is organized as follows. In next section, we formulate a more reasonable multi-group alcoholism model with public health education and alcoholism age. Some preliminary setting for the multi-group and age-dependent alcoholism model are presented in Section 3. In Section 4, we state our main results of this paper. We prove the global asymptotic stability of the alcohol-free equilibrium P0 for R0≤1 by using the theory of non-negative matrices and the classical method of Lyapunov functional. By applications of the graph-theoretic approach to the method of Lyapunov functionals, we prove the existence, uniqueness and global asymptotic stability of the alcohol-present equilibrium P∗ for R0>1. In Section 5, the effects of the public health education and alcoholism age are given. A brief discussion is also given in last section.
2.
Model formulation
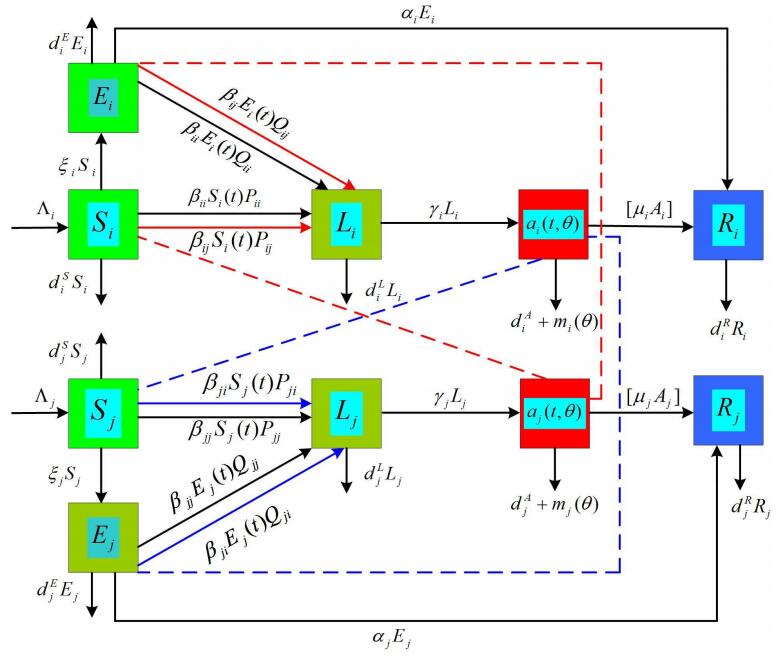
Let n∈N be the number of groups, the heterogeneous host population is divided into n homogeneous groups. For i-th (1≤i≤n) group, it is further classified as five compartments: the uneducated susceptible individuals Si(t) who do not drink or drink only moderately and do not accepted the public health education, but may one day develop light drinkers, the educated susceptible individuals Ei(t) who do not drink or consume alcohol in moderation and accepted the public health education, but may one day also develop light drinkers, the light drinkers Li(t) who often consume alcohol but they don't influence other people, the alcoholics Ai(t) who have drinking problems or addictions (i.e. heavy drinkers) and they will influence susceptible individuals, and the recovered drinkers Ri(t) who are the recovered drinkers and permanently quit drinking. In i-th (1≤i≤n) group, we assume that the susceptible individuals, the light drinkers and the recovered drinkers are homogeneous at time t. The alcoholics individuals is structured by the age of alcoholism θ, and ai(t,θ) be the alcoholism age density of the individuals in the alcoholism individuals with alcoholism age θ at time t. Suppose that ai(t,θ)=0 for all sufficiently large θ>θ+, where θ+ is the maximum ages of alcoholism which is finite. Then Ai(t)=∫∞0ai(t,θ)dθ is the number of heavy drinkers at time t. Furthermore, we make the following assumptions:
(H1) In group i, at any moment in time, new recruits enter the uneducated susceptible population at a rate Λi>0.
(H2) In group i, via public health education, the uneducated susceptible individuals in Si class turn into the educated susceptible individuals Ei class at a constant rate ξi>0.
(H3) In group i, the coefficient of alcoholism transmission for susceptible individuals (Si or Ei) turn into light drinkers (Li) is βij≥0 for some reasons (e.g. through peer pressure), in which a susceptible individual contact with heavy drinkers Aj come from the j-th group.
(H4) Some educated individuals who never have a drink due to the effect of the public health educational campaigns, and the proportion αi>0 is that fraction of Ei who move into the Ri class.
(H5) The heavy drinkers in Ai class can recover (due to counselling, health reasons, treatment, prohibition, or tax hiked on alcohol beverages, etc.) and will permanently quit drinking. The proportion μi>0 is that fraction of Ai who move into the Ri class.
(H6) The n-square contact matrix B(n)=(βij)n×n is irreducible [41], where βij≥0.
Remark 1: Assumption (H6) implies that every pair of groups is joined by an infectious path so that the presence of a heavy drinking individual in the first group can cause "infection" in the second group.
The other parameters description of the model are presented in Table 1.
Here, all the parameters are positive. Furthermore, the following assumptions are made.
(H7) We assume that pij(θ),qij(θ),mi(θ)∈L1+(0,+∞) are nonnegative and essential bounded, and they are all non-decreasing functions of the age of alcoholism θ. If θ≥θ0, then pij(θ)≡pmax, qij(θ)≡qmax, and mi(θ)≡mmax, where 0<θ0≤θ+.
Because the effect of public health education, here the infectivity qij(θ) is reduced relatively for educated individuals, i.e., qij(θ)≤pij(θ) (the smaller, the better). For simplicity, we set Pij=∫∞0pij(θ)aj(t,θ)dθ, Qij=∫∞0qij(θ)aj(t,θ)dθ, and [μiAi] stands for ∫∞0μiai(t,θ)dθ. Under assumptions (H1)-(H6), the alcoholism transmission diagram is depicted in Figure 1.
The transfer diagram leads to the following multi-group age structured SELAR alcoholism model with public health education:
The initial conditions and boundary conditions of model (2.1) are respectively given by:
where L1+(0,+∞) is the space of functions that are nonnegative and Lebesgue integrable.
Remark 2: Neglecting the heterogeneity and alcoholism age, Xiang et al. [36] constructed a SEARQ drinking model with public health educational campaigns, its threshold stability was achieved.
3.
Preliminaries
In this section, some primary results are presented for establishing our main conclusions.
3.1. Volterra formulation
Note that the variables Ri(t) does not appear in the first four equations of (2.1), it suffices to study the dynamical behaviors of the following reduced sub-system (3.1):
Let
then Γ0i(θ) is the probability of an alcoholism individual in the i-th group surviving to alcoholism-age θ. Using the approach introduced by Webb [53], integrating along the characteristic lines and incorporating the boundary conditions, we can obtain that
Substituting (3.3) into the equations for S′i(t),E′i(t) and L′i(t) of system (3.1), we have
where
It is easy to check that limt⟶∞F1(t)=0,limt⟶∞F2(t)=0. Furthermore, set
Obviously, hi(θ)≥gi(θ),i=1,2,⋯,n. Then, by application of the results in [54], any equilibrium of system (3.4) (if it exists), must be a solution of the following limiting system associated with (3.4):
The behavior of system (3.1) is equivalent to the system (3.5). Once the solution of system (3.5) is determined, we can obtain ai(t,θ) from (3.3). So that the stability of the equilibrium of system (2.1) is the same as that of system (3.5). In this paper, we focus on the system (3.5).
3.2. State space
For system (3.5), we need the appropriate fading memory space of continuous functions as follows (see Atkinson et al. [52]):
which is a Banach space endowed with the norm ∥ϕ∥λi:=sups≤0∣ϕ(s)∣eλis<+∞, where R+=[0,+∞], λi is a positive constant. Let ϕit(s)∈Cλi be such that ϕit(s)=ϕi(t+s),s∈(−∞,0]. Thus, we consider the system (3.5) in the phase space
For system (3.5), the existence and uniqueness of the solution with the initial and boundary conditions (2.2) can be checked by the standard approaches in [53]. Furthermore, we clams that any solution of system (3.5) with nonnegative initial conditions remains nonnegative.
We define a continuous solution semi-flow Φ:R+×X⟶X associated with (3.5):
Let
where ηi=min{dSi,dEi,dLi,dAi,dRi}. And denote the interior of Ω as:
Lemma 3.1. For system (3.5), Ω is positively invariant for Φ, i.e., Φ(t,x0)∈Ω,∀x0∈Ω,t≥0.
Proof. First, we prove that all solutions of system (3.5) with the initial and boundary conditions (2.2) remains nonnegative. By continuity of the solutions of system (3.5) and Si(0)=ϕi1≥0, we claim Si(t)≥0 for all t≥0,i=1,2,⋯,n. In fact, we assume that there exists a i1∈{1,2,,⋯,n} such that Si1(t) lost its positivity for the first time at t1>0, i.e., Si1(t1)=0. However, from the first equation of (3.5), we can see dSi1(t1)dt=Λi1>0 which is a contradiction to the fact that Si1(t)>Si1(t1)=0 for any 0≤t<t1.
Similarly, we shall show that Ei(t)≥0 for all t≥0,i=1,2,⋯,n. Otherwise, we assume that there exists a i2∈{1,2,,⋯,n} and the first time t2>0 such that Ei2(t2)=0. However, from the second equation of (3.5) one obtains dEi2(t2)dt=ξi2Si2(t2)>0 which follows from the nonnegativity of Si(t) for all t≥0,i=1,2,⋯,n. Thus, Ei(t)≥0 for all t≥0,i=1,2,⋯,n.
Furthermore, we conclude that there is a i3∈{1,2,,⋯,n} and the first time t3>0 such that Li3(t3)=0. Note that 0<t3−θ<t3, thus, Li3(t3−θ)>0. Using the similar arguments and the third equation of (3.5), we gets dLi3(t3)dt>0. This is a contradiction which implies the nonnegativity of Li(t) for all t≥0,i=1,2,⋯,n.
Second, we prove that all solutions of system (3.5) with the initial and boundary conditions (2.2) remains bounded. The total population size is N(t)=∑ni=1Ni(t). In the i-th group, Ni(t)=Si(t)+Ei(t)+Li(t)+∫∞0ai(t,θ)dθ+Ri(t). Let ηi=min{dSi,dEi,dLi,dAi,dRi}, then
this suggesting
Then, we have
Therefore, we can obtain that Ω is positively invariant for Φ, i.e., Φ(t,x0)∈Ω,∀x0∈Ω,t≥0. This proof is completed.
Our results in this paper will be stated for system (3.5) in Ω, and can be translated straightforwardly to system (3.1). Moreover, all positive semi-orbits in Ω have compact closure in X (see [52]), and thus have non-empty ω-limit sets. We have the following result.
Lemma 3.2. All positive semi-orbits in Ω have non-empty ω-limit sets.
Lemma 3.3. System (3.5) is point dissipative, that is, there exists constants Mi>0 such that for each solution of (3.5) there is a Ti>0 such that ∥Sit∥λi≤Mi,∥Eit∥λi≤Mi and ∥Lit∥λi≤Mi for all t≥Ti,i=1,2,⋯,n.
Proof. Let Wi=maxt∈[0,Ti]Si(t)>0, then, for any t≥Ti and any εi>0, we get
where the last estimation was obtained by three separations to u≤0,0≤u≤Ti and Ti≤u≤t. Hence, we can choose MSi>0 such that ∥Sit∥λi≤MSi. Similary, we can also prove that ∥Eit∥λi≤MEi and ∥Lit∥λi≤MLi. Set Mi=max{MSi,MEi,MLi}>0, this proof is completed for all t≥Ti,i=1,2,⋯,n.
3.3. Equilibria
System (3.5) always has a alcohol-free equilibrium P0=(S01,E01,0,...,S0n,E0n,0)∈R3n+, where
Let
The alcohol-present equilibrium of system (3.5) is given by
then it is determined by the following system of equations
The basic reproduction number R0 (see [38,55,56]) is defined as the spectral radius of a matrix M0, that is,
It acts as a threshold as is shown in the following result. We shall establish that the dynamical behaviors of system (3.5) are completely determined by values of R0.
4.
Main results
In this section we state and prove our main results concerning the global dynamics of system (3.5).
4.1. Global stability of P0
Theorem 4.1. Assume that the matrix B(n)=(βij)n×n is irreducible.
(1) If R0≤1, then the unique alcohol-free equilibrium P0 of system (3.5) is globally asymptotically stable in Ω;
(2) If R0>1, then P0 is unstable and system (3.5) is uniformly persistent, i.e., there exists a positive constant c>0 such that lim supt⟶∞Si(t)≥c,lim supt⟶∞Ei(t)≥c,lim supt⟶∞Li(t)≥c,i=1,2,…,n. Furthermore, system (3.5) has at least one alcohol-present equilibrium P∗ in Ωo.
Proof. (1) Let S=(S1,S2,...,Sn)T,E=(E1,E2,...,En)T, we define a matrix-valued function
It is easy to see that M(S0,E0)=M0, where S0=(S01,S02,...,S0n)T, E0=(E01,E02,...,E0n)T. We also have
First, we will prove the uniqueness of alcohol-free equilibrium in Ω. If R0≤1, (S,E)≠(S0,E0), then we obtain
Since B(n)=(βij)n×n is irreducible, we know nonnegative matrix M(S,E) and M0 are also irreducible. Using the Perron-Frobenius theorem (see Theorem 2.1.4 or Corollary 2.1.5 in [58]), we get
Therefore, ρ(M(S,E))<1 holds when R0=ρ(M0)≤1 and (S,E)≠(S0,E0). This implies that the vector equation M(S,E)L=L has only the trivial solution L=0, where L=(L1,L2,...,Ln)T. Thus P0 is the unique equilibrium of system (3.5) in Ω if R0≤1.
Next, we claim that the alcohol-free equilibrium P0 is globally asymptotically stable in Ω. Since M0 is irreducible, there exists a positive left eigenvector ω of M0 corresponding to ρ(M0), i.e.,
where ω=(ω1,ω2,...,ωn) and ωi>0,i=1,2,...,n. We introduce the Volterra-type function φ(x)=x−1−lnx,x>0, which is positive definite and attains its global minimum φ(1)=0 at x=1. Note that ω is a strictly positive left eigenvector. We define a Lyapunov functional Lafe(t):(Si,Ei,Li)→R as
which is nonnegative and continuously differentiable. Here
where ci=ωidLi+γi, mj(θ)=∫∞θhj(s)ds and nj(θ)=∫∞θgj(s)ds. Obviously, mj(0)=∫∞0hj(s)ds=Bj,dmj(θ)dθ=−hj(θ), and nj(0)=∫∞0gj(s)ds=Cj,dnj(θ)dθ=−gj(θ).
It follows from Lemmas 3.1 and 3.3 that Lafe(t) is nonnegative and bounded for big enough positive t>0. It is clear that Lafe(t) with the equality holds if and only if Si=S0i,Ei=E0i,Li=0. First, calculating the time derivative of H1(t) along with the solutions of system (3.5), using the equation Λi=(dSi+ξi)S0i, we have
Second, calculating the time derivative of H2(t) along with the solutions of system (3.5). Let t−θ=s, then −∞<s<t, we obtain
Similar to H′2(t), we get derivative of H3(t) along with the solutions of system (3.5). Let t−θ=s, then −∞<s<t, we obtain
Using (4.1)-(4.3), we have
If R0=ρ(M0)<1, then (4.4) implies L=0, and dLafe(t)dt=0 if and only if L=0.
If R0=ρ(M0)=1, from (4.4), we see dLafe(t)dt=0 implies (ω1,ω2,…,ωn)⋅(M0L−L)=0, that is
where In denote the n-dimensional identity matrix. Hence, if (S,E)≠(S0,E0), then we have
so the solution of (4.5) is only the trivial value L=0. Summarizing the above statements, we see that dLafe(t)dt=0 implies that either L=0 or R0=1 and (S,E)=(S0,E0). It can be verified that the largest compact invariant subset of {(S1,E1,L1,...,Sn,En,Ln)∈Ω:dLafe(t)dt=0} is the singleton {P0}. Therefore, by Lemma 3.2 and the Lyapunov-LaSalle invariance principle (see Theorem 3.4.7 in [59]), we can see that P0 is globally attractive in Ω if R0≤1. The local asymptotical stability of the disease-free equilibrium P0 comes from the relationship between the eigenvalues of the linearized matrix and R0, which can be proved using the same proof as one for Corollary 5.3.1 in [60] (The detailed process is omitted here). Then, together with the local asymptotical stability of P0, we know that the alcohol-free equilibrium P0 is globally asymptotically stable in Ω when R0≤1.
(2) Suppose that R0=ρ(M0)>1, and L≠0. By continuity and (4.4), we have
which implies that dLafe(t)dt>0 in a sufficiently small neighborhood of the alcohol-free equilibrium P0 in Ω0. This implies that the alcohol-free equilibrium P0 is unstable when R0>1.
Next, with a uniform persistence result in [61] and a similar argument as in the proof of Proposition 3.3 of [62], we can show that the instability of P0 implies that system (3.5) is uniformly persistent when R0>1, i.e., there exists a positive constant c>0 such that
provided (S1(0),E1(0),L1(0),...,Sn(0),En(0),Ln(0))∈Ω0.
Furthermore, using the uniform persistence of system (3.5), together with the uniform boundedness of solutions, we shall establish the existence of the alcohol-present equilibrium P∗ (see Theorem 2.8.6 in [63] or Theorem D.3 in [64]). This completes the proof of theorem 4.1.
4.2. Global stability of P∗
In following section, we devote to the alcohol-present equilibrium of system (3.5) is globally asymptotically stable. In order to prove our results, we will construct the proper Lyapunov functional and apply subtle grouping technique in estimating the derivatives of Lyapunov functional guided by graph theory, which was recently developed by Guo et al. in [38,39] and Li et al. [40]. Here, we quote some results from graph theory which will be used in the proof of our main results. We refer the reader to [38,39,40] and the references cited therein for more details of these concepts and results.
Given a non-negative matrix A=(aij)n×n, the directed graph G(A) associated with A has vertices {1, 2, ..., n} with a directed arc (i,j) from i to j if and only if aij≠0. It is strongly connected if any two distinct vertices are joined by an oriented path. Matrix A is irreducible if and only if G(A) is strongly connected.
A tree is a connected graph with no cycles. A subtree T of a graph G is said to be spanning if T contains all the vertices of G. A directedtree is a tree in which each edge has been replaced by an arc directed one way or the other. A directed tree is said to be rooted at a vertex, called the root, if every arc is oriented in the direction towards to the root. An orientedcycle in a directed graph is a simple closed oriented path. A unicyclicgraph is a directed graph consisting of a collection of disjoint rooted directed trees whose root are on an oriented cycle.
For a given nonnegative matrix (ˉβij)n×n, ˉβij≥0,1≤i,j≤n. Let
be the Laplacian matrix of the directed graph (ˉβij)n×n, and Cij denote the cofactor of the (i,j) entry of ˉB. For the linear system
the following results hold (see Lemma 2.1 in [38] and Theorem 2.2 in [40]).
Lemma 4.1 ([38]) Assume that (ˉβij)n×n is irreducible and n≥2. Then following results hold:
(1) The solution space of system (4.7) has dimension 1;
(2) A basis of the solution space is given by
where Cii denotes the cofactor of the k-th diagonal entry of ˉB, 1≤i≤n;
(3) For all 1≤i≤n,
where Ti is the set of all directed spanning subtrees of G(ˉB) that are rooted at the i−th vertex, w(T) is the weight of a directed tree T, and E(T) denotes the set of directed arcs in a directed tree T;
(4) For all 1≤i≤n,
Lemma 4.2. ([40]) Assume that vi is the same meaning in Lemma 4.1, and n≥2, then
where Fij(xi,yj),1≤i,j≤n is an arbitrary function, T is the set of all spanning unicyclic graphs of G(ˉB), w(T) is the weight of a directed tree T, CT denotes the oriented cycle in a unicyclic graph T, and E(CT) denotes the set of directed arcs in CT.
Let P∗=(S∗1,E∗1,L∗1,...,S∗n,E∗n,L∗n) be the alcohol-present equilibrium of system (3.5), then S∗i,E∗i,L∗i(i=1,2,...,n) are determined by the following equations
In the following section, we prove that the alcohol-present equilibrium P∗ is globally asymptotically stable when R0>1. In particular, this proof implies that the endemic equilibrium is unique in the region Ω0 when it exists. Therefore, we have the following main result on the uniqueness and global stability of the positive equilibrium P∗ when R0>1.
Theorem 4.2. Assume that B(n)=(βij)n×n is irreducible. If R0>1, then the alcohol-present equilibrium P∗ of system (3.5) is globally asymptotically stable in Ω0 and thus is the unique positive equilibrium.
Proof. Case Ⅰ: n=1
The multi-group system (3.5) reduces to a single-group system as follows
where h(θ)=γp(θ)Γ0(θ),∫∞0h(θ)dθ=B and g(θ)=γq(θ)Γ0(θ),∫∞0g(θ)dθ=C. The alcohol-present equilibrium P∗ of system (4.9) satisfies the following equations
Let (S(t),E(t),L(t)) be any solution of system (4.9) with non-negative initial data, we construct a Lyapunov functional Vape(t):(S,E,L)→R as follows
which is nonnegative and continuously differentiable. Here we define
where φ(x)=x−1−lnx, m(θ)=∫∞θh(s)ds,m(0)=B,dm(θ)dθ=−h(θ), and n(θ)=∫∞θg(s)ds,n(0)=C,dn(θ)dθ=−g(θ). It is clear that Vape(t) is bounded for all t≥0, and Vape(t)≥0 with the equality holds if and only if S(t)=S∗,E(t)=E∗,L(t)=L(t−θ)=L∗.
Using the equations in (4.9) and Λ=(dS+ξ)S∗+βS∗L∗B, and differentiating V1(t) along system (4.8), we have
Further, let s=t−θ and base on fact that dm(θ)dθ=−h(θ), we obtain
Similarly, base on fact that dn(θ)dθ=−g(θ), we have
Combining (4.10)-(4.13), we get
From (4.14), we conclude that dVape(t)dt≤0 and with the equality holds if and only if S(t)=S∗,E(t)=E∗,L(t)=L(t−θ)=L∗ for all t≥0. Thus, the largest invariant set {dVape(t)dt=0}={P∗}. Therefore, using the LaSalle's Invariance Principle, we get the alcohol-present equilibrium P∗ is globally attractive in Ω0 if R0>1 for n=1.
Case Ⅱ: n≥2
In the following, we are going to consider the case n≥2. Let
and matrix ˉB as given in (4.6). Since B(n)=(βij)n×n is irreducible, we know the matrix ˉB is also irreducible. Let v={v1,…,vn},vi>0 be a basis for the solution space of linear system (4.7), i.e., ˉBv=0 as described in Lemma 4.1.
Let (Si(t),Ei(t),Li(t))(1≤i≤n) be any solution of system (3.5) with non-negative initial data. For such v={v1,…,vn}, we define a Volterra-type Lyapunov functional Uape(t):(Si,Ei,Li)→R as follows
which is nonnegative and continuously differentiable, where
where mj(θ)=∫∞θhj(s)ds,mj(0)=Bj,dmj(θ)dθ=−hj(θ), and nj(θ)=∫∞θgj(s)ds,nj(0)=Cj,dnj(θ)dθ=−gj(θ).
By the definition of the fading memory space, Lemmas 3.2 and 3.3, we known that Uape(t) is well-defined, that is, Uape(t) is bounded for all t≥0. It is clear that Uape(t)≥0 with the equality holds if and only if Si(t)=S∗i,Ei(t)=E∗i,Li(t−θ)=Li(t)=L∗i. Differentiating U1(t),U2(t),U3(t) along the solutions of system (3.5), and using the equilibrium equations (4.8), we get
Further, let s=t−θ and base on fact that dmj(θ)dθ=−hj(θ), we obtain
Similarly, base on fact that dnj(θ)dθ=−gj(θ), we have
Combining (4.15)-(4.17), we get
Using φ(x)=x−1−lnx and ˉβij=βijL∗j(S∗iBj+E∗iCj), we rearrange the terms in (4.18) as follows
Now, we are going to show that dUape(t)dt≤0. From the properties of function φ(x), it is easy to see that we only need to consider the last two items in (4.19). In what follows, we first prove that the penultimate item
In fact, from (4.7) ˉBv=0, we get
Note that ˉβij=βijL∗j(S∗iBj+E∗iCj), we obtain
Using this equation, we can obtain
Therefore, we have
holds for all L1,L2,⋯,Ln>0.
Next, we will show that the last item is also equal to zero. Let
Then, we will find that
holds for all L1,L2,⋯,Ln>0.
In fact, by the Kirchhoff's Matrix-Tree Theorem in Lemmas 4.1 and 4.2 (see [38]-[40]), we known that vi=Cii is a sum of weights of all directed spanning subtrees T of G that are rooted at vertex i. So, each term viˉβij is the weight w(Q) of a unicyclic subetaaph Q of G, obtained from such a tree T by adding a directed arc (i,j) from the root i to vertex j. Thus, the meaning of double sum in (4.22) can be considered as a sum over all unicyclic subetaaphs Q containing vertices {1,2,…,n}, that is
For each unicyclic subetaaph Q, we can see that
which implies that
For example, we set n=2, the unique cycle CQ has two vertices with the cycle 1→2→1, and E(CQ)={(1,2),(2,1)}. Then we can get v1=ˉβ21, v2=ˉβ12, and
So, we have Wn≡0 holds for all L1,L2,⋯,Ln>0.
Therefore, combining with (4.19)-(4.20), (4.22) and using properties of φ(x), we have dUape(t)dt≤0 for all (S1,E1,A1,…,Sn,En,An)∈Ω0, and with the equality holds if and only if Si(t)=S∗i,Ei(t)=E∗i,Li(t)=Li(t−θ)=L∗i for all t≥0, θ∈[0,θ+], i=1,…,n. We conclude that the largest invariant set {dUape(t)dt=0}={P∗}. The ω-limit set of Ω0 consist of just the alcohol-present equilibrium P∗. Therefore, using the LaSalle's Invariance Principle, we see that the alcohol-present equilibrium P∗ is globally attractive in Ω0 if R0>1 for n≥2.
As for the local asymptotical stability of the alcohol-present equilibrium P∗, which can be proved by the way of Corollary 5.3.1 in [60]. Therefore, using an argument similar to that in the proof of Theorem 4.1 (1), the alcohol-present equilibrium P∗ is globally asymptotically stable in Ω0 if R0>1, which consequently implies that the alcohol-present equilibrium P∗ is unique. The proof of Theorem 4.2 is completed.
5.
Education and alcoholism age effects
In this section, we will discuss the effects of the public health education and alcoholism age on the alcohol control. It follows from Theorems 4.1 and 4.2 that the global dynamics of system (3.5) are completely determined by the basic reproduction number
where S0i=ΛidSi+ξi, E0i=ξiΛi(dSi+ξi)(dEi+αi), and Bj=∫∞0hj(θ)dθ, Cj=∫∞0gj(θ)dθ.
For convenience, we assume that the natural death rate of susceptible population (including uneducated and educated susceptible population) is the same, that is, dSi=dEi. To investigate the effect of the public health education, we consider the special case with ξi=0, which is the rate of the susceptible population who accepted the public health education entering into the educated class. Then for ξi=0, we have ^E0i=0 and ^S0i=ΛidSi. Note that ΛidSi−ΛidSi+ξi=ξiΛidSi(dSi+ξi)>ξiΛi(dSi+ξi)(dEi+αi) and Bj≥Cj, we can obtain that
This implies that public health education leads to the basic reproduction number decline. On the other hand, the reproduction number R0 is an increasing function of transmission coefficient βij. By increasing public health educational campaigns at all social levels, the value of ξi will be increase. Hence, the awareness about drinking will alert the susceptible individuals so that they isolate themselves and decline to drink or drink moderately. This leads to a decrease in the value of βij. In this case, the value of the reproduction number R0 will be decrease. Considering both the cost and the practical purposes, efforts to increase public health education are more effective in controlling the spread of alcohol problems than efforts to increase the number of individuals who have access to treatment. Therefore, public health education is one of the effective measures to control the spread of alcohol problems and it is beneficial for alcoholism control in the whole society.
However, R0 is a decreasing function of γi which is the rate of the light drinkers enter into the alcoholism compartment. From the assumption (H7), alcoholism age θ will cause the value of the reproduction number R0 increase, which is bad for alcoholism control. It suggests that the longer the light drinkers stay in their compartment, the better alcohol problems will be controlled.
6.
Discussion
The goal of this paper is to analysis threshold dynamics of a multi-group alcoholism epidemic model with public health education and alcoholism age in heterogeneous populations. Our results expands the previous related works which have been obtained in single-group models without alcoholism age. Mathematical analysis shown that the global asymptotic behavior of multi-group alcoholism model is completely determined by the size of the basic reproduction number R0. By using the theory of non-negative matrices and the classical method of Lyapunov functional, Theorem 4.1 implies that the alcohol problems dies out in the sense that alcoholism fractions go to zero from all the groups if R0≤1. By applications of the graph-theoretic approach to the method of Lyapunov functionals, we proved the existence, uniqueness and global asymptotic stability of the alcohol-present equilibrium P∗ for R0>1, see Theorem 4.2. Our results implies that the alcoholism will persist in all the groups of the population and will eventually settle at a constant level in each group.
Our main results indicate that the dynamics of alcoholism model (2.1) is similar to that for the models without considering multi-group and alcoholism age. That is, heterogeneity of populations does not alter the dynamical behaviors as shown in [36]. However, our model is more realistic than the corresponding models already established. Because by decomposing the heterogeneous population into several subetaoups, the effects of both the intra-group and inter-group are considered. Moreover, inclusion of alcoholism population with alcoholism age leads to the basic reproduction number R0 is increase. So the heterogeneity of populations plays an important role on R0, it affects the global dynamics of the model. Strengthening public health education and controlling the age of drinking have a positive role in alcoholism control. In addition, there is an innovation in the method of study in this paper. Our findings in this paper may be valuable for the health workers who are performing alcoholism control. These results are provided with intention to inform and assist policy-makers in targeting education and treatment resources for maximum effectiveness.
Of course, other factors, such as time lag for alcoholism or nonlinear transmission rate, taking into account the model which will make the model more realistic. In addition, relapse is very common when it comes to drinking. Research on these issues remains to be completed in the future.
Acknowledgments
This research is supported by the NNSF of China (11461041 and 11661050), the NSF of Gansu Province (148RJZA024 and 061706), and the Development Program for HongLiu Outstanding Young Teachers in Lanzhou University of Technology. We are grateful to the anonymous referees and the editors for their valuable comments and suggestions which improved the quality of the paper.
Conflict of interest
The authors declare that there is no conflict of interests regarding the publication of this paper.









 DownLoad:
DownLoad: