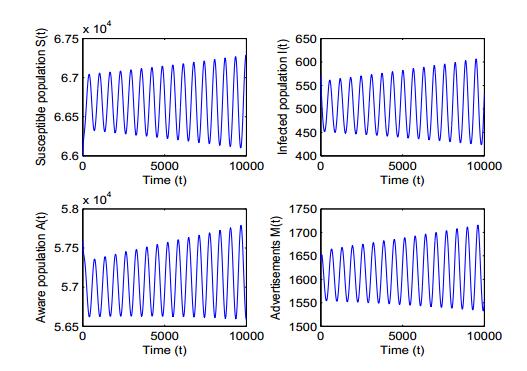
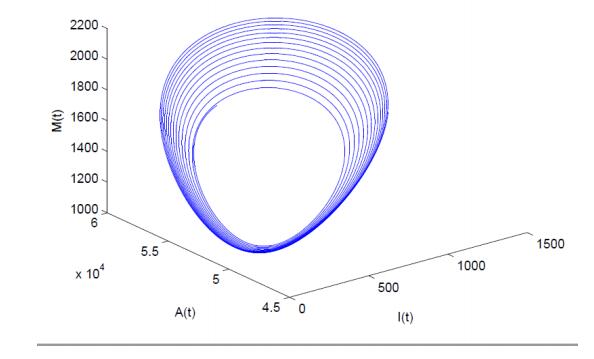
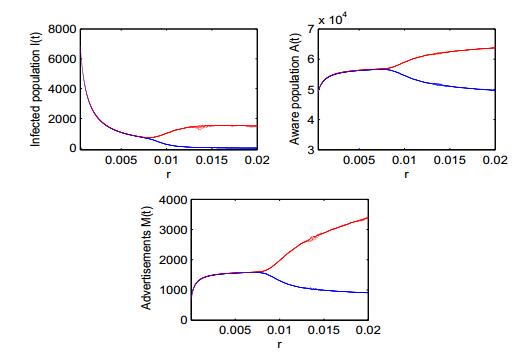
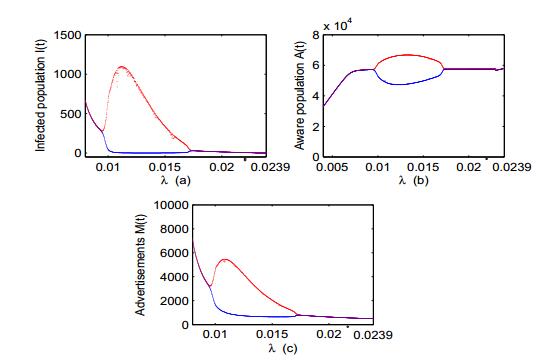
Public health information through media plays an important role to curb the spread of various infectious diseases as most of the populations rely on what media projects to them. Social media and TV advertisements are important mediums to communicate people regarding the spread of any infectious disease and methods to prevent its spread. Therefore, in this paper, we propose a mathematical model to see how TV and social media advertisements impact the dynamics of an infectious disease. The susceptible population is assumed vulnerable to infection as well as information (through TV and social media ads). It is also assumed that the growth rate of TV and social media ads is proportional to the number of infected individuals with decreasing function of aware individuals. The feasibility of possible equilibria and their stability properties are discussed. It is shown that the increment in growth rate of TV and social media ads destabilizes the system and periodic oscillations arise through Hopf-bifurcation. It is also found that the increase in dissemination rate of awareness among susceptible population also gives rise interesting dynamics about the stability of endemic equilibrium and causes stability switch. It is observed that TV and social media advertisements regarding the spread of infectious diseases have the potential to bring behavioral changes among the people and control the spread of diseases. Numerical simulations also support analytical findings.
1.
Introduction
Population dynamics has always been an important research object of biomathematics. Various groups often have complex interspecific relationships, such as predation, competition, parasitism and mutualism [1]. Predation behavior, as a widespread interspecies relationship, has been widely studied. Lotka and Volterra were the first to propose a predator-prey system to describe the widespread interspecies relationship of predation [2]. Holling further proposed three functional responses of predators to describe the energy transfer between predators and prey [3]. These three functional responses have been applied and perfected by many scientists [4]. In 1991, Hastings and Powell proposed a food chain system with chaotic dynamics and studied the dynamics of the model [5]. In recent years, many mathematicians have also studied the development and improvement of Hastings-Powell food chain models [6,7,8,9].
Fractional calculus is a generalization of traditional calculus, and its order can be composed of integers, fractions or complex numbers[10]. Fractional calculus can better describe some systems or processes with memory and hereditary properties, and it has been widely used in many fields, such as physics, secure communication, system control, neural networks, and chaos[11,12]. The method of solving the fractional model has also been widely studied[13,14]. In [15], the Caputo fractional derivative operator is used instead of the integer first derivative to establish an effective numerical method for solving the dynamics of the reaction-diffusion model based on a new implicit finite difference scheme. In [16], a numerical approximation for the Caputo-Fabrizio derivative is used to study the dynamic complexity of a predator-prey system with a Holling-type functional response. In [17], a new fractional chaotic system described by the Caputo fractional derivative is presented, and how to use the bifurcation diagram of this chaotic system to detect chaotic regions is analyzed. In [18], the generalization of Lyapunov's direct method applying Bihari's and Bellman-Gronwall's inequalities to Caputo-type fractional-order nonlinear systems is proposed. In [19], the Fourier spectral method is introduced to explore the dynamic richness of two-dimensional and three-dimensional fractional reaction-diffusion equations. In [20], the spatial pattern formation of the predator-prey model with different functional responses was studied. [21] studied the numerical solution of the space-time fractional reaction-diffusion problem that simulates the dynamic and complex phenomena of abnormal diffusion.
Since most biological mathematical models have long-term memory, fractional differential equations can more accurately and reliably describe the actual dynamic process [1,22]. [23] proposed fractional predator-prey models and fractional rabies models and studied their equilibrium points, stability and numerical solutions. In [24], the authors studied the stability of a fractional-order system by the Lyapunov direct method, which substantially developed techniques to study the stability of fractional-order population models. In [9], the authors extended the Hastings-Powell food chain system to fractional order and analyzed its dynamic behavior.
As an important research object of biological mathematics and control theory, population model control has received extensive research and development in recent years [25,26,27]. In [28], the authors conducted random detection and contacted tracking on the HIV/AIDS epidemic model and used the Adams-type predictor-corrector method to perform fractional optimal control of the model, which significantly reduced the number of AIDS patients and HIV-infected patients. In [29], the authors applied the time-delay feedback controller to the fractional-order competitive Internet model to solve the bifurcation control problem of this model. In [30], the author considered the influence of additional predators on the Hastings-Powell food chain model and studied the control of chaos in this model.
Biological models are widely studied by scientists, but many classic models study food chain models composed of herbivores and carnivores, and omnivores are rarely considered. In fact, omnivores are widespread in nature and play an important role in the food chain. In this article, the existence of omnivores is fully considered, and a food chain model in which herbivores, omnivores and carnivores coexist is studied. Based on these works, this paper proposes a fractional food chain model with a Holling type-II functional response. The main contributions of this paper are as follows. First, this paper proves the existence and uniqueness of the solution and the nonnegativity and boundedness of the solution. Second, the equilibrium point of the model is calculated, and the local stability of the equilibrium point is proven. Third, a controller is designed to prove the global asymptotic stability of the system by using the Lyapunov method.
This paper is organized as follows. In Section 2, the definitions and lemmas are given, and the food chain model is established. In Section 3, the existence, uniqueness, nonnegativity and boundedness are proven, and the local stability of the equilibrium point of the model is studied. The global stability of the model is studied through the controller. In Section 4, numerical simulations are performed to verify the theoretical results. The conclusion of this article is given in Section 5.
2.
Model description and preliminaries
In this section, some basic knowledge about fractional equations and the theorems and lemmas used in this paper are given, and the fractional food chain system is introduced.
Definition 1. [10]. The Caputo fractional derivative of order α of a function f, R+→R, is defined by
where Γ(⋅) is the Gamma function. When 0<α<1,
Definition 2. [31]. When the order a>0, for a function f:(0,∞)→R, the Riemann-Liouville representation of the fractional integral operator is defined by
where a>0 and Γ(⋅) is the Gamma function.
Lemma 1. (Generalized Gronwall inequality) [32]. Assume that m≥0, γ>0, and a(t) are nonnegative, locally integrable, and nondecreasing functions defined on 0≤t≤T(T≤∞). In addition, h(t) is a nonnegative, locally integrable function defined in 0≤t≤T and satisfies
then,
where the Mittag-Leffler function Eγ(z)=∞∑k=0zkΓ(kγ+1).
Lemma 2. [10]. Consider the fractional-order system
where f(t,x(t)) defined in R+×Rn→Rn and α∈(0,1].
The local asymptotic stability of the equilibrium point of this system can be derived from |arg(λi)|>απ2, where λi are the eigenvalues of the Jacobian matrix at the equilibrium points.
Lemma 3. [24]. Consider the system
where α∈(0,1], f:[t0,∞)×Ω→Rn, and Ω∈Rn; if f(t,x) satisfies the local Lipschitz condition about x on [t0,∞)×Ω, then there exists a unique solution of (2.2).
Lemma 4. [33]. The function x(t)∈R+ is continuous and derivable; then, for any t≥t0
There are a variety of complex biological relationships in nature. Predation is the most important biological relationship, and it has received attention from and been studied by many scientists. In [5], the author proposed a three-species food chain model. The model consists of one prey ˆX and two predators ˆY and ˆZ. The top predator ˆZ feeds on the secondary predator ˆY, and the secondary predator ˆY feeds on the prey ˆX. This is the famous Hastings-Powell model:
where ˆR and ˆK represent the intrinsic growth rates and environmental carrying capacity, respectively. For i=1,2, parameters^Ai, ^Bi, ^Ci and ^Di are the predation coefficients, half-saturation constant, food conversion coefficients and death rates.
The Hastings-Powell model considers a food chain composed of herbivores, small carnivores and large carnivores but does not consider the existence of omnivores. We consider a food chain consisting of small herbivores X, medium omnivores Y and large carnivores Z. Among them, omnivores Y prey on herbivores X, and carnivores Z prey on omnivores Y. They all respond according to Holling II type. This system can be expressed mathematically as
where X, Y and Z represent the densities of the prey population, primary predator population and top-predator population, respectively. For i=1,2, parameters Ri, Ki, Ai, Bi and Ci are the intrinsic growth rates, environmental carrying capacity, predation coefficients, half-saturation constant and food conversion coefficients, respectively. The parameter D is the death rates for Z.
Then, we obtain the following dimensionless version of the food chain model:
the independent variables x, y and z are dimensionless population variables; t represents a dimensionless time variable; and ai, bi(i=1,2) and d are positive.
Research results show that using fractional derivatives to model real-life biological problems is more accurate than classical derivatives[15]. To better analyze the dynamics between these three populations, we studied the following fractional-order Hastings-Powell System food chain model:
where α∈(0,1) is the fractional order.
3.
Main results
3.1. Properties of the solution
Theorem 1. The fractional-order Hastings-Powell System food chain model (2.6) has a unique solution.
Proof: We will study the existence and uniqueness of the system (2.6) in [0,T]×Ω, where Ω={(x,y,z)∈R3:0≤x,y,z≤H}. Let S=(x,y,z), ˉS=(ˉx,ˉy,ˉz), F(S)=(F1(S),F2(S),F3(S)) and
For any S,ˉS∈Ω, it follows from (3.1) that
where L=max{r1+r1MK1+M,r2+r2MK2+2M+b1M2,d+M+b2M2}, Based on Lemma 3, F(S) satisfies the Lipschitz condition with respect to S in Ω. According to the Banach fixed point theorem in [34], system (2.6) has a unique solution in Ω.
Theorem 2. Set A={x,y,z)∈R3:0<x+y+z<K1(r1+v)24r1v+K2(r2+v)24r2v} as a positively invariant set of system (2.6), and the solutions are bounded.
Proof: let g(t)≜g(x(t),y(t),z(t))=x(t)+y(t)+z(t),
let
According to the positive knowledge of all parameters and the nonnegativity of the solutions,
we obtain
Since Eα(−vtα)≥0, when g(0)≤uv, limt→∞supg(t)≤uv. According to the nonnegativity of the system (2.6), g(t)≥0(∀t≥0); hence, A={x,y,z)∈R3:0<x+y+z<K1(r1+v)24r1v+K2(r2+v)24r2v} is a positively invariant set of system (2.6), and the solutions are bounded.
3.2. Equilibria and their stability
Let
Then, the equilibrium points are E0=(0,0,0), E1=(K1,0,0), E2=(0,K2,0) and E∗=(x∗,y∗,z∗), where
For system (2.6), the Jacobian matrix at the equilibrium point (x′,y′,z′) is
where e1=a2−b2d, e2=b2de1+1, e3=1+b1x′, e4=2r1x′K1+a1de1e23, e5=a1x′e3.
Theorem 3.3. For system (2.6), the equilibrium points E0 and E1 are saddle points.
Proof: The Jacobian matrices evaluated at E0 and E1 are
According to Lemma 2, when the eigenvalues are all real numbers and all negative, the equilibrium points are locally asymptotically stable.
The eigenvalues of J(E0) are λ01=r1, λ02=r2 and λ03=−d. The eigenvalues of J(E1) are λ11=−r1, λ12=r2+a1K1b1K1+1 and λ13=−d.
Then, we have λ01,λ02>0, λ03,λ11<0, and λ13<0, so the equilibrium points E0 and E1 are saddle points.
Theorem 4. For system (2.6), if r1<a1K2 and a2K2(b2K2+1)<d, then the equilibrium point E2=(0,K2,0) is locally asymptotically stable.
Proof: The Jacobian matrix evaluated at E2 is
The eigenvalues of J(E2) are λ1=r1−a1K2, λ2=−r2 and λ3=a2K2b2K2+1−d.
Therefore, if r1<a1K2 and a2K2(b2K2+1)<d, the equilibrium point E2 is locally asymptotically stable.
The characteristic equation of equilibrium points E∗=(x∗,y∗,z∗) is given as
where
A=2f4−2r1−e5+f2z∗+f1de23+f5−f2b2dz∗e1e2,
B=(B1+B2),
B1=e4e5+e5r1+r21−2r1f4−f2r1z∗−2e5f4+e21dz∗a2−f6e5−f6r1−2r1f5,
B2=2e23f1f5+2f2f4z∗+a1f3e23z∗+4dr2f4e1k1+r1f7−f1f7de3−2f4f7,
C=dz∗(a1dK1e23−e1K1r1+2e1r1x∗)f1K1,
where f1=a1e1, f2=a2e2, f3=f2de1, f4=r1x∗K1, f5=r2de1K2, f6=a1de23e1, f7=b2f3z∗e2.
For Eq. (3.2), define the discriminant as
With reference to the results of [35] and [36], we obtain the following fractional Routh-Hurwitz conditions:
1. If D(P)>0, A>0, C>0, and AB−C>0, E∗ is locally asymptotically stable.
2. If D(P)<0 and A≥0, B≥0, and C>0, when α<23, E∗ is locally asymptotically stable.
3. If D(P)<0, A>0, B>0, and AB=C, then for all α∈(0,1), E∗ is locally asymptotically stable.
Theorem 5. If D(P)<0, C>0 and AB≠C, then α∗∈(0,1) exists; when α∈(0,α∗), E∗ is locally asymptotically stable; when α∈(α∗,1), E∗ is unstable. The system diverges at the critical value E∗.
Proof: If D(P)<0, then the eigenvalues of Eq. (3.2) have one real root λ1=a and two complex conjugate roots λ2,3=b±ci. Then, Eq. (3.2) can be written as
where A=−a−2b, B=b2+c2+2ab, C=−a(b2+c2), c>0, a,b,c∈R.
From C>0, then a<0, and then |arg(λ1)|=π>απ2.
From AB≠C, then −a2b+b(b2+c2)≠−2ab2 ⟹ −2b[(a+b)2+c2]≠0 ⟹ b≠0 and (a+b)2+c2≠0.
Thus, we can obtain |arg(λ2,3)|=|arctan(cb)|=arctan|cb|∈(0,π2).
Then, α∗∈(0,1) exists; when α∈(0,α∗), απ2<arctan|cb|, according to Lemma 2, E∗ is locally asymptotically stable, and when α∈(α∗,1), απ2>arctan|cb|, E∗ is unstable.
3.3. Global asymptotic stability
To study the asymptotic stability of system (2.6), three controllers will be added. The controller is proposed as follows: μ1=m1x(x−x∗), μ2=m2y(y−y∗), and μ3=m3z(z−z∗). where m1, m2 and m3 represent negative feedback gains, which are defined as real numbers. Clearly, if mi=0(i=1,2,3) or x=x∗(y=y∗,z=z∗), then μi=0(i=1,2,3), so it will not change the equilibrium point of system (2.6).
Controllers added into system (2.6) as follows
One gives a Lyapunov function as:
then,
Consider E∗ to be the equilibrium point:
According to Lemma 4, we can obtain
When m1≥a1b1(2y∗+x∗)2(1+b1x∗)−r1K1, m2≥a2b2(2z∗+y∗)2(1+b2y∗)+a1b1x∗2(1+b1x∗)−r2K2, and m3≥a2b2y∗2(1+b2y∗), it follows that DαV≤0. We can show that the equilibrium point E∗ is uniformly asymptotically stable.
4.
Numerical examples
In this section, we use the Adams-Bashforth-Molton predictor-corrector algorithm numerical simulation. This method is described in detail in [37] and [38].
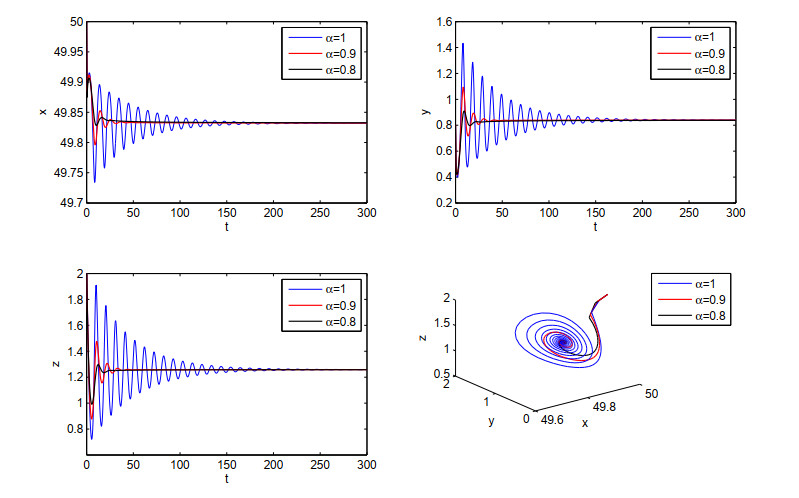
Example 1. In system (2.6), let r1=1, r2=0.6, K1=50, K2=10, a1=1, a2=0.6, b1=5, b2=0.01 and d=0.5. System (2.6) has a positive equilibrium point E∗=(49.8479,0.8403,1.2597). According to Theorem 3.5, when α=1, α=0.9, α=0.8, and E∗ is locally asymptotically stable, it can be seen from Figure 1 that the order α will affect the speed at which the system converges to the equilibrium point. The relevant results are shown in Figure 1.
Example 2. In system (2.6), let r1=1, r2=0.6, K1=50, K2=30, a1=1, a2=0.6, b1=5, b2=0.2 and d=0.2. System (2.6) has a positive equilibrium point E∗=(49.9382,0.3571,1.4158). It follows from Theorem 3.5 that system (2.6) has a bifurcation at α∗. When α=0.95 and α=0.8, E∗ is locally asymptotically stable, and when α=0.98, E∗ is unstable. The relevant results are shown in Figure 2.
Example 3. To verify the sensitivity of the system (2.6) to initial conditions and other parameters, according to the method in [39], apply the positive Euler format to transform the differential model into the following discrete form:
where δ is the time step size. We use the parameters of Example 1 to study Lyapunov exponents. Figure 3 shows that system (2.6) is in a stable state and is less sensitive to initial conditions.
5.
Conclusions
This paper studies a new fractional-order food chain model with a Holling type-II functional response. First, the existence, uniqueness, nonnegativity and boundedness of the solution of the model are discussed. Second, the local stability of each equilibrium point is discussed. Third, controllers μ1=m1x(x−x∗), μ2=m2y(y−y∗) and μ3=m3z(z−z∗) are proposed and added to the system. Using the Lyapunov method, sufficient conditions for the positive equilibrium point to reach the global uniformly asymptotically stable state are obtained. Finally, we use numerical simulations to verify the theoretical results.
Acknowledgments
This work was supported by the Shandong University of Science and Technology Research Fund (2018 TDJH101).
Conflict of interest
The author declares no conflicts of interest in this paper.









 DownLoad:
DownLoad: