1.
Introduction
System reliability and availability have been extensively used in numerous fields, such as industry, power, aviation, computers and networks, etc. With the increasing demand for the reliability of the system, system designers and managers adopt various methods to improve the system's reliability. Redundant standby design is a simple and effective way to improve system reliability, which is widely used in a variety of practical systems, such as, mission-critical systems, including hospital emergency care systems, power supply systems, and flight control systems. Based on the failure characteristics of the standby period, the types of standby can be classified into hot standby, warm standby, and cold standby. We consider a discrete-time stochastic model of the system with retrial and cold standbys.
The reliability model for repairable retrial system is a kind of stochastic model developed based on retrial queueing theory in recent years. Early studies of retrial queueing systems can be seen in [2,6,10,11,33]. Retrial mechanism is widely used in modeling many practical problems such as call centers, computer networks and communication networks. The retrial mechanism is a feature that some automation systems need to consider during the design phase. The retrial mechanism of a repairable system means that if the repair equipment is idle when the failed component gets to the maintenance station, the repair equipment repairs it immediately. Otherwise, the component failure information is automatically stored in the failure information repository (retrial orbit), and the maintenance request is repeatedly sent after some time. Given the fully automated characteristics of some future system equipment, it is sometimes necessary to consider the retrial mechanism of failed components when designing, modeling and analyzing the system reliability. Continuous-time warm standby repairable retrial systems with N-policy have been researched by Chen and Wang [9]. Yang and Tsao [42] studied a continuous-time standby retrial system with multiple vacations using the matrix-analytic and Laplace transform methods. Yen et al. [43] considered machine repair problems for systems with retrial and working breakdowns based on the F-policy.
In recent years, scholars have realized that retrial mechanism has certain potential application value in some repairable system reliability model designs, so the research on retrial mechanism has been investigated in some reliability models [14,17,18,38,44]. Due to the repair equipment will inevitably fail in engineering practice, Gao and Wang [12] analyzed a continuous-time model for retrial systems with unreliable repair equipment. Wu et al. [39] analytically compared the stationary availability and cost-benefit ratio of four warm standby retrial systems with general repair times and imperfect coverage. Li et al. [20] studied a circular consecutive k-out-of-n: F system with retrial and analyzed some critical system reliability measures. Wang et al. [37] studied linear consecutive k-out-of-n: F systems with retrial and two maintenance activities using the Laplace transform and Runge- Kutta methods. In general, the running time, repair time, and inspection time for some components are measured in discrete time. In view of this situation, we propose a discrete-time model of the system with retrial and cold standbys. Compared with the above literature, the similarity is that the retrial mechanism is considered. The difference is that the above research works focus on continuous-time system models, while this paper focuses on discrete-time system models, and multiple events can occur simultaneously.
Compared with continuous-time reliability models, discrete-time reliability models have been studied relatively late. Early studies of reliability models for discrete-time systems can be seen in [24,25,32,34,41]. Since then, the research of discrete-time reliability models has attracted extensive attention from plentiful scholars. Bracquemond and Gaudoin [7] dealt with discrete lifetime distributions for non-repairable systems. Liu and Kapur [22] investigated discrete-time models for non-repairable systems with multi-state. Habib et al. [15] studied a discrete-time Markov consecutive r-out-of-n: F system model. In engineering practice, managers often through repair method to improve the reliability of the system. Studies on discrete time reliability models for repairable systems have been paid more and more attention by scholars. The discrete-time 2-out-of-(N+1): F repairable system model was investigated by Bruning [8]. Alfa and Castro [1] studied the discrete-time repairable machine reliability and obtained the optimal time to replace the machine. The discrete-time models for systems with repair and cold standbys were studied by Ruiz-Castro et al. [31] based on the matrix-analytic method. Ruiz-Castro et al. [29] utilized matrices with low order to calculate reliability measures for discrete-time cold standby repairable systems by using the RG-factorization method. Subsequently, Ruiz-Castro et al. [30] proposed discrete-time models for repairable systems with external and internal failures. Ruiz-Castro and Fernández-Villodre [27] studied a discrete-time model for repairable systems with warm standbys and provided the cost-benefit analysis of the system. In addition, Li et al. [21] conducted a discrete-time model for repairable systems with multi-state. Kan and Eryilmaz [16] evaluated the reliability and hazard rate functions for discrete-time models for repairable systems with cold standbys. Ruiz-Castro and Li [28] introduced several types of failure into a discrete-time model for k-out-of-n: G repairable system with multiple repairmen. Ruiz-Castro [26] investigated discrete-time models for systems with multi-state, warm standbys and preventive maintenance.
The research on discrete-time reliability for repairable systems rarely considers the retrial of failed components. The research on discrete-time models for retrial systems mainly focuses on queueing performance measures. Atencia and Moreno [4] studied a discrete-time model for Geo/G/1 retrial queueing systems. Atencia and Moreno [5] studied a discrete-time single-server model for the Geo/Geo/1 queue with negative arrivals. Artalejo et al. [3] analyzed a discrete-time model for Geo[X]/G/1 retrial queueing systems with batch arrivals. Wu et al. [40] studied a discrete-time model for Geo/G/1 retrial queue subject to preferred and impatient customers. Considering the inevitable failure of servers in practical engineering systems, the discrete-time models for queueing systems with unreliable servers have also been considered in [13,19,23,35,36]. It can be noticed that the discrete-time models for retrial queueing systems in queueing theory have been thoroughly studied by scholars. In view of the correlation between repairable system reliability and queueing theory, the retrial mechanism is considered in the reliability model for a discrete-time cold standby repairable system in this paper.
Based on the above literature review, it can be observed that massive works have investigated continuous-time reliability models for repairable systems, but there are relatively few studies on the discrete-time reliability models for repairable systems. Although discrete-time reliability models for repairable systems have been studied by some researchers, the retrial mechanism of failed components in discrete-time repairable systems has not been studied. The running time, repair time, and inspection time of some repairable systems in engineering practice are measured in discrete time, and the failure information of system components is not successfully sent to the repair equipment in some cases. Therefore, the retrial mechanism is introduced into the reliability model of a discrete-time system with repair and cold standbys for modeling and evaluating the system reliability in this paper. The reliability measures of the system with retrial and cold standbys are obtained using the discrete-time Markov process theory, the iterative algorithm of the difference equation, and generating function method. In this work, it is assumed that the lifetime of each component, the repair time and the retrial time of each failed component are distributed geometrically. The geometric distribution in discrete time and the exponential distribution in continuous time have similar effects in stochastic modeling and analysis of the system. Therefore, using geometric distribution to describe random variables related to components will be more conducive to mathematical processing and analysis. In addition, the failure rate, repair rate, and retrial rate are approximately constant during the stable operation of the system, so the geometric distribution can be used to model the reliability of the discrete-time system.
The design of a discrete-time standby retrial system model can be applied in the field of communication. We show a potential application of the developed model in cloud data processing. In this application, the cloud data processing center contains one operating virtual machine and multiple cold standby virtual machines. The Cloudstack cloud management tool is installed as a virtual machine repair server. The repair server has a storage system for storing failure signals of the virtual machine. When the operating virtual machine fails, the cold standby virtual machine immediately substitutes the failed one and becomes the operating virtual machine. The super supervisor sends the failure signal to the virtual machine repair server or saves it to the storage system on the virtual machine repair server. If the virtual machine repair server is idle, it will repair the failed virtual machine immediately. Otherwise, the storage system continuously sends repair requests to the virtual machine repair server. Through the modeling of practical engineering application problems, this paper makes a certain contribution to the extended research of discrete-time cold standby systems. The Major contributions of this paper are as follows:
● A new discrete-time model for reliability systems with retrial and cold standbys is proposed based on geometric distribution.
● The priority order of simultaneous occurrence of multiple events is defined for the case in which multiple events can occur simultaneously at the same time in the discrete-time system model.
● Based on the system's reliability measures and other performance measures, the expected cost function and cost-benefit ratio function of the system are formulated.
● An algorithm is designed to calculate the stationary and transient probabilities of the system, and the steps for solving the cost-benefit ratio optimization model based on the PSO algorithm are given.
The remainder of this paper is structured as follows. In Section 2, the system model is given. In Section 3, we provide crucial reliability measures derivation from the system model. In Section 4, we provide system cost-benefit ratio function construction and optimization. Numerical analysis is provided to demonstrate the influence of each parameter on system reliability, the cost-benefit ratio, and the system measures with and without retrial mechanism in Section 5. Finally, in Section 6, we provide the findings and presents future research directions.
2.
The model
2.1. Description and assumptions
We consider a cold standby n-system with one operating component and n−1 cold standbys. There is only one repair equipment. When the operating component fails, the cold standby one (if there is a cold standby) immediately substitutes the failed one and becomes the operating one. If the repair equipment is idle when the failed component arrives, the repair equipment repairs it immediately. Otherwise, the failed one enters the retrial orbit and tries again after a time until it is repaired (first come, first out). Specific model assumptions are established as
(1) The operating component's lifetime, X, is distributed geometrically with parameter p, given by
where 0<p<1, ˉp=1−p.
(2) Each failed component's repair time, Y, is distributed geometrically with parameter δ, given by
where 0<δ<1, ˉδ=1−δ.
(3) Each failed component's retrial time, Z, is distributed geometrically with parameter r, given by
where 0<r<1, ˉr=1−r.
(4) All events occur at the time point, when events occur at the same time, the priority order of simultaneous occurrence of multiple events is defined, including the following two models:
Model A: Repair of failed component, failure of operating component and retrial of failed component.
Model B: Failure of operating component, retrial of failed component and repair of failed component.
(5) At the initial moment, all components are brand new, the operating component is working, and the repair equipment is idle. Switch time between an operating component and a cold standby component can be neglected.
(6) All of the failed components are repaired as new. The operation time, repair time and retrial time for all components are mutually independent.
2.2. Model state analysis
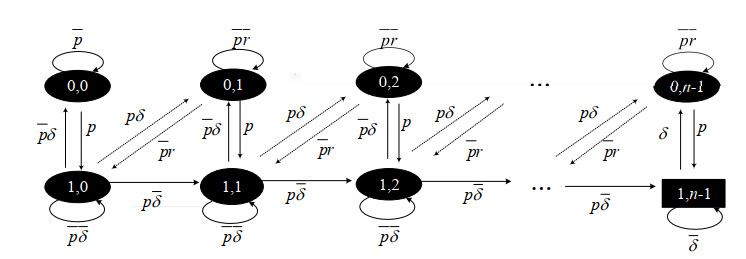
At time k, let J(k) represent the state of the repair equipment, and I(k) be the number of failed components in orbit. Here, J(k)=1 means that the repair equipment is busy, and J(k)=0 means that the repair equipment is idle. The value of I(k) is 0,1,⋯,n−1. System state can be expressed as D(k)={J(k),I(k),k=0,1,2,⋯}. Apparently, D(k) is a discrete-time Markov chain with state space Ω including working states set W and failed states set F, where, Ω={(j,i),j=0,1,i=0,1,⋯,n−1}, W={(j,i),j=0,1,i=0,1,⋯,n−2}∪{(0,n−1)}, and F={(1,n−1)}.
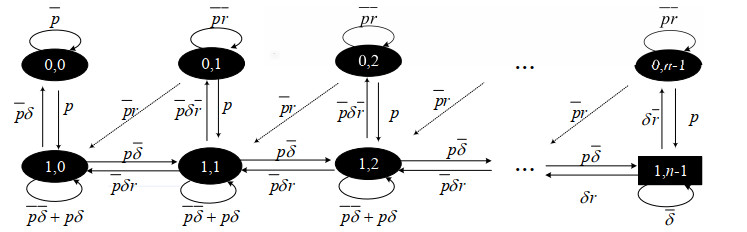
Two state transition diagrams of models A and B are depicted in Figures 1 and 2, respectively. The states in the ellipse and rectangle are the system working states and failure states, respectively. According to the system state definitions and the relevant model assumptions, all the transitions between different states are determined. Taking model A as an example, six types of transition probabilities are described (see Appendix).
2.3. Transition probability matrix
● The transition probability matrix Q of model A
● The transition probability matrix Q′ of model B
3.
Model reliability analysis
Taking model A as an example, this section presents the derivation process of several reliability indices.
3.1. Availability
● Transient availability
At time k, let Pj,i(k)(j=0,1,i=0,1,⋯,n−1) represent the probability of the system in the state (j,i), and the system state probability vector is denoted as
Based on the discrete-time Markov process theory, the system state probability equation is given by
and the initial distribution of the system state probability is
Based on the iterative algorithm of the difference equation, using Eqs (3.1) and (3.2), we have
Thus, the system state probability P(j,i)(k) is obtained.
Once P(j,i)(k) is determined, the system transient availability, denoted by A(k), can be expressed as
where cu is a 2n dimensional column vector whose u-th row element is equal to 1 and the others are equal to 0. c2n is a 2n dimensional column vector whose 2n-th row element is equal to 1 and the others are equal to 0.
● Stationary availability
The system will enter a stable state after a long period of operation. When the system is in a steady state, the probability that each state stops in each state during the process of mutual transfer is defined as the stationary probability. According to Figure 1, all state probabilities of the system satisfy the following stationary probability equations
The above equations for the stationary probability π(j,i) can also be expressed as a matrix for the stationary probability vector π, π=(π(0,0),π(1,0),π(0,1),π(1,1),⋯,π(0,n−1),π(1,n−1)), and then combined with the normalization condition, The linear system of π is obtained as
where e2n is a 2n dimensional column vector with whole elements being 1. The system stationary probability π(j,i),(j,i)∈Ω is obtained by solving Eq (3.5).
Hence, the stationary availability A(∞) can be written as
The calculation of the stationary and transient probability of the system can be realized by programming, as shown in Table 1.
3.2. Conditional probability of failure
At time k, the conditional probability of failure of the operating component (system) is defined as the probability of the component (system) operating normally at time k−1 and failure at time k. Based on Eq (3.3), the following two performance measures can be obtained as
● The conditional probability of the operating component failure at time k is
● The conditional probability of the system failure at time k is
where c2n−2 is a 2n dimensional column vector whose (2n−2)-th row element is equal to 1 and the others are equal to 0. c2n−1 is a 2n dimensional column vector whose (2n−1)-th row element is equal to 1 and the others are equal to 0.
In the stationary situation, the above two performance measures can be expressed by the following Eqs (3.9) and (3.10) according to Eq (3.5), respectively.
● The stationary conditional probability of the operating component failure is
● The stationary conditional probability of the system failure is
3.3. Reliability function and MTTFF
For analyzing the system reliability function R(k), suppose the system failure state be the absorption state of the Markov process, then a new Markov chain ˜D(k)={˜J(k),˜I(k),k=0,1,2,⋯} can be obtained. Let S(j,i)(k)=P{˜D(k)=(j,i)},(j,i)∈Ω. Under the newly defined Markov chain, at time k, the system probability vector in working states can be written as
The system transition probability matrix from working states to working states is
The system transition probability matrix from working states to failure states is
According to Eq (3.1), the following matrix equation can be obtained as
From Eq (3.11), we have
According to Eq (3.12), SW(k)=SW(0)Bk can be obtained by the iterative algorithm of the difference equation, that is, the system reliability function is
where e2n−1 is a 2n−1 dimensional column vector with whole elements being 1.
Since B is the transition probability matrix of the system from working states to working states, it has its spectral radius ρ(B)≤1. When s<1, ρ(sB)<1, then matrix I2n−1−sB is reversible. By using Eq (3.12), the probability generating function for each working state of the new Markov chain can be given by S∗W(s)=SW(0)(I2n−1−sB)−1, where I2n−1 is a 2n−1 order identity matrix.
By calculating the generating function at both ends of Eq (3.13), we can obtain
From MTTFF=R∗(1)=lims→1R∗(s), MTTFF can be written as
4.
Cost-benefit ratio analysis
In this section, model A is taken as an example to give the construction and optimization of the system cost-benefit ratio function.
4.1. System cost-benefit ratio function construction
According to the system state probability, the following stationary state performance measures are obtained as
● The probability of the repair equipment being free, denoted by Pf can be written as
● The probability of the repair equipment being busy, denoted by Pb can be written as
Let E[N] be the expected number of components in the retrial orbit, πi is the stationary probability vector corresponding to the i number of failed components in the system retrial orbit, where πi=(π(0,i),π(1,i))(i=0,1,⋯,n−1), e2 is a 2-dimensional column vector with whole elements being 1, then we have
The stationary busy cycle Tc is defined as the interval from the time when whole components are normal and the repair equipment is idle to the time when whole components are normal and the repair equipment is idle again. Suppose T(0,0) be the interval length for repair equipment idle and no failed component in orbit. In the stationary E[T(0,0)]=1p, according to the alternating renewal process, the stationary probability of the system in state (0,0) is determined as π(0,0)=E[T(0,0)]E[Tc], and stationary expected busy cycle can be written as
The improvement of system reliability often requires higher cost input, so the system managers will be more concerned about the optimization of the cost-benefit ratio (CBR). The cost-benefit ratio can be defined as the cost per unit time of expected total (TC) and A(∞) ratio in the stationary situation. In order to establish an optimization model about CBR, cost elements can be defined as:
C0≡ cost per unit time of per failed component in the retrial orbit,
C1≡ cost per unit time of the repair equipment is free,
C2≡ cost per unit time of per failed component to be repaired at a repair rate δ,
C3≡ cost per unit time of per failed component successfully retried at a retrial rate r,
Cs≡ setup cost per cycle.
Based on the above definition of cost elements and the corresponding performance measures of the system, take δ and r as variables to construct the following cost per unit time of expected total function and the system cost-benefit ratio function, respectively:
4.2. CBR optimization
Compared with other intelligent optimization algorithms, the PSO algorithm is easier to implement, so it is commoner in practical applications. In this section, we aim to search for the optimal values of repair rate δ∗ and retrial rate r∗, and minimize the value of CBR by using the PSO algorithm. The steps are as follows:
Step 1. Initialization of (δ,r) scheme set.
The (δ,r) scheme set corresponding to the CBR minimization question min(δ,r)CBR(δ,r) contains the number of (δ,r) scheme is D, among which 0<δ,r<1. The initial (δ,r) scheme set is denoted as ϑ0=(ψ10,ψ20,⋯,ψD0), and the ιth (δ,r) scheme in ϑ0 is denoted as vector ψι0=(δι0,rι0). The velocity vector corresponding to ψι0 is denoted as vι0=(vι01,vι02), and the initial velocity set corresponding to ϑ0 is denoted by v0=(v10,v20,⋯,vD0).
Step 2. Calculation of fitness.
Equation (4.6) is used to calculate the fitness of each (δ,r) scheme ψικ (fitness refers to the value of Eq (4.6) in this paper), denoted by Fικ, Fικ=CBRικ(δικ,rικ).
Step 3. Update of optimal (δ,r) scheme of the individual and the κth generation.
The fitness Fικ is compared with the fitness FιOP of the ιth (δ,r) scheme ψιOP, which is the best in the first κ−1 (δ,r) schemes set. If the fitness Fικ is lower, then the ψιOP is updated to ψικ, that is, the fitness FιOP=min{Fι0,Fι1,⋯,Fικ} corresponding to ψιOP. The fitness of all (δ,r) schemes in individual optimal (δ,r) scheme set ψOP=(ψ1OP,ψ2OP,⋯,ψDOP) is compared, and the (δ,r) scheme corresponding to the lowest fitness is defined as the κth generation optimal (δ,r) scheme ψκbest.
Step 4. Update of (δ,r) scheme and velocity.
The ιth (δ,r) scheme will update the content and velocity of the scheme by tracking the ψιOP and ψκbest. The updated formula is
where, w is the inertia factor, w=wmax−(wmax−wmin)×ℓ/ℓmax, ℓ represents the number of iterations, q1 and q2 are the learning factors, and rand(χ−η) represents generating a random number between χ and η. If the generated vικ+1 or ψικ+1 is not within the value range, the vικ+1 or ψικ+1 that meet the conditions is randomly generated anew.
Step 5. Termination of update.
The update terminates until the (δ,r) scheme set has been updated ℓmax times. The optimal (δ,r) configuration scheme is ψ∗=(δ∗,r∗).
5.
Numerical analysis
In this section, we take a 3-component system as an example to illustrate the numerical results of the obtained performance indices. Let p=0.3,δ=0.8 and r=0.5 be the basic parameters of the system. Model A is taken as an example to illustrate the numerical analysis.
5.1. Reliability measures
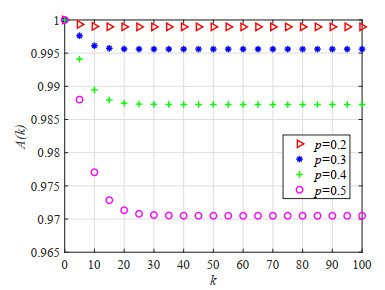
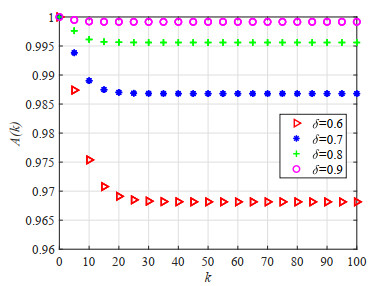
Figures 3–5 show the change of the system transient availability A(k) with time k under different parameters. The transient availability curve decreases sharply in the time interval [0,15] and decreases slowly in the time interval [15,30]. After time k=30, the availability curve gradually becomes steady, and the stationary value is the stationary availability of the system. The parameters p and δ have a substantial impact on A(k), while parameter r has a relatively small impact on A(k).
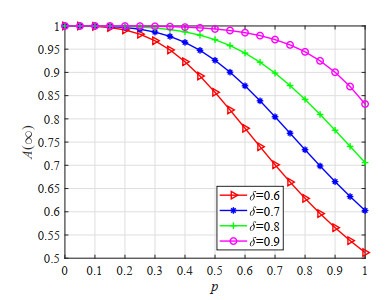
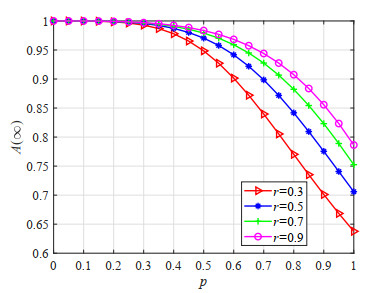
The impact of the parameter p on A(∞) for different parameters δ and r are respectively given in Figures 6 and 7. A(∞) decreases as p increases, and increases as δ or r increases. That is, the smaller the rate p, the longer the normal operating time of the system. The higher the rate δ, the shorter the component's repair time in repair state, and the more the normal components. The higher the rate r, the shorter the failed component's retrial time.
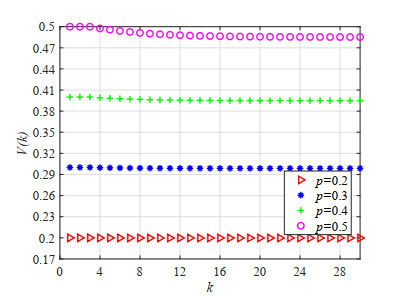
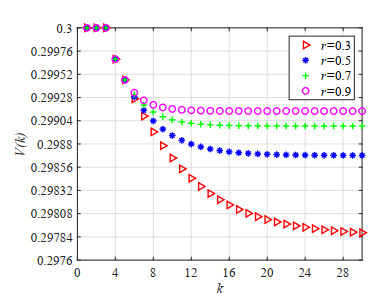
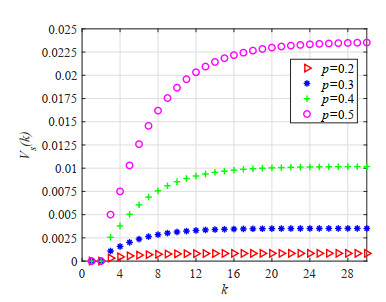
Figures 8–10 and Figures 11–13 show the change of conditional failure probability of the operating component and system under different parameters with time k, respectively. The numerical results in Figure 8 show that V(k) does not change significantly with time k, and the curve becomes steady soon. The corresponding stationary value is the conditional probability of operating component failure when the operating component is in the stationary situation, and the change of the parameter p has a great influence on V(k). Figures 9 and 10 show that V(k) first decreases with time k, and then the curve gradually becomes stable. When the parameter δ or r is relatively small, the corresponding value V(k) is smaller. Figures 11–13 show that Vs(k) first increases sharply with time k and then increases slowly, and then the curve gradually becomes stable. The corresponding stationary value is Vs when the system is in the stationary situation. Parameter p has a significant impact on Vs(k), parameter δ has a moderate impact on Vs(k), and parameter r has a relatively small impact on Vs(k).
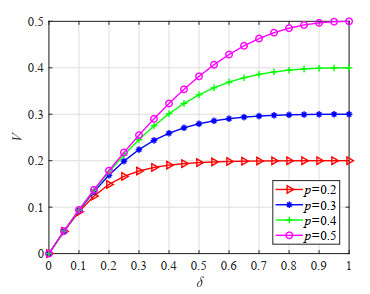
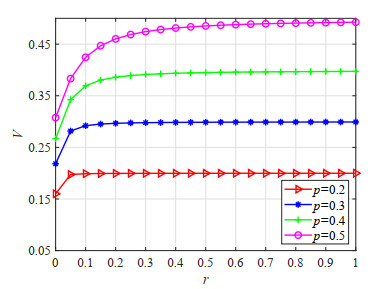
Figures 14 and 15 show the variations of V with parameters δ and r respectively when parameter p is different. Figure 14 presents the impact of parameter δ on V for different p. As can be seen from the numerical results in Figure 14, with the increase of parameter δ, the curve of V first increases sharply, then increases slowly, and finally the curve of V gradually becomes stable. The smaller the failure rate of components, the smaller the stable value can be reached when the value of the δ is relatively small, and the smaller the corresponding stable value will be. Figure 15 presents the impact of parameter r on V for different p. As can be seen from the numerical results in Figure 15, with the increase of parameter r, the curve of V first increases sharply, then increases slowly, and finally the curve of V gradually becomes stable. The smaller the parameter p, the smaller the stationary value is reached when the parameter r is relatively small, and the smaller the corresponding stationary value will be. In addition, it can be observed from Figures 14 and 15 that V increases as p increases. When the parameter δ is small relative to the parameter p, V is not obvious to the change of the parameter p. When the parameter δ is large relative to the parameter p, the parameter p has a significant influenceon V.
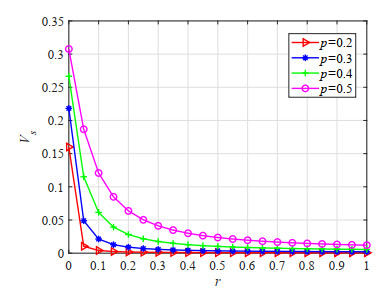
Figure 16 presents the impact of parameter δ on Vs for different p. From Figure 16, Vs increases as p increases. When parameter δ is small, Vs increases as δ increases. When parameter δ is relatively large, Vs decreases as δ increases. Figure 17 presents the impact of parameter r on Vs for different p. From Figure 17, as r increases, the curve first sharply decreases and then slowly decreases, then gradually becomes stable. The smaller the failure rate p is, the stable value will be reached when the value of r is relatively small, and the smaller the corresponding stable value will be.
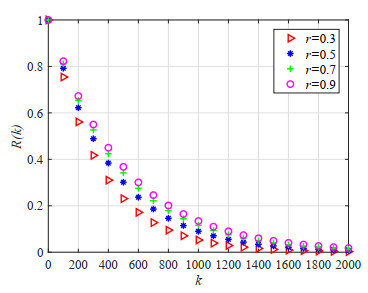
Figures 18–20 respectively show the change of R(k) with time k under different parameters p,δ and r. It can be seen that parameters p and δ significantly affect R(k), and R(k) decreases as p increases and increases as δ increases, because the less likely the operating component is to fail and the less time it takes to repair the failed component, the higher the system reliability. R(k) is not sensitive to the change of the parameter r, and R(k) increases as r increases, because the easier the failed component is to retry in the retrial orbit, the less time the failed component has to wait for repair, and the higher the reliability of the system.
To better show the impact of the number of system components on a series of system performance indices, we adjust the system parameters to p=0.39,δ=0.6,r=0.5 in this section. Table 2 shows the major reliability measures for different numbers of components. The numerical results show that A(∞), MTTFF of the systems, and V increase with the number of components climbs up. However, Vs decreases as the number of components climbs up. The reason is that with the increase of the number of system components, that is, the number of cold standby components increases, and the system is in the working state for a longer time. By analyzing the change of system reliability measures with the number of system components, we get that system reliability can improve by increasing the number of system components.
The impact of parameters p,δ and r on MTTFF are given in Tables 3 and 4. Since MTTFF is most sensitive to the parameter p, we consider the parameter p in each table. From Tables 3 and 4, MTTFF increases as δ or r increases. As the value of δ or r climbs up, the repair time and waiting time of failed components become shorter, that is, there will be sufficient cold standby components to replace the failed components when the operating component fails. MTTFF decreases as the rate p climbs up. It decreases fastly for the smaller value of p, however, MTTFF decreases slowly for the larger value of p. To sum up, MTTFF is significantly influenced by parameter p, while parameters δ and r have relatively little influence.
5.2. Comparative analysis of some transient reliability indices of two models
Several system transient reliability indices of models A and B are contrast displayed. By observing Table 5, it can be found that the reliability index values of models A and B have almost no difference, that is, changing the priority order of multiple events hardly affects the reliability of the system studied in this paper.
5.3. Comparison of system measures with and without retrial mechanism
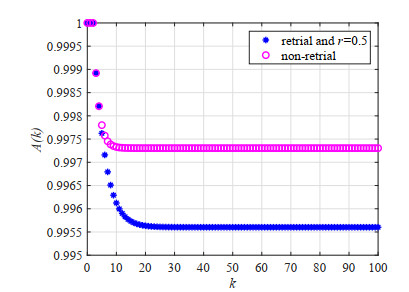
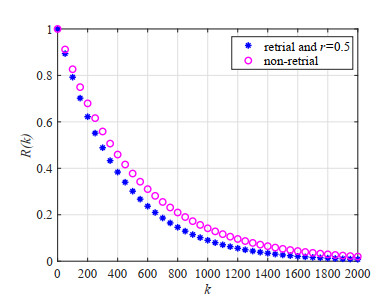
The number of components in the systems with and without retrial mechanism is set to 3, and the reliability and availability of the two systems are compared. Figures 21 and 22 show that the two systems are highly reliable. The stationary availability is greater than 0.995, and the reliability is still close to 0.9 when the systems operate to time k=50.
5.4. Cost-benefit ratio analysis
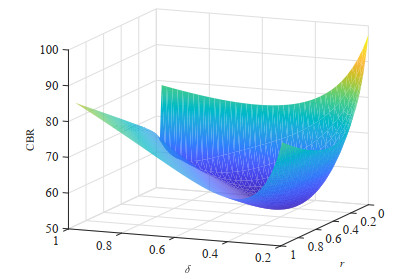
Let C0=8,C1=15,C2=30,C3=30 and Cs=180 be the basic values of the parameters. The numerical results in Figure 23 show that CBR(δ,r) shifts with parameters δ and r at the same time. By observing the numerical results in Figure 23, it is found that, on the one hand, when the parameter r is fixed, the CBR first decreases and then increases with the increase of the parameter δ; on the other hand, when the parameter δ is fixed, the CBR first decreases and then increases with the increase of the parameter r. As can be seen from the general trend in Figure 23, it is found that with the increase of parameters δ and r, the surface of the graph demonstrates a trend of first decreasing and then ascending, and the graph has a lowest point. Based on the PSO algorithm in Section 4.2, let D=200,ℓmax=200,q1=0.4,q2=0.3,wmax=0.8,wmin=0.6,vmax=0.6 and vmin=−0.6. We can obtain the optimal value (δ∗,r∗)=(0.4671,0.2362) and the corresponding minimum value of cost-benefit ratio CBR(δ∗,r∗)=50.9226.
6.
Conclusions
Based on the discrete-time Markov process theory, two reliability models for systems with retrial and cold standbys are investigated in this study. To begin with, some reliability measures of the system such as availability, reliability function, MTTFF and other performance measures are obtained. In addition, the impact of failure rate, repair rate, and retrial rate on system critical reliability measures is performed. Then, the impact of parameters δ and r on the CBR of the system is analyzed, and the two values of the repair rate δ∗ and retrial rate r∗ corresponding to the minimum value of CBR are obtained using the PSO algorithm. Moreover, the system transient reliability measures with and without retrial mechanism are analytically compared. Furthermore, the design of the system state probability algorithm can improve the calculation efficiency of system performance measures, especially when the number of system components is large. Last, this work is aimed at the situation of complete reliability of repair equipment. In the future, the unreliability situation of repair equipment or the vacation strategy of repairmen can be introduced into the discrete-time cold standby repairable retrial system.
Appendix
Six types of transition probabilities of model A are described as follows.
(1) (j,i)→(j,i)
The one-step transition probability of (0,0)→(0,0) is ¯p. The operating component is normal and the repair equipment remains idle at the next time.
The one-step transition probability of (1,i)→(1,i),(i=0,1,⋯,n−2) is ¯p¯δ+pδ. The first item of the sum indicates that the operating component is normal and the repair equipment is still repairing the failed component at the next time. When there are failed components in the retrial orbit, the failed component may or may not retry in the retrial orbit. The second item of the sum indicates that the repair equipment completed the repair of the failed component and the operating component failed. When there are failed components in the retrial orbit, the failed component may or may not retry in the retrial orbit.
The one-step transition probability of (0,i)→(0,i),(i=1,2,⋯,n−1) is ¯p¯r. The operating component is normal and the failed component in the retrial orbit does not retry.
The one-step transition probability of (1,n−1)→(1,n−1) is ¯δ. System failure and the repair equipment is still repairing the failed components at the next time, and the failed component may or may not retry in the retrial orbit.
(2) (0,i)→(1,i)
The one-step transition probability of (0,i)→(1,i),(i=0,1,⋯,n−1) is p. Failure of operating component, repair equipment priority repair of failed components. When there are failed components in the retrial orbit, the failed component may or may not retry in the retrial orbit.
(3) (1,i)→(1,i+1)
The one-step transition probability of (1,i)→(1,i+1),(i=0,1,⋯n−2) is p¯δ. The repair equipment is repairing a previously failed component when the operating component fails and enters retrial orbit. When there are failed components in the retrial orbit, the failed component may or may not retry in the retrial orbit.
(4) (0,i)→(1,i−1)
The one-step transition probability of (0,i)→(1,i−1),(i=1,2,⋯,n−1) is ¯pr. The operating component is normal and the failed component in the retrial orbit retries successfully at the next time.
(5) (1,i)→(0,i)
The one-step transition probability of (1,0)→(0,0) is ¯pδ. The operating component is normal and the repair equipment completes the repair of the failed component at the next time.
The one-step transition probability of (1,i)→(0,i),(i=1,2,⋯,n−2) is ¯pδ¯r. The operating component is normal and the repair equipment completes the repair of the failed component at the next time, the failed component in the retrial orbit does not retry.
The one-step transition probability of (1,n−1)→(0,n−1) is δ¯r. The repair equipment completes the repair of the failed component and the failed component in the retrial orbit does not retry. The system starts to operate normally.
(6) (1,i)→(1,i−1)
The one-step transition probability of (1,i)→(1,i−1),(i=1,2,⋯,n−2) is ¯pδr. The operating component is normal and the repair equipment completes the repair of the failed component at the next time, the failed component in the retrial orbit retries successfully.
The one-step transition probability of (1,n−1)→(1,n−2) is δr. System failure and the repair equipment completes the repair of the failed component and the failed component in the retrial orbit retries successfully at the next time, the system starts to operate normally.
Author contribution
Mengrao Ma: Methodology, Software, Writing-original draft, Visualization; Linmin Hu: Conceptualization, Writing-review & editing, Funding acquisition; Yuyu Wang: Validation, Writing-review & editing; Fang Luo: Validation, Date curation. All authors have read and approved the final version of the manuscript for publication.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This work was supported by the National Natural Science Foundation of China [grant number 72071175], Shijiazhuang Science and Technology Project [grant number 241790737A], and the Basic Innovative Research and Cultivation Project of Yanshan University [grant number 2023LGZD003].
Conflict of interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.









 DownLoad:
DownLoad: