1. Introduction

Dealing with natural hazards involves working with natural processes or phenomena “that may cause loss of life, injury or other health impacts, property damage, loss of livelihoods and services, social and economic disruption, or environmental damage” ^[1]. To determine the landslide activity and/or slope stability or to recognize the possibility of failure in the future are goals of most investigations. It is known that landslides can result in large numbers of casualties and great economic losses particularly in regions with steep slopes ^[2]. The most disastrous landslides have claimed as many as 100,000 lives ^[3] and, for example, in the United States, landslides cause estimated damages of about US $1-2 billion in economic losses and about 25-50 deaths annually ^[2]. Thus, another important aspect is: What does the landslide mean for people, property and infrastructure? Thus, while the nature and dimensions of a landslide has to be examined to obtain information about stability, the landslide risk must also be investigated to get information about the influence and impact of such events on end-users. Lee and Jones ^[4] defined risk as “the potential of adverse consequences, loss, harm or detriment or the chance of loss”. Thus risk is a human and human-centred concept and is applied in those instances where “humans and the things that human values could be adversely impacted at a foreseeable future date” ^[4]. In general, the term risk describes the combination of the probability of an event and its negative consequences and thus risk can be expressed mathematically by the product of probability and consequences ^[5].

The process that determines the quantitative and qualitative value of risk related to a specific hazard is risk assessment. Risk assessment consists of a review of the technical characteristics of the hazard (including intensity, frequency and probability), analyses of exposure and vulnerability (physical, social, economic and environmental conditions), and evaluation of the effectiveness of existing/alternative capacities to absorb a possible event ^[6]. In this process the identification and characterisation of the potential landslides together with evaluation of their corresponding frequency of occurrences have to be done ^[7]. Landslide hazard characterisation is a key task that requires an understanding of slope processes and potential failure mechanisms in relation to geomorphology, geology, hydrogeology, climate and vegetation.

Bedrock landslides are primarily controlled by the orientation of discontinuities, such as joints, faults and bedding, and their relationships with hillslope angle and direction. The combinations of multiple discontinuities, termed wedge failures, are the most frequently observed rock slope failures that can occur over a wide range of geological and geomorphological conditions ^[8]. To assess the potential occurrence of wedge failure, the orientation of discontinuities is determined by means of stereographic projection techniques. This first step is known as kinematic analysis and it contributes to the risk assessment process by identifying the feasibility of a failure process. In addition to contributing to the characterisation of the landslide hazard, the kinematic analysis stage gives an initial indication of the probability of occurrence as well as the scale of failure that contributes to understanding the consequences. After kinematic analysis comes stability analysis of the identified slope failure mechanism. For clarity, the kinematic analysis stage is also referred to as stability assessment.

This study focuses on the characteristics of rock slopes and the contribution of rock structures in related slope stability problems. Recently published methods improving the assessment of structures using the stereograph are outlined and illustrated with a range of field examples.

---

2. Assessing Rock Slope Hazards

Shallow, near surface slope instability is mainly influenced by soil properties, especially soil- water interaction. Larger, deeper slope instability typically involves rock and is strongly influenced by the orientation and spacing of discontinuities present in the rock mass ^[9,10,11]. These features are most noticeable in deeper, fresh rock but are also important in weathered bedrock slopes. To assess the risk associated with such slopes requires knowledge of the potential mechanisms of failure and the analysis of the probability of failure. Slope stability can be analysed by calculating a factor of safety or a probability of failure based on techniques including static methods, limit-equilibrium and finite element methods ^[12]. For soil slopes continuum finite element models can be applied ^[13]. For rock slopes discontinuous numerical models are typically required ^[14]. It is important to note that the risk is being determined for the mechanism of failure not for the slope itself. It remains possible that the slope could fail by a different mechanism with a different level of risk. Identifying potential instability mechanisms and ensuring that the most problematic mechanisms are considered is an especially important stage of slope stability analysis where rock masses are involved in the failed slope system. The field of structural geology provides a framework for data collection and interpretation of discontinuities within a rock mass ^[15]. New techniques are also being developed to allow data collection for regional investigations of slope stability in rocky terrains ^[16].

Conducting detailed analysis of a large number of potential mechanisms is not an optimal allocation of resources. It is necessary to commence investigation with an assessment of the feasibility of commonly occurring rock slope instability mechanisms in order to develop an optimal stability analysis approach for a given location. The stereographic method is the tool typically used for kinematic analysis ^[17,18]. One of the major strengths of stereographic methods is to represent the orientations of discontinuity planes by the orientation of the line (known as a pole on the stereograph) perpendicular to the plane and thus represent large data sets in a clear way ^[19].

The stereographic assessment of failure mechanisms and analysis of relevant mechanisms is the first stage in quantification of the probability and consequences of potential slope failures. The consequences of rock slope failure can be quantified in terms of the size (volume and dimensions) and run-out distance of the debris interpreted in the context of the habitation and development of the ground below the slope. These attributes of landslide consequences are not directly addressed in this study. Nevertheless, stereographic techniques are a robust method of identification of rock slope failure mechanisms.

---

3. Methodology

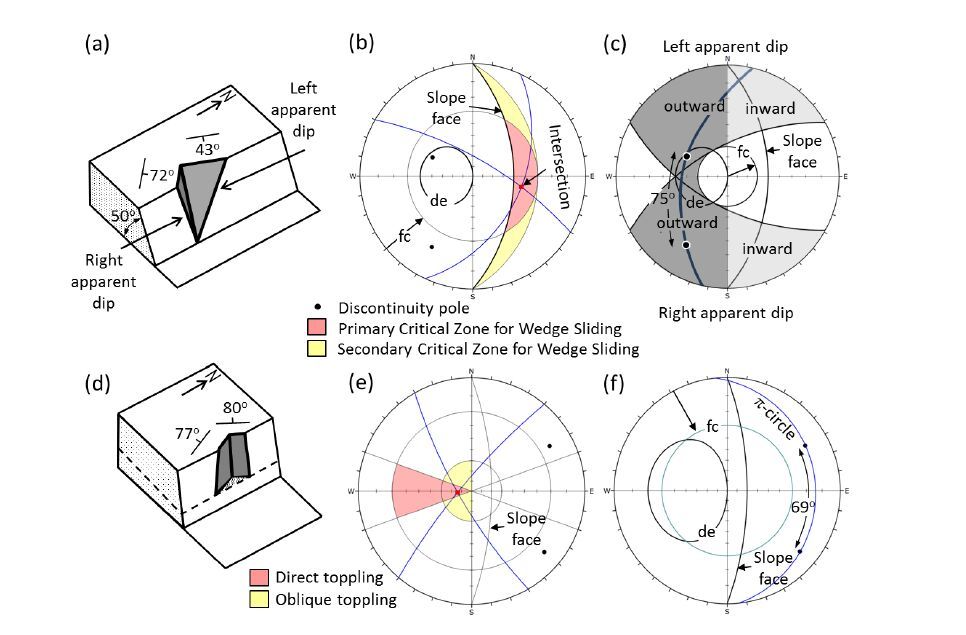
The stereographic method of kinematic analysis is particularly useful for assessment of the stability of pairs of discontinuity planes known as wedges. Such wedges can fail by sliding or by toppling ^[20]. The stability of wedges of rock is conventionally assessed by finding the orientation of the lines of intersection of the pairs of planes ^[20,21]. An alternative method of stereographic assessment involves finding the orientation of great circles linking pairs of discontinuity poles ^[22]. These two methods will be referred here as the intersection method and the circle method, respectively.

---

3.1. IntersectionMethod

An example of a wedge formed by two discontinuities is shown in Figure 1(a). The slope face dips directly east at 50° and the discontinuities dip outward from the slope in a northeast (NE) and southeast (SE) direction, respectively. The NE-dipping discontinuity has a right apparent dip on the slope face and the SE-dipping discontinuity has a left apparent dip on the slope face. The resulting intersection between the discontinuities plunges outward from the slope at an angle less than the slope face angle allowing the wedge to slide from the slope face.

The conventional kinematic analysis for wedge sliding involves constructing the great circle for each pole and observing the location of the intersection (Figure 1b). According to a commonly used stereographic software (Rocscience DIPS V 6.016) primary and secondary critical zones can be identified on the stereograph. The primary critical zone for wedge sliding is the crescent shaped area inside the plane friction cone (measured from the outer perimeter of the stereograph) and outside the slope face plane. The secondary critical zone for wedge sliding is the area between the slope face plane and a plane (great circle) inclined at the friction angle as shown on Figure 1b. The secondary critical zone is required to include examples where sliding occurs on one of the two discontinuity planes. In the primary critical zone sliding typically occurs along the direction of the line of intersection of the two planes. The intersection method is illustrated on the stereograph in one of two ways. Either all the great circles are shown or only the intersection points are shown. The problem with showing all great circles is that the stereograph becomes unreadable even with only a modest amount of data. The problem with showing only the intersections is that the relationship between each intersection and the discontinuities that form the intersection is lost. This is considered a problem in that there is no information retained on the shape or other geometric attributes of the wedge.

In the case of toppling, a wedge can form where two discontinuities dip into the slope face (Figure 1d). The intersection of these discontinuities plunges into the slope face also. The kinematic analysis of direct toppling of wedges is commonly conducted by observing intersections on a stereograph. According to a commonly used stereographic software (Rocscience DIPS V 6.016) direct toppling and oblique toppling zones can be identified on the stereograph. The direct toppling zone is the sector from the vertical to the friction circle (measured from the outer perimeter of the stereograph). The direct toppling sector is inside the plane friction cone (as measured from the outer perimeter of the stereograph) in the direction opposite to the slope face. The sector is typically assigned a width of ±20°. The oblique toppling zone is the semi-circle from vertical to the friction angle (measured from the vertical). The oblique toppling zone accounts for the high variability of intersection lines that can be expected near the vertical direction. An example of these features is illustrated in Figure 1e.

The intersection method, in general, identifies all the intersections which occur between all the discontinuity planes present. The number of wedges identified by the intersection method, i, is given by:

$i\text{ }=\text{ }\left[ n\left( n-1 \right) \right]/2$

(1)

Where n is the total number of discontinuities and i is the number of intersections formed ^[21]. The number of intersections becomes very great even with a modest number of discontinuities in a dataset.

---

3.2. CircleMethod

The circle method involves constructing a great circle through a pair of poles and observing the position of the great circle (p-circle) relative to the slope to assess kinematic feasibility ^[22]. For wedge sliding the position of the p-circle relative to the daylight envelope of the slope face plane and the friction circle (measured from the centre of the stereograph) is used to assess kinematic feasibility. If the p-circle passes through the daylight envelope without touching the friction circle the wedge is kinematically feasible.

An example of application of the circle method to wedge sliding is illustrated in Figure 1(c). The wedge formed by the two discontinuities is assessed as kinematically feasible because the p-circle passes through the daylight envelope of the slope face without touching the friction circle. The angle measured between the discontinuity poles is 75° which indicates that the opening of the wedge on the slope is the supplement of this angle, 105°. Thus the shape of the wedge can be readily observed directly from the p-circle on the stereograph. This angle, termed the wedge aperture by Markland ^[21] and the wedge sharpness by Hudson and Harrison ^[20], is particularly important as it is the primary control on the volume of rock in a wedge and plays an important role in wedge stability ^[20]. The visibility of this attribute on the stereograph is a benefit of using the circle method rather than using intersections to illustrate and assess wedges.

The circle method can also be applied to wedge toppling as illustrated in Figure 1(f). A p-circle is constructed through the pair of discontinuity poles. The p-circle dips in the same direction as the slope face indicating toppling of the wedge is feasible. The angle measured between the discontinuity poles is 69° which indicates that the opening of the wedge on the slope is the supplement of this angle, 111°. Thus the shape of the toppling wedge can be readily observed directly from the p-circle on the stereograph.

The circle method has an additional benefit in that it gives the opportunity to confirm that two discontinuities will form a wedge of practical significance. In theory, two discontinuities can form a kinematically feasible sliding wedge even if one of the discontinuities is dipping inward relative to the slope face or if the two discontinuities have the same apparent dip on a slope. However, such cases are not likely to form a wedge of practical significance as they are typically very thin, as recognised by Markland in 1972 ^[21]. The circle method provides for restricting the kinematic analysis to pairs of poles both dipping out of the slope face and with opposite apparent dips on the slope face. The stereograph can be readily separated into zones in which the orientation of poles relative to the slope face can be defined (Figure 1c). An analysis can be restricted to wedges formed by pairs of poles lying in each of the outward-dipping zones. Such wedges are considered to be the most relevant to practical slope stability analysis. The number of wedges of practical significance identified by the circle method, c, is given by:

$c\text{ }=\text{ }{{n}_{1}}{{n}_{2}}$

(2)

where n₁ and n₂ are the number of discontinuities in each of two sub-sets with opposing apparent dips on a given slope face. The number of such wedges is much smaller than the number of wedges identified in a conventional intersection analysis. In a case where the only discontinuity poles considered were in the outward-dipping wedge-forming part of the stereograph [as shown on Figure 1(c)], a conventional intersection analysis would generate twice the number of intersections compared to the circle method because the intersection method would include wedges formed by pairs of discontinuities with the same apparent dip on the slope face. In general it can be stated that:

$c\text{ }＜\text{ }i/2$

(3)

The difference between the two methods is likely to be significantly greater than a factor of two in most cases. It is proposed that the intersection method is highly conservative in this respect as it includes many wedges which are not of practical significance.

---

4. Field Examples

---

4.1. Guinsaugon, Philippines

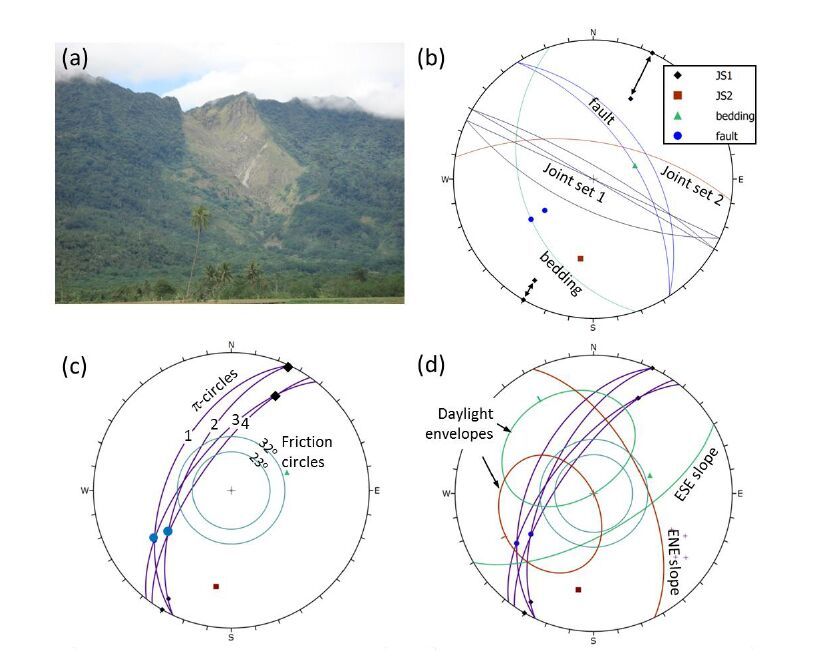
On 17 February 2006 a catastrophic landslide occurred in southern Leyte (Philippines) burying the village of Guinsaugon. The landslide, releasing 15 million m³ of debris, resulted in loss of 1,221 lives and displacement of approximately 19,000 people. It was considered to be caused by progressive degradation of the rock mass due to long-term conditions rather than being attributable to a single causative event ^[23]. An investigation of the Guinsaugon rock slide-debris avalanche identified its deposit characteristics and failure mechanisms ^[24]. The relationship between the orientation of geological structures in the rock and the ground slope was found to be critical to the initiation and propagation of the failure. The landslide initiated on an approximately 800 m high escarpment associated with the Philippine Fault that bisects the major islands of the Philippines (Figure 2a). Bedding layers of the sedimentary and volcanic rocks dip into the hillslope and are not considered to be relatively stable structures. Faults and two sets of joints were identified as significant contributors to the instability of the rock mass.

As is common in the collection and reporting of discontinuity data, the orientations were reported as ranges ^[24]. The implication is that most or all data lies within the ranges stated. This is not typically expressed in a statistical manner unless a very large amount of data is available. The discontinuities were plotted on a stereograph using data published by Catane et al ^[24] (Figure 2b). One of the main mechanisms identified in that investigation is the presence of sliding wedges comprising two discontinuities. In particular, the fault (referring to multiple near-parallel fault structures) and joint set 1 intersect in the southeast quadrant of the stereograph. The plunge of the intersection indicates the instability of the wedge relative to friction and its exposure (daylighting) in the slope face. As can be seen in Figure 2b, even the two fault planes shown and the four joint set 1 planes shown produce numerous intersections. It is also difficult to see clearly which intersections are formed by one fault plane and one joint plane rather than two joint planes. The 6 poles present combine to give 15 intersections (Eq. 1). However this includes pairs of discontinuities that are nearly parallel and would not form wedges of practical significance.

The circle method will be used to study the kinematics of the rock slide. Assessment of the frictional characteristics of the surfaces need to be included in all kinematic analysis ^[25,26,27]. Friction is represented by small circles centred on the vertical axis. Surfaces with friction angles of 32° and 23° were identified in the study by ^[24]. A set of p-circles has been constructed between the two fault poles and two joint set 1 poles which are on opposite sides of the stereograph (Figure 2c). A p-circle which passes through the friction circle represents a wedge which will not slide due to friction. Three of the p-circles pass through the higher friction circle indicating those wedges are stable for that friction condition. One of the p-circles pass through the lower friction circle indicating that wedge is stable for that friction condition. One of the p-circles does not pass through either friction circle indicating that wedge is kinematically feasible if the slope angle and slope direction are unfavourable.

To complete the assessment the slope faces also need to be considered. The Guinsaugon landslide was seen to comprise a SE facing slope and a NE facing slope (Figure 2a). The slope faces are represented by great circles and each slope has a daylight envelope that defines the distribution of poles to planes exposed in that slope face. A wedge of practical significance should comprise two discontinuities dipping outward from the slope face but with opposite apparent dip on the slope face ^[22].

For the SE facing slope the poles to the faults and the part of joint set 1 which dips SSW lie on opposite sides of the stereograph relative to the slope face. Therefore pairs of these structures can potentially form kinematically feasible sliding wedges. One of the four p-circles passes through the daylight envelope for the SE slope without passing through either of the friction circles and therefore represents a kinematically feasible wedge for that slope (Figure 2d). Two of the p-circles passes through the daylight envelope for the SE slope without passing through the 23° friction circles and therefore represents a kinematically feasible wedge for that slope and that friction angle (but these wedges would be stable if the friction angle were the higher value of 32°). The p-circle which passes through the 23° friction circle therefore represents a kinematically stable wedge for that slope at both friction angles.

For the NE slope, the SSW-dipping parts of joint set 1 dip into the slope face and the poles to faults, joint set 2 and the part of joint set 1 which dips NNE are all in the same part of the stereograph. Therefore these discontinuities cannot combine to form wedges of practical significance in this slope face. The fault planes are close to dipping directly out of the slope face and lie within the daylight window for the slope so they represent potential planar sliding structures for this slope.

In summary, the circle method finds kinematically feasible wedges with marginal frictional stability in the SE slope face and no kinematically feasible wedges in the NE slope face. This is in contrast, to the conventional intersection-based wedge kinematic analysis which found wedges to be feasible for both the SE and NE slope faces ^[24]. This erroneous result of the intersection method occurs because there is no test to check that the intersections are formed by planes which together can form a wedge of practical significance.

---

4.2. Sydney, Australia

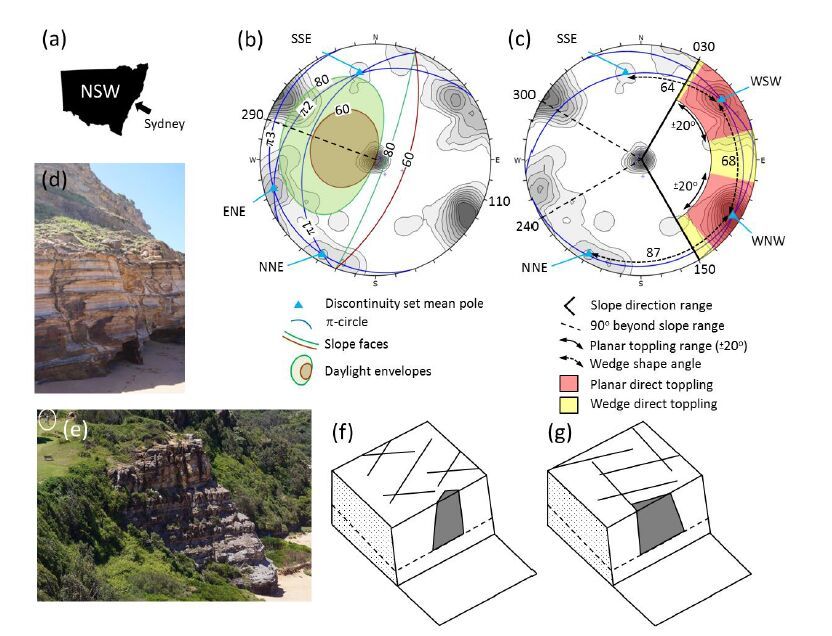
The Northern Beaches district of Sydney, New South Wales, Australia has numerous coastal cliffs which are prone to instability ^[28]. The Sydney basin, specifically the Early Triassic Narrabeen Group bedded sandstones and shales and the lower parts of the overlying Middle Triassic Hawkesbury Sandstone form near-horizontal strata which outcrop along the coast ^[29]. The data collected in this case study was obtained by John V Smith from various sites some of which were also studied by Kotze ^[30]. The orientations of bedding partings and joints (n = 109) measured using a magnetic compass, and corrected for magnetic declination, are shown on stereographs (Figure 3b&c). Bedding is approximately horizontal and the main joint set strikes NE-SW and dips steeply. Steeply dipping joint sets striking NNW-SSE and WNW-ESE are also present. The presence of these steeply dipping, to vertical, joints is a major influence on the stability of the cliffs of the Sydney Northern Beaches district (Figure 3d&e).

Relationships between discontinuities observed on the stereograph can be used to infer the shapes and orientations of potentially unstable blocks of rock along the coast. Large amounts of data can be combined so that the mean orientations of the main discontinuity sets can be used in the kinematic assessment. The p-circle method can be used to make a rapid assessment of the potential failure mechanisms and the kinematic stability of wedge blocks. The orientation of the northern Sydney coastline is variable but an azimuth of 110 (ESE) will be considered here as the direction the coastal cliffs are facing for the wedge sliding kinematic analysis. Relative to this slope direction, three sets of joints dip outward from the face with orientations that could potentially form sliding wedges (Figure 3b).

Two p-circles have been constructed, based on combining set means with opposite apparent dips on the slope face (Figure 3b). The set of joints dipping toward the SSE have a SSW apparent dip on the slope face whereas the ENE- and NNE-dipping sets have a NNE apparent dip on the slope face. The daylight envelope for an 80° slope face is shown on Figure 3b and it can be observed that the two p-circles each cross the daylight envelope indicating kinematic feasibility of wedge sliding. The daylight envelope for a 60° slope face is also shown on Figure 3b and it can be observed that neither of the two p-circles cross the daylight envelope indicating wedge sliding of the mean orientations is not kinematically feasible for that slope face (Figure 3b). The friction of the discontinuities is estimated to be 35° but has not been shown on the stereograph as it does not influence the stability of the wedges identified.

A third p-circle has been constructed, based on combining set means for the ENE and NNE dipping sets (Figure 3b). That p-circle does not pass through the daylight envelope so would not be kinematically feasible for the slope face angles on Figure 3b. It is also relevant that the two set means would have the same apparent dip on the slope face and are not considered to form wedges of practical significance.

The method of constructing p-circles can also be applied to assessing toppling failure mechanisms involving multiple discontinuities. For the purpose of the case study the range of dips of planes capable of undergoing direct toppling is taken as 60° to 90°. This range can vary depending on the friction angle of block interfaces, slope face angle, and spacing of discontinuity sets (block size and shape) and has not been directly considered in this study. The flexural toppling mechanism, which involves confinement of the layers, is not addressed in this example. For the assessment of direct toppling, where blocks are free to move out of a slope, a range of slope directions can be considered simultaneously. In order to include all discontinuity sets dipping into the slope faces being considered the range is extended to the directions 90° from each of the slope direction limits (Figure 3c). Considering a range of slope face directions from azimuth 30 to 150, the joint set means dipping toward WSW-, WNW- SSE- and NNE- are included in the toppling assessment (Figure 3c). A range of 20° each side of the mean has been arbitrary selected as the range of planar toppling and the WSW- and WNW-dipping set means are marked as such (Figure 3c). There is a zone between the WSW and WNW set means where wedge direct toppling formed by a combination of those two discontinuity sets as shown by the presence of the p-circle (Figure 3c). Wedge direct toppling comprising the WSW- and SSE-dipping set means occurs at the northern limit of the slope direction range. Wedge direct toppling formed by the WNW set mean and the NNE set mean occurs to the south of the slope direction range (Figure 3c).

The circle method removes the effect of intersections formed by near parallel planes or by planes with dip directions not conducive to the failure mechanism being considered which are commonly included in the conventional intersection methods. In addition, the circle method displays the poles and on the connecting great circle and thus retains information about the shape of blocks. For example the location of the discontinuity poles relative to the slope face direction allows symmetrical and asymmetrical wedge shapes to be inferred directly from the stereograph (Figure 3f&g). These features make the circle method useful for assessing the kinematics of potential wedge sliding and wedge direct toppling and the geometry of wedge-shaped blocks capable of producing rockfalls on joint-controlled cliffs in the northern beaches area of Sydney, Australia.

---

4.3. Tarkwa, Ghana

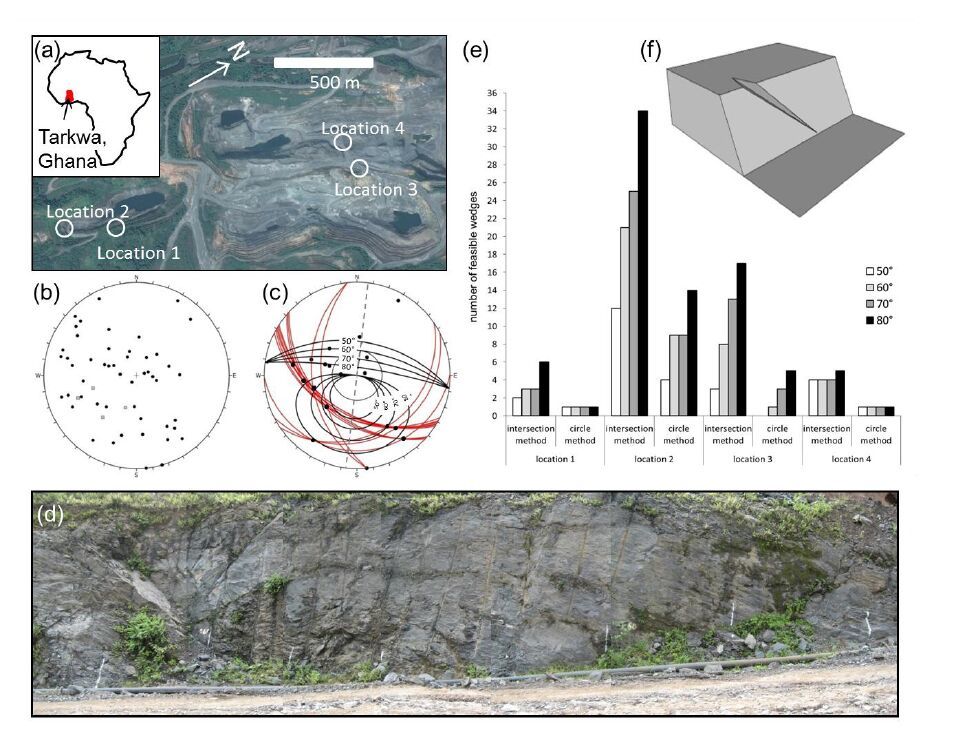
Open pit mine slope design in rock requires a detailed understanding of structural failure mechanisms including tetrahedral wedge sliding and wedge toppling ^[31]. The methodology and case studies above presented some of the general features of the circle method and contrasted these with the conventional intersection method. The rock slope design data in this case study was collected by John V Smith at an open pit manganese mine in Ghana, West Africa (Figure 4a). The mine is located in rocks of the Proterozoic greenstones of the Birimian belt ^[32,33].

At selected locations around the exposed open pit walls, the orientations of bedding and joints were measured (n = 55), using a magnetic compass and corrected for magnetic declination (Figure 4b).Kinematic analysis for wedge sliding using the intersection method and circle method was conducted for the discontinuity data from each location. The analysis was performed for four slope face angles from 50° to 80°. The p-circles for one location compared to the daylight envelope of the slope face are shown in Figure 4c&d. It was found that the intersection method identified from 2 to 8 times more wedges than the circle method (Figure 4e). This is because many intersections are formed by planes which are not capable of forming wedges of practical significance, such as planes which are nearly parallel to each other or are dipping into the slope face (Figure 4f). The circle method ^[22] can be used to restrict the discontinuities to those which dip out of the plane and to pairs of planes which have opposing apparent dip directions on a slope face.

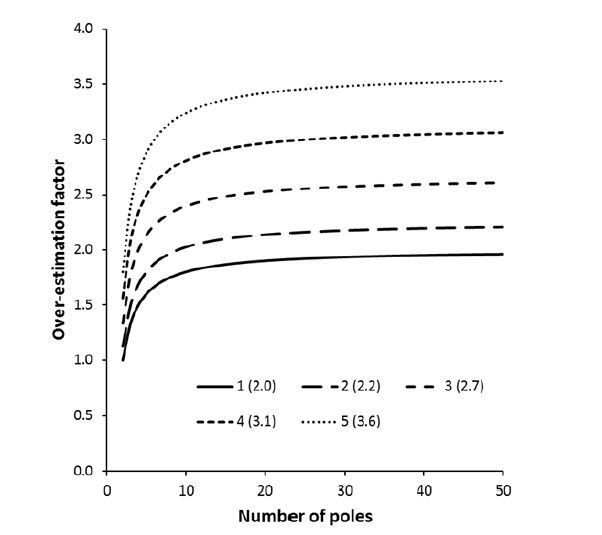
The intersection method will identify many more feasible intersections than the circle method since it includes all discontinuity poles. The circle method restricts discontinuity poles to those which lie within a zone capable of forming wedges and can be further restricted to those discontinuities which dip outward from the slope face ^[22]. A simple comparison can be made between the two methods for the case where only poles meeting the requirements of the circle method are included in analysis. The data are considered to be in two sub-sets with opposing apparent dip on the slope face. With this limitation and Equation (1) and Equation (2), the proportion of wedges found by the intersection method compared to the circle method can be determined (Figure 5). The relationship rapidly moves to an asymptote of 2.0 for an equal distribution of the two sub-sets. For an unequal distribution of 1:5 between the two sub-sets the difference between the intersection and circle methods reaches an asymptote of 3.6. The minimal value of 2.0 represents the characteristic that the circle method does not include any pairs of discontinuities which would have the same apparent dip on the exposed face, which halves the number of wedges identified (Equation 3). With regard to uneven distributions of poles in each hemisphere of the stereograph (relative to the plane perpendicular to the slope face) the difference between the two methods becomes greater. These values represent the minimum difference between the methods as the intersection method does not restrict which poles are included in the analysis.

Graphical representationof the minimum over-estimation of wedges identified in the intersection method compared to the circle method. Curves represent various ratios (1 to 5) of abundance between two sub-sets of data on each hemisphere of the stereograph. Asymptotic values (at 1000 poles) is given in brackets with the key.

---

5. Discussion

The features of each of the rock-related slope instability case studies is summarised in Table 1. The case studies represent a range of slope types mainly differentiated by slope angle. For the slopes at Guinsaugon in the Philippines, some parts of the slope are steep when compared to hillslopes generally, while other parts of the slope are at the low end of slopes where large masses of rock are involved. The frictional strength of the rock mass, or the frictional properties of the rock discontinuity interfaces if they are unfavourably oriented, form a lower limit to the instability of rock slopes. The structural data showed that the sliding wedges assessed to be the critical failure mechanism were close to the frictional strength of the discontinuities. The p-circle method was used to show this relationship stereographically.

The slopes at Sydney, Australia are very steep and include wedge sliding but are dominated by toppling. The toppling assessment using p-circles allowed consideration of planar and wedge toppling over a wide range of slope directions, simultaneously.

The mine at Tarkwa, Ghana, like most open pit mines, has been excavated at slope angles close to the limit of stability. Mine slopes comprise steep faces of stepped benches within a less steep overall slope angle. Geotechnical mine design involves selection of slope angles that will be sufficiently stable relative to the orientation of weak structures (including combinations of structures such as wedges) in the rock mass. The assessment of the data as p-circles showed that wedges occur on a range of slope angles from approximately 50° to 80°. Together with other geotechnical data, this information informs mine slope design and is the main influence of probability of failure of the slope. It was observed in the data that the intersection method identifies a significantly greater number of wedges and would lead to more conservative mine slope designs.

In each of the cases described here the mechanism of failure has been assessed using stereographic methods. The assessment is followed by more detailed analysis of slope stability — not included in this review. However, the stereographic stability assessment provides information on the nature of rock-slope failures and first-order information on the likelihood of their occurrence. This information is essential to an informed investigation of the risk presented by each slope.

---

6. Conclusions

Wedge-shaped blocks of rock bounded by two discontinuities within a rock mass represent an important type of hazard. These types of hazard are difficult to recognise compared to hazards involving a single dominant discontinuity. Awareness of wedge sliding is more advanced than awareness of wedge toppling processes. The conventional stereographic method for wedge failure mechanisms, both sliding and toppling, involves plotting the lines of intersections of the planes. The disadvantage of this method is that the lines of intersection are typically shown without reference to the planes which formed them. This makes it difficult to assess the shape of the wedge block which is an important contributor to its stability. Also, the intersection method does not incorporate a test to confirm that each intersection comprising two planes which both dip out of the slope face with opposing apparent dip directions in the slope face. The effect of this omission is that the number of intersections generated in such an analysis can be significantly greater than the number of wedges of practical significance, resulting in an exaggerated probability of failure.

In contrast, the recently published circle method ^[22] allows assessment of stability directly from the poles of the discontinuity planes. In this way individual planes and pairs of planes forming wedges can be assessed for sliding and toppling mechanisms for a range of slope orientations all within a small number of stereographic representations. The circle method also incorporates a test for the validity of discontinuities to form a wedge prior to considering the kinematic feasibility of the wedge. This test avoids the tendency of the intersection method to include intersections of discontinuities which may have similar dip directions and therefore not be capable of forming a wedge of practical significance.

The circle method of structural stability assessment has been shown to be an improved stereographic method of assessing failure mechanisms in a range of case studies including a large rock slide-debris avalanche in the Philippines, coastal cliffs in Australia and mining excavation slopes in Ghana, West Africa. The method illustrates how the critical failure mechanisms in rock slopes transition from sliding-dominated to mixed sliding and toppling to toppling-dominated as slope steepness increases.

---

Acknowledgements

Christian Arnhardt conducted fieldwork at the Guinsaugon landslide site in November 2010 as part of the Environmental and Rural Developement Program —Disaster Risk Management (EnRD-DRM) of the German Society for International Cooperation (GIZ).

---

Conflict of Interest

All authors declare no conflicts of interest in this paper.

---