1.
Introduction
The behavior between two species, predator and prey coexisting in the same environment, is a subject of study in ecology and several researchers have proposed mathematical models that describe the dynamics of both species. These models are usually described by systems of continuous ordinary differential equations
where u∈R2 is the size for each population, measured in number of individuals or density per unit area or volume at any instant t≥0, α∈Rp, with p≥1 and non-negative inputs, a vector of parameters and f:Ω⊂R2×Rp→R2 a continuous and differentiable function. The qualitative analysis of the model (1.1) determines the conditions in its parameters to achieve a possible stabilization in both species, or an extinction of at least one of them. This process is called bifurcation, where its possible cases were listed in [1].
In particular, Leslie-Gower models have been used to describe the dynamics of both species, which assumes that, in addition to the carrying capacity of the environment for the intrinsic growth of prey, the environmental carrying capacity in predators should be proportional to the abundance of prey [2]. However, several researchers have made modifications to the Leslie-Gower model, considering, for example, alternative food for predators, various functions describing the predator functional response, or harvesting for one or both species [3,4,5,6,7].
In the simplest case, if x(t),y(t)≥0 are the population sizes of prey and predators, respectively, whitch prey growth is subject to the carrying capacity of the environment K>0 and, the growth of predators under the quality of the utilization of consumed prey n>0 and of the possible alternative food available c>0 in the environment for new births, the dynamics of prey x(t) and predators y(t) is given by:
where r,s>0 are the intrinsic growth rates of prey and predators, respectively, q>0 is the harvest coefficient, E>0 the harvest effort, and a>0 the hunting success rate of the predator affecting the prey. In this case, it is assumed that predators can consume the prey without any limitation or resistance, so the predator functional response is linear [8], that is, h(x)=ax.
On the other hand, the effort of prey refuge from predators could play an important role in the stabilization of an ecological system, which generates an additional interest on the part of ecologists, and of which the effect of the reduction in the quantity of prey that will not be consumed by the predator is analyzed with respect to the different dynamics that could be presented by the model proposed.
In general, there are two types of prey refuge: the quantity of refuge is proportional to the population size of the prey [9,10,11] or it is a fixed quantity [12,13]. However, the qualitative analysis in predator-prey models when considering a proportion of refuged prey is equivalent to the model with no refuge [9,10], as opposed to considering a fixed quantity of refuge in the prey [14]. In particular, if 0<m<K denotes a fixed quantity of refuge for the prey, the model (1.2) is modified by:
where the initial prey condition x(0) must be greater than m, that is, m<x(0)≤K.
However, if x(0)≤m, prey and predators do not interact in the environment, so predators must feed only on resources provided by the environment and the growth of both species must be modeled by logistic differential equations of the form
In particular, the model (1.3) for m<x≤K and the model (1.4) for 0≤x≤m can be generalized by systems of discontinuous differential equations, generally known as Filippov's planar systems [15,16], described by
where fi:Si×Rp→R2, with 1≤i≤k, are continuous functions in an open and non-overlapping region Si, separated from Sj by a differentiable curve Σij. The discontinuous models (1.5) are called Filippov systems, whose bifurcation cases, which in addition to the possible cases shown in [1] for vector fields fi, were listed by Kuznetsov [15].
In addition, since there are species, such as cardumens [17,18], that hide from the predator when their population size is critical, so that the predator is forced to change its diet to avoid extinction [19,20,21], and when the desired population size is exceeded again, they leave their hiding place to search for food or explore the environment, and become accessible to the predator again [22,23,24], researchers have focused additional interest in proposing mathematical models that describe such interaction between prey and predators, when the predator functional response is deactivated if the population size of prey is below a threshold value M>0 [25,26,27,28,29].
However, and in contrast to discontinuous models proposed [25,26,27,28,29], by assuming that there are a quantity of prey that do not hide from the predator when it is below its critical population size M>0, for example, because they move away from other species to explore the environment undetected, we are interested in analyzing the behavior between prey and predators when a fixed quantity of prey m>0 is protected by being below its critical value M>0. Therefore, and given that there are species with a linear predator functional response [8,30], in this paper we propose, and perform a local and global qualitative analysis, a discontinuous Lesli-Gower model of the form,
where
and 0<m<M<K. In this case, if m<x<M then the prey is protected by a refuge constant m>0, and from which they become accessible to the predator if x>M. However, if x<m, prey are totally protected from the predator, so predators must subsist on alternative food provided by the environment.
For this purpose, the Section 2 presents the necessary tools to carry out a qualitative analysis of a Filippov system [15,16,28,29]. In Sections 3–5, a qualitative and bifurcation analysis is performed on the models (1.3), when m∈(0,K) and m=0, and (1.4) respectively, and whose results are used to perform the local and global analysis of the Filippov systems (1.6), shown in Section 6. A contrast to the Filippov systems (1.6) is performed with a bifurcation analysis with respect to the parameters M,E>0.
2.
Preliminaries
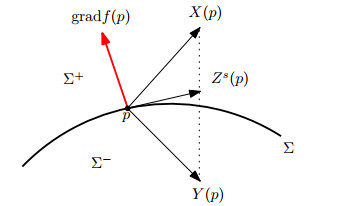
Let a planar Filippov system Z=(X,Y) a vector field in an open set U⊂R2 defined by
where X,Y are vector fields of class Cr, with r>1, and Σ={(x,y)∈U:f(x,y)=0,gradf(x,y)≠0}, with f:U→R a function of class Cr, is a differentiable curve that divides U into two open regions
If p=(x,y)∈Σ+, the local trajectory φZ(t,p) in Z=(X,Y), with initial point in p, is defined by trajectory φX(t,p) in the vector fields X. Analogously, if p=(x,y)∈Σ−, then φZ(t,p)=φY(t,p), where φY(t,p) is the trajectory in the vector fields Y.
However, a trajectory φZ(t,p) must also be defined for p=(x,y)∈Σ. The following result determines a division of Σ that depends on the behavior of the vector fields X and Y.
Definition 1. Let Xf(p)=⟨X(p),gradf(p)⟩ and Yf(p)=⟨Y(p),gradf(p)⟩, with p=(x,y)∈Σ, then Σ is divided into three disjoint regions given by:
● Crossing region: Σc={p∈Σ:Xf(p)⋅Yf(p)>0},
● Sliding region: Σs={p∈Σ:Xf(p)<0,Yf(p)>0},
● Escaping region: Σe={p∈Σ:Xf(p)>0,Yf(p)<0}.
By the Definition 1, for the case in which there exists p∈Σ such that Xf(p)=0 or Yf(p)=0, the following result defines the tangent point at Z=(X,Y).
Definition 2. If p∈∂Σc∪∂Σs∪∂Σe such that Xf(p)=0 or Yf(p)=0, where ∂Σc,e,s are the boundaries of the regions Σc,e,s, the point p is called tangency point, and it can be classified as:
● quadratic if Xf(p)=0 and X2f(p)=⟨X(p),gradXf(p)⟩≠0, or Yf(p)=0 and Y2f(p)=⟨Y(p),gradYf(p)⟩≠0. A quadratic tangency p∈Σ is regular if Xf(p)=0, X2f(p)≠0 and Yf(p)≠0; or Yf(p)=0, Y2f(p)≠0 and Xf(p)≠0. For the first case, a regular quadratic tangency is visible if X2f(p)>0 and invisible if X2f(p)<0. For the second case, p∈Σ is visible if Y2f(p)<0 and invisible if Y2f(p)>0.
● cubic if Xf(p)=X2f(p)=0 and X3f(p)=⟨X(p),gradX2f(p)⟩≠0 or Yf(p)=Y2f(p)=0 and Y3f(p)=⟨Y(p),gradY2f(p)⟩≠0.
We will now define the trajectory φZ(t,p) for a initial point p in Σc, Σs or Σe. According to Filippov's method [15,16,28,29], the trajectory φZ(t,p) with p∈Σs∪Σe is given by a convex combination of the vector fields X and Y tangent to Σ, that is,
In view of the Figure 1, the sliding vector field Zs is defined below.
Definition 3. The sliding vector field Zs is given by
defined in Σe∪Σs. For p∈Σe∪Σs, the local trajectory φZ(t,p) of p is given by this vector field. The point p∈Σs∪Σe is called pseudo-equilibrium if Zs(p)=0.
Keeping in mind this background, the trajectory φZ(t,p) in Z=(X,Y) is defined as follows.
Definition 4. Let φX and φY the trajectories in the vector fields X and Y defined for t⊂I∈R, respectively. The local trajectory φZ in Z=(X,Y) through a point p is defined as follows:
● For p∈Σ+ or p∈Σ− such that X(p)≠0 or Y(p)≠0 respectively, the trajectory is given by φZ(t,p)=φX(t,p) or φZ(t,p)=φX(t,p) respectively, for t⊂I∈R.
● For p∈Σc such that Xf(p),Yf(p)>0, and taking the origin of time at p, the trajectory is defined as φZ(t,p)=φY(t,p) for t⊂I∩{t≤0} and φZ(t,p)=φX(t,p) for t⊂I∩{t≥0}. For the case Xf(p),Yf(p)<0, the trajectory is defined as φZ(t,p)=φY(t,p) for t⊂I∩{t≥0} and φZ(t,p)=φX(t,p) for t⊂I∩{t≤0}.
● For p∈Σe∪Σs such that Zs(p)≠0, the trajectory is given by φZ(t,p)=φZs(t,p) for t∈I⊂R, where Zs is the sliding vector field given in (2.1).
● For p∈∂Σc∪∂Σs∪∂Σe such that the definitions of trajectories for points in Σ in both sides of p can be extended to p and coincide, the trajectory through p is this common trajectory. We will call these points regular tangency points.
● For any other point φZ(t,p)={p} for all t∈I⊂R. This is the case of the tangency points in Σ which are not regular and which will be called singular tangency points and are the critical points of X in Σ+, Y in Σ− and Zs in Σe∪Σs.
● As observed in Figure 2(a), a regular periodic trajectory is a trajectory Γ={φZ(t,p):t∈R}, which therefore belongs to Σ+∪Σ−∪¯Σc such that φZ(t+T,p)=φZ(t,p) for some T>0.
● A limit cycle in Σ+, or in Σ−, is a limit cycle in Z=(X,Y) which is represented in Figure 2(b).
● A cycle in Z=(X,Y) is a limit cycle formed by the union of a sequence of curves γ1,⋯,γn, such that γ2k⊂Σs and γ2k+1⊂Σ+∪Σ−, where the arrival and departure points belong to the closures of γ2k and γ2k+1, respectively. Figure 2(c) is an example of a cycle where n=2.
● A pseudo-cycle is the union of a set of trajectories γ1,⋯,γn, contained in Σ+ or Σ−, such that the end point of some γi coincides with the end point of the next curve and the initial point of γi coincides with the initial point of the previous curve. Figure 2(d) shows a pseudo-cycle.
With the basic notions for Filippov systems, we can perform the qualitative analysis for the model (1.6). For this purpose, the qualitative analysis of the vector fields W, X and Y will be performed, the results of which are used to analyze the dynamics of the discontinuos model (1.6), where the discontinuity in the vector fields W and X will be analyzed, and subsequently the discontinuity of X and Y.
3.
Prey refuge model
Let x(t)≥0 and y(t)≥0 the population sizes of prey and predator, respectively, whose dynamics are represented by the model (1.3) and defined in the region of biological sense
Before performing a mathematical analysis to determine possible behaviors in the dynamics of prey and predators, one must verify that the model (1.3) is mathematically correct and biologically feasible. Indeed,
Lemma 1. The model (1.3) has a unique trajectory φX, with initial condition (x(0),y(0))∈Ωm. Moreover, Ωm is invariant.
Proof. Since the vector field (1.3) is continuously differentiable for all (x,y)∈Ωm, the existence and uniqueness of the trajectory φX is satisfied. On the other hand, it must be guaranteed that the trajectories φX of the model (2.1) do not escape from Ωm. For this purpose, the change in the trajectories φX of the model (1.3) on the boundary is analyzed. Indeed, if x=m then ˙x=rm(1−mK)≥0 for all y≥0. Similarly, if x=K then ˙x=−a(K−m)y≤0 for all y≥0. On the other hand, if y=0 then ˙y=0 for all x≥0. If y=n(K−m)+c, we have that ˙y≤−qE[n(K−m)+c]≤0 for all 0≤x≤K. Therefore, the trajectories φX do not cross the boundary of Ωm.
Lemma 2. The trajectories φX of the model (1.3) are uniformly bounded.
Proof. Note that
which corresponds to a logistic differential equation, that is,
with x(0)∈Ωm the initial condition of the solution x(t). Consequently, x(t)≤K for all t≥0.
On the other hand, note that,
If S=n(K−m)+c, we have that y(t)≤S. Thus, when considering the function W(t):R+→R+ given by
then 0≤W(t)≤K+S and the trajectories φX of the model (1.3) are uniformly bounded.
3.1. Local and global qualitative analysis
The equilibrium in the model (1.3) on the x-axis is given by P1=(K,0)∈Ωm. Moreover, the model (1.3) could have a maximum of two interior equilibriums P3,4=(x1,2,y1,2), with
and x1,2 as positive solutions of the polynomial Ax2+Bx+C=0, with
that is,
To determine the number of interior equilibrium, we proceed by analyzing the following cases.
● Case c−mn>0: If s−qE≤0, the model (1.3) does not exhibit interior equilibriums, since y1,2<0. If s−qE>0, then A>0 and C<0, so the model (1.3) has an interior equilibrium P3=(x1,y1).
● Case c−mn=0: The model (1.3) has an interior equilibrium P3=(x1,y1) if s−qE>0 and B<0.
● Case c−mn<0: If ˙x=0, the model (1.3) has as an isocline
which is a positive and decreasing function for m<x<K, with asymptote at x=m.
If ˙y=0, the model (1.3) has as isoclines y=0,
and
Note that the function (3.5) intercepts with (3.6) at y=0, so the functions (3.4) and (3.5) do not intercept if s−qE≤0, and implies that the model (1.3) has no interior equilibriums. On the other hand, if s−qE>0, then the functions (3.4) and (3.5) intercept at a single point P3=(x1,y1). Figure 3 shows the behavior of the isoclines for the case where c−mn<0.
Therefore, the existence of the interior equilibrium P3∈Ωm is summarized in the following result.
Lemma 3. If s−qE≤0, the model (1.3) has no interior equilibrium. If s−qE>0, the model (1.3) has a unique interior equilibrium P3∈Ωm.
On the other hand, the following result determines the conditions on the local stability of the equilibrium on the model (1.3).
Lemma 4. Local stability of equilibriums P1 and P3 in the model (1.3).
1) If s−qE>0 then P1 is a saddle point. If s−qE<0, then P1 is a locally stable node.
2) If P3 is an interior equilibrium, then P3 is locally stable.
Proof. Indeed,
1) As the eigenvalues of the Jacobian matrix J(x,y) of the model (1.3) calculated in P1,
are given by λ1=−r<0 and λ2=s−qE. If s−qE<0 then P1 is a locally stable node. For s−qE>0, then P1 is a saddle point.
2) From the Jacobian matrix J(x,y) of the model (1.3) calculated in P3,
we have that
where A>0 and C<0 if c−mn>0 and, A,B,C>0 if c−mn<0, with A, B and C as described in (3.2). Let △m:=tr J(P3)2−4det J(P3). If △m<0, then P3 is locally a stable focus. If △m>0, P3 is locally a stable node.
Before calculating and determining the global stability of the possible equilibrium points of the model (1.3), the following result shows the non-existence of limit cycles in Ωm.
Lemma 5. The model (1.3) has no limit cycles in Ωm.
Proof. Let
and
Since
for all (x,y)∈Ωm, that is, for m≤x, by the Bendixon - Dilac criterion [31], the model (1.3) has no limit cycles in Ωm.
Consequently, since the model (1.3) has no limit cycles in Ωm invariant, as observed in Lemmas 1 and 5, in view of the Poincare - Bendixson Theorem [31] and the local stability of the equilibriums on the model (1.3), shown in Lemma 4, the following result shows conditions for establishing the global stability of the equilibriums P1 or P3 in Ωm.
Theorem 1. If s−qE<0, then P1 is a globally asymptotically stable node in Ωm. If s−qE>0, then P3 is a globally asymptotically stable equilibrium in Ωm.
3.2. Existence of a transcritical bifurcation
The model (1.3) has a transcritical bifurcation when P3 and P1 collide, which P1 transforms from a saddle point to a stable node and P3 changes from stable to non-existent at Ωm. The existence of the bifurcation is characterized by the existence of a null eigenvalue in the Jacobian matrix J(P1), that is, when s−qE=0, shown in the following result.
Theorem 2. If s−qE=0, then model (1.3) has a transcritical bifurcation around P1.
Proof. Let F(x,y,s) and Fs(x,y,s) as the vector field Y and its derivative with respect to s, respectively, that is,
and V, W as the eigenvectors associated with the null eigenvalue in the Jacobian matrix J(P1) and J(P1)T, that is,
If s=s0:=qE, by the transcritical bifurcation Theorem [32], three conditions must be guaranteed:
1) WTFs(P1,s0)=0: Since Fs(P1,s0)=(0,0)T, the result is guaranteed.
2) WT[DFs(P1,s0)V]≠0: Since DFs(P1,s0)V=(0,−1)T, then WT[DFs(P1,s0)V]=1>0.
3) WT[D2F(P1,s0)(V,V)]≠0, where V=(v1,v2)T: Since
then WT[D2F(P1,s0)(V,V)]=2n(K−m)+c>0.
Therefore, the model (2.1) has a transcritical bifurcation at s=qE.
3.3. Bifurcation diagram
We analyze the cases in which the parameters m and E can modify the phase diagrams of the model (1.3) using the results found by the qualitative analysis presented in Subsection 3.1. Indeed, in Figure 4 we observe all possible dynamics of the model (1.3) in Ωm with respect to the bifurcation curve in the plane (m,E), shown in Figure 4(a).
In this case, and as observed in Figure 4(b)–(d), the phase portraits in Ωm representing each bifurcation region, shown in Figure 4(a), are given by:
● In region Ⅰ, the trajectories φX, regardless of the initial condition (x(0),y(0))∈Ωm, converge to the equilibrium P1 in Ωm. Note that P3∉Ωm as seen in Figure 4(b).
● In regions Ⅱ and Ⅲ, the equilibrium P3∈Ωm and the trajectories φX converge to P3, where P3 is a node in region Ⅱ and a focus in region Ⅲ. Figure 4(c) and (d) show the dynamics of the model (1.3) in Ωm for regions Ⅱ and Ⅲ, respectively.
4.
Non-constant refuge of prey
If the refuge constant for prey to be consumed by the predator is removed, i.e., when m=0, the dynamics of prey x(t) and predators y(t) is given by the model (1.2) defined in the biological sense region
The following result determines that the model (1.2) is mathematically correct and biologically feasible, and that the trajectories φW are uniformly bounded, whose proof is equivalent to that shown in Lemmas 1 and 2 and will be omitted for brevity.
Lemma 6. The model (1.2) has a unique trajectory φW, with initial condition (x(0),y(0))∈Ωm. Moreover, Ω0 is invariant and the trajectories φW of the model (1.2) are uniformly bounded.
4.1. Local and global qualitative analysis
Before calculating and determining the local and global stability of the possible equilibrium points of the model (1.2), the following result shows the non-existence of limit cycles in Ω0.
Lemma 7. The model (1.2) has no limit cycles in Ω0.
Proof. Let
and h(x,y) defined in (3.8). Since
for all (x,y)∈Ω0, by the Bendixon-Dilac criterion, the model (1.2) has no limit cycles in Ω0.
On the other hand, the equilibriums in the model (1.2) on the (x,y) axes are given by: ¯P0=(0,0), ¯P1=(K,0) and ¯P2=(0,c(s−qE)s) if s−qE>0. Let
if s−qE>0 and 1<ϕ, the model (1.2) has an interior equilibrium ¯P3=(¯x,¯y)∈Ω0, with
The following result determines the conditions on the local stability of the equilibrium on the model (1.2).
Lemma 8. Local stability of equilibriums ¯P0, ¯P1, ¯P2 and ¯P3 in the model (1.2).
1) If s−qE>0, then ¯P0 is a locally unstable node. If s−qE<0, then ¯P0 is a saddle point.
2) If s−qE>0, then ¯P1 is a saddle point. If s−qE<0, then ¯P1 is a locally stable node.
3) Let s−qE>0. If ϕ<1, then ¯P2 is locally a stable node. If 1<ϕ, then ¯P2 is a saddle point.
4) If ¯P3 is an interior equilibrium, then ¯P3 is locally stable.
Proof. Indeed,
1) From the Jacobian matrix ¯J(x,y) of the model (1.2) calculated at ¯P0, that is,
we have as eigenvalues: λ1=r>0 and λ2=s−qE. Consequently, if s−qE>0 then ¯P0 is a locally unstable node. However, for s−qE<0, then ¯P0 is a saddle point.
2) As the eigenvalues of the Jacobian matrix ¯J(x,y) of the model (1.2) calculated in ¯P1,
are given by λ1=−r<0 and λ2=s−qE. If s−qE<0 then ¯P1 is a locally stable node. If s−qE>0, then ¯P1 is a saddle point.
3) From the Jacobian matrix ¯J(x,y) of the model (1.2) calculated in ¯P2,
we have as eigenvalues: λ1=ϕ−1s and λ2=−(s−qE)<0. Therefore, if ϕ<1, then ¯P2 is locally a stable node. If 1<ϕ, then ¯P2 is a saddle point.
4) From the Jacobian matrix ¯J(x,y) of the model (1.2) calculated in ¯P3,
we have that
Therefore, ¯P3 is locally stable. Let ¯△:=tr ¯J(¯P3)2−4det ¯J(¯P3). If ¯△<0, then ¯P3 is locally a stable focus. If ¯△>0, ¯P3 is locally a stable node.
Consequently, and equivalently to what is shown by Theorem 1, the following result shows conditions for establishing the global stability of the equilibriums ¯P1, ¯P2 or ¯P3.
Theorem 3. If s−qE<0, then ¯P1 is a globally asymptotically stable node. If s−qE>0 and ϕ<1 then ¯P2 is a globally asymptotically stable node. If s−qE>0 and 1<ϕ, then ¯P3 is a globally asymptotically stable equilibrium.
In particular, model (1.2) has two transcritical bifurcations as observed in the following result, whose proof is analogous to Theorem 3.2.
Theorem 4. Existence of transcritical bifurcation in the model (1.2).
1) If s−qE=0, the model has a bifurcation around ¯P1.
2) If ϕ=1 and s−qE>0, the model has a bifurcation around ¯P2.
4.2. Bifurcation diagram
We analyze the cases in which the parameters K and E can alter the behaviors in the trajectories of the model (1.2). Indeed, Figure 5 we observe all possible dynamics of the model (1.2) with respect to the bifurcation curve in the plane (K,E), shown in Figure 5(a), whose phase portraits representing each bifurcation region are described as follows:
● In region Ⅰ, as observed in Figure 5(b), ¯P2,3∉Ω0 and the trajectories φW, regardless of the initial condition (x(0),y(0))∈Ω0, converge to the equilibrium ¯P1∈Ω0.
● In regions Ⅱ and Ⅲ, the equilibriums ¯P2,3∈Ω0 but φW converges to ¯P3, where ¯P3 is a node in region Ⅱ and a focus in region Ⅲ as observed in Figure 5(c) and (d), respectively.
● In region Ⅳ, ¯P3∉Ω0 and φW converges to ¯P2 as seen in Figure 5(e).
5.
Non-predatory model
In contrast to the assumptions made for the formulation of the model (1.3) with x(0)<m, if the prey x(t) are protected at all times from being consumed by the predator, the dynamics between the two species is given by the model (1.4) defined in the biological sense region
The following result, without proof, shows the non-existence of limit cycles in the model (1.4), so there are no periodic trajectories in the dynamics of x(t) and y(t).
Lemma 9. In the model (1.4) there are no limit cycles on ˜Ω.
On the other hand, the model (1.4) has as equilibriums ˜P0=(0,0) and ˜P1=(K,0). However, if s−qE>0, the model (1.4) has two additional equilibrium given by ˜P2=(0,c(s−qE)s) and ˜P3=(K,c(s−qE)s). Local and global stability of each equilibrium point are shown in the following results, whose phase portraits are observed in Figure 6.
Lemma 10. If s−qE<0, that is, if ˜P2,3∉˜Ω, the equilibrium ˜P0 is locally a saddle point and ˜P1 is locally a stable node. If s−qE>0 we have that ˜P1,2 are locally a saddle point, ˜P3 and ˜P0 are locally a stable and unstable node, respectively.
Theorem 5. If s−qE>0, then ˜P3 in the model (1.4) is globally asymptotically stable. If s−qE<0 then ˜P1 is globally asymptotically stable.
6.
Protection of prey subject to the threshold value
From the hypotheses proposed to relate the growth and interaction of prey and predators given in Sections 3–5, if a constant quantity of prey m>0 take refuge, which avoids being consumed by the predator, when its population size is lower than its critical value M>0, where 0<m<M<K, and does not maintain contact with the predator if x<m, the final dynamics between prey and predators is described by the discontinuous model (1.6), equivalent to:
where
To perform a qualitative analysis of the discontinuous model (6.1) we must calculate the equilibrium points, the regions Σs,e,c1,2 that divide Σ1,2 and, the sliding segment Zs1,2 in Σ1,2.
6.1. Local stability of equilibrium points
From the equilibrium in the vector fields W, X, Y, as observed in Section 4, 3 and 5 respectively, we have that the equilibriums of the discontinuous model (6.1) on the coordinate axes (x,y) are given by: ˜P0=(0,0)∈Σ−, ˜P2=(0,c(s−qE)s)∈Σ− if s−qE>0 and, ¯P1=(0,K)∈Σ+.
Moreover, if s−qE>0 and m<x1<M, the discontinuous model (6.1) has an interior equilibrium given by P3=(x1,y1)∈Σ∗, with x1 and y1 as described in (3.3) and (3.1), respectively. Also, if 1<ϕ and M<¯x, the discontinuous model (6.1) has another interior equilibrium given by ¯P3=(¯x,¯y)∈Σ+, with ¯x and ¯y as described in (4.3). The local stability of each equilibrium is shown in the Lemmas 4, 8 and 10.
However, the interior equilibriums P3 and ¯P3 do not coexist in the discontinuous model (6.1), as shown in the following result.
Lemma 11. If s−qE>0 and 1<ϕ, then P3 and ¯P3 do not coexist in the discontinuous model (6.1).
Proof. Since ac(s−qE)>a(c−2m)(s−qE), if 1<ϕ then ac(s−qE)>rs>a(c−2m)(s−qE) or ac(s−qE)>a(c−2m)(s−qE)>rs. In either case, ¯x<x1. Therefore, if M<¯x then ¯P3∈Σ+ and P3∉Σ∗. If ¯x<M<x1, then ¯P3∉Σ+ and P3∉Σ∗. If x1<M then ¯P3∉Σ+ and P3∈Σ∗.
6.2. Existence of pseudo-equilibrium and tangent points in Σ1
If f1(x,y)=x−M, then for all p∈Σ1,
Since r(K−M)aK<rM(K−M)aK(M−m), then
In addition, the sliding segment Σs1 has two tangent points given by:
where the tangent point ¯T is visible if W2f(¯T)=⟨W(¯T),gradWf1(¯T)⟩>0, that is,
and invisible if
Similarly, the tangent point T is visible if X2f1(T)=⟨X(T),gradXf1(T)⟩<0, that is,
and invisible if
The following result summarizes the behavior of the tangent points on the model, whose proof will be omitted.
Lemma 12. Let T and ¯T tangent points of the discontinuous model (6.1), with ϕ as described in (4.2).
1) If s−qE<0 then ¯T is visible and T is invisible to all m<M<K.
2) For s−qE>0,
(a) If ϕ<1, ¯T is invisible to all m<M<K. If 1<ϕ, ¯T is invisible if ¯P3∉Σ+, and visible if ¯P3∈Σ+.
(b) The tangent point T is visible if P3∈Σ∗, and invisible if P3∉Σ∗.
On the other hand, the vector field of the sliding segment Zs1(p)=(Zs1x,Zs1y)T, with p∈Σs1, is given by
with pseudo-equilibrium PN=(M,yp)∈Σs1, where
which corresponds to a stable pseudo-node because Zs1y>0 if y<yp and Zs1y<0 if y>yp.
The following result shows conditions for the existence of the stable pseudo-node PN.
Lemma 13. Conditions for the existence of the stable pseudo-node PN in the discontinuous model (6.1), with ϕ as described in (4.2).
1) If s−qE<0 then PN∉Σs1.
2) For s−qE>0,
(a) If ϕ<1, then PN∈Σs1 if, and only if, P3∉Σ∗.
(b) If 1<ϕ, the pseudo-nodo PN∈Σs1 if, and only if, P3∉Σ∗ and ¯P3∉Σ+.
Proof. Note that PN∈Σs1 if, and only if, r(K−M)aK<yp<rM(K−M)aK(M−m), that is, rs(K−M)<aK(nM+c)(s−qE) and rsM(K−M)>aK[n(M−m)+c](M−m)(s−qE). In this case, if s−qE<0, then PN∉Σs1. However, if s−qE>0, PN∈Σs1 if, and only if,
and
with A, B and C as described in (3.2).
Therefore, if ϕ<1 then (6.2) is obtained for all M>0 and (6.3) is satisfied if, and only if, ¯P3∉Σ+. However, if 1<ϕ, then PN∈Σs1 if, and only if, ¯x<M<x1, that is, P3∉Σ∗ and ¯P3∉Σ+.
In summary, and in view of the results shown in Lemmas 12 and 13 it follows that if ¯P3∈Σ+, then ¯T is visible, T is invisible and Σs1=→¯TT. On the other hand, if P3∈Σ∗, then ¯T is invisible, T is visible and Σs1=←¯TT. Conversely, if PN∈Σs1, then T and ¯T are invisible and Σs1=→TPN←PN¯T.
6.3. Non-existence of pseudo-equilibrium and tangent points in Σ2
In this case, if f2(x,y)=x−m, then for all p∈Σ2,
and Σ2=Σc2, that is, the trajectories φV with initial condition (x(0),y(0))∈Σ− must cross Σ2 and reach Σ∗, so there are no pseudo-equilibriums and tangent points in Σ2.
6.4. Global stability of equilibrium and pseudo-equilibrium
It is important to determine the global stability of the equilibriums ¯P1, P3, ¯P3 and of the pseudo-equilibrium PN. Indeed, for the case where the pseudo-equilibrium PN∈Σs1, the trajectories φV of the discontinuous model (6.1) converge to PN for all (x(0),y(0))∈Σ−∪Σ2∪Σ∗∪Σ1∪Σ+, and thus PN is globally asymptotically stable, as observed in the following result, whose proof was inspired by [28,33,34].
Theorem 6. If PN∈Σs, then PN is globally asymptotically stable.
Proof. Since PN∈Σs1 is locally stable, ˜P0∈Σ− is unstable, P1∈Σ+ and ˜P2∈Σ− are saddle points, P3∉Σ∗ and ¯P3∉Σ+, to guarantee the global asymptotic stability of PN five conditions must be proved:
1) There are no limit cycles in Σ+, Σ∗ and Σ−: By the Lemmas 7, 5 and 9 the non-existence of limit cycles in Σ+, Σ∗ and Σ−, respectively, is guaranteed.
2) There is no periodic trajectory for discontinuous model (6.1) which contains a part of Σs1: Since the pseudo-equilibrium PN∈Σs1 is stable, there is not closed orbit for discontinuous model (6.1) containing a part of Σs1.
3) There is no periodic trajectory which contains Σ− and Σ∗: Since Σ2=Σc2 and from the dynamics of the vector field Y, formed by systems of autonomous and independent differential equations, no closed trajectory can be formed by unions of trajectories in Σ∗ and Σ−.
4) There is no periodic trajectory which contains Σ−, Σ∗, Σs1 and Σ+ : Analogous to the previous reasoning.
5) There is no closed trajectory which contains Σ∗, Σs1 and Σ+: Indeed, if it is assumed that there is a closed trajectory Γ of discontinuous model (6.1), which passes through Σ1 and encloses Σs1, let Q and N as the intersection points of Γ and Σ1, respectively, as observed in Figure 7. Similarly, let Q1=Q+a1(θ) and N1=N−a2(θ), with intersection points of Γ and the line x=M−θ, respectively, and Q2=Q+b1(θ) and N2=N−b2(θ) as the intersection points of Γ and x=Q+θ, where θ>0 is sufficiently small. Moreover, a1,2(θ) and b1,2(θ) are continuous with respect θ and a1,2(θ),b1,2(θ)→0 when θ→0.
As shown in Figure 7, the Γ1 and →N1Q1 is locate in the region Σ∗. Similarly, the Γ2 and →Q2N2 is locate in the region Σ+. Furthermore, the dynamics of discontinuous model (6.1) in region Σ+ is represented by (4.1) If ∂Σ+ denote the boundary of Σ+, by using Green's Theorem [31], with h(x,y) as in (3.8), we have
where dx=¯f(x,y)dt, dy=¯g(x,y)dt, and there is no change of x in →Q2N2, then
Similarly, if the dynamics in Σ∗ is represented by (3.7), by Green's Theorem,
Suppose that Σ∗10⊂Σ∗ and
From Lemma 5, and based in (6.6), we have
As observed in Figure 7, if θ→0 in the sum of (6.4) and (6.5) we have that
Then (6.7) holds, which contradicts to (6.8). Thus there is no closed trajectory containing Σs1 and PN. Therefore, PN is globally asymptotically stable.
Similarly, if ¯P3∈Σ+, by Theorem 4.1 and Lemmas 11 and 13, it follows that the trajectories φV of the Filippov system (6.1) converge to ¯P3 for all (x(0),y(0))∈Σ−∪Σ2∪Σ∗∪Σ1∪Σ+. Therefore, the following result shows that the equilibrium ¯P3 is globally asymptotically stable, whose proof was inspired by [28,33,34].
Theorem 7. If ¯P3∈Σ+, then ¯P3 is globally asymptotically stable.
Proof. Since PN∉Σs, ˜P0 is unstable, ¯P1 and ˜P2 are saddle points and P3∉Σ∗, to determine that ¯P3 is globally asymptotically stable five conditions must be proved:
1) There are no limit cycles in Σ+, Σ∗ and Σ−: By the Lemmas 7, 5 and 9 we guarantee the non-existence of limit cycles in Σ+, Σ∗ and Σ−, respectively.
2) There is no closed trajectory for discontinuous model (6.1) which contains a part of Σs1: Since Σ2=Σc2, ¯T is visible and T is invisible, any trajectory φV, with initial condition (x(0),y(0))∈Σs1, slides over Σs2 to ¯T and escapes Σ+, therefore, we will prove that the trajectory φV of discontinuous model (6.1) starting from the tangent point ¯T cannot enter the Σs1 again. Indeed, if the trajectory φV starting at ¯T, encircles ¯P3 and intercepts with ¯T forming a periodical trajectory Γ, then any φV with initial condition outside Γ will not be able to cross Γ, and certainly cannot converge to the equilibrium ¯P3, which contradicts the stability of ¯P3. Similarly, if the φV starting at ¯T and encircling the equilibrium ¯P3, intercepts Σs1 at some point other than ¯T, then ¯P3 must be unstable, which also contradicts to the statement that ¯P3 is a stable equilibrium. Therefore, there is no closed trajectory of discontinuous model (6.1) containing part of Σs1.
3) There is no periodic trajectory which contains Σ− and Σ∗: Analogous to that shown by the Theorem 6.
4) There is no periodic trajectory which contains Σ−, Σ∗, Σs1 and Σ+ : Analogous to that shown by the Theorem 6.
5) There is no closed trajectory which contains Σ∗, Σs1 and Σ+: This step is similar to that of Theorem 6, and we omit it here for brevity.
If s−qE<0 and ϕ<1, that is, if P3∉Σ∗ and ¯P3∉Σ+, by Theorem 1 and Lemmas 12 and 13, the trajectories φV of the discontinuous model (6.1) converge to ¯P1∈Σ+ as shown in the following result.
Theorem 8. If s−qE<0, then ¯P1∈Σ+ is globally asymptotically stable.
Proof. A similar procedure to that of Theorem 7 can be used to prove this theorem, and we omit it here for brevity.
6.5. Bifurcation diagram
Finally, we analyze the cases in which the parameters M and E can modify the phase diagrams of the discontinuous model (6.1) using the results found by the qualitative analysis presented in Subsection 6.1. Indeed, in Figure 8(b)–(g) show the changes in their phase portraits of the discontinuous model (6.1) with respect to the bifurcation curves in the plane (M,E), represented in Figure 8(a), described as follows:
● In region Ⅰ, ¯P1∈Σ+ is globally asymptotically stable, this is, φV converge to ¯P1, regardless of the initial condition (x(0),y(0))∈Σ−∪Σ2∪Σ∗∪Σ1∪Σ+, as observed in Figure 8(b).
● In regions Ⅱ and Ⅲ, represented in Figure 8(c) and (d) respectively, shows that φV converge to ¯P3∈Σ+, where ¯P3 is node in region Ⅱ and a focus in region Ⅲ.
● In region Ⅳ, shown in Figure 8(e), we have that φV→PN∈Σs1 when t→∞.
● In regions Ⅴ and Ⅵ, represented in Figure 8(f) and (g) respectively, shows that P3∈Σ∗ is a globally asymptotically stable equilibrium, which is a node in region Ⅴ and a focus in region Ⅵ.
7.
Conclusions
Since many researchers assume the existence of prey that completely hide from the predator when their population size is below a critical threshold, the proposed discontinuous predator-prey models focus on completely deactivating the predator functional response, which is generally assumed to be nonlinear to enrich the qualitative analysis of the model, if the population size of prey is below the critical threshold [25,26,27,28,29]. However, it could be the case that a constant population size of prey does not manage to hide from the predator, because, for example, they escape from the prey group unnoticed to explore the environment, so the predator functional response should not be deactivated, but modified in such a way that it considers the interaction between the population size of the predator and the unrefuged quantity of prey, where the predator functional response should not necessarily be considered as nonlinear [8,30].
Therefore, in this work a mathematical model was proposed, by means of a system of discontinuous differential equations, which represents the scientifically observable behavior between a pair of species, prey and predator. From the qualitative analysis of the models (1.2), (1.3) and (1.4), it is determined that the general behavior of the species described in the discontinuous model (1.6) remain in equilibrium over time and no extinction of any species occurs.
Regarding the qualitative analysis of model (1.2), the extinction of predators is determined when the intrinsic birth rate is less than or equal to the product between the harvest coefficient and the capture effort, that is s−qE<0. If this condition among its parameters is not met, the predator species does not become extinct and converges to an equilibrium. However, if the model (1.2) has no internal equilibrium for s−qE>0, the prey should become extinct over time. Moreover, the model (1.2) has two transcritical bifurcations, characterized by the collision of the interior equilibrium with respect to one of its equilibriums located on the coordinate axes, and subsequently, it causes a change in the stability of the equilibrium on the coordinate axis and the disappearance of the interior equilibrium in Ω0.
On the other hand, and with respect to the qualitative analysis of the model (1.3), this model makes biological sense if the initial population size of prey is greater than or equal to the quantity of refugia, i.e., m≤x(0). However, for the hypothetical case where x(0)<m, the initial condition in the model (1.3) is not constrained if c−mn≤0, otherwise, if the initial population size of prey is less than −(c−mn)n, the population size of predators grows exponentially, which is biologically absurd. To mitigate this problem, if the initial prey population size is less than m>0, the interaction between prey and predators ceases, and both species attain carrying capacity by logistic growth (1.4), with harvesting for predators, and in that case, the model (1.3) should be applicable when the growth of prey, without interaction with the predator, exceeds the constant refuge of prey.
Unlike the cases of bifurcations in the model (1.2), the model (1.3) has a transcritical bifurcation, associated by the collision, and subsequent change of stability, of the interior equilibrium and the only equilibrium located on the x-axis. The non-existence of the equilibrium on the y-axis in the model (1.3) is associated with the parameter of constant prey refuge m>0, which guarantees the non-extinction of prey. However, the predators described in the model (1.3) could become extinct if the condition of the parameters associated with the model (1.2) are satisfied. In addition, the bifurcation cases for the models (1.2) and (1.3) disagree on an additional bifurcation region in the model (1.2), characterized by prey extinction, that is s−qE>0 and ϕ<1, since m>0 prey refuge is not considered in the model (1.3). However, in both models, the predator may become extinct over time if s−qE≤0.
On the other hand, the transcritical bifurcation in the model (1.3) is transferred to the cases of bifurcations in the discontinuous model (1.6), so the predators, in the same way as in models (1.2) and (1.3), could become extinct if s−qE≤0, but the value of m>0 guarantees the non-extinction of prey in the discontinuous model (1.6), unlike the model (1.2) in which prey can become extinct over time if s−qE>0 and ϕ<1. For the case where the equilibrium P3 or ¯P3 collides with Σ, a Σ-node bifurcation occurs and the pseudo-equilibrium PN∈Σs1. Note that regardless of the parameter conditions in the discontinuous model (1.6), same as models (1.2) and (1.3), there are no limit cycles, so that both species could stabilize their growth over time. In addition, a sliding segment is formed in the discontinuous model (1.6) regardless of the alteration of its parameters, so there are trajectories that slide along the sliding segment and converge to equilibrium or pseudo-equilibrium.
Comparing the models (1.2) and (1.3) with the discontinuous model (1.6), we have that the bifurcation cases for the models (1.2) and (1.3) are added to the bifurcations in the discontinuous model (1.6) whenever s−qE>0 and P3, or ¯P3, is the unique interior equilibrium in the discontinuous model (1.6), that is, when x1<M, or 1<ϕ and M<¯x, which guarantees that both species subsist over time. Furthermore, if P3 is the only equilibrium in the discontinuous model (1.6), then the population size of prey is below the critical value M>0. Similarly, if ¯P3 is the only interior equilibrium, then the population size of prey is above the critical value M>0. However, if s−qE>0, ϕ<1 and x1>M, we have that PN exists, so the population size of prey converges to the critical value M. Similarly, if s−qE>0, 1<ϕ, x1>M and ¯x<M, then P3 and ¯P3 are not interior equilibriums in the discontinuous model (1.6), so the population size of prey converges to the critical value M>0.
Note that the predator functional response described in the discontinuous model (1.6), shown in Figure 9, could be viewed as a kind of sigmoid, or Holing Ⅲ type, if we ignore the discontinuity at x=M. Although predator-prey models with Holing Ⅲ predator functional response have limit cycles [35,36], it does not give guarantee to assume the existence of limit cycles in the discontinuous model (1.6). A possible formation of limit cycles in Filippov systems arises from the existence of limit cycles in one of the conforming vector fields, together with the possible collision of these limit cycles with the sliding segment Σs, or cycles crossing Σc, as observed in Figure 2. Similarly, and naturally for continuous dynamical systems, the existence of at least one stable limit cycle could be deduced when all equilibriums of the discontinuous model are unstable [26,29].
For the case where all prey take refuge from the predator when they are below the threshold value M>0, the predators cannot consume the prey and the discontinuous model (1.6) is re-formulated by
where
of which has a single tangent point ¯T and a stable pseudo-equilibrium PN. Moreover, the bifurcation cases are equivalent to that shown in Figure 8(a), in which region Ⅳ is interposed over regions Ⅴ and Ⅵ, so that there are only 4 different behaviors in the dynamics between prey and predators. In this case and independent of the alterations on the parameters in the discontinuous model (7.1), the population size of prey could not be below the critical value M>0 and the predators would lead to extinction if s−qE<0.
Similarly, if there are a population size of prey m>0 that is refugeed from the predator at all times, the predator, upon consuming the remaining prey, must subsist solely on alternative food provided by the environment. Thus, the discontinuous model (1.6) is modified by:
where
and the bifurcation cases are equivalent to those shown in Figure 4. Unlike the model (1.3), the discontinuous model (7.2) does not provide any constraint with respect to the behavior between the initial prey population size and quantity of prey refuges, i.e., m<x(0) or x(0)>m.
Acknowledgments
This research was supported by MCIN/AEI/10.13039/501100011033 through grant BADS, no. PID2019-109320GB-100, and the associated FPI contract PRE2019-088899 to Christian Cortés García. The Spanish MICINN has also funded the "Severo Ochoa" Centers of Excellence to CNB, SEV 2017-0712.
Conflict of interest
The author declare there is no conflict of interest.









 DownLoad:
DownLoad: