1. Introduction
Photosynthesis
a divided into two types: oxygenic and anoxygenic. In oxygenic photosynthesis, which is carried out by plants and cyanobacteria, oxygen is produced. However, anoxygenic photosynthesis, which is carried out by various, types of green and
purple bacteria and produces no oxygen [1]. The origin of oxygenic
photosynthesis is estimated to be in the range of 3.4 to 2.3 billion years ago
where the oxygen-rich atmosphere of the earth today was initiated [2]. Oxygenic
photosynthesis involves two large membrane protein complexes, photosystems Ⅰ
and Ⅱ (PS Ⅰ and PS Ⅱ), which perform the first step in the conversion of the
light absorbed from the sun into chemical energy and by producing molecular
oxygen and consuming carbon dioxide.
Photosystems contain the reaction centers (the sites where
photochemical reactions occur) which are typically divided into groups. PS Ⅱ
has a quinone type reaction center (known as Type Ⅱ), while PS Ⅰ has an
iron-sulphur (FeS) type reaction center (type Ⅰ). Moreover, photosystems are
connected by an intermediate Cytochrome b6f (Cytb6f)
super-complex and two electron carriers, the plastoquinone (PQ) that transports
electrons between PSⅡ and Cytb6f, and the plastocyanin (PC) which links
Cytb6f to PSI [3]. On the other hand, non-oxygenic phototrophic
organisms generally contain either a Type Ⅱ reaction center (e.g. purple
Bacteria) or a Type Ⅰ reaction center (e.g. green-sulphur or Heliobacteria)
[1]. A common organization has been assigned for the two photosystems, however, functionally they are organized in two main moieties: a core complex and a
peripheral antenna system (light harvesting antenna). During evolution, core
complexes
have been well conserved evident by the fact that most of the subunits are
similar in prokaryotic and eukaryotic photosystems. In contrast, the light
harvesting antenna is highly variable where it is composed of peripheral
associated membrane proteins in cyanobacteria, the phycobilisomes, and integral
LHC membrane proteins in eukaryotic cells [4].
During
photosynthesis, the light harvesting antenna to drive electron transport from
the low-potential electron donor water to the high-potential electron
end-acceptor NADP+ absorbs light. ATP and NADPH representing energy
and reduction equivalents are generated which will be subsequently used in carbon
dioxide fixation and reduction in the Calvin-Benson cycle, the dark reaction
(Figure 1). Changes in abiotic factors such as the intensity and quality of
light, the nutrient, the temperature and the water availability have a great
impact on photosynthetic efficiency and thereafter on plant yield. When the
photon utilization capacity of the chloroplast is overloaded with the photon
fluence rate, photosynthesis become inhibited and irreversible damage of the
reaction centers i.e. PS Ⅱ occurs [5,6]. Moreover, elevated excitation
pressure increases the production of ROS that will cause damaging effects on
protein, lipids and enzymes necessary for the function of the chloroplast and
the cell [7,8]. For a long time, ROS accumulation was solely considered
indicator of deleterious effects that is termed oxidative stress [9,10].
However, this concept where ROS cause indiscriminate damaging effects became
undoubtedly anchored in the literature and the signaling function of ROS was
raised as a fundamental principle in cellular communication [11]. Genetic
evidence has demonstrated unequivocally that ROS are signaling molecules with
important and specific roles in gene expression regulation and that a balance
between redox metabolism and ROS formation is needed and that's why cells
operate a redox signaling network [12,13]. The network senses environmentally
induced redox imbalances and initiates compensatory responses either to
readjust redox homeostasis and/or to repair oxidative damage [14]. ROS and
redox signals are among several signals that comprise the retrograde signaling
pathways (signals that originate in the organelles, e.g. chloroplast and
mitochondria, which modulate nuclear gene expression (NGE). Despite decades of
research work on retrograde signaling and the clear ultimate effects on NGE, the initiation and transmission of the signals, as well as their mode of action
still a subject of controversy debate [15].
The
regulation of gene expression by oxidants, antioxidants, and the redox state
has emerged as a novel sub-discipline in molecular biology that has promising
wide implications for different organisms. Several redox-dependent mechanisms
that control the activity of TFs and that control transcription have been
investigated in detail in bacteria and mammals. However, only
in the past
two decades redox-responsive transcription factors and co-activator proteins
have emerged in plants. In this review, we will introduce the recent progress
that has been made in plants to identify transcription factors whose activities
are directly or indirectly regulated by redox as well as redox-dependent
mechanisms that control transcription.
2. Redox
signal transduction from the chloroplast to the nucleus (retrograde signaling)
and its impact on gene expression
Chloroplasts
and mitochondria evolved and became part of the eukaryotic cell through
endosymbiosis. During the course of such evolution dramatic reduction in the
genome size of these organelles occurred and these organelles became dependent
on their eukaryotic host. Currently, the plant plastid genome encodes ~80
proteins and the mitochondrial genome encodes ~57 proteins in the organelles
and the rest of ~4000 chloroplast and ~1000 mitochondrial proteins are
nuclear-encoded and imported into the organelles from the cytosol [16,17].
Because the organellar genomes encode now a limited number of proteins and the
vast majority of the genes are encoded in the nucleus, there is a requirement
for coordinated expression of organellar and nuclear genomes to ensure correct
assembly of complexes that contain proteins encoded in each genome [18]. From a
historical point of view, the first report, indicating the presence of
retrograde signaling and describing the existence of communication between the
chloroplast and the nucleus, has been provided by Bradbeer et al [19]. In that
report Bradbeer et al. have shown severe decrease in chloroplast protein
synthesis associated with decreased expression of nucleus-encoded chloroplast
genes using the barley (Hordeum vulgare) chloroplast ribosome-deficient mutant
[19]. Subsequent reports using inhibitors of plastid protein synthesis (e.g.
streptomycin, lincomycin and chloramphenicol) and various plant species (e.g.
mustard, barley, pea and Arabidopsis) confirmed the findings in Bradbeer et al.
[20,21,22,23].
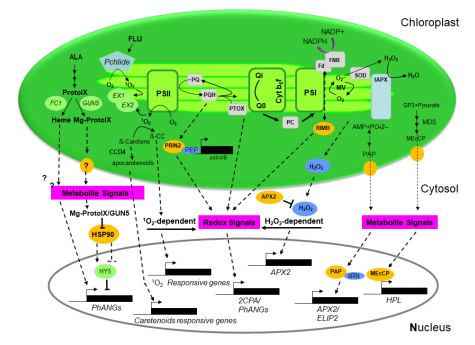
Recent
advances in recent years have identified several putative retrograde signals
and signaling pathways (Figure 2). Pogson et al. classified them into two
groups: "biogenic signals" required in the early stages of chloroplast
development and "operational signals" in mature plants to cope with the
changes in the environment [24]. Some of these retrograde signals include
metabolites and metabolic intermediates. Others include photosynthesis-dependent
signals [25,26]. In this section we will briefly concentrate on the metabolic
retrograde signals and discuss in details the redox-dependent signal
transduction pathways.
2.1. Metabolite dependent signals impact on nuclear gene expression
Several
metabolites, which are synthesized in the chloroplast and then translocated
into the cytosol or to other cellular compartments, have been suggested to be
involved in chloroplast-to-nucleus communication. The classical metabolite
signaling pathway is associated with tetrapyrrole biosynthesis intermediates
[27]. More recently, secondary metabolites such as the isoprenoid precursor
methylerythritol cyclodiphosphate (MEcPP) [28], 3′-phosphoadenosine
5′-phosphate (PAP) [29], oxidation products of carotenes, such as the volatile
β-cyclocitral [30], and heme [31] have also been discovered and proposed as
retrograde plastid signals which affects NGE in plants.
2.1.1. Tetrapyrrole
intermediates: the classical retrograde signals that affect expression of
photosynthesis-associated nuclear genes (PhANG) in green algae and plants
Mg-ProtoporphyrinIX
(Mg-ProtoIX), a tetrapyrrole biosynthesis intermediate in the synthesis of the
chlorophyll photosynthetic pigments, have been proposed to act as a plastid
signal that regulate the expression of PhANG in algae and plants [32,33,34,35].
Isolation of genomes uncoupled (gun) mutants was very helpful in deciphering
the retrograde signaling during chloroplast biogenesis [36]. In gun mutants the
expression of Light Harvesting Complex b (LHCB) nuclear gene is maintained
following chloroplast damage using the herbicide norflurazon (NF) treatment
[36]. So far, six guns mutants have been identified and four of them (gun 2–6)
have a mutation/lesion in enzymes involved in or associated with tetrapyrroles
biosynthesis [34,36,37,38]. Gun 1 mutant results from a mutation in a gene
encoding a chloroplast-localized pentatricopeptide repeatcontaining protein
(PPR) that binds nucleic acids [36,39]. The GUN2, GUN3, and
GUN5 encode heme oxygenase, phytochromobilin synthase, and the H subunit of Mg-chelatase
(CHLH), respectively [39], while GUN4 is a regulator of Mg-chelatase activity
[38].
During
oxidative stress e.g. after NF treatment Mg-ProtoIX accumulates in the cytosol
and act as a mobile signal mediating chloroplast regulation of PhANG [34,40].
However, the specific accumulation of Mg-ProtoIX has been challenged due to the
lack of correlation between the metabolite levels and gene expression [41,42].
It is interesting that Zhang et al. reported that oxidative stress induced by
NF can induce transient accumulation of tetrapyrroles with concomitant
repression of LHCB in adult plants re-addressing the importance of Mg-ProtoIX
accumulation in the regulation of PhANG. Interestingly, the authors have shown
that following exposure to oxidative stress (NF treatment) accumulation of
Mg-ProtoIX/Mg-ProtoIX-ME was rapid and transient [35]. Similar findings were
also shown for Mg-ProtoIX/Mg-ProtoIX-ME transient accumulation after methyl
viologen (MV) treatment [43].
Although
the movement of Mg-ProtoIX into the cytosol is debatable [34,40,41,42], a possible
mechanism of action for Mg-ProtoIX have been described by Kindgren et al. [43].
Mg-ProtoIX binds the cytosolic heat shock 90-type proteins (HSP90) both in
vitro and in vivo [43,44], and that the oxidative stress-triggered GUN phenotype is partially suppressed
when HSP90 is down-regulated [43]. Moreover, HY5 was suggested to be part of the GUN5/HSP90 signaling pathway which
regulates PhANG expression [43]. This is analogous to the regulatory
HSP70-HSP90-HAP1 complex in yeast that responds to heme accumulation and
controls the oxidative response [45].
2.1.2. Methylerythritol cyclodiphosphate (MEcPP) is a retrograde signal that affect
expression of stress-associated nuclear genes in plants
One
major biosynthetic pathway in plants is the isoprenoid metabolism [46]. The
isoprenoid precursor methylerythritol cyclodiphosphate (MEcPP) was suggested as
a plastid-derived retrograde signal regulating HPL nuclear gene [28]. In a
genetic screen designed to identify genes involved in the regulation of
hydroperoxide lyase (HPL), a stress-inducible nuclear gene encoding a
plastid-localized protein in the oxylipin pathway, constitutively expressing
HPL (CEH1) mutant was isolated [28]. The conversion of MEcPP to hydroxymethylbutenyl
diphosphate (HMBPP) catalyzed by CEH1, which encodes
1-hydroxy-2-methyl-2-(E)-butenyl4-diphosphate synthase (HDS), is the major step
in the methylerythritol phosphate (MEP) pathway [47]. CEH1 mutant results in
high levels of MEcPP accumulation, increases in stress-related salicylic acid
(SA), increased resistance to the Pseudomonas pathogen and induced the
expression of a subset of stress-associated genes, including ISOCHORISMATE
SYNTHASE 1, a key plastidial enzyme in SA-biosynthetic pathway, and HPL.
Moreover, high light and wounding abiotic stresses increased MEcPP levels which
induce the expression of the aforementioned genes [28], demonstrating MEcPP
involvement in a retrograde pathway involved in abiotic stresses (operational
control) which is distinct from the chlorophyll intermediates (gun) signaling
pathway. Indeed, NF treatment of WT and ceh1 seedlings resulted in reduced
levels of LHCB transcripts and photo-bleaching. In addition, ceh1 mutant do not
alter PhANG expression [28]. This is distinct from the gun mutants which have
high levels of LHCB expression in response to NF treatment [21].
2.1.3. SAL1-PAP
act as a retrograde signal during high light and drought
stress
In a
screen for elevated expression of the antioxidant enzyme ascorbate peroxidase
(APX2) under low light (LL) and high light (HL), the alx8/sal1 mutant was
isolated [48]. In alx8/sal1 mutant 25% of the HL stress inducible genes e.g.
APX2 and Early Light Induced Protein 2 (ELIP2), are up-regulated. Moreover, sal1 showed altered metabolome and 50% increased survival under water limiting
conditions [48,49].
SAL1 enzyme, which is localized in both the chloroplast and the mitochondria, is an inositol polyphosphate 1-phosphatase which regulates the steady-state
level of 3'-phosphoadenosine 5'-phosphate (PAP) by dephosphorylating it to an
adenosine monophosphate (AMP). Interestingly, PAP accumulated 20-fold more in
the sal1 mutant and increased by up to 30-fold in wild type plants in response
to drought and HL stresses. Accordingly, PAP accumulation triggered changes in
the expression of APX2 and ELIP2 nuclear genes [50]. The demonstrated PAP
movement between the chloroplast and nucleus [50] probably through the PAPS/PAP
chloroplastic antiporter that might be responsible to facilitate the exchange
of PAP between the chloroplast and cytosol [51]. PAP most likely regulates
nuclear gene expression by altering RNA metabolism mediated by
5-3'exoribonucleases (XRNs) [50]. There are two nuclear-localized XRNs, XRN2
and XRN3 homologues to the yeast Xrn2p/Rat1p. The sal1 and
xrn mutants have been shown similar morphological phenotypes [52]. By
repressing the activity of XRNs, PAP may stimulate expression of HL and
drought-responsive genes, leading to increased tolerance [50].
2.1.4. Carotenoid
derived retrograde signaling
Because 1O2
is highly reactive and have short half-life it is unlikely that it functions as
a signal that is translocated to the chloroplast envelop, but rather it
interacts with a chloroplastic component/molecule, likely close to its site of
production, and to act then as a signaling molecule [53]. In
plants, β-cyclocitral (β-CC), one of the carotenes oxidation products in the
chloroplast act as a stress signal that mediate gene responses to singlet
oxygen (1O2) [30]. Eighty percent of gene expression
profiles of the flu mutant (1O2-responsive genes) [54], are similar to those genes affected in the β-CC plants [30], suggesting that
β-CC is an intermediate in the 1O2 signaling pathway.
Moreover, the effects of β-CC appear to be specific because the related
molecule β-Ⅰ, a volatile derivative of β-carotene that is accumulated in
response to light stress induced oxidation of the carotenoid β-carotene, did
not reveal similar results [30]. The β-carotene
volatile is a lipid soluble compound which makes it able to cross lipid
membranes and therefore it's a strong candidate to function is retrograde
chloroplastic signal. Its exact mechanism of action needs further investigation, however at this stage it seems that its impact on nuclear gene expression is
independent from the 1O2 signaling pathway within the
chloroplast which depends on EXECUTER1 and 2 (EX1/2) [54].
2.1.5. Heme, a derived retrograde
signaling, regulates nuclear gene expression in algae and
higher plants
Heme, another product of tetrapyrrole biosynthesis, acts as a retrograde signal from
the chloroplast to regulate nuclear gene expression in both algae and higher
plants [31,55,56]. Feeding experiments with Mg-ProtoIX and heme triggered
global changes in the gene expression in Chlamydomonas reinhardtii (almost 1000
genes changed their expression level significantly upon feeding of Mg-ProtoIX
or heme) [55]. In Arabidopsis a gain-of-function genetic screen using activation-tagging
mutagenesis was used and a candidate mutant (gun 6-1D) was identified [31]. The gun 6-1D
encodes and overexpresses the plastid Ferrochelatase 1 (FC1, heme synthase)
which leads to the accumulation of PhANGs in the presence of NF. The responses
obtained seems to be specific to the activity of FC1 because over-expression of
the other chloroplast-localized FC2 did not increase PhANG expression [31].
Biochemical and genetic experiments suggest that increased flux through the
heme branch of the plastid tetrapyrrole biosynthetic pathway increases PhANG
expression. Moreover, a model was proposed in which heme is exported from
chloroplasts to increase PhANG expression. There is no correlation between the
gun phenotype and the free heme levels indicating that the signaling heme may
be bound to specific targets [57]. One possibility is that heme interacts with cytosolic
or nuclear factors similar to the yeast [58]. The other is that heme interacts
with heme-binding proteins to regulate gene expression.
2.2. Photosynthesis
dependent signals modulate nuclear gene expression
In the context of photosynthesis, chloroplasts act both as
source and target of redox regulation [59]. Initiators of retrograde signaling
have been proposed for diverse components of the chloroplast. Among them
components of the photosynthetic electron transport chain (PETC) [60]. Photosynthetic electron transport (PET) requires the
absorption of light by chlorophyll. Then excitation of the pigments and
electron transfer reactions in an oxygen rich environment lead to the
production of ROS. Moreover, changes in the redox state of PETC trigger retrograde
signaling. The photosynthesis redox-dependent signals are classified into the
redox state of the plastoquinone (PQ) pool, the redox state of the acceptor or
the donor side of PSI and the accumulation of ROS (1O2, O2– and H2O2)
[60,61,62,63].
2.2.1. The
redox state of PQ pool, an early proposed retrograde signal in the control of
NGE
More
than two decades ago photosynthetic control of nuclear and plastid gene
expression has been linked to the redox state of PQ pool. First studies have
been demonstrated for cab genes in green algae such as Dunaliella salina and
Chlorella vulgaris [64,65]. Inhibitors of electron transport such as 3-(3′, 4′-dichlorophenyl)-1, 1-dimethyl
urea (DCMU) and 3-methyl-6-isopropyl-p-benzoquinone (DBMIB) and different light
intensities were used to modify the redox state of the PQ pool. A threefold
increase in the amount of cellular chlorophyll occurred in Dunaliella cells
within 24 hours of transfer from high light (HL) to low light (LL). More
studies with Dunaliella salina and Chlorella vulgaris demonstrated similar
acclimatory responses in which photosynthetic adjustment is regulated by the
redox poise of inter system electron transport [66,67]. Not only expression of
photosynthesis genes encoded in the nucleus, but also expression of some
photosynthetic genes encoded in the chloroplast was regulated by the redox
state of PQ pool in green algae and higher plants [68]. Analysis of tobacco
plants using specific PS-specific light sources (PSI and PSⅡ light) and DCMU
and DBMIB inhibitors suggested that the redox state of the PQ pool affects also
PhANG (e.g. plastocyanin (petE) gene) in higher plants [69]. Additionally, Oswald
et al. [70] investigated
the relationship between PET and sugar signaling using transgenic Arabidopsis
lines carrying luciferase reporter genes driven by CAB2 promoter or petE
promoter. The transcriptional activation of PhANG was found to be dependent on
PET but not on the sugar status of the cells which suggested that a redox
signal from the plastid overrides the sugar-related expression of PhANG [70].
However, subsequent analysis of cyanobacteria and Arabidopsis using the same
PSI and PSⅡ light and inhibitors, demonstrated that the redox state of the PQ
pool was not the major source of the HL mediated plastid signal [71,72,73]. In
Arabidopsis only 54 genes were redox regulated directly by the reduction state
of PQ and only 2 genes encoded components directly associated with
photosynthesis suggesting a weak indication for PQ-dependent regulation of
genes for light-harvesting complex proteins [72]. Using microarrays and
metabolic profiling to study the role of the redox state of the PQ, Brautigam
et al. demonstrated that the reductive redox signal has a faster kinetics than
the oxidative signal and that the effect on the transcriptome and metabolome
were not complementary [74]. These findings suggested the presence of two
different pathways involved in the redox state of the PETC. Although the redox
state of PQ is an important part of retrograde signaling, a much more complex
signaling mechanism is present rather than a simple switch between oxidized and
reduced PQ pool [74]. In addition to redox signals that are generated on the
acceptor side of PSⅡ through the action of PQ, Cytb6f complex appears to be
critical for redox regulation of genes involved in chlorophyll biosynthesis. The
accumulation of GSA, ALAD, HEMA, HLD, CPX1, CHLH1, CTH1 and CHLI transcripts is
lost upon illumination of Cytb6f Chlamydomonas mutants [75]. Although at this
stage little is known about the specific elements involved, it is suggested
that the Cytb6f complex activity through quinole binding to the complex impacts
on the activity of STT7 and STN7 protein kinases (which are required for state
transitions) [76]. Evidence about PETC redox-dependent retrograde signals that
regulate the expression of a large number of genes involved in stress response, metabolism and gene expression was also provided through stn7 [77] but no significant differences in the
expression levels of PhANGs excluding the STN7 kinase direct effect on the
transcription of PhANGs in Arabidopsis. Moreover, several hundred
miss-regulated genes, in plants lacking the STN7 kinase and mutants with severe
PETC over-reduction, were recently identified using Meta-analysis of several
studies on NGE [78].
2.2.2. The
acceptor availability at PSI, the previously ignored retrograde signal
In heterotrophic organisms and non-photosynthetic
compartments of plant cells, thioredoxins (Trx) reduction is provided by NADPH
in a reaction catalyzed by an NADPH-dependent Trx reductase (NTR) [79]. In
contrast the ferredoxin-thioredoxin reductase /thioredoxin (FTR/Trx) system has
been considered the only pathway regulating redox reactions in chloroplasts of
photosynthetic plant cells [80]. Redox signals are generated on the acceptor
side of PSI through the action of ferredoxins (Fdx), Trx, and NADPH. Trxs are
reduced via the FTR in a light-dependent manner, using photosynthetic electrons
provided by the PSI electron acceptor Fdx, and are able to reductively cleave
disulfide bonds in many stromal target proteins. Thus, modulate their functions
i.e. to increase or decrease their activity. Chloroplast Trxs, such as Trx-m, Trx-f, Trx-y, Trx-x and Trx-z [81], chloroplast glutaredoxins (Grx) such
as GrxC5, S12, S14, and S16 [82] and chloroplast cyclophilins [83,84] mediate
thiol-disulphide redox interchange of various chloroplast proteins like
fructose-1, 6-bisphosphase (FBPase), a key enzyme of the Calvin–Benson cycle and
the most studied target enzyme of the CO2 assimilation pathway [85], NADP-dependent malate dehydrogenase (NADP-MDH) a key enzyme of the malate valve
and represents a classical target of m-type
Trxs [86], and peroxiredoxins which are central elements of the antioxidant
defense system and the dithiol-disulfide redox regulatory network of the plant
cell [87,88,89]. In the chloroplast, in addition to the light-dependent Fdx/Trx
system, a separate Trx system which uses NADPH as electron donor has been
identified. This system consists of NADPH-dependent Trx reductase (NTRC) and
contains both NTR and a Trx domain on the same protein [90]. Target enzymes of
NTRC are AGPase involved in starch biosynthesis [91], the nuclear-encoded 2-Cys
peroxiredoxins (2CP) involved in H2O2 detoxification
[92], and Mg-chelatase Ⅰ sub-unit [93]. At first NTRC was suggested to function
in the chloroplast in a light-independent manner, as a separate thiol-redox
system. However, recent finding in planta suggested that NTRC is able to
regulate chloroplast FBPase and the Calvin-Benson cycle in response to varying
light conditions [94,95]. Although NTRC is a key redox protein responsible for
regulatory functions distinct from those of the FTR/Trx system, it was found as
an electron donor to Trx-z, an important regulator of chloroplasts gene
expression (Figure 1). Moreover, the cooperative control of chloroplast
functions via the FTR/Trx and NTRC pathways which is essential for plant
viability has been demonstrated [96].
Until
the last decade the role of carbon metabolism in chloroplast-mediated signals
in nuclear gene expression regulation has been ignored in most studies. Piippo
et al. used short-term illumination with different qualities (PSⅡ light that
preferentially excites photosystem Ⅱ and PSI light that excites predominantly
photosystem Ⅰ) and quantities (darkness, low light and high but not excessive
light) of light to modulate the redox state of PETC [73]. In their expirements
emphasis was focused on the role of the redox state of the PQ pool, the stromal
redox compounds, and the CO2 fixation products as sources of
chloroplast signals during the short term treatment period. The genes
responding to different light treatments found in their study were among genes
involved in protein synthesis and carbohydrate metabolism. Hence, they
suggested that the metabolic activity of the chloroplast could be a source of plastid signals whereas the role of the redox
state of the PQ pool in nuclear gene expression appears to be only marginal
[73]. Another line of support to these findings came from a study by Baier et
al. [97] who reported that the acceptor availability at PSI and the cellular
redox poise but not the redox state of the PQ pool control the 2-Cys
peroxiredoxin A (2CPA) transcription activity within the nucleus. In all plants
analysed so far the expression of 2CP was found to be redox-regulated [87].
Among several enzymatic components of the chloroplast antioxidant system the
most detailed analysis of expressional/transcriptional regulation has been
performed for 2CPA gene [98,99,100]. Moreover, a screen for redox-imbalanced
(rimb) mutants was set up in Arabidopsis inorder to identify further elements
involved in transcriptional regulation of genes for chloroplast
antioxidant enzymes [101] using a reporter gene line expressing luciferase
under control of the 2CPA promoter [98]. In the rimb mutants, the expression of
2CPA is uncoupled from the redox state of the PSI acceptor side. Identification
of the RIMB genes will impact on our understanding of the redox-mediated
retrograde signaling pathway(s). Indeed, recently the rimb1 mutation was mapped
to a 167 kb region on chromosome1 containg candidate genes and compared the
phenotypes of T-DNA insertion lines with rimb1. RIMB1, a major factor involved
in redox-regulation of genes for chloroplast antioxidant enzymes and protection
against photooxidative stress, was identical to the radical-induced cell death
1 (RCD1), a regulator of disease response reactions and cell death [102].
2.2.3. ROS-dependent
signals in the retrograde signaling from chloroplast to the nucleus
HL has
been demonstrated to increase the production of ROS and to induce a strong
expression of various antioxidant genes, cellular chaperones and heat shock
proteins [103,104]. The role of H2O2 as signaling
molecule in the regulation of gene expression networks in plants has been given
special attention and was the focus of several studies [10,105,106,107,108,109,110]. In
Arabidopsis exposure to H2O2 resulted in a change of ~1/3
of the transcriptome [111]. Additionally, 113 genes were induced and 62 genes
were repressed from those 175 genes regulated by H2O2
[107]. Studies of genes involved in H2O2 signal
transduction, mitogen-activated protein kinases (MAPKs) and various TFs such as
NAC, ZAT, and WRKY families have been identified [112,113]. Moreover, by studying
catalase-deficient Arabidopsis plants, Vandenabeele et al. [114] have shown
that H2O2 was inferred to regulate the expression of
genes encoding specific small heat shock proteins and several TFs. The question
arises: as a signaling molecule to what extent it is able to diffuse out of the
compartments and to cross organelle membranes? This is still debatable and
there is no general agreement on the rate and concentration of H2O2
production in the cell different compartments [115]. However, Henzler and Steudle
and Bienert et al. proposed that H2O2 is able to diffuse
through membranes, possibly through aquaporins [116,117] and later on
Mubarakshina et al. [118] demonstrated that part of the chloroplast-derived H2O2
diffuses out of the chloroplasts which might be sufficient to trigger signaling
processes i.e. by a change in the expression level of responsive genes.
However, it was hypothesized that H2O2 itself is unlikely
to be the retrograde signaling molecule that directly regulates expression of
nuclear-encoded chloroplast genes but rather compartment-specific
redox-sensitive components such as oxidized proteins/peptides would act as
second messengers or down-stream mediators of such H2O2
signaling [119,120]. Most recently, stromules have been proposed to have a role
in the translocation of proteins and H2O2 from the
chloroplast to the nucleus and to be part of retrograde signaling [121].
To study the specific role of 1O2
as a signaling molecule the conditional Arabidopsis flu mutant was used. In the
flu mutant induction of 1O2 is allowed in the plastids in
a controlled manner. FLU is a nucleus-encoded plastid protein which acts as a
negative regulator of the synthesis of δ-aminolevulinic acid (ALA) in the
tetrapyrrole biosynthetic pathway (the chlorophyll (Chl) biosynthesis) [122].
Inactivation of this protein in the flu mutant demonstrates an
over-accumulation of protochlorophyllide (Pchlide) in plastids upon
transfer to the dark. However, shifting back to the light Pchlide acts as a
photosensitizer and generates 1O2. The mature flu plants
stopped growing after the release of 1O2, the seedlings
bleached and died and the differential regulation of specific sets of nuclear
genes [54]. It was also suggested that the 1O2 pathway is
active under moderate light stress conditions which could be part of the
acclimation response because of the limited cell death occured. Another report
demonstrated high levels of 1O2 production in response to
norflurazon and lincomycin treatments.
Therfore, 1O2 molecule was suggested to be a putative
signal for the modulation of NGE in response to these inhibitors supporting a
link between 1O2 and GUN1 and ABI4 transcription factors
because this regulation is no longer observed in gun1 and abi4 mutants [42].
Screens for flu suppressor mutations that abrogate 1O2-mediated
cell death and/or growth inhibition of flu revealed the identification of EXECUTER1
(EX1). EX1 encodes a plastid protein of unknown function and unrelated to known
proteins. Moreover, EX1 was shown to be indispensable for the transfer of 1O2
dependent signals that mediate growth inhibition and cell death from the
plastid to the nucleus. Inactivation of the EX1 gene in the flu mutant
background is not sufficient to fully suppress 1O2‐induced
changes in nuclear gene expression i.e. inactivation of EX1 attenuated the
upregulation of 1O2‐responsive nuclear genes but did not
fully eliminate the changes [123]. Another plastid nuclear‐encoded protein, EX2, has been identified and is also implicated in the changes in signalling of
1O2‐dependent nuclear gene expression [124]. When both
EXECUTER proteins are inactive in the ex1/ex2/flu triple mutant, most of the 1O2‐responsive
gene transcripts are close to wild‐type level suggesting that the singlet
oxygen derived plastid signal requires concerted action of both EXECUTER1 and
EXECUTER2 [124].
Another suppressor screen with the flu mutant have led to the identification of dubbed singlet oxygen-linked
death activator (soldat) mutants that abrogate 1O2-dependent
cell death without affecting Pchlide accumulation. Soldat8 affects a gene
encoding the SIGMA6 factor of the plastid RNA polymerase (PEP) while soldat10
affects a gene encoding a plastid-localized protein related to the human
mitochondrial transcription termination factor mTERF [125,126]. On the one hand
inactivation of SIGMA6 in soldat8 mutants disturbed plastid homeostasis, non-photochemical quenching capacity was reduced drastically and light
sensitivity of young soldat8 seedlings was enhanced. Moreover, Suppression of 1O2-mediated
cell death in young flu/soldat8 seedlings caused by the initial disturbance of
plastid homeostasis seems to be due to a transiently enhanced acclimation at
the beginning of seedling development [125]. On the other hand the soldat10
mutation does not interfere directly with the transfer of 1O2-derived
signals but disturbs chloroplast homeostasis and suppresses a subsequent
1O2-mediated cell death response in flu seedlings [126].
In short, constitutive acclimation to light stress results in the suppression
of 1O2-mediated cell death in soldat8 and soldat10
mutants [125,126]. In the above sections we mentioned that plastids may act as
sensors of stress and via plastid-to-nucleus signaling they trigger stress
responses, therefore, it is not surprising that the perturbation of plastid
homeostasis in soldat mutants is perceived as stress and triggers an
acclimatory response.
Overall, by comparing the transcriptional responses to 1O2 and H2O2
using the flu mutants and plants treated with MV, transcriptome analyses have differentiated
O2–/H2O2-dependent
regulation from 1O2-dependent regulation and demonstrated
the specific and the antagonistic effect of these two chloroplastic ROS on gene
expression [54,106,111]. However, cross-talk between H2O2-and 1O2-dependent signaling pathways was proposed in
which it might contribute to the overall stability and robustness of plants
exposed to adverse environmental stress conditions [106].
3. Compartmentalisation
is a major factor in ROS/redox signal specificity
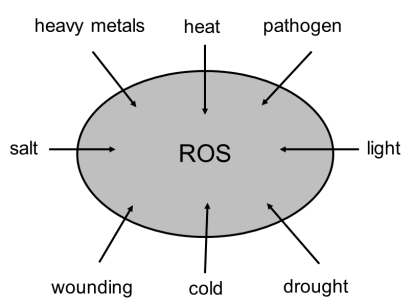
Because
ROS, which is produced in different sites of the cell, represents a common
plant response to almost all environmental stresses (Figure 3) it drives
someone's thinking that it could be the primary source of the signaling battery
in plants. There are different sources for the production of ROS within the
cell such as the plasma and apoplastic membranes, and in the organelles such as
the chloroplasts, mitochondria, and peroxisomes (Figure 4).
The
compartmentalization of ROS production within cells, aside from the specificity
and selectivity of ROS on their targets, is an important determinant of whether
damage or redox signaling occurs. H2O2-dependent
oxidation of a given protein is likely to occur close to the source of H2O2
production so an effective redox signaling can take place. A good example is
the protein targets of H2O2 generated from plasma
membrane NADPH oxidases is likely also located at the plasma membrane. In a
similar way, superoxide accumulation in the mitochondrial matrix has different
outcomes from superoxide accumulation in the cytosol. Accordingly, both the
type of ROS and its local concentration collectively determine whether redox
signaling or oxidative-stress-induced damage occurs [59,60,61]. Thus, for
understanding cell redox physiology, ROS generation within specific subcellular
compartments and their redox status appear to be of major importance.
Additionally, more and more methods for the study of redox compartmentalization
have been developed. For example, GSH/GSSG and NAD(P)H/NAD(P)+ redox
potentials are quantified by high-performance liquid chromatography (HPLC).
However, in order to determine the redox state of several proteins, mass
spectrometry and redox Western blotting, in association with labeling of free
thiols, are frequently used. Despite the high specificity present in these
methods for the redox couple examination and the quantification of both the
oxidized and reduced forms, they often require tissue/cell fractionation.
Fractionation can result in redistribution and artifactual oxidation/reduction.
Molecular biology techniques using epitope-tagged versions of nuclear NLS and
NES proteins have been developed to allow measurements without fractionation.
Approaches involve fluorescent imaging techniques of ROS and major redox
couples within organelles in intact cells or organisms in vivo have been
developed more recently.
3.1. ROS/REDOX
signal initiation and signal transduction in plants and its impact on NGE
A linear
signaling pathway pattern for each stress is unlikely due to the increasing
evidences of cross-talk between different signaling pathways. However, if ROS
are the points at which different branches of signaling pathways converge then
what are the ROS receptors/sensors? the downstream signaling components? the
signaling specificity? and the cross-talk between the different signaling
pathways? A signal transduction pathway consists of several components starting
from the stimulus sensing (sensor/receptor), then the transducer (transmitter)
and to the final response.
3.1.1. The
receptors or sensors of the redox signals in plants
To
activate signaling pathway(s) H2O2, O2–, and 1O2, as
signaling molecules, must interact with a cellular target (receptor/sensor).
The life time of ROS is quite short and therefore, the sensor has to be quite
efficient and this rises several possibilities about ROS sensing by the plant
cell which occurs possibly through different mechanisms (ⅰ) receptor proteins
that is yet not identified or may be at the apoplastic site of the plasma membrane
such as two members of the cysteine-rich receptor-like kinase gene family and a
leucine-rich repeat (LRR-RLK) protein in Arabidopsis which are
transcriptionally induced after ozone treatment, and thus they could sense ROS
through redox modifications of their extracellular domain [127]. Moreover, a
tight link between G proteins and ROS in plant signaling has been suggested in
which exposure of Arabidopsis leaves to ozone resulted in an oxidative burst
which was attenuated or even completely absent in mutant plants lacking Gα
protein or Gβ protein. The study suggested that the extracellular ROS which is
produced by ozone in the apoplastic part activate the G protein either directly
or indirectly [128]. In fact, NADPH oxidases D and F that receive initial
signals from G proteins to mediate ozone responses in Arabidopsis guard cells
was suggested [129]. Moreover, AtRbohD and AtRbohF, which are membrane-bound
ROS producing enzymes, work in the same pathway
with the Gβ subunit of the heterotrimeric G protein for full disease resistance
[130]. (ⅱ) Modulation of kinases directly by ROS. Mitogen-activated protein
kinases (MAPKs) exist in all eukaryotes and are considered to be evolutionary
conserved. MAPKs are interlinked protein kinases: MAP kinase kinase kinase
(MAPKKK), MAP kinase kinase (MAPKK), and MAP kinase (MAPK) where one activates
the other by phosphorylation of the tyrosine and therionine residues in the TXY
motif. The transmission of oxidative signals is controlled through MAPKs where
MAPKs can be activated by accumulation of H2O2, or they
can trigger an H2O2-induced oxidative burst [131]. (ⅲ) ROS activate signaling molecules within the
cell or the organelles i.e. redox sensitive compound such as transcription
factors such as WRKY, ZAT, RAV, GRAS and MYB. Regulation of TFs by ROS occurs
at several levels (ⅰ) TF expression up-regulation i.e. increasing mRNA
stability and translation; (ⅱ) decreasing TF degradation by inhibiting the
ubiquitin E3 ligase complex or by decreasing its association with this complex
(ⅲ) Translocation of TFs from for e.g. cytoplasm to the nucleus or by
releasing the TF from partners or from membrane anchors and (ⅳ) modulation of
DNA binding and/or transactivation potential of TFs, modulation of co-activators
or repressors, and by regulating chromatin to activate individual genes.
3.1.2. The
transmitters or transducers of the redox signals in plants
Trxs and
Grxs as well as the antioxidant enzymes are key players not only in keeping ROS
levels low but also may themselves act as signal transmitters [132]. Indeed, König et al. proposed that PRXs can be transducers in redox signaling in plants
and animals. They act as floodgates for H2O2 under strong
oxidative conditions [133]. The mammalian GPX7 functions as an oxidative stress
sensor and transmitter. When cells expose to oxidative stress, GPx7 acts as an
intracellular sensor that detects redox level and transmits ROS signals to
redox-sensitive, thiol-containing proteins to facilitate the regulation of
multiple biologic processes [134]. In addition, some TFs were also reported as
signal transmitters such as RAP2.4a which acts as an efficient redox-sensor and
transducer of the redox state of the cell to the nucleus to control
transcriptional activity of chloroplast antioxidant enzymes [99].
3.1.3. The
Responses to the redox signals in plants: the expressional/transcriptional
level
ROS/redox
networks play essential roles in the acclimation of plants to abiotic stresses.
A delicate balance of homeostasis is contributed by these signals within the
different organelles. Moreover, cross-talk between the different
organellar/cellular components is overseen by regulating different biological
pathways including gene expression, protein expression and modification for
e.g. phosphorylation, energy metabolism in response to stress. ROS-dependent
signal propagation as result of abiotic stresses i.e. HL, heat and wounding
reqiures RbohD [135]. Cross-talk between the regulatory mechanisms of
chloroplast redox signaling and systemic responses dependent on RbohD suggested
a great overlap between them although no direct evidence of interaction between
these responses was provided [135]. In response to HL and/or fluctuating light
changes in PQ pool redox state correlated with expression of antioxidant genes, pathogen defense genes, and thylakoid proteins phosphorylation. Dark and
norflurazon treatments (conditions which suppress chloroplast development)
resulted in amplified heat stress-induced apoptotic-like (AL) programmed cell
death (PCD) in Arabidopsis cell lines. Knock-out mutant plants dificient in
APX1 exhibited increased sensitivity to photo-oxidative stress [136]. Altered
gene expression of the stress-induced ZAT10 and ZAT12 in response to HL and
increased sensitivity to heat stress was reported in seedlings of abi4 and gun1
mutants which suggested that retrograde signalling could exert influence on
abiotic stress response and acclimation through the GUN1-ABI4.
4. Mechanisms of redox-regulated
transcription: examples from non-plant and plant organisms
Whereas redox-regulated transcription is
studied extensively in bacteria, yeast and animals (mammalian) systems, our
knowledge of redox-regulated transcription in plants is rather limited and only
emerging. Recent biochemical, molecular, physiological and genetic studies have
revealed parts of these mechanisms in plants and broadened our understanding of
how redox signals controlling transcription in plants. An elevation of a
specific redox signal may cause redox to either activate or repress the
activity of a redox-responding protein (e.g. protein kinase or phosphatase)
which then transfer the signal to a TF or it may directly affect target protein
like TFs, and thus cause it to activate or repress transcription of target
genes, depending on whether the TF itself is a transcriptional activator or
repressor. Some of the downstream target genes of such redox-regulated TF are
themselves transcription activators or repressors that affect the expression of
many more downstream genes. There are several redox-dependent mechanisms which
control TFs activity (ⅰ) redox-dependent conformational changes (ⅱ)
metal-S-clusters e.g. [Fe-S] cluster (ⅲ) subcellular localization and
compartmentation (ⅳ) disulfide formation and other Cys modifications such as
S-glutathionylation, S-nitrosylation (ⅴ) redox regulation of proteolytic
processes (ⅵ) regulation of PTMs such as phosphorylation, ubiquitination and
sumoylation (ⅶ) protein-protein interaction (ⅷ) direct redox control of
DNA binding [137,138]. The examples shown above are from non-plant and plant
systems and in the following sections will describe briefly the non-plant TFs
and in details plant TFs. Several of these TFs, their cis-element DNA binding
sites, their function and their mechanisms (Table 1) will be discussed.
Table 1. Cis-elements, transcription factors and their function in possible redox-mediated signaling
pathways.
| Allele |
Protein family/subfamily/domain |
cis-element/DNA binding site |
Function |
References |
| Athb-9 At1g30490 |
Homeodomain (HD) TFs/HD-zip Ⅲ class |
5'-CTAA(G/C)ATTAC-3' |
Role in plant developmental processes |
141,142 |
| Rap2.4a At1g36060 |
AP2/ERF-type TFs/ type Ib-ERF class |
CE3-like motif 5'-CACGCGATTC-3' |
Control of 2CPA and other antioxidant genes expression in response to oxidative stress |
56 |
| AtHSFA8 At1g67970 |
Heat shock factors (HSFs) |
HSE 5'-AGAAnnTTCT-3' |
Responses to abiotic stress |
147 |
| ANAC089 At5g22290 |
NAC family TFs |
5'-GCACGTCTAGT-3' |
Suppressor of stromal ascorbate peroxidase gene; control of ER-stress induced PCD in plants |
149 |
| TCP15 At1g69690 |
bHLH TFs |
as-1 5'-AATTCAGATCTGTGGGACCGGGAG-3' |
Modulation of anthocyanin biosynthesis |
154 |
| TGA1 At5g65210 |
bZIP-TFs/ TGA subclass |
as-1 5'-TCGAGCTGACGTAAGGGATGAC-3' |
Regulation of pathogenesis related (PR) genes during SAR |
159 |
| AtbZIP16 At2g35530 |
bZIP -TFs/G-group |
G-box 5'-CACGTG-3' |
Regulates early stages of Arabidopsis development |
163,168 |
4.1. Redox-dependent
conformational changes
• The
bacterial OxyR: a similar mechanism to the plant redox-regulated Rap2.4a
In the prokaryotic cell, e.g. bacteria, which lack the
presence of membrane-bound organelles, such as nucleus, chloroplasts, mitochondria
etc., a fast response is required and, therefore, it is obligatory to have
highly reactive proteins that are able to sense ROS/redox signals and to
trigger a response. During stress, bacteria initiate signaling that coordinate
the activation of TFs that control the expression of defense-regulated genes.
In response to H2O2, the redox-regulated TF OxyR, which belongs to the LysR family of TFs, control
the expression of the stress responsive genes such as catalase (KatG), alkylhydroperoxide reductase (AhpcF), glutaredoxin (Grx), glutathione reductase
(GorA), and the small regulatory RNA (OxyS) in response to elevated levels of
hydrogen peroxide. [139,140]. Among the six Cys residues in OxyR, Cys199 and
Cys208 are conserved and form a disulfide bond which leads to its activation in
responses to H2O2. Moreover, it was shown that the
tetrameric form of oxidized OxyR binds to target promoters [141,142,143]. No
apparent homologs of OxyR have been found in plants, however, the Arabidopsis
Rap2.4a TF similarly play a role in regulating the expression of
nuclear-encoded chloroplast antioxidant genes in response to oxidative stress.
Rap2.4a, which belongs to activator protein 2/ethylene response factor (AP2/ERF) TF family [144], was isolated from a
yeast-one-hybrid screen using a redox-sensitive DNA promoter region that
control the expression of 2-cysteine peroxidoxin A (2CPA) gene [98]. Rap2.4a
was proposed as redox sensor and/or transducer of redox information
in the cell to control the expression of 2CPA and other chloroplast antioxidant
proteins [99]. The redox environment leads to profound transitions
(dithiol/disulfide) of the quaternary structure of Rap2.4a where under
intermediate redox potentials Rap2.4a protein forms homo-dimeric structure by
intermolecular disulfide bond and binds to the DNA promoter target and activates
transcription. DNA-binding and transactivation activities are impaired under
both highly reducing and highly oxidizing conditions. The midpoint redox
of Rap2.4a was found to be about –270 mV which is
more negative than the midpoint potential of glutathione (–230
mV), but less than that of most thioredoxins (–290
to –300
mV) suggesting that moderate oxidation of the glutathione pool might be
sufficient to activate Rap2.4a-dependent gene expression [99]. In high light
response, detailed transcriptional profiling and clustering assigned RAP2.4a to
a network of extremely fast-responding transcription factors [145,146].
4.2. Metal-S-clusters
in functional assem bly
• Bacterial
SoxR, SoxS and plant SBP and WRKY
While
OxyR is involved in H2O2 responses, SoxR
and SoxS orchestrate the bacterial response to superoxide. Superoxide modify
the activity of SoxR, which, in turn, induces expression of SoxS. SoxR
is divergently transcribed from SoxS and SoxR promoter is embedded
within the SoxS gene. SoxR binds to SoxS promoter as
homodimer to prevent enhanced transcription in the absence of an oxidative
stress signal. In the absence of an induction signal, SoxR repression in combination
with the proteases, Lon and FtsH, results in low levels of SoxS protein, however, oxidation of SoxR [2Fe-2S] cluster converts SoxR to an activator of SoxS transcription. When SoxS levels increase, this will lead to
increased expression of genes related to DNA repair (such as nfo) and genes
involved in detoxification (such as sodA). The activation, as a result of
oxidization by the superoxide, has been recently challenged after it has been
considered for long time. Superoxide has been shown not to be the oxidizer of
SoxR; rather the redox cycling oxidizes SoxR [147,148,149]. Proteins that carry Fe, Cu, Mn, and Zn metal ions as cofactors mediate diverse biochemical processes, including energy conversion, synthesis, and DNA regulation, reactive oxygen
species (ROS) detoxification, as well as signaling events that trigger
molecular, cellular, and systemic responses. The well-studied example of the
bacterial oxidative stress sensor SoxR with its [2Fe-2S] cluster is compared to
the plant-specific TFs that also contain sulfur-based metal-binding sites (Zn), e.g. WRKY proteins and SQUAMOSA promoter binding proteins (SBP) TFs [150,151]. Because
metal-S-clusters often serve as redox sensory systems, it is likely these TFs
are sensitive to oxidative modifications and redox regulation. Like in the case
of WRKY8 [152], expression of WRKY30, WRKY75, WRKY48, WRKY39, WRKY6, WRKY53, WRKY22 was triggered in Arabidopsis in response to H2O2
treatment. WRKY63 was suggested to function as an activator in HL stress
tolerance where it triggers downstream components that quenches ROS formed by
HL stress [153]. CBSX (single cystathionine β-synthase domain–containing
proteins), CC-type GRXs (ROXY1 and ROXY2) and SBP TFs, which are targets of
miR156 and miR157, were implicated in redox clean up during male reproductive
development [154,155].
4.3. Subcellular
localization/Compartmentation
• Yeast
YAP1, a good example to compare with the plant HSFA8
In
unicellular eukaryotic organisms, such as yeast, TFs have been found to respond
to ROS and to function in a redox regulated manner. AP1 protein family in yeast
(yAP-1) is particularly interesting. S. cerevisiae YAP1 TFs contain a bZIP
DNA-binding and dimerization domain. YAP1 exists both in the cytosol in its
inactive form and in the nucleus, where it is active. Under non-stress
conditions YAP1 is preferentially localized in the cytoplasm however upon cells
exposure to oxidative stress YAP1 is quickly translocated to the nucleus [156,233].
Oxidation of the H2O2
sensor Oxidant Receptor Peroxidase 1 (ORP1/Gpx3) triggers a thiol–disulphide
relay mechanism that ultimately leads to nuclear accumulation of the yeast YAP1
transcription factor [138]. Like in the case of OxyR, SoxR and SoxS, no
apparent homologs have been assigned to the plant system and instead other
plant TFs e.g. HSFA8 have been reported to adopt a mechanism that rely on
compartmentation. Heat-responsive genes counteract exposure of plants to heat
stress. Expression of heat-responsive genes and heat shock proteins (HSPs) is
regulated by the heat shock transcription factors (HSFs) which binds to the
heat shock element (HSE; (5′-nAGAAnnTTCTn-3′) in the promoter region of the
heat-responsive genes. H2O2 have been proposed to
possibly directly modify HSFs and induce HSF trimerization [157]. HSFA1A
contains one Cys residue within the trimerization domain [158]. The early response
to high light has been shown to be mediated by a subset of HSFs where the HSF, e.g. HSFA1D, translocates from the cytoplasm to the nucleus [159]. Furthermore, it has been recently shown that translocation of HSFA8 to the nucleus in
response to oxidative stress is mediated through the Cys residue. Indeed, H2O2
induced wild-type HSFA8, but not HSFA8 C24S mutant variant, translocations from
the cytosol to the nucleus in the protoplasts [160].
4.4. Disulfide
formation and other Cys modifications such as S-glutathionylation, S-nitrosylation
• AP-1
involves Cys modification in the DNA binding domain a good example to compare with
plant HD-ZIP and
Strong
evidences for redox-regulation of mammalian TFs have been demonstrated.
Moreover, binding sites of the redox-regulated TFs such as activator protein-1
(AP-1) are present in promoters of genes directly involved in the pathogenesis
of diseases e.g. cancer, AIDS, and diabetes. The AP-1 protein family consists
of several groups of bZIP TFs subfamilies such as Jun (v-Jun, c-Jun, JunB, JunD), Fos (v-Fos, c-Fos, FOSB, FRA-1, FRA2), small MAF (MAFG, MAFF, and MAFK)
and large MAF (cMAF, MAFB, MAFA, and NRL), and ATF/CREB (CREB, ATF1, ATF2, ATF4, ATF5, ATF6a, ATF6b, ATF7, ATF3/LRF1, B-ATF, and ATFa0), JDP1/2). AP-1
regulates several cellular processes such as cell proliferation, survival, differentiation and apoptosis [161]. The AP-1 proteins form heterodimers and
bind to target DNA sequences such as TPA-responsive element (TRE;
5-TGAG/CTCA-3) or cAMP response elements (CRE; 5-TGACGTCA-3). One mechanism of
AP-1 regulation involves reversible oxidation of specific Cys residues in c-Jun
and c-Fos basic regions. The AP-1 activity can be restored in vitro using
reducing agents. Furthermore, the DNA-binding activity of AP-1 is suppressed by
nitric oxide through S-glutathionylation [162,163].
In
plant the homeodomain (HD) containing TFs have been divided into six families, namely (1) homeodomain leucine zipper (HD-Zip); (2) homeodomain with a finger
domain (PHD); (3) Bell domain (Bell); (4) zinc finger with homeodomain (ZF-HD);
(5) Wuschel homeobox (WOX); and (6) Knotted homeobox (KNOX). Among these the
HD-Zip are only present in plants and therefore they were suggested to have
plant specific developmental role [164]. Moreover, HD-Zip proteins are divided
into four classes, Ⅰ–Ⅳ, where each of them contains several members in the
different plant species. Redox regulation has been investigated for members
from HD-Zip Ⅱ and HD-Zip Ⅲ classes because they contain conserved Cys
residues. HAHR1 and HABH-10 that belong to the HD-Zip Ⅱ of the sunflower (H.
annuus) undergo dithiol/disulfide exchanges, which affects their DNA-binding
activity evident by using oxidizing, and reducing agents, Cys directed
mutagenesis and the use of NADPH/TRX/TR system. The authors suggested that in
response to environmental signals a redox-dependent mechanism may operate in
the plants to modulate the activity of these TFs [165]. ATHB-9 /PHV of the
HD-Zip Ⅲ class was investigated for the role of the 4 Cys residues in the
HD-zip domain and it was shown that the redox sensitivity is conferred from Cys
residues located at the positions 23, 38 and 42. Cys23 and Cys38 in ATHB-9 are
conserved in all HD-Zip Ⅲ class. ATHB-9 is actively binding DNA targets in
the presence of reducing conditions or thioredoxin system. Oxidation
inactivates ATHB-9 and impairs its DNA-binding activity while TRX reactivate
it. Because of sequence conservation, redox-dependent control of target gene
expression can be expected in other HD TFs during developmental processes
[166].
4.5. Redox
regulation of proteolytic processing
• NRF2
and ANAC089
NF-E2-related factor 2 (NRF2) is a member of p45 NF-E2-related
proteins (p45 NF-E2, NRF1, NRF2, and NRF3. NRF2 is involved in haematopoiesis, differentiation, proliferation, growth, apoptosis, drug metabolism, and the
oxidative stress response. In unstressed (normal) conditions, NRF2 is localized
in the cytoplasm where it interacts with Kelch-like ECH-associating protein 1
(KEAP1) which targets NRF2 for rapid degradation by the ubiquitin-proteasome.
However, in response to stress NRF2 translocate to the nucleus and
heterodimerizes with small masculoaponeurotic fibrosarcoma (MAF) proteins and
bind to the Antioxidant Response Element (ARE), a cis-acting enhancer sequence
(TCAG/CXXXGC) that is present in the promoter region of NRF2-regulated genes.
Moreover, NRF2 was found to possess ARE in its own promoter to initiate its own
transcription. It is interesting that KEAP1 contains several active Cys
residues that are targets for modification by ROS and electrophiles. Such
modification results in dissociation between KEAP1 and NRF2 which allows of
NRF2 translocation to the nucleus to regulate gene expression and suggesting
that the activity of NRF2 is negatively regulated by KEAP1-dependent cytosolic
sequestration pathway [167]. Other mechanisms were suggested to activate NRF2, other than targeting KEAP1, in which oxidation can activate NRF2 through
phosphorylation by PKC and PERK that leads to NRF2 dissociation from KEAP1, allowing the free NRF2 to translocate to nucleus [168]. Redox regulation of TFs
in terms of shuttling between different compartments i.e. the plasma membrane
and the nucleus have been reported for ANAC089. NAC transcription factors have
been shown to be involved in responses to biotic and biotic stresses, hormonal
signaling and developmental processes. ANAC089 fusion
protein localizes to vesicle-like structures and peripheral membranes [169,170].
The reductive signal lead to the translocation of ANAC089 from the membrane to
the nucleus [149] where it binds to the oxidative responsive element in the promoter
region of sAPX to suppress its gene expression i.e. ANAC089 functions as a
negative regulator of sAPX expression if the cell encounters highly reducing
conditions [170]. More recently ANAC089 was shown to be an important player in
the ER-stress induced PCD which can be summarized as follows: bZIP28 and bZIP60
upregulate ANAC089 gene expression, the ANAC089 translocates from the ER
membrane to the nucleus, PCD induction by ANAC089, the ability of ANAC089 to
bind the promoter of many downstream targets and finally the evidence deduced
from NAC089 knock-down (KD) mutants which suppresses the ER-stress-induced
expression of several PCD regulators [171].
4.6. Regulation
of PTMs: phosphorylation, ubiquitination, and sumoylation
• NF-κB
and ABI5
Nuclear
factor kappa B (NF-κB) is a heterodimeric ubiquitous redox-sensitive TF that
coordinates regulators of immunity, inflammatory responses, cell proliferation, cellular growth and development, and apoptosis. The activity NF-kB is
dysregulated in a number of chronic inflammatory diseases and certain types of
cancers. That's why NF-kB signaling is an attractive target for the development
of anti-inflammatory and anti-cancer drugs [172]. The mammalian NF-κB family
consists of NF-κB1 (p50/p105), NF-κB2 (p52/p100), RelA (p65), c-Rel, and RelB
which are characterized by the presence of Rel homology domain (RHD). There are
three functions mediated by RHD (1) NF-κB DNA
binding (2) homo-and hetero-dimerization between NF-κB family
members (3) association of
the inhibitors of kappa B (IκB) with NF-κB dimers. While NF-kB exists as an
inactive cytoplasmic complex with inhibitory kB (IκB) proteins in
non-stimulated cells, its transcriptional activation occurs through distinct
steps. Different stimuli such as cytokines and ROS leads to NF-κB activation
through activation of IκB kinase (IKK) which then phosphorylates IκB resulting
in dissociation of NF-κB from the IκB inhibitor and of IκB degradation by
ubiquitin/proteasome system. Then unbound NF-κB translocates to the nucleus
where it binds to DNA and activates the transcription of target genes [173,174].
In plants, the phytohormone abscisic acid (ABA) regulates plant growth and
development as well as response to abiotic and biotic stresses. Cellular
responses to ABA are mediated by a suite of transcription factors including
members of the B3, AP2, and bZIPs domain families. Abscisic Acid Insensitive 5
(ABI5), a bZIP TF and one of the most studied ABA-responsive TFs, functions as
a regulator of abiotic stress responses and integrator of ABA crosstalk with
other phytohormones [175]. PTMs such as phosphorylation, ubiquitination, and
sumoylation play significant roles in regulating ABI5 and thus ABA signaling.
In unstressed conditions, ABI5 is maintained at low levels via degradation by
the 26S proteasome. However, upon exposure to stress conditions, proteasome-dependent turnover decreases and ABI5 becomes more stable which
leads to its accumulated and then induces expression of ABA-responsive genes
required for growth inhibition and stress tolerance [175]. ABI5 degradation by
the 26S proteasome depends on the ubiquitination pathway by a number of
ubiquitin ligases. Ubiquitin ligases which directly target ABI5 include Keep on
Going (KEG), a single subunit RING-type E3, which interacts with the E2 and
ABI5 via its RING domain and ankyrin repeats, respectively [176].
While
phosphorylation of ABI5, which is necessary for its activation, is mediated by
members of the Sucrose Non-fermenting1-related protein kinases (SnRK) 2 and
CBL-interacting protein kinase (CIPK) families [177], Serine/Threonine Protein
Phosphatase 6 (PP6) dephosphorylates ABI5 and negatively regulate ABA signaling
[178]. Moreover, low levels of ABI5 in siz1 mutant compared with wild type in
response to ABA treatment suggested that sumoylation regulates ABI5 abundance [179].
4.7. Protein-protein
interaction
• Mammalian
Ref1-Trx-AP1 and the best studied redox-regulated transcription system in
plants NPR1-TGA
Redox
effector factor 1 (Ref1) was identified in a search for nuclear factors
responsible for the AP-1 TF reduction. Subsequently, Ref-1 was reported to
reduce several TFs such as NF-κB, HIF-1α, p53, PAX. The redox regulation of
AP-1 depends on a direct interaction between Trx and Ref1 [180]. The scenario
is the following: Ref-1 was co-purified with AP-1 and interaction between them
was stable. Moreover, TRX is one of the endogenous redox molecules that
regulates the AP-1 transcriptional activity through its direct association with
Ref1 [181]. Previously, solution structure showed direct association of TRX and
the partial peptide of Ref-1 using oligopeptide comprising amino acid residues
59–71 of Ref-1 and TRX complex [182]. Cys-32 and Cys-35 of TRX, which
constitute the catalytic center, were shown to be involved in the association
using whole recombinant proteins in in vivo experiments. Moreover, Site-directed mutagenesis Cys-63 and Cys-95 in the redox domain of Ref-1, suggested that they are redox sensitive and can be targets of TRX [181].
TGA
TFs belong to the group of bZIP factors binds to the cis element TGACGTGG and
they are implicated as regulators of pathogenesis-related (PR) genes [182,183].
Among the 10 TGA genes in Arabidopsis 7 of them (TGA1-TGA7) have been
characterized based on their interaction with the nonexpresser of PR gene1
(NPR1). Redox Regulation of the NPR1-TGA1 System is one of the best studied
redox-controlled signaling. During systemic acquired resistance (SAR), SA-induced redox change regulates NPR1-TGA interaction [184]. SA-mediated redox
changes lead to reduction in the intermolecular disulfide bonds of Cys-156 of
NPR1 oligomers to its active monomeric form which is then translocated to the
nucleus to fulfill its coactivator function by interacting with TGA1 which is
required for the induction of PR genes [185]. SA triggers monomerization of
NPR1 by a reduction catalyzed by TRX-h
proteins that counter the effect of GSNO-facilitated oligomeri-zation [186].
Among the four Cys residues in TGA1, Cys-260 and Cys-266 form an intramolecular
bond in response to oxidation however redox changes do not directly regulate
the DNA binding activity of TGA1. However, reduction of this disulfide bond is
critical for the interaction between TGA1 and NPR1 [184]. Further
investigations suggested that TGA1 Cys-172 and Cys-287 are also
redox-responsive and form intermolecular disulfide bond [187]. The authors also
demonstrated that the DNA-binding activity of TGA1 was enhanced by GSNO in
presence of NPR1. Not only NPR1 and TGA1 proteins were S-nitrosylated with the
treatment by NO donor, but also the nuclear translocation of NPR1 was promoted
by NO [187]. The plant-specific CC-type glutaredoxins (ROXYs), involved in
anther development [188], contains this leucine rich motif which mediates the
interactions between ROXYs and TGA TFs [189].
4.8. Direct
redox control of DNA binding
Several
plants of the TFs are regulated through direct redox control of their DNA
inding such as the R2R3 MYB [190]. While vertebrate MYB genes encode proteins
with MYB domains formed by three MYB repeats (R1R2R3 MYB), the majority of
plant MYB genes encode proteins with only two MYB repeats (R2R3 MYB) most
similar to the vertebrate R2 and R3 MYB. Most plant R2R3 MYB domains contain
two cysteines, Cys-49 and Cys-53. Cys-53 is located at the equivalent position
as Cys-130 in R1R2R3 MYB. In vertebrate MYB TFs Cys-130 was shown to serve as a
redox sensor because when it was mutated the DNA binding and transcriptional
activities of c-MYB were significantly impaired [191]. It is interesting that
Cys-53, established for vertebrate MYB proteins, is not essential for the
DNA-binding activity of P1 TF (R2R3 MYB) from maize while Cys-49 acted as the
redox sensor [190]. Moreover, Cys-49 and Cys-53 form an intramolecular
disulfide bond that can be important for modulating DNA-binding activity [191].
TCP
is plant specific family of TFs that share a conserved DNA-binding domain, which contains bHLH motif, called the TCP domain. This family is grouped into
class Ⅰ (TCP-P) and class Ⅱ (TCP-C) that are involved in plant growth and
development [192]. In TCP class Ⅰ of different plants species, a shared
conserved cysteine residue at position 20 within the TCP DNA binding domain
(Cys-20) was found. However, this conserved Cys-20 is not present in TCP class
Ⅱ. Interestingly, in vitro assays (EMSAs) suggested that Cys-20 is not
required for DNA binding but rather it is responsible for the redox sensitivity
of class Ⅰ TCP proteins and that mutating Cys-20 in TCP15 abolished its redox
sensitivity probably though intermolecular disulfide formation [193]. Moreover, H2O2 not only significantly decreased
the transcriptional activity of TCP15 in the transactivation assays in yeast
but also in the plants [193]. It's interesting
that TCP proteins have in the second helix of the DNA-binding domain a
leucine-rich short motif (L**LL) which has been shown to mediate protein
interactions both in animals and plants.
It is Another group of plant proteins which is under redox
control is group G of bZIP TF family [194]. Ten
groups are classified in Arabidopsis bZIP family according to sequence
similarities of their basic region, conserved motifs, and
other features such as the size of the leucine zipper [195].
The G group comprises 5 members namely AtbZIP16, AtbZIP68, GBF1, GBF2 and GBF3
and all of them binds to the G-box cis element [194,196,197].
The G-box element was reported to be enriched in high light regulated genes
such as LHCB2.4 [198].
Because LHCB2.4 represents the genes responding to light and/or redox
changes, part of its promoter region containing G-box was used to isolate TF(s)
targets [194]. Interestingly, the conserved
Cys330 in the DNA binding domain of bZIP16, bZIP68 and GBF1 is sensitive to
redox treatment and should be reduced for optimal binding of target genes [194]. While Shaikhali et al. reported bZIP16 as
transcriptional repressor in the light regulated
photomorphogenesis response [194], Hsieh et
al. reported bZIP16 as a newly identified G-box binding TF which integrates the
light and hormone pathways to promote seed germination and hypocotyl elongation [199].
Subsequently, GIP1 has been identified as a regulator for the TFs bZIP16, bZIP68 and GBF1 in early stages of Arabidopsis
seedling development. In response to redox treatments GIP1 undergoes functional
switching from redox to chaperone activity [200]. GIP1 was
also reported to may act as a transcriptional coactivator of LBD18. LBD18
belongs to plant-specific lateral organ boundaries domain and plays a key role
in lateral organ development of plants [201].
5. Redox-regulation
of the Mediator complex
The
transcriptional regulation in eukaryotes is complex and involve many components
such as RNA polymerase Ⅱ (RNP Ⅱ), general transcription factors (GTFs), transcription factors and the mediator complex. The mediator complex provides
the essential link between TFs and RNP Ⅱ
where it transduces diverse signals to genes involved in different pathways.
The first reports on the mediator complex came from the yeast Saccharomyces
cerevisiae where about 20 subunits were estimated [202].
Subsequently the mediator complex was identified in almost all organisms.
Therefore, a unified nomenclature for the different protein subunits of the
mediator complexes across the different species has been proposed. Backström et
al. isolated for the first time the mediator complex
from Arabidopsis using column chromatography accompanied with
immunoprecipitation using antibody raised against MED6 subunit [203]. The
authors reported the isolation of 21 conserved and six specific subunits of the
mediator complex [203]. Subsequent comparative genomics and bioinformatics work
identified the mediator complex in 16 plant species represented across the
plant kingdom [204]. In their work, Mathur et al. identified for the first time
MED26 subunits that was not
identified earlier in any of the plant species. Moreover, Arabidopsis MED32, MED33, MED34, MED35, MED36, and MED37 orthologues were identified in all plant
groups [204].
The different mediator complex subunits have been
assigned to three modular structures called the head, the middle and the tail
modules which form the core of the complex. In addition to these modules, there
is a separable kinase or CDK8 module, which consists of CDK8, cyclin C, MED12, and MED13. Depends on the cellular context the mediator complex shuttle between
two different states: The Mediator core complex and Mediator core-kinase
complex in which the former favors transcription (the mediator core associates
with RNAP Ⅱ) and the latter represses transcription (the kinase module-bound
Mediator complex dissociates from RNAP Ⅱ). The first studies on the mediator
suggested that it is mainly linked to the initiation step of transcription [205,206], however, later on the mediator has be
linked to different step in the transcription [207,208,209,210,211]. Specific functions in signaling pathways
which are important during development and in response to biotic and abiotic
stress have been reported for several plant mediator complex subunits [212,213,214,215,216,217]. For instance, MED21 has been proposed to
function in defense against necrotrophic pathogens through interaction with
HUB1 [218]. MED14, MED15, and MED16 are
considered as key players in plant defense signaling crosstalk [219]. MED18 and MED20 have been recently suggested
as factors that confer susceptibility for Fusarium oxysporum infection in
Arabidopsis [220]. MED12 and MED13 regulates
early embryo patterning [221]. Cell number
during primordia initiation is controlled by
SWP/MED14 [222]. PFT1/MED25 has been shown to
regulate
flowering, jasmonate signaling, light signaling and photomorphogenesis and
biotic and abiotic stress tolerance [212,213,216,223].
It is interesting that both the mediator complex and
ROS/redox regulate transcription during growth, development and
stress, however, no direct linkage between them have been reported until
recently [224,225,226,227,228,229,230]. Analysis of npr1 single
mutant and tga6 tga2 tga5 triple mutant suggested that NPR1 and the TGA factors (TGA2, TGA5, and TGA6) are essential
positive regulators of SAR [231,232]. Later on, induction of SAR was abolished completely in
med16/sfr6 mutant plants similar to that in npr1 and tga6 tga2 tga5 triple
mutant suggesting that MED16 is an essential positive regulator of SAR [234]. The decreased levels of NPR1 protein in med16
mutants suggested that MED16 may regulated SA signaling and basal immunity at
least partly through modulating NPR1 accumulation [234].
PFT1/MED25 was suggested to control ROS balance in roots and is critical for
root hair differentiation and elongation probably by regulating the expression of redox-related genes, the Class Ⅲ
peroxidases [224]. In their working model the authors suggested that, possibly, PFT1/MED25
induces the expression of class Ⅲ peroxidases where H2O2 is produced in the elongation zone. On contrary, NADPH
oxidases produces O2– in the
meristematic zone. When a threshold concentration of the distribution of H2O2 and O2– is reached, it acts as a signal which determines the differentiation of root hair cells
[224]. The fact that PFT1/MED25 is involved in different signaling pathways and mechanisms such as flowering time and light signaling [212,213,214,215,216], JA signaling [217], JA-mediated pathogen defense [223], abiotic stress [213] and root hair
development [224] prompted the authors to suggest that PFT1 probably control these
processes by regulating redox homeostasis [224]. Involvement of the mediator
complex in redox signaling and regulation has been also proposed through the
interaction of MED18 and YIN YANG1 (YY1), a zinc finger transcription factor, which associates with the promoter regions of genes encoding proteins known for
long to be involved in redox regulation and signaling i.e. GRXS13/ROXY18, GRXC9/ROXY19 and TRXh5. Moreover, the deregulated expression of GRXS13/ROXY18, GRXC9/ROXY19 and TRXh5 in yy1 and med18 mutants suggested a synergistic role
for GRXs and TRXs in plant immunity [227]. More recently we reported that
representative members of the head, middle and tail modules of the
mediator complex, MED28, MED10a and MED32 are targets of redox-regulation
[228]. These proteins form intermolecular disulfide bonds, which are reduced by
at least one representative member of GRXs
and TRXs families, although with different efficiencies [228]. In particular, MED28 oligomer formation involved both covalent and non-covalent interactions
[229]. Moreover, med32 and med28 mutants display phenotypes (altered root
development and senescence) associated with redox changes [230].
6. The power of Mass Spectrometry and Proteomics
in developing the study of redox regulated transcription
Recent
studies acknowledged proteomic as a powerful technique to characterize induced
protein modifications involved in the regulation of several redox-regulated
signaling pathways involved in cellular physiological processes [235,236]. Identification of the general basis the
polypeptide targets of redox activity and how these modifications may control
protein function and the cellular pathways of which they are a part of is the
intention of redox proteomics. Nowadays, a quantitative evaluation of protein oxidation
under different cellular conditions is provided by the field of redox
proteomics that is increasing. Moreover, nowadays combined nano-liquid
chromatography, electrophoretic protein/peptide separation techniques, mass
spectrometry, and affinity based methodologies have provided a better
understanding of the protein oxidative modifications present in various
biological systems under different physiological conditions.
Carbonyl
formation, oxidation of side chains of amino acids, products of glycation reactions, reactive alkenal product of lipid peroxidation are common oxidative
post-translational modifications (oxPTMs) to proteins [237].
To this end, the sensitive Cys residue to e.g. nitrosylation and oxidation
states of the sulfur atom of the Cys thiol may be is the prime target of redox
modification [238]. Furthermore, the
involvement of the oxidation of Cys residues leading to oligomerization of
subunits of the Mediator complex may play an important regulatory role [228]. However, the methods that analyze redox
regulation of plant transcription have only partly reached the necessary
sensitivity to address the level of complexity in vivo. Thus, there is an
urgent need to improve proteome sensitivity to characterize low abundant
proteins with various oxPTMs [137].
One
possibility to characterize low abundant TFs proteins with mass spectrometry is
to use nucleus fractionation to enhance the sensitivity in the mass
spectrometry analysis [239,240,241]. Examples from
nucleus extraction procedures and using nucleus recombinant proteins in
Arabidopsis have identified S-nitrosylations of different of proteins [187,242,243]. A nuclear overexpressed Thioredoxin
(Trxo 1) was identified to interact with the
proliferating cell nuclear antigen (PCNA) and thus to be a potential target [245]. Chaki et al. treated Arabidopsis suspension
cell cultures with pathogens before nuclear proteins were extracted and treated
with the S-nitrosylating agent GSNO [244]. A
biotin switch assay was performed and biotin-labelled proteins were purified by
neutravidin affinity chromatography and identified by mass spectrometry. Most
of the S-nitrosylated candidates were involved in protein and RNA metabolism, stress response, and cell organization
and division. In addition, plant-specific histone deacetylases were
identified suggesting that nitric oxide regulated epigenetic processes in
plants [244].
Although
much work has been carried out using untargeted or discovery mass spectrometry
approaches, identification of oxPTMs has supported from the development of
targeted or semi-targeted scanning routines, combined with chemical labeling
and enrichment approaches [245]. However, many
pitfalls exist and both advantages and limitations in redox analysis by mass
spectrometry has been discussed in several review articles [235,236,245,247]. Limitations and pitfalls can be
summarized as follows: the lack of standard proteomics methodology (lack of
well-established validation protocols for oxPTM) when oxPTMs analysis is
performed. Adding to this the wide variety of methodologies, and the complex
data analysis. The lack of competence of the invistigator, as well as the
poor bioinformatic background can be other factors. Finding solutions to these
limitations and pitfalls in the future will certainly advance our way in
studying nuclear proteins and hence redox-regulated transcription.
7. Conclusions
The
redox-signaling pathway involves several players (receptors/acceptors, transmitters/transducers, responders) and the redox network involves inputs
from various redox cues. In this review, a special
attention has been given to redox-regulation of transcription that is an
evolutionary conserved mechanism in which changes in the ROS and/or the redox
state of the cell leads to changes or alterations in the gene expression. We
discussed several examples of the redox regulated TFs from different organisms
and their response to stress/environmental conditions. Moreover, PTMs of TFs
such as covalent interaction (disulfide bond formation) are important for their
regulation to help the plants to cope with external stresses. The recent
findings, as highlighted in this review, have made it clear that proteomics and
mass spectroscopic have to be improved to identify the low abundant nuclear
proteins and to increase the sensitivity to identify PTMs such as Cys residues
involved in covalent interactions.
Acknowledgment
The
authors apologize for the many colleagues' reports not included in this review.
Conflict of interest
The
authors declare no conflict of interest.









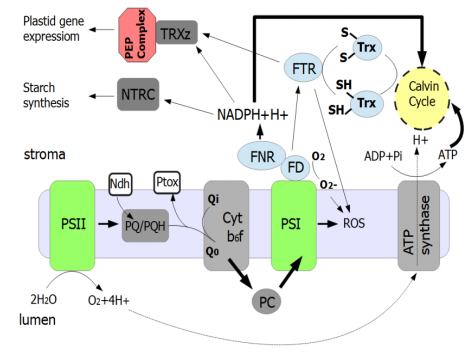
 DownLoad:
DownLoad: