1.
Introduction
Decision makers tackle and, despite the complications in every field of life, are in different challenging situations. It is not easy to express the attributed value due to its uncertain nature. Scholars introduce many tools to handle its fuzzy nature. A fuzzy set is a generalized form of a well-defined set, which is known as a truth set having components the members of truth degrees [1]. A truth set only has two values 0, for (no) and 1 for (yes), so, with the help of the truth set, it cannot be easy, and sometimes solving real-world problems may become impossible. To get a better result, fuzzy set (FS) allows adjustment of truth membership value from 0−1 instead of only consisting of 0 or 1. Father of fuzzy set theory, Zadeh put forward an idea of the fuzzy set in 1965 [2], which is a generalization of a truth set in which entries of a set have different membership values. We know in this generalization no concept of a falsity-membership or negative value is defined. In 1985, Atanassov gave the idea of falsity and truth membership value, which is defined in the form of an intuitionistic fuzzy set (IFS) [3] based on having constraint
In this context, the symbols α and β are used to denote the degree of membership in the concepts of truth and falsehood, respectively. But in IFS there are some cases where the sum of truth membership and falsity-membership degrees increases from 1 [4]. The Pythagorean fuzzy set was proposed for such cases by Zhang and Xu with new limitations of
which expands the area of uncertainty. Q-rung orthopair fuzzy set was proposed for more generalization by Yager [5] with a condition of
For solving real-world problems more generally [6] gives an idea of picture fuzzy set (PFS) in which human opinions are of type yes, no, abstain, and refusal is discussed as more sufficient in situations. The PFS gave truth membership, falsity-membership, and abstain degrees as well, with the condition
where
is a refusal degree. Then some fuzzy logic operators — disjunction, conjunction, implication, and complements — were broadened by Coung and Hai.
As PFS well expresses uncertainty and ambiguity to obtain more extension, the concepts of interval-valued spherical fuzzy set (IVSFS) and IV T-spherical fuzzy set (IVTSFS) are introduced along with the condition
which strengthens the idea of PFS. The choice of parameters is increased by IVTSFS and also plays an effective role in real-world issues. Bonferroni mean operators are discussed in [7] for TSFHG, and are used to evaluate search and rescue robots performance [8]. The strategy used for evaluating the performance of solar cells under uncertain conditions was the multi-attribute group decision-making (DM) approach using IVTSF information [9].
The graph is the easiest way of graphical representation of different relationships between different elements. The researcher of [10] discussed the idea of fuzzy graph (FG) and its structure. He also introduced and highlighted the characteristics of paths, cycles, connectivity, subgraphs, trees, and forests. Parvathi gave the idea of an intuitionistic fuzzy graph (IFG) by increasing the falsity membership value [11]. In [12], degree, size, and order of IFG are also discussed. The generalization of IFS Pythagorean fuzzy graph (PyFG) is also introduced in [13], which also introduces and discuss a number of its properties. By the generalization of PyFG, we get a new concept of a q-rung ortho-pair fuzzy graph (q-ROFG) [14] and also discussed its applications in soil system [15]. In 2019, Zuo proposed a picture fuzzy graph in which truth membership, falsitymembership, and, abstinence degrees are involved [16]. In a study conducted by [17], a spherical fuzzy graph (SFG) was developed, with an exponent increase of two. Similarly, [18] presented the notion of a T-spherical fuzzy graph (TSFG) based on the work of [19], which included an extension of exponent T. In 2011 Akram gave an idea of IV fuzzy graphs (IVFG)[20]. The authors in [21] introduced the concept of interval-valued intuitionistic fuzzy graph (IVIFG) due to the expansion of IV fuzzy graphs (IVFG). In [22], some certain operations and applications are discussed. The generalized forms of (IVIFG) of IV Pythagorean fuzzy set (IVPyFG) [23], IV q-Rung orthopair fuzzy set (IVqRFG) [24], IV picture fuzzy graph (IVPFG) [25], and IV spherical fuzzy graph (IVSFG), IV T-spherical fuzzy graph (IVTSFG) [26]. There are some other topics related to these fuzzy graph theoretical parameters like, metric dimension [27,28,29], topological descriptors [30,31,32], and their applications [33,34,35,36,37].
In chemistry, Gutman introduced [38] the idea that the energy of a graph is determined by the interplay between the π energy of electrons and the summation of these energies in certain molecules, and also discovered the upper energy limit and lower energy limit of the graph. The energy of a complete graph is usually calculated as 2 times the difference between the total count of nodes (represented by n) and 1. In the case of a graph with no edges, the energy is equal to 0. The energy of splitting graph [39] and the energy of shadow graph [40]. The authors in [41] calculated the Laplacian energy for the graph. If the vertices are adjacent, then the Randić matrix
is defined as
here dχ represents the degree of vertex χ otherwise
Researchers gave the idea of Randić energy, which can be calculated as the sum of absolute values of eigenvalues[42]. The Laplacian energy of a fuzzy graph is presented by [43]. The intuitionistic fuzzy graphs IFG are started; after that, researcher worked on the Laplacian graph and found the energy of an IFG [44]. Akram presents the energy and Laplacian energy of PFGs; the researchers established various DM methods and techniques to tackle the challenges involved in various disciplines. In general, the IVTSFSs are better in comparison to interval-valued intuitionistic fuzzy set (IVIFS) and IVqROFS as they also examine the abstinence degree.
When there is more than one method for the solution of a particular problem, to get a more efficient answer, concept of an aggregation operator is more beneficial. Hamacher examined a specific category of t-operators [45]. t-norm (TN) and Hamacher t-conorm (TCN) by Hmacher are a generalization of algebraic TN and TCN, and they are highly effective and more flexible. When comparing various notions, it is evident that the interval-valued T-spherical fuzzy Hamacher graphs (IVTSFHG) exhibit primarily flexibility and parameterization in representing the perspectives of decision-makers. These are some situations that caused us to write that article. Spectrum graph theory is an inclusive application in branches of mathematics like physics, chemistry, computer science, and many others. Graph spectrum helps in combinatorial optimization problems and has a remarkable role in mathematics. The concept of graph energy comes from energy in chemistry. Multiple mathematical properties of graph energies are being explored.
Another idea of energy, referred to as the FG energy, is introduced in this study. This concept expands upon the existing definitions of energy and Randić energy to include the visualization of FGs and IV FGs. To handle the situation, the modified form of IVIFS, q−ROFSG, is an effective tool, which is IVTSFS, which can increase the human opinion's flexibility. In this article, the Hamacher aggregation operators have been used to calculate the energy and Randić energy of IVFG, thereby providing a comprehensive assessment of its effect. The article makes a number of contributions: The concept of IVTSFHG is used in the context of the Hamacher TN and TCN. In this discourse, we engage in a discussion on the energy associated with the division of IVTSFHG, as well as its corresponding shadow. We provide compelling findings pertaining to this topic. A numerical problem-solving method is provided using IVTSF data. The provided approach has the potential to be employed in many problem-solving scenarios in order to get optimal decision-making outcomes [46].
1.1. Importance, novelty and research gap of the chosen topic
The investigation of Randic energies in the context of DM for human trafficking, using IVTSFHGs, is a novel and influential addition to the domains of graph theory and decision science. The significance of this paper rests in its capacity to provide a comprehensive and adaptable framework for the modeling and analysis of intricate systems, namely within the domain of human trafficking. The new contribution may be delineated as follows:
Multifaceted modeling approach: The use of IVTSFHGs enables a more accurate and adaptable depiction of uncertainties linked to human trafficking data. This comprehensive modeling methodology takes into account both the inherent imprecision of data and the IV attributes, so offering a more nuanced and exact representation of the DM context. Randic energies as descriptors: The integration of Randic energies introduces an additional dimension of structural analysis to the process of DM. The research presents a unique approach to quantifying the structural features of networks implicated in people trafficking via the use of graph-based descriptors. This phenomenon has the potential to provide valuable knowledge on the structure, interconnections, and possible weaknesses inherent in human trafficking networks. Decision support system: The incorporation of Randic energies within the context of IVTSFHGs makes a significant contribution to the advancement of a reliable decision support system. The use of this system has the potential to aid policymakers, law enforcement agencies, and many other stakeholders in making well-informed choices. This is achieved by offering a more extensive comprehension of the fundamental structures and dynamics that govern human trafficking networks. Handling uncertainty effectively: The issue of human trafficking is characterized by its intricate and ever-evolving nature, which gives rise to inherent uncertainty. The study places significant importance on IVTSFHGs, demonstrating a very advanced methodology for managing uncertainty. This technique enables decision-makers to efficiently negotiate the intricacies associated with incomplete or inaccurate information. Cross-disciplinary impact: The work presented in this study is characterized by its cross-disciplinary approach, which effectively combines graph theory with decision science. Moreover, the study's focus on the pressing social problem of human trafficking further amplifies its importance. The aforementioned contribution encompasses both theoretical improvements in mathematical modeling and practical implementations, hence bearing immediate consequences for addressing a significant worldwide concern.
The significance of the paper stems from its novel integration of IVTSFHGs and Randic energies, which together provide a robust DM framework within the domain of human trafficking. The new contributions of this study go beyond the conventional uses of graph theory, providing a complete and influential framework for comprehending and tackling intricate social problems.
1.2. Structure of the study
This study presents an analysis of our notions in a specific structure: Section 2 provides an overview of fundamental ideas. In Section 3, the concept of IVPFHGs was introduced, and an analysis was conducted to determine their respective energy levels. Additionally, the energy associated with the division of IVTSFHG and the shadow IVTSFHG was a topic of discussion. Section 4 made a contribution to the Randić energy calculations of IVTSFHGs and IVTSFHDGs. Section 5 of the paper presents an examination of the use of the IV T-spherical fuzzy Hamacher averaging (IVTSFHA) operator and the interval-valued T-spherical fuzzy Hamacher weighted geometric (IVTSFHWG) operator. The discussion is supported by the use of numerical examples. In the following part, the conclusion is examined.
2.
Preliminaries
In this section, we will discuss some basic definitions of our research work. In this article, we denote integers by I.
Definition 2.1. A TSFS Γ on a fixed set I is defined as:
where αv(Υ) shows the truth membership degree, βv(Υ) shows abstinence degree, γv(Υ) denotes the falsity membership degree.
and
in such a way that
is called the hesitancy degree.
Definition 2.2. Let three TSFs, V1,V2, and V3. Then
Definition 2.3. A TSF relation (TSFR), A on I×I is defined as:
where, αA denotes truth membership degree, βA denotes abstinence degree and γA represents falsity membership degree.
in such a way that
Definition 2.4. The TSFPR (TSF preference relation) can be explained as: Suppose
be a set of alternatives. A TSFPR on Θ is symbolized by a matrix
where
for all crisp values of 1≤a,b≤n in I. The symbol αab is used to symbolize the extent to which the attribute Υa is chosen in relation to the component Υb. Similarly, βab symbolizes the extent to which this Υa is not chosen in relation to Υb. Lastly, γab indicates the degree of stance among the elements Υa and Υb and
is hesitancy degree, which follows the condition αTab,βTab,γTab∈[0,1],
Definition 2.5. For comparing two TSF values (TSFV), we also use the score function and accuracy function.
Consider
is a TSFV. The score function S(♢) is symbolized and defined as
where S(♢)∈[−1,1].
Definition 2.6. Consider I is a fixed set,
and
are two TSFVs on I. Then
(ⅰ) If S(♢1)≤S(♢2) then ♢1≤♢2;
(ⅱ) If S(♢1)≥S(♢2) then ♢1≥♢2.
Definition 2.7. Let
is called TN, which is a binary function. If ∀Υ,ζ,n∈[0,1] meets the observing criteria
(ⅰ) (Υ,ζ)=Υ.
(ⅱ) Γ(Υ,ζ)=Γ(ζ,Υ).
(ⅲ) Γ(Υ,F(ζ,n))=(Γ(Υ,ζ),n).
(ⅳ) Γ(Υ,ζ)≤Γ(n,b) if Υ≤n and b≥ζ.
Definition 2.8. A TN X is said to be a TCN such that
The aforementioned statement of TNs along with TCN is established as the Hamacher product and Hamacher sum defined by
and
Definition 2.9. A TSFS X on a fixed set Z is defined as:
where αv(Υ) denotes the truth membership degree, βv(Υ) denotes the abstinence degree, γv(Υ) represents the falsity membership degree [47],
in such a way that
is called upper and lower hesitancy degree, respectively.
Definition 2.10. The TSF preference relation TSFPR can be explained as: Suppose
be a set of alternatives. A TSFPR on Θ is symbolized by a matrix
where
for all crisp values of 1≤a,b ≤n from I. Along with αab denotes the degree to which the element Υa is close to the element Υb and βab denotes the degree to which the element Υa is not selected to the element Υb and γab represents the abstinence degree between the element Υa and Υb and
is a hesitancy degree which follows the condition
3.
Interval-valued T-spherical fuzzy Hamacher graphs (IVTSFHG)
During the aforementioned part, we have presented the IVTSFG by using the Hamacher TN and TCN. In addition, we determine the energy associated with the variable IVTSFG and analyze its significant characteristics. In this context, we are further curious to see the energy associated with the splitting of IVTSFG and the shadow IVTSFG. It extends traditional fuzzy sets by incorporating interval values for membership, non-membership, abstention, and refusal degrees, offering a richer structure to model uncertainty with four-dimensional information. This makes it particularly suited for scenarios involving complex human judgments.
Definition 3.1. If
is a graph, then by an IV fuzzy relationBon a setEwe mean an IV fuzzy set such that
for all xy∈A [20].
Definition 3.2. Let
is an IVTSFHG on a fixed set I, where
is an IVTSFS in I and
is an IVTSFR on I in such a away that:
∀Υ,c∈I, where
Here V and E are the IVTSF vertex set and IVTSF edge set of G, respectively, where A is symmetric IVTSFR on V. If A is not symmetric on V, then
is called IVTSFHDG.
Definition 3.3. The notion (AM) is used for the adjacency matrix of the
is developed as
which is a square matrix
where A(αE,u(ΥχΥτ)),A(αE,l(ΥχΥτ)),A(βE,u(ΥχΥτ)),A(βE,l(ΥχΥτ)),A(γE,u(ΥχΥτ)), and A(γE,l(ΥχΥτ)) represent the IV truth, abstinence, and falsity membership degree between Υχ and Υτ, respectively.
Definition 3.4. Consider an
The adjacency matrix based on the spectrum of an IVTSFHGA(ϝ) is developed as (χ,H,Q), given χ,H,Q are eigenvalues' set of A(αA(ΥχΥτ)),A(βA(ΥχΥτ)),A(γA(ΥχΥτ)), respectively.
Definition 3.5. The energy of an
is developed by:
where ϕχ∈χ,υ∈H,ψ∈Q.
Theorem 3.1. Suppose
is an IVTSFHG with adjacency matrix A(ϝ). Consider ϕ1,ϕ2,ϕ3,…,ϕℵ are the eigenvalues of A(αA(ΥχΥτ)) having the condition
are the eigenvalues of A(βA(ΥχΥτ)) having the condition
and ψ1,ψ2,ψ3,…,ψℵ are the eigenvalues of A(γA(ΥχΥτ)) having the condition
then
(i)∑ℵχ=1[ϕχ,u,ϕχ,l]=[0,0], ∑ℵχ=1[υχ,u,υχ,l]=[0,0], ∑ℵχ=1[ψχ,u,ψχ,l]=[0,0];
(ii)∑ℵχ=1[ϕ2χ,u,ϕ2χ,ζ]=2∑1≤χ<τ≤m[α2A,u(ΥχΥτ),α2A,ζ(ΥχΥτ)],
∑ℵχ=1[υuχ,u,υuχ,ζ]=2∑1≤χ<τ≤m[β2A,u(ΥχΥτ),β2A,ζ(ΥχΥτ)],
∑ℵχ=1[ψ2χ,u,ψ2χ,ζ]=2∑1≤χ<τ≤m[γ2A,u(ΥχΥτ),γ2A,ζ(ΥχΥτ)];
where ϕχ,u,ϕχ,ζ∈χ,υχ,u,υχ,ζ∈H and ψχ,u,ψχ,ζ∈Q.
Proof. (i) It is obvious that the trace of a matrix is the same as the sum of eigenvalues of that matrix, and in the case of simple a graph, diagonal entries of a matrix are always 0.
(ii) Through the use of the trace properties in the matrix, we've obtained
Hence,
Likewise, we can show that
□
Definition 3.6. A graph in which a new vertex Υ′ is added to each vertex Υ, such that Υ′ is connected to every vertex that is connected to Υ in ϝ with the same truth, abstinence, and falsity membership degree is known as the splitting IVTSFHG S(ϝ) of an IVTSFHG.
Theorem 3.2. Let S(ϝ) be a splitting IVTSFHG of an IVTSFHGϝ. Then
Consider an IVTSFHG having the vertex sets Υ1,Υ2,…,Υℵ. The AM of ϝ is a:
and
Proof. Suppose new vertexs Υ′1,Υ′2,…,Υ′N corresponding to the vertex Υ1,Υ2,…,ΥN are built in ϝ to obtain S[αA,u(ΥχΥτ),αA,ζ(ΥχΥτ)](ϝ) is in a manner that
along the crisp values of
The notion's A[αA,u(ΥχΥτ),αA,ζ(ΥχΥτ)](ϝ) block matrix is shown following:
where
That is,
The eigenvalues of [1110] and [A(αA,u(ΥχΥτ)),A(αA,u(ΥχΥτ))](S(G)) are 1±√52 and ϕχ, where χ=1 where χ is from 1 to m. This implies that
Similarly, we can show that
and
By this, we obtain
□
Definition 3.7. A connected IVTSFHG is said to be a shadow IVTSFHG of Sh(ϝ) If it can be shown by making duplicates of G, it is referred to as G′ and G′′. Link every Upsilon prime node in G′ to the nearby residents of the associated Υ′ node in G′ using the identical truth, abstinence, and negative degree.
Theorem 3.3. Let Sh(ϝ) be a shadow IVTSFHG of an IVTSFHGϝ. Then
Consider an IVTSFHG having the vertex sets Υ1,Υ2,…,Υℵ. The AM of the graph is a
where,
Proof. Suppose new vertexs Υ′1,Υ′2,…,Υ′n corresponding to the vertex Υ1,Υ2,…,Υn are built in ϝ to obtain Sh[(αE,u(ΥχΥτ)),(αE,l(ΥχΥτ))](ϝ) in such a way that
where
The block matrix of A[(αE,u(ΥχΥτ)),(αE,l(ΥχΥτ))](Sh(ϝ)) is given below:
where
That is,
The eigenvalues of [1111] and A[(αE,u(ΥχΥτ)),(αE,l(ΥχΥτ))](ϝ) are 0,2 and ϕχ, where χ is from 1 to m, respectively. For that reason,
Similarly, we can also express that
and
The outcome is
□
Definition 3.8. Consider a TSFHDG,
The spectrum of the adjacency matrix of a TSFHDG A(→ϝ) is explained as (χ,H,Q), where P, H and Q are the eigenvalues of A(α→A(ΥχΥτ)),A(β→A(ΥχΥτ)) and A(γ→A(ΥχΥτ)), respectively.
Definition 3.9. Consider an IVTSFHDG,
The spectrum of the adjacency matrix of an IVTSFHDG A(→ϝ) is explained as (χ,H,Q), where χ, H, and Q are the set of eigenvalues of A[(α→E,u(ΥχΥτ)),(α→E,l(ΥχΥτ))],A[(β→E,u(ΥχΥτ)),(β→E,l(ΥχΥτ))] and A[(γ→E,u(ΥχΥτ)),(γ→E,l(ΥχΥτ))], respectively.
Definition 3.10. The energy of a TSFHDG,
on ℵ vertices is formulated as:
where tχ∈χ, uχ∈ H, and vχ∈Q. Record that Re(tχ), Re(uχ), and Re(vχ) denotes the real value of the eigenvalues of tχ, uχ, and vχ.
Definition 3.11. The energy of an IVTSFHDG,
on ℵ vertices is formulated as:
where tχ,u,tχ,l∈χ, uχ,u,uχ,l∈ H and vχ,u,vχ,l∈Q. Record that Re([tχ,u,tχ,l]), Re([uχ,u,uχ,l]) and Re([vχ,u,vχ,l]) denotes the real value of the eigenvalues of tχ,u,tχ,l, uχ,u,uχ,l,vχ,u and vχ,l.
4.
Randić energy of IVTSFG
The characteristics and Randić energy of IVTSFG are discussed in this section.
Definition 4.1. Let an
on ℵ vertices. An ℵ×ℵ matrix
of ϝ is defined as a Randić matrix with these condition:
Definition 4.2. Consider an IVTSFHG,
The spectrum of Randić matrix of an IVTSFHG R(ϝ) is formulated as (χR,HR,QR), where χR, HR, and QR are the set of eigenvalues of R[(αE,u(ΥχΥτ)),(αE,l(ΥχΥτ))], R[(βE,u(ΥχΥτ)),(βE,l(ΥχΥτ))], and R[(γE,u(ΥχΥτ)),(γE,l(ΥχΥτ))], respectively.
Definition 4.3. The Randić energy of an IVTSFHG,
is formulated as:
where ϕχ,u,ϕχ,l∈χ, υχ,u,υχ,l∈H and ψχ,u,ψχ,l∈Q.
Lemma 4.1. Let on m vertices an IVTSFHG,
and
be its Randić matrix. Then,
(1) Tr(R(ϝ))=[0,0];
(2) Tr(R2(ϝ))=2∑χ∼τ[1Dϝ(Υχ,u)Dϝ(Υτ,u),1Dϝ(Υχ,l)Dϝ(Υτ,l)];
(3) Tr(R3(ϝ))=2∑χ∼τ[1Dϝ(Υχ,u)Dϝ(Υτ,u)(∑s∼χ,s∼τ1Dϝ(Υs,u)),1Dϝ(Υχ,l)Dϝ(Υτ,l)(∑s∼χ,s∼τ1Dϝ(Υs,l))];
(4)
Proof. (1) Due to the fact that R(ϝ)'s orthogonal elements add to 0. So,
(2) Furthermore, we establish the Tr(R2(ϝ)) when
when
Additionally, it is useful to state that
Consequently,
(3) For R3[(α(Υχ,uΥχ,u)),(α(Υχ,lΥχ,l))](ϝ), we have
so,
Additionally, it is useful to state that
and
Hence,
(4) We must locate
Likewise, it is easy to express that
and
The outcome is,
□
Theorem 4.1. Let on m order an IVTSFHG,
Then
Additionally, the equality is valid if and only if the graph G is an IVTSFHG with a dsingle degree node and end vertices.
Proof. Let an IVTSFHG,
on m vertices. The number's range of variation |ϕχ|, where χ is from 1 to m, is defined as
Further,
Now,
Likewise,
Consequently,
More, if a IVTSFHG,
having a single degree node, then ϕχ=0 for all χ is from 1 to m, and thus
so
is an IVTSFHG having no link consequently
If IVTSFHG is ϝ possesses a single degree and a single terminal vertex, then
so the variance of
χ is from 1 to m.
Therefore,
□
Theorem 4.2. Let an
possessing at least one edge and being on m vertex. Then
Proof. Using the Holder inequality
Here the above inequality holds for any real number [gχ,uhχ,u,gχ,lhχ,l], where χ is from 1 to m. Picking
i=32 and j=3, thus, we obtain
All ϕ,χs≠0, if there is at least one edge in G. So
along with
and
Likewise,
Consequently,
□
Theorem 4.3. Let
be an IVTSFHG on ℵ vertices. Then for any graph ϝ,
Proof. Using the convex function specification as a guide, h(a)=a2, we will acquire,
Subsequently,
thus,
with i=2, we obtain
Similarly, we can simply say that
The outcome is,
□
Theorem 4.4. If
is a TSFHG with order m, then for any graph ϝ,
Proof. Every falsity-negative [a1,u,a1,l],[a2,u,a2,l],…,[am,u,am,l] holds the inequality
Then,
Consequently,
Similarly, we can also show that
The outcome is
□
Theorem 4.5. If
is a TSFHG with order m, then for any graph ϝ,
Proof. We know that
Thus, AM≥GM we concluded,
and
Thus,
Likewise, we can also express that,
The outcome is
□
Definition 4.4. Let an IVTSFHDG,
on m vertices. An m×m matrix
of →ϝ is explained as a Randić matrix with these conditions
Definition 4.5. Consider an IVTSFHDG,
The spectrum of Randić matrix of an IVTSFHG R(→ϝ) is formulated as (PR,HR,QR), where PR, HR, and QR are eigenvalues of
and
respectively.
Definition 4.6. The Randić energy of an IVTSFHDG,
is formulated as:
where ϕχ,u,ϕχ,l∈χ, υχ,u,υχ,l∈H and ψχ,u,ψχ,l∈Q.
5.
DM for human trafficking
To check the authenticity and applicability of we formulate an application that deals with human trafficking. We also perform a comparative study for checking its authenticity with the current approach. Begin by imagining a professional who wants to research the issue of human trafficking and assemble a committee of four decision-makers {Γ1,Γ2,Γ3,Γ4} from different countries to select the worst country among the five countries {Y1,Y2,Y3,Y4,Y5}. Decision makers use the criteria of IVTSFHG when comparing five countries in pairs.
Every year, millions of men, women, and children are trafficked worldwide — including right here in the United States, can happen in any community, and victims can be of any age, race, gender, or nationality. Traffickers might use the following methods to lure victims into trafficking situations:
(1) Violence;
(2) Manipulation;
(3) False promises of well-paying jobs.
Language barriers, fear of their traffickers, and/or fear of law enforcement frequently keep victims from seeking help, making human trafficking a hidden crime.
Traffickers look for people who are easy targets for a variety of reasons, including:
(1) Psychological or emotional vulnerability;
(2) Economic hardship;
(3) Lack of a social safety net;
(4) Natural disasters;
(5) Political instability.
The four administrators evaluated every pair of nations, Yχ and ♢τ, where χ and τ possess the values from 1 to 6, separately and given IVTSFV
where b is from 1 to 4 with PD [αbχτ,u,αbχτ,l], prioritize Yχ over ♢τ. The AD [βbχτ,u,βbχτ,l], where Yχ, ♢τ both have the preference. The ND [γbχτ,u,γbχτ,l], because ♢τ is not given preference over ♢χ. The suggested analytical techniques for selecting a country to rank worst are listed here.
5.1. Algorithm
Input: A unique group of nations
is set of executives
to complete the objective and infrastructure of IVTSFHPR
for each executive.
Output: Choosing the worst countries to live in.
(1) Initially determine every IVTSFHDG's energy and Randić energy. Qb (b is from 1, to a).
(2) Calculate the executive weight vector for the energy and Randi's energy of IVTSFHDGs by using
respectively.
(3) By applying IVTSFA operator and IVTSFGA operator, aggregate all [d(b)χτ,u,d(b)χτ,l] where τ is from 1 to m, corresponding to the industry Yχ and obtain the T-spherical fuzzy elements (IVTSFE), d(b)χ of the country Yχ on the whole the all other countries for the executives.
(4) Compute the collective IVTSFE dχ for countries Yχ by aggregating all d(b)χτ (b is from 1, to a) using the IVTSFWA operator and the IVTSFWG operator.
(5) Calculate the score function S(bχ,u,bχ,l) of bχ where χ is from 1 to m.
(6) Rank all the countries Yχ where χ is from 1 to m.
(7) Output the suitable country.
The TSFPRs
(b is from 1 to 4) are shown as follows:
The energy of each IVTSFHDG is formulated below:
E(Q1)=([3.890641,3.3108107],[3.944952,3.493447],[3.508376,2.75356]),
E(Q2)=([3.928878,3.431376],[3.853645,3.1132687],[3.70876,3.406579]),
E(Q3)=([3.183152,2.732871],[3.789294,3.341306],[3.852292,3.0448514]),
E(Q4)=([3.856494,3.358533],[4.0919205,3.5953166],[3.661323,3.168921]).
The weight vector of each executive can be calculated as:
The IVTSFHA operator is formulated as:
For ⋄=1 the IVTSFHA operator is d(b)χ:
All the Tables 1–4 and 6–9 are the values from theorems and definitions of results section. The aggregation results are computed in Table 5.
The TSFHWA operator is formulated as:
For ⋄=1 the IVTSFHWA operator is d(b)χ:
The cumulative IVTSFE applying IVTSFHWA operator are formulated as:
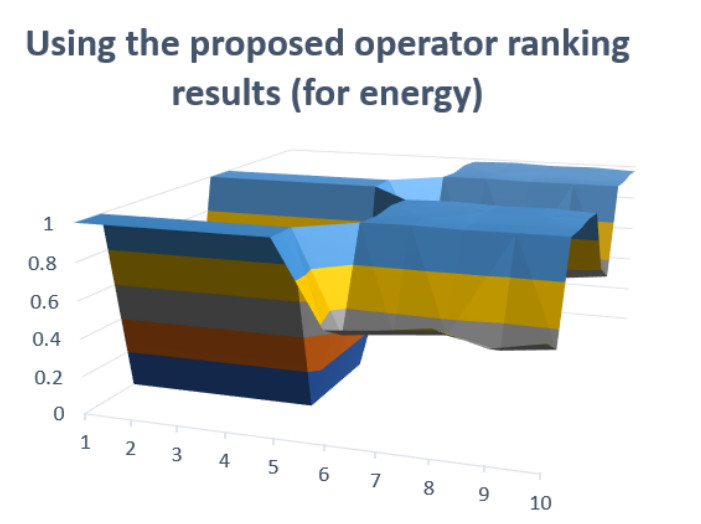
The graphical depiction shown in the Figure 1.
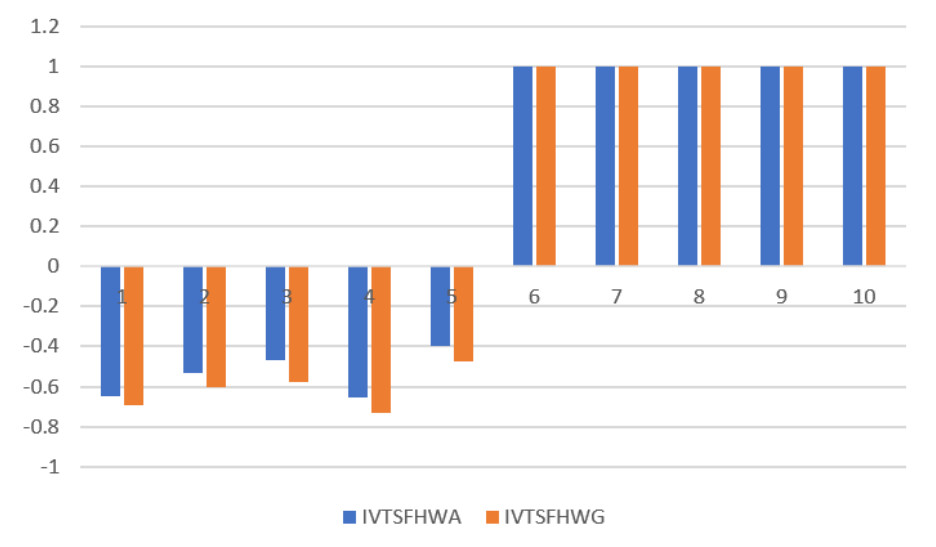
The score function S(dχ) of dχ, where χ is from 1 to 5, is calculated as:
The worst country with respect to human trafficking is ♢3.
Then, similarly, with the same procedure using the above (IVTSFHG) operator, we obtain these results:
The score function S(dχ) of dχ, where χ is from 1 to 5, is calculated as:
The worst country regarding human trafficking is ♢5.
The IVTSFHG operator is formulated as:
The Randić matrix of the IVTSFPRs
where (b is from 1, to 4) are shown as follows:
The Randić energy of each IVTSFHDG is formulated below:
The weight vector of each executive can be calculated as:
Weight vector of four executive xb where (b is from 1, to 4) is:
The IVTSFHA operator is formulated as:
The aggregation result is computed in Table 10.
The cumulative IVTSFE applying IVTSFHWA operator is formulated as:
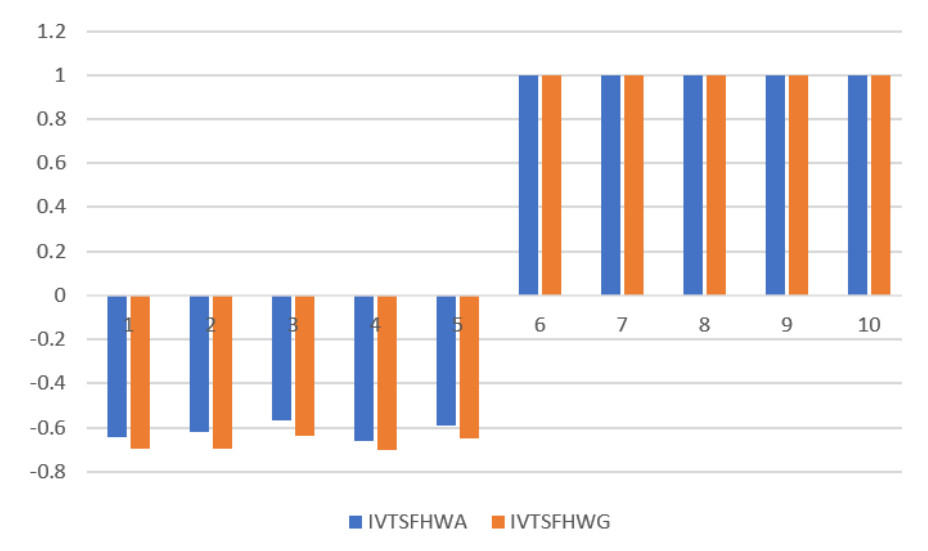
The score function S(dχ) of dχ, where χ is from 1 to 6, si calculated as:
Then,
The worst country is♢2. The IVTSFHG operator is formulated as:
Then, similarly, with the same procedure using the above (IVTSFHG) operator, we obtain these results:
The score function S(dχ) of dχ, where χ is from 1 to 6, is calculated as:
so this implies ♢3 is the worst country among all.
5.2. Comparitive study
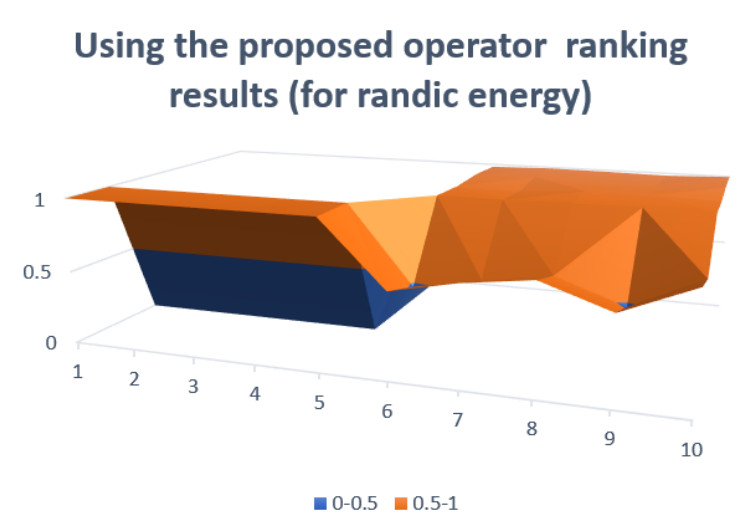
This subsection presents the results of the previous numerical example using specifically established operators. The IVTSF Dombi weighted arithmetic (IVTSFDWA) and IVTSF Dombi weighted geometry (IVTSFDWG) operators are utilized to compare the outputs of the currently employed model. The rankings generated by these operators are summarized in Tables 11 and 12. Table 11 confirms that our proposed method aligns with the rankings obtained from the IVTSFDWA of energy, thus validating our approach's effectiveness. However, the results in Table 12 do not align with our approach. This discrepancy results from Randic's energy and the energy of IVTSFHG being calculated using various aggregation techniques or slightly changing the value produced by the scoring function.
Figures 2–4 show graphical representations to contrast the suggested technique with the current approach. To generalize algebraic operations, it is essential to use the TNs and TCNs developed by Hamacher and Einstein. The Hamacher operator can be either an algebraic TN or TCN depending on the value of parameter 1, and it can be either an Einstein's TN or TCN depending on the value of parameter 2.
5.3. Comparative experiments with existing methods
The Hamacher parameter μ plays a crucial role in the aggregation process. We propose analyzing its impact through:
Table 13 shows how varying μ affects the decision outcomes:
The analysis reveals that while absolute scores vary with μ, the relative ranking remains stable, demonstrating the robustness of our method.
Comparative analysis. We compare IVTSFHG with IVIFS and IVPFS approaches using the same dataset shown in the Table 14:
5.4. Discussion
Interval-valued T-spherical (IVT−spherical) serves as an enhanced version of the spherical fuzzy set (SFS) model, which provides greater flexibility in handling spaces with larger values. When considering truth order (α), abstinence order (β), and negative order (γ), the constraint α,uT+β,uT+γ,uT≤1 (T≥1) in the TSFS model facilitates more precise value definitions. In the PFS model, the energy and Randić energy cannot be computed using the HWA and HWG operators, resulting in an inability to rank the outcomes. This occurs when the sum of PD, AD, and ND exceeds 1. Tables 11 and 12 display the rankings of energy and Randić energy achieved through the PFS, SFS, and TSF models. Due to the sum of squares of PD, AD, and ND being greater than 1, analysis using the PFS model is impractical. Nevertheless, the calculation is feasible when T=3. Specifically, TSFS is PFV for T=1 and SFV for T=2. As T increases, so does the space and its corresponding value. For instance, (0.9,0.4,0.5) represents a 3−SFV. In the case of
but
and
Consequently, the PFS and SFS models lack the capability to handle this data adequately. Hence, (0.9)+(0.4)+(0.5) is neither SFV nor PFV. Thus, all PFVs are SFVs and TSFS. However, the PFS and SFS classes do not fall under the TSF category. The assessment of the energy and Randić energy in TSFHDG designates Y3 as the least favorable country.
6.
Conclusions
In order to enhance the efficacy of our methodology, we incorporated investigations on data mining, encompassing topics such as human trafficking, as well as algorithms. The IVTSF Hamarcher operator was employed in the study to ascertain the energy values of both IVTSFG energy and Randić energy. The arrangement of characteristics is contingent upon the Randić energy and the energy of IVTSFHDG. The optimal choice is the variable ♢, as it remains consistent across all circumstances. The study yields a novel way for addressing challenges encountered in the data management process, while also facilitating the establishment of new frameworks for measuring information.
The current state of the discourse about DM frequently exhibits characteristics of illogicality and ambiguity. The accurate prediction of future outcomes may be hindered by the presence of insufficient knowledge. The concept of IVFS offers a theoretical foundation for effectively managing the provided data. The effectiveness of IVTSFG surpasses that of IVIFS, IVPFS, PFS, and IFS due to its ability to address a diverse range of real-world problems based on triplets. We have introduced a novel notion, denoted as IVTSFHG, by employing the Hamacher operator. This idea allows us to investigate the Randić energy as well as the energy associated with IVTSFHG. In addition, the division of IVTSFHG and the allocation of shadow IVTSFHG energy are established. In addition, our discussion also encompassed the examination of the energy of the molecular structure denoted as IVTSFHG, as well as the Randić energy associated with IVTSFHG.
6.1. Future research directions
● Conduct systematic sensitivity analysis of the Hamacher parameter μ to understand its impact on decision outcomes.
● Extend the methodology to other complex decision-making problems beyond human trafficking assessment.
● Develop hybrid models combining IVTSFHG with machine learning techniques for enhanced predictive capabilities.
● Investigate the scalability of the approach for high-dimensional decision problems.
6.2. Limitations of the existing methodologies
Limitations of the existing methodologies are summarized as follows:
(1) q-ROFG, IVq-ROFG were considered good additions in the fuzzy world, but these concepts are not sufficient to symbolize human opinion properly due to the statistics of the scenario which will have an element of hesitant degree in a variety of issues and applications. In such situations, the decision-makers are not sure to give results according to the provided data.
(2) The scenario we discussed in this article gave data in the form of all four fuzzy components, which makes it unsolvable for either an IVIFG, IVTFS, or IVPyFG.
(3) Furthermore, the q-ROFG, IVq-ROFG and their operations which are currently used to solve real-world issues are not enough to deal with the information having a refusal degree as well.
(4) SFS, TSFS are comparatively more convenient ways to solve complex real-world problems. But IVTSFS is much better than these two methods.
Author contributions
Ali Ahmad: conceptualization, methodology, investigation, writing — original draft, funding acquisition; Humera Rashid: conceptualization, methodology, software, data curation, writing — review & editing; Hamdan Alshehri: project administration, supervision, writing — review & editing, funding acquisition; Muhammad Kamran Jamil: data curation, investigation, validation, verification, writing — original draft; Haitham Assiri: formal analysis, investigation, resources, visualization, writing — original draft. All authors have read and agreed to the published version of the manuscript.
Use of Generative-AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The authors gratefully acknowledge the funding of the Deanship of Graduate Studies and Scientific Research, Jazan University, Saudi Arabia, through project number: RG24-M039.
Conflict of interest
The authors declare that there are no conflicts of interest.









 DownLoad:
DownLoad: