1.
Introduction
Energy is one of the needs of human life. Increasing energy demand is an increasing prosperity indicator, but there are still problems in the energy supply business. Energy use increases with population growth. Large energy consumption causes energy availability to run low. Electricity consumption in Indonesia reaches 202,845.82 GWh. It is a quite large nominal compared to some other countries in Southeast Asia.
Indonesia is a tropical country that has considerable solar energy potential. The potential of solar energy in Indonesia is around 4.8 kWh/m2/day. There are two types of technology used to exploit the potential of solar energy, namely thermal solar energy technology and solar panel energy. Solar panel energy is used to meet the needs of electricity, water pumps, televisions, telecommunications, and refrigerators in remote areas. The alternative electrical energy produced by solar panels is usually stored first in a storage system (battery) before use. Solar panels become a renewable energy source that offers many advantages, including the energy used, comes from the sun, does not produce pollution, low maintenance costs, and does not produce noise.
The conversion of solar energy into electrical energy depends on the angle of reception of solar radiation. There are two types of use of panels, namely the PV fixed and solar tracker. Nowadays a lot of optimization has been done on solar panels, with solar tracking systems. The benefit of solar tracker systems is to optimize the collection of solar energy [1]. There are two types of solar tracking based on the axis of the system, namely a single axis and double axis [2,3,4]. The efficiency of dual-axis solar tracking systems is known to be 81.68% higher than in fixed panels, while the efficiency of single-axis tracking is only 32.17% higher than in fixed panels [5]. Solar tracking research focuses on adding the Maximum Power Point Tracking (MPPT) algorithm to the PV panel system. The output power of PV is influenced by the level of solar irradiation and the temperature received by the PV panel surface. If the irradiation received by the PV panel is higher then the resulting current will be higher, but if the panel temperature is too high the voltage obtained will be lower. There have been many studies on MPPT, one of which uses fuzzy Particle Swarm Optimization (PSO) [6]. From the results of experiments and simulations it is known that tracking time and accuracy increase by 0.88% and 0.93% of fuzzy without PSO [7].
The construction of solar power plants requires very good planning so as not to cause excessive impact on the environment. To build a solar power plant requires an initial investment that is quite expensive, so it needs to be designed components of solar power plants, one of them is a solar charge controller that is reliable, optimal, efficient, and economical so that solar power plant development planning obtained efficiently and optimally. The sepic converter already design in 2008 without a controller [8]. The MPPT algorithm using Perturb and Observe (P & O) technique can get maximum power from the PV panel so that it can charge the battery to the maximum [9,10]. The battery charging using a fuzzy logic controller can track setpoint with a minimum time [11]. The method can resolve problem P & O technique with better result or more accurate. The MPPT based on Particle Swarm Fuzzy can increase the power output of solar tracker than using MPPT based fuzzy [6]. In this research, apply the MPPT Particle Swarm Fuzzy on the battery charging system of solar tracker. The purpose of this research can absorb maximum energy from the sun to the charging system. The design of a charging system controller where the setpoint charge will adjust to the state of the battery voltage so that this controller will work efficiently and optimally. This research is expected to be able to good contribute to solar power plants development planning.
2.
Methodology
2.1. System specifications
In this research, the PV module used is a type of polycrystalline solar module with a nominal power of 250 Wp. Table 1 presents the PV specifications as provided by the manufacturer datasheet.
Figure 1 is a characteristic curve of solar panels resulting from simulation under Standard Temperature Condition (STC).
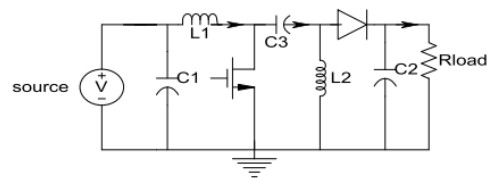
Meanwhile, the converter used is a Single-Ended Primary-Inductor (SEPIC) type converter. The Sepic converter is capable of producing above or below the input voltage without reversing the input voltage pole.
Figure 2 explains a SEPIC converter circuit with the component parameters. The component parameter value of a SEPIC converter can show in Table 2.
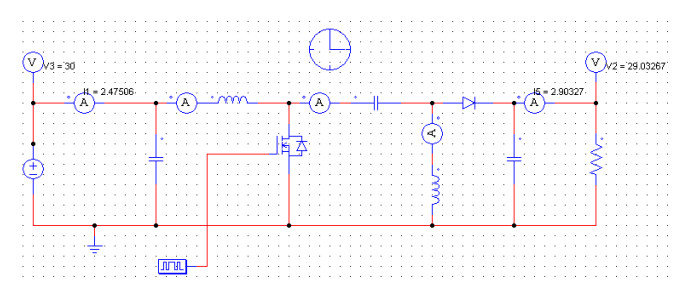
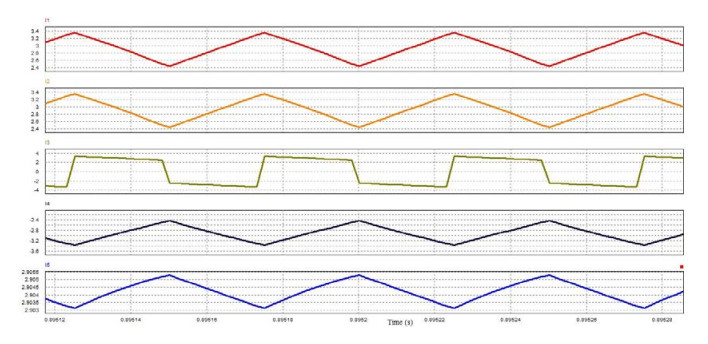
After entering the SEPIC converter parameter values in the circuit, the circuit modeled on PSIM as shown Figure 3. Converter modeling in PSIM aims to determine the voltage and current characteristics through the component without taking into account the specifications used. Figure 4 explains the current characteristics of SEPIC circuit.
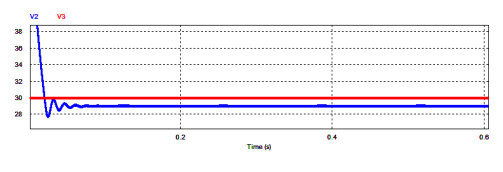
Figure 5 shows the results of the SEPIC converter modeling response. The result of the input voltage is 30 V, while the output voltage is 29 V. The SEPIC converter can produce lower or higher output voltages than the input voltage. The output voltage polarity is directly proportional to the input voltage. It shows that the modeling is already appropriate with existing conditions. The MPPT Algorithm using the P & O technique can only get Maximum Power Point (MPP) on the converter. The MPPT based fuzzy logic controller can maintain the MPP even though there is the load changes. Increased power generated by MPPT sought by optimizing fuzzy membership using PSO. This method can increase maximum power than using fuzzy MPPT [6].
2.2. Retrieval of primary data
Primary data collection includes data irradiation, panel temperature, voltage, and current. The 12 Ah battery function to load that first connected to the Solar Charge Controller (SCC). The data collected on 17 April 2018 at 3rd floor of the S2 building in the Department of Physics Engineering ITS start from 6:00 to 17:00 WIB. This location avoid from shading that can obstruct irradiation to the solar panel surface. Irradiation measurements are carried out using a pyranometer. Panel temperature measurements are performed using an infrared thermometer. Current and voltage measurements are carried out with a digital multimeter. The retrieval data results of solar irradiation and panel temperatures for one day (11 hours of operation) can show in Table 3. The highest panel irradiation and temperature obtain at 11:30 with a value of 1011,333 W/m2 and 61,667 ℃. Meanwhile, the maximum power of solar panel occurs at 12:30 with value 196.99 watt or 28.86 volt and 6.826 ampere. The measurement result can be influenced by some factor as wind, wheater, solar panel position, etc at the time.
2.3. Design of Fuzzy-MPSO controls
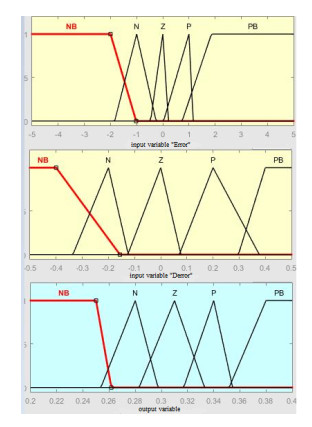
There are three elements of fuzzy control, namely fuzzification, inference system, and defuzzification. The fuzzy control system is used to track the output of the SEPIC converter to match the battery's charging voltage. Inputs given to the controller are voltage errors (error) and voltage delta error (derror), while the output is the duty cycle (D). The voltage error is the difference between the setpoint and the actual voltage, while voltage delta error is the difference between the actual voltage and the previous voltage. The duty cycle of Pulse Width Modulation (PWM) is the pulse width used to set the switching speed on the converter. To set the duty cycle, a Linear Decreasing Weight Particle Swarm Optimization (LDWPSO) control is needed [12]. The Membership Function (MF) used is the type of triangle and trapezoid with 3 MF triangles and 2 MF trapezoid. The MPSO (Modified Particle Swarm Optimization) used is LDWPSO. LDWPSO is a modification of PSO which modifies the weight of inertia to decrease with each additional iteration. Figure 6 is the MF of fuzzy input and output that has been optimized by LDWPSO.
The MPPT with the P & O technique only has two inputs. If the voltage is less than zero, then the PWM will go up. If the voltage is more than zero, then the PWM will go down. Meanwhile, the fuzzy logic controller has every two inputs and one output divided by the five membership functions of fuzzy. This method can increase the power at the PV output rather than the P & O technique. The MPPT fuzzy MPSO is applied to control the output of the converter [6]. The rule base is used to control the pulse width with two inputs that are errors and delta errors. The rule base design of fuzzy control can explain in Table 4. The rule base that has been designed using the AND operator. For example, a rule (1), if the error value enters the Negative Big (NB) membership function range and the delta error enter Negative Big (NB) membership function range, then the duty cycle value enter Negative Big (NB) membership function range. If occur errors and delta errors are very big, then the duty cycle of PWM will go up very fast. (IF e(NB) AND de(NB) THEN duty cycle(PB) etc and applies to all rules).
3.
SEPIC converter system
Characteristics test of the SEPIC converter designed with several variations of the switching frequency and duty cycle. The purpose of this test is to determine the characteristics of the converter. Frequency variation testing is performed to determine the effect of switching frequencies used on the output voltage. Frequency Switching testing is carried out in the range of 10,000 Hz–60,000 Hz. The tests use a DC voltage source in the form of a power generator (16 V/2 A), a 6.8 Ohm converter load and a 30% duty cycle.
Table 5 shows the effect of switching frequency on the SEPIC converter output. NA (Not Available) is a condition where the generator cannot produce the desired input voltage. The switching frequency not visible significantly influence the output voltage produced by the converter. The converter starts properly working when given a switching frequency of 30 kHz or more.
The duty cycle variation aims to determine the duty cycle effect on the output voltage generated by the converter. The tests use a DC generator (16 V/2 A), a resistor load of 6.8 Ohm and a switching frequency of 50 kHz. The results of testing the duty cycle variations shown in Table 6. It shows the SEPIC converter output voltage response with duty cycle variations. The output voltage value is high if the duty cycle value is also high.
4.
Results and discussion
4.1. Setpoint tracking simulation of fuzzy and Fuzzy-LDWPSO
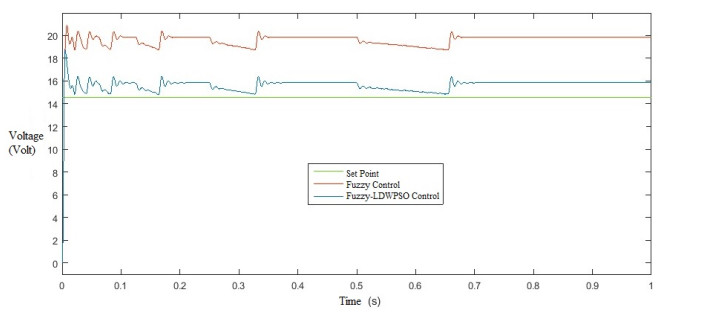
The function of tracking simulation is to find out the comparison between fuzzy controller and fuzzy-LDWPSO that has a design for tracking setpoint charging (14.55 V). The simulation is carried out on STC conditions, namely irradiation conditions of 1000 W/m2 and Temperature 25 ℃. Figure 7 shows the red line is SEPIC converter output voltage using fuzzy control. The blue line is the output voltage with fuzzy-LDWPSO control while the green line is setpoint voltage 14.55 V. The voltage ratio experienced by the fuzzy control is in the numbers 15.8 V to 19.8 V while the fuzzy-LDWPSO has an output voltage principle at 14.7 V to 16.3 V.
Table 7 shows that fuzzy-LDWPSO control has a faster response than fuzzy control. It can be seen based on the decrease in rise time, settling time, and peak time. The maximum overshoot and steady-state error values of the fuzzy-LDWPSO control are smaller than fuzzy. Based on the data in Table 7, fuzzy-LDWPSO is better in tracking the charging voltage by reducing the maximum overshoot by 16.14% and reducing the steady-state error by 27.49% from the fuzzy control.
4.2. Tracking test simulation of fuzzy and Fuzzy-LDWPSO with variations in climatic conditions
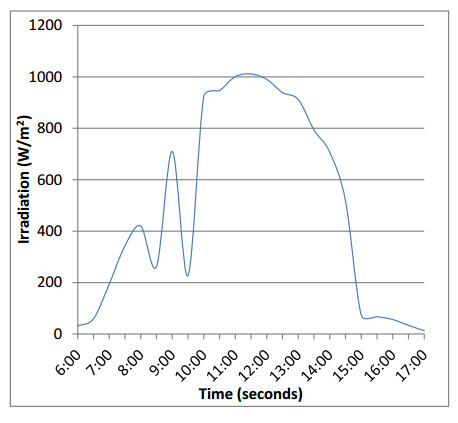
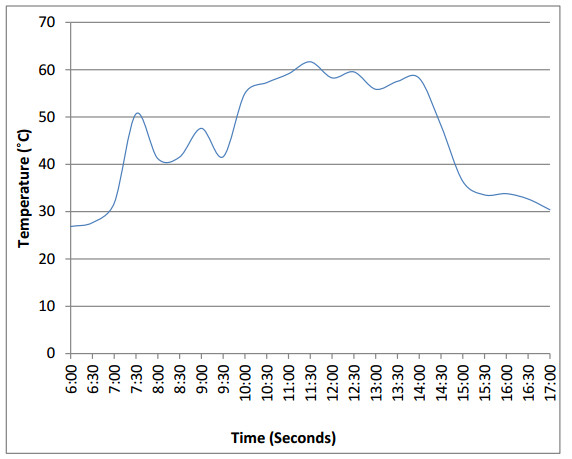
The test is performed to compare between fuzzy and fuzzy-LDWPSO control in tracking setpoint voltage with variations conditions of temperature and irradiation. Temperature and irradiation variations are used based on the results of secondary irradiation and temperature data collection on 17 April 2018. Figure 8 shows the results of irradiation measurements for 6 hours, start from 6.00 WIB until 17.00 WIB. Based on the graph, the biggest irradiation occurred at 11:30 WIB. The panel surface temperature measured for 10.5 hours shown in Figure 9. The highest panel temperature reached at 11:30 with the temperature recorded on the measurement of 61.66 ℃. The charging voltage tracking test is performed to determine the performance of the fuzzy and fuzzy-LDW-PSO controls that designed. The results of the tracking setpoint test with variations in climatic conditions shown in Figure 10.
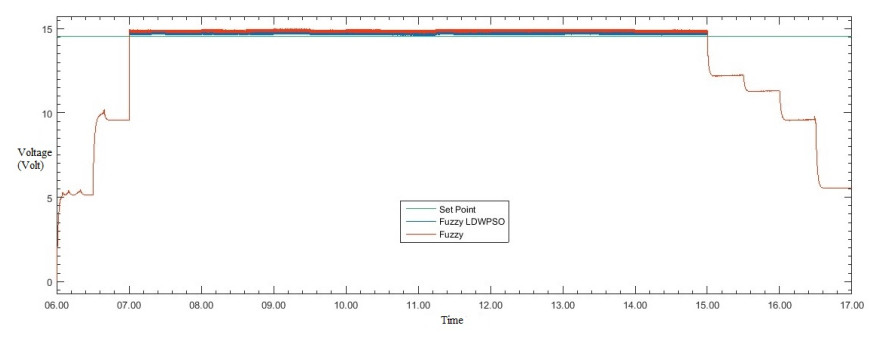
In Figure 10, the red line is the results of the output voltage control generated by fuzzy control while the blue line is the fuzzy-LDWPSO output voltage. The voltage ripple that generated Fuzzy located between 14.8 V and 15 V, while the ripple voltage of fuzzy-LDWPSO is in the numbers 14.6 V to 14.8 V. The charging voltage using the fuzzy-LDWPSO control is closer to the setpoint than the fuzzy control. At 15.00, the voltage decreases below the set point because when the irradiation data is collected and the temperature at that hour the sun is covered with thin clouds, so the control cannot track the setpoint voltage.
4.3. Hardware test with PV fix and tracking conditions
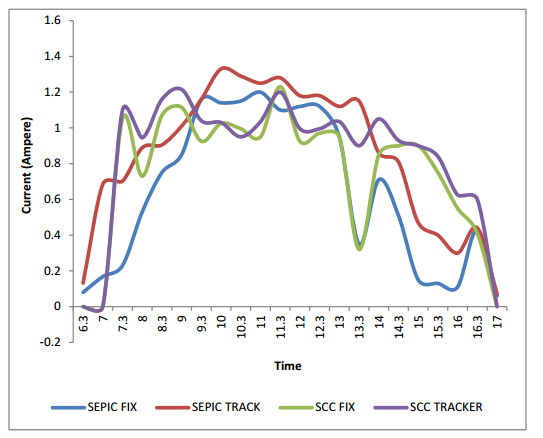
Hardware testing for fix and tracking conditions was carried out on 1 June 2018 in the car park area of the ITS Physics Engineering Department. The tests use 2 PVs with a capacity of 250 WP each with fix and track conditions. The function of retrieval data using SCC is to compare with the converter that has designed. Data collected during sunny cloudy.
The charging current that enters using SCC is very volatile, so it records the maximum and minimum currents that are read by the ampere meter. The charging current entering using the SEPIC experiences a ripple of around ± 0.01 A that read on the ampere meter. PV with a tracking condition produces a charging current is greater than the PV condition fix. The increase in charging current generated by the tracking conditions using the SEPIC converter with fuzzy-LDWPSO Control was 36.15% and using SCC increased by 11.61% from the fix condition panel.
5.
Conclusions
To find out the response of the fuzzy output can be approached by finding the value of rise time, settling time, maximum overshoot, peak time, and steady-state error. After knowing the results of their responses, we can find out which controller has a good response with the smallest error. The fuzzy control has a value of rise time 0.0027 seconds, settling time 0.0189 seconds, maximum overshoot 43.64%, peak time 0.008 and steady-state error 36.08%. fuzzy-LDWPSO control has a value of rise time 0.0021 seconds, settling time 0.0135 seconds, maximum overshoot 27.5%, peak time 0.0056 seconds, and steady-state error of 8.59%. The fuzzy-LDWPSO control has a better [13] response than the fuzzy controller with response faster and has error smaller. After getting the best controller, the fuzzy-LDWPSO control entered to battery charging system. The SEPIC charging circuit uses a fuzzy-LDWPSO control with a panel tracking condition capable of increasing the battery charging current by 36.15% from the panel condition fix.
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: