This paper tries to empirically examine the exchange rate deviations to its level under the purchasing power parity (PPP) and transaction costs hypotheses using a battery of newly developed nonlinear approaches. To explain the persistent differences in exchange rates,we use the half-life function analysis with quarterly data over the period 1988Q1–2018Q2 for a panel of 23 developed and emerging countries. Our results show that some deviations to PPP relation may be governed by non-linear dynamics. The adjustment toward the fundamental equilibrium seems to depend on the size and the sign of the gap from the PPP equilibrium. The foreign exchange rate adjustment can be modeled by symmetric process with the ESTAR model and asymmetric process with LSTAR model.
1.
Introduction
Alzheimer's disease (AD) is a fatal neurodegenerative disorder that is the most prevalent form of dementia. The neuropathological and neurochemical hallmarks of AD include: Synaptic loss and selective neuronal cell death; decreases in markers for certain neurotransmitters; and abnormalities in neurons and their processes as well as in the extracellular space. Two of the main features associated with AD are neurofibrillary tangles comprised of the protein tau and cerebrovascular, diffuse, and neuritic plaques composed predominantly of the amyloidogenic peptide amyloid-β (Aβ). These proteinaceous deposits of tau and Aβ consist of stable amyloid fibrils, which are β-sheet rich fibrous protein agammaegates. Similar deposition of amyloid is associated with numerous other diseases [1].
In the early 90's, the amyloid cascade hypothesis was introduced, which postulated that Aβ agammaegation and deposition directly lead to neuronal death, resulting in AD [2]. Yet, the correlation between plaques and cognitive dysfunction in AD has been questionable for years [3]–[7], and with Aβ-directed therapeutic strategies failing in numerous clinical trials, the role of Aβ in AD progression is being re-evaluated [8]–[11]. This has led to an enhanced research focus on diffuse, soluble agammaegates of Aβ. Various small, globular agammaegates of Aβ, referred to broadly as Aβ oligomers (AβO), were detected in AD patients a few decades ago [12]–[14], and these AβO were originally classified as being intermediates toward the formation of amyloid fibrils. Over the years, increasing evidence points toward AβOs playing a central role in AD, as AβOs correlate more strongly with AD progression in patients and animal models [15]–[19]. For example, AβO formation and buildup occurs early compared with plaque buildup (much earlier than plaque deposition) in the AD brain [20]–[22]and CSF [23], which has led to extensive efforts to develop assays to detect AβOs for potential application as biomarkers [24]–[31].
An extensive body of evidence has linked high levels of AβOs in the brain to a variety of pathogenic consequences associated with AD (summarized in Table 1). As such, there has been significant effort made to characterize AβO formation, structure, and biochemical/biophysical characteristics (such as interactions with other proteins and lipids) in the hope that underlying modes of AβO-related toxicity could be revealed [32]–[37]. As AβOs are metastable, highly heterogeneous in nature, and can form via a variety of different pathways, this remains a challenging task, yet progress has been made. Here, we review AβOs with a focus on biophysical characterization of their formation and structure.
2.
The Aβ Peptide
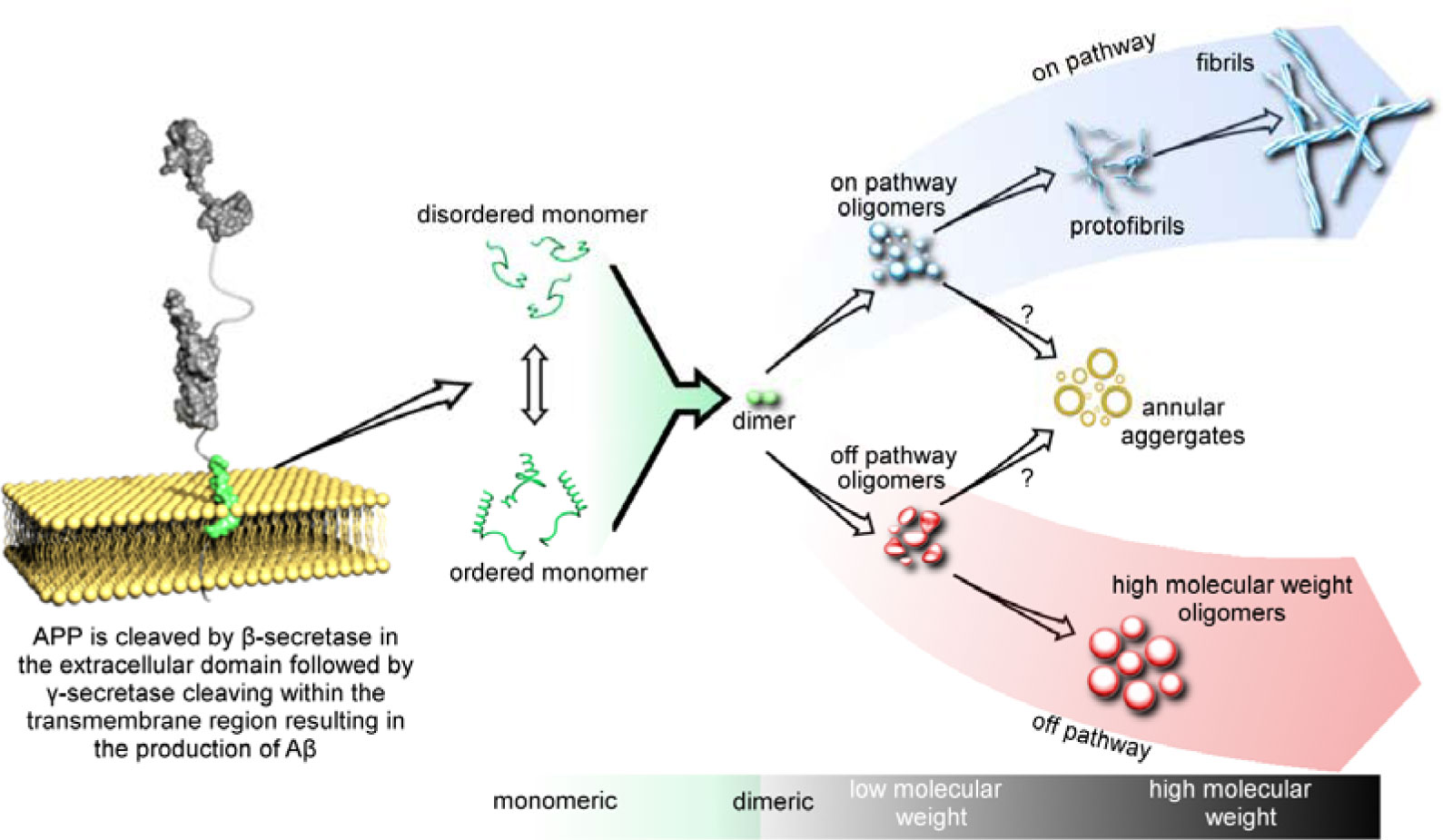
Aβ is an approximately 4 kDA peptide (typically 40–42 amino acids long) that is derived from the transmembrane portion of the amyloid precursor protein (APP; Figure 1). The production of Aβ is achieved by the sequential cleavage of APP by two membrane-bound endoprotease activities, β- and γ-secretase. The two predominant Aβ peptides produced are 40 and 42 amino acids in length, and these peptides are referred to as Aβ40 and Aβ42 respectively. Aβ is amphipathic in nature (having a predominately hydrophilic N-terminus and a predominately hydrophobic C-terminus), which is thought to drive its agammaegation. As the C-terminal end of Aβ coincides with the transmembrane portion of APP, Aβ42 has a larger hydrophobic domain, making it more fibrillogenic compared to Aβ40 and deposits to a much greater extent in the brain [68]–[70]. Only about 10% of APP is processed via this Aβ producing pathway. Most APP is cleaved by the α-secretase, generating a series of much more benign peptide fragments.
The hydrophilic N-terminal region of Aβ can adopt both an α-helical or β-sheet structure dependent on solution conditions, for example pH [71],[72]. The hydrophobic C-terminal end of Aβ has a propensity to adopt β-sheet structure upon agammaegation independent of solvent conditions [71],[72]. Beyond its amphipathic nature, several other domains have been identified in Aβ. The different polyomorphic fibril structures of Aβ are comprised of bundled β-sheets with backbones orthogonal to the fiber axis creating a cross-β structure [73], and two β-strand forming domains (residues 11–21 and 29–39 respectively) that are separated by a turn/bend region (around residues 23–26) identified through various experimental and computational studies [74]–[77]. The central region of Aβ (residues 16–21), contained within one of the β-strands, has enhanced amyloidogenic properties and represents a hydrophobic core [78].
3.
Aβ aggregation—a complex mechanism
The agammaegation of Aβ (and other amyloid-forming proteins) is typically characterized in terms of fibril formation (Figure 2). Aβ fibril formation occurs via a complex agammaegation pathway. Fundamentally, AβOs can be subdivided into species that are intermediates in fibrils formation (referred to as being on pathway) or species that do not directly lead to fibrils (referred to as being off pathway). This is a contributing factor to the immense heterogeneity observed in AβO populations as will be discussed in more detail later. In general, amyloid formation proceeds via a nucleation dependent polymerization mechanism [79]–[81]. With this mechanism, agammaegation initially occurs via a slow nucleation phase (often called the lag phase) that involves the formation of a thermodynamically unfavorable critical nucleus that is associated with a transition from a native to non-native protein conformation. For Aβ, the critical nucleus is likely a multimeric species [82]–[84]. Once the critical nucleus has formed, an elongation or growth phase (characterized by a relatively rapid extension of fibril agammaegates) occurs. While numerical models can extract important parameters (lag phase times, elongation rates, critical nucleus size) from experimental data [85], the actual agammaegation pathway toward fibrils can be complicated. For example, other on pathway intermediates, like protofibrils, are also observed in Aβ agammaegation. Protofibrils are amyloid-like, elongated agammaegates with filament-like morphologies and are late-stage intermediate precursors on the agammaegation pathway to fibrils. A key aspect that facilitates on and off pathway agammaegation routes and complicates investigations of AβOs is that they possess structural plasticity and are metastable and transient in nature.
Further complicating the issue is the observation that Aβ can agammaegate into a variety of morphologically distinct fibril structures, referred to as polymorphs [86]–[90]. This phenomenon is predicated on subtle changes of environmental conditions associated with agammaegation, and as a result, preparatory protocols employed in experiments determines the resulting Aβ fibril morphology [87]. While polymorphic agammaegates are readily observed with in vitro studies using synthetic Aβ, polymorphic structures have been observed in amyloids derived from tissue, and it is thought that variations in Aβ agammaegate morphologies may play an important role in AD [91],[92]. For example, polymorphic agammaegates and fibrils may result in distinct biological activities and levels of toxicity that could underlie variations in AD [76], and distinct fibril structures can be directly associated with individual AD patients and clinical phenotype [93],[94].
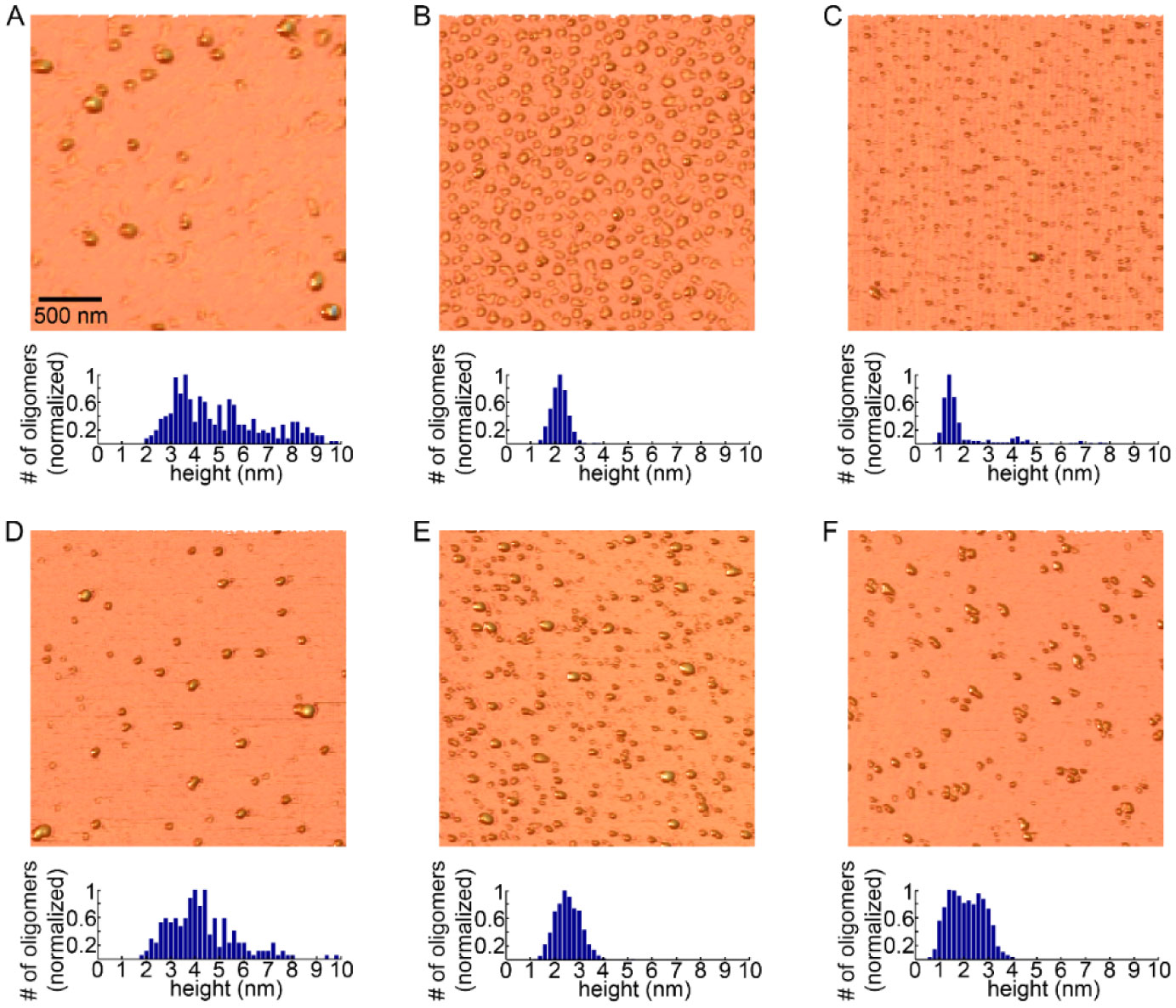
A complicating factor in evaluating and comparing studies aimed at elucidating AβO formation, structure, and physiological impact is divergent experimental conditions, such as Aβ preparation protocols, heavily influences experimental outcomes. In terms of oligomers, the emergence of distinct fibril structure strongly suggests that there would also be distinct oligomeric precursors associated with their formation. Indeed, distinct AβO species can be observed within in vitro agammaegation assays under conditions that result in fibril polymorphs [95] (Figure 3). For studies conducted with synthetic peptide, there are a variety of protocols used to solubilize Aβ (Table 2). Typically, these protocols consist of a disagammaegation step and a reconstitution step. The disagammaegation steps usually involves the use of hydrogen bond disrupting solvents, i.e., hexafluoroisopropanol (HFIP) or trifluoroacetic acid (TFA), that break down pre-existing agammaegates within lyophilized stocks of Aβ. These solvents are often removed under vacuum, leaving a peptide film. The reconstitution step involves dissolving these peptide films into a solvent that facilitates dilution into an appropriate buffer. Dimethyl sulfoxide (DMSO) is often used, creating a concentrated stock that is diluted into the desired buffer. Disagammaegation and reconstitution can also be facilitated by changes in pH. Sometimes reconstitution is performed directly into the final buffer. Importantly, variations in preparatory protocols indeed result in different populations of oligomers (Table 2 and Figure 3), and these variations can complicate direct comparisons between different reports within the literature. Further complicating the issue, there are often distinctions observed between studies performed with synthetic Aβ and naturally derived Aβ [96]. In many studies aimed at elucidating activity of AβOs, specific preparations are used to obtain a particular population of oligomer species. These are then directly applied to different model systems, ranging from cell culture to animal models. However, there is often a lack of effort to verify that once added to the model system (which can often be a pronounced change in chemical environment) that these AβO species do not dissociate or agammaegate into a different AβO or Aβ agammaegate. To truly relate specific AβOs to a neurotoxic activity, effort should be invoked to attempt additional controls of this type.
4.
Classifying AβOs
Enormous efforts have been extended in identifying the most toxic/disease-relevant AβO species and the relevant underlying structure [9],[106],[107]. This is a daunting endeavor due to the transient nature and extensive heterogeneity associated with AβOs [108]–[110]. It is possible that different AβO species may activate different deleterious changes associated with AD [9],[106],[107]; however, there may also be relatively benign AβO species or even experimental artifacts [96],[108],[111]. Unraveling the physiological activity of AβOs may require separate analysis of these different species, which may be altered by preparatory protocols. The ability of smaller AβO (like dimers and trimers) to further agammaegate into large, more stable synaptotoxic assemblies [107] must be accounted for in assessing the toxic effects of specific AβO species. That is, upon the addition of preparations of AβO to cell culture or other models, AβOs may further assemble into other higher order species that may influence the associated toxic effects. Despite the inherent complexity of this endeavor, progress has been made. Aβ trimers have been linked to playing a role in inducing pathological conformational changes in tau [112]. However, crosslinked Aβ dimers were shown not to be toxic themselves, but rather contributed to toxicity by further assembling into larger assemblies [113]. A 56 kDa SDS-stable Aβ0 (referred to as Aβ*56) has been identified as a prominent specie in the AD brain [16], CSF [22], and in transgenic mouse models of AD [32],[114]. In terms of an actual biological activity, Aβ*56 interacts with N-methyl-D-aspartate receptors (NMDARs), increasing NMDAR-dependent Ca2+ influx and activation of Ca2+/calmodulin-dependent kinase IIα (CAMKIIα) [115]. Activation of CAMKIIα correlates with enhanced site-specific phosphorylation and mis-sorting of tau [115]. Smaller AβOs, namely dimers and trimers, do not appear to elicit these specific effects.
There appears to be some common themes emerging with respect to toxicity. Toxic AβOs appear to react with oligomer specific antibodies like A11 (generic for amyloid oligomers in general, [116]) and NU4 (specific for AβOs) [117]; whereas, nontoxic AβOs demonstrate reactivity with anti-fibril antibodies like OC [116]. Importantly, toxic AβOs appear to be unrelated to plaques [116],[118]. AβOs related to amyloid plaques temporally, spatially, and structurally are nontoxic [118]. A number of toxic AβO species are larger than 50 kDa [21],[54],[118], like the previously mentioned Aβ*56 [32]. Smaller AβOs appear to be less toxic or even benign [21],[54],[118],[119], except for their ability to further agammaegated into larger assemblies. This has led to distinguishing between high molecular weight (HMW) and low molecular weight (LMW) oligomers [120]. Agammaegation mechanisms differentiating between the eventual formation of HMW and LMW AβOs already appear to deviate at the dimer stage [121]. Furthermore, LMW and HMW Aβ oligomers differentially impact synapses and memory [122],[123]; although, LMW AβOs are not always associated with memory dysfunction [116],[117]. HMW AβOs are the predominant Aβ species in the native soluble protein fraction of AD brains [124]. These HMW species in the AD brain sometimes appear to be constructed from smaller ∼7 kDa Aβ species [125]. Neurohistopathological and biochemical analyses of AβOs in the temporal cortex of AD brains implicated an Aβ dodecamer (∼55 kDa) [126]. HMW AβOs bind cultured synapses [21],[54],[118], induce reactive oxygen species (ROS) production [123], and disrupt memory function [116],[117]. With respect to the previously defined agammaegation pathways, LMW AβOs are typically on pathway to fibril formation; HMW AβOs are off-pathway [127],[128]. This is consistent with HMW AβOs being potent, as off pathway AβOs appear more toxic [129]. Collectively, these observations point to the complex interplay between different AβO species and their specific activity with respect to neurotoxicity.
5.
Characterizing AβO structure and activity
To facilitate structure/activity analysis, significant efforts have been made to obtain structural details of AβOs using a variety of methods (Table 3). This is required to fully elucidate the modes of interaction of AβOs with other biomolecules and related toxic mechanisms [32],[33],[35]–[37],[130]. Structural characterization of specific AβOs is challenging due to their transient nature and heterogeneity. AβOs can exhibit conformational plasticity that can be heavily influenced by environmental factors, further complicating such analysis. Despite these inherent challenges, efforts have been made to separate, isolate, and characterize distinct AβO species obtained from synthetic Aβ or from AD brain tissue and cell cultures [18],[32],[35]–[37],[114],[129],[131]–[136]. With regard to HMW and LMW AβOs, they are separable in vitro by size exclusion chromatography [137] or ultrafiltration with a 50 kDa molecular weight cutoff [21],[54],[118]. Quick characterization of AβOs can be accomplished by chromatographic techniques, SDS PAGE, and by the use of a variety of oligomer specific antibodies (Table 4).
One strategy that has been used to overcome obstacles associated with characterizing AβOs has been to stabilize oligomers via cross-linking. Such methods can lead to not only structural but also functional characterization of AβOs. Photo-induced crosslinking (PICUP) was initially used to stabilize and characterize LMW AβOs of Aβ40 and Aβ42 [33],[141]–[143].While Aβ40 primarily formed an equilibrium of dimers, trimers, and tetramers, Aβ42 agammaegated into pentamers/hexamers that further assembled into protofibrils [143]. Using mutated Aβ42 (F10, Y42), AβOs ranging from dimer to dodecamers were stabilized using PICUP [141], opening up the ability to perform structure/activity analysis on AβOs up to 50 kDa in size. AβOs can also be stabilized using dityrosine crosslinking, which occurs under elevated copper concentrations and oxidative stress [144]. Both of these conditions have been linked to AD, suggesting that the crosslinking associated with this method may be more physiologically relevant [145],[146]. In fact, dityrosine crosslinked proteins are observed in amyloid plaques and CSF from AD patients [145]. Dityrosine linkages are associated with copper-mediated stabilization of AβOs [147]. Copper stabilization is effective enough to allow for 3D structural characterization of AβOs by small-angle x-ray scattering [148], and the copper to Aβ ratio could push agammaegation toward ellipsoidal oligomers of 38 peptides (excess copper) or fibrils (excess Aβ) [119].
Another method to overcome the metastability of Aβ species and control the agammaegation process is to complex/fuse Aβ sequences within other protein. Aβ sequences are often based on previously identified regions of the peptide that have been identified as playing a role in Aβ agammaegation (Figure 1). Such a strategy has been successful in studying monomeric structure of Aβ sequences [149]. With careful design, this method has been applied to stabilizing oligomers derived from Aβ fragments for structural characterization. Fusion of Aβ18–41 with the CDR3 loop region of a shark Ig new antigen receptor single variable domain antibody resulted in the formation and stabilization of tightly associated Aβ dimers, which could pair to form tetramers [150]. This dimer had a compact structure rather than a β-turn/β-sheet structure. Several engineered peptide macrocycles that incorporate Aβ sequences (Aβ15–23 and Aβ17–36) have been designed to contain agammaegation to the oligomeric state, and these systems have been structurally characterized by X-ray crystallography and NMR spectroscopy [151]–[153]. Collectively, this macrocycle strategy has revealed numerous dimers, trimers, tetramers, and higher order oligomer species that display a variety of β-sheet based structural heterogeneity.
Due to the complex nature of Aβ agammaegation, techniques that allow for distinguishing and characterizing distinct morphological features of AβOs within heterogeneous agammaegation reactions are of enormous benefit. Both atomic force microscopy (AFM) and electron microscopy (EM) provide this capability. In particular, AFM has emerged as a particularly useful technique in studying AβO formation and morphology [95],[101],[134],[135],[154]–[160]. As AFM can be operated in solution, it has the ability to observe and track the behavior of individual AβOs on surfaces under physiological buffer conditions [158],[160]. The surfaces used in AFM experiments have become progressively more biologically relevant and include lipid membranes [135],[161]–[164]. With regard to Aβ agammaegation on lipid membranes, in solution AFM studies have demonstrated the formation of distinct oligomeric agammaegates associated with point mutation in Aβ [165], the formation of pore-like AβO morphologies [154]–[156], that preparation history influences AβO formation on bilayers [95], and that mechanical changes occur in bilayers associated with the presence of AβOs [166]. AFM based force spectroscopy has even been used to understand the energetics of AβO formation and stability [167],[168].
With the recent development of high-speed AFM in solution, insights into the dynamics and fate of individual AβOs has been achieved [128],[169]. Using high-speed AFM to track the dynamics of PICUP-stabilized LMW AβOs demonstrated that these AβOs were highly dynamic in structure, fluctuating between single and multi-globular assemblies [169]. Direct visualization of Aβ agammaegation with high-speed AFM imaging demonstrates that LMW AβOs much more quickly transition to form fibrils with distinct morphologies compared with HMW AβOs [128]. Despite being classified as being off-pathway, HMW AβOs can still contribute to fibril formation by serving as a reservoir of Aβ. That is, HMW AβOs may dissociate into smaller LMW AβO that seed fibrillization [128]. This again points to the necessity to track the fate of AβO species when evaluating their physiological activities. For example, the LMW AβOs that are dissociation products of HMW AβOs may actually be more toxic [170].
Another technique that is capable of characterizing heterogeneous populations of AβOs is ion mobility mass spectrometry (IM-MS). These IM-MS studies ascertained qualitative differences in AβO structure associated with Aβ40 and Aβ42 [131],[132]. Aβ40 tetramers displayed an enclosed ring-shaped configuration that would inhibit additional contacts required to assemble into larger AβO species [131],[132]. Aβ42 tetramers preferentially had a bent structure that would provide oligomer ends capable of additional contacts and enabling further agammaegation. Indeed, Aβ42 was capable of forming larger donut-shaped dodecamers.
Another strategy to perform structural analysis of AβOs is utilizing specific conditions to stabilize them via a kinetic trap. Such an approach has been successfully used to enable NMR spectroscopy of AβOs [171],[172]. By incubation of Aβ at 4 °C and freeze-trapping with liquid nitrogen, heterogeneous, spherical AβOs were analyzed with 2D NMR and shown to possess in-register parallel β-strand structure similar to fibrils [172]. Based on NMR analysis, Aβ42 pentamers stabilized at a low 4 °C and 10 mM salt concentration were disordered [171]. These Aβ42 pentamers displayed enhanced toxicity compared with protofibrils or fibrils [171]. An atomic model of Aβ42 oligomers consisting of approximately 15–24 peptides has been proposed from a combination of biophysical techniques [173]. These oligomers were prepared by disagammaegation in HFIP followed by resuspension in dilute ammonium hydroxide, preventing fibril formation. These were not end-stage AβOs, as subsequent dilution in PBS resulted in fibril formation. Powder X-ray diffraction patterns of the AβOs were consistent with helical β-sheet pairs wrapped together into a super-helix. This wrapping results in a hole along the super-helix axis, which is consistent with proposed toxic mechanisms in which Aβ forms pathogenic pores. Another proposed structure, based on site-directed spin labeling and electron paramagnetic resonance, shares similarities with this structure [174]. This study used Aβ42 fused to GroES-ubiquitin that formed stable oligomers that were A11 positive. Based on the EPR data, an AβO model was proposed that consisted of a β-sheet with three antiparallel strands with these strands being arranged head to tail. These sheets are further packed face to back as a group of four.
Even just tracking AβO formation has been challenging. A number of straight forward spectroscopic assays are well-established to track formation and kinetic parameters of fibrils (e.g., ThT), but methods to easily track AβO formation have been lacking. Recently, the use of 4,4-difluoro-4-bora-3a,4a-diaza-s-indacene (BODIPY) dyes have been utilized to fluorescently detect the AβOs in vitro [175],[176]. These BODIPY fluorescent probes have high quantum yields with the capability of selectively binding to AβOs [177]. Importantly, increases and decreases in the “BD-Oligo” dye fluorescence also correlated with increasing and decreasing intensities of A11 staining, directly relating this signal to an established methods to detect AβOs [175]. In addition, the BD-Oligo dye can be used in parallel with ThT assays that detect fibril formation, allowing for the direct investigation of the correlation between oligomer and fibril formation [175]. A BODIPY-based probe (BAP-1) has also facilitated the direct visualization of Aβ plaques in transgenic mice [177], and rational modifications of BAP-1 also allowed for near-infrared selective detection of tau neurofibrillary tangles [178]. The ability to rationally modify BODIPY dyes for fluorescent detection of specific agammaegate species, the tunability of their spectroscopic properties, and their insensitivity to solvent and pH changes have made BODIPY dyes a recently promising avenue for high throughput studies of AβO formation and stability.
6.
Conclusion
While the agammaegation of Aβ has been extensively studied, there is still much to understand at the molecular level about AβO formation, structure, and activity. Due to the transient nature of AβOs, their morphological heterogeneity, and the continuing debate concerning specific toxic agammaegate species associated with AD, structural details and physiological activities of the variety of AβOs still need to be fully elucidated. The exact mechanisms associated of how AβOs lead to cellular dysfunction and death have not fully been explained. Understanding these phenomenon may prove crucial in the effectiveness of therapeutic strategies based on manipulating Aβ production, clearance, and agammaegation. Here, we highlighted some specific features of AβOs and techniques that have provided insight into their structure and formation. While this review is far from exhaustive, we hope that collectively they provide a compelling argument toward the importance of understanding the nature of AβOs, highlight some of the intrinsic obstacles associated with studying AβOs, and provide some insight into methods that will play a role in pushing our knowledge of AβOs further.









 DownLoad:
DownLoad: