1.
Introduction
Due to the new improvements in medical services and longer life expectancy, there is a growing need for rehabilitative and assistive devices for at-home nursing due to the rise in clients with gait impairments [1]. Lower-limb exoskeletons are now possible as a home-care tool for both rehabilitation and assistive purposes, thanks to rapidly expanding methods of monitoring and combining multi-modal biomedical data. Improved assistive and rehabilitative performance can be obtained by combining multi-modal biomedical signals that were used to decode human motor intent, i.e., to recognize subject-specific gait features.
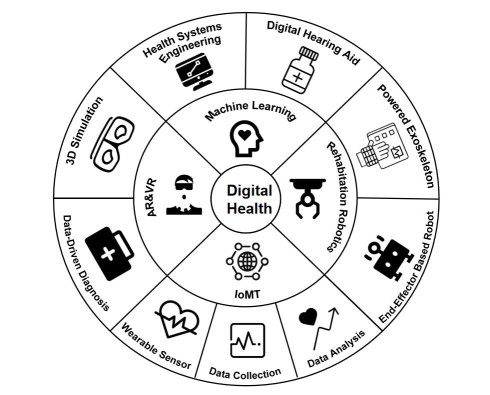
In order to enhance the effectiveness of service delivery and make medication more individualized and specific, the field of digital health integrates digital clinical services, innovations, and lifestyle with illness, care, and social culture [2]. It makes use of technology-based information and communication to enable a more individualized and accurate assessment of the health issues and difficulties experienced by patients undergoing proper help and social prescription [3]. The application areas of digital health management are shown in Figure 1. There are various ways in which the concepts of digital health and its scope intersect with those of medical and medical informatics. Global adoption of electronic health files has grown since 1990, and this development is closely related to the accessibility of universal health-care coverage [4]. The interdisciplinary area of digital health involves a wide range of participants, including physicians and scientists with a variety of specialties in healthcare, architecture, social science, global health, economics, and database administration. Telemedicine, wearable technology, AR, along with VR are just a few examples of the software applications and products that make up digital health technologies. In general, digital health links disparate medical activities to increase the use of technology, intelligent objects, analytical methods, and messaging applications to help physicians and clients control diseases and health risks and also advance wellness. By digital health management, we refer to the approach that gives users the power to manage their own health while utilizing technology to connect them to their care teams. This is about fusing human ties with digital experiences, not about replacing human interactions with them. There are basically three steps for digital health management, which include data monitoring, data processing, as well as rehabilitation intervention. Digital health technologies play a key role in each part via the use of multi-modal signal monitoring [5].
For lower-limb data monitoring, IoT plays a major role. Specifically, technologies related to IoT, such as wearable sensors, machine learning, AI, IoMT, 5G wireless communication, and telemedicine devices, can all be employed in gathering biological signals.
For lower-limb data analysis, digital technologies exert a great influence. All studies demonstrated that a digital health based classification approach is an effective data analysis approach for this task.
For lower-limb rehabilitation, there is much that digital health technologies can do. Rehabilitation robots, which help patients move their bodies, have emerged as one of the most popular trends in the field of digital health.
This manuscript consists of several parts. The first section covers a general overview of digital health management, wherein the history of development, the application area, as well as its sub-fields are fully discussed. The second section is a brief introduction of the digital health management, and the third part covers the major biological signal monitoring technologies employed in digital health management. The fourth part is a list of the recent advancement of the application of digital health technology in lower-limb symptoms diagnosis, analysis, and rehabilitation. Part five is a demonstration of related works. Part six discusses the uses of digital health and its future prospect, and part seven concludes the article by summarizing the findings and applications in the field of digital health management.
2.
Digital health management
Digital health management, which lays its groundwork on digital health technologies, covers a lot of areas of medical research and application and will be introduced in the following paragraphs in terms of telemedicine, wearable technology, AR and VR, as well as rehabilitation robotics.
2.1. Telemedicine
One of the most inclusive subfields of e-health is telemedicine [6]. It includes remote healthcare, scheduling appointments, self-symptom checking, reporting patient outcomes, and the digitalization of medical data, among many other things [7]. Quick, non-urgent consultations are constantly provided through digital and remote clinics, saving an enormous amount of time. This approach, which has take place of in-person consultations as the primary way physicians visit their clients, has grown increasingly popular, particularly in light of the COVID-19 epidemic [8]. Physicians want to utilize this form of digital therapy for routine checkups even after the epidemic is over, since it is a trustworthy procedure that keeps all parties secure. The swivel motion reconstruction approach was employed in conjunction with the kinematic mapping in robot redundancy to mimic human-like behavior [9]. Online health records are a part of telemedicine as well, giving doctors and clients constant access to the necessary data. With so much digital data available, healthcare professionals may access patient information and use it to evaluate patient data to develop more effective and intelligent treatment regimens. This paves the way for a more personalized healthcare system, which may help patients understand their ailments and yield better results.
2.2. Wearable technology
Smart watches and on-body sensors are two examples of wearable innovation. Smart watches were one of the first wearable gadgets that encourage self-monitoring and were commonly connected with fitness tracking [11]. Many track health-related information, such as "body mass index, calories burned, heart rate, and physical activity patterns." Aside from smart watches, scientists are working on intelligent bodywear, such as patches, garments, and accessories, to provide "on-demand medication delivery." [12]. Figure 2 provides a perfect example. This innovation has the potential to be broadened into smart implantation for both acute and non-severe medical problems, allowing clinicians to develop better, more dynamic treatment procedures that would not have been conceivable without such digital innovation.
These innovations are employed to capture information about patients all day long. The statistics can result in more effective treatment planning and monitoring because practitioners no longer have to ask their patients to visit their offices to obtain the essential data. Therapists will have a better understanding of how well a specific drug is working. They are also capable of continuing to learn from this data and building on their initial treatment regimens, allowing them to act when necessary.
2.3. AR and VR
AR technology is utilized to create smart gadgets for healthcare practitioners and enriches real-world encounters with digital sensory data [13]. Because hand-held devices now collect the majority of patient-related data, wearable technology provides a novel, hands-free enhanced method for a doctor to evaluate their patient's health history. Wearing a pair of smart glasses while treating a person enables the use of this technology for data-driven diagnostics, increased patient record, and even improved care plans.
VR, which provides interactive simulations that imitate actual settings and may be customized for specific therapies, is another area of related technology [14]. Since the lower limb is usually the focus of therapy, many stroke victims experience loss of range of motion, and according to established treatment procedures, 55 to 75% of patients experience chronic upper muscle impairment. The two key indicators of recovery progress are repeated behaviors and the period of treatment. In order to assist patients retrain their motor motions, virtual reality technology may provide a variety of 3D situations that are difficult to replicate in the real world. These scenarios can not only target particular bodily regions, but they can also grow more intense as the patient gets better and needs to perform more difficult duties.
2.4. Rehabilitation robotics
Studies in the subject of rehabilitation robotics are focused on learning about and improving rehabilitation through the use of robots [15,16,17]. Robotics for rehabilitation comprises the creation of tools designed to support various sensorimotor processes [18] (e.g., arm, hand, [19,20] leg, ankle [21]), development of different schemes of assisting therapeutic training [22], and assessment of sensorimotor performance of patient [23]; here, robots are used mainly as therapy aids instead of assistive devices [24], as is shown in Figure 3. Robotic treatment has been demonstrated to be a successful supplement to therapy in people with motor deficits, especially those caused by stroke, and is often well tolerated by patients.
Although the number of rehabilitation robots has grown over time, their availability is still limited due to clinical trials. Numerous clinics do experiments but choose not to deploy the bots since they prefer remote control. There are several advantages to involving bots in a participant's rehabilitation. The ability to repeat the procedure or workout as often as desired is one of its benefits. You can obtain precise measures of their advancement or deterioration, which is another advantageous element. Figure 4 provides a perfect example. Through the use of the device's sensors, you could obtain precise measurements. You must be cautious whereas the gadget is performing the measurement since the varied motions the person makes to exit the room after it is finished might cause the instrument to malfunction [27]. The therapeutic bot can provide continuous care for longer. The rehabilitation robots are unable to comprehend the patient's demands during the healing process like an expert clinician would [28]. Although the robots are currently unable to comprehend, they will be capable of doing so in the long term. Some other benefit of using a robot for recovery is that the practitioner doesn't have to exert any extra exertion, as is shown in Figure 5.
The use of rehabilitative robots in learning for medical, operations, telemedicine, and other fields has recently increased; however, there have been numerous concerns about the robots' inability to be operated by a computer. Contrary to popular belief, employing an animatronic robot for rehabilitation does not equate to utilizing one for manufacturing. Robots used in rehabilitation must be configurable and customizable since they may be applied in a variety of ways. An industrial robot, on the other hand, is always the same; until the item it is handling gets larger or smaller, the robot doesn't need to be changed. An industrial robot would need to be more adaptable to its new mission in order to function.
3.
Multi-modal signal processing
Multi-modal signal processing is a crucial area of study and innovation that mixes and analyzes data from audio, sight, speech, and messages in order to better comprehend, model, and conduct HCI systems and devices that benefit human interaction [30,31,32]. A broad range of uses that aid in improving group relations and consequently efficacy before or during surgical treatments are made possible by multi-modal signal processing [33].
More effective monitoring gadgets have been made due to the technology advancements. These devices can typically record a few physiological signals simultaneously, including the electrocardiogram (ECG) [34], blood pressure (BP), arterial blood pressure (ABP), electroglottography (EGG), electroencephalogram (EEG), electrooculogram (EOG), electromyogram (EMG), mechanomyogram (MMG), magnetoencephalogram (MEG), respiration (RESP), and photoplethysmogram (PPG). The IoT has progressively merged with both the practitioner and patient sides of the healthcare industry. There are three main instruments used in signal analysis: the IoMT, machine learning, and medical sensors, which will be introduced in the following text. Moreover, Figure 6 provides an example of its application.
3.1. IoMT-based remote health monitoring
The health sector is not an exemption to the growth of IoT devices across domains. The Internet of Things (IoT) currently has a significant influence on the health-care industry [36]. The Internet of Medical Things(IoMT), which was created by the creation of intelligent sensors, smart gadgets, and sophisticated light communication protocols, enables healthcare equipment to be linked in order to track biological signals and assess client ailments without the use of humans (IoMT) [37]. The ability to perform routine duties while clients have been continuously monitored for their well-being and the benefits of reduced hospital costs are the key advantages of IoMT-based remote monitoring. Given the bulk of the body-mounted units and the need for regular battery charging or backup, traditional distant monitoring devices are uncomfortable for the clients.
The IoMT breakthrough addresses the aforementioned problems by creating small, extremely low-power sensor devices and streamlined messaging, which can be seen in Figure 7. The portable patient monitoring unit (PPMU) at the client's home or in an acute ambulance, along with real-time monitoring and a decision-making system at the doctor's office, make up the bulk of the remote health surveillance system. The portable telemonitoring device is primarily made of electronic sensors and circuits that can collect signs like heart rate, heart rate variability, pulse rate, respiration rate, systolic blood pressure, diastolic blood pressure, oxygen saturation, body temperature, body mass index, level of consciousness, muscular activation, total lung volume, height, blood sugar level, and a urine report. The data is then processed by a processing unit, and a report is generated.
The synergistic expansion of machine learning (ML) and artificial intelligence (AI) is increasing the usefulness of medical IoT. Information analytics and machine learning process vast volumes of continually streaming data from sensor-assisted medical devices, delivering actionable results more quickly and assisting the therapeutic process. Using streaming data, preventive treatment could drastically reduce hospital stays and emergency care costs. This would increase productivity while also improving treatment experiences and happiness. However, certain information security hazards both in movement and at rest need to be carefully considered. Furthermore, the possibility of false positive results might stress out individuals and the healthcare system excessively. The three crucial components of IoMT that should always take precedence are precision, repetition, and dependability.
3.2. Wearable sensors in medical application
Flexible materials, the building blocks of biosensors, have advanced significantly over the past ten years to give electronic gadgets skin-like qualities such as slimness, stretchability, bio - compatibility, biodegradability, and self-healing capacity [38], as is shown in Figure 8. Furthermore, a variety of tissue engineering applications for sensing devices have entered the digital health space [39,40,41,42,43,44], making it possible to monitor vital signs (blood pressure [45], respiration rate [46], skin temperature, pulse, etc [47].), physiological signals (electrocardiography (ECG), electromyography (EMG), electroencephalography (EEG), etc.) [48,49], body kinetics(strain, pressure, etc.) [50,51], and dynamic biomolecular state via accessible biofluids (sweat, etc.) [52,53]. Biosensors go beyond only monitoring biomedical signals to provide remarkable reinforcement or assistance for body movements [54,55,56]. The robotic exoskeleton, a bot that directly assists or strengthens human muscular motions, has emerged as a viable platform in a wide variety of uses, including prostheses, physiotherapy, rehabilitation, and human capacity enhancement. [57,58,59].
3.3. Machine learning
The research of pc systems which can identify complicated connections that exist from empirical evidence and reach reliable conclusions is known as machine learning [61]. The computer is "taught" by utilizing vast amounts of information and formulas that give it the capability to comprehend what to do to complete the work, as opposed to being given particular sets of commands to carry out a task. In contrast to conventional algorithms, learning takes place without explicit instructions since the data "tells" the computer what the "correct response" is. ML issues may be divided into supervised learning and unsupervised learning categories [62]. In supervised ML algorithms like face identification, the machine is given several examples of "faces" or "non-faces, " and the algorithm learns to predict whether an unknown image is a face or not [63]. ML and active shape modeling have been used for understanding biomechanics and orthopaedic implant design in musculoskeletal medicine [64], bone tumour resection [65], prediction of progression of osteoarthritis based on anatomical shape assessment [66], and robotic surgery [67]. In clients with spinal impairment, extensive physiological data analysis using machine learning has been applied [68].
4.
Recent advancements in digital health management using multi-modal signal monitoring
4.1. Lower-limb health data collection through digital health management
IoT is crucial for lower-limb data monitoring. In particular, IoT-related technologies like wearable sensors, AI, IoMT, 5G wireless connectivity, and telemedicine equipment may all be used to collect biological signals.
To improve the reliability and precision of gait detection utilizing sEMG signals from the lower limbs, Ting Yao et al. suggested an approach based on deep neural networks using sEMG data in [69]. Electroencephalography (EEG) and surface electromyography (sEMG) data were captured by Xiebing Chen et al. in [70] from volunteers who were instructed to stroll on flat ground and up stairs. In order to simultaneously simulate EEG and sEMG signals, this work introduces a unique technique based on vine copula. To integrate various information and build intra-lead as well as inter-lead connections in multi-lead data, MingHao Zhong et al. in [71] modelled the multi-lead data as a heterogeneous graph, offering a suitable and efficient data model. The application domains, application processes, typical signals, common approaches, and outcomes of smart wearable devices for the identification of mental health issues were thoroughly reviewed by Nannan Long et al. in [72]. A lightweight DC-DSCNN model for gait identification for wearable technology was suggested by Xiaoguang Liu et al. in [73].
In order to forecast the growth of major lower limb amputations in the United Kingdom, Meffen et al. Additionally, sources of regularly gathered electronic health-care data that detail the epidemiology of significant lower limb amputations will be identified in [74]. In [75], the goal of Hyun Kyung Kim and Li-Shan Chou was to investigate the contributions of the lower-limb muscles to the velocity of the total body center of mass while walking in obese people. A biomechanical model was used to evaluate the simulation of walking weight delivery for five overweight and five non-overweight people. In [76], wearable Inertial Measurement Units (IMUs) were utilized to derive objective gait characteristics, according to research by Anwary et al. The growth of digital care is aided by this method, which makes it possible to measure gait at residence without the need for or price of a complex laboratory setup. In [77], Jean Won Kwak and colleagues created a pressure gauge with a smooth, 3D layout that merges into a tiny, adaptable, battery-free, wireless system with an incorporated thermometer to enable non-invasive, undetectable operation just at skin-prosthesis interface. The robotic exoskeleton in Figure 9 can be used to achieve that goal.
In [10], Luís Moreira et al. give a comprehensive database that contains the aforementioned raw and analyzed information for 16 healthy volunteers walking on a ten meter-flat ground at seven regulated speeds. This paper describes the research setup in depth and provides a quick confirmation of information quality. Cristina Floriana Pană et al. in [79] offered a novel approach for an intelligent ankle prosthesis grounded on intelligent fluids that would mimic the functioning of the normal ankle during walking and jogging movements. The investigation in this sector focuses on enhancing the properties of the mechanical ankle in order to accurately imitate the function of the human ankle. From 2012 to 2019, Yergali Nabiyev et al. in [80] examined the prevalence of lower-limb wounds and related risks in Kazakhstan. They discovered a link between age and the probability of lower extremity injuries. Lower extremity injuries are on the rise in both sexes over the age of 85. Analyzing the cohort impacts revealed the risk's proclivity for both sexes. In [81], Hudson Kaleb Dy and Chelsea Yeh highlighted the use of ML and IoT technology to measure the lower-limb strength of people undergoing recovery or therapy. They wanted to evaluate and analyze people's development by attaching sensors to chairs and analyzing the data with the Google GPU Tensorflow CoLab.
In [82], Kai Zhao et al. developed a fatigue state graded system on the basis of surface EMG signals of human lower-limb muscles and analyzed the fatigue condition of clients' lower limbs by capturing surface EMG signals of aim muscles of human lower limbs, in in order to guarantee that sick people can not only carry out training sessions but also do not cause additional injuries due to overtraining. In [83], Thomas M. Doering et al. studied the changes in individual muscle protein abundance and related gene sets in otherwise healthy lads following 3 and 14 days of unilateral lower-limb immobilization. It is the inaugural research to demonstrate that unilateral lower limb immobility causes mitochondrial malfunction, excitotoxicity, and proteolysis using data independent proteomics and GSEA. In [84], Sean Sadler et al. gathered published reports or studies that investigated views, meanings, or attitudes about the feet and lower-limb health. Multiple complicated linked constituents were discovered to impact Aboriginal and Torres Strait Islander Peoples' views of foot and lower-limb health. In [85], Takuro Ikeda et al. set out to explore the impact of short-term lower-limb immobilization on postural sway in the vertical position after cast release. 22 healthy young individuals were enlisted, and each user's lower leg on one leg was secured for 10 hours with a soft bandage as well as a medical splint constructed of metal and soft urethane. The findings imply that short-term disuse may produce acute alterations in COP motions during silent standing.
LR Souto et al. in [86] conducted a study to determine the immediate effects of the hip strap and foot orthoses during level-ground walking and the single-leg squat test on self-reported outcomes. The secondary aim is to investigate whether the hip strap and foot orthoses result in the kinematic changes that these devices are purported to cause. The Surface EMG technique was used to investigate the activities of the vastus lateralis (VL), rectus femoris (RF), vastus medialis (VM), and the medial head of the gastrocnemius (MHGM) with the increase of age. [87] In a study on physically fit females, Farhah Nadhirah AimanSahabuddin et al. in [88] examined the effects of four weeks of hip- and ankle-focused workouts on lower limb mechanics during single-leg squats (SLS). 36 healthy and active ladies with high DKV were divided into categories for hip, ankle, as well as control. The intervention groups participated in activities that targeted either the hip or ankle muscles over the course of twelve sessions spread over four weeks. After athletes were given the all-clear to return to competition, Argyro Kotsifaki et al. in [89] assessed their lower-limb status throughout the propulsive and landing phases of a SLHD task. It was discovered that symmetry in SLHD standardized tests does not guarantee symmetry in lower-limb biomechanics.
In [90], the current human body stance surveillance model was refined by Yinman Zhang and Lulu Wang, who also created a human body model with four degrees of freedom for the lower limbs. The configuration of the monitoring nodes and micro-sensor assembling technique for getting the model description parameters were chosen as a result. The processing of sensor information and the assessment of correctional effects use techniques like collaborative filtering as well as support vector machines. In [91], a smartphone-based method for capturing and recognizing lower limb movements in humans was suggested by Lin-Tao Duan et al. We create a movement logger that uses two motion sensors to record five different types of limb activity. The 10-fold cross-validation method is used to train and validate these classifiers with 670 lower-limb motion examples. The results of the experiments demonstrate that our low-cost method can accurately identify human lower-limb actions.
To help patients precisely adjust their left and right legs, Fangyan Dong et al. in [92] devised a categorization system based on EEG signals of motor imagery. This study offers a solid theoretical foundation for the development and use of brain-computer interfaces in instruction for recovery. In [93], in order to protect passengers' overall fitness while they are sleeping while seated during flights, Huizhong Zhang et al. investigate the lower-limb edema and tissue compression. Twenty volunteers took part in a field study of lower limb edema and tissue compression during sitting sleep using a Boeing 737 airplane seat as the model. According to the study, travelers can obtain dynamic comfort by making certain seated position adjustments. A reward-modulated multitasking learning strategy and a recurrent neural network that resembles the motor cortex are suggested in the study by Jiahao Chen et al. in [94]. Also, a neurological control on the basis of muscle synergies is proposed by Jiahao Chen in [95]. This article also advocates for the advancement of musculoskeletal robots and the blending of robotics and neurology.
4.2. Statistical analysis of lower-limb data through digital health technology
Digital technologies have a significant impact on the analysis of data for the lower limb. All investigations showed that a classification strategy based on digital health is a useful data analysis strategy for this assignment. Figure 10 provides us with a perfect example.
An overview of the following topics is given section by section by Ankit Vijayvargiya et al. in [97]: 1) Methods for removing artifacts from lower limb sEMG signals. 2) A review of lower limb sEMG datasets already in existence. 3) A succinct explanation of the different methods for categorizing and processing sEMG data for a range of applications involving lower limb activity. By sit-stand-sit motions, Md. Moznuzzaman et al. in [87] evaluated the effects of aging on the lower-limb muscles connected to OA knees. Fifty-one healthy subjects and 33 OA sufferers were included in the study's total of 84 volunteers. The vastus lateralis, rectus femoris, vastus medialis, and medial head of the gastrocnemius were examined for changes in activity with aging using the surface EMG technique. A three-dimensional gait analysis is shown in Figure 11.
A methodology for forecasting an exoskeleton's contralateral lower-limb joint angles was put forth by Can Wang et al. in [99]. The model supports exoskeleton-assisted hemiplegia rehabilitation by using data from several signal - based sensors. For individuals with hemiplegia, a monitoring system based on bioelectric and acoustic data is being developed to enhance joint angle prediction in the afflicted leg. In [96], Bingzhu Wang et al. used a variety of feature analyses and their combination to enhance the performance of sEMG-based lower-limb movement categorization. In the clinical study, nine subjects executed four distinct moves. The trained movement decoders collected the sEMG by extracting features and recognizing patterns. A study on the assessment of adolescent lower-limb posture correction using collaborative filtering and microsensors is conducted in the paper by Yinman Zhang and Lulu Wang in [90]. This paper simplifies the current human body stance surveillance model and creates a human body model with six degrees of freedom for the lower limbs in an effort to address the issue that the human body posture monitoring system requires several nodes.
Using electrical stimulation at frequencies of 20, 35 and 50 Hz, Paulo Broniera Jnior et al. in [100] examined the impact of reducing the number of EEG channels on the outcomes of lower limbs' motor imagery classification. In [101], Ying Zhang suggests a hardware approach for the steadiness feature of lower-limb exercise using the theory of smart wearable sensors in response to the need for real-time monitoring. In terms of software design, his study splits the software system into two components: the lower computer and the upper computer. The Virtual Peg Insertion Test and a previously developed core set of 10 digital health measures were utilized by Christoph M. Kanzler et al. in [102] to describe upper body motion and grasp force variations during a pick-and-place activity. With ARSACS completing three repeated assessment sessions on twenty-three participants, they assessed reliability, measurement error, and learning outcomes. Figure 12 shows the Noraxon's myoMotion and myoMuscle sensor locations.
In [104], Sali Issa and Abdel Rohman Khaled demonstrated an improved extraction characteristic for surface EMG signals used in lower limb movement identification applications. In order to evaluate the system, participants are separated into abnormal and normal groups based on the normalcy of their knees. The goal of Alexander Meigal et al. in [105] was to investigate the value of nonlinear surface electromyogram (sEMG) characteristics in characterizing neuromuscular activity in relation to space flight (SF) time and stepping mode. For describing the neuromuscular activity of skeletal muscles in SF circumstances, nonlinear sEMG parameters appear promising. In [106], a ML classification approach was used by Payam Zandiyeh et al. to explore if changes in muscle function patterns between ACLR patients and healthy controls could be identified ten to fifteen years after surgery. In [107], a transfer entropy assessment while walking was used in the article by Tonghun Hwang et al. to determine the causation between head vertical motion and lower-limb joint movements. The gait patterns of all 12 subjects were examined. This discovery could pave the way for easy secondary assessment of lower-limb joint issues using just head-worn sensors.
To accurately recognize lower limb motions, Chunfeng Wei et al. in [108] suggested a precise method of feature extraction for single-channel sEMG signals. By variational mode decomposition (VMD), the single-channel sEMG signal was divided into several variational modal functions (VMFs), and entropy characteristics were recovered from the VMFs to highlight the meaningful data of the sEMG signal. An experimental technique for motor imaging was developed in the study by Fangyan Dong et al. in [92]. They relied on multi-joint path planning for movement of the left and right legs, aiming at the volatile properties of EEG data. This study offers a solid theoretical foundation for the development and use of brain-computer interfaces in recovery instruction. In [109], an intelligent lower-limb prosthesis hierarchy planner based on sensor fusion and a central pattern generator was proposed by Yansong Wang and colleagues. Signals from the inertial measurement unit (IMU) and electromyographic (EMG) were captured and merged at the feature and decision levels. The gait phase dependent cascade classifiers make up the senior-level planner.
4.3. lower-limb rehabilitation through digital health management
Digital health technology provide a lot of potential for lower-limb rehabilitation innovation. One of the biggest innovations in the world of digital health is rehabilitation robots, which help patients move their bodies.
A set of wearable orthoses for at-home physiotherapy were designed, developed, and evaluated, according to a presentation by Kevin Hung et al. in [110]. It can provide a low-to-medium range of resistive torques suited for isotonic, isometric, and open-chain resistance exercises because it originally used tiny electromagnetic brakes. Using the kinematic data from a Wearable Sensor System, Javier Conte Alcaraz et al. in [111] presented Deep Convolutional Neural Network for tracking the advancement of the rehabilitation. The WSS offers 3D linear acceleration and rotational velocity during strolling at any pace on flat ground from numerous body areas, including the lower back and lower limbs. In [112], Rongguo Yan et al. dealt with a three-axis accelerometer-based physical exercise monitoring system for the sufferers. The system creates a data collecting platform out of a three-axis accelerometer, a microcontroller, and a wireless Bluetooth module in order to collect accelerations of the lower-limb movement. The wireless Bluetooth module then transmits the data to a smart phone.
A rehabilitative method based on a lower-limb exoskeleton combined with a human-machine interface was presented by Susanna Yu. Gordleeva et al (HMI) in [113]. The project produced algorithms for the gathering, processing, and classification of multimodal HMI data. The system is capable of real-time, simultaneous analysis of up to fifteen signals throughout a motion. Automated foot strikes from a 6MWT have been used successfully by Pascale Juneau et al. in [114] to determine step-based characteristics for injury risk categorization in lower-limb amputees. A smartphone app might use automated foot strike detection and fall risk level to offer clinical assessment right away after a 6MWT. EEG signals and user feedback have been shown by Daniela Camargo-Vargas et al. in [115] to have advantages in terms of cost, efficacy, adequate education, and user engagement. As a result, there is a need to keep creating user-friendly interfaces that incorporate feedback approaches. In order to control and activate a new Dynamic Ankle-Foot Orthosis designed to rehabilitate the dorsiflexion and plantarflexion movements of the ankle, Mohd Nor Azmi Ab Patar et al. developed a straightforward yet effective technique in [116]. Figure 13 shows the process of rehabilitation by a robotic device.
To provide a low cost and effective method of monitoring the patient 's health with prosthetic lower limbs, Neha Mathur et al. proposed a whole mobile sensor system based on easily available consumer items in [117]. The state-of-the-art of lower-limb exoskeletons, which are mostly utilized for physical movement support and rehabilitation, was evaluated and described by Weiguang Huo in [118]. A description of the most popular actuation systems was also given. A Long-term Recurrent Convolution Network based on transfer-learning, called "MyoNet", was developed by Arvind Gautam et al. for the categorization of lower limb motions and the forecasting of the related knee joint angle in [119]. In the study by Jie Li et al. in [120], an efficient motion measuring technique premised on an inertial sensor network is suggested to assess children's motor skills with the goal of validating the efficacy of therapy for children with cerebral palsy.
In [121], the unique rehabilitation robot Hunova's development process and technical foundation were detailed by Jody A. Saglia et al. The report summarizes the clinical investigations conducted to validate the innovation and explains in full the software and hardware design of the system. Quan Zhang et al. used a lower-limb rehabilitation robot in [122] to prove the sensory system's performance in user recognition, movement tracking, and training with robots and video games, demonstrating its potential for Internet of Things-based intelligent application areas. In their literature analysis, Trinnachoke Eiammanussakul and Viboon Sangveraphunsiri examined the training activities carried out by rehabilitation robots in [123]. The control system of the lower-limb rehabilitation robot in sitting posture, which was described in previous work, is covered in detail to illustrate the robot's behavior while instructing a participant.
In [124], a robot that blends on-site and telerehabilitation was created by Mingda Miao et al. The goal is to make the patient's walking easier. They incorporate a gantry mechanism, body-weight support network, data review system, and a man-machine interaction process control into the design of the electromechanical system. In [125], a preliminary investigation is undertaken in the paper proposed by Nurhazimah Nazmi et al. to enhance the health of post-stroke victims in physiological functions, particularly on the lower-limb rehabilitation, with a minimum amount of therapist monitoring. To control the movement of a mobile LLAO, DusthonLlorente-Vidrio in [126] proposed an event-driven automated controller. The LLAO is activated using data gathered from electromyographic signals, which are recorded from the patient's triceps and biceps muscles. Margarida Florindo et al. in [127] demonstrated the therapeutic significance of basic dynamic activities like gait and confirmed that perfusion is age dependent. This deep-level loss in dorsal foot perfusion—which becomes more significant with movement intensity—suggests a broad range of applications, including early diagnosis and rehabilitation.
5.
Related work
Recent years have seen rapid development of digital health, and this section will go over the recently published reviews or surveys on the topic of digital health management through multi-modal signal monitoring as well as its related concepts. Obtaining high performance in common robots is one of the most difficult challenges in robotics research. It is typically expensive and challenging to do various high-precision jobs with regular robots, and improving their performance typically requires the coordinated development of several academic disciplines. Comparatively speaking, humans are capable of achieving outstanding overall performance when their body's individual units are sensed and controlled with low absolute accuracy and modest computational energy consumption. So, one viable way to enhance the performance of robotic systems is to create robotic systems and algorithms that are inspired by humans. The most recent research on intelligent robots with human-inspired decision-making, cognition, motion control, and system design is outlined in the review by Hong Qiao et al. in [128] for features that are inspired by behavior and the brain. In order to further the fusion of neurology, technology, and control and create a new generation of robotic systems, this review intends to offer a substantial insight into intelligent robots that are inspired by humans. Adrienne Kline et al.
In the health industry, machine learning is widely used to address issues, including clinical decision-support. Its use has traditionally been concentrated on single-mode data. In the biomedical discipline of machine learning, attempts to enhance prediction and emulate the multimodal aspect of clinical expert decision-making have been met by integrating dissimilar data. The goal of [129] was to synthesize the most recent studies in the area and pinpoint areas that might use more investigation. To describe multi-modal data fusion in the context of health, they carried out this review in compliance with the PRISMA extension for Scoping Reviews. From 2011 to 2021, search terms were created and utilized in the databases PubMed, Google Scholar, and IEEE Xplore. The analysis was conducted on a final batch of 128 articles. Oncology and neurology were the two health fields that used multi-modal approaches the most frequently. The most popular method of data merging was early fusion. Notably, adopting data fusion resulted in an increase in predicting performance. Lacking from the publications were analyses of how using multimodal techniques from various subpopulations may alleviate biases and healthcare inequalities, as well as clear clinical deployment plans, FDA approval, etc. These findings offer an overview of multimodal data fusion as it relates to issues with health diagnosis and prognosis. There aren't many studies that contrast multimodal and unimodal predictions for output. However, those that did saw an average improvement in prediction accuracy of 6.4%. While multi-modal machine learning offers more accurate estimates than unimodal approaches, it is less scalable and requires more time to concatenate the input.
Sensing devices that are both personal and omnipresent, like cellphones, have made it possible to gather data continuously and covertly. To forecast user contextual information such as location, emotion, physical activity, etc., machine learning techniques have been applied to continuous sensor data. Recently, there has been an increase in interest in using ubiquitous sensing technology for applications in mental health care, enabling automatic, continuous monitoring of various mental illnesses such as depression, anxiety, stress, and so on. The survey by Enrique Garcia-Ceja et al. [130] reviews current studies employing sensor data and machine learning in mental health monitoring systems (MHMS). We concentrated on studies related to mental illnesses and disorders such as depression, anxiety, bipolar disorder, stress, etc. To help with the study of relevant research and to outline the main phases of MHMS, they suggest a categorization taxonomy. Additionally, the field's research problems and potential future prospects are highlighted.
6.
Future prospect
A person's health is crucial for living a pleasant and fulfilling life. The WHO defines health as a condition of physical and mental fitness free from illness and disability. Healthcare is the process of preserving or enhancing health with the aid of sickness and injury prevention, diagnosis, and treatment. The majority of traditional healthcare practices involve manual management and upkeep of patient demographic information, case histories, diagnoses, medications, billing, and drug stock maintenance, which can result in human mistakes that have an impact on patients. By linking all the devices that monitor vital signs through a network to a decision support system, Internet of Things (IoT)-based smart healthcare eliminates human error and aids the doctor in making more accurate and timely diagnoses [131].
The primary area for expanding outreach and offering prompt assistance is digital health. We need to add digital health management assistance to healthcare systems at a time when they are under a lot of stress, and we should work to lower the hazards that lead to extra costs.
7.
Conclusions
Technology improvements have led to the development of more efficient monitoring tools that can often record multiple physiological signals at once. The relationship between multi-modal signal monitoring and digital health management, however, has not received significant attention. This article examines the most recent developments in multi-modal signal monitoring for digital health management to close the gap. The most recent advancements in multi-modal signal monitoring for digital health management are examined in this article. In order to thoroughly review the current use of digital health technology in the recovery of lower-limb symptoms, three specific digital health processes—lower-limb data collection, statistical analysis of lower-limb data, and lower-limb rehabilitation via digital health management—are covered in this article. By listing and analyzing the latest papers, we fully review the current application of digital health technology in lower-limb symptoms recovery. Still, there are certain limitations for digital health management using multi-modal signal monitoring. Future work will focus on broadening the application area and depth of research.
Acknowledgments
This study was supported in part by the Open Project of China International Science and Technology Cooperation Base on Intelligent Equipment Manufacturing in Special Service Environment (ISTC2021KF07), in part by 2020 Anhui Province Education and Teaching Research Project: Osaka Medical College Sino-japanese cooperation in running a new school model (2020jyxm1897).
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad:














