1.
Introduction
In this paper we are interested in finding some description of the non-abelian group F21 of order 21 as the group of symmetries of some geometrical object (just like, for instance, S3 is the group of symmetries of the equilateral triangle), or as the group of automorphisms of some combinatorial object (recall, for instance, that the non-abelian group F39 of order 39 is the group of automorphisms of the cyclic STS(13)). Finding such representations is not in general a surprise, since any finite group G is the automorphism group of a suitable simple graph by Frucht's theorem [13]. On the other hand, in the case where G=F21, Frucht's theorem requires a quite big graph (with more than 100 vertices). The goal of this paper is to provide three different (but related) geometrical representations of F21, each of which uses only 7 vertices.
In Section 2 we show that F21 is the group of common automorphisms of two orthogonal Fano planes. Orthogonal STSs were first introduced by O'Shaughnessy [30] as a means of constructing Room squares, although the terminology "orthogonal Steiner system" was first introduced in [27, §5]. Formally, the notion of a Fano plane and the orthogonality between two Steiner triple systems can be defined as follows.
Definition 1.1. (see, e.g., [1,4,6,7]) A Steiner triple system of order v, denoted as STS(v), is a pair (V,B), where V is a set of v elements (points), and B is a collection of (unordered) triples of elements of V (blocks), with the property that each (unordered) pair of points occurs as a subset of precisely one block in B. A Steiner triple system of order 7 is also called a Fano plane.
An isomorphism from an STS (V1,B1) to an STS (V2,B2) is a one-to-one map σ from V1 onto V2 that maps B1 onto B2. An automorphism is an isomorphism from an STS to itself. The group of all the automorphisms of a Steiner triple system D=(V,B) is denoted by Aut(D).
Definition 1.2. Two STSs (V,B1) and (V,B2) on the same point-set are disjoint if B1∩B2=∅, that is, if they have no blocks in common. They are said to be orthogonal if, in addition, they satisfy the following property:
In the special case where the order of the STS is equal to 7, it is easy to see that two Fano planes are orthogonal if and only if they are disjoint.
Example 1.3. Let ˉB1 be the family of the 3-subsets of Z7={0,1,2,3,4,5,6} that are obtained by translating {0,1,3} (mod 7). More precisely,
Similarly, we define ˉB2 as the set of translates (mod 7) of the triple {0,1,5} or, more precisely,
Since ˉB1∩ˉB2=∅, it is immediate that ˉF1=(Z7,ˉB1) and ˉF2=(Z7,ˉB2) are orthogonal Fano planes on the common point-set Z7.
Note that, up to replacing 0 by 7,ˉB1 and ˉB2 coincide precisely with the families S1 and S2, respectively, defined in [30] as a first example of orthogonal Fano planes (where the triple 713 was mistakenly reported as 712), which were introduced in order to construct a 7×7 array (a Room square of side 7) whose rows and columns were determined by S1 and S2, respectively (this is probably the reason why the two Fano planes were later said to be orthogonal).
Example 1.4. One can readily verify by inspection that the map λ2(x)=2x (mod 7) is a common automorphism of the orthogonal Fano planes ˉF1 and ˉF2.
In the final part of Section 2, we apply the main result on the group of common automorphisms of two orthogonal Fano planes to show that F21 is also the automorphism group of a notable Kirkman triple system of order 15.
Subsequently, in Section 3, we investigate the automorphism group of an oriented Fano plane, and show that it is isomorphic to F21 as well. Formally, an oriented Fano plane and its automorphism group can be defined as follows.
Definition 1.5. A Fano plane F=(V,B) is oriented if there exists a binary antisymmetric relation → on V such that
● for any triple {x1,x2,x3}∈B, either x1→x2→x3→x1 or x1→x3→x2→x1 (in particular, for any distinct x,y in V, either x→y or y→x);
● for any x∈V, if {x,y1,z1}, {x,y2,z2}, and {x,y3,z3} are the three triples in B through x, and if x→y1, x→y2, and x→y3, then {y1,y2,y3}∈B.
If this is the case, then we say that the relation → is an orientation on F, and that the pair (F,→) is an oriented Fano plane.
Example 1.6. Let ˉF1=(Z7,ˉB1) be the Fano plane introduced in Example 1.3. We define an orientation → on ˉF1 by first defining 0→1→3→0 on the base triple {0,1,3}, and then extending the orientation by translation (mod 7) on the other triples. In other words, for any x in V=Z7, we let x→x+1→x+3→x.
The definition is well posed, since any two distinct points in V belong to exactly one triple in ˉB1. Note that, equivalently, for any two distinct points x,y in V,x→y if and only if y−x is a non-zero quadratic residue (mod 7), that is, if and only if y−x∈{1,2,4} (mod 7), that is, if and only if y∈{x+1,x+2,x+4} (mod 7). Therefore, for any x∈V, the orientation on the three blocks through x is
Now {x+1,x+2,x+4}, being a translate of the block {1,2,4}, is a block in ˉB1, hence it follows, by Definition 1.5, that (ˉF1,→) is an oriented Fano plane.
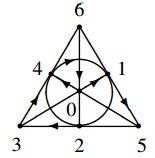
Note that the orientation → on ˉF1 can also be represented graphically as in Figure 1. For the sake of simplicity, we indicate only one arrow mark for each triple, but it is understood that the orientation is extended cyclically to all the 2-subsets of the triple. For instance, the arrow mark at the base of the triangle indicates the orientation 2→3→5→2 on the triple {2,3,5}.
Definition 1.7. Let (F,→) be an oriented Fano plane with point-set V, and let σ be an automorphism of F. We denote by either σ(→) or →σ the orientation on F induced by σ and → as follows: for any x,y in V,σ(x)→σσ(y) if and only if x→y. We say that σ is an automorphism of (F,→) if σ preserves the orientation →, that is, if σ(→)=→ (equivalently, for any x,y in V,σ(x)→σ(y) if and only if x→y).
Remark 1.8. The map λ2(x)=2x (mod 7), introduced in Example 1.4, is an automorphism of the oriented Fano plane (ˉF1,→) defined in Example 1.6, since the set {1,2,4} of quadratic residues (mod 7) is invariant under λ2. Note that this map can be visualized in Figure 1 as a clockwise 120-degree rotation of the triangle around its center. Moreover, the map τ1(x)=x+1(mod7) is an automorphism of (ˉF1,→) as well, by definition of →.
The concept of an oriented Fano plane was originally introduced as a mnemonic rule for the multiplication table of the octonions. In [18, §4.4], Peter L. Killgore investigated the group of the automorphisms of the octonions that permute the imaginary basis units e1,e2,e3,e4,e5,e6,e7, and proved that this group is a non-abelian group of order 21 and is isomorphic to the automorphism group of an oriented Fano plane. In Section 3, we give an alternative and more direct proof of this result, by constructing a one-to-one correspondence between the family of the orientations on a given Fano plane F and the family of the Fano planes orthogonal to F.
In Section 4, in order to provide an additional geometrical representation of F21, we study the triangular embeddings of the complete graph K7 (i.e., those whose faces have all length three).
Here graphs are finite, undirected and simple; loops and multiple edges are not allowed. A surface is a compact connected 2-manifold without boundary. Let us recall that a graph embedding into a surface can be defined as follows [25,26].
Definition 1.9. Given a graph Γ and a surface Σ, an embedding of Γ in Σ is a continuous injective mapping ψ:Γ→Σ, where Γ is viewed as a 1-dimensional simplicial complex, with the usual topology.
The connected components of Σ∖ψ(Γ) are called ψ-faces. If every ψ-face is homeomorphic to an open disc, then the embedding ψ is said to be cellular. Also, we say that a circuit T=(x1,x2,x3,…,xℓ) of Γ is a face of length ℓ (induced by the embedding ψ) if ψ([x1,x2]∪[x2,x3]∪…∪[xℓ,x1]) is the boundary of a ψ-face (where [xi,xi+1] denotes the arc with endpoints xi and xi+1 in the 1-dimensional simplicial complex). For instance, the faces of the classical toroidal embedding of K7 in Figure 2 (see also [2,16,35]) are precisely the blocks of the orthogonal Fano planes ˉF1 and ˉF2 introduced in Example 1.3.
Definition 1.10. We say that two graph embeddings ψ:Γ→Σ and ψ′:Γ′→Σ′ are isomorphic whenever there exists a graph isomorphism σ:Γ→Γ′ such that σ(T) is a face in Γ′ (induced by ψ′) if and only if T is a face in Γ (induced by ψ). The map σ will be called an embedding isomorphism (embedding automorphism in the case where ψ′=ψ).
Note that, in Definition 1.10, σ is a graph isomorphism and not a surface homeomorphism.
Let us recall that the classical embedding of K7 into the 2-torus admits a face 2-coloring, that is, a coloration of the faces with two colors and with the property that any edge belongs to two faces of different colors. In Figure 2 we color in gray the face {0,1,3} and its translates (which are the elements of ˉB1 in Example 1.3), and in white the face {0,1,5} and its translates (which are the elements of ˉB2). We also recall that, in general, a graph embedding that admits such a coloring is said to be a biembedding.
We give an independent proof that any triangular embedding of K7 into a surface is isomorphic to the classical toroidal one and hence is face 2-colorable. Moreover, we show that, for any such embedding ψ, the group Aut(ψ,Col) of the embedding automorphisms that fix the color classes is isomorphic to the automorphism group of two orthogonal Fano planes and hence is F21.
Some of the results in this paper may be at least partially known, but we include direct and independent proofs for the convenience of the reader, in order to make the paper self-contained and offer a unified view on the subject.
2.
Orthogonal Fano planes
2.1. Automorphisms of two orthogonal Fano planes
In this section, we aim to determine the group of common automorphisms of two orthogonal Fano planes.
First of all, we introduce some notation and terminology. From now on, we identify the point-set of two orthogonal Fano planes with Z7={0,1,2,3,4,5,6}, and we often indicate the triple {x,y,z}, briefly, by xyz. In the special case where the triple xyz belongs to the family of blocks of a Fano plane F, we also say, for short, that F contains the triple xyz, or that xyz is a triple in F.
We begin by proving that the group of common automorphisms of two orthogonal Fano planes does not depend on the choice of the two Fano planes.
Proposition 2.1. There exist precisely eight orthogonal Fano planes to a given Fano plane F. Moreover, Aut(F) acts transitively on the family of the eight orthogonal Fano planes.
Proof. Up to isomorphism, we may assume that F is the Fano plane ˉF1 introduced in Example 1.3.
Any Fano plane orthogonal to F contains a triple 01x, with x in {2,4,5,6}, and a triple x3y, where y can only be one of the two points different from 0,1,3,x and from the third point in the same triple with x and 3 in F. The choice of x and y determines a unique Fano plane S orthogonal to F. Indeed, up to permutation, we may assume that x=5. Then y∈{4,6}, say y=6 (resp., y=4). In this case, S contains the triples 01x=015,x3y=536, and 524 (resp., 015,534, and 526). Since F contains 602 (resp., 124), S necessarily contains 604 and 023 (resp., 146 and 123), and, finally, 126 and 134 (resp., 024 and 036). Hence S is uniquely determined by x and y, as claimed. Since there are four possible choices for x and two possible choices for y, it follows that there exist precisely eight orthogonal Fano planes to the given Fano plane F.
Now let S1 and S2 be two distinct Fano planes orthogonal to F. If S1 contains 01x and S2 contains 01x′, for some x,x′ in {2,4,5,6}, then we may assume that x′=x, else we replace S2 by σ(S2), where σ is the unique automorphism of F that fixes 0 and 1 and maps x′ to x. Note that σ(S2) is orthogonal to F, since S2 is orthogonal to F and σ(F)=F. Hence we may assume that S1 and S2 are precisely the only two Fano planes that are orthogonal to F and that contain 01x. If S1 contains x3y and S2 contains x3y′ (with y′ necessarily different from y), then it is easy to show that the permutation (01)(yy′) is an automorphism of F and an isomorphism between S1 and S2. This finally proves our claim. □
Corollary 2.2. The group of common automorphisms of two orthogonal Fano planes does not depend on the choice of the two Fano planes.
Proof. Since the Fano plane is unique up to isomorphism, it suffices to show that if (F,S1) and (F,S2) are two pairs of orthogonal Fano planes, then the group of common automorphisms of F and S1 is (isomorphic to) the group of common automorphisms of F and S2. This is now, in turn, an immediate consequence of Proposition 2.1. □
We can now state the main result of this section. An alternative proof can be found in our earlier draft [11].
Theorem 2.3. The group of common automorphisms of two orthogonal Fano planes is isomorphic to the Frobenius group F21 of order 21.
Proof. Let F1 and F2 be two orthogonal Fano planes. Then, by Proposition 2.1, Aut(F1) acts transitively on the eight Fano planes orthogonal to F1. This means that the group of the common automorphisms of F1 and F2 can be seen as the stabilizer of F2 under this action.
On the other hand, Aut(F1) is isomorphic to PSL2(7), and it is well known that PSL2(7) can act transitively in a unique way on a set of cardinality 8 (it admits a unique irreducible permutation representation of length 8). This action can be seen also as the conjugation action of PSL2(7) on its eight Sylow 7-subgroups. Since the stabilizer of a given Sylow 7-subgroup under this action is its normalizer, which is F21, it follows by uniqueness that the group of common automorphisms of two orthogonal Fano planes is isomorphic to F21 as well. □
Remark 2.4. It is well known that the Frobenius group of order 21 is the group of permutations of Z7={0,1,2,3,4,5,6} generated by λ2(x)=2x and τ1(x)=x+1(mod7). Equivalently, F21 is the group of all the affine transformations of the form x↦ax+b,x∈Z7, with b∈Z7 and a∈{1,2,4}. Indeed, if ˉF1 and ˉF2 are the two orthogonal Fano planes defined in Example 1.3, then, as we noted in Example 1.4, and by definition of ˉF1 and ˉF2, λ2 and τ1 are two common automorphisms of ˉF1 and ˉF2. Since λ2 and τ1 have order 3 and 7, respectively, they generate a group which necessarily coincides with the order-21 group of common automorphisms of ˉF1 and ˉF2.
Remark 2.5. It would be interesting to investigate the group of common automorphisms of two orthogonal STS(v)s also for v>7. The smallest order v>7 for which there exist two orthogonal Steiner triple systems is v=13 [28], and, for this order, there exists, up to isomorphism, only one pair of orthogonal STSs (see, for instance, [14]). Such a pair can be constructed as follows. The common point-set is the cyclic group Z13, and the blocks of the first STS (which is known as the cyclic STS(13)) are the twenty-six triples that are cyclically generated (mod 13) by the two base blocks {1,3,9} and {2,5,6} (see, e.g., [7, Theorem 2.11]). Also, the group of automorphisms of the STS is the order-39 Frobenius group of all affine transformations of the form
with b∈Z13 and a∈{1,3,9}.
The blocks of the second STS are the triples {−u,−v,−w} (mod 13), as {u,v,w} ranges over the blocks of the first STS. It is easy to check that the two STSs are orthogonal. Also, since the map x↦−x is an isomorphism between the two STSs, the automorphisms of the second STS are all the transformations of the form x↦−φa,b(−x)=ax−b, with b∈Z13 and a∈{1,3,9}, hence the group of the common automorphisms of the two STSs simply coincides with the automorphism group of the first STS.
2.2. The STS(15) #61 and the KTS(15) #61
Here we apply Theorem 2.3 to show that the Frobenius group F21 is also the automorphism group of a notable resolvable Steiner triple system of order 15 and of the corresponding Kirkman triple system.
We first need some preliminary definitions (see, e.g., [4,6,7]).
Definition 2.6. Given an STS(v) (V,B) with v multiple of 3, a parallel class is a subcollection of v/3 mutually disjoint blocks in B that partition the point-set V. When the entire collection B of blocks can, in turn, be partitioned into parallel classes, such a partition R is called a resolution of the STS, and the STS is said to be resolvable. If this is the case, then (V,B,R) is called a Kirkman triple system of order v, denoted as KTS(v), and (V,B) is its underlying STS.
An isomorphism from a KTS (V1,B1,R1) to a KTS (V2,B2,R2) is an isomorphism σ from the STS (V1,B1) to the STS (V2,B2) that, in addition, preserves the parallel classes: for any parallel class C in R1, the set {σ(T)|T∈C} is a parallel class in R2. An automorphism is an isomorphism from a KTS to itself.
Note that any KTS of order 15 is a solution of the famous fifteen schoolgirl problem [19, p. 48].
There exist eighty non-isomorphic Steiner triple systems of order 15 [36], four of which are resolvable [8] (cf. [6, p. 66], [7, p. 370]). Three of the four resolvable STS(15)s underlie two non-isomorphic KTSs, whereas the fourth resolvable STS, usually known as #61, underlies a unique Kirkman triple system of order 15. Moreover, the system #61 is one of the sixteen STS(15)s containing a unique Fano plane, and, among these, it is the system with the least number of Pasch configurations (see, for instance, [6, Table 1.29], [7, Table 5.11]). Finally, the corresponding KTS, which is also denoted by #61, is related to Sylvester's problem of the 15 schoolgirls: can the 35×13 (unordered) triples of elements of a 15-set be grouped into the blocks of 13 different KTS(15)s? Denniston's 1974 solution [12] (see also [1, Example 7.3.2], [6, Example 2.71, p. 66]) contains 13 KTS(15)s all isomorphic to the KTS #61.
It is well known that the group of automorphisms of the STS(15) #61 is isomorphic to the Frobenius group of order 21. This property, however, to the best of our knowledge, is reported in the literature as a mere "fact", without an actual proof (the same can be said about the automorphism group of the corresponding KTS). The two main references are [8] and [24], where the automorphism group is given as the group generated by two explicit permutations on fifteen symbols, with no further details or references. Here we prove that the group is isomorphic to the group of common automorphisms of two orthogonal Fano planes and hence is isomorphic, by Theorem 2.3, to the Frobenius group of order 21.
Theorem 2.7. The automorphism group of the STS(15) #61 is isomorphic to the Frobenius group of order 21.
Proof. Up to isomorphism, the STS(15) #61 is the Steiner triple system D=(V,B) with point-set
and block-set B consisting of the 35 triples cyclically generated (mod 7) by the base blocks 00′∞,013,01′6′,02′5′, and 03′4′ (see, for instance, [32, §3, Example 5]). Let us set
Let ˉF1 be the Fano plane with point-set P and block-set consisting of the seven triples cyclically generated (mod 7) by the base block 013. Then ˉF1 is the unique Fano plane contained in D, and hence it is invariant under any automorphism of D. It follows, in particular, that P and Pc are both invariant under any automorphism of D. Also, let ˉF2 be the Fano plane, with point-set P, whose blocks are the seven triples cyclically generated (mod 7) by the triple 015. Then, as we already noted before (see Example 1.3), ˉF1 and ˉF2 are orthogonal Fano planes.
By construction, B does not contain any 3-subset of Pc. It follows, by definition of an STS, that for any 2-subset {x,y} of Pc there exists a unique block axy in B, with a in P. Therefore, for any of the fifty-six 3-subsets {x,y,z} of Pc, there exists a unique 3-subset {a,b,c} of P such that axy,bxz, and cyz are blocks in B. Since P contains thirty-five 3-subsets, the map {x,y,z}↦{a,b,c} is not injective.
One can readily verify, by inspection, that the seven blocks of ˉF1 and the seven blocks of ˉF2 are the only 3-subsets {a,b,c} of P that are associated with a unique 3-subset {x,y,z} of Pc (and that any other 3-set {a,b,c} is associated with exactly two 3-sets {x,y,z}). More precisely, each block {i,i+1,i+3} in ˉF1 is associated only with {x,y,z}={(i+2)′,(i+4)′,(i+5)′}, and each block {i,i+1,i+5} in ˉF2 is associated only with {x,y,z}={(i+3)′,(i+4)′,(i+6)′} (mod 7). It follows that the family of the fourteen blocks in ˉF1 and ˉF2 is invariant under any automorphism of D. Since the same holds for ˉF1, we conclude that ˉF2 is invariant under any automorphism of D as well.
It follows from the properties above that the element ∞ is the only point in Pc that never occurs in a triple {x,y,z} associated with a block {a,b,c} in either ˉF1 or ˉF2. Therefore ∞ is invariant under any automorphism of D. This immediately implies, in turn, that any automorphism of D is uniquely determined by its restriction to P. Indeed, for any n in P, and for any automorphism σ of D,σ(n) is in P and σ(nn′∞)=σ(n)σ(n′)∞, hence σ(n′) necessarily coincides with σ(n)′.
It follows that the restriction map σ↦σ|P is an isomorphism between AutD and a subgroup of the group of common automorphisms of the orthogonal Fano planes ˉF1 and ˉF2. Hence AutD has at most 21 elements. On the other hand, the map ∞↦∞,n↦n+1,n′↦(n+1)′ (mod 7) is by construction an order-7 automorphism of D, and it can be readily verified that the map ∞↦∞,n↦2n,n′↦(2n)′ (mod 7) is an order-3 automorphism of D. Therefore AutD has at least 21 elements, and AutD≅F21 by Theorem 2.3.
This finally completes the proof of the theorem. □
As we mentioned earlier, the STS D=(V,B) in the proof of Theorem 2.7 is a resolvable Steiner triple system of order 15, usually known as #61. The thirty-five blocks of the system can be collected in seven parallel classes, which are generated (mod 7) by the base parallel class {00′∞,124,31′5′,54′6′,62′3′} (see, for instance, [32, §3,Example 5]). The corresponding Kirkman triple system, which is also denoted by #61, has the same automorphisms as D. Indeed, the Steiner triple system D only contains seven parallel classes [24] (cf. [6, Table 1.29], [7, p. 370]), which are necessarily permuted by any automorphism of D. It follows, by definition, that any automorphism of D is also an automorphism of the KTS #61 (cf. [8, System VII]). As a consequence of Theorem 2.7 above, we obtain the following well-known result (see, e.g., [8]), for which no complete formal proof seems to have appeared in print in the literature.
Corollary 2.8. The automorphism group of the KTS(15) #61 is isomorphic to the Frobenius group of order 21.
3.
Automorphisms of an oriented Fano plane
The goal of this section is to prove that the automorphism group of an oriented Fano plane is F21. This result will be proved by showing that the group under consideration is isomorphic to the automorphism group of two orthogonal Fano planes.
Let us recall that, if F is a Fano plane, then by saying that {a,b,c} is a triple in F we mean that {a,b,c} is a block in F.
Lemma 3.1. Let F and S be two orthogonal Fano planes with point-set V. Then for any triple {a,b,c} in F there exists a unique triple {x,y,z} in S such that {a,b,c} and {x,y,z} are disjoint.
Also, for any v in V, there exist a unique triple {a,b,c} in F and a unique triple {x,y,z} in S such that {v}∪{a,b,c}∪{x,y,z} is a partition of V.
Proof. If {a,b,c} is a triple in F, then S contains a triple through a and b, a triple through a and c, a triple through b and c, three additional triples with exactly one element in {a,b,c}, and a unique triple disjoint from {a,b,c}. Now let v be in V. Each of the three triples in F containing v is disjoint from a (unique) triple in S, not containing v, and each of the three triples in S containing v is disjoint from a (unique) triple in F, not containing v. This leaves a unique pair of disjoint triples T1, T2, not containing v, with T1 in F and T2 in S. □
Proposition 3.2. Let F=(V,B) be a Fano plane, and let → be an orientation on F. For each v in V, let T(v) be the triple of the three points x,y,z in V such that x→v, y→v, z→v. If B→ is the family of the seven triples T(v), as v ranges over V, then F→=(V,B→) is a Fano plane orthogonal to F.
Moreover, the map φ(→)=F→ defines a bijection between the family of the orientations on F and the family of the Fano planes orthogonal to F.
Proof. Suppose, for instance, that F contains the triples abc,ade,afg,bdf,beg,cdg, and cef. Let → be an orientation on F. We may assume, up to permutation, that a→b→c→a and a→d→e→a. Since bdf is a triple in F, this implies, by Definition 1.5, that the orientation on the triple afg is a→f→g→a. Since c→a and adf is not a triple in F, the conditions c→d and c→f cannot both hold, hence either d→c or f→c. If the two latter conditions were both satisfied, then the orientation on the two triples cdg and cef would be d→c→g→d and f→c→e→f, against the hypotheses that c→a and age is not a triple in F. Therefore one and only one of the two conditions d→c and f→c occurs. We assume that d→c and c→f, the other case being examined similarly.
The orientation on the two triples cdg and cef is now d→c→g→d and c→f→e→c. Since f→e and f→g (resp., g→a and g→d), and since beg (resp., ade) is a triple in F, forcing f→b (resp., g→e), the orientation on the triple bdf (resp., beg) is f→b→d→f (resp., g→e→b→g). This finally specifies the orientation on all seven triples in F (and hence on any pair of distinct elements of V). Also, B→ is, by definition, the family {T(a),T(b),…,T(g)}={ceg,aef,bde,abg,dfg,acd,bcf}, hence F→ is a Fano plane orthogonal to F.
We now need to prove that the map φ(→)=F→ is a bijection. Let S be a Fano plane orthogonal to F. We need to show that there exists a unique orientation → on F such that F→=S.
Let v be a given point in V. Since F and S are orthogonal Fano planes, by Lemma 3.1 there exist a unique triple αβγ in F and a unique triple xyz in S such that {v}∪{α,β,γ}∪{x,y,z} is a partition of V. Therefore, by Definition 1.5, if S=F→ for some orientation → on F, then the triple T(v) coincides necessarily with xyz, hence x→v, y→v, z→v, and v→α, v→β, v→γ. Since v was an arbitrary point in V, it follows that S determines the orientation → on F uniquely (that is, the map φ is injective).
We are now left to prove that the above definition of the orientation → induced by S is well posed (if this is the case, then S=F→ by construction, that is, φ is surjective). Equivalently, we need to show that, as v ranges over V, the partitions {v}∪{α,β,γ}∪{x,y,z} of V determine an orientation on F unambiguously. Given a triple ijk in F, it suffices to prove that the choices v=i, v=j, and v=k produce the same orientation on ijk.
Since any triple in F different from ijk intersects ijk in precisely one point, we may assume that, for v=i, there exist a triple kmn in F and a triple juw in S that partition V together with i, thereby inducing the orientation j→i→k→j. Also, for v=j, there exist a triple TF in F and a triple TS in S that partition V together with j. Now TS and juw, being distinct triples in S, have precisely one element in common (necessarily different from j), hence we may assume that TS contains u but not w, that is, w∈TF and u∉TF. This implies, in turn, that k∉TF, since kuw is the unique triple in F containing k and w. Hence TF∩{i,j,k}={i} and k∈TS, say TF=imw and TS=kun, thus inducing again the orientation j→i→k→j. Finally, in addition to kuw and imw,F must also contain jnw, whereas S, in addition to juw and kun, must also contain ium, which is disjoint from jnw and {k}. Therefore the choice v=k produces again the orientation j→i→k→j. It follows that → is a well-defined orientation on F, as claimed. □
Propositions 2.1 and 3.2 now immediately imply the following results.
Corollary 3.3. A given Fano plane admits exactly eight distinct orientations.
Corollary 3.4. Let →1 and →2 be two orientations on a Fano plane F. Then there exists an automorphism σ of F such that σ(→1)=→2. As a consequence, the group of automorphisms of an oriented Fano plane does not depend on the choice of the orientation.
Proof. Let →1 and →2 be two orientations on F, corresponding to the two pairs of orthogonal Fano planes (F,F→1) and (F,F→2), respectively, by Proposition 3.2. Now, by Proposition 2.1, there exists an automorphism σ of F that is an isomorphism from F→1 onto F→2. In particular, σ(F→1)=F→2. On the other hand, it is immediate by Proposition 3.2 that σ(F→1)=Fσ(→1), hence σ(→1)=→2.
Therefore, if μ is an automorphism of F, then μ is an automorphism of (F,→2) if and only if σ−1μσ is an automorphism of (F,→1). It follows that the group of automorphisms of (F,→2) is (isomorphic to) the group of automorphisms of (F,→1). □
Corollary 3.5. Let F be a Fano plane, let → be an orientation on F, and let F→ be the associated orthogonal Fano plane. If σ is an automorphism of F, then σ is an automorphism of (F,→) if and only if σ is an automorphism of F→. As a consequence, the automorphism group of the oriented Fano plane (F,→) is isomorphic to the group of common automorphisms of the two orthogonal Fano planes F and F→.
Proof. Let σ be an automorphism of F. Since F→ is the orthogonal Fano plane associated with →, σ(F→) is the orthogonal Fano plane associated with σ(→). Hence σ(→)=→ if and only if σ(F→)=F→. Equivalently, by Definition 1.7, σ is an automorphism of (F,→) if and only if σ is an automorphism of F→. □
In view of Corollaries 3.4 and 3.5, and of Theorem 2.3, we can now state the main result of this section.
Theorem 3.6. The group of automorphisms of an oriented Fano plane is isomorphic to the Frobenius group of order 21.
Remark 3.7. Let (F,→) be an oriented Fano plane. In particular, by Definition 1.5, → induces a cyclic orientation on each of the seven triples in F. Moreover, the first part of the proof of Proposition 3.2 shows that → induces a cyclic orientation also on each of the seven triples T(a),T(b),…,T(g) in F→. In fact, the orthogonal Fano plane F→ can be characterized as the unique Fano plane S, orthogonal to F, with the property that the orientation → on F induces a cyclic orientation on each of the seven triples in S, as well.
As a consequence, besides the definition of F→ in Proposition 3.2, the triples in F→ can also be determined as follows. Given two distinct points a,b in the point-set V, if abc is the triple through a and b in F, say a→b→c→a, then the triple through a and b in F→ is precisely the triple abx, where x is the unique point in V, different from c, such that a→b→x→a. Indeed, if x were not unique, then a→b→y→a for an additional point y, and thus, by Definition 1.5 and Proposition 3.2, cxy (=T(a)) would be a triple in both F and F→, against the fact that the two Fano planes are orthogonal.
For instance, if (ˉF1,→) is the oriented Fano plane in Figure 1 (see Example 1.6), then it is clear from the picture that the cyclic orientation 0→1→x→0 occurs if and only if either x=3 or x=5, which corresponds to the fact that 013 is a triple in ˉF1 and 015 is a triple in the orthogonal Fano plane (ˉF1)→. Also, one easily finds that (ˉF1)→ is precisely the Fano plane ˉF2 introduced in Example 1.3. With a different viewpoint, the property that the orientation → induces a cyclic orientation on the triples of both the orthogonal Fano planes ˉF1 and ˉF2 is an immediate consequence of the fact that, for any two distinct points x,y in Z7,x→y if and only if y−x∈{1,2,4} (mod 7), which, in turn, yields the cyclic orientation x→x+1→x+3→x and x→x+1→x+5→x on all the triples in ˉF1 and ˉF2, respectively. A nice graphical visualization of this property will be proposed in the subsequent Remark 4.6 in Section 4.
In an earlier draft [11, Remark 3.7(b), (c), (d)], we give other properties of the orthogonal Fano plane F→, together with alternative proofs of Corollary 3.3 and Theorem 3.6. Also, we characterize the orientations on a given Fano plane in terms of the Hamiltonian circuits in K7 with the property that the seven edges corresponding to pairs of consecutive vertices "cover" all the seven blocks of the plane. In the present paper we omitted all these observations for the sake of brevity.
Remark 3.8. It is well known that the oriented Fano plane completely describes the algebraic structure of the octonions, which are an 8-dimensional algebra over the real numbers, with basis 1,e1,e2,e3,e4,e5,e6,e7, and a multiplication table given as in [3, Table 1] (which is known as the Cartan-Schouten-Coxeter octonion multiplication table). The octonions constitute, up to isomorphism, the unique non-associative and non-commutative normed division algebra. The basis element 1 is also the multiplicative identity, e1,…,e7 are square roots of −1, and the remaining products are cyclically generated (mod 7) by the basic products e1e2=e4 and e2e1=−e4.
If we replace the symbols 1,2,…,6 in Figure 1 by e1,e2,…,e6, respectively, and the symbol 0 by e7, then the orientation of the blocks of the Fano plane completely determines the product of any two distinct basis elements different from the multiplicative identity 1: if {ei,ej,ek} is a block with orientation ei→ej→ek, then eiej=ek and ejei=−ek [3, p. 152] (an earlier version of the same construction can be found in [23, Figure 2]. See also [5]).
It is then immediate that Theorem 3.6 can be seen as a reformulation of the following result on the automorphisms of the octonions. This result was probably known before the undergraduate thesis [18] that we are quoting here, although no earlier proof seems to have appeared in print.
Corollary 3.9. [18, §4.4] The group of the automorphisms of the octonions that permute the imaginary basis units e1,e2,e3,e4,e5,e6,e7 is isomorphic to the Frobenius group of order 21.
4.
Combinatorial embeddings of graphs
In this section we give an independent proof that any triangular embedding of the complete graph K7 into a surface is isomorphic to the classical biembedding into the 2-torus (in particular, any such embedding is face 2-colorable). Moreover, we show that the group of the embedding automorphisms that preserve the color classes is isomorphic to F21.
Let us recall that, if ψ is a biembedding, then the group of the embedding automorphisms that fix the color classes is denoted by Aut(ψ,Col) (see the terminology introduced in Definition 1.10).
Theorem 4.1. If φ:K7→T2 is the classical biembedding of K7 into the 2-torus, then Aut(φ,Col) is isomorphic to the Frobenius group of order 21.
Proof. Let Z7={0,1,2,3,4,5,6} be the vertex-set of K7, and let φ be the embedding of K7 into the 2-torus presented in Figure 2. By Definition 1.10, an embedding automorphism (of φ) is a permutation σ of Z7 that permutes the 14 faces of K7 induced by φ, which are, in turn, the 7 blocks of the Fano plane ˉF1=(V,ˉB1) and the 7 blocks of the Fano plane ˉF2=(V,ˉB2) introduced in Example 1.3. Since the two color classes of φ are precisely ˉB1 and ˉB2, we conclude that σ is in Aut(φ,Col) if and only if σ is a common automorphism of the orthogonal Fano planes ˉF1 and ˉF2. The thesis is now an immediate consequence of Theorem 2.3. □
More in general, in the remaining part of this section, we investigate the triangular embeddings of K7 and their automorphisms. Following [10,15,17,35], we provide an equivalent, but purely combinatorial, definition of a graph embedding into a surface, assuming that the surface is orientable. Given a graph Γ=(V,E), we denote by D(Γ) the set of all the ordered pairs (x,y) and (y,x), as {x,y} ranges over the edge-set E, and we say that the elements of D(Γ) are the oriented edges of Γ (note that the cardinality of D(Γ) is twice the cardinality of E, and that we do not assume any prescribed orientation on the edges of Γ). Also, given a vertex x in V, we denote by N(Γ,x) the set of all the vertices adjacent to x in Γ.
Definition 4.2. Let Γ=(V,E) be a connected graph. A combinatorial embedding of Γ is a pair Π=(Γ,ρ), where ρ:D(Γ)→D(Γ) is a map satisfying the following property: for any x in V, there exists a permutation ρx of N(Γ,x) that is a cycle of order |N(Γ,x)| and is such that, for any y∈N(Γ,x), ρ(x,y)=(x,ρx(y)). The map ρ is said to be a rotation of Γ.
As reported in [15], a cellular embedding of a (connected) graph Γ into an orientable surface is equivalent to a combinatorial embedding Π=(Γ,ρ) (see also [2, Theorem 3.1] and [31]). This yields, in particular, a purely combinatorial description of the faces of Γ induced by the embedding. Indeed, in the case of a combinatorial embedding (Γ,ρ), an edge {x,y} belongs to exactly two faces T1 and T2, which can be determined via the so-called face-tracing algorithm as follows:
We also recall that the notions of embedding isomorphism and embedding automorphism can be defined purely combinatorially as follows (see [20, p. 61]).
Definition 4.3. Let Γ and Γ′ be two connected graphs, and let Π=(Γ,ρ) and Π′=(Γ′,ρ′) be two combinatorial embeddings of Γ and Γ′, respectively. We say that Π is isomorphic to Π′ if there exists a graph isomorphism σ:Γ→Γ′ such that either
or
We also say that σ is an embedding isomorphism between Π and Π′. Moreover, if Eq (4.2) holds, then σ is said to be an orientation-preserving isomorphism, whereas, if (4.3) holds, then σ is said to be an orientation-reversing isomorphism. Also, if Γ′=Γ and ρ′=ρ (that is, Π′=Π), then we say that σ is an embedding automorphism of Π. We denote by Aut(Π) the group of all the embedding automorphisms of Π. Finally, if Π admits a face 2-coloring, then we denote by Aut(Π,Col) the group of the embedding automorphisms of Π that fix the color classes.
Let us first consider the classical toroidal embedding of K7, seen as a combinatorial embedding. As explained in [26], the rotation ρ associated with the biembedding of K7 in Figure 2, with vertex-set Z7={0,1,2,3,4,5,6}, can be obtained through the two following steps.
1) Given x∈Z7, the map ρx is defined by looking at the neighbors of x in a clockwise order. For example, for x=3,ρ3 is the cyclic permutation (025641) of Z7.
2) By Definition 4.2, ρ(x,y)=(x,ρx(y)) for any (x,y)∈D(Γ).
These two steps are enough to determine the map ρ. Alternatively, however, for a given x∈Z7, one can define the map ρ by clockwise rotation just on the oriented edges starting from x, and then extend the map ρ on the remaining oriented edges as follows.
3) Given a vertex x in Z7, every oriented edge of K7 can be written in the form (x+z,y+z), where the sum is performed modulo 7. Then we set
By doing so, one can easily check that the expression (4.4) for ρ is independent of the initial vertex x. Also, if τz is the permutation of Z7 defined by τz(x)=x+z(mod7), then property (4.4) can be written as
By Definition 4.3, this means that, for any z∈Z7, the map τz is an orientation-preserving automorphism of the combinatorial embedding ˉΠ=(K7,ρ). In particular, following the notation introduced in the same definition, τz∈Aut(K7,ρ) for any z∈Z7. In addition, the embedding (K7,ρ) is Z7-regular, that is, it admits Z7 as a sharply vertex-transitive automorphism group of K7. Note that, on the torus, the permutations τz can be visualized as one-step translations along the "lines" in Figure 2 (see Remark 4.5). Finally recall that, by Definition 4.3, Aut(ˉΠ,Col) denotes the group of the embedding automorphisms of ˉΠ=(K7,ρ) that fix the color classes.
We can now state an equivalent version of Theorem 4.1. One can also give a direct and independent proof of Theorem 4.4 as in an earlier draft of this paper [11].
Theorem 4.4. If ˉΠ=(K7,ρ) is the combinatorial embedding of Figure 2, then Aut(ˉΠ,Col)≅F21.
Proof. Since the combinatorial embedding ˉΠ=(K7,ρ) is equivalent to the embedding φ:K7→T2 of Figure 2, the thesis immediately follows from Theorem 4.1. □
Remark 4.5. It is immediate that the group Aut(ˉΠ,Col)≅F21 is generated by the permutations λ2(x)=2x and τ1(x)=x+1(mod7) of Z7={0,1,2,3,4,5,6} (note the analogy with Remark 2.4). This gives, by means of Figure 2, a very simple and elegant way to visualize the 21 permutations x↦ax+b (a∈{1,2,4},b∈Z7) in F21 as a group of transformations of the torus.
Indeed, every permutation of the form x↦2x+b (resp., x↦4x+b) can be seen as an order-3 counterclockwise (resp., clockwise) rotation around the point −b (resp., 2b), whereas every permutation of the form x↦x+b,b≠0, can be seen as a one-step translation along some "line" in Figure 2. For instance, the permutation x↦2x+4 (resp., x↦4x+5) represents an order-3 counterclockwise (resp., clockwise) rotation around the point 3, whereas the permutation x↦x+3 (resp., x↦x−3) can be visualized as a one-step upward (resp., downward) translation along the "lines" in Figure 2 parallel to the line containing 0,3, and 6.
Remark 4.6. We noted in Remark 3.7(a) that the orientation → defined in Example 1.6 (also represented in Figure 1) induces a cyclic orientation on the triples of both the orthogonal Fano planes ˉF1 and ˉF2 introduced in Example 1.3. This property can be easily visualized by means of the representation of the blocks in ˉF1 and ˉF2 as the gray and the white triangular faces in Figure 2, respectively. Indeed, for any pair of distinct points x,y in Z7={0,1,2,3,4,5,6}, let us reproduce on the edge {x,y} in Figure 2 the same arrow sign that appears in Figure 1 (equivalently, the arrow mark points from x to y if and only if y−x∈{1,2,4} (mod 7)). Then, as a result, every gray (resp. white) face ends up displaying a cyclic counterclockwise (resp. clockwise) orientation. For instance, there is a counterclockwise orientation 0→1→3→0 on the gray face {0,1,3} in the bottom-right corner of the picture, and a clockwise orientation 0→4→6→0 on the white face {0,4,6} in the upper-left corner.
In the final part of this section, we generalize Theorems 4.1 and 4.4 to an arbitrary triangular embedding of K7 into a surface. Note that the uniqueness of such an embedding, up to isomorphism, is well known [34, Theorem 0.2, p. 81, and Remark 0.3.(2), p. 84]. In the special case of triangular embeddings of K7 into the 2-torus, the uniqueness is proved in [22] as a consequence of more general results on toroidal graphs [29] or triangulations of the torus [21]. Here, in the spirit of the whole paper, we give a direct and self-contained proof that any triangular embedding of K7 into a surface is face 2-colorable and isomorphic to the classical toroidal (combinatorial) embedding ˉΠ=(K7,ρ).
We first consider the case where the surface is orientable. As we recalled earlier in this section, this is equivalent to considering a triangular combinatorial embedding of K7.
Lemma 4.7. Let Π′=(K7,ρ′) be a triangular combinatorial embedding of K7. If ρ′x(y)=z, then ρ′z(x)=y and ρ′y(z)=x.
Proof. Suppose that ρ′x(y)=z. Because of the face-tracing algorithm (see (4.1)), the face containing x,y, and z is
Since the embedding is triangular, it follows that ρ′z(x)=y. Similarly, starting from z, the same face can be written as
Since, again, the embedding is triangular, we conclude that ρ′y(z)=x. □
Proposition 4.8. Let Π′=(K7,ρ′) be a triangular combinatorial embedding of K7. Then Π′ is isomorphic to the classical toroidal embedding ˉΠ=(K7,ρ).
Proof. As usual, we identify the vertex-set of K7 with Z7={0,1,2,3,4,5,6}. In view of Definition 4.3, up to replacing ρ′ by σρ′σ−1, where σ is a suitable permutation of the vertices, we may assume that
as in ˉΠ. In particular, ρ′0(5)=4 and ρ′0(1)=5. Therefore, since the embedding is triangular, it follows from Lemma 4.7 that ρ′5 is of the form (401xyz), where {x,y,z}={2,3,6}. Suppose, by contradiction, that ρ′5(1)=3 (resp., ρ′5(6)=4). Together with ρ′0(1)=5 and ρ′0(3)=1 (resp., ρ′0(5)=4 and ρ′0(4)=6), this would imply, by Lemma 4.7, that the permutation ρ′1 (resp., ρ′4) contains the 3-cycle (350) (resp., (560)), contradicting the fact that ρ′1 (resp., ρ′4) has order six by Definition 4.2. Hence x≠3 and z≠6. Now ρ′0(6)=2 implies, by Lemma 4.7, that ρ′2(0)=6; hence ρ′2(5)≠6, which implies, in turn, that ρ′5(6)≠2. It finally follows that ρ′5 is either (401632) or (401263).
In the former case, the conditions ρ′5(1)=6, ρ′0(1)=5, and ρ′0(3)=1 imply, by Lemma 4.7, that ρ′1(6)=5, ρ′1(5)=0, and ρ′1(0)=3, respectively, hence ρ′1 is of the form (6503xy), where {x,y}={2,4}. Now ρ′5(3)=2⇒ρ′3(2)=5⇒ρ′3(2)≠1⇒x=ρ′1(3)≠2, thus ρ′1=(650342). By reasoning in the same way, one finds that ρ′2=(061453),ρ′3=(410256),ρ′4=(605213), and ρ′6=(351204). Hence ρ′=ρ, and Π′ is isomorphic to ˉΠ.
Let us finally assume that ρ′5=(401263). By arguing as in the previous case, one finds that
Now the permutation σ=(24)(35) of the vertex-set {0,1,2,3,4,5,6} is such that
for all oriented edges (x,y) in K7, as it can be easily checked by inspection. Hence, by Definition 4.3, Π′=(K7,ρ′) is isomorphic to ˉΠ=(K7,ρ), as claimed. □
We finally consider the case of an arbitrary triangular embedding of K7 into a surface, without assuming that the surface be orientable.
Corollary 4.9. Let ψ be a triangular embedding of K7 into a (non-necessarily orientable) surface Σ. Then the embedding admits a unique face 2-coloring and
Proof. As in the orientable case, each edge of K7 belongs to exactly two faces. Indeed, the two faces can be determined by means of the face-tracing algorithm induced by a suitable combinatorial embedding, which can be associated to ψ also in the non-orientable case (by generalizing [9, Definition 5.2]). Since the faces of ψ are triangular, the total number of faces is 14. Then, by Euler's formula, the Euler characteristic of Σ is
Therefore Σ is either the torus or the Klein bottle. On the other hand, it is well known that K7 does not admit embeddings into the Klein bottle (see, e.g., Theorem 4.11, p. 69, in [33]). Therefore Σ is orientable and hence, because of Proposition 4.8, ψ is isomorphic to the classical toroidal (combinatorial) embedding ˉΠ=(K7,ρ). The thesis now follows immediately from Theorem 4.4 and from the uniqueness of the face 2-coloring of ˉΠ. □
Author contributions
Simone Costa: Investigation, Writing – review and editing; Marco Pavone: Conceptualization, Investigation, Writing – original draft, Writing – review and editing. All authors have read and agreed to the published version of the manuscript.
Acknowledgments
The authors are grateful to the anonymous referees for their useful comments and suggestions. The authors were partially supported by INdAM–GNSAGA. This work was supported in part by the European Union under the Italian National Recovery and Resilience Plan (NRRP) of NextGenerationEU, Partnership on "Telecommunications of the Future, " Program "RESTART" under Grant PE00000001, "Netwin" Project (CUP E83C22004640001). The second author was supported also by Università di Palermo (FFR2024 Pavone).
Use of Generative-AI tools declaration
The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.
Conflict of interest
The authors declare no conflict of interest in this paper.









 DownLoad:
DownLoad: