Approximate controllability of Hilfer fractional neutral stochastic systems of the Sobolev type by using almost sectorial operators

1.   Introduction

Fractional calculus was introduced as a significant area of advanced calculus in 1695. The idea of fractional calculus has been effectively applied to a number of fields. Researchers in the fields of physics and mathematics have demonstrated that this calculus may accurately reflect a variety of non-local dynamics. The most common domains in which fractional calculus is used include elasticity, kinetic oscillations in identical and homogeneous constructions, aqueous waterways, imaging, viscoelasticity and other areas. The success of fractional structures has caused several researchers to re-evaluate their mathematical estimation techniques, because diagnostic configurations may not be available in many domains. The readers can discover some interesting findings on fractional dynamical systems in many research works about the theory and applications of fractional differential systems ^{[1,2,3,4,5,6]}. Particularly, partial neutral constructions with or without delays act as an overview of several partial neutral systems that appear in problems concerning heat transfer in components, viscoelasticity and a variety of natural events. Additionally, interested readers are able to review various books ^[7,8,9,10] and research articles ^{[11,12,13,14,15,16,17]} that focus on the most popular neutral structures.

Hilfer ^[18] pioneered fractional derivatives, including the Riemann-Liouville (RL) and Caputo derivatives. Additionally, some theoretical discussions on thermoelasticity in solid compounds, pharmaceutical manufacturing, rheological adaptive computing, mechanics and related areas have revealed the applicability of Hilfer fractional derivatives (HFDs). Gu and Trujillo ^[19], in 2015, used a measure of noncompactness method, along with the fixed-point criterion, to prove that the HFD evolution problem has an integral solution. They considered a new variable $\mathfrak{r}\in [0, 1]$, together with a fractional variable $\mathfrak{s}$, to indicate the derivative's order. As a result, $\mathfrak{r} = 0$ gives the RL derivative, while $\mathfrak{r} = 1$ gives the Caputo derivative. Numerous papers have been written in the context of Hilfer fractional calculus ^{[20,21,22,23]}. Jaiswal and Bahuguna ^[24] and Karthikeyan et al. ^[25] turned to the existence of a mild solution in relation to the Hilfer differential systems by using almost sectorial operators.

Due to the numerous applications of neutral differential equations in fields including electronics, chemical kinetics, biological modelling and fluid dynamics, this form of equation has attracted a lot of interest recently. We cite the publications ^[26,27,28] and the references therein for the theory and applications of neutral partial differential equations with non-local and classical circumstances. Due to the fact that neutral structures are prevalent in several areas of applied mathematics, recent years have seen an increase in interest in them.

According to what we already know, the condition of controllability is an essential qualitative and quantitative property of the control construction, and its characteristics are important in a range of control challenges for both restricted and limitless networks. Recently, this notion has sparked a lot of interest from researchers in the area of controllability of a wave equation of fractional order. See ^[29] for significant new findings on the exact and approximate controllability of nonlinear delay or non-delay dynamical systems. The approximate controllability of Atangana-Baleanu fractional neutral delay integro-differential stochastic systems with non-local conditions was established by Ma et al. ^[30] by using the fixed-point approach. A novel approach for such controllability of Sobolev-type Hilfer fractional (HF) differential equations was recently unveiled by Pandey et al. ^[31] in 2023.

In contrast to deterministic models, stochastic ones should be investigated since both natural and artificial systems are prone to noise and uncontrolled perturbations. Differential equations with stochastic components contain unpredictability in their mathematical depiction of a specific event. Recently, much attention has been paid to the application of stochastic differential equations (SDEs) to describe a variety of occurrences in population motion, science, technological engineering, environment, neuroscience, biological science and several other domains of science and technology. Infinite and finite dimensions can both be employed with SDEs. An overview of SDEs and their applications may be found in ^[32,33].

Numerous physical phenomena, like fluid movement through fractured rocks and thermodynamics, have mathematical structures that often reveal the Sobolev differential system. The debate about the approximate controllability of Hilfer neutral fractional stochastic differential inclusions of the Sobolev type was presented and developed by Dineshkumar et al. ^[32] in 2022. Also, a study on the existence of mild solutions has been carried out for the Hilfer neutral fractional SDE by Sivasankar and Udhayakumar ^[34] with the help of almost sectorial operators with a delay. Nevertheless, as far as we are aware, the literature does not describe any research on the topic of the approximate controllability of Hilfer neutral fractional stochastic differential systems of the Sobolev type under the condition of an almost sectorial operator with delay.

By taking inspiration from previous research, this study intends to address this gap. In other words, the aim of this manuscript is to establish the approximate controllability of Hilfer neutral fractional stochastic differential systems of the Sobolev type by using an almost sectorial operator with the delay in the following form:

where $D^{\mathfrak{r}, \mathfrak{s}}_{0^+}$ represents the HF derivative of order $\mathfrak{r}\in (0, 1)$ and of type $\mathfrak{s}\in [0, 1]$. The state parameter $\mathtt{u}(\cdot)$ takes values in a real separable Hilbert space ${\bf{Z}}$. Moreover, $\varkappa(\cdot)\in L^2_{\mathfrak{F}}(\mathfrak{I}, \mathcal{U})$ is the control parameter ($\mathcal{U}$ is a real Hilbert space) and ${\bf{Y}}\in L(\mathcal{U}, {\bf{Z}})$ is bounded. Let $\mathfrak{I}: = [0, \mathrm{c}]$ and $\mathfrak{I}': = (0, \mathrm{c}]$. Let ${\bf{A}}: D({\bf{A}})\subset {\bf{Z}}\rightarrow {\bf{Z}}$ be the almost sectorial operator that denotes a strongly continuous semigroup $\{T(\mathfrak{l})\}_{\mathfrak{l}\geq 0}$ on ${\bf{Z}}$ that is uniformly bounded in ${\bf{Z}}$. The function $\mathtt{u}_{\mathfrak{l}}:(-\infty, 0]\rightarrow {\bf{Z}}$ is given by $\mathtt{u}_{\mathfrak{l}} = \mathtt{u}(\mathfrak{l}+\theta), \ \theta\in (-\infty, 0]$. Note that $\mathtt{u}_{\mathfrak{l}}\in \mathcal{B}$, and it is defined axiomatically. The functions $\aleph, \ \mathcal{G}$ and multi-function ${\bf{H}}$ will be subject to satisfying some suitable criteria to be defined in the sequel.

The following describes the manuscript's structure: We give the theoretical principles in relation to fractional calculus that are relevant to our investigation in Section 2. We focus on the approximate controllability of the Hilfer neutral fractional stochastic differential system (1.1) in Section 3. To help our discussion be as applicable as possible, we offer the theoretical application in Section 4.1.

2.   Preliminaries

The complete probability space $(\Lambda, \mathfrak{F}, {\bf{P}})$ is introduced by a complete family of right-continuous, non-decreasing sub-$\sigma$-algebras $\{\mathfrak{F}_{\mathfrak{l}}\}_{\mathfrak{l}\in \mathfrak{I}}$ fulfilling the condition that $\mathfrak{F}_{\mathfrak{l}}\in \mathfrak{F}$. We denote a collection of all strongly measurable, mean square-integrable ${\bf{Z}}$-valued random parameters by

which is a Banach space associated with the norm $\|\mathtt{u}(\cdot)\|_{L_2(\Lambda, {\bf{Z}})} = (E\|\mathtt{u}(\cdot, W)\|^2_{{\bf{Z}}})^{\frac{1}{2}}$, where $E$ denotes the expectation satisfying that $E(\mathtt{u}) = \int_{\Lambda}\mathtt{u}(W)d{\bf{P}}$.

Take a real-valued sequence $\{W_n(\mathfrak{l}), \ \mathfrak{l}\geq 0, \ n\in \mathbb{N}\}$ of one-dimensional standard Wiener processes, which are mutually independent in $\Lambda$. Let $\mathbb{K}$ be a real distinct Hilbert space, and define

so that $\{\beta_n\geq 0, \ n\in \mathbb{N}\}$ and $\{\delta_n, \ n\in \mathbb{N}\}$ is a complete orthonormal basis of $\mathbb{K}$. Moreover, take $Q\in L(\mathbb{K}, \mathbb{K})$ as an operator formulated by $Q\delta_n = \beta_n\delta_n, \ (n\in \mathbb{N})$, along with the finite trace $Tr(Q) = \sum_{n = 1}^{\infty}\beta_n(<\infty)$. Let $\psi\in L(\mathbb{K}, {\bf{Z}})$ and set

If $\|\psi\|_{L_2^0} < \infty$, in this case, $\psi$ will be called the $Q$-Hilbert-Schmidt operator. Here, $L_2^0(\mathbb{K}, {\bf{Z}})$ is the space of all Hilbert-Schmidt operators endowed with the norm $\|\psi\|^2_{L_2^0} = \langle \psi, \psi\rangle$. Clearly, $L_2^0(\mathbb{K}, {\bf{Z}})$ is a real separable Hilbert space. Furthermore, $D({\bf{A}}^{\gamma})$ is dense in ${\bf{Z}}$.

Some important properties of ${\bf{A}}^{\gamma}$ are listed below.

Theorem 2.1. ^[35]

(1) Let $0 < \gamma\leq 1$. ${\bf{Z}}_{\gamma} = D({\bf{A}}^{\gamma})$ is a Banach space with $\|\mathtt{u}\|_{\gamma} = \|{\bf{A}}^{\gamma} \mathtt{u}\|, \, (\mathtt{u}\in {\bf{Z}}_{\gamma})$.

(2) Let $0 < \kappa < \gamma\leq 1$. $D({\bf{A}}^{\gamma})\rightarrow D({\bf{A}}^{\kappa})$ is compact whenever ${\bf{A}}$ is compact.

(3) $\forall\, \gamma\in (0, 1]$ and $\exists$ $C_{\gamma} > 0$ such that

The linear operators ${\bf{A}}, \mathcal{F}:D({\bf{A}})\subset {\bf{Z}}\rightarrow {\bf{Z}}$ are identified now based on the following criteria ^[36]:

$(A1)$ $\mathcal{F}$ is bijective and $D(\mathcal{F})\subset D({\bf{A}})$.

$(A2)$ ${\bf{A}}$ and $\mathcal{F}$ are closed.

$(A3)$ $\mathcal{F}^{-1}:{\bf{Z}}\rightarrow D(\mathcal{F})$ is continuous.

Additionally, for $(A1)$ and $(A2)$, $\mathcal{F}^{-1}$ is closed. Also, by $(A3)$, along with the closed graph theorem, ${\bf{A}}\mathcal{F}^{-1}:{\bf{Z}}\rightarrow {\bf{Z}}$ is bounded. Set $\|\mathcal{F}^{-1}\| = \mathcal{F}_1$ and $\|\mathcal{F}\| = \mathcal{F}_2$.

Definition 2.2. ^[37] The RL-fractional integral of order $\mathfrak{r}$ for $\hbar:[\mathrm{c}, \infty)\rightarrow \mathbb{R}$ is defined as

Definition 2.3. ^[37] The RL-fractional derivative of order $\mathfrak{r}\in [m-1, m), \ m\in \mathbb{Z}$ for $\hbar:[\mathrm{c}, \infty)\rightarrow \mathbb{R}$ is

Definition 2.4. ^[37] The Caputo derivative of order $\mathfrak{r}\in [m-1, m), \ m\in \mathbb{Z}$ for $\hbar:[c, \infty)\rightarrow \mathbb{R}$ is defined as

Definition 2.5. ^[37] The HF derivative of order $0 < \mathfrak{r} < 1$ and $0\leq \mathfrak{s}\leq 1$ for $\hbar:[\mathrm{c}, \infty)\rightarrow \mathbb{R}$ is given by

Remark 2.6. (1) If $\mathfrak{s} = 0, \ 0 < \mathfrak{r} < 1$ and $c = 0$, then the HF derivative corresponds to the classical RL-fractional derivative:

(2) If $\mathfrak{s} = 1, \ 0 < \mathfrak{r} < 1$ and $c = 0$, then the HF derivative is equal to the classical Caputo fractional derivative:

The abstract phase space $\mathcal{B}_{{\bf{p}}}$ is now described. Consider ${\bf{p}}:(-\infty, 0]\rightarrow (0, +\infty)$ to be continuous, along with $\ell = \int_{-\infty}^0{\bf{p}}(\mathfrak{l})d\mathfrak{l} < +\infty$. Now, for every $n > 0$, we have

For $\mathcal{B}_{{\bf{p}}}$, we consider

Therefore, $(\mathcal{B}_{{\bf{p}}}, \|\cdot\|)$ is a Banach space.

We assume that the space of all continuous ${\bf{Z}}$-valued stochastic processes $\{\mathfrak{z}(\mathfrak{l}), \ \mathfrak{l}\in (-\infty, \mathrm{c}]\}$ is $C((-\infty, \mathrm{c}], {\bf{Z}})$ and

Moreover, set the seminorm $\|\cdot\|_\mathrm{c}$ in $\mathcal{B}_{{\bf{p}}}'$ as

Lemma 2.7. ^[21] Let $\mathtt{u}\in \mathcal{B}_{{\bf{p}}}'$. Then, $\forall\, \mathfrak{l}\in \mathfrak{I}, \ \mathtt{u}_\mathfrak{l}\in \mathcal{B}_{{\bf{p}}}$ and

so that $\ell = \int_{-\infty}^0{\bf{p}}(\mathfrak{l})d\mathfrak{l} < +\infty$.

Definition 2.8. ^[24,38] Let $0 < \vartheta < 1$ and $0 < \varpi < \dfrac{\pi}{2}$. We denote $\Xi_{\varpi}^{-\vartheta}({\bf{Z}})$ as a family of the closed linear operators ${\bf{A}}:D({\bf{A}})\subset {\bf{Z}}\rightarrow {\bf{Z}}$ such that

(1) $\sigma({\bf{A}})\subset {\bf{S}}_{\varpi} = \{\nu\in \mathbb{C}\backslash\{0\}:|\arg \nu|\leq \varpi\}\cup\{0\}$, and

(2) $\forall$ $\mu\in (\varpi, \pi)$, $\exists$ $C_{\mu}$ such that

where $R(\nu; {\bf{A}}) = (\nu{\bf{A}}-{\mathcal I})^{-1}, \ \nu\in \varrho({\bf{A}})$ is the resolvent operator of ${\bf{A}}$. If the linear operator ${\bf{A}}$ is in the range $\Xi_{\varpi}^{-\vartheta}({\bf{Z}})$, it will be referred to as an almost sectorial operator on ${\bf{Z}}$.

Definition 2.9. ^[39] The Wright function $\mathcal{W}_\mathfrak{r}(\beta)$ is specified by the formula

with the following characteristic:

Proposition 2.10. ^[38] Let $\mathcal{O}(\mathfrak{l})$ be the compact semigroup and ${\bf{A}}\in \Xi_{\varpi}^{-\vartheta}$, where $0 < \varpi < \frac{\pi}{2}$ and $0 < \vartheta < 1$. Then,

(1) $\mathcal{O}(\mathfrak{l})$ is analytic and $\dfrac{d^n}{d\mathfrak{l}^n}T(\mathfrak{l}) = (-{\bf{A}})^n\mathcal{O}(\mathfrak{l}), \ \mathfrak{l}\in {\bf{S}}_{\frac{\pi}{2}-\varpi};$

(2) $\mathcal{O}(\mathfrak{l}+\mathfrak{e}) = \mathcal{O}(\mathfrak{l})\mathcal{O}(\mathfrak{e})$ for all $\mathfrak{e}, \mathfrak{l}\in {\bf{S}}_{\frac{\pi}{2}-\varpi};$

(3) $\|\mathcal{O}(\mathfrak{l})\|_{L({\bf{Z}})}\leq \mathcal{S}_0 \mathfrak{l}^{\vartheta-1}$, $\mathfrak{l} > 0,$ where $\mathcal{S}_0 > 0$ is a constant;

(4) $\Sigma_\mathcal{O} = \{\mathtt{u}\in {\bf{Z}}:\lim_{\mathfrak{l}\rightarrow 0^+}\mathcal{O}(\mathfrak{l})\mathtt{u} = \mathtt{u}\}$ gives $D({\bf{A}}^{\gamma})\subset\Sigma_\mathcal{O}$ whenever $\gamma > 1+\vartheta;$

(5) $(\mu-{\bf{A}})^{-1} = \int_0^{\infty}e^{-\mu\mathfrak{e}}\mathcal{O}(\mathfrak{e})d\mathfrak{e}$, $\mu\in \mathbb{C}$, and $Re(\mu) > 0$.

Lemma 2.11. ^[39] For any fixed $\mathfrak{l} > 0$, $\mathcal{O}_{\mathfrak{r}}(\mathfrak{l}), \ \mathcal{N}_{\mathfrak{r}}(\mathfrak{l})$ and $\mathcal{M}_{\mathfrak{r}, \mathfrak{s}}(\mathfrak{l})$ are linear operators and $\forall$ $\mathtt{u}\in {\bf{Z}}$,

where

Proposition 2.12. ^[34] Let $\mathfrak{r}\in (0, 1), \ \gamma\in (0, 1]$ and $\mathtt{u}\in D({\bf{A}})$; then, some $\mathcal{S}_{\gamma} > 0$ exists such that

Definition 2.13. An $\mathfrak{F}_{\mathfrak{l}}$-adopted and measurable stochastic process $\{\mathtt{u}(\mathfrak{l})\}_{\mathfrak{l}\in \mathfrak{I}'}$ is named as a mild solution of the system (1.1) if $\mathtt{u}(0) = \xi\in L_2^0(\Lambda, {\bf{Z}})$ and $\varkappa(\cdot)\in L^2_{\mathfrak{F}}(\mathfrak{I}, \mathcal{U})$; also $\forall$ $\mathfrak{e} \in [0, \mathrm{c})$, the function ${\bf{A}}\mathcal{N}_{\mathfrak{r}}(\mathfrak{l}-\mathfrak{e})\aleph(\mathfrak{e}, \mathtt{u}_{\mathfrak{e}})$ is integrable and

Since $\mathcal{N}_{\mathfrak{r}}(\mathfrak{l}) = \mathfrak{l}^{\mathfrak{r}-1}\mathcal{O}_{\mathfrak{r}}(\mathfrak{l})$, then (2.2) is equivalent to

where $\mathcal{M}_{\mathfrak{r}, \mathfrak{s}}(\mathfrak{l}) = I_{0^+}^{\mathfrak{s}(1-\mathfrak{r})}\mathcal{N}_{\mathfrak{r}}(\mathfrak{l}),$ and accordingly,

We introduce the state value of (1.1) at the end time $\mathrm{c}$ related to the control $\varkappa$ and the actual value $\xi$ by $\mathtt{u}_{\mathrm{c}}(\mathtt{u}_0;\varkappa)$. Set

which is the admissible set of system (1.1) at the end time $\mathrm{c}$. Note that $\overline{R(\mathrm{c}, \xi)}$ stands for the closure of $R(\mathrm{c}, \xi)$ in ${\bf{Z}}$.

Definition 2.14. ^[30] The Hilfer neutral fractional stochastic differential system of the Sobolev type (1.1) is approximately controllable on $\mathfrak{I}$ if $\overline{R(\mathrm{c}, \xi)} = {\bf{Z}}$.

To conduct an analysis of the approximate controllability of the supposed nonlinear Sobolev-type Hilfer control system (1.1), in the first step, we should establish the property of the approximate controllability in the linear case, that is,

To do this, we first need to introduce the pertinent operator

and the set

In the aforementioned notions, $\mathcal{O}^*_{\mathfrak{r}}(\mathfrak{l})$ and ${\bf{Y}}^*$ represent the adjoints of $\mathcal{O}_{\mathfrak{r}}(\mathfrak{l})$ and ${\bf{Y}}$, respectively. It is notable that the linear operator $\Gamma_0^{\mathrm{c}}$ is easily proven to be bounded. Consider the following hypothesis:

$(H_{\alpha})$ $\alpha R(\alpha, \Gamma_0^{\mathrm{c}})\rightarrow 0$ as $\alpha\rightarrow 0^+$ w.r.t. the strong operator topology.

Based on (Theorem 2^[14]), the linear Sobolev-type Hilfer control system (2.4) is approximately controllable on $[0, \mathrm{c}]$, which is close to the hypothesis $(H_{\alpha})$.

Lemma 2.15. ^[40,41] Let $P_{cv, cl, bd}({\bf{Z}})$ be the collection of nonempty bounded closed and convex sets in ${\bf{Z}}$ and $\mathfrak{I}$ be a compact real interval. Consider the $L^2$-Caratheodory multi-valued function

which is nonempty. Moreover, let $\Sigma$ be a linear continuous function that maps $L^2(\mathfrak{I}, {\bf{Z}})$ to $\complement$. Then,

is a closed graph operator in $\complement\times \complement$.

3.   Approximate controllability

Here, the property of approximate controllability is studied in relation to the given nonlinear Sobolev type Hilfer stochastic control system (1.1).

The following hypotheses are required to prove the main theorems.

$(H_{\mathcal{O}})$ $\mathcal{O}(\mathfrak{l})$ is compact for every $\mathfrak{l}\geq 0$.

$(H_{\aleph})$ The function $\aleph:\mathfrak{I}\times \mathcal{B}_{{\bf{p}}}\rightarrow {\bf{Z}}$ is continuous and $\exists$ $0 < \gamma < 1$ such that $\aleph\in D({\bf{A}}^{\gamma})$. For any $\mathtt{u}\in {\bf{Z}}$ and $\mathfrak{l}\in \mathfrak{I}$, ${\bf{A}}^{\gamma}\aleph(\cdot, \mathtt{u})$ is strongly measurable. Moreover, $\exists$ $\mathcal{S}_{\aleph}, \mathcal{S}'_{\aleph} > 0$ such that $\forall\, \mathtt{u}_1, \mathtt{u}_2\in {\bf{Z}}$ and ${\bf{A}}^{\gamma}\aleph(\mathfrak{l}, \cdot)$ satisfies

Take $\|{\bf{A}}^{-\gamma}\| = \mathcal{S}$.

$(H_{\mathcal{G}})$ For the function $\mathcal{G}:\mathfrak{I}\times \mathcal{B}_{{\bf{p}}}\rightarrow {\bf{Z}}$:

$(1)$ $\mathfrak{l}\rightarrow \mathcal{G}(\mathfrak{l}, \mathtt{u})$ is measurable for any $\mathtt{u}\in \mathcal{B}_{{\bf{p}}}$,

$(2)$ $\mathtt{u}\rightarrow \mathcal{G}(\mathfrak{l}, \mathtt{u})$ is continuous for almost every $\mathfrak{l}\in \mathfrak{I}$,

$(3)$ For almost every $\mathfrak{l}\in \mathfrak{I}$ and any $\mathtt{u}\in \mathcal{B}_{{\bf{p}}}$,

where $\mathfrak{q}_1\in L^1(\mathfrak{I}, \mathbb{R}^+)$ and we have the continuous increasing function $\mathcal{S}_{\mathcal{G}}: \mathbb{R}^+\rightarrow (0, \infty)$.

$(H_{{\bf{H}}})$ For each $(\mathfrak{l}, \mathfrak{e})\in \mathfrak{I}$, an $L^2$-Caratheodory function ${\bf{H}}(\mathfrak{l}, \cdot)$ mapping from $\mathcal{B}_{{\bf{p}}}$ into $\mathscr{P}_{cl, bd, cv}L(\mathscr{K}, {\bf{Z}})$ is continuous, and for any $\mathtt{u}\in \mathcal{B}_{{\bf{p}}}$, the function ${\bf{H}}(\cdot, \mathtt{u}):\mathfrak{I}\rightarrow \mathscr{P}_{bd, cl, cv}L(\mathscr{K}, {\bf{Z}})$ is strongly measurable. An integrable function $\mathfrak{q}_2:\mathfrak{I}\rightarrow [0, \infty)$ and $\bar{\mathfrak{q}} > 0$ exist such that

Note that $\mathcal{S}_{{\bf{H}}}:[0, \infty)\rightarrow [0, \infty)$ is continuous and increasing.

$(H_{I})$ The following inequality holds:

where

and

Remark 3.1. ^[30] The following implications hold:

$(a)$ $\forall$ $\mathtt{u}\in {\bf{Z}}$, $S_{{\bf{H}}, \mathtt{u}} = \emptyset$ if $\dim {\bf{Z}} < \infty$.

$(b)$ $S_{{\bf{H}}, \mathtt{u}}$ is nonempty $\iff$ for $\eta:\mathfrak{I}\rightarrow \mathbb{R}$, we have

Note that the Hilfer stochastic control system of the Sobolev type (1.1) is approximately controllable if a continuous function $\mathtt{u}$ exists such that $\forall\, \alpha > 0$:

and

where

We first state an auxiliary lemma (it will be used later).

Lemma 3.2. ^[30] For any $\bar{\mathtt{u}}_{\mathrm{c}}\in L^2(\Gamma_{\mathrm{c}}, {\bf{Z}})$, some $\phi(\cdot)\in L^2_{\mathfrak{F}}(\Lambda; L^2(\mathfrak{I}; L_2^0))$ exists such that

Define the operator $\Psi$ mapping from $\mathcal{B}'_{{\bf{p}}}$ into $2^{\mathcal{B}'_{{\bf{p}}}}$, denoted by $\Psi\mathtt{u}$, as the set $\mathtt{y}\in \mathcal{B}'_{{\bf{p}}}$ so that

where $\hbar\in S_{{\bf{H}}, \mathtt{u}}$. We shall show that $\Delta$ admits a fixed point that is the mild solution of the Hilfer stochastic control system of the Sobolev type (1.1). Obviously, $\mathtt{u}_{\mathrm{c}} = \mathtt{u}(\mathrm{c})\in (\Delta\mathtt{u})(\mathrm{c})$, which means that $\varkappa_{\mathtt{u}}(\mathtt{u}, \mathfrak{l})$ gives (1.1) as $\mathtt{u}_0\rightarrow \mathtt{u}_{\mathrm{c}}$ in the finite time $\mathrm{c}$.

Since $\varphi\in \mathcal{B}_{{\bf{p}}}$, we introduce $\widehat{ \varphi}$ as follows:

Thus, $\widehat{\varphi}\in \mathcal{B}'_{{\bf{p}}}$. Let $\mathtt{u}(\mathfrak{l}) = \mathfrak{l}^{1-\mathfrak{s}+\mathfrak{r}\mathfrak{s}-\mathfrak{r}\vartheta}[\mathtt{v}(\mathfrak{l})+\widehat{\varphi}(\mathfrak{l})], \ -\infty < \mathfrak{l}\leq \mathrm{c}$. We consider $\mathtt{v}$ to satisfy (3.1) if and only if $\mathtt{v}$ satisfies $\mathtt{v}_0 = 0$ and

Consider $\mathcal{B}''_{{\bf{p}}} = \{\mathtt{v}\in \mathcal{B}'_{{\bf{p}}}:\mathtt{v}_0 = 0\in \mathcal{B}_{{\bf{p}}}\}$. For any $\mathtt{v}\in \mathcal{B}''_{{\bf{p}}}$, we have

Hence, $(\mathcal{B}''_{{\bf{p}}}, \|\cdot\|)$ is a Banach space. Set $\mathcal{D}_{r} = \{\mathtt{v}\in \mathcal{B}''_{{\bf{p}}}:\|\mathtt{v}\|^2_{\mathrm{c}}\leq r\}$ for some $r > 0$. Accordingly, $\mathcal{D}_{r}\subseteq \mathcal{B}''_{{\bf{p}}}$ has the uniform boundedness property. If $\mathtt{v}\in \mathcal{D}_{r}$, from Lemma 2.7, we obtain

Define $\Delta:\mathcal{B}''_{{\bf{p}}}\rightarrow 2^{\mathcal{B}''_{{\bf{p}}}}$, denoted by $\Delta\mathtt{v}$, as the set $\widehat{\mathtt{y}}\in \mathcal{B}''_{{\bf{p}}}$ such that

We begin the proofs by stating some theorems that will allow us to prove the main theorem on the approximate controllability.

Theorem 3.3. If the hypotheses $(H_{\mathcal{O}}), \ (H_{\aleph}), \ (H_{\mathcal{G}}), \ (H_{{\bf{H}}})$ and $(H_{I})$ are to be held, then the multi-valued map $\Delta:\mathcal{B}''_{{\bf{p}}}\rightarrow 2^{\mathcal{B}''_{{\bf{p}}}}$ has the complete continuity and upper semi-continuity properties with the closed and convex values.

Proof. We know that a fixed point of $\Delta$ exists if and only if a fixed point of $\Pi$ exists. We break the proof into several steps for the sake of simplicity.

Step 1: $\Delta\mathtt{v}$ is convex, $\forall$ $\mathtt{v}\in \mathcal{B}'_{{\bf{p}}}$: Indeed, when $\varphi_1, \varphi_2\in \Delta\mathtt{v}$, then $\exists$ $\hbar_1, \hbar_2\in S_{{\bf{H}}, \mathtt{u}}$ such that

for $\mathfrak{l}\in \mathfrak{I}, \ i = 1, 2.$ Let $\mu\in [0, 1]$. In this case, $\forall$ $\mathfrak{l}\in \mathfrak{I}$, we have

Since $S_{{\bf{H}}, \mathtt{u}}$ is convex, $\mu\varphi_1+(1-\mu)\varphi_2\in S_{{\bf{H}}, \mathtt{u}}$. Thus, $(\mu\varphi_1+(1-\mu)\varphi_2)\in \Delta\mathtt{v}.$

Step 2: Boundedness of $\Delta\mathtt{v}$ on the bounded sets of $\mathcal{B}''_{{\bf{p}}}$:

It is enough to prove that some $\pi > 0$ exists such that $\forall$ $\varphi\in \Delta\mathtt{v}, \ \mathtt{v}\in \mathcal{D}_{r}$, we have $\|\varphi\|_c\leq \pi$.

Subject to $\varphi\in \Delta\mathtt{v}$, there exists $\hbar\in S_{{\bf{H}}, \mathtt{u}}$ such that, for any $\mathfrak{l}\in \mathfrak{I}$, and from $(H_{\aleph}), \ (H_{\mathcal{G}}), \ (H_{{\bf{H}}})$ and $(H_{I})$, we obtain

where

Thus, for all $\varphi\in \Delta(\mathcal{D}_r)$, we have that $\|\varphi\|_{\mathrm{c}}\leq \pi$.

Step 3: $\Delta$ maps the bounded sets into equicontinuous sets of $\mathcal{B}''_{{\bf{P}}}$:

Assume that $0 < \mathfrak{l}_1 < \mathfrak{l}_2\leq \mathrm{c}$. For every $\varphi\in \Delta\mathtt{v}$ in which $\mathtt{v}$ belongs to $\mathcal{D}_r = \{\mathtt{v}\in \mathcal{B}''_{{\bf{p}}}:\|\mathtt{v}\|^2_{\mathrm{c}}\leq r\}$, there exists $\hbar\in S_{{\bf{H}}, \mathtt{u}}$ such that for any $\mathfrak{l}\in \mathfrak{I}$, we obtain

When $\mathfrak{l}_2\rightarrow \mathfrak{l}_1$, the right-hand side of the above inequality tends to $0$, because $\mathcal{O}_{\mathfrak{r}}(\mathfrak{l})$ is an operator with the strong continuity, and because the compactness of $\mathcal{O}_{\mathfrak{r}}(\mathfrak{l})$ requires uniform continuity. As a result, the set $\{\Delta\mathtt{v}:\mathtt{v}\in \mathcal{D}_r\}$ is equicontinuous. The Arzela-Ascoli theorem and Steps 2 and 3 allow us to conclude that $\Delta$ is compact.

Step 4: $\Delta$ has a closed graph:

Suppose that $\{\mathtt{v}^n\}\subset \mathcal{B}''_{{\bf{p}}}$ is a sequence such that $\mathtt{v}^n\rightarrow \mathtt{v}^*$, and assume that $\{\varphi^n\}$ is a sequence belonging to $\Delta \mathtt{v}^n$ for any $n\in \mathbb{N}$ such that $\varphi^n\rightarrow \varphi^*$. We shall demonstrate that $\varphi^*\in \Delta \mathtt{v}^*$. Since $\varphi^n\in \Delta \mathtt{v}^n$, then there exists $\hbar^n\in S_{{\bf{H}}, \mathtt{u}^n}$ such that

We must show that $\exists$ $\hbar^*\in S_{{\bf{H}}, \mathtt{u}^*}$ such that

Now, $\forall$ $\mathfrak{l}\in \mathfrak{I}$, since $\mathcal{G}$ is continuous, we have

Consider the linear continuous operator $\mho:L^2(\mathfrak{I}; {\bf{Z}})\rightarrow C(\mathfrak{I}; {\bf{Z}})$ by

Accordingly, by referring to Lemma 2.15, $\mho\circ S_{\hbar}$ is a closed graph. Moreover,

Since $\mathtt{v}^n\rightarrow \mathtt{v}^*$, because of Lemma 2.15, we may write

Thus, $\Delta$ has a closed graph.

In view of the four previous steps, $\Delta$ is a completely continuous multi-valued map with upper semi-continuity and closed values that are convex. □

Now, in order to use the Martelli fixed-point theorem, we choose a parameter $\eta > 1$ and establish the following auxiliary problem:

Thus, by Definition 2.13, the mild solution of (3.2) can be defined in the following form:

where $\hbar\in S_{{\bf{H}}, \mathtt{u}} = \{\hbar\in L^2(L(\mathbb{K}, {\bf{Z}})):\hbar(\mathfrak{l})\in {\bf{H}}(\mathfrak{e}, \mathtt{u}_{\mathfrak{e}})\ {\text{for}}\ \mathfrak{l}\in \mathfrak{I}\}$.

Now, in this lemma, we can be sure of the above structure in relation to the mild solution of (3.2).

Lemma 3.4. Assume that $(H_{\mathcal{O}}), \ (H_{\aleph}), \ (H_{\mathcal{G}}), \ (H_{{\bf{H}}})$ and $(H_{I})$ are satisfied. Then, $\mathtt{u}$ is a mild solution of (3.2). Moreover, the priori bound $\epsilon > 0$ exists such that $\|\mathtt{u}_{\mathfrak{l}}\|_{\mathcal{B}_{{\bf{p}}}}\leq \epsilon$, $\forall\, \mathfrak{l}\in \mathfrak{I}$, where $\epsilon$ is only dependent on $\mathrm{c}$, $\mathfrak{q}_1(\cdot), \ \mathfrak{q}_2(\cdot), \ \mathcal{S}_{\mathcal{G}}$ and $\mathcal{S}_{{\bf{H}}}$.

Proof. From the structure (3.3), we may write

Therefore, by Lemma 2.7, we get

Assume that $\upsilon(\mathfrak{l}) = \sup\{\mathfrak{l}^{2(1-\mathfrak{s}+\mathfrak{r}\mathfrak{s}-\mathfrak{r}\vartheta)}\|\mathtt{u}_{\mathfrak{e}}\|^2_{\mathcal{B}_{{\bf{p}}}}:0\leq \mathfrak{e}\leq \mathfrak{l}\}$. Furthermore, the function $\upsilon(\mathfrak{l})\in \mathfrak{I}$ is non-decreasing, and we have

We can conclude from the right-hand side of the above inequality that

and

where

These inequalities imply, for each $\mathfrak{l}\in \mathfrak{I}$, that

where $\mu(0) = \kappa$ and $\beta(\mathfrak{e}) = (\ell(\mu(\mathfrak{l}))^{\frac{1}{2}}+\|\xi\|_{\mathcal{B}_{{\bf{p}}}})^2$.

Hence, $\mu(\mathfrak{l}) < \infty$ and there exists a constant $d$ such that $\mu(\mathfrak{l})\leq d$ for all $\mathfrak{l}\in [0, \mathrm{c}]$. Thus, we have that $\|\mathtt{u}_{\mathfrak{l}}\|^2_{\mathcal{B}_{{\bf{p}}}}\leq \upsilon(\mathfrak{l})\leq \mu(\mathfrak{l})\leq d, \forall\, \mathfrak{l}\in \mathfrak{I}$, where $d$ is only dependent on $\mathrm{c}$ and the functions $\mathfrak{q}_1(\cdot), \ \mathfrak{q}_2(\cdot), \ \mathcal{S}_{\mathcal{G}}(\cdot)$ and $\mathcal{S}_{{\bf{H}}}(\cdot)$. This ends the proof. □

Theorem 3.5. If $(H_{\mathcal{O}}), \ (H_{\aleph}), \ (H_{\mathcal{G}}), \ (H_{{\bf{H}}})$ and $(H_{I})$ hold, then the Hilfer stochastic control system of the Sobolev type (1.1) admits at least one mild solution on $(-\infty, \mathrm{c}]$.

Proof. Let $\Phi = \{\mathtt{v}\in \mathcal{B}''_{{\bf{p}}}:\eta\mathtt{v}\in \Lambda\mathtt{v}, \ {\text{for some}}\ \eta > 1\}$. Then, for all $\mathtt{v}\in \Phi$, we have

Then, the function $\mathtt{u} = \mathtt{v}+\widehat{\varphi}$ will be a mild solution of the system (3.3); thus, by Lemmas 3.4 and 2.7, we estimate the following:

which gives the boundedness of $\Phi$.

Therefore, it gives, by Lemma 2.7 and the Martelli fixed-point theorem, that $\Lambda$ admits a fixed point $\mathtt{v}^*\in \mathcal{B}''_{{\bf{p}}}$. Set $\mathtt{u}(\mathfrak{l}) = \mathtt{v}^*+\widehat{\varphi}(\mathfrak{l}), \ \mathfrak{l}\in [0, \mathrm{c}]$. Then, $\mathtt{u}$ is a fixed point of $\Psi$, which is a mild solution of the Hilfer stochastic control system of the Sobolev type (1.1). □

By considering the previous theorems, we can now prove the approximate controllability for the main given stochastic system.

Theorem 3.6. If the hypotheses $(H_{\mathcal{O}}), \ (H_{\aleph}), \ (H_{\mathcal{G}}), \ (H_{{\bf{H}}})$ and $(H_{I})$ are satisfied and $\mathcal{G}$ and ${\bf{H}}$ have the uniform boundedness property, then the Hilfer stochastic control system of the Sobolev type (1.1) is approximately controllable on $\mathfrak{I}$.

Proof. Let $\mathtt{u}^{\alpha}(\cdot)\in \mathcal{D}_r$ be a fixed point of the operator $\Pi$. But, based on Theorem 3.3, we know that every fixed point of $\Pi$ is a mild solution of the Hilfer stochastic control system of the Sobolev type (1.1). This shows that there is a $\mathtt{u}^{\alpha}$ such that $\mathtt{u}^{\alpha}\in \Pi(\mathtt{u}^{\alpha})$; that is, by the stochastic Fubini theorem, $\exists$ $\hbar^{\alpha}\in S_{\mathcal{G}, \mathtt{u}^{\alpha}}$ so that

Moreover, using the Dunford-Pettis theorem and the existing conditions on $\aleph, \ \mathcal{G}$ and $\hbar$, we find that $\aleph(\mathrm{c}, \mathtt{u}_{\mathrm{c}}), \ \mathcal{G}(\mathfrak{e}, \mathtt{u}_{\mathrm{c}})$ and $\hbar(\omega, \mathtt{u}_{\omega})$ are weakly compact, respectively, in $L^2(\mathfrak{I}, {\bf{Z}})$, $L^2(\mathfrak{I}, {\bf{Z}})$, and $L^2(L_Q(\mathbb{K}, {\bf{Z}}))$. So, there are subsequences, denoted by $\aleph(\mathrm{c}, \mathtt{u}_{\mathrm{c}}), \ \mathcal{G}(\mathfrak{e}, \mathtt{u}_{\mathrm{c}})$ and $\hbar(\omega, \mathtt{u}_{\omega})$, weakly converging to $\aleph, \ \mathcal{G}$ and $\hbar$, respectively, in $L^2(\mathfrak{I}, {\bf{Z}}), \ L^2(\mathfrak{I}, {\bf{Z}})$ and $L^2(L_Q(\mathbb{K}, {\bf{Z}}))$. Now, we write

From $(H_{\alpha})$, for each $0\leq \mathfrak{e}\leq \mathrm{c}$, we get that $\alpha(\alpha I+\Gamma_0^{\mathrm{c}})^{-1}\rightarrow 0$ strongly as $\alpha\rightarrow 0^+$. Accordingly, $\alpha(\alpha I+\Gamma_0^{\mathrm{c}})^{-1}\leq 1$. Consequently, we have that $E\|\mathtt{u}^{\alpha}(\mathrm{c})-\bar{\mathtt{u}}_{\mathrm{c}}\|^2\rightarrow 0$ as $\alpha\rightarrow 0^+$ from Lebesgue's dominated convergence theorem and the compactness of $\mathcal{O}_{\mathfrak{r}}(\mathfrak{l})$. Hence, the Hilfer stochastic control system of the Sobolev type (1.1) is approximately controllable which completes the proof. □

4.   Example

4.1.   Example Ⅰ

Here, we simulate the given Hilfer stochastic control system of the Sobolev-type (1.1) by defining some operators.

Let $\mathcal{U} = L^2[0, \pi]$ and ${\bf{Y}}:D({\bf{Y}})\subset \mathcal{U}\rightarrow \mathcal{U}$ be an operator defined as

so that

Suppose that ${\bf{A}}:D({\bf{A}})\subset {\bf{Z}}\rightarrow {\bf{Z}}$ and $\mathcal{F}:D(\mathcal{F})\subset {\bf{Z}}\rightarrow {\bf{Z}}$ are two operators respectively given by ${\bf{A}}\mathtt{z} = \mathtt{z}''$ and $\mathcal{F}\mathtt{z} = \mathtt{z}-\mathtt{z}''$, in which, accordingly,

Moreover, ${\bf{A}}$ and $\mathcal{F}$ respectively take the following forms:

where $\varkappa_n(\mathtt{y}) = \sqrt{\frac{2}{\pi}}\sin(n\mathtt{y}), \ n = 1, 2, 3, \cdots$ denotes the orthonormal vectors of ${\bf{A}}$. Additionally, for $\mathtt{u}\in {\bf{Z}}$, we have

and

Note that ${\bf{Y}}$ admits the eigenvalues $\beta_n = -n^2, \ n\in \mathbb{N}$, and that the corresponding eigenfunction is given by $\varkappa_n$. Therefore, the spectral representation of ${\bf{Y}}$ is formulated by

Further, define

Specify that

where $\widehat{\mathcal{U}}$ is a space with the infinite dimension under the norm

In this step, we can define ${\bf{Y}}:\widehat{\mathcal{U}}\rightarrow \mathcal{U}$ as

so that ${\bf{Y}}$ is a linear continuous map.

Now, by the above definitions, consider the following Hilfer stochastic control system of the Sobolev type as follows:

where $W(\mathfrak{l})$ is the standard one-dimensional Brownian motion in ${\bf{Z}}$ belonging to the filtered probability space $(\Lambda, \mathfrak{F}, {\bf{P}})$. Obviously, all assumptions $(H_{\mathcal{O}}), \ (H_{\aleph})$, $(H_{\mathcal{G}}), \ (H_{{\bf{H}}})$ and $(H_{I})$ hold; thus, the above Hilfer stochastic control system of the Sobolev type (4.1) is approximately controllable based on Theorem 3.6.

4.2.   Example Ⅱ

In this part, we examine the approximate controllability of Hilfer neutral fractional stochastic differential systems of the Sobolev type by using an almost sectorial operator with delay. Consider the mild solution of the system (2.3):

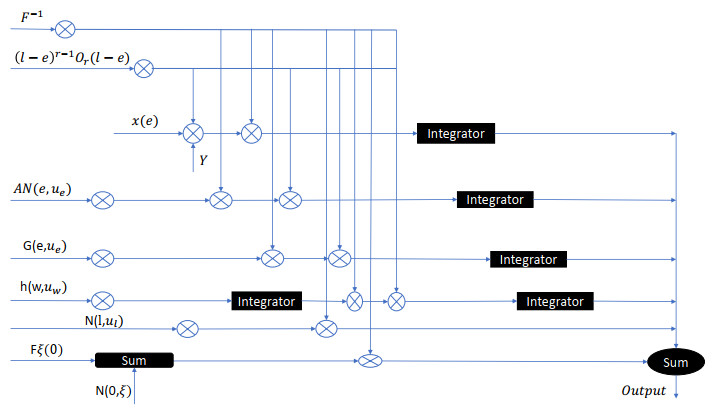
Motivated by the filter system presented in ^[22,42,43], we present the digital filter system corresponding to the mild solution in Figure 1. Digital filters are the backbone for any signal processing applications. Many biomedical signals related to the human body are currently being acquired for various informative feature extractions. Most of the aforementioned signals generally possess a low frequency by nature. These signals describe the information pertaining to various disorders or diseases for which the accuracy is of high concern. The efficiency of any digital signal-processing filtering system relies on the ability to reject the noise.

Figure 1 describes the following:

(1) The product modulator 1 accepts the input $[\mathcal{F}\xi(0)-\aleph(0, \xi)]$, and $\mathcal{M}_{\mathfrak{r}, \mathfrak{s}}$ at time $\mathfrak{l} = 0$ produces the output $\mathcal{M}_{\mathfrak{r}, \mathfrak{s}}(\mathfrak{l})[\mathcal{F}\xi(0)-\aleph(0, \xi)]$.

(2) The product modulator 2 accepts the input $\aleph(\mathfrak{l})$, produces the output $\aleph(\mathfrak{l}, \mathtt{u}_{\mathfrak{l}})$.

(3) The product modulator 3 accepts the input $\varkappa(\mathfrak{e})$ and ${\bf{Y}}$ and produces the output ${\bf{Y}}\varkappa(\mathfrak{e})$.

(4) The product modulator 4 accepts the input $\mathtt{u}(\mathfrak{e})$ and $\aleph$ and gives output $\aleph(\mathfrak{e}, \mathtt{u}_{\mathfrak{e}})$.

(5) The product modulator 5 accepts the input $\mathtt{u}(\mathfrak{e})$ and $\mathcal{G}$ and gives output $\mathcal{G}(\mathfrak{e}, \mathtt{u}_{\mathfrak{e}})$.

(6) The product modulator 6 accepts the input $\mathtt{u}(\omega)$ and $\hbar$ and gives output $\hbar(\omega, \mathtt{u}_{\omega})$.

(7) The integrator performs the integral of

over the period $\xi$.

Furthermore,

(1) Inputs $\mathcal{F}^{-1}(\mathfrak{l}-\mathfrak{e})^{\mathfrak{r}-1}\mathcal{O}_{\mathfrak{r}}(\mathfrak{l}-\mathfrak{e})$ and ${\bf{A}}\aleph(\mathfrak{e}, \mathtt{u}_{\mathfrak{e}})$ are combined and multiplied with an output of the integrator over $(0, \mathfrak{e})$.

(2) Inputs $\mathcal{F}^{-1}(\mathfrak{l}-\mathfrak{e})^{\mathfrak{r}-1}\mathcal{O}_{\mathfrak{r}}(\mathfrak{l}-\mathfrak{e})$ and $\mathcal{G}(\mathfrak{e}, \mathtt{u}_{\mathfrak{e}})$ are combined and multiplied with an output of the integrator over $(0, \mathfrak{e})$.

(3) Inputs $\mathcal{F}^{-1}(\mathfrak{l}-\mathfrak{e})^{\mathfrak{r}-1}\mathcal{O}_{\mathfrak{r}}(\mathfrak{l}-\mathfrak{e})$ and $\int_0^{\mathfrak{e}}\hbar(\omega, \mathtt{u}_{\omega})dW(\omega)$ are combined and multiplied with an output of the integrator over $(0, \mathfrak{e})$.

(4) Inputs $\mathcal{F}^{-1}(\mathfrak{l}-\mathfrak{e})^{\mathfrak{r}-1}\mathcal{O}_{\mathfrak{r}}(\mathfrak{l}-\mathfrak{e})$ and ${\bf{Y}}\varkappa(\mathfrak{e})$ are combined and multiplied with an output of the integrator over $(0, \mathfrak{e})$.

Finally, we move all of the outputs from the integrators to the summer network. Therefore, the output of $\mathtt{u}(\mathfrak{l})$ is attained; it is bounded and controllable.

5.   Conclusions

This paper focuses on the approximate controllability of a Hilfer stochastic neutral control system of the Sobolev type by using an almost sectorial operator with delay. The concepts of stochastic analysis, fractional calculus, semigroup theory and fixed-point technique are used to find the mild solutions of the mentioned system. More precisely, by defining some operators, and under some control conditions, we could prove the existence result for the mild solutions. Finally, we provided a theoretical example and filter system to effectively analyse our results. In future works, one can extend the control Hilfer stochastic neutral systems under some well-known boundary value conditions.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to Prince Sattam bin Abdulaziz University for funding this research work through the project number (PSAU/2023/01/9010).

Conflict of interest

The authors declare no conflict of interest.