1.
Introduction
Let K be a field and S=K[x1,x2,…,xd] be the polynomial ring over K with standard grading, that is, deg(xi)=1, for all i. Let M be a finitely generated graded S-module. Suppose that M admits the following minimal free resolution:
Let pdim(M) denotes the projective dimension of M. Then
Depth is an algebraic invariant of module M denoted by depth(M) and is defined as the common length of maximal regular sequences on M in the ideal m=(x1,x2,…,xd), where m is the unique graded maximal ideal of S. If M is a finitely generated Zd graded module over the Zd graded ring S, then for a homogeneous element u∈M and a subset W⊂{x1,x2,…,xd}, uK[W] denotes the K-subspace of M generated by all homogeneous elements of the form uv, where v is a monomial in K[W]. Such a linear K-subspace uK[W] is called a Stanley space of dimension |W| if it is a free K[W]-module, where |W| denotes the number of indeterminates in W. A Stanley decomposition of M is a presentation of the K-vector space M as a finite direct sum of Stanley spaces
The Stanley depth of decomposition D is sdepth(D)=min{|Wi|:i=1,2,…,s}. The Stanley depth of M is
We refer the readers to [1] for a detailed introduction to Stanley depth. In 1982 Stanley conjectured in [2] that, if M is a finitely generated Zd-graded S-module, then sdepth(M)≥depth(M). Ichim et al. gave an algorithm for computing Stanley depth in [3]. Let I⊂J be monomial ideal of S, then Ichim et al. [4] reduced the Stanley's conjecture for the module of the type J/I to the case when I and J are square free monomial ideals. This conjecture was proved for some special classes of modules; see for instance [5,6,7]. However, this conjecture was later disproved by Duval et al. in [8]. For some other interesting results on Stanley depth we refer the readers to [9,10,11,12].
Let G=(V(G),E(G)) be a graph with vertex set V(G)={x1,x2,…,xd} and edge set E(G). All graphs considered in this paper are simple and undirected. The degree of a vertex in a graph G is the number of edges incident to that vertex. A vertex of a graph is a pendant vertex if it is of degree one. An edge ideal of a graph G is a square free monomial ideal of polynomial ring S, that is, I(G)=(xixj:{xi,xj}∈E(G)). Several papers have been written on depth and Stanley depth of S/I(G); see for instance [13,14,15]. A graph is known as a path if it is a sequence of vertices such that each vertex in the sequence is adjacent to the vertex next to it. A tree is a graph in which there is a unique path between any two vertices of it. A tree in which one vertex is selected as a root vertex and all other vertices are directed away from it, is known as a rooted tree. A vertex of tree is known as parent, if at least one edge is directed from it to another vertex of a tree. A vertex to which edge is directed from the parent is known as a child. A tree is called an h-ary tree if each of its vertex has at most h children. An h-ary tree is called a perfect h-ary tree of height t if each of its parent vertex has h children, and all non parent vertices are at distance t from the root vertex. B. Shaukat et al. [16] computed formulas for the values of depth, Stanley depth and projective dimension of residue class rings of the edge ideals of perfect h-ary trees.
In this article we define a tree which we call a {perfect [h,d]-ary tree} and a unicyclic graph closely related to the perfect [h,d]-ary tree. We compute exact values of depth, Stanley depth and projective dimension for quotient rings associated to the edge ideals of perfect [h,d]-ary trees and quotient rings associated to the edge ideals of considered unicyclic graphs, except for the one special case of unicyclic graphs in which we compute tight bounds for the Stanley depth. For the values of depth see Theorem 3.4, 3.7, 4.2 and 4.5, and for the results related to Stanley depth see Theorem 3.5, 3.8, 4.3 and 4.6.
2.
Preliminaries
Here we discuss some of the terminologies of Graph Theory. A graph is called simple if it is loopless and contains no multiple edges. Let n≥1, if Pd represents a path graph on d vertices say x1,x2,…,xd, then E(Pd)=⋃d−1i=1{{xi,xi+1}} (if d=1, then E(P1)=∅ and I(P1)=(0)). A graph denoted by Cd with edge set E(Cd)=⋃d−1i=1{{xi,xi+1}}⋃{x1,xd} is called a cycle on d vertices. The vertices x1 and x2 in a graph G are said to be fused or merged or identified if x1 and x2 are replaced by a single new vertex x, such that, every edge that was adjacent to either x1 or x2 or both, is adjacent to x. A path with end vertices xi and xj is known as xixj-path. A graph G is connected if it has xixj-path for each xi,xj∈V(G). A subgraph of a graph G is maximal connected if it is connected and is not contained in any other connected subgraph of G. A maximal connected subgraph of a graph G is called its component. For vertices xi and xj of a graph G, the length of a shortest path from xi to xj is called the distance between xi and xj denoted by dG(xi,xj). If no such path exists between xi and xj, then dG(xi,xj)=∞. As defined earlier a tree is a graph in which there is a unique path between any two vertices of it. A forest is a graph whose all components are trees. A caterpillar is a tree in which all the vertices are at distance at most one from the central path. A lobster graph is a tree in which all the vertices are at distance at most two from the central path.
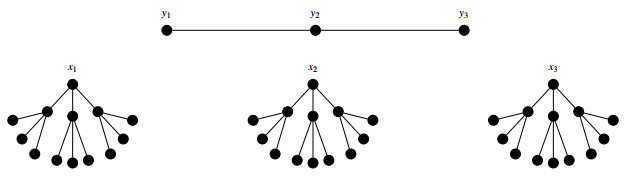
If h=1, then a perfect h-ary tree is a path, and we designate one of its pendant vertex as a root. If h≥2, then there is only one vertex of degree h in a perfect h-ary tree, and we designate that unique vertex as a root. Let h≥2, d≥1 and H1,H2,…,Hd be perfect (h−1)-ary trees such that Hi≅Hj for all i and j. Let x1,x2,…,xd be the root vertices of H1,H2,…,Hd, respectively, and y1,y2,…,yd be the vertices of the path Pd. Let H be a forest with d+1 components H1,H2,…,Hd and Pd. If we fuse the vertex xi with yi for all i∈{1,2,…,d} in H, then we get a tree which we call a perfect [h,d]-ary tree. If t is the common height of all perfect (h−1)-ary trees in H, then we denote such a perfect [h,d]-ary tree by Ph,t,d. See Figures 1 and 2 for an example of Ph,t,d. Now let d≥3. If we add an edge between the vertices x1 and xd of Ph,t,d, then we get a unicyclic graph and we denote this unicyclic graph by Ch,t,d.
See Figures 3 and 4 for examples and labeling of vertices of Ph,t,d and Ch,t,d.
Remark 2.1. Note that
(1) If h≥2, t≥1, and d=1, then Ph,t,1 is a perfect (h−1)-ary tree.
(2) If h≥2, t=1 and d≥1, then Ph,1,d belongs to the class of caterpillar trees.
(3) If h≥2, t=2 and d≥1, then Ph,2,d is a special class of lobster trees.
Now we state some results that will be used frequently throughout this article.
Lemma 2.2. Let I=I(Pd)⊆S=K[x1,x2,…,xd] be an ideal of S, then
(1) depth(S/I)=⌈d3⌉ ([17,Lemma 2.8]).
(2) sdepth(S/I)=⌈d3⌉ ([18,Lemma 4]).
Proposition 2.3. [19,Proposition 1.3 and 1.8] Let I=I(Cd)⊆S=K[x1,x2,…,xd] be an ideal of S, then depth(S/I)=⌈d−13⌉ and sdepth(S/I)≥⌈d−13⌉.
Lemma 2.4. [20,Theorem 2.6] Let h≥2, t=1 and d=1. If S=K[V(Ph,1,1)], then depth(S/I(Ph,1,1))= sdepth(S/I(Ph,1,1))=1.
Theorem 2.5. [21,Theorem 3.5 and 3.6] Let S=K[V(Ph,2,d)], h≥2, t=2 and d≥1, then depth(S/I(Ph,2,d))= sdepth(S/I(Ph,2,d))=(h−1)d.
Theorem 2.6. [21,Theorem 4.3 and 4.4] Let S=K[V(Ch,2,d)], h≥2, t=2 and d≥3, then depth(S/I(Ch,2,d))= sdepth(S/I(Ch,2,d))=(h−1)d.
Proposition 2.7. [16,Proposition 2.2] Let h≥3 and t≥1. If S=K[V(Ph,t,1)], then
Theorem 2.8. [22,Theorem 1.3.3] (Auslander–Buchsbaum formula) Let R be a commutative Noetherian local ring and M be a non-zero finitely generated R-module. If pdim(M)<∞, then
Now we give some results that play important role while proving our main results of this article.
Lemma 2.9. [23,Lemma 2.2] Let 0→R1→R2→R3→0 be a short exact sequence of finitely generated Zd-graded S−modules, then sdepth(R2)≥min{ sdepth(R1), sdepth(R3)}.
Proposition 2.10. [24,Proposition 2.7] Let J⊂S be a monomial ideal and w be a monomial such that w∉J, then sdepth(S/(J:w))≥ sdepth(S/J).
Lemma 2.11. [22,Proposition 1.2.9] (Depth Lemma) Let 0→R1→R2→R3→0 be a short exact sequence of Zd-graded S-modules, then
(1) depth(R2)≥min{depth(R1),depth(R3)}.
(2) depth(R1)≥min{depth(R2),depth(R3)+1}.
(3) depth(R3)≥min{depth(R1)−1,depth(R2)}.
Remark 2.12. Let J⊂S be a monomial ideal. Then for x∉J, the short exact sequence
implies that
(1) depth(S/J)≥min{depth(S/(J:x)),depth(S/(J,x))},
(2) sdepth(S/J)≥min{ sdepth(S/(J:x)), sdepth(S/(J,x))}.
Moreover, if depth(S/(J:x))≤depth(S/(J,x)), then by Depth Lemma depth(S/J)=depth(S/(J:x)).
The following results will be used frequently in our proofs and we will not be referring it again and again.
Lemma 2.13. [25,Lemma 3.6] Let I⊂S=K[x1,x2,…,xd] be a monomial ideal. If S′=S⊗KK[xd+1]≅S[xd+1], then depth(S′/IS′)=depth(S/I)+1 and sdepth(S′/IS′)= sdepth(S/I)+1.
Lemma 2.14. [26,Lemma 2.12 and 2.13] Let J1⊂S1=K[x1,x2,…,xt] and J2⊂S2=K[xt+1,xt+2,…,xd] be monomial ideals where 1≤t<d. If S=S1⊗KS2, then
(1) depthS(S1/J1⊗KS2/J2)=depthS(S/(J1S+J2S))=depthS1(S1/J1)+depthS2(S2/J2).
(2) sdepthS(S1/J1⊗KS2/J2)≥ sdepthS1(S1/J1)+ sdepthS2(S2/J2).
The following lemma is proved by B. Shaukat et al. [27]. Since the paper is not yet published, we present here a short proof for the sake of completeness.
Lemma 2.15. [27] Let h≥2, t=1.
(1) If d≥1 and S=K[V(Ph,1,d)], then
(2) If d≥3 and S=K[V(Ch,1,d)], then
Proof. (1) Result is proved by induction on d. If d=1, then the result follows from Lemma 2.4. Now let d≥2. We have
(if d=2, K[V(Ph,1,d−2)]/I(Ph,1,d−2)≅K) and
By Lemma 2.13 we have,
and
Then again by Lemma 2.13 and induction, we have
and
Hence by Remark 2.12 we have depth(S/I(Ph,1,d))=d+(h−2)⌈d−12⌉. Now we discuss the proof for Stanley depth. For lower bound we use the same arguments as used in the proof of depth and we use Lemma 2.9 instead of Lemma 2.11. And we compute the desired upper bound by using Proposition 2.10.
(2) The proof of this result is similar to the proof given above and it involves the use of values of depth and Stanley depth of S/I(Ph,1,d) which are computed above.
3.
Depth, Stanley depth and projective dimension of cyclic modules associated to perfect [h,d]-ary trees
In this section we compute depth, Stanley depth and projective dimension of the quotient ring K[V(Ph,t,d)]/I(Ph,t,d). We also prove that the depth and Stanley depth are equal.
Remark 3.1. In this remark we introduce some terms that appear in some special cases of our proofs when we use induction.
(1) If d=0, we define K[V(Ph,t,0)]/I(Ph,t,0):=K, hence
(2) If d≥2, we define K[V(Ph,0,d)]/I(Ph,0,d):=K[V(Pd)]/I(Pd), hence by Lemma 2.2
Remark 3.2. While doing the computations of depth and Stanley depth for the quotient ring associated to edge ideals of both perfect [h,d]-ary trees and a unicyclic graph, it was observed that the patterns of computed values were different for h=2 and h≥3. So our results are based on two cases of h, that is for h=2 and h≥3. Further, these two cases are classified on the basis of variable t.
Remark 3.3. Let I⊂S be a squarefree monomial ideal whose minimal generating set comprises of monomials of degree at most two. We associate a graph GI to an ideal I with vertex set and edge set defined as V(GI)=supp(I) and E(GI)={{xa,xb}:xaxb∈G(I)}, respectively. We know that for xi∉I, (I:xi) and (I,xi) are also squarefree monomial ideals minimally generated by monomials of degree at most two. The graphs G(I:xi) and G(I,xi) are subgraphs of graph GI. See Figure 4 for examples of G(I(P3,3,3):x(0)3) and G(I(P3,3,3),x(0)3)that are subgraphs of GI(P3,3,3). By using the structures of these subgraphs we have the following isomorphisms:
and
Theorem 3.4. Let t≥1 and d≥1. If S=K[V(P2,t,d)], then
Proof. We will prove this result by induction on d. For d=1 and d=2, K[V(P2,t,1)]/I(P2,t,1)≅K[V(Pt+1)]/I(Pt+1) and K[V(P2,t,2)]/I(P2,t,2)≅K[V(P2(t+1))]/I(P2(t+1)), respectively. Then by Lemma 2.2, depth(S/I(P2,t,1))=⌈t+13⌉ and depth(S/I(P2,t,2))=⌈2(t+1)3⌉, as desired. Now let d≥3. For t=1 the result follows from Lemma 2.15 and for t=2 the result follows from Theorem 2.5. Now let t≥3, we have the following cases:
(1) Let t≡0(mod3). We have
and
Then by induction, Lemma 2.14 and Lemma 2.2 we have
and
Since t≡0(mod3) implies ⌈t−13⌉=⌈t3⌉=(t3) and ⌈t+13⌉=⌈t3⌉+1. Thus we have depth(S/(I(P2,t,d):x(0)d−1))=dt3+⌈d3⌉ and depth(S/(I(P2,t,d),x(0)d−1))=dt3+⌈d−23⌉+1=dt3+⌈d+13⌉. Hence by Remark 2.12 depth(S/I(P2,t,d))=dt3+⌈d3⌉.
(2) Let t≡1(mod3). We have
and
By induction, Lemma 2.14 and Lemma 2.2 we have
since t≡1(mod3) implies ⌈t−13+1⌉=⌈t3⌉ so depth(S/(I(P2,t,d):x(0)d))=⌈t3⌉⋅d and
Hence by Remark 2.12 depth(S/I(P2,t,d))=⌈t3⌉⋅d.
(3) Let t≡2(mod3). We have
Now let J=(I(P2,t,d),x(1)d). We have the following isomorphisms:
and
Then by induction, Lemma 2.14 and Lemma 2.2 we have
and
Since t≡2(mod3) implies ⌈t+13⌉=⌈t3⌉, ⌈t−13+1⌉=⌈t3⌉+1 and ⌈t+13−23⌉=⌈t3⌉. So we have depth(S/(I(P2,t,d):x(1)d))=⌈t3⌉⋅d, depth(S/(J:x(0)d))=⌈t3⌉⋅d+1 and depth(S/(J,x(0)d))=⌈t3⌉⋅d. Thus by Remark 2.12 depth(S/J)≥⌈t3⌉⋅d and also J=(I(P2,t,d),x(1)d) implies depth(S/(I(P2,t,d),x(1)d))≥⌈t3⌉⋅d. Hence again by Remark 2.12 depth(S/I(P2,t,d))=⌈t3⌉⋅d.
Theorem 3.5. Let t≥1 and d≥1. If S=K[V(P2,t,d)], then
Proof. For d=1,2 result holds and proof is similar to as done in Theorem 3.4 by using Lemma 2.2. Now let d≥3. With similar arguments as in Theorem 3.4 and using Lemma 2.14 and Remark 2.12 we get the lower bounds
Now we will compute upper bound by induction on t. If t=1, by Lemma 2.15 we have sdepth(S/I(P2,t,d))=d, as desired. For t=2, by Theorem 2.5 sdepth(S/I(P2,t,d))=d satisfies the result. Now let t≥3 and u be a monomial such that u:=x(t−1)1x(t−1)2…x(t−1)d. We have the following isomorphism:
S/(I(P2,t,d):u)≅K[V(P2,t−3,d)]/I(P2,t−3,d)⊗KK[x(t−1)1,x(t−1)2,…,x(t−1)d], then we have
By Proposition 2.10 we have sdepth(S/I(P2,t,d))≤ sdepth(S/(I(P2,t,d):u)).
(1) Let t≡0(mod3). Since t−3≡0(mod3), then from Eq (3.1) and by induction on t we have
(2) Let t≡1,2(mod3). Since t−3≡1,2(mod3), then from Eq (3.1) and by induction on t we have
Corollary 3.6. Let h=2, t≥1 and d≥1. If S=K[V(P2,t,d)], then
Proof. We have |V(P2,t,d)|=d(t+1), therefore, depth(S)=d(t+1). Hence we get the required result by using Theorem 3.4 and Theorem 2.8.
Theorem 3.7. Let h≥3, t≥1 and d≥1. If S=K[V(Ph,t,d)], then
Proof. We will prove this result by induction on d. If d=1, the result follows from Proposition 2.7. Now let d≥2. For t=1 the result follows from Lemma 2.15 and for t=2 the result follows from Theorem 2.5. Now let t≥3, we consider the following three cases:
(1) Let t≡0(mod3). We consider two subcases
(i) d=2.
(ii) d≥3.
If d=2, then we have the following isomorphisms:
and
Now if d≥3, then we have
and
First we prove the result for subcase (ii) and the proof for subcase (i) is similar and will be discussed later. Since t−1≡2(mod3) and t−2≡1(mod3), then by Lemma 2.14, Proposition 2.7 and induction we have
and
Hence by Remark 2.12 depth(S/(I(Ph,t,d))=d((h−1)t+2−(h−1)2)(h−1)3−1+⌈d3⌉.
For subcase (i) the proof is similar, therefore we omit the detailed proof. We have the values depth(S/(I(Ph,t,2):x(0)1))=2((h−1)t+2−(h−1)2)(h−1)3−1+1 and depth(S/(I(Ph,t,2),x(0)1))=2((h−1)t+2−(h−1)2)(h−1)3−1+1. The required result follows by using Remark 2.12.
(2) Let t≡1(mod3). We again consider two subcases that is d=2 and d≥3.
(i) Let d=2. We have the following isomorphims:
and
Since t−1≡0(mod3) and t−2≡2(mod3), then by Lemma 2.14 and Proposition 2.7
and
Hence by Remark 2.12, depth(S/(I(Ph,t,2))=2((h−1)t+2−1)(h−1)3−1+(h−2), as desired.
(ii) Now let d≥3. We have the following isomorphisms:
Then similarly as done for subcase (i) by using same arguments for each terms of above isomorphisms except for first terms on which induction on d is applied and we have the values depth(S/(I(Ph,t,d):x(0)d))=d((h−1)t+2−1)(h−1)3−1+(h−2)⋅⌈d−12⌉ and depth(S/(I(Ph,t,d),x(0)d))=d((h−1)t+2−1)(h−1)3−1+(h−2)⋅⌈d2⌉. Hence by Remark 2.12 we have depth(S/I(Ph,t,d))=d((h−1)t+2−1)(h−1)3+(h−2)⋅⌈d−12⌉.
(3) Let t≡2(mod3).
(i) Let d=2. We have the following isomorphisms:
Now let J=(I(Ph,t,2),x(1)2(h−1)). We have
Since t−1≡1(mod3) and t−3≡2(mod3), then by Lemma 2.14 and Proposition 2.7 we have
and
Thus by Remark 2.12 we have depth(S/J)≥2((h−1)t+2−(h−1))(h−1)3−1)+(h−2) and also J=(I(Ph,t,2),x(1)2(h−1)) implies depth(S/(I(Ph,t,2),x(1)2(h−1)))≥2((h−1)t+2−(h−1))(h−1)3−1+(h−2). Hence again by Remark 2.12 we have, depth(S/I(Ph,t,2))=2((h−1)t+2−(h−1))(h−1)3−1.
(ii) Now let d≥3.
Let J=(I(Ph,t,d),x(1)(h−1)d). We have the following isomorphisms:
and
Then similarly as done for d = 2 by using the same arguments for each terms of above isomorphisms except for the first terms on which induction on d is applied we get the values \text{depth}(S/(I(\mathscr{P}_{h, t, d}):x^{(1)}_{(h-1)d})) = \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1} , \text{depth}(S/(J:x^{(0)}_{d})) = \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}+(h-1)(h-2)+1 and \text{depth}(S/(J, x^{(0)}_{d})) = \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}+(h-2) . And so we have \text{depth}(S/I(\mathscr{P}_{h, t, d})) = \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}.
Theorem 3.8. Let d\geq1 , t\geq1 and h\geq3 . If S = K[V(\mathscr{P}_{h, t, d})] , then
Proof. If d = 1 the result follows from Proposition 2.7. Now let d\geq2 . With similar arguments as in Theorem 3.7 and using Lemma 2.14 and Remark 2.12 we get lower bounds that is
Now we will compute upper bound by induction on t . If t = 1 , by Lemma 2.15
If t = 2 , by Theorem 2.5 \text{ sdepth}(S/I(\mathscr{P}_{h, t, d})) = (h-1)(d) , as desired. Now let t\geq3 and u be a monomial such that u: = x_{1}^{(t-1)}x_{2}^{(t-1)}\dotso x_{d(h-1)^{t-1}}^{(t-1)} . We have the following isomorphism:
then we have
By Proposition 2.10 we have \text{ sdepth} (S/I(\mathscr{P}_{h, t, d}))\leq \text{ sdepth} (S/(I(\mathscr{P}_{h, t, d}):u)) .
\bf (1) Let t\equiv0(\mod3) . Since t-3\equiv0(\mod3) thus by Eq (3.2) and induction on t , \text{ sdepth}(K[V(\mathscr{P}_{h, t-3, d})]/I(\mathscr{P}_{h, t-3, d}))+d(h-1)^{t-1} = \frac{d((h-1)^{t+2-3}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d}{3}\rceil + d (h-1)^{t-1} = \frac{d((h-1)^{t+2}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d}{3}\rceil.
\bf (2) Let t\equiv1(\mod3) . Since t-3\equiv1(\mod3) thus by Eq (3.2) and induction on t we have, \text{ sdepth}(K[V(\mathscr{P}_{h, t-3, d})]/I(\mathscr{P}_{h, t-3, d}))+d(h-1)^{t-1} = \frac{d((h-1)^{t+2-3}-1)}{(h-1)^{3}-1}+(h-2)\lceil\frac{d-1}{2}\rceil + d(h-1)^{t-1} = \frac{d((h-1)^{t+2}-1)}{(h-1)^{3}-1}+(h-2)\lceil\frac{d-1}{2}\rceil.
\bf (3) Let t\equiv2(\mod3) . Since t-3\equiv2(\mod3) thus by Eq (3.2) and induction on t we have, \text{ sdepth}(K[V(\mathscr{P}_{h, t-3, d})]/I(\mathscr{P}_{h, t-3, d}))+d(h-1)^{t-1} = \frac{d((h-1)^{t+2-3}-(h-1))}{(h-1)^{3}-1} + d(h-1)^{t-1} = \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}.
Corollary 3.9. Let h\geq 3 , t\geq1 and d\geq 3 . If S = K[V(\mathscr{P}_{h, t, d})] , then
Proof. We have |V(\mathscr{P}_{h, t, d})| = \Big(\frac{(h-1)^{t+1}-1}{(h-2)}\Big)(d) , therefore, \text{depth}(S) = \Big(\frac{(h-1)^{t+1}-1}{(h-2)}\Big)(d) . Hence we get the required result by using Theorem 3.7 and Theorem 2.8.
4.
Depth, Stanley depth and projective dimension of cyclic modules associated to some unicyclic graphs
In this section we compute depth, Stanley depth and projective dimension of quotient ring K[V(\mathscr{C}_{h, t, d})]/I(\mathscr{C}_{h, t, d}) .
Remark 4.1. In this remark we introduce some terms that appear in special cases of our proofs.
(1) If d = 0 we define K[V(\mathscr{C}_{h, t, 0})]/I(\mathscr{C}_{h, t, 0}): = K , hence
(2) If d\geq3 we define K[V(\mathscr{C}_{h, 0, d})]/I(\mathscr{C}_{h, 0, d}): = K[V(C_d)]/I(C_d) , hence by Proposition 2.3
Theorem 4.2. Let t\geq1 and d\geq3 . If S = K[V(\mathscr{C}_{2, t, d})] , then
Proof. Let d\geq3 . For t = 1 the result follows from Lemma 2.15 and for t = 2 the result follows from Theorem 2.6. Now let t\geq3 .
\bf (1) Let t\equiv0(\mod3) . We have
and
Then by Lemma 2.14, Lemma 2.2 and Theorem 3.4
and
Since t\equiv0(\mod3) implies \lceil\frac{t-1}{3}\rceil = \lceil\frac{t}{3}\rceil = \frac{t}{3} . Hence \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}):x_{d}^{(0)})\right) = \frac{dt}{3}+\lceil\frac{d-3}{3}+1\rceil = \frac{dt}{3}+\lceil\frac{d}{3}\rceil and \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}), x_{d}^{(0)})\right) = \frac{dt}{3}+\lceil\frac{d-1}{3}\rceil. Since for d\equiv0, 2(\mod3) \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}):x_{d}^{(0)})\right) = \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}), x_{d}^{(0)})\right) . Thus by Remark 2.12 \text{depth}\left(S/I(\mathscr{C}_{2, t, d})\right) = \frac{dt}{3}+\lceil\frac{d-1}{3}\rceil .
Now let d\equiv1(\mod3) . We have the following isomorphism:
where
and
Indeed, if w is a monomial such that w\in(I(\mathscr{C}_{2, t, d}):x_{d}^{(0)}) but w \notin I(\mathscr{C}_{2, t, d}) , then w is divisible by at least one variable from the set \{x_{1}^{(0)}, x_{d-1}^{(0)}, x_{d}^{(1)}\} . If w is not divisible by any variable form the set \{x_{1}^{(0)}, x_{d-1}^{(0)}, x_{d}^{(1)}\} , then w \in I(\mathscr{C}_{2, t, d}) , a contradiction. Let w be a monomial such that w\in \frac{(I(\mathscr{C}_{2, t, d}):x_{d}^{(0)})}{I(\mathscr{C}_{2, t, d})} . In order to establish the isomorphism as given in Eq (4.1) one has to consider the following cases.
Case 1. If x_{1}^{(0)}|w , then w = (x_{1}^{(0)})^{\alpha}u , where \alpha\geq1 , u\in K[x_{3}^{(0)}, x_{4}^{(0)}, \dotso, x_{d-1}^{(0)}, x_{2}^{(1)}, x_{2}^{(1)}, \dotso, x_{d}^{(1)}, x_{1}^{(2)}, x_{2}^{(2)}, \dotso, x_{d}^{(2)}, \dotso, x_{1}^{(t)}, x_{2}^{(t)}, \dotso, x_{d}^{(t)}] and u\notin \big(G(I(\mathscr{C}_{2, t, d}))\setminus \{x_{1}^{(0)}x_{1}^{(1)}, x_{1}^{(1)}x_{1}^{(2)}, x_{1}^{(0)}x_{2}^{(0)}, x_{2}^{(0)}x_{2}^{(1)}, x_{2}^{(0)}x_{3}^{(0)}, x_{d-1}^{(0)}x_{d}^{(0)}, x_{1}^{(0)}x_{d}^{(0)}, x_{d}^{(0)}x_{d}^{(1)} \}\big) . Thus w \in x_{1}^{(0)}A_{0} and it is easy to see that
Case 2. If x_{d-1}^{(0)}|w and x_{1}^{(0)}\nmid w , then w = (x_{d-1}^{(0)})^{\beta}v , where \beta\geq1 , v\in K[x_{2}^{(0)}, x_{3}^{(0)}, \dotso, x_{d-3}^{(0)}, x_{1}^{(1)}, x_{2}^{(1)}, \dotso, x_{d-2}^{(1)}, x_{d}^{(1)}, x_{1}^{(2)}, x_{2}^{(2)}, \dotso, x_{d}^{(2)}, \dotso, x_{1}^{(t)}, x_{2}^{(t)}, \dotso, x_{d}^{(t)}] and v\notin \big(G(I(\mathscr{C}_{2, t, d}))\setminus \{x_{1}^{(0)}x_{1}^{(1)}, x_{1}^{(0)}x_{2}^{(0)}, x_{1}^{(0)}x_{d}^{(0)}, x_{d-2}^{(0)}x_{d-3}^{(0)}, x_{d-2}^{(0)}x_{d-2}^{(1)}, x_{d-2}^{(0)}x_{d-1}^{(0)}, x_{d-1}^{(0)}x_{d}^{(0)}, x_{d-1}^{(0)}x_{d-1}^{(1)}, x_{d}^{(0)}x_{d}^{(1)}, x_{d-1}^{(1)}x_{d-1}^{(2)} \}\big)\Big) . Thus w\in x_{d-1}^{(0)}A_{1} and we have
Case 3. If x_{d}^{(1)}|w , x_{1}^{(0)}\nmid w and x_{d-1}^{(0)}\nmid w , then w = (x_{d}^{(1)})^{\gamma}z , where \gamma\geq1 , z\in K[x_{2}^{(0)}, x_{3}^{(0)}, \dotso, x_{d-2}^{(0)}, x_{1}^{(1)}, x_{2}^{(1)}, \dotso, x_{d-1}^{(1)}, x_{1}^{(2)}, x_{2}^{(2)}, \dotso, x_{d-1}^{(2)}, x_{1}^{(3)}, x_{2}^{(3)}, \dotso, x_{d}^{(3)}, \dotso, x_{1}^{(t)}, x_{2}^{(t)}, \dotso, x_{d}^{(t)}] and z\notin \big(G(I(\mathscr{C}_{2, t, d}))\setminus \{x_{1}^{(0)}x_{1}^{(1)}, x_{1}^{(0)}x_{2}^{(0)}, x_{1}^{(0)}x_{d}^{(0)}, x_{d-1}^{(0)}x_{d-2}^{(0)}, x_{d-1}^{(0)}x_{d}^{(0)}, x_{d-1}^{(0)}x_{d-1}^{(1)}, x_{d}^{(0)}x_{d}^{(1)}, x_{d}^{(1)}x_{d}^{(2)}, x_{d}^{(2)}x_{d}^{(3)} \}\big) . Hence w\in x_{d}^{(1)}A_2 and
It is also easy to see that x_{1}^{(0)}A_{0}\cong A_0 , x_{d-1}^{(0)}A_{1}\cong A_1 and x_{d}^{(1)}A_{2}\cong A_2 . Thus by Lemma 2.2, Lemma 2.14 and Theorem 3.4
Hence, \text{depth}\big(\frac{(I(\mathscr{C}_{2, t, d}):x_{d}^{(0)})}{I(\mathscr{C}_{2, t, d})} \big) = \min\{\text{depth}(A_0), \text{depth}(A_1), \text{depth}(A_2)\} = \frac{dt}{3}+\lceil\frac{d-1}{3}\rceil. Now by applying Depth Lemma on following short exact sequence
we get \text{depth}(S/I(\mathscr{C}_{2, t, d})) = \frac{dt}{3}+\lceil\frac{d-1}{3}\rceil.
\bf (2) Let t\equiv1(\mod3) . In a similar way as done in Case 1 we have
and
Since t+2\equiv0(\mod3) implies \lceil\frac{t+2}{3}\rceil = \lceil\frac{t}{3}\rceil . Hence \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}):x_{d}^{(0)})\right) = \lceil\frac{t}{3}\rceil\cdot d and \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}), x_{d}^{(0)})\right) = \lceil\frac{t}{3}\rceil \cdot d . Hence by Remark 2.12 we have \text{depth}\left(S/I(\mathscr{C}_{2, t, d})\right) = \lceil\frac{t}{3}\rceil \cdot d.
(3) Let t\equiv2(\mod3)
We have
Now let J = (I(\mathscr{C}_{2, t, d}), x_{d}^{(1)}) . We have the following isomorphisms:
and
Then by Lemma 2.14, Lemma 2.2 and Theorem 3.4 we have
and
Since t+1\equiv0(\mod3) implies \lceil\frac{t+1}{3}\rceil = \lceil\frac{t}{3}\rceil . Hence \text{depth}\left(S/(I(\mathscr{C}_{2, t, d}):x_{d}^{(1)})\right) = \lceil\frac{t}{3}\rceil\cdot d , \text{depth}\left(S/(J:x_{d}^{(0)})\right) = \lceil\frac{t}{3}\rceil\cdot d+1 and \text{depth}\left(S/(J, x_{d}^{(0)})\right) = \lceil\frac{t}{3}\rceil\cdot d . Thus by Remark 2.12 \text{depth}\left(S/J\right)\geq\lceil\frac{t}{3}\rceil\cdot d and also J = (I(\mathscr{C}_{2, t, d}), x_{d}^{(1)}) implies \text{depth}(S/(I(\mathscr{C}_{2, t, d}), x_{d}^{(1)})) \geq \lceil\frac{t}{3}\rceil\cdot d . Hence again by Remark 2.12 \text{depth}(S/I(\mathscr{C}_{2, t, d})) = \lceil\frac{t}{3}\rceil \cdot d.
Theorem 4.3. Let d\geq3 and t\geq1 . If S = K[V(\mathscr{C}_{2, t, d})] , then \text{ sdepth}\left(S/I(\mathscr{C}_{2, t, d})\right) = \lceil\frac{t}{3}\rceil\cdot d for t\equiv1, 2(\mod3) . Otherwise, \frac{dt}{3}+\lceil\frac{d-1}{3}\rceil \leq \text{ sdepth}\left(S/I(\mathscr{C}_{2, t, d})\right) \leq \frac{dt}{3}+\lceil\frac{d}{3}\rceil .
Proof. We compute lower bound by using similar arguments as in Theorem 4.2 and using Lemma 2.14 and Remark 2.12 that is \text{ sdepth}\left(S/I(\mathscr{C}_{2, t, d})\right)\geq \lceil\frac{t}{3}\rceil\cdot d for t\equiv1, 2(\mod3) otherwise, \text{ sdepth}\left(S/I(\mathscr{C}_{2, t, d})\right) \geq \frac{dt}{3}+\lceil\frac{d-1}{3}\rceil . Now we compute upper bound by induction on t . If t = 1 , then by Lemma 2.15 \text{ sdepth}(S/I(\mathscr{C}_{2, t, d})) = d , as desired. For t = 2 , by Theorem 2.6 \text{ sdepth}(S/I(\mathscr{C}_{2, t, d})) = d , as desired. For t = 3 we have the following isomorphism:
Then we have
Also by Proposition 2.10 we have \text{ sdepth}(S/I(\mathscr{C}_{2, 3, d})) \leq d+\lceil\frac{d}{3}\rceil , and as already we have computed lower bound that is \text{ sdepth}(S/I(\mathscr{C}_{2, 3, d})) \geq d+\lceil\frac{d-1}{3}\rceil. Hence
Now let t\geq4 and u be a monomial such that u: = x_{1}^{(t-1)}x_{2}^{(t-1)}\dotso x_{d}^{(t-1)} . We have the following isomorphism:
Then we have
By Proposition 2.10, \text{ sdepth}(S/I(\mathscr{C}_{2, t, d})) \leq \text{ sdepth}(S/(I(\mathscr{C}_{2, t, d}):u)) .
\bf (1) Let t\equiv1, 2(\mod3) . Since t-3\equiv1, 2(\mod3) , then by using induction on Eq (4.2) we have \text{ sdepth}(K[V(\mathscr{C}_{2, t-3, d})]/I(\mathscr{C}_{2, t-3, d})) + d = \lceil\frac{t-3}{3}\rceil\cdot d + d = \lceil\frac{t}{3}\rceil\cdot d.
\bf (2) Let t\equiv0(\mod3) . Since t-3\equiv0(\mod3) , then by using induction on Eq 4.2 we have \text{ sdepth}(K[V(\mathscr{C}_{2, t-3, d})]/I(\mathscr{C}_{2, t-3, d})) + d = \frac{dt}{3}+\lceil\frac{d}{3}\rceil.
Corollary 4.4. Let h = 2 , t\geq1 and d\geq 3 . If S = K[V(\mathscr{C}_{2, t, d})] , then
Proof. We have |V(\mathscr{C}_{2, t, d})| = d(t+1) , therefore, \text{depth}(S) = d(t+1) . Hence we get the required result by using Theorem 4.2 and Theorem 2.8.
Theorem 4.5. Let d\geq3 , t\geq1 and h\geq3 . If S = K[V(\mathscr{C}_{h, t, d})] , then
Proof. If t = 1 the result follows from the Lemma 2.15. If t = 2 the result follows from Theorem 2.6. Now let t\geq3 .
\bf (1) Let t\equiv0(\mod3) . We have the following isomorphisms:
and
Since t-1 \equiv 2(\mod3) and t-2 \equiv 1(\mod3) , then by Lemma 2.14, Theorem 3.7 and Proposition 2.7 we have
and
For d\equiv 0, 2(\mod3), \lceil\frac{d-1}{3}\rceil = \lceil\frac{d}{3}\rceil . Then by Remark 2.12 we have
Now let d\equiv1(\mod3) . We have the following isomorphism:
where
Indeed if w is a monomial such that w\in (I(\mathscr{C}_{h, t, d}):x_{d}^{(0)}) but w \notin I(\mathscr{C}_{h, t, d}) , then w is divisible by at least one variable from the set \{x_{1}^{(0)}, x_{d-1}^{(0)}, x_{(h-1)d-(h-2)}^{(1)}, x_{(h-1)d-(h-3)}^{(1)}, \dotso, x_{(h-1)d}^{(1)}\} . If w is not divisible by any variable from the set \{x_{1}^{(0)}, x_{d-1}^{(0)}, x_{(h-1)d-(h-2)}^{(1)}, x_{(h-1)d-(h-3)}^{(1)}, \dotso, x_{(h-1)d}^{(1)}\} , then w\in I(\mathscr{C}_{h, t, d}) , a contradiction. Let w be a monomial such that w \in \frac{(I(\mathscr{C}_{h, t, d}):x_{d}^{(0)})}{I(\mathscr{C}_{h, t, d})} . In order to establish the isomorphism as given in Eq (4.3) we adopt the similar strategy of Theorem 4.2. If x_{1}^{(0)}|w then w = ({x_{1}^{(0)}})^{\alpha}u , where \alpha\geq1 , u\notin(G(I(\mathscr{C}_{h, t, d}))\setminus \underset{l = 0}{\overset{1}{\bigcup }}\underset{p = 1}{\overset{(h-1)^{l}}{\bigcup }}\underset{q = (h-1)p-(h-2)}{\overset{(h-1)p}{\bigcup }}\{x_{p}^{(l)}x_{q}^{(l+1)}\}\cup\underset{q = h}{\overset{2(h-1)}{\bigcup }}\{x_{2}^{(0)}x_{q}^{(1)}\}\cup\underset{q = (h-1)d-(h-2)}{\overset{(h-1)d}{\bigcup }}\{x_{d}^{(0)}x_{q}^{(1)}\}\cup\{x_{1}^{(0)}x_{2}^{(0)}, x_{2}^{(0)}x_{3}^{(0)}, x_{1}^{(0)}x_{d}^{(0)}, x_{d-1}^{(0)}x_{d}^{(0)}\}) and u\in K[x_{3}^{(0)}, x_{4}^{(0)}, \dotso, x_{d-1}^{(0)}, x_{h}^{(1)}, x_{h+1}^{(1)}, \dotso, x_{(h-1)d}^{(1)}, x_{1}^{(2)}, x_{2}^{(2)}, \dotso, x_{(h-1)^{2}d}^{(2)}, \dotso, x_{1}^{(t)}, x_{2}^{(t)}, \dotso, x_{(h-1)^{t}d}^{(t)}]. Thus w \in x_{1}^{(0)}A_{0} where
Now proceeding in a similar way if x_{d-1}^{(0)}|w and x_1^{(0)}\nmid w then we have w\in x_{d-1}^{(0)}A_1 . Now let x_{(h-1)d-(h-2)}^{(0)}|w , x_1^{(0)}\nmid w and x_{d-1}^{(0)}\nmid w we have w\in x_{(h-1)d-(h-2)}^{(1)}B_{0} . Continuing in the same fashion we get the required isomorphism and
Now by Lemma 2.14, Theorem 3.7 and Proposition 2.7 we have
Similarly
Thus \text{depth}\big(\frac{(I(\mathscr{C}_{h, t, d}):x_{d}^{(0)})}{I(\mathscr{C}_{h, t, d})} \big) = \frac{d((h-1)^{t+2}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d-1}{3}\rceil. Now by applying Lemma 2.11 on the following short exact sequence
we have the required result that is \text{depth}(S/I(\mathscr{C}_{h, t, d})) = \frac{d((h-1)^{t+2}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d-1}{3}\rceil.
\bf (2) Let t\equiv1(\mod3) . In a similar way as done in Case 1 we have
and
Hence by Remark 2.12 we have \text{depth}(S/I(\mathscr{C}_{h, t, d}) = \frac{d((h-1)^{t+2}-1)}{(h-1)^{3}-1} + (h-2)\lceil\frac{d}{2}\rceil.
\bf (3) Let t\equiv2(\mod3) . We have the following isomorphism:
Now let J = (I(\mathscr{C}_{h, t, d}), x^{(1)}_{(h-1)d}) , then we have
and
Since t-1 \equiv 1(\mod3) , t-2 \equiv 0(\mod3) and t-3 \equiv 2(\mod3) , then by Lemma 2.14, Theorem 3.7 and Proposition 2.7 we have
and
Hence by Remark 2.12 we have \text{depth}(S/J)\geq \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}+(h-2) and also J = (I(\mathscr{C}_{h, t, d}), x^{(1)}_{(h-1)d}) implies \text{depth}(S/(I(\mathscr{C}_{h, t, d}), x^{(1)}_{(h-1)d}))\geq \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}+(h-2) . Thus again by Remark 2.12 we have \text{depth}(S/I(\mathscr{C}_{h, t, d})) = \frac{d((h-1)^{t+2}-(h-1))}{(h-1)^{3}-1}.
Theorem 4.6. Let d\geq3 , t\geq0 and h\geq3 . If S = K[V(\mathscr{C}_{h, t, d})] , then
Otherwise, \frac{d((h-1)^{t+2}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d-1}{3}\rceil \leq \text{ sdepth}\left(S/I(\mathscr{C}_{h, t, d})\right) \leq \frac{d((h-1)^{t+2}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d}{3}\rceil.
Proof. We compute lower bound by using similar arguments as in Theorem 4.5 and using Lemma 2.14 and Remark 2.12 we get.
otherwise \text{ sdepth}\left(S/I(\mathscr{C}_{h, t, d})\right) \geq \frac{d((h-1)^{t+2}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d-1}{3}\rceil .
Now we compute upper bound by induction on t . If t = 1 result follows from Lemma 2.15 and for t = 2 result follows from Theorem 2.6. For t = 3 we have the following isomorphism:
Then we have
then by Lemma 2.2 we have
Also by Proposition 2.10 we have \text{ sdepth}(S/I(\mathscr{C}_{h, 3, d})) \leq (h-1)^{2}d+\lceil\frac{d}{3}\rceil and already we have computed lower bound that is \text{ sdepth}(S/I(\mathscr{C}_{h, 3, d})) \geq (h-1)^{2}d+\lceil\frac{d-1}{3}\rceil. Now let t\geq4 and u be a monomial such that u: = x_{1}^{(t-1)}x_{2}^{(t-1)}\dotso x_{d(h-1)^{t-1}}^{(t-1)} . We have
Then we have
By Proposition 2.10 we have \text{ sdepth}(S/I(\mathscr{C}_{h, t, d})) \leq \text{ sdepth}(S/(I(\mathscr{C}_{h, t, d}):u)) .
\bf (1) Let t\equiv1(\mod3) implies t-3\equiv1(\mod3) then from Eq (4.4) and by induction we have \text{ sdepth}(K[V(\mathscr{C}_{h, t-3, d})]/I(\mathscr{C}_{h, t-3, d})) + d(h-1)^{t-1} = \frac{{d((h-1)^{t+2-3}}-1)}{(h-1)^{3}-1}+(h-2)\lceil\frac{d}{2}\rceil + d(h-1)^{t-1} = \frac{{d((h-1)^{t+2}}-1)}{(h-1)^{3}-1}+(h-2)\lceil\frac{d}{2}\rceil , as desired.
\bf (2) Let t\equiv2(\mod3) implies t-3\equiv2(\mod3) then from Eq (4.4) and by induction we have \text{ sdepth}(K[V(\mathscr{C}_{h, t-3, d})]/I(\mathscr{C}_{h, t-3, d})) + d(h-1)^{t-1} = \frac{{d((h-1)^{t+2-3}}-(h-1))}{(h-1)^{3}-1}+ d(h-1)^{t-1} = \frac{{d((h-1)^{t+2}}-(h-1))}{(h-1)^{3}-1} , as desired.
\bf (3) Let t\equiv0(\mod3) implies t-3\equiv0(\mod3) then from Eq (4.4) and by induction we have \text{ sdepth}(K[V(\mathscr{C}_{h, t-3, d})]/I(\mathscr{C}_{h, t-3, d})) + d(h-1)^{t-1} = \frac{{d((h-1)^{t+2}}-(h-1)^{2})}{(h-1)^{3}-1}+\lceil\frac{d}{3}\rceil, as desired.
Corollary 4.7. Let h\geq 3 , t\geq1 and d\geq 3 . If S = K[V(\mathscr{C}_{h, t, d})] , then
Proof. We have |V(\mathscr{C}_{h, t, d})| = \Big(\frac{(h-1)^{t+1}-1}{(h-2)}\Big)(d) , therefore, \text{depth}(S) = \Big(\frac{(h-1)^{t+1}-1}{(h-2)}\Big)(d) . Hence we get the required result by using Theorem 4.5 and Theorem 2.8.
Conflict of interest
It is declared by the authors that there is no conflict of interest in this paper.









 DownLoad:
DownLoad:








