1.
Introduction
The idea of metric spaces, as well as the Banach contraction principle, provide the foundation of fixed point theory. Thousands of academics are drawn to spaciousness by axiomatic interpretation of metric space. There have been several generalizations on metric spaces thus far. This demonstrates the beauty, allure, and growth of the notion of metric spaces.
Zadeh [1] developed the concept of fuzzy sets. The adjective "fuzzy" appears to be a popular and common one in recent investigations of the logical and set theoretical underpinnings of mathematics. The key explanation for this rapid rise, in our opinion, is simple. The world around us is full of uncertainty for the following reasons: the information we gather from our surroundings, the concepts we employ, and the data arising from our observations or measurements are, in general, hazy and erroneous. As a result, every formal representation of the real world, or some of its properties, is always an approximation and idealization of the actual reality. Fuzzy sets, fuzzy orderings, fuzzy languages, and other concepts enable us to handle and investigate the degree of uncertainty indicated above in a strictly mathematical and formal manner. The fuzzy set notion has succeeded in moving many mathematical structures within its concept. The concept of continuous norms was established by Schweizer and Sklar [2] The concept of fuzzy metric spaces was developed by Kramosil and Michalek [3]. They extended the concept of fuzziness to traditional conceptions of metric and metric spaces via continuous norms and contrasted the results to those derived from other, particularly probabilistic, statistical extensions of metric spaces. The fuzzy version of the Banach contraction principle in fuzzy metric spaces was introduced by Garbiec [4]. UrReham et al. [5] demonstrated several α−ϕ fuzzy cone contraction findings using an integral type.
Only membership functions are dealt with in fuzzy metric spaces. Park [6] constructed an intuitionistic fuzzy metric space that is utilized to deal with both membership and non-membership functions. Konwar [7] introduced the idea of an intuitionistic fuzzy b-metric space and demonstrated various fixed point theorems. In [8], Kiricsci and Simsek established the concept of neutrosophic metric spaces, which are utilized to deal with membership, non-membership, and naturalness. Simsek and Kiricsci [9] demonstrated some incredible fixed-point solutions in the framework of neutrosophic metric spaces. In the setting of neutrosophic metric spaces, Sowndrarajan et al. [10] demonstrated certain fixed point findings. Hussain, Al Sulami, and Ishtiaq [11] developed the concept of neutrosophic rectangular metric space and established fixed point theorems on it.
The idea of an orthogonal set, as well as many various types of orthogonality, has several applications in mathematics. In 2017, Eshaghi Gordji, Ramezani, De la Sen, and Cho [12] proposed a new notion of orthogonality in metric spaces and offered a framework to expand the findings in the setting of metric space with new orthogonality and also proved several fixed point theorems. Eshaghi Gordji and Habibi [13] modified the concept in 2017 to establish the fixed point theorem in generalized orthogonal metric space. Many writers [14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29] have explored orthogonal contractive type mappings and gotten significant results.
In this paper, we present the concept of an orthogonal neutrosophic rectangular metric space and prove fixed-point theorems.
2.
Preliminaries
In this section, the authors provide some definitions to understand the main section.
Definition 2.1. (See[6,Definition 2.1]) A binary operation ∗:[0,1]×[0,1]→[0,1] is called a continuous triangle norm if:
(1) ι∗ν=ν∗ι, for all ι,ν∈[0,1];
(2) ∗ is continuous;
(3) ι∗1=ι, for all ι∈[0,1];
(4) (ι∗ν)∗η=ι∗(ν∗η), for all ι,ν,η∈[0,1];
(5) If ι≤η and ν≤d, with ι,ν,η,d∈[0,1], then ι∗ν≤η∗d.
Definition 2.2. (See[6,Definition 2.2]) A binary operation ∘:[0,1]×[0,1]→[0,1] is called a continuous triangle co-norm if:
(1) ι∘ν=ν∘ι, for all ι,ν∈[0,1];
(2) ∘ is continuous;
(3) ι∘0=0, for all ι∈[0,1];
(4) (ι∘ν)∘η=ι∘(ν∘η), for all ι,ν,η∈[0,1];
(5) If ι≤η and η≤d, with ι,ν,η,d∈[0,1], then ι∘ν≤η∘d.
Definition 2.3. (See[7,Definition 2.1]) Take Γ≠∅. Let ∗ be a continuous t-norm, ∘ be a continuous t-co-norm, b≥1 and Ψ,Φ be fuzzy sets on Γ×Γ×(0,+∞). If (Γ,Ψ,Φ,∗,∘) fullfils all ϱ,M∈Γ and υ,ζ>0:
(I) Ψ(ϱ,M,ζ)+Φ(ϱ,M,ζ)≤1;
(II) Ψ(ϱ,M,ζ)>0;
(III) Ψ(ϱ,M,ζ)=1 if and only if ϱ=M;
(IV) Ψ(ϱ,M,ζ)=Ψ(M,ϱ,ζ);
(V) Ψ(ϱ,μ,b(ζ+υ))≥Ψ(ϱ,M,ζ)∗Ψ(M,μ,υ);
(VI) Ψ(ϱ,M,⋅) is a non-decreasing function of R+ and limζ→+∞Ψ(ϱ,M,ζ)=1;
(VII) Φ(ϱ,M,ζ)>0;
(VIII) Φ(ϱ,M,ζ)=0 if and only if ϱ=M;
(IX) Φ(ϱ,M,ζ)=Φ(M,ϱ,ζ);
(X) Φ(ϱ,μ,b(ζ+υ))≤Φ(ϱ,M,ζ)∘Φ(M,μ,υ);
(XI) Φ(ϱ,M,⋅) is a non-increasing function of R+ and limζ→+∞Φ(ϱ,M,ζ)=0.
Then, (Γ,Ψ,Φ,∗,∘) is an intuitionistic fuzzy b-metric space.
Definition 2.4. (See[8,Definition 3.1]) Let Γ≠∅,∗ is a continuous t-norm, ∘ be a continuous t-co-norm, and Ψ,Φ,χ are neutrosophic sets on Γ×Γ×(0,+∞) is said to be a neutosophic metric on Γ, if for all ϱ,M,μ∈Γ, the following conditions are satisfied:
(1) Ψ(ϱ,M,ζ)+Φ(ϱ,M,ζ)+χ(ϱ,M,ζ)≤3;
(2) Ψ(ϱ,M,ζ)>0;
(3) Ψ(ϱ,M,ζ)=1 for all ζ>0, if and only if ϱ=M;
(4) Ψ(ϱ,M,ζ)=Ψ(M,ϱ,ζ);
(5) Ψ(ϱ,μ,ζ+υ)≥Ψ(ϱ,M,ζ)∗Ψ(M,μ,υ);
(6) Ψ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞Ψ(ϱ,M,ζ)=1;
(7) Φ(ϱ,M,ζ)<1;
(8) Φ(ϱ,M,ζ)=0 for all ζ>0, if and only if ϱ=M;
(9) Φ(ϱ,M,ζ)=Φ(M,ϱ,ζ);
(10) Φ(ϱ,μ,ζ+υ)≤Φ(ϱ,M,ζ)∘Φ(M,μ,υ);
(11) Φ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞Φ(ϱ,M,ζ)=0;
(12) χ(ϱ,M,ζ)<1;
(13) χ(ϱ,M,ζ)=0 for all ζ>0, if and only if ϱ=M;
(14) χ(ϱ,M,ζ)=χ(M,ϱ,ζ);
(15) χ(ϱ,μ,ζ+υ)≤χ(ϱ,M,ζ)∘χ(M,μ,υ);
(16) χ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞χ(ϱ,M,ζ)=0;
(17) If ζ≤0, then Ψ(ϱ,M,ζ)=0,Φ(ϱ,M,ζ)=1 and χ(ϱ,M,ζ)=1.
Then, (Γ,Ψ,Φ,χ,∗,∘) is called a neutrosophic metric space.
Definition 2.5. (See[11,Definition 12]) Let Γ≠∅ and ∗ be a continuous t-norm, ∘ be a continuous t-co-norm and (Ψ,Φ,D) be neutrosophic sets on Γ×Γ×(0,+∞) is said to be a neutrosophic rectangular metric on Γ, if for any ϱ,μ∈Γ and all distinct x,M∈Γ∖{ϱ,μ}, then the following conditions are satisfied:
(i) Ψ(ϱ,M,ζ)+Φ(ϱ,M,ζ)+D(ϱ,M,ζ)≤3;
(ii) Ψ(ϱ,M,ζ)>0;
(iii) Ψ(ϱ,M,ζ)=1 for all ζ>0, if and only if ϱ=M;
(iv) Ψ(ϱ,M,ζ)=Ψ(M,ϱ,ζ);
(v) Ψ(ϱ,μ,ζ+υ+ϖ)≥Ψ(ϱ,M,ζ)∗Ψ(M,x,υ)∗Ψ(x,μ,ϖ);
(vi) Ψ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞Ψ(ϱ,M,ζ)=1;
(vii) Φ(ϱ,M,ζ)<1;
(viii) Φ(ϱ,M,ζ)=0 for all ζ>0, if and only if ϱ=M;
(ix) Φ(ϱ,M,ζ)=Φ(M,ϱ,ζ);
(x) Φ(ϱ,μ,ζ+υ+ϖ)≤Φ(ϱ,M,ζ)∘Φ(M,x,υ)∘Φ(x,μ,ϖ);
(xi) Φ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞Φ(ϱ,M,ζ)=0;
(xii) D(ϱ,M,ζ)<1;
(xiii) D(ϱ,M,ζ)=0 for all ζ>0, if and only if ϱ=M;
(xiv) D(ϱ,M,ζ)=D(M,ϱ,ζ);
(xv) D(ϱ,μ,ζ+υ+ϖ)≤D(ϱ,M,ζ)∘D(M,x,υ)∘D(x,μ,ϖ);
(xvi) D(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞D(ϱ,M,ζ)=0;
(xvii) If ζ≤0, then Ψ(ϱ,M,ζ)=0,Φ(ϱ,M,ζ)=1 and χ(ϱ,M,ζ)=1.
Then, (Γ,Ψ,Φ,D,∗,∘) is called a neutrosophic rectangular metric space.
Example 2.1. Let Γ=D∪Υ, where D={0,12,13,14}, Υ=[1,2] and d:Γ×Γ→[0,+∞) as follows:
and
Define Ψ,Φ,D:Γ×Γ×(0,+∞)→[0,1] as
Then, we have
Then (Γ,Ψ,Φ,D,∗,∘) is a neutrosophic rectangular metric space with continuous t-norm ι∗Λ=ιΛ and continuous t-co-norm ι∘Λ=max{ι,Λ}.
On the other hand, Eshaghi Gordji et al. [12] introduced the basic concept as follows:
Definition 2.6. (See[12,Definition 2.1]) Let Γ be a non-empty set and binary relation as ⊥⊆Γ×Γ. If ⊥ satisfies condition
then, (Γ,⊥) is said to be an orthogonal set(O-set).
Example 2.2. (See[12,Example 2.4]) Let Γ=Z. Define the binary relation ⊥ on Γ by m⊥n if there exists k∈Z such that m=kn. It is easy to see that 0⊥n for all n∈Z. Hence, (Γ,⊥) is an O-set.
Definition 2.7. (See[12,Definition 3.1]) Let (Γ,⊥) be an O-set. A sequence {ϱβ}β∈N is called an orthogonal sequence (O-sequence) if
Definition 2.8. (See[12,Definition 3.2]) A mapping ω:Γ→Γ is orthogonal continuous (O-continuous) in ϱ∈Γ if for each O-sequence {ϱβ}β∈N⊂Γ such that ϱβ→ϱ, ωϱβ→ωϱ. Also ω is said to be ⊥-continuous on Γ if ω is ⊥-continuous at each ϱ∈Γ.
Definition 2.9. (See[12,Definition 3.10]) Let (Γ,⊥) be an O-set. A mapping ω:Γ→Γ is said to be ⊥-preserving if ωϱ⊥ωM, then ϱ⊥M.
Ishtiaq, Javed, Uddin, De la Sen, Ahmed, and Ali [30] introduced the notion of an orthogonal neutrosophic metric spaces and proved fixed point results on orthogonal neutrosophic metric spaces as follows
Theorem 2.1. (See[30,Theorem 3]) Let (Γ,Ψ,Φ,D,∗,∘,⊥) be an O-complete neutrosophic metric space such that
for all ϱ,M∈Γ and ζ>0. Let ω:Γ→Γ be an ⊥-continuous, ⊥-contraction and ⊥-preserving mapping. Then ω has a unique fixed point say ϱ⋆∈Γ. Furthermore
for all ϱ,M∈Γ and ζ>0.
Motivated by the above work, we introduce the notion of an orthogonal neutrosophic rectangular metric space and prove fixed-point theorems.
3.
Main results
In this part, we present orthogonal neutrosophic rectangular metric space and demonstrate some fixed-point results.
Definition 3.1. Let Γ≠∅ and ∗ be a continuous t-norm, ∘ be a continuous t-co-norm and Ψ,Φ, and D be neutrosophic sets on Γ×Γ×(0,+∞) is said to be a orthogonal neutrosophic rectangular metric on Γ, if for any ϱ,μ∈Γ and all distinct x,M∈Γ∖{ϱ,μ}, the following conditions are satisfied:
(i) Ψ(ϱ,M,ζ)+Φ(ϱ,M,ζ)+D(ϱ,M,ζ)≤3 such that ϱ⊥M and M⊥ϱ;
(ii) Ψ(ϱ,M,ζ)>0 such that ϱ⊥M and M⊥ϱ;
(iii) Ψ(ϱ,M,ζ)=1 for all ζ>0, if and only if ϱ=M such that ϱ⊥M and M⊥ϱ;
(iv) Ψ(ϱ,M,ζ)=Ψ(M,ϱ,ζ) such that ϱ⊥M and M⊥ϱ;
(v) Ψ(ϱ,μ,ζ+υ+ϖ)≥Ψ(ϱ,M,ζ)∗Ψ(M,x,υ)∗Ψ(x,μ,ϖ) such that ϱ⊥μ, ϱ⊥M, M⊥x and x⊥μ;
(vi) Ψ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞Ψ(ϱ,M,ζ)=1 such that ϱ⊥M and M⊥ϱ;
(vii) Φ(ϱ,M,ζ)<1 such that ϱ⊥M and M⊥ϱ;
(viii) Φ(ϱ,M,ζ)=0 for all ζ>0, if and only if ϱ=M such that ϱ⊥M and M⊥ϱ;
(ix) Φ(ϱ,M,ζ)=Φ(M,ϱ,ζ) such that ϱ⊥M and M⊥ϱ;
(x) Φ(ϱ,μ,ζ+υ+ϖ)≤Φ(ϱ,M,ζ)∘Φ(M,x,υ)∘Φ(x,μ,ϖ) such that ϱ⊥μ, ϱ⊥M, M⊥x and x⊥μ;
(xi) Φ(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞Φ(ϱ,M,ζ)=0 such that ϱ⊥M and M⊥ϱ;
(xii) D(ϱ,M,ζ)<1 such that ϱ⊥M and M⊥ϱ;
(xiii) D(ϱ,M,ζ)=0 for all ζ>0, if and only if ϱ=M such that ϱ⊥M and M⊥ϱ;
(xiv) D(ϱ,M,ζ)=D(M,ϱ,ζ) such that ϱ⊥M and M⊥ϱ;
(xv) D(ϱ,μ,ζ+υ+ϖ)≤D(ϱ,M,ζ)∘D(M,x,υ)∘D(x,μ,ϖ) such that ϱ⊥μ, ϱ⊥M, M⊥x and x⊥μ;
(xvi) D(ϱ,M,⋅):(0,+∞)→[0,1] is continuous and limζ→+∞D(ϱ,M,ζ)=0 such that ϱ⊥M and M⊥ϱ;
(xvii) If ζ≤0, then Ψ(ϱ,M,ζ)=0,Φ(ϱ,M,ζ)=1 and χ(ϱ,M,ζ)=1 such that ϱ⊥M and M⊥ϱ.
Then, (Γ,Ψ,Φ,D,∗,∘,⊥) is called an orthogonal neutrosophic rectangular metric space(O-neutrosophic rectangular metric space).
Example 3.1. Let Γ={1,2,3,4} and a binary relation ⊥ by ϱ⊥M iff ϱ+M≥0. Define Ψ,Φ,D:Γ×Γ×(0,+∞)→[0,1] as
and
Then, (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space with continuous t-norm ι∗ν=ιν and continuous t-co-norm, ι∘Λ=max{ι,Λ}.
Proof. Here, we prove (v), (x) and (xv) others are obvious.
Let ϱ=1,M=2, x=3 and μ=4. Then
On the other hand,
and
That is,
Then, the above is satisfies for all ζ,υ,ϖ>0. Hence,
Now,
On the other hand,
and
That is,
Hence,
for all ζ,υ,ϖ>0. Now,
On the other hand,
and
That is,
Hence,
for all ζ,υ>0. Hence, (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space.
Remark 3.1. The preceding example also satisfies for continuous t-norm ι∗Λ=min{ι,Λ} and continuous t-co-norm ι∘Λ=max{ι,Λ}.
Example 3.2. Let Γ=D∪Υ, where D={0,12,13,14} and Υ=[1,2]. Define a binary relation ⊥ by ϱ⊥M iff ϱ+M≥0 and d:Γ×Γ→[0,+∞) as follows:
and
Define Ψ,Φ,D:Γ×Γ×(0,+∞)→[0,1] as
Then, we have
Then (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space with continuous t-norm ι∗Λ=ιΛ and continuous t-co-norm ι∘Λ=max{ι,Λ}.
Definition 3.2. Let (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space and {ϱβ} be an O-sequence in Γ. Then {ϱβ} is said to be:
(a) an orthogonal convergent(O-convergent) exists if there exists ϱ∈Γ such that
(b) an orthogonal Cauchy sequence(O-Cauchy sequence), if and only if for each Λ>0,ζ>0, there exists β0∈N such that
If every O-Cauchy sequence is convergent in Γ, then (Γ,Ψ,Φ,D,∗,∘,⊥) is called a complete orthogonal neutrosophic rectangular metric space.
Definition 3.3. Let (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space, an open ball is then defined B(ϱ,r,ζ) with center ϱ, radius r,0<r<1 and ζ>0 as follows:
Theorem 3.1. Every open ball is an open set in an orthogonal neutrosophic rectangular metric space.
Proof. Consider B(k,r,ζ) be an open ball with center k and radius r. Assume r∈B(k,r,ζ). Therefore, ℜ(k,d,ζ)>1−r,ℵ(k,d,ζ)<r,B(k,d,ζ)<r. There exists ζ3∈(0,ζ) such that ℜ(k,d,ζ3)>1−r,ℵ(k,d,ζ3)<r, D(k,d,ζ3)<r due to ℜ(k,d,ζ)>1−r. If we take r0=ℜ(k,d,ζ3), then for r0>1−r,ϵ∈(0,1) will exist such that r0>1−ϵ>1−r. Given r0 and ϵ such that r0>1−ϵ. Then r1,r2,r3,r4,r5,r6∈(0,1) will exist such that r0∗r1∗r2>1−ϵ,(1−r0)∘(1−r3)∘(1−r4)≤ϵ and (1−r0)∘(1−r5)∘(1−r6)≤ϵ. Choose r7=max{r1,r2,r3,r4,r5,r6}. Consider the open ball B(d,1−r7,ζ3). We will show that B(d,1−r7,ζ3)⊂B(k,r,ζ). If we take v∈B(d,1−r7,ζ3), then ℜ(g,d,ζ3)>r7,ℵ(g,d,ζ3)<r7,B(g,d,ζ3)<r7 and ℜ(d,v,ζ3)>r7,ℵ(d,v,ζ3)<r7,B(d,v,ζ3)<r7. Then
It shows that v∈B(k,r,ζ) and B(d,1−r7,ζ3)⊂B(k,r,ζ).
Theorem 3.2. Every orthogonal neutrosophic rectangular metric space is Hausdorff.
Proof. Let (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space. Let ρ and M be any distinct points in Γ. Then, 0<Ψ(ϱ,M,ζ)<1, 0<Φ(ϱ,M,ζ)<1 and 0<D(ϱ,M,ζ)<1. Put r1=Ψ(ϱ,M,ζ), 1−r2=Φ(ϱ,M,ζ), 1−r3=D(ϱ,M,ζ) and r4=Ψ(ϱ,g,ζ3), 1−r5=Φ(ϱ,g,ζ3),1−r6=D(ϱ,g,ζ3) and r=max{r1,1−r2,1−r3,r4,1−r5,1−r6}. For each r0∈(r,1), there exists r7 and r8 such that r4∗r7∗r7≥r0, (1−r5)∘(1−r8)∘(1−r8)≤1−r0 and (1−r6)∘(1−r8)∘(1−r8)≤1−r0. Put r9=max{r7,r8} and consider the open balls B(ρ,1−r9,ζ3) and B(M,1−r9,ζ3). Then, clearly
Suppose that v∈B(ρ,1−r9,ζ3)∩B(M,1−r9,ζ3). Then,
which is a contradiction. Hence, (Γ,Ψ,Φ,D,∗,∘,⊥) is Hausdorff.
Lemma 3.1. Let {ϱβ} be an O-Cauchy sequence in orthogonal neutrosophic rectangular metric space (Γ,Ψ,Φ,D,∗,∘,⊥) such that ϱβ≠ϱα whenever α,β∈N with β≠α. Then the O-sequence {ϱβ} can converge to, at most, one limit point.
Proof. Contrarily, assume that ϱβ→ϱ and ϱβ→M, for ϱ≠M. Then, limβ→+∞Ψ(ϱβ,ϱ,ζ)=1,limβ→+∞Φ(ϱβ,ϱ,ζ)=0,limβ→+∞D(ϱβ,ϱ,ζ)=0, and limβ→+∞Ψ(ϱβ,M,ζ) = 1, limβ→+∞Φ(ϱβ,M,ζ)=0,limβ→+∞D(ϱβ,M,ζ)=0, for all ζ>0. Suppose
That is Ψ(ϱ,M,ζ)≥1∗1∗1=1,Φ(ϱ,M,ζ)≤0∘0∘0=0 and D(ϱ,M,ζ)≤0∘0∘0=0. Hence, ϱ=M.
Lemma 3.2. Let (Γ,Ψ,Φ,D,∗,∘,⊥) is an orthogonal neutrosophic rectangular metric space. If for some 0<σ<1 and for any ϱ,M∈Γ,ζ>0,
then ϱ=M.
Proof. (3.1) implies that
Now,
Also, by Definition of (iii), (viii), (xiii), that is, ϱ=M.
Definition 3.4. Let (Γ,Ψ,Φ,D,∗,∘,⊥) be an orthogonal neutrosophic rectangular metric space. A mapping ω:Γ→Γ is an othogonal neutrosophic rectangular contraction type-1(⊥-neutrosophic rectangular contraction type-1) if there exists 0<σ<1 such that
for all ϱ,M∈Γ with ϱ⊥M and ζ>0.
Theorem 3.3. Let (Γ,Ψ,Φ,D,∗,∘,⊥) be a complete orthogonal neutrosophic rectangular metric space and ω:Γ→Γ be a mapping satisfying
(a) ω is an ⊥-neutrosophic rectangular contraction type-1;
(b) ω is an ⊥-preserving.
Then ω has a unique fixed point.
Proof. Since (Γ,⊥) is an O-set,
It follows that ϱ0⊥ωϱ0 or ωϱ0⊥ϱ0. Let
for all β∈N∪{0}.
If ϱβ0=ϱβ0+1 for any β0∈N∪{0}, then it is clear that ϱβ0 is a fixed point of ω. Assume that ϱβ0≠ϱβ0+1 for all β0∈N∪{0}. Since ω is ⊥-preserving, we have
for all β0∈N∪{0}. This implies {ϱβ} is an O-sequence. Since ⊥-neutrosophic rectangular contraction type-1, we obtain
and
We obtain
Using (v), (x) and (xv), we have the following cases:
Case 1. When i=2α+1, i.e., i is odd, then
and
Using (3.3) in the above inequalities, we deduce
Case 2. When i=2α, i.e., i is even, then
and
Using (3.3) in the above inequalities, we deduce
and
As β→+∞, we deduce
and
Therefore, {ϱβ} is a Cauchy sequence. Since (Γ,Ψ,Φ,D,∗,∘,⊥) is a complete orthogonal neutrosophic rectangular metric space, we can find
Using (v),(x) and (xv), we get
and
Hence, ωϱ=ϱ. Let ϱ,η∈Γ be two fixed points of ω and suppose that ωβϱ=ϱ≠η=ωβη for all β∈N. By choice of ϱ0, we obtain
Since ω is ⊥-preserving, we have
for all n∈N. Since ⊥-neutrosophic rectangular contraction type-1, we have
and
by using (iii),(viii) and (xiii), ϱ=η.
Definition 3.5. Let (Γ,Ψ,Φ,D,∗,∘,⊥) be an orthogonal neutrosophic rectangular metric space. A map ω:Γ→Γ is an orthogonal neutrosophic rectangular contraction type-2 (⊥-neutrosophic rectangular contraction type-2) if there exists 0<σ<1, such that
and
for all ϱ,M∈Γ with ϱ⊥M and ζ>0.
Now, we prove the theorem for O-NRT(orthogonal neutrosophic rectangular) contraction.
Theorem 3.4. Let (Γ,Ψ,Φ,D,∗,∘,⊥) be a complete orthogonal neutrosophic rectangular metric space. and ω:Γ→Γ be a mapping satisfying
(a) ω is an ⊥- neutrosophic rectangular contraction type-2,
(b) ω is an ⊥-preserving.
Then ω has a unique fixed point.
Proof. Since (Γ,⊥) is an O-set,
It follows that ϱ0⊥ωϱ0 or ωϱ0⊥ϱ0. Let
for all β∈N∪{0}.
If ϱβ0=ϱβ0+1 for any β0∈N∪{0}, then it is clear that ϱβ0 is a fixed point of ω. Assume that ϱβ0≠ϱβ0+1 for all β0∈N∪{0}. Since ω is ⊥-preserving, we have
for all β0∈N∪{0}. This implies {ϱβ} is an O-sequence. Since ω is an ⊥-neutrosophic rectangular contraction type-2, we have
Continuing in this way, we get
We obtain
and
Using (v),(x) and (xv), we have the following cases:
Case 1. When i=2α+1, i.e., i is odd, then
and
Using (3.3) in the above inequalities, we deduce
and
Case 2. When i=2α, i.e., i is even, then
and
Using (3.3) in the above inequalities, we deduce
As β→+∞, we deduce
and
Therefore, {ϱβ} is a Cauchy sequence. Since (Γ,Ψ,Φ,D,∗,∘,⊥) be a complete orthogonal neutrosophic rectangular metric space, we can find
Using (v),(x) and (xv), we get
Using the above inequality, we obtain
and
Hence, ωϱ=ϱ. Let ϱ,η∈Γ be two fixed points of ω and suppose that ωβϱ=ϱ≠η=ωβη for all β∈N. By choice of ϱ0, we obtain
Since ω is ⊥-preserving, we have
for all n∈N. Since ⊥-neutrosophic rectangular contraction type-2, we have
which is a contradiction.
which is a contradiction and
which is a contradiction. Therefore, we must have Ψ(ϱ,η,ζ)=1,Φ(ϱ,η,ζ)=0 and D(ϱ,η,ζ)=0, hence, ϱ=η.
Example 3.3. Let Γ=[0,1]. Define the binary relation ⊥ on Γ by ϱ⊥M iff ϱ+M≥0 and Ψ,Φ,D:Γ×Γ×(0,+∞)→[0,1] by
for all ϱ,M∈Γ with ϱ⊥M and ζ>0. Then, (Γ,Ψ,Φ,D,∗,∘,⊥) is a complete orthogonal neutrosophic rectangular metric space with continuous t-norm ι∗ν=ιν and continuous t-co-norm ι∘ν=max{ι,ν}.
Define ω:Γ→Γ by ω(ϱ)=1−5−ϱ7 and take σ∈[12,1), then
and
Therefore ω is an orthogonal neutrosophic contraction type-1. Clearly ω is an ⊥-preserving. Hence, all the hypothesis of Theorem 3.3 are fulfilled, and 0 is the only fixed point for ω.
4.
Application to Fredholm integral equation
Suppose Γ=C([c,a],R) is the set of real value continuous functions defined on [c,a].
Suppose the integral equation:
where δ>0,∧(v) is a fuzzy function of v:v∈[c,a] and ℧:C([c,a]×R)→R+. Define the binary relation ⊥ on Γ by ϱ⊥M iff ϱ+M≥0 and Ψ,Φ,D:Γ×Γ×(0,+∞)→[0,1] by
and
for all ϱ,M∈Γ with ϱ⊥M and ζ>0, continuous t-norm and continuous t-co-norm define by ι∗ν=ι⋅ν and ι∘ν=max{ι,ν}. Then (Γ,Ψ,Φ,D,∗,∘) is a complete orthogonal neutrosophic rectangular metric space. Suppose that |℧(τ,v)ϱ(τ)−℧(τ,v)M(τ)|≤|ϱ(τ)−M(τ)| for ϱ,M∈Γ,σ∈(0,1) and ∀τ,v∈[c,a]. Also, let ℧(τ,v)(δ∫acdv)≤σ<1. Then, the integral Eq (4.1) has a unique solution.
Proof. Define ω:Γ→Γ by
Clearly ω is an ⊥-preserving. Now, for all ϱ,M∈Γ with ϱ⊥M, we deduce
and
Therefore, \omega is an orthogonal neutrosophic contraction type-1. Hence, all the conditions of Theorem 3.3 are satisfied and operator \omega has a unique fixed point.
Example 4.1. Assume the following non-linear integral equation.
Then it has a solution in \varGamma .
Proof. Let \omega\colon\varGamma\rightarrow \varGamma be defined by
and set \mho(\tau, \mathfrak{v})\varrho(\tau) = \frac{1}{7}\mathfrak{v}\varrho(\mathfrak{v}) and \mho(\tau, \mathfrak{v})\mathcal{M}(\tau) = \frac{1}{7}\mathfrak{v}\mathcal{M}(\mathfrak{v}) , where \varrho, \mathcal{M}\in\varGamma , and for all \tau, \mathfrak{v}\in[0, 1] . Then, we have
Furthermore, see that \frac{1}{7}\int_{0}^{1}\mathfrak{v}\mathfrak{d}\mathfrak{v} = \frac{1}{7}\big(\frac{(1)^2}{2}-\frac{(0)^2}{2}\big) = \frac{1}{7} = \sigma\leq1 , where \delta = \frac{1}{7} . Hence, it is easy to see that all other conditions of the above application are easy to examine and the above problem has a solution in \varGamma .
5.
Application to electric circuit differential equation
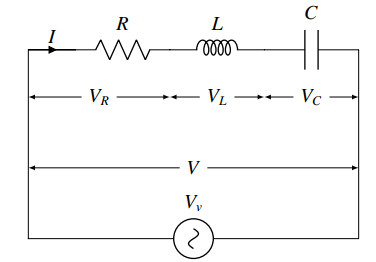
Let us consider a series electric circuit which contain a resistor ( \mathcal{R} , Ohms) a capacitor ( \mathcal{C} , Faradays), an inductor ( \mathcal{L} , Henries) a voltage ( \mathcal{V} , Volts) and an electromotive force ( \mathcal{E} , Volts), as in the following scheme, Figure 1.
Considering the definition of the intensity of electric current \mathcal{I} = \frac{d\mathcal{M}}{d\mathfrak{t}} , where \mathcal{M} denote the electric charge and \mathfrak{t} -the time, let us recall the following usual formulas
● \mathcal{V}_{\mathcal{R}} = \mathcal{I}\mathcal{R};
● \mathcal{V}_{\mathcal{C}} = \frac{\mathcal{M}}{\mathcal{C}};
● \mathcal{V}_{\mathcal{L}} = \mathcal{L}\frac{d\mathcal{I}}{d\mathfrak{t}}.
Since in a series circuit there is only one current flowing, then \mathcal{I} have the same value in the entire circuit. Kirchhoff's Voltage Law is the second of his fundamental laws we can use for circuit analysis. His voltage law states that for a closed loop series path the algebraic sum of all the voltages around any closed loop in a circuit is equal to zero. The Kirchhoff's Voltage Law states: "the algebraic sum of all the voltages around any closed loop in a circuit is equal to zero".
The main idea of the Kirchhoff's Voltage Law is that as you move around a closed loop/circuit, you will end up back where you started in the circuit. Therefore you back to the same initial potential without voltage losses around the loop. Therefore, any voltage drop around the loop must be equal to any voltage source encountered along the way. The mathematical expression of this consequence of the Kirchhoff's Voltage Law is: "the sum of the voltage rises across any loops is equal to the sum of voltage drops across that loop". Then we have the following relation:
We can write this voltage equation in the parameters of a second-order differential equation as follows.
where \mathcal{C} = \frac{4\mathcal{L}}{\mathcal{R}^{2}} and \tau = \frac{\mathcal{R}}{2\mathcal{L}} - the nondimensional time for the resonance case in Physics. The Green function associated with Eq (5.1) is the following:
The seccond order differential Eq (5.1) can be rewrite as the following integral equation by using the above conditions, we have
and \mathfrak{f} (\mathfrak{s}, \cdot) : [0, 1] \times \mathbb{R} \rightarrow \mathbb{R} is a monotone non decreasing mapping for all \mathfrak{s} \in [0, 1] .
Let \varGamma = (C[0, 1], \mathbb{R}) be the set of all continuous functions defined on [0, 1] . Define the binary relation \bot on \varGamma by \varrho\bot\mathcal{M} iff \varrho+\mathcal{M}\geq 0 and \varPsi, \varPhi, \mathcal{D}\colon\varGamma\times\varGamma\times(0, +\infty)\rightarrow [0, 1] by
and
for all \varrho, \mathcal{M}\in\varGamma with \varrho\bot\mathcal{M} and \zeta > 0 , continuous t-norm and continuous t-co-norm define by \mathfrak{e}\ast\flat = \mathfrak{e}\flat and \mathfrak{e}\circ\flat = \max\{\mathfrak{e}, \flat\} . Then (\varGamma, \varPsi, \varPhi, \mathcal{D}, \ast, \circ, \bot) is a complete orthogonal neutrosophic rectangular metric space. Further, let us give the main result of the section.
Theorem 5.1. Let \omega\colon\varGamma\rightarrow \varGamma be a mapping such that the following assertions hold:
(i) \mathcal{G}\colon[0, 1]^2\rightarrow [0, \infty) is a continuous function;
(ii) \mathfrak{f}(\mathfrak{s}, \cdot)\colon[0, 1]\times\mathbb{R}\rightarrow \mathbb{R} is a monotone non decreasing function for all \mathfrak{s}\in[0, 1] such that \varrho, \mathcal{M}\in\varGamma , we have the inequality:
(iii) \int_{0}^{\mathfrak{t}}\mathcal{G}(\mathfrak{t}, \mathfrak{s})d\mathfrak{s}\leq \sigma < 1.
Then the voltage differential Eq (5.1) has a unique solution.
Proof. Define \omega\colon\varGamma\rightarrow \varGamma by
Clearly \omega is an \bot -preserving. Now, for all \varrho, \mathcal{M}\in \varGamma with \varrho\bot\mathcal{M} , we deduce
and
Therefore, all the hypothesis of Theorem 3.3 are satisfied and \omega has a unique fixed-point and the differential voltage Eq (5.1) has a unique solution.
6.
Conclusions and future work
In this paper, we introduced the concept of orthogonal neutrosophic rectangular metric space and prove fixed point theorems. Recently, Khaelehoghli, Rahimi and Eshaghi Gordji [24,25] introduced R-metric spaces and obtained a generalization of Banach's fixed point theorem. It is an interesting open problem to study the relation R instead of orthogonal relation and obtained neutrosophic rectangular metric space results on R-complete neutrosophic rectangular metric spaces.
Conflict of interest
The authors declare no conflicts of interest.









 DownLoad:
DownLoad:



