Analytical solutions of incommensurate fractional differential equation systems with fractional order $ 1 &lt; \alpha, \beta &lt; 2 $ via bivariate Mittag-Leffler functions

Yong Xian Ng; Chang Phang; Jian Rong Loh; Abdulnasir Isah; Yong Xian Ng; Chang Phang; Jian Rong Loh; Abdulnasir Isah

doi:10.3934/math.2022130

AIMS Mathematics

2022, Volume 7, Issue 2: 2281-2317. doi: 10.3934/math.2022130

Previous Article Next Article

Research article

Analytical solutions of incommensurate fractional differential equation systems with fractional order $1 < \alpha, \beta < 2$ via bivariate Mittag-Leffler functions

1.
Department of Mathematics and Statistics, Faculty of Applied Sciences and Technology, Universiti Tun Hussein Onn Malaysia, Malaysia
2.
Foundation in Engineering, Faculty of Science and Engineering, University of Nottingham Malaysia, Semenyih, Selangor, Malaysia
3.
Department of Mathematics Education, Tishk International University, Erbil, Iraq
4.
Center for Computational Applied Mathematics, Faculty of Applied Sciences and Technology, Universiti Tun Hussein Onn Malaysia, Malaysia

Received: 03 September 2021 Accepted: 01 November 2021 Published: 10 November 2021
MSC : 34A08, 34A25, 26A33

In this paper, we derive the explicit analytical solution of incommensurate fractional differential equation systems with fractional order $1 < \alpha, \beta < 2$ . The derivation is extended from a recently published paper by Huseynov et al. in ^[1], which is limited for incommensurate fractional order $0 < \alpha, \beta < 1$ . The incommensurate fractional differential equation systems were first converted to Volterra integral equations. Then, the Mittag-Leffler function and Picard's successive approximations were used to obtain the analytical solution of incommensurate fractional order systems with $1 < \alpha, \beta < 2$ . The solution will be simplified via some combinatorial concepts and bivariate Mittag-Leffler function. Some special cases will be discussed, while some examples will be given at the end of this paper.

Keywords:

Citation: Yong Xian Ng, Chang Phang, Jian Rong Loh, Abdulnasir Isah. Analytical solutions of incommensurate fractional differential equation systems with fractional order $1 < \alpha, \beta < 2$ via bivariate Mittag-Leffler functions[J]. AIMS Mathematics, 2022, 7(2): 2281-2317. doi: 10.3934/math.2022130

Related Papers:

[1]	E. Bonyah, C. W. Chukwu, M. L. Juga, Fatmawati . Modeling fractional-order dynamics of Syphilis via Mittag-Leffler law. AIMS Mathematics, 2021, 6(8): 8367-8389. doi: 10.3934/math.2021485
[2]	Saima Rashid, Fahd Jarad, Fatimah S. Bayones . On new computations of the fractional epidemic childhood disease model pertaining to the generalized fractional derivative with nonsingular kernel. AIMS Mathematics, 2022, 7(3): 4552-4573. doi: 10.3934/math.2022254
[3]	Muhammad Altaf Khan, Muhammad Ismail, Saif Ullah, Muhammad Farhan . Fractional order SIR model with generalized incidence rate. AIMS Mathematics, 2020, 5(3): 1856-1880. doi: 10.3934/math.2020124
[4]	Rahat Zarin, Abdur Raouf, Amir Khan, Aeshah A. Raezah, Usa Wannasingha Humphries . Computational modeling of financial crime population dynamics under different fractional operators. AIMS Mathematics, 2023, 8(9): 20755-20789. doi: 10.3934/math.20231058
[5]	Ahu Ercan . Comparative analysis for fractional nonlinear Sturm-Liouville equations with singular and non-singular kernels. AIMS Mathematics, 2022, 7(7): 13325-13343. doi: 10.3934/math.2022736
[6]	Gulalai, Shabir Ahmad, Fathalla Ali Rihan, Aman Ullah, Qasem M. Al-Mdallal, Ali Akgül . Nonlinear analysis of a nonlinear modified KdV equation under Atangana Baleanu Caputo derivative. AIMS Mathematics, 2022, 7(5): 7847-7865. doi: 10.3934/math.2022439
[7]	Sabri T. M. Thabet, Reem M. Alraimy, Imed Kedim, Aiman Mukheimer, Thabet Abdeljawad . Exploring the solutions of a financial bubble model via a new fractional derivative. AIMS Mathematics, 2025, 10(4): 8587-8614. doi: 10.3934/math.2025394
[8]	Mohammed A. Almalahi, Satish K. Panchal, Fahd Jarad, Mohammed S. Abdo, Kamal Shah, Thabet Abdeljawad . Qualitative analysis of a fuzzy Volterra-Fredholm integrodifferential equation with an Atangana-Baleanu fractional derivative. AIMS Mathematics, 2022, 7(9): 15994-16016. doi: 10.3934/math.2022876
[9]	Fatmawati, Muhammad Altaf Khan, Ebenezer Bonyah, Zakia Hammouch, Endrik Mifta Shaiful . A mathematical model of tuberculosis (TB) transmission with children and adults groups: A fractional model. AIMS Mathematics, 2020, 5(4): 2813-2842. doi: 10.3934/math.2020181
[10]	M. Mossa Al-Sawalha, Osama Y. Ababneh, Rasool Shah, Amjad khan, Kamsing Nonlaopon . Numerical analysis of fractional-order Whitham-Broer-Kaup equations with non-singular kernel operators. AIMS Mathematics, 2023, 8(1): 2308-2336. doi: 10.3934/math.2023120

Abstract

1. Introduction

To show the dynamics of an epidemic, the susceptible-infected-recovered (SIR) fractional model was proposed. Despite the fact that the SIR model is approaching its centenary and its wholly analytical solutions have just been computed, the ADM represents one of them. The SIR model gives details and predictions on the spread of a virus in societies that recorded data alone cannot. This model is a system of FDEs. FDEs have numerous applications in science and engineering: for example, electrical networks ^[1,2], control theory, fluid flow, fractal theory ^[3,4], electromagnetic theory ^[5,6,7], chemistry, optical and neural network systems, potential theory, and biology. The ADM ^[8,9] was used in this study to solve a critical model of arbitrary orders: the SIR epidemic model. This technique has several benefits: It efficiently solves various classes of equations, whether they are linear or nonlinear, in stochastic and deterministic areas, and provides an analytical solution with no discretization or linearization ^{[10,11,12,13]}.

In recent works, the SIR model involving the Riemann-Liouville derivative and CD for childhood disease has been proposed ^[14]. There, the authors used the homotopy analysis method to derive a numerical solution for their model. In ^[15], the solution for the SIR model involving time fractional CD by utilizing the Laplace ADM (LADM) was illustrated graphically for unequal values of order $\alpha$ , which showed that the recovered population increases with a decreasing rate of infection in the population. In ^[13], the author proposed an epidemic model and used the fractional interpolated variation iteration method to find an efficient approximate solution. In ^[16], numerical solutions for the SIR model involving CD were obtained by using the Adams-type predictor-corrector method. In ^[17], the authors considered the fractional-order SIR epidemic model involving conformable FDs and used the conformable differential transform method (CFDTM) to calculate an approximate solution that is in the form of a rapidly convergent series. In ^[18], they found the numerical solutions for the epidemic model involving fractional CD using the Euler method. In ^[19], the author considered the time fractional epidemic model of childhood disease involving fractional CD and presented the numerical results by using the Adams-type implicit fractional linear multistep method. In ^[20], the author presented an epidemic model of non-fatal disease involving fractional CD and computed the analytical solution for the corresponding system of nonlinear FDEs by applying the LADM. The SIR model in CD was solved using the residual power series in ^[21]. In ^[22], the SIR epidemic model with the Mittag-Leffler fractional derivative was discussed. In ^[23], a fractional SIR model with delay in the context of the generalized Liouville-Caputo fractional derivative was given. The stability and equilibrium points of this model when containing CD were discussed before in many works, such as ^{[24,25,26,27,28]}.

In this research, the SIR model was used to solve problems involving three different FDs: CFD, ABD, and CD derivatives. A comparison between the solutions of the SIR system, containing these three derivatives, is given. Important applications of ABD and CFD can be found in ^[29,30].

This research is organized as follows: In Section 2, the main definitions are given. In Section 3, the first definition (CFD) is discussed. Then, in Section 4, the second definition (CD) is given. In Section 5, the third definition (ABD) is discussed. In Section 6, a comparison between these three different definitions is discussed. Finally, in Section 7, a conclusive summary to this research is given. All the results of the SIR model are obtained and compared to all of these using the fourth order Runge-Kutta 4 (RK4) solution from the Mathematica 5.2 package.

2. Main definitions

The definitions of the three fractional derivatives used in this research and the main properties and advantages of using each definition are given here.

(1) The definition of the CD of order $\upsilon$ is ^[31]

$\begin{equation} _{0}^{C}D_{t}^{\upsilon }f(t) = \frac{1}{\Gamma (n-\upsilon )}\int_{0}^{t} \frac{f\text{ }^{\left( n\right) }(\tau )d\tau }{(t-\tau )^{\upsilon -n+1}} , \quad n-1 < \upsilon < n. \end{equation}$

(2.1)

Its corresponding fractional integral (FI) is ^[31]

$\begin{equation} ^{C}I^{\upsilon }\digamma \left( t\right) = \frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\digamma (\tau )\left( t-\tau \right) ^{\upsilon -1}d\tau , { \ \ }0 < \upsilon < 1. \end{equation}$

(2.2)

Moreover,

$\begin{equation} \left( ^{C}I^{\upsilon }\right) \left( ^{C}D^{\upsilon }\right) \digamma \left( t\right) = \digamma \left( t\right) -\digamma \left( a\right) . \end{equation}$

(2.3)

This definition is considered one of the classical FDs and is one of the most well known and famous FDs, as follows ^[31]:

ⅰ) Its main advantage is that the initial conditions for FDEs containing CD take an identical form as for integer-order DEs, such as

$\begin{equation} f^{^{\text{ }\prime }}(a), f^{\text{ }^{\prime \prime }}(a), \cdots , \end{equation}$

(2.4)

and they have well-known physical meaning.

ⅱ) The derivative of a constant by using the Caputo definition is equal to zero, whereas the Riemann-Liouville derivative of a constant is not equal to zero, as follows:

$\begin{equation} _{0}^{RL}D_{t}^{\upsilon }\left( C\right) = \frac{Ct^{-\upsilon }}{\Gamma \left( 1-\upsilon \right) }. \end{equation}$

(2.5)

So, the properties of this definition coincide with the properties of the integer-order derivative definition.

(2) The definition of the CFD of order $\upsilon$ is ^[30]

$\begin{equation} ^{CF}D_{a}^{\upsilon }\digamma \left( t\right) = \frac{B\left( \upsilon \right) }{1-\upsilon }\int_{a}^{t}\exp \left( \frac{-\upsilon \left( t-\tau \right) }{1-\upsilon }\right) \digamma ^{\prime }(s)ds, \end{equation}$

(2.6)

where the normalization function $B(\upsilon)$ $> 0$ satisfies $B(0) = B(1) = 1$ . Its corresponding FI is ^[32]

$\begin{equation} ^{CF}I_{a}^{\upsilon }\digamma \left( t\right) = \frac{1-\upsilon }{B\left( \upsilon \right) }\digamma \left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) }\int_{a}^{t}\digamma (s)ds, \ { \ \ }\upsilon \in (0, 1), \end{equation}$

(2.7)

where

$\begin{equation} \left( ^{CF}I_{a}^{\upsilon }\right) \left( ^{CF}D_{a}^{\upsilon }\right) \digamma \left( t\right) = \digamma \left( t\right) -\digamma \left( a\right) . \end{equation}$

(2.8)

The main advantage of using this definition is that there is no singularity in the definition, as shown in (2.6) and (2.7).

(3) The ABD of order $\upsilon$ of $\digamma (t)$ is ^[32]

$\begin{equation} ^{AB}D^{\upsilon }\digamma \left( t\right) = \frac{B\left( \upsilon \right) }{ 1-\upsilon }\int_{0}^{t}E_{\upsilon }\left( \frac{-\upsilon \left( t-s\right) }{1-\upsilon }\right) ^{\upsilon }\digamma ^{\prime }(s)ds, \end{equation}$

(2.9)

where $E_{\upsilon }$ is the Mittag-Leffler function of one variable, and its reduced FI is ^[9]

$\begin{equation} ^{AB}I^{\upsilon }\digamma \left( t\right) = \frac{1-\upsilon }{B\left( \upsilon \right) }\digamma \left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\digamma (s)\left( t-s\right) ^{\upsilon -1}ds, \ { \ \ }0 < \upsilon < 1. \end{equation}$

(2.10)

Also,

$\begin{equation} \left( ^{AB}I^{\upsilon }\right) \left( ^{AB}D^{\upsilon }\right) \digamma \left( t\right) = \digamma \left( t\right) -\digamma \left( a\right) . \end{equation}$

(2.11)

In this research, we aim to see which one of the two new FDs (CFD and ABD) is closer to the classical fractional CD because we can deduce the properties of the integer derivatives from the properties of the CD, as we can see from the relations (2.4) and (2.5).

3. First definition: Caputo-Fabrizio derivative (CFD)

The general form of the nonlinear FDE system with the CFD takes the following form:

$\begin{equation} ^{CF}D_{t}^{\upsilon }y_{k}\left( t\right) +h_{k}\left( t\right) \text{ } f_{k}\left( \overline{y}\right) = \chi _{k}\left( t\right) , \end{equation}$

(3.1)

subject to

$\begin{equation} y_{k}^{(j-1)}(0) = c_{k}, \ { \ }k, j = 1, 2, \ldots , n, \end{equation}$

(3.2)

and

$\begin{equation*} \overline{y} = \{y_{1}(t), y_{2}(t), \ldots , y_{n}(t)\}, \ \ 0 < \upsilon < 1. \end{equation*}$

The SIR epidemic model is a special case of this system. Now, taking the FI (2.7) of order $\upsilon$ , and letting $a = 0$ with the system (3.1)-(3.2), we get

$\begin{eqnarray} y_{k}\left( t\right) = c_{k}+\frac{1-\upsilon }{B\left( \upsilon \right) } \chi _{k}\left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}\chi _{k}(s)ds -\frac{1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) f_{k}\left( \overline{y}\right) -\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{k}\left( s\right) f_{k}\left( \overline{y}\right) ds. \end{eqnarray}$

(3.3)

Let $\chi _{k}(t)$ be bounded $\forall t\in J = [0, T], \, T\in R^{+}$ , $\left\vert h_{k}(\tau)\right\vert \leq M_{k}$ for all $0\leq \tau \leq t\leq T, \ M_{k}$ be finite constants, and $f_{k}(\overline{y})$ satisfy the Lipschitz condition, having Lipschitz constants $L_{k}$ as

$\begin{equation} \left\vert f_{k}(\overline{y})-f_{k}(\overline{z})\right\vert \leq L_{k}\left\vert \overline{y}-\overline{z}\right\vert . \end{equation}$

(3.4)

Furthermore, it has Adomian polynomials (APs) presented as

$\begin{equation} f_{k}(\overline{y}) = \sum\limits_{m = 0}^{\infty }A_{km}(y_{k0}, y_{k1}, \ldots , y_{kn}), \end{equation}$

(3.5)

where

$\begin{equation} A_{km} = \frac{1}{m!}\frac{d^{m}}{d\lambda ^{m}}\left[ f_{k}\left( \sum\limits_{j = 0}^{\infty }\lambda ^{j}y_{j}\right) \right] _{\lambda = 0}. \end{equation}$

(3.6)

Substituting (3.5) into (3.3), we get

$\begin{eqnarray} y_{k}\left( t\right) = c_{k}+\frac{1-\upsilon }{B\left( \upsilon \right) } \chi _{k}\left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}\chi _{k}(s)ds -\frac{1-\upsilon }{B\left( \upsilon \right) }h_{i}\left( t\right) \sum\limits_{i = 0}^{\infty }A_{ki}-\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{k}\left( s\right) \sum\limits_{i = 0}^{\infty }A_{ki}\, ds. \end{eqnarray}$

(3.7)

Let $y_{k}(t) = \sum\limits_{i = 0}^{\infty }y_{ki}(t)$ in (3.7). Then,

$\begin{eqnarray} y_{k0}(t) & = &c_{k}+\frac{1-\upsilon }{B\left( \upsilon \right) }\chi _{k}\left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}\chi _{k}(s)ds, \end{eqnarray}$

(3.8)

$\begin{eqnarray} y_{ki}(t) & = &-\frac{1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) A_{k(i-1)}-\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{i}\left( s\right) A_{k(i-1)}ds, \quad k\geq 1. \end{eqnarray}$

(3.9)

The final solution will be

$\begin{equation} y_{k}(t) = \sum\limits_{i = 0}^{\infty }y_{ki}(t). \end{equation}$

(3.10)

3.1. Convergence

3.1.1. Existence of a unique solution

Take the mapping $\Psi :\Omega \rightarrow \Omega$ , where $\Omega$ is the Banach space ( $C^{\left(n\right) }\left(J\right), \left\Vert \cdot \right\Vert$ ). $C^{\left(n\right) }\left(J\right)$ is a class of continuous column vectors $Y = \left(\overline{y}\right) ^{\prime }$ taking norm $\left\Vert Y\right\Vert = \sum\limits_{k = 1}^{n}\underset{t\in J}{\max } \left\vert y_{k}(t)\right\vert$ , and $\left(.\right) ^{\prime }$ is the matrix transpose.

Theorem 3.1. There exists a unique solution to the system (3.1)-(3.2) at $0 < \phi < 1$ , $\phi = \frac{LMT}{ B\left(\upsilon \right) }$ , where $L = \sum\limits_{m = 1}^{n}L_{m}$ , $M = \max \left\{ M_{1}, M_{2}, \ldots, M_{n}\right\}$ .

Proof. Rewrite Eq (3.7) as

$\begin{eqnarray*} Y\left( t\right) = C+\frac{1-\upsilon }{B\left( \upsilon \right) }\chi \left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) }\int_{0}^{t}\chi (s)ds -\frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) F\left( \overline{y}\right) -\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}H\left( s\right) F\left( \overline{y}\right) ds, \end{eqnarray*}$

where

$\begin{eqnarray*} C & = &\left( c_{1}, c_{2}, \ldots , c_{n}\right) ^{\prime }, \\ \chi (t) & = &\left( \chi _{1}, \chi _{2}, \ldots , \chi _{n}\right) ^{\prime }, \\ H\left( t\right) & = &diag\left\{ h_{1}, h_{2}, \ldots , h_{n}\right\} , \\ F(\overline{y}\left( t\right) ) & = &\left( f_{1}\left( \overline{y}\right) , f_{2}\left( \overline{y}\right) , \ldots , f_{n}\left( \overline{y}\right) \right) ^{\prime }. \end{eqnarray*}$

The mapping $\Psi :\Omega \rightarrow \Omega$ is defined as

$\begin{eqnarray*} \Psi Y\left( t\right) = C+\frac{1-\upsilon }{B\left( \upsilon \right) } \chi \left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}\chi (s)\text{ }ds -\frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) F\left( \overline{y}\right) -\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}H\left( s\right) F\left( \overline{y}\right) ds. \end{eqnarray*}$

Let $Y, Z\in \Omega$ .

$\begin{eqnarray*} \left\Vert \Psi Y\left( t\right) -\Psi Z\left( t\right) \right\Vert & = &\left\Vert -\frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) \left( F(\overline{y})-F(\overline{z})\right) -\frac{\upsilon }{B\left( \upsilon \right) }\int_{0}^{t}H\left( s\right) \left( F(\overline{y})-F( \overline{z})\right) ds\right\Vert \\ &\leq &\left\Vert \frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) \left( F(\overline{y})-F(\overline{z})\right) \right\Vert +\left\Vert \frac{\upsilon }{B\left( \upsilon \right) }\int_{0}^{t}H\left( s\right) \left( F(\overline{y})-F(\overline{z})\right) ds\right\Vert \\ &\leq &\frac{1-\upsilon }{B\left( \upsilon \right) }\left\Vert H\left( t\right) \right\Vert \left\Vert F(\overline{y})-F(\overline{z})\right\Vert + \frac{\upsilon }{B\left( \upsilon \right) }\int_{0}^{t}\left\Vert H\left( s\right) \right\Vert \left\Vert F(\overline{y})-F(\overline{z})\right\Vert ds \\ &\leq &\frac{\left( 1-\upsilon \right) M}{B\left( \upsilon \right) }\left( \sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert f_{m}(\overline{y} )-f_{m}(\overline{z})\right\vert \right) +\frac{\upsilon M}{B\left( \upsilon \right) }\int_{0}^{t}\left( \sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert f_{m}(\overline{y} )-f_{m}(\overline{z})\right\vert \right) ds \\ &\leq &\frac{M}{B\left( \upsilon \right) }\left( \sum\limits_{m = 1}^{n} \underset{t\in J}{\max }\left\vert f_{m}(\overline{y})-f_{m}(\overline{z} )\right\vert \right) \left[ \left( 1-\upsilon \right) +\upsilon \int_{0}^{t}ds\right] \\ &\leq &\frac{M}{B\left( \upsilon \right) }\left[ 1-\upsilon +\upsilon T \right] \sum\limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{M\left[ 1+\upsilon \left( T-1\right) \right] }{B\left( \upsilon \right) }\sum\limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{MT}{B\left( \upsilon \right) }\sum\limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \leq \frac{LMT}{B\left( \upsilon \right) }\left\Vert Y-Z\right\Vert \\ &\leq &\phi \left\Vert Y-Z\right\Vert. \end{eqnarray*}$

If $0 < \phi < 1$ , the mapping $\Psi$ will be a contraction, so, there is a unique solution to the system (3.1)-(3.2). □

3.1.2. Convergence proof

Theorem 3.2. The series solution (3.10) will be convergent if $\left\vert y_{k1}\right\vert < \infty$ and $0 < \phi < 1, \, \phi = \frac{LMT}{ B\left(\upsilon \right) }$ , as $L = \sum\limits_{k = 1}^{n}L_{k}$ , $M = \max \left\{ M_{1}, M_{2}, \ldots, M_{n}\right\}$ .

Proof. Take a sequence $\left\{ S_{kr}\right\}$ such that $S_{kr} = \sum \limits_{i = 0}^{r}y_{ki}(t)$ is partial sums sequence of $\sum\limits_{i = 0}^{\infty }y_{ki}(t)$ . We have

$\ f(S_{kr}) = \sum\limits_{i = 0}^{r}A_{ki}(y_{k0}, y_{k1}, \ldots , y_{kr}).$

If $S_{kr}$ and $S_{kw}$ are two partial sums where $r > w$ , our goal is to prove that $\left\{ S_{ir}\right\}$ is a Cauchy sequence in this Banach space.

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert & = &\sum\limits_{i = 1}^{n}\underset{t\in J }{\max }\left\vert S_{ir}-S_{iw}\right\vert = \sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \sum\limits_{j = w+1}^{r}y_{ij}(t)\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \sum\limits_{j = w+1}^{r}\frac{1-\upsilon }{B\left( \upsilon \right) } h_{k}\left( t\right) A_{k(i-1)}+\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{k}\left( s\right) A_{k(i-1)}ds\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) \sum\limits_{j = w+1}^{r}A_{i(j-1)}+\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{k}\left( s\right) \sum\limits_{j = w+1}^{r}A_{i(j-1)}ds\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) \sum\limits_{j = w}^{r-1}A_{ij}+\frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{k}\left( s\right) \sum\limits_{j = w}^{r-1}A_{ij}ds\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]\right. \\ &&\left. +\frac{\upsilon }{B\left( \upsilon \right) }\int_{0}^{t}h_{k}\left( s\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]ds\right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left[ \left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]\right\vert \right. \\ &&\left. +\left\vert \frac{\upsilon }{B\left( \upsilon \right) } \int_{0}^{t}h_{k}\left( s\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]ds\right\vert \right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left[ \frac{1-\upsilon }{B\left( \upsilon \right) }\left\vert h_{k}\left( t\right) \right\vert \left\vert f(S_{i(r-1)})-f(S_{i(w-1)})\right\vert \right. \\ &&\left. +\frac{\upsilon }{B\left( \upsilon \right) }\int_{0}^{t}\left\vert h_{k}\left( s\right) \right\vert \left\vert f(S_{i(r-1)})-f(S_{i(w-1)})\right\vert ]ds\right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left[ \frac{1-\upsilon }{B\left( \upsilon \right) }\left\vert h_{k}\left( t\right) \right\vert \left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) \right. \\ &&\left. +\frac{\gamma }{B\left( \gamma \right) }\int_{0}^{t}\left\vert h_{k}\left( s\right) \right\vert \left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) ds\right] \\ &\leq &\sum\limits_{k = 1}^{n}\underset{t\in J}{\max }\left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) \left[ \frac{1-\upsilon }{B\left( \upsilon \right) }M+\frac{\upsilon M}{B\left( \upsilon \right) }\int_{0}^{t}ds\right] \\ &\leq &\sum\limits_{k = 1}^{n}\underset{t\in J}{\max }\left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) \left[ \frac{1-\upsilon }{B\left( \upsilon \right) }M+\frac{\upsilon M}{B\left( \upsilon \right) }\int_{0}^{t}ds\right] \\ &\leq &\frac{LMT}{B\left( \upsilon \right) }\left\Vert S_{k(r-1)}-S_{k(w-1)}\right\Vert \\ &\leq &\phi \left\Vert S_{k(r-1)}-S_{k(w-1)}\right\Vert . \end{eqnarray*}$

Let $r = w+1$ . Then,

$\left\Vert S_{k(w+1)}-S_{kw}\right\Vert \leq \phi \left\Vert S_{kw}-S_{k(w-1)}\right\Vert \leq \phi ^{2}\left\Vert S_{k(w-1)}-S_{k(w-2)}\right\Vert \leq \cdots \leq \phi ^{w}\left\Vert S_{k1}-S_{k0}\right\Vert.$

From the triangle inequality, we get

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert &\leq &\left\Vert S_{k(w+1)}-S_{kw}\right\Vert +\left\Vert S_{k(w+2)}-S_{k(w+1)}\right\Vert +\cdots +\left\Vert S_{kr}-S_{k(r-1)}\right\Vert \\ \ &\leq &\left[ \phi ^{w}+\phi ^{w+1}+\cdots +\phi ^{r-1}\right] \left\Vert S_{k1}-S_{k0}\right\Vert \\ &\leq &\phi ^{w}\left[ 1+\phi +\cdots +\phi ^{r-w-1}\right] \left\Vert S_{k1}-S_{k0}\right\Vert \\ \ &\leq &\phi ^{q}\left[ \frac{1-\phi ^{r-w}}{1-\phi }\right] \left\Vert y_{k1}(t)\right\Vert . \end{eqnarray*}$

Since $0 < \phi < 1$ and $r > w$ , $(1-\phi ^{r-w})\leq 1$ . Hence,

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\beta } \left\Vert y_{k1}(t)\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert . \end{eqnarray*}$

If $\left\vert y_{k1}(t)\right\vert < \infty$ and $q\rightarrow \infty$ , then $\left\Vert S_{kr}-S_{kw}\right\Vert \rightarrow 0$ . So, $\left\{ S_{kr}\right\}$ will be a Cauchy sequence in this Banach space, and the series $\sum\limits_{i = 0}^{\infty }y_{ki}(t)$ will converge. □

3.1.3. Error estimation

Theorem 3.3. For the series solution (3.10), the maximum absolute error can be estimated as

$\begin{equation*} \underset{t\in J}{\max }\left\vert y_{k}(t)-\sum\limits_{i = 0}^{w}y_{ki}(t)\right\vert \leq \frac{\phi ^{w}}{ 1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert . \end{equation*}$

Proof. Using Theorem 3.2, we get

$\ \left\Vert S_{kr}-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset {t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

However, $S_{ir} =$ $\sum\limits_{i = 0}^{r}y_{ki}(t)$ as $r\rightarrow \infty$ . Then, $S_{kr}\rightarrow y_{k}(t)$ and we get

$\left\Vert y_{k}(t)-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset {t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

So, the maximum absolute error will be

$\underset{t\in J}{\max }\left\vert y_{k}(t)-\sum\limits_{i = 0}^{w}y_{ki}(t)\right\vert \leq \frac{\phi ^{w}}{ 1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

3.2. SIR epidemic model of arbitrary orders with CFD

The simple epidemic model of arbitrary orders of a dangerous sickness in a wide range of populations, known as the SIR model, was presented in ^{[14,33,34,35,36,37]}, by considering that the populace consists of three types of individuals: susceptible ( $S$ ), referring to individuals who are not infected although they can be severely affected in an easy way; infected ( $I$ ), the individual individuals who carry the diseases and are able to transmit the sickness to the susceptible; and recovered ( $R$ ). The formal SIR model is presented as

$\begin{eqnarray} \frac{dS}{dt} & = &\left( 1-\eta \right) \pi -\varphi SI-\pi S, \\ \frac{dI}{dt} & = &\varphi SI-\left( \gamma +\pi \right) I, \\ \frac{dR}{dt} & = &\eta \pi +\gamma I-\pi R. \end{eqnarray}$

(3.11)

This model assumes that immunization is completely effective. Individuals are added to the population with a constant birth rate, and there is an extremely low youth sickness death rate. Every year, the proportion of citizens immunized at childbirth is expressed as $\eta$ . Infected individuals are approximated by constant rate $\varphi$ and recover at a rate $\gamma$ from infection.

The SIR epidemic model of arbitrary orders containing CFDs is

$\begin{eqnarray} ^{CF}D_{0}^{\alpha }S\left( t\right) & = &\left( 1-p\right) \pi -\beta SI-\pi S, \\ ^{CF}D_{0}^{\alpha }I\left( t\right) & = &\beta SI-\left( \gamma +\pi \right) I, \\ ^{CF}D_{0}^{\alpha }R\left( t\right) & = &p\pi +\gamma I-\pi R, \end{eqnarray}$

(3.12)

subject to

$\begin{equation*} S(0) = N_{1}, \ I(0) = N_{2}, \ R(0) = N_{3}. \end{equation*}$

Using the ADM on system (3.11), we get the ADM recurrence relation as

$\begin{eqnarray} S_{0} & = &N_{1}+\text{ }^{CF}I^{\alpha }\left( 1-p\right) \pi , \quad S_{j+1} = -\beta \text{ }^{CF}I^{\alpha }(A_{j})-\pi \text{ }^{CF}I^{\alpha }\left( S_{j}\right) , \end{eqnarray}$

(3.13)

$\begin{eqnarray} I_{0} & = &N_{2}, \quad I_{j+1} = \beta \text{ }^{CF}I^{\alpha }\left( A_{j}\right) -\text{ }^{CF}I^{\alpha }\left[ \left( \gamma +\pi \right) I_{j} \right] , \end{eqnarray}$

(3.14)

$\begin{eqnarray} R_{0} & = &N_{3}+\text{ }^{CF}I^{\alpha }\left( p\pi \right) +, \quad R_{j+1} = \gamma \text{ }^{CF}I^{\alpha }\left( I_{j}\right) -\pi \text{ } ^{CF}I^{\alpha }\left( R_{j}\right) . \end{eqnarray}$

(3.15)

The series solution is

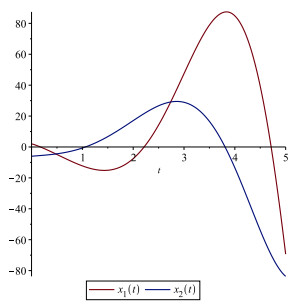
$\begin{equation} S(t) = \sum\limits_{k = 0}^{n}S_{k}(t), I(t) = {\ }\sum\limits_{k = 0}^{n}I_{k}(t), \text{ and } R(t) = {\ }\sum\limits_{k = 0}^{n}R_{k}(t). \end{equation}$

(3.16)

– show the ADM solution of the SIR system when $\alpha = 1, 0.95, 0.85, 0.75$ and $n = 5$ .

Figure 1. ADM solution of

$S\left(t\right)$ with CFD.

DownLoad: Full-Size Img PowerPoint

Figure 2. ADM solution of

$I\left(t\right)$ with CFD.

DownLoad: Full-Size Img PowerPoint

Figure 3. ADM solution of

$R\left(t\right)$ with CFD.

DownLoad: Full-Size Img PowerPoint

– show the absolute differences (ADs) between the ADM and RK4 solutions for the SIR system at $\alpha = 1$ , respectively.

Table 1. ADM and RK4 solutions of

$S(t)$ .

$t$	Solution of ADM	Solution of RK4	AD
$0$	1	1	0
$0.1$	0.949	0.949	0.00004
$0.2$	0.901	0.901	4 $\times 10^{-6}$
$0.3$	0.854	0.854	0.00001
$0.4$	0.810	0.810	0.00004
$0.5$	0.768	0.768	0.00003
$0.6$	0.729	0.729	0.00006
$0.7$	0.691	0.691	4 $\times 10^{-6}$
$0.8$	0.655	0.655	0.00008
$0.9$	0.621	0.621	0.0003

| Show Table

DownLoad: CSV

Table 2. ADM and RK4 solutions of

$I(t)$ .

$t$	Solution of ADM	Solution of RK4	AD
$0$	0.2	0.2	0
$0.1$	0.207	0.207	0.00001
$0.2$	0.214	0.214	0.00003
$0.3$	0.2195	0.2195	0.00002
$0.4$	0.225	0.225	0.00005
$0.5$	0.22933	0.229	0.00003
$0.6$	0.233	0.233	0.00004
$0.7$	0.237	0.237	4 $\times 10^{6}$
$0.8$	0.239	0.244	0.005
$0.9$	0.241	0.239	0.002

| Show Table

DownLoad: CSV

Table 3. ADM and RK4 solutions of

$R(t)$ .

$t$	Solution of ADM	Solution of RK4	AD
$0$	0	0	0
$0.1$	0.03589	0.0359	0.00001
$0.2$	0.0704	0.0704	0.00001
$0.3$	0.1036	0.1036	0.00004
$0.4$	0.1354	0.1354	0.00004
$0.5$	0.1661	0.1661	0.00002
$0.6$	0.1955	0.1955	0.00004
$0.7$	0.2239	0.2229	0.00096
$0.8$	0.2511	0.2511	0.00003
$0.9$	0.2772	0.2772	0.00002

| Show Table

DownLoad: CSV

From –, for $\alpha = 1$ , ADM and RK4 have close values as shown from the values of ADs between them.

shows the ADM solution of the SIR system at $\alpha = 0.5$ and $n = 5$ .

Figure 4. ADM solution of the SIR Model with CFD.

DownLoad: Full-Size Img PowerPoint

Figure 4 shows that the susceptible population decreases, whereas the infected population and the recovered population increase for a long time. In –, we see the effect of using different values of $\alpha$ on the SIR system.

4. Second definition: Caputo derivative (CD)

The general form of the nonlinear FDE system with a CD is

$\begin{equation} ^{c}D_{t}^{\upsilon }y_{k}\left( t\right) +h_{k}\left( t\right) f_{k}\left( \overline{y}\right) = \chi _{k}\left( t\right) , \end{equation}$

(4.1)

with

$\begin{equation} y_{k}^{(j-1)}(0) = c_{kj}, \ \quad k, j = 1, 2, \ldots , n, \end{equation}$

(4.2)

$\begin{equation} \overline{y} = \{y_{1}(t), y_{2}(t), \ldots , y_{n}(t)\}, \ \quad 0 < \upsilon < 1. \end{equation}$

(4.3)

The SIR epidemic model is a special case of this system. Applying fractional integration of order $\upsilon$ , this minimizes (4.1)-(4.2) in the system of fractional integral equations (FIEs).

$\begin{eqnarray} y_{k}\left( t\right) = \sum\limits_{j = 1}^{m}\frac{c_{kj}}{\Gamma \left( \upsilon \right) }t^{\upsilon -1}+\frac{1}{\Gamma \left( \upsilon \right) } \int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\chi _{k}(\tau )d\tau -\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) f_{k}\left( \overline{y} \right) d\tau . \end{eqnarray}$

(4.4)

Assume that $\chi _{k}(t)$ is bounded, $\forall \ t\in J = [0, T], \, T\in R^{+}$ , $\left\vert h_{k}(\tau)\right\vert \leq K_{k}, \ \forall \ 0\leq \tau \leq t\leq T, \ K_{k}$ are finite constants, and $f_{k}(\overline{y})$ satisfy the Lipschitz condition with Lipschitz constants $L_{k}$ as

$\begin{equation} \left\vert f_{k}(\overline{y})-f_{k}(\overline{z})\right\vert \leq L_{k}\left\vert \overline{y}-\overline{z}\right\vert . \end{equation}$

(4.5)

Substituting (3.5) into (4.4), we have

$\begin{eqnarray} y_{k}\left( t\right) = \sum\limits_{j = 1}^{m}\frac{c_{kj}}{\Gamma \left( \upsilon \right) }t^{\upsilon -1}+\frac{1}{\Gamma \left( \upsilon \right) } \int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\chi _{k}(\tau )d\tau -\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) \sum\limits_{m = 0}^{\infty }A_{km}d\tau .\ { \ \ \ \ \ } \end{eqnarray}$

(4.6)

Let $y_{k}(t) = \sum\limits_{m = 0}^{\infty }x_{km}(t)$ substitute in (4.6) and we get

$\begin{eqnarray} y_{k0}(t) & = &\sum\limits_{j = 1}^{m}\frac{c_{kj}}{\Gamma \left( \upsilon \right) } t^{\upsilon -1}+\frac{1}{\Gamma \left( \beta \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\chi _{k}(\tau )d\tau , \\ y_{km}(t) & = &-\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) \sum\limits_{m = 0}^{\infty }A_{k(m-1)}d\tau , \quad m\geq 1.\ { \ \ \ \ \ } \end{eqnarray}$

(4.7)

The final solution will be

$\begin{equation} y_{k}(t) = \sum\limits_{m = 0}^{\infty }y_{km}(t). \end{equation}$

(4.8)

5. Convergence

5.1. Existence of a unique solution

Take the mapping $\Psi :\Omega \rightarrow \Omega$ . $\Omega$ is the Banach space ( $C^{\left(n\right) }\left(J\right), \left\Vert \cdot \right\Vert$ ), where $C^{\left(n\right) }\left(J\right)$ is the class of continuous column vectors $Y = \left(\overline{y}\right) ^{\prime }$ with $\left\Vert Y\right\Vert = \sum\limits_{m = 1}^{n}\underset{t\in J}{\max } \left\vert y_{m}(t)\right\vert$ , and $\left(.\right) ^{\prime }$ denotes the matrix transpose.

Theorem 5.1. The system (4.1)-(4.2) has a unique solution when $0 < \phi < 1\mathit{, }$ $\phi = \frac{KLT^{\upsilon }}{ \upsilon \Gamma \left(\upsilon \right) }$ , {where } $L = \sum\limits_{m = 1}^{n}L_{m}\mathit{}$ , $K = \max \left\{ K_{1}, K_{2}, \ldots, K_{n}\right\}$ .

Proof. Equation (4.4) can be described as

$\begin{eqnarray*} Y\left( t\right) = A+\frac{1}{\Gamma \left( \upsilon \right) } \int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}G(\tau )d\tau -\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}H\left( \tau \right) F\left( \overline{y}\right) d\tau , \end{eqnarray*}$

where

$\begin{eqnarray*} A & = &\left( a_{1}, a_{2}, \ldots , a_{n}\right) ^{\prime }, \\ Y(t) & = &\left( y_{1}, y_{2}, \ldots , y_{n}\right) ^{\prime }, \\ H\left( t\right) & = &diag\left\{ h_{1}, h_{2}, \ldots , h_{n}\right\} , \\ F(\overline{y}\left( t\right) ) & = &\left( f_{1}\left( \overline{y}\right) , f_{2}\left( \overline{y}\right) , \ldots , f_{n}\left( \overline{y}\right) \right) ^{\prime }. \end{eqnarray*}$

Let $X, Z\in \Omega$ .

$\begin{eqnarray*} \left\Vert \Psi Y\left( t\right) -\Psi Z\left( t\right) \right\Vert & = &\left\Vert -\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}H\left( \tau \right) \left( F(\overline{y})-F( \overline{z})\right) d\tau \right\Vert \\ &\leq &\left\Vert \frac{1}{\Gamma \left( \upsilon \right) } \int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}H\left( \tau \right) \left( F(\overline{y})-F(\overline{z})\right) d\tau \right\Vert \\ &\leq &\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\left\Vert H\left( \tau \right) \right\Vert \left\Vert F(\overline{y})-F(\overline{z})\right\Vert d\tau \\ &\leq &\frac{K}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\left( \sum\limits_{m = 1}^{n}\underset{t\in J}{\max } \left\vert f_{m}(\overline{y})-f_{m}(\overline{z})\right\vert \right) d\tau \\ &\leq &\frac{K}{\Gamma \left( \upsilon \right) }\left( \sum\limits_{m = 1}^{n} \underset{t\in J}{\max }\left\vert f_{m}(\overline{y})-f_{m}(\overline{z} )\right\vert \right) \int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}d\tau \\ &\leq &\frac{KT^{\upsilon }}{\Gamma \left( \upsilon \right) } \sum\limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{KLT^{\upsilon }}{\upsilon \Gamma \left( \upsilon \right) } \left\Vert Y-Z\right\Vert \\ &\leq &\phi \left\Vert Y-Z\right\Vert . \end{eqnarray*}$

With the condition $0 < \phi < 1$ , the mapping $\Psi$ is a contraction, and then there exists a unique solution $Y$ $\in$ $C^{\left(n\right) }\left(J\right)$ . □

5.2. Convergence

Theorem 5.2. The series solution of the system (4.1)-(4.2) using ADM converges if $\left\vert y_{i1}\right\vert < \infty$ , $0 < \phi < 1$ , and $\, \phi = \frac{LKT^{\upsilon }}{\upsilon \Gamma \left(\upsilon \right) }$ , where $L = \sum\limits_{m = 1}^{n}L_{m}$ , $K = \max \left\{ K_{1}, K_{2}, \ldots, K_{n}\right\}$ .

Proof. Take a sequence $\left\{ S_{kr}\right\}$ such that $S_{kr} = \sum \limits_{m = 0}^{r}y_{km}(t)$ is the partial sums sequence from the series solution $\sum\limits_{m = 0}^{\infty }y_{km}(t)$ . We get

$\ f(S_{kr}) = \sum\limits_{m = 0}^{r}A_{km}(y_{k0}, y_{k1}, \ldots , y_{kr}).$

Let $S_{kr}$ and $S_{kw}$ be two partial sums where $r > w$ . Our goal is to show that $\left\{ S_{kr}\right\}$ is a Cauchy sequence in this Banach space.

$\begin{eqnarray*} \left\Vert S_{kp}-S_{kq}\right\Vert & = &\sum\limits_{m = 1}^{n}\underset{t\in J }{\max }\left\vert S_{mr}-S_{mw}\right\vert = \sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert \sum\limits_{j = w+1}^{r}y_{mj}(t)\right\vert \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{1}{ \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) A_{m(j-1)}d\tau \right\vert \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{1}{ \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) \sum\limits_{j = w+1}^{r}A_{m(j-1)}d\tau \right\vert \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{1}{ \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) \sum\limits_{j = w}^{r-1}A_{mj}d\tau \right\vert \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{1}{ \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}h_{k}\left( \tau \right) [f(S_{m(r-1)})-f(S_{m(w-1)})]d\tau \right\vert \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\left\vert h_{k}\left( \tau \right) \right\vert \left\vert \left( f(S_{m(r-1)})-f(S_{m(w-1)})\right) \right\vert d\tau \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\frac{1}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}\left\vert h_{i}\left( \tau \right) \right\vert \left( L_{m}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) d\tau \\ &\leq &\sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\frac{LK}{\Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-\tau \right) ^{\upsilon -1}d\tau \left\Vert S_{j(r-1)}-S_{j(w-1)}\right\Vert \\ &\leq &\frac{LKT^{\upsilon }}{\Gamma \left( \upsilon \right) }\left\Vert S_{j(r-1)}-S_{j(w-1)}\right\Vert \\ &\leq &\phi \left\Vert S_{j(r-1)}-S_{j(w-1)}\right\Vert . \end{eqnarray*}$

Let $p = q+1$ . Then,

$\left\Vert S_{k(q+1)}-S_{kq}\right\Vert \leq \phi \left\Vert S_{kq}-S_{k(q-1)}\right\Vert \leq \phi ^{2}\left\Vert S_{k(q-1)}-S_{k(q-2)}\right\Vert \leq \cdots \leq \phi ^{q}\left\Vert S_{k1}-S_{k0}\right\Vert .$

Using the triangle inequality,

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert &\leq &\left\Vert S_{k(w+1)}-S_{kw}\right\Vert +\left\Vert S_{k(w+2)}-S_{k(w+1)}\right\Vert +\cdots +\left\Vert S_{kr}-S_{k(r-1)}\right\Vert \\ \ &\leq &\left[ \phi ^{w}+\phi ^{w+1}+\cdots +\phi ^{r-1}\right] \left\Vert S_{k1}-S_{k0}\right\Vert \\ &\leq &\phi ^{w}\left[ 1+\phi +\cdots +\phi ^{r-w-1}\right] \left\Vert S_{k1}-S_{k0}\right\Vert \\ \ &\leq &\phi ^{w}\left[ \frac{1-\phi ^{r-w}}{1-\phi }\right] \left\Vert y_{k1}(t)\right\Vert , \end{eqnarray*}$

where $0 < \phi < 1$ , and $r > w$ . Consequently, $(1-\phi ^{r-w})\leq 1$ . Then,

$\begin{equation} \left\Vert S_{kr}-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\left\Vert y_{k1}(t)\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset{t\in J}{\max } \left\vert y_{k1}(t)\right\vert . \end{equation}$

(5.1)

However, $\left\vert y_{k1}(t)\right\vert < \infty$ , and as $w\rightarrow \infty$ , $\left\Vert S_{kr}-S_{kw}\right\Vert \rightarrow 0$ . Therefore, $\left\{ S_{kr}\right\}$ is a Cauchy sequence in this Banach space. □

5.3. Error estimation

Theorem 5.3. The maximum absolute error of system (4.1)-(4.2) can be estimated as

$\begin{equation*} \underset{t\in J}{\max }\left\vert y_{k}(t)-\sum\limits_{m = 0}^{w}y_{km}(t)\right\vert \leq \frac{\phi ^{w}}{ 1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert . \end{equation*}$

Proof. From the convergence theorem inequality (5.1), we have

$\ \left\Vert S_{kr}-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset {t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

However, $S_{kp} =$ $\sum\limits_{m = 0}^{r}x_{km}(t)$ as $r\rightarrow \infty$ . Then, $S_{kr}\rightarrow y_{k}(t)$ , so

$\left\Vert y_{k}(t)-S_{kq}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset {t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

So, the maximum absolute error will be

$\underset{t\in J}{\max }\left\vert y_{k}(t)-\sum\limits_{m = 0}^{w}y_{km}(t)\right\vert \leq \frac{\phi ^{w}}{ 1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

5.4. SIR epidemic model with arbitrary order containing CD

The SIR epidemic model of arbitrary orders with CD is

$\begin{eqnarray} ^{C}D_{0}^{\alpha }S\left( t\right) & = &\left( 1-\eta \right) \pi -\varphi SI-\pi S, \\ ^{C}D_{0}^{\alpha }I\left( t\right) & = &\varphi SI-\left( \gamma +\pi \right) I, \\ ^{C}D_{0}^{\alpha }R\left( t\right) & = &\eta \pi +\gamma I-\pi R, \end{eqnarray}$

(5.2)

subject to

$\begin{equation*} S(0) = N_{1}, \ I(0) = N_{2}, \ R(0) = N_{3}. \end{equation*}$

Applying the ADM to the system (5.2), we get the following algorithm:

$\begin{eqnarray} S_{0} & = &N_{1}+\text{ }^{C}I^{\alpha }\left( 1-\eta \right) \pi , \ { \ } S_{j+1} = -\varphi \text{ }^{C}I^{\alpha }(A_{j})-\pi \text{ }^{C}I^{\alpha }\left( S_{j}\right) , \\ I_{0} & = &N_{2}, \ { \ \ }I_{j+1} = \varphi \text{ }^{C}I^{\alpha }\left( A_{j}\right) -\text{ }^{C}I^{\alpha }\left[ \left( \gamma +\pi \right) I_{j} \right] , \\ R_{0} & = &N_{3}+\text{ }^{C}I^{\alpha }\left( \eta \pi \right) , \ { \ \ } R_{j+1} = \gamma {\ }^{C}I^{\alpha }\left( I_{j}\right) -\pi \text{ } ^{C}I^{\alpha }\left( R_{j}\right) . \end{eqnarray}$

(5.3)

From the relations (5.3), the solution of the system (5.2) will be

$\begin{equation} S(t) = \sum\limits_{m = 0}^{n}S_{m}(t), \; I(t) = {\ }\sum\limits_{m = 0}^{n}I_{m}(t), \text{ and } R(t) = {\ }\sum\limits_{m = 0}^{n}R_{m}(t). \notag \end{equation}$

– show ADM solutions of the SIR system with different values of $\alpha$ ( $\alpha = 1, 0.9, 0.8, 0.7$ ). It is essential here to note that all the Parameters depend on the fractional order $\alpha$ of the model. The model consists of three variables, subject to time and $n = 5$ . The parameters can be identified as follows: Parameters ( $N_{1}$ , $N_{2}$ , and $N_{3}$ ) are the initial conditions of the SIR system, taking $N_{1} = 1$ , $N_{2} = 0.2$ , $N_{3} = 0$ . Also, $\eta = 0.9$ is the therapy rate, $\pi = 0.4$ is the birth rate $\varphi = 0.8$ is the infected individual rate, and $\gamma = 0.03$ is the recovery from infection rate.

Figure 5. ADM solution of

$S(t)$ with CD.

DownLoad: Full-Size Img PowerPoint

Figure 6. ADM solution of

$I(t)$ with CD.

DownLoad: Full-Size Img PowerPoint

Figure 7. ADM solution of

$R(t)$ with CD.

DownLoad: Full-Size Img PowerPoint

– give ADs between the ADM and RK4 solutions of the SIR system at $\alpha = 1$ , respectively.

Table 4. ADM and RK4 solutions of

$S(t)$ .

$t$	ADM solution	RK4 solution	AD
$0$	1	1	0
$0.1$	0.949	0.949	4.4 $\times$ 10 $^{-5}$
$0.2$	0.901	0.901	4 $\times$ 10 $^{-6}$
$0.3$	0.854	0.854	1.2 $\times$ 10 $^{-5}$
$0.4$	0.810	0.810	3.7 $\times$ 10 $^{-5}$
$0.5$	0.768	0.768	3.3 $\times$ 10 $^{-5}$
$0.6$	0.729	0.729	5.9 $\times$ 10 $^{-5}$
$0.7$	0.691	0.691	4 $\times$ 10 $^{-6}$
$0.8$	0.655	0.655	0.00008
$0.9$	0.621	0.621	0.0003

| Show Table

DownLoad: CSV

Table 5. ADM and RK4 solutions of

$I(t)$ .

$t$	ADM solution	RK4 solution	AD
$0$	0.2	0.2	0
$0.1$	0.2071	0.207	0.00001
$0.2$	0.2136	0.214	0.00003
$0.3$	0.2195	0.2195	0.00002
$0.4$	0.225	0.225	0.00005
$0.5$	0.229	0.229	0.00003
$0.6$	0.233	0.233	0.00004
$0.7$	0.237	0.237	4 $\times$ 10 $^{-6}$
$0.8$	0.239	0.244	0.005
$0.9$	0.241	0.239	0.002

| Show Table

DownLoad: CSV

Table 6. ADM and RK4 solutions of

$R(t)$ .

$t$	ADM solution	RK4 solution	AD
$0$	0	0	0
$0.1$	0.036	0.036	0.00001
$0.2$	0.070	0.070	0.00001
$0.3$	0.104	0.104	0.00004
$0.4$	0.135	0.135	0.00004
$0.5$	0.166	0.166	0.00002
$0.6$	0.196	0.196	0.00004
$0.7$	0.224	0.223	0.00096
$0.8$	0.251	0.251	0.00003
$0.9$	0.277	0.277	0.00002

| Show Table

DownLoad: CSV

From –, for $\alpha = 1$ , ADM and RK4 are given enclosed values, as shown by the values of ADs between them.

shows the ADM solution of the SIR system at $\alpha = 0.5$ and $n = 5$ .

Figure 8. ADM Solution of the SIR model in the Caputo sense at

$\alpha = 0.5$ .

DownLoad: Full-Size Img PowerPoint

Figure 8 shows that the susceptible population decreases, whereas the infected population and the recovered population increase for a long time. In –, we see the effect of using different values of $\alpha$ on the SIR system.

6. Third definition: Atangana-Baleanu derivative (ABD)

The general form of the nonlinear FDE system with the ABD is

$\begin{equation} ^{AB}D_{t}^{\upsilon }y_{k}\left( t\right) +h_{k}\left( t\right) f_{k}\left( \overline{y}\right) = \chi _{k}\left( t\right) , \end{equation}$

(6.1)

subject to

$\begin{equation} x_{k}^{(j-1)}(0) = c_{k}, \ \quad k, j = 1, 2, \ldots , n, \end{equation}$

(6.2)

$\begin{equation*} \overline{y} = \{y_{1}(t), y_{2}(t), \ldots , y_{n}(t)\}, \ \quad 0 < \upsilon < 1. \end{equation*}$

The SIR epidemic model is a special case of this system. Now, applying the FI of order $\upsilon$ , this reduces the system (6.1)-(6.2) to the system of FIEs,

$\begin{eqnarray} x_{k}\left( t\right) & = &c_{k}+\frac{1-\upsilon }{B\left( \upsilon \right) } \chi _{k}\left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\chi _{k}(s)ds \\ &&-\frac{1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) f_{k}\left( \overline{y}\right) -\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) f_{k}\left( \overline{y}\right) ds. \end{eqnarray}$

(6.3)

Assume that $\chi _{k}(t)$ is bounded $\forall t\in J = [0, T], \, T\in R^{+}, \; \left\vert h_{k}(\tau)\right\vert \leq M_{k}, \forall 0\leq \tau \leq t\leq T, \ M_{k}$ are finite constants, and $f_{k}(\overline{y})$ satisfy Lipschitz condition with the Lipschitz constants $L_{k}$ such as

$\begin{equation} \left\vert f_{k}(\overline{y})-f_{k}(\overline{z})\right\vert \leq L_{k}\left\vert \overline{y}-\overline{z}\right\vert . \end{equation}$

(6.4)

Substituting Eq (3.5) into Eq (6.3), we get

$\begin{eqnarray} y_{k}\left( t\right) & = &c_{k}+\frac{1-\upsilon }{B\left( \upsilon \right) } \chi _{k}\left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\chi _{k}(s)ds \\ &&-\frac{1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) \sum\limits_{i = 0}^{\infty }A_{ki}-\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) \sum\limits_{i = 0}^{\infty }A_{ki}\, ds.\ { \ \ \ \ \ } \end{eqnarray}$

(6.5)

Let $x_{k}(t) = \sum\limits_{i = 0}^{\infty }x_{ki}(t)$ in (6.5) and we get

$\begin{eqnarray} x_{k0}(t) & = &c_{k}+\frac{1-\upsilon }{B\left( \upsilon \right) }\chi _{k}\left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\chi _{k}(s)ds, \end{eqnarray}$

(6.6)

$\begin{eqnarray} x_{ki}(t) & = &-\frac{1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) A_{k(i-1)}-\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) A_{k(i-1)}ds, \quad i\geq 1.\ { \ \ \ \ \ } \end{eqnarray}$

(6.7)

The final solution will be

$\begin{equation} x_{k}(t) = \sum\limits_{i = 0}^{\infty }x_{ki}(t). \end{equation}$

(6.8)

6.1. Analysis of convergence

6.1.1. Existence of a unique solution

Define the mapping $\Psi :\Omega \rightarrow \Omega$ . $\Omega$ is the Banach space ( $C^{\left(n\right) }\left(J\right), \left\Vert \cdot \right\Vert$ ), where $C^{\left(n\right) }\left(J\right)$ is the class of continuous column vectors $Y = \left(\overline{y}\right) ^{\prime }$ with norm $\left\Vert Y\right\Vert = \sum\limits_{k = 1}^{n}\underset{t\in J}{\max } \left\vert y_{k}(t)\right\vert$ , and $\left(.\right) ^{\prime }$ denotes the matrix transpose.

Theorem 6.1. The system (6.1)-(6.2) has a unique solution if $0 < \phi < 1$ , $\phi = \frac{LM\left[\Gamma \left(\upsilon \right) +T^{\upsilon }\right] }{B\left(\upsilon \right) \Gamma \left(\upsilon \right) }$ , {where } $L = \sum\limits_{m = 1}^{n}L_{m}$ , $M = \max \left\{ M_{1}, M_{2}, \ldots, M_{n}\right\}$ .

Proof. Equation (6.5) can be described as

$\begin{eqnarray*} Y\left( t\right) & = &C+\frac{1-\upsilon }{B\left( \upsilon \right) }\chi \left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\chi (s)ds \\ &&-\frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) F\left( \overline{y}\right) -\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}H\left( s\right) F\left( \overline{y}\right) ds, \end{eqnarray*}$

where

The mapping $\Psi :\Omega \rightarrow \Omega$ is defined as

$\begin{eqnarray*} \Psi X\left( t\right) & = &C+\frac{1-\upsilon }{B\left( \upsilon \right) } \chi \left( t\right) +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\chi (s)ds \\ &&-\frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) F\left( \overline{y}\right) -\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}H\left( s\right) F\left( \overline{y}\right) ds. \end{eqnarray*}$

Let $X, Z\in \Omega$ .

$\begin{eqnarray*} \left\Vert \Psi Y\left( t\right) -\Psi Z\left( t\right) \right\Vert & = &\left\Vert -\frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) \left( F(\overline{y})-F(\overline{z})\right) -\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}H\left( s\right) \left( F(\overline{y})-F(\overline{ z})\right) ds\right\Vert \\ &\leq &\left\Vert \frac{1-\upsilon }{B\left( \upsilon \right) }H\left( t\right) \left( F(\overline{y})-F(\overline{z})\right) \right\Vert +\left\Vert \frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}H\left( s\right) \left( F(\overline{y})-F(\overline{z})\right) ds\right\Vert \\ &\leq &\frac{1-\upsilon }{B\left( \upsilon \right) }\left\Vert H\left( t\right) \right\Vert \left\Vert F(\overline{x})-F(\overline{z})\right\Vert + \frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) } \int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\left\Vert H\left( s\right) \right\Vert \left\Vert F(\overline{x})-F(\overline{z})\right\Vert ds \\ &\leq &\frac{\left( 1-\upsilon \right) M}{B\left( \upsilon \right) }\left( \sum\limits_{m = 1}^{n}\underset{t\in J}{\max }\left\vert f_{m}(\overline{x} )-f_{m}(\overline{z})\right\vert \right) \\ &&+\frac{\upsilon M}{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\left( \sum\limits_{m = 1}^{n} \underset{t\in J}{\max }\left\vert f_{m}(\overline{y})-f_{m}(\overline{z} )\right\vert \right) ds \\ &\leq &\frac{M}{B\left( \upsilon \right) }\left( \sum\limits_{m = 1}^{n} \underset{t\in J}{\max }\left\vert f_{m}(\overline{y})-f_{m}(\overline{z} )\right\vert \right) \left[ \left( 1-\upsilon \right) +\frac{\upsilon }{ \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}ds\right] \\ &\leq &\frac{M}{B\left( \upsilon \right) }\left[ 1-\upsilon +\frac{\upsilon T^{\upsilon }}{\Gamma \left( \upsilon \right) }\right] \sum \limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{M}{B\left( \upsilon \right) }\left[ 1+\frac{T^{\upsilon }}{ \Gamma \left( \upsilon \right) }\right] \sum\limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{M\left[ \Gamma \left( \gamma \right) +T^{\gamma }\right] }{ B\left( \gamma \right) \Gamma \left( \gamma \right) }\sum \limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{M\left[ \Gamma \left( \gamma \right) +T^{\gamma }\right] }{ B\left( \gamma \right) \Gamma \left( \gamma \right) }\sum \limits_{m = 1}^{n}L_{m}\left\Vert Y-Z\right\Vert \\ &\leq &\frac{LM\left[ \Gamma \left( \upsilon \right) +T^{\upsilon }\right] }{ B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\left\Vert Y-Z\right\Vert \\ &\leq &\phi \left\Vert Y-Z\right\Vert . \end{eqnarray*}$

If $0 < \phi < 1$ , the mapping $\Psi$ will be a contraction, and then there exists a unique solution of the system (6.1)-(6.2). □

6.1.2. Convergence proof

Theorem 6.2. The series solution (6.8) will converge if $\left\vert y_{k1}\right\vert < \infty$ and $0 < \phi < 1, \, \phi = \frac{LM\left[\Gamma \left(\upsilon \right) +T^{\upsilon }\right] }{B\left(\upsilon \right) \Gamma \left(\upsilon \right) }$ , where $L = \sum\limits_{k = 1}^{n}L_{k}$ , $M = \max \left\{ M_{1}, M_{2}, \ldots, M_{n}\right\}$ .

Proof. Take a sequence $\left\{ S_{kr}\right\}$ such that $S_{kr} = \sum \limits_{i = 0}^{r}y_{ki}(t)$ is a partial sums sequence of $\sum\limits_{i = 0}^{\infty }y_{ki}(t)$ . We have

$\begin{equation*} \ f(S_{kr}) = \sum\limits_{i = 0}^{p}A_{ki}(y_{k0}, y_{k1}, \ldots , y_{kr}). \end{equation*}$

Let $S_{kr}$ and $S_{kw}$ be two partial sums where $r > w$ . Our goal is to prove that $\left\{ S_{kr}\right\}$ is a Cauchy sequence in this Banach space.

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert & = &\sum\limits_{i = 1}^{n}\underset{t\in J }{\max }\left\vert S_{ir}-S_{iw}\right\vert = \sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \sum\limits_{j = w+1}^{r}x_{ij}(t)\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \sum\limits_{j = w+1}^{r}\frac{1-\upsilon }{B\left( \upsilon \right) } h_{k}\left( t\right) A_{k(i-1)}+\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) A_{k(i-1)}ds\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) \sum\limits_{j = w+1}^{r}A_{i(j-1)}+\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\gamma -1}h_{k}\left( s\right) \sum\limits_{j = w+1}^{r}A_{i(j-1)}ds\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) \sum\limits_{j = w}^{r-1}A_{ij}+\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) \sum\limits_{j = w}^{r-1}A_{ij}ds\right\vert \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]\right. \\ &&\left. +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]ds\right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left[ \left\vert \frac{ 1-\upsilon }{B\left( \upsilon \right) }h_{k}\left( t\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]\right\vert \right. \\ &&\left. +\left\vert \frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}h_{k}\left( s\right) [f(S_{i(r-1)})-f(S_{i(w-1)})]ds\right\vert \right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left[ \frac{1-\upsilon }{B\left( \upsilon \right) }\left\vert h_{k}\left( t\right) \right\vert \left\vert f(S_{i(r-1)})-f(S_{i(w-1)})\right\vert \right. \\ &&\left. +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\left\vert h_{k}\left( s\right) \right\vert \left\vert f(S_{i(r-1)})-f(S_{i(w-1)})\right\vert ]ds \right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left[ \frac{1-\upsilon }{B\left( \upsilon \right) }\left\vert h_{k}\left( t\right) \right\vert \left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) \right. \\ &&\left. +\frac{\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\int_{0}^{t}\left( t-s\right) ^{\upsilon -1}\left\vert h_{k}\left( s\right) \right\vert \left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) ds\right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left( L_{i}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) \left[ \frac{1-\upsilon }{B\left( \upsilon \right) }M+\frac{ M\upsilon }{B\left( \upsilon \right) \Gamma \left( \upsilon \right) } \int_{0}^{t}\left( t-s\right) ^{\upsilon -1}ds\right] \\ &\leq &\sum\limits_{i = 1}^{n}\underset{t\in J}{\max }\left( L_{k}\sum\limits_{j = 1}^{n}\left\vert S_{j(r-1)}-S_{j(w-1)}\right\vert \right) \left[ \frac{1-\upsilon }{B\left( \upsilon \right) }M+\frac{ MT^{\upsilon }}{B\left( \upsilon \right) \Gamma \left( \upsilon \right) } \right] \\ &\leq &\frac{LM\left[ \Gamma \left( \upsilon \right) +T^{\upsilon }\right] }{ B\left( \upsilon \right) \Gamma \left( \upsilon \right) }\left\Vert S_{k(r-1)}-S_{k(w-1)}\right\Vert \\ &\leq &\phi \left\Vert S_{k(r-1)}-S_{k(w-1)}\right\Vert . \end{eqnarray*}$

Let $r = w+1$ . Then,

$\begin{equation*} \left\Vert S_{k(w+1)}-S_{kw}\right\Vert \leq \phi \left\Vert S_{kw}-S_{k(w-1)}\right\Vert \leq \phi ^{2}\left\Vert S_{k(w-1)}-S_{k(w-2)}\right\Vert \leq \cdots \leq \phi ^{w}\left\Vert S_{k1}-S_{k0}\right\Vert . \end{equation*}$

From the triangle inequality,

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert &\leq &\left\Vert S_{k(w+1)}-S_{kw}\right\Vert +\left\Vert S_{k(w+2)}-S_{k(w+1)}\right\Vert +\cdots +\left\Vert S_{kr}-S_{k(r-1)}\right\Vert \\ \ &\leq &\left[ \phi ^{w}+\phi ^{w+1}+\cdots +\phi ^{r-1}\right] \left\Vert S_{k1}-S_{k0}\right\Vert \\ &\leq &\phi ^{w}\left[ 1+\phi +\cdots +\phi ^{r-w-1}\right] \left\Vert S_{k1}-S_{k0}\right\Vert \\ \ &\leq &\phi ^{w}\left[ \frac{1-\phi ^{r-w}}{1-\phi }\right] \left\Vert y_{k1}(t)\right\Vert . \end{eqnarray*}$

Since $0 < \phi < 1$ and $r > w$ , $(1-\phi ^{r-w})\leq 1$ . Consequently,

$\begin{eqnarray*} \left\Vert S_{kr}-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi } \left\Vert y_{k1}(t)\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert , \end{eqnarray*}$

but $\left\vert y_{k1}(t)\right\vert < \infty$ . As $w\rightarrow \infty$ , then, $\left\Vert S_{kr}-S_{kw}\right\Vert \rightarrow 0$ . Therefore, $\left\{ S_{kr}\right\}$ is a Cauchy sequence in this Banach space, so the series $\sum\limits_{i = 0}^{\infty }y_{ki}(t)$ will converge. □

6.1.3. Error estimation

Theorem 6.3. The maximum absolute error of the series solution (6.8) is estimated as

Proof. From Theorem 6.2, we have

$\ \left\Vert S_{kr}-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset {t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

However, $S_{ir} =$ $\sum\limits_{i = 0}^{r}y_{ki}(t)$ as $r\rightarrow \infty$ . Then, $S_{kr}\rightarrow y_{k}(t)$ . So

$\left\Vert y_{k}(t)-S_{kw}\right\Vert \leq \frac{\phi ^{w}}{1-\phi }\underset {t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

So, the maximum absolute error will be

$\underset{t\in J}{\max }\left\vert y_{k}(t)-\sum\limits_{i = 0}^{w}y_{ki}(t)\right\vert \leq \frac{\phi ^{w}}{ 1-\phi }\underset{t\in J}{\max }\left\vert y_{k1}(t)\right\vert .$

□

6.2. SIR epidemic model of arbitrary orders containing ABD

The SIR epidemic model of arbitrary orders involving the ABD is

$\begin{eqnarray} ^{AB}D_{0}^{\alpha }S\left( t\right) & = &\left( 1-p\right) \pi -\beta SI-\pi S, \\ ^{AB}D_{0}^{\alpha }I\left( t\right) & = &\beta SI-\left( \gamma +\pi \right) I, \\ ^{AB}D_{0}^{\alpha }R\left( t\right) & = &p\pi +\gamma I-\pi R, \end{eqnarray}$

(6.9)

subject to

$\begin{equation*} S(0) = N_{1}, \ I(0) = N_{2}, \ R(0) = N_{3}. \end{equation*}$

Applying the ADM to the system (6.9), we get the following solution algorithm:

$\begin{eqnarray} S_{0} & = &N_{1}+\text{ }^{AB}I^{\alpha }\left( 1-p\right) \pi , \quad S_{j+1} = -\beta \text{ }^{AB}I^{\alpha }(A_{j})-\pi \text{ }^{AB}I^{\alpha }\left( S_{j}\right) , \end{eqnarray}$

(6.10)

$\begin{eqnarray} I_{0} & = &N_{2}, \quad I_{j+1} = \beta \text{ }^{AB}I^{\alpha }\left( A_{j}\right) -\text{ }^{AB}I^{\alpha }\left[ \left( \gamma +\pi \right) I_{j} \right] , \end{eqnarray}$

(6.11)

$\begin{eqnarray} R_{0} & = &N_{3}+\text{ }^{AB}I^{\alpha }\left( p\pi \right) , \quad R_{j+1} = \gamma \text{ }^{AB}I^{\alpha }\left( I_{j}\right) -\pi \text{ } ^{AB}I^{\alpha }\left( R_{j}\right) . \end{eqnarray}$

(6.12)

Using the relations (6.10)–(6.12), the series solution of the system (6.9) will be

$\begin{equation} S(t) = \sum\limits_{k = 0}^{n}S_{k}(t), I(t) = {\ }\sum\limits_{k = 0}^{n}I_{k}(t), R(t) = \ { \ }\sum\limits_{k = 0}^{n}R_{k}(t). \notag \end{equation}$

– show the ADM solution of the SIR system at different values of $\alpha$ ( $\alpha = 1, 0.95, 0.85, 0.75$ ) and $n = 5$ , respectively.

Figure 9. ADM solution of

$S\left(t\right)$ in the Atangana-Baleanu sense at different values of

$\alpha$ .

DownLoad: Full-Size Img PowerPoint

Figure 10. ADM solution of

$I\left(t\right)$ in the Atangana-Baleanu sense at different values of

$\alpha$ .

DownLoad: Full-Size Img PowerPoint

Figure 11. ADM solution of

$R\left(t\right)$ in the Atangana-Baleanu sense at different values of

$\alpha$ .

DownLoad: Full-Size Img PowerPoint

– show ADs between the ADM and RK4 solutions of the SIR system at $\alpha = 1$ , respectively.

Table 7. ADM and RK4 solutions of

$S(t)$ .

$t$	ADM solution	RK4 solution	AD
$0$	1	1	0
$0.1$	0.949	0.949	0.00004
$0.2$	0.901	0.901	4 $\times 10^{-6}$
$0.3$	0.854	0.854	0.00001
$0.4$	0.810	0.810	0.00004
$0.5$	0.768	0.768	0.00003
$0.6$	0.729	0.729	0.00006
$0.7$	0.691	0.691	4 $\times 10^{-6}$
$0.8$	0.655	0.655	0.00008
$0.9$	0.621	0.621	0.00027

| Show Table

DownLoad: CSV

Table 8. ADM and RK4 solutions of

$I(t)$ .

$t$	ADM solution	RK4 solution	AD
$0$	0.2	0.2	0
$0.1$	0.207	0.207	0.00001
$0.2$	0.214	0.214	0.00003
$0.3$	0.2195	0.2195	0.00002
$0.4$	0.225	0.225	0.00005
$0.5$	0.229	0.229	0.00003
$0.6$	0.233	0.233	0.00004
$0.7$	0.237	0.237	4 $\times 10^{-6}$
$0.8$	0.239	0.244	0.005
$0.9$	0.241	0.239	0.002

| Show Table

DownLoad: CSV

Table 9. ADM and RK4 solutions of

$R(t)$ .

$t$	ADM solution	RK4 solution	ADs
$0$	0	0	0
$0.1$	0.036	0.0359	0.00001
$0.2$	0.070	0.0704	0.00001
$0.3$	0.104	0.1036	0.00004
$0.4$	0.135	0.1354	0.00004
$0.5$	0.166	0.1661	0.00002
$0.6$	0.196	0.1955	0.00004
$0.7$	0.224	0.2229	0.00096
$0.8$	0.251	0.2511	0.00003
$0.9$	0.277	0.2772	0.00002

| Show Table

DownLoad: CSV

From –, for $\alpha = 1$ , ADM and RK4 are given enclosed values, as shown from the values of ADs between them.

shows the ADM solution of the SIR system at $\alpha = 0.5$ and $n = 5$ .

Figure 12. ADM solution of the SIR model in the Atangana-Baleanu sense at

$\alpha = 0.5$ .

DownLoad: Full-Size Img PowerPoint

From Figure 12, we see that the susceptible population reduces, whereas the infected population and the recovered population increase for a long time. In –, we see the effect of using different values of $\alpha$ on the SIR system.

7. Comparison between the three definitions

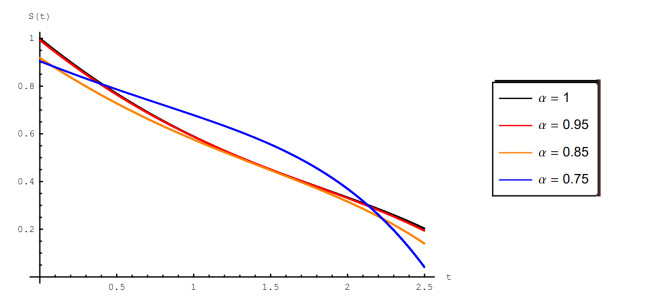
In this section, we aim to give a comparison between the previous three different FDs, as shown in the following figures. In –, we show the solution of the SIR system at multiple values of $\alpha$ ( $\alpha = 1, 0.95, 0.85, 0.75$ ) and $n = 5$ , as follows:

Figure 13.

$S(t)$ solution of CFD, CD, ABD at

$\alpha = 0.95$ .

DownLoad: Full-Size Img PowerPoint

Figure 14.

$S(t)$ solution of CFD, CD, ABD at

$\alpha = 0.85$ .

DownLoad: Full-Size Img PowerPoint

Figure 15.

$S(t)$ solution of CFD, CD, ABD at

$\alpha = 0.75$ .

DownLoad: Full-Size Img PowerPoint

Figure 16.

$I(t)$ solution of CFD, CD, ABD at

$\alpha = 1$ .

DownLoad: Full-Size Img PowerPoint

Figure 17.

$I(t)$ solution of CFD, CD, ABD at

$\alpha = 0.95$ .

DownLoad: Full-Size Img PowerPoint

Figure 18.

$I(t)$ solution of CFD, CD, ABD at

$\alpha = 0.85$ .

DownLoad: Full-Size Img PowerPoint

Figure 19.

$I(t)$ solution of CFD, CD, ABD at

$\alpha = 0.75$ .

DownLoad: Full-Size Img PowerPoint

Figure 20.

$R(t)$ solution of CFD, CD, ABD at

$\alpha = 1$ .

DownLoad: Full-Size Img PowerPoint

Figure 21.

$R(t)$ solution of CFD, CD, ABD at

$\alpha = 0.95$ .

DownLoad: Full-Size Img PowerPoint

Figure 22.

$R(t)$ solution of CFD, CD, ABD at

$\alpha = 0.85$ .

DownLoad: Full-Size Img PowerPoint

Figure 23.

$R(t)$ solution of CFD, CD, ABD at

$\alpha = 0.75$ .

DownLoad: Full-Size Img PowerPoint

(1) The solution of $S(t)$ is given in Figures 13–15.

(2) The solution of $I(t)$ is given in Figures 16–19.

(3) The solution of $R(t)$ is given in Figures 20–23.

From Figures 13–23, we see the following:

ⅰ) In the integer case ( $\alpha = 1$ ), the three FDs give the same solution (see Figures 13, 16 and 20).

ⅱ) For fractional orders, we see that the ABD is closer to the CD than the CFD.

8. Conclusions

This research considered analytical and numerical solutions of an important fractional order model of epidemic childhood diseases (the SIR model) with three different definitions of fractional derivatives: CD, CFD, and ABD. The analytical solution was obtained using the ADM, while the numerical solution was obtained using the RK4 method. By calculating the absolute differences between these two methods, we see that the two solutions coincide (see –). A comparison is made between the solutions obtained with the three different definitions, and we see that, for integer order ( $\alpha = 1$ ), the three different FDs give the same solution (see Figures 13, 16 and 20). Meanwhile, for fractional orders, we see that the ABD is closer to the CD than the CFD (see Figures 14–15, 17–19 and 21–23).

Author contributions

Eman A. A. Ziada and Osama Moaaz: Conceptualization, Methodology, Formal analysis; Salwa El-Morsy and Ahmad M. Alshamrani: Investigation, Writing-original draft; Sameh S. Askar and Monica Botros: Software, Writing-review & editing. All authors have read and approved the final version of the manuscript for publication.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This research was supported by the Researchers Supporting Project number (RSPD2024R533), King Saud University, Riyadh, Saudi Arabia.

Conflict of interest

The authors declare no competing interests.

References

[1]	I. T. Huseynov, A. Ahmadova, A. Fernandez, N. I. Mahmudov, Explicit analytical solutions of incommensurate fractional differential equation systems, Appl. Math. Comput., 390 (2021), 125590. doi: 10.1016/j.amc.2020.125590. doi: 10.1016/j.amc.2020.125590
[2]	A. Hajipour, H, Tavakoli, Analysis and circuit simulation of a novel nonlinear fractional incommensurate order financial system, Optik, 127 (2016), 10643–10652. doi: 10.1016/j.ijleo.2016.08.098. doi: 10.1016/j.ijleo.2016.08.098
[3]	I. Pan, S. Das, S. Das, Multi-objective active control policy design for commensurate and incommensurate fractional order chaotic financial systems, Appl. Math. Model., 39 (2015), 500–514. doi: 10.1016/j.apm.2014.06.005. doi: 10.1016/j.apm.2014.06.005
[4]	K. Zourmba, A. A. Oumate, B. Gambo, J. Y. Effa, A. Mohamadou, Chaos in the incommensurate fractional order system and circuit simulations, Int. J. Dyn. Control, 7 (2019), 94–111. doi: 10.1007/s40435-018-0442-y. doi: 10.1007/s40435-018-0442-y
[5]	X. Wang, Z. Wang, J. Xia, Stability and bifurcation control of a delayed fractional-order eco-epidemiological model with incommensurate orders, J. Franklin Inst., 356 (2019), 8278–8295. doi: 10.1016/j.jfranklin.2019.07.028. doi: 10.1016/j.jfranklin.2019.07.028
[6]	B. Daşbaşi. Stability analysis of the HIV model through incommensurate fractional-order nonlinear system, Chaos, Solitons Fractals, 137 (2020), 109870. doi: 10.1016/j.chaos.2020.109870.
[7]	N. Debbouche, A. O. Almatroud, A. Ouannas, I. M. Batiha, Chaos and coexisting attractors in glucose-insulin regulatory system with incommensurate fractional-order derivatives, Chaos, Solitons Fractals, 143 (2021), 110575. doi: 10.1016/j.chaos.2020.110575. doi: 10.1016/j.chaos.2020.110575
[8]	M. Tavazoei, M. H. Asemani, On robust stability of incommensurate fractional-order systems, Commun. Nonlinear Sci. Numer. Simul., 90 (2020), 105344. doi: 10.1016/j.cnsns.2020.105344. doi: 10.1016/j.cnsns.2020.105344
[9]	M. Tavazoei, M. H. Asemani, Robust stability analysis of incommensurate fractional-order systems with time-varying interval uncertainties, J. Franklin Inst., 357 (2020), 13800–13815. doi: 10.1016/j.jfranklin.2020.09.044. doi: 10.1016/j.jfranklin.2020.09.044
[10]	Y. Shen, Y. Wang, N. Yuan, A graphical approach for stability and robustness analysis in commensurate and incommensurate fractional-order systems, Asian J. Control, 22 (2020), 1241–1252. doi: 10.1002/asjc.1980. doi: 10.1002/asjc.1980
[11]	R. Luo, H. Su, The stability of impulsive incommensurate fractional order chaotic systems with Caputo derivative, Chinese J. Phys., 56 (2018), 1599–1608. doi: 10.1016/j.cjph.2018.06.017. doi: 10.1016/j.cjph.2018.06.017
[12]	C. M. Chang, H. K. Chen, Chaos and hybrid projective synchronization of commensurate and incommensurate fractional-order Chen-Lee systems, Nonlinear Dyn., 62 (2010), 851–858. doi: 10.1007/s11071-010-9767-6. doi: 10.1007/s11071-010-9767-6
[13]	C. Ma, J. Mou, J. Liu, F. Yang, H. Yan, X. Zhao, Coexistence of multiple attractors for an incommensurate fractional-order chaotic system, Eur. Phys. J. Plus, 135 (2020), 1–21. doi: 10.1140/epjp/s13360-019-00093-0. doi: 10.1140/epjp/s13360-019-00093-0
[14]	C. Huang, J. Cao, M. Xiao, A. Alsaedi, F. E. Alsaadi, Controlling bifurcation in a delayed fractional predator-prey system with incommensurate orders, Appl. Math. Comput., 293 (2017), 293–310. doi: 10.1016/j.amc.2016.08.033. doi: 10.1016/j.amc.2016.08.033
[15]	K. Diethelm, N. J. Ford, A. D. Freed, A predictor-corrector approach for the numerical solution of fractional differential equations, Nonlinear Dyn., 29 (2002), 3–22. doi: 10.1023/A:1016592219341. doi: 10.1023/A:1016592219341
[16]	K. Diethelm, N. J. Ford, Multi-order fractional differential equations and their numerical solution, Appl. Math. Comput., 154 (2004), 621–640. doi: 10.1016/S0096-3003(03)00739-2. doi: 10.1016/S0096-3003(03)00739-2
[17]	H. Liao, Y. Ding, L. Wang, Adomian decomposition algorithm for studying incommensurate fractional-order memristor-based Chua's system, Int. J. Bifurcat. Chaos, 28 (2018), 1850134. doi: 10.1142/S0218127418501341. doi: 10.1142/S0218127418501341
[18]	H. N. Soloklo, N. Bigdeli, Direct approximation of fractional order systems as a reduced integer/fractional-order model by genetic algorithm, Sadhana, 45 (2020), 1–15. doi: 10.1007/s12046-020-01503-1.
[19]	A. Ahmadova, I. T. Huseynov, A. Fernandez, N. I. Mahmudov, Trivariate Mittag-Leffler functions used to solve multi-order systems of fractional differential equations, Commun. Nonlinear Sci. Numer. Simul., 97 (2021), 105735. doi: 10.1016/j.cnsns.2021.105735. doi: 10.1016/j.cnsns.2021.105735
[20]	N. I. Mahmudov, I. T. Huseynov, N. A. Aliev, F. A. Aliev, Analytical approach to a class of Bagley-Torvik equations, TWMS J. Pure Appl. Math., 11 (2020), 238–258.
[21]	I. T. Huseynov, A. Ahmadova, N. I. Mahmudov, Fractional Leibniz integral rules for Riemann-Liouville and Caputo fractional derivatives and their applications, 2020. Available from: https://arXiv.org/abs/2012.11360.
[22]	M. A. Özarslan, A. Fernandez, On the fractional calculus of multivariate Mittag-Leffler functions, Int. J. Comput. Math., 2021, 1–27. doi: 10.1080/00207160.2021.1906869.
[23]	B. Bira, H. Mandal, D. Zeidan, Exact solution of the time fractional variant Boussinesq-Burgers equations, Appl. Math., 66 (2021), 437–449. doi: 10.21136/AM.2021.0269-19. doi: 10.21136/AM.2021.0269-19
[24]	D. Zeidan, C. K. Chau, T. T. Lu, W. Q. Zheng, Mathematical studies of the solution of Burgers' equations by Adomian decomposition method, Math. Methods Appl. Sci., 43 (2020), 2171–2188. doi: 10.1002/mma.5982. doi: 10.1002/mma.5982
[25]	J. R. Loh, C. Phang, Numerical solution of Fredholm fractional integro-differential equation with right-sided Caputo's derivative using Bernoulli polynomials operational matrix of fractional derivative, Mediterr. J. Math., 16 (2019), 1–25. doi: 10.1007/s00009-019-1300-7. doi: 10.1007/s00009-019-1300-7
[26]	J. R. Loh, C. Phang, K. G. Tay, New method for solving fractional partial integro-differential equations by combination of Laplace transform and resolvent kernel method, Chinese J. Phys., 67 (2020), 666–680. doi: 10.1016/j.cjph.2020.08.017. doi: 10.1016/j.cjph.2020.08.017
[27]	M. A. Ebadi, E. Hashemizadeh, A new approach based on the Zernike radial polynomials for numerical solution of the fractional diffusion-wave and fractional Klein-Gordon equations, Phys. Scripta, 93 (2018), 125202. doi: 10.1088/1402-4896/aae726
[28]	L. N. Kaharuddin, C. Phang, S. S. Jamaian, Solution to the fractional logistic equation by modified Eulerian numbers, Eur. Phys. J. Plus, 135 (2020), 1–11. doi: 10.1140/epjp/s13360-020-00135-y. doi: 10.1140/epjp/s13360-020-00135-y
[29]	P. Roul, V. M. K. Prasad Goura, A high order numerical scheme for solving a class of non-homogeneous time-fractional reaction diffusion equation, Numer. Methods Partial Differ. Equ., 37 (2021), 1506–1534. doi: 10.1002/num.22594. doi: 10.1002/num.22594
[30]	P. Roul, A high accuracy numerical method and its convergence for time-fractional Black-Scholes equation governing European options, Appl. Numer. Math., 151 (2020), 472–493. doi: 10.1016/j.apnum.2019.11.004. doi: 10.1016/j.apnum.2019.11.004
[31]	S. Kumar, D. Zeidan, An efficient Mittag-Leffler kernel approach for time-fractional advection-reaction-diffusion equation, Appl. Numer. Math., 170 (2021), 190–207. doi: 10.1016/j.apnum.2021.07.025. doi: 10.1016/j.apnum.2021.07.025
[32]	F. Sultana, D. Singh, R. K. Pandey, D. Zeidan, Numerical schemes for a class of tempered fractional integro-differential equations, Appl. Numer. Math., 157 (2020), 110–134. doi: 10.1016/j.apnum.2020.05.026. doi: 10.1016/j.apnum.2020.05.026
[33]	A. Fernandez, C. Kürt, M. A. Özarslan, A naturally emerging bivariate Mittag-Leffler function and associated fractional-calculus operators, Comput. Appl. Math., 39 (2020), 1–27. doi: 10.1007/s40314-020-01224-5. doi: 10.1007/s40314-020-01224-5
[34]	H. Seybold, R. Hilfer, Numerical algorithm for calculating the generalized Mittag-Leffler function, SIAM J. Numer. Anal., 47 (2009), 69–88. doi: 10.1137/070700280. doi: 10.1137/070700280
[35]	R. Garrappa, Numerical evaluation of two and three parameter Mittag-Leffler functions, SIAM J. Numer. Anal., 53 (2015), 1350–1369. doi: 10.1137/140971191. doi: 10.1137/140971191
[36]	C. Kürt, M. A. Özarslan, A. Fernandez, On a certain bivariate Mittag-Leffler function analysed from a fractional-calculus point of view, Math. Methods Appl. Sci., 44 (2021), 2600–2620. doi: 10.1002/mma.6324. doi: 10.1002/mma.6324
[37]	A. A. Kilbas, H. M. Srivastava, J. J. Trujillo, Theory and applications of fractional differential equations, Vol. 204, Elsevier, 2006.
[38]	A. A. Hamou, E. Azroul, Z. Hammouch, A. Lamrani alaoui, On dynamics of fractional incommensurate model of Covid-19 with nonlinear saturated incidence rate, medRxiv, 2021. doi: 10.1101/2021.07.18.21260711.

This article has been cited by:

1.	Manish Goyal, Haci Mehmet Baskonus, Amit Prakash, Regarding new positive, bounded and convergent numerical solution of nonlinear time fractional HIV/AIDS transmission model, 2020, 139, 09600779, 110096, 10.1016/j.chaos.2020.110096
2.	Majid Bagheri, Ali Khani, Soheil Salahshour, Analytical Method for Solving the Fractional Order Generalized KdV Equation by a Beta-Fractional Derivative, 2020, 2020, 1687-9139, 1, 10.1155/2020/8819183
3.	Mehvish Fazal Ur Rehman, Yongyi Gu, Wenjun Yuan, Soheil Salahshour, Exact Analytical Solutions of Nonlinear Fractional Liouville Equation by Extended Complex Method, 2020, 2020, 1687-9139, 1, 10.1155/2020/8815363
4.	Dumitru Baleanu, Amin Jajarmi, Hakimeh Mohammadi, Shahram Rezapour, A new study on the mathematical modelling of human liver with Caputo–Fabrizio fractional derivative, 2020, 134, 09600779, 109705, 10.1016/j.chaos.2020.109705
5.	Amit Prakash, Vijay Verma, Two efficient computational technique for fractional nonlinear Hirota–Satsuma coupled KdV equations, 2020, 38, 0264-4401, 791, 10.1108/EC-02-2020-0091
6.	Harendra Singh, Numerical simulation for fractional delay differential equations, 2020, 2195-268X, 10.1007/s40435-020-00671-6
7.	Wei Gao, Hatıra Günerhan, Haci Mehmet Baskonus, Analytical and approximate solutions of an epidemic system of HIV/AIDS transmission, 2020, 59, 11100168, 3197, 10.1016/j.aej.2020.07.043
8.	D.G. Prakasha, P. Veeresha, Analysis of Lakes pollution model with Mittag-Leffler kernel, 2020, 5, 24680133, 310, 10.1016/j.joes.2020.01.004
9.	Amit Prakash, Ajay Kumar, Haci Mehmet Baskonus, Ashok Kumar, Numerical analysis of nonlinear fractional Klein–Fock–Gordon equation arising in quantum field theory via Caputo–Fabrizio fractional operator, 2021, 2008-1359, 10.1007/s40096-020-00365-2
10.	Mutaz Mohammad, Carlo Cattani, A collocation method via the quasi-affine biorthogonal systems for solving weakly singular type of Volterra-Fredholm integral equations, 2020, 59, 11100168, 2181, 10.1016/j.aej.2020.01.046
11.	V. Padmavathi, A. Prakash, K. Alagesan, N. Magesh, Analysis and numerical simulation of novel coronavirus (COVID‐19) model with Mittag‐Leffler Kernel, 2021, 44, 0170-4214, 1863, 10.1002/mma.6886
12.	Manish Goyal, Vinod Kumar Bhardwaj, Amit Prakash, Investigating new positive, bounded, and convergent numerical solution for the nonlinear time‐dependent breast cancer dynamic competition model, 2021, 44, 0170-4214, 4636, 10.1002/mma.7057
13.	Hülya Durur, Asif Yokuş, Exact solutions of (2 + 1)-Ablowitz-Kaup-Newell-Segur equation, 2020, 0, 2444-8656, 10.2478/amns.2020.2.00074
14.	Uttam Ghosh, Susmita Sarkar, Bapin Mondal, Study of Stability and Bifurcation of Three Species Food Chain Model with Non-monotone Functional Response, 2021, 7, 2349-5103, 10.1007/s40819-021-01017-2
15.	Shao-Wen Yao, Muhammad Farman, Maryam Amin, Mustafa Inc, Ali Akgül, Aqeel Ahmad, Fractional order COVID-19 model with transmission rout infected through environment, 2022, 7, 2473-6988, 5156, 10.3934/math.2022288
16.	Amit Kumar Saraswat, Manish Goyal, Numerical simulation of time-dependent influenza model with Atangana–Baleanu non-integer order derivative in Liouville–Caputo sense, 2022, 96, 0973-7111, 10.1007/s12043-022-02335-w
17.	V. Padmavathi, N. Magesh, K. Alagesan, M. Ijaz Khan, Samia Elattar, Mamdooh Alwetaishi, Ahmed M. Galal, Numerical Modeling and Symmetry Analysis of a Pine Wilt Disease Model Using the Mittag–Leffler Kernel, 2022, 14, 2073-8994, 1067, 10.3390/sym14051067
18.	Amit Prakash, Numerical simulation of SIR childhood diseases model with fractional Adams–Bashforth method, 2022, 0170-4214, 10.1002/mma.8785
19.	Amit Prakash, Hardish Kaur, 2022, Chapter 38, 978-981-16-7663-5, 465, 10.1007/978-981-16-7664-2_38
20.	Manish Goyal, Amit Prakash, Shivangi Gupta, An Efficient Perturbation Sumudu Transform Technique for the Time-Fractional Vibration Equation with a Memory Dependent Fractional Derivative in Liouville–Caputo Sense, 2021, 7, 2349-5103, 10.1007/s40819-021-01068-5
21.	Amit Prakash, Hardish Kaur, Manish Goyal, 2022, Chapter 31, 978-981-16-7663-5, 383, 10.1007/978-981-16-7664-2_31
22.	Manish Goyal, Haci Mehmet Baskonus, A Reliable Solution of Arbitrary Order Nonlinear Hunter–Saxton Equation with Time Dependent Derivative in Liouville–Caputo Sense, 2021, 7, 2349-5103, 10.1007/s40819-021-01065-8
23.	Manish Goyal, Amit Prakash, Dumitru Baleanu, An efficient hybrid computational technique for the time dependent Lane-Emden equation of arbitrary order, 2022, 7, 24680133, 131, 10.1016/j.joes.2021.07.004
24.	Rania Saadeh, Aliaa Burqan, Ahmad El-Ajou, Reliable solutions to fractional Lane-Emden equations via Laplace transform and residual error function, 2022, 61, 11100168, 10551, 10.1016/j.aej.2022.04.004
25.	Viswanathan Padmavathi, Kandaswami Alagesan, Awatif Alhowaity, Muhammad Ijaz Khan, Haneen Hamam, Manivelu Angayarkanni, Vediyappan Govindan, Analysis and numerical simulation of fractional order pine wilt disease model, 2022, 36, 0217-9792, 10.1142/S0217979222502046
26.	Shivangi Gupta, Manish Goyal, Amit Prakash, A Hybrid Computational Scheme with Convergence Analysis for the Dependent Rosenau-Hyman Equation of Arbitrary Order Via Caputo-Fabrizio Operator, 2021, 7, 2349-5103, 10.1007/s40819-021-01182-4
27.	Hamadou Halidou, Alphonse Houwe, Souleymanou Abbagari, Mustafa Inc, Serge Y. Doka, Thomas Bouetou Bouetou, Optical and W-shaped bright solitons of the conformable derivative nonlinear differential equation, 2021, 20, 1569-8025, 1739, 10.1007/s10825-021-01758-9
28.	Manish Goyal, Amit Kumar Saraswat, Amit Prakash, Numerical analysis of fractional coronavirus model with Atangana-Baleanu derivative in Liouville-Caputo sense, 2023, 97, 0973-1458, 147, 10.1007/s12648-022-02409-w
29.	Kazem Nouri, Hassan Ranjbar, Dumitru Baleanu, Leila Torkzadeh, Investigation on Ginzburg-Landau equation via a tested approach to benchmark stochastic Davis-Skodje system, 2021, 60, 11100168, 5521, 10.1016/j.aej.2021.04.040
30.	Vinod Kumar Bhardwaj, Manish Goyal, A Reliable Solution of Nonlinear Time Dependent Fractional Model of Ebola Virus Disease with Arbitrary Order Derivative in Liouville–Caputo Sense, 2021, 7, 2349-5103, 10.1007/s40819-021-01200-5
31.	A. DLAMINI, EMILE F. DOUNGMO GOUFO, M. KHUMALO, CHAOTIC BEHAVIOR OF MODIFIED STRETCH–TWIST–FOLD FLOW UNDER FRACTAL-FRACTIONAL DERIVATIVES, 2022, 30, 0218-348X, 10.1142/S0218348X22402071
32.	Amit Prakash, Hardish Kaur, Numerical simulation of coupled fractional‐order Whitham‐Broer‐Kaup equations arising in shallow water with Atangana‐Baleanu derivative, 2022, 0170-4214, 10.1002/mma.8238
33.	Ajay Kumar, Haci Mehmet Baskonus, Amit Prakash, 2023, Chapter 14, 978-3-031-29958-2, 232, 10.1007/978-3-031-29959-9_14
34.	MUHAMMAD AZEEM, MUHAMMAD FARMAN, MARWAN ABUKHALED, KOTTAKKARAN SOOPPY NISAR, ALI AKGÜL, EPIDEMIOLOGICAL ANALYSIS OF HUMAN LIVER MODEL WITH FRACTIONAL OPERATOR, 2023, 31, 0218-348X, 10.1142/S0218348X23400479
35.	Amit Prakash, Vijay Verma, Dumitru Baleanu, Two Novel Methods for Fractional Nonlinear Whitham–Broer–Kaup Equations Arising in Shallow Water, 2023, 9, 2349-5103, 10.1007/s40819-023-01497-4
36.	Viswanathan Padmavathi, Kandaswami Alagesan, Samad Noeiaghdam, Unai Fernandez-Gamiz, Manivelu Angayarkanni, Vediyappan Govindan, Tobacco smoking model containing snuffing class, 2023, 9, 24058440, e20792, 10.1016/j.heliyon.2023.e20792
37.	A. Dlamini, E. F. Doungmo Goufo, M. Khumalo, Strongly perturbed bondorbital attractors for generalized systems, 2025, 35, 1054-1500, 10.1063/5.0249237

Reader Comments

Your name:*

Email:*
© 2022 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.1

Metrics

Article views(3137) PDF downloads(129) Cited by(5)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(1)

AIMS Mathematics

Analytical solutions of incommensurate fractional differential equation systems with fractional order $1 < \alpha, \beta < 2$ via bivariate Mittag-Leffler functions

Related Papers:

Abstract

1. Introduction

2. Main definitions

3. First definition: Caputo-Fabrizio derivative (CFD)

3.1. Convergence

3.1.1. Existence of a unique solution

3.1.2. Convergence proof

3.1.3. Error estimation

3.2. SIR epidemic model of arbitrary orders with CFD

4. Second definition: Caputo derivative (CD)

5. Convergence

5.1. Existence of a unique solution

5.2. Convergence

5.3. Error estimation

5.4. SIR epidemic model with arbitrary order containing CD

6. Third definition: Atangana-Baleanu derivative (ABD)

6.1. Analysis of convergence

6.1.1. Existence of a unique solution

6.1.2. Convergence proof

6.1.3. Error estimation

6.2. SIR epidemic model of arbitrary orders containing ABD

7. Comparison between the three definitions

8. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Analytical solutions of incommensurate fractional differential equation systems with fractional order 1<α,β<2 1 < \alpha, \beta < 2 via bivariate Mittag-Leffler functions

Related Papers:

Abstract

1. Introduction

2. Main definitions

3. First definition: Caputo-Fabrizio derivative (CFD)

3.1. Convergence

3.1.1. Existence of a unique solution

3.1.2. Convergence proof

3.1.3. Error estimation

3.2. SIR epidemic model of arbitrary orders with CFD

4. Second definition: Caputo derivative (CD)

5. Convergence

5.1. Existence of a unique solution

5.2. Convergence

5.3. Error estimation

5.4. SIR epidemic model with arbitrary order containing CD

6. Third definition: Atangana-Baleanu derivative (ABD)

6.1. Analysis of convergence

6.1.1. Existence of a unique solution

6.1.2. Convergence proof

6.1.3. Error estimation

6.2. SIR epidemic model of arbitrary orders containing ABD

7. Comparison between the three definitions

8. Conclusions

Author contributions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog

Analytical solutions of incommensurate fractional differential equation systems with fractional order $1 < \alpha, \beta < 2$ via bivariate Mittag-Leffler functions