An analysis for a special class of solution of a Duffing system with variable delays

1.   Introduction

As it is known, Duffing equations explain the motion of a mechanical system in the field of twin well potential. There are many mathematical methods and techniques to solve Duffing equation and Duffing oscillator (see ^{[18,19,22,23]}). However, due to their potential applications in mechanics, physics, electric distribution lines, and engineering, topic of the dynamic behaviors of Duffing differential equations has also gained considerable importance. (see ^{[4,5,7,11,14,17,20]}). Considering literature, it can be seen that many studies have been applied on qualitative behavior of solutions such as oscillation, periodicity, almost periodicity (AP), and pseudo almost periodicity (PAP) ^{[1,2,3,6,7,8,9,10,12,13,15,16,21,24,25]}. Authors of ^[10] considered the following constant coefficients Duffing equation with variable delay:

where $l(t)$ and $e(t)$ are almost periodic functions and $n$ is an integer, $a, {\kern 1pt} {\kern 1pt} b$ and $c$ are constants. By defining $p(t) = \varsigma '(t) + \xi \varsigma (t) - {\Psi _1}(t)$ and ${\Psi _2}(t) = - {\Psi '_1}(t) + e(t) + (\xi - c){\Psi _1}(t), {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} \xi > 1$.

Researchers achieved some results for almost periodic solutions of (1.2). ^[9] extended (1.2) to the following system

where $b(t) \ne 0$ and $\gamma (t) > 0.$ In ^[9], some suitable conditions were obtained on PAP solutions of (1.3). S. Bochner in ^[3] introduced the theory of AA to the literature. Additionally, Xiao et al. ^[12] introduced the concept of PAA, which is a generalization of the AP, AA, and PAP. If the considered output is not showing periodic, AP or AA can check whether its behavior is PAA or not ^[26].

In this study, we consider the following variable coefficient Duffing system:

Let $\tilde c \in {R^ + }$. Define $p(t) = \varsigma^{\prime}(t)+\tilde{c} \varsigma(t)$, (1.4) can be converted into the following equivalent system

As far as we know from the literature, there is no study related to the PAA solutions of the system (1.5). Our purpose in this study is to obtain some suitable criteria for the existence, uniqueness, and globally exponential stability of PAA solutions of system (1.5). The results obtained are new and complementary to the previous studies.

2.   Preliminary results

Define the following notations: $\left\{ {{w_i}(t)} \right\} = \left({{w_1}(t), {w_2}(t)} \right) \in {R^2}$, $\left| w \right| = \left\{ {\left| {{w_i}(t)} \right|} \right\}$ and $\left\| {w(t)} \right\| = \mathop {\max }\limits_{1 \leqslant i \leqslant 2} \left\{ {\left| {{w_i}(t)} \right|} \right\}.$ Let $BC(R, R)$ denote collection of bounded continuous functions. $BC(R, R)$ is Banach space with norm ${\left\| \theta \right\|_\infty } = {\sup _{t \in R}}\left| {\theta (t)} \right|$. Also, we use the notations ${\theta ^ + } = {\sup _{t \in R}}\left| {\theta (t)} \right|, {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\theta ^ - } = {\inf _{t \in R}}\left| {\theta (t)} \right|, {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$ where $\theta \left(t\right)\in BC(\mathbb{R}, \mathbb{R}).$

Definition 2.1. ^[8] Let $f\in C(\mathbb{R}, \mathbb{R}).$ For $f$ to be AP it is necessary and sufficient that the family of functions $H = \left\{ {{f^h}} \right\} = \left\{ {f(t + h)} \right\},$ $- \infty < h < \infty,$ is compact in $C(R$).

Definition 2.2. ^[2] $Q \in C(R, R)$ is called AA if for every real sequence $\left(v_{n}\right)$ there exists a subsequence $\left(v_{n_{k}}\right)$ such that$\lim \limits_{n \rightarrow \infty} \mathrm{Q}\left(t+v_{n_{k}}\right)=P(t)$ and $\lim \limits_{n \rightarrow \infty} P\left(t-v_{n_{k}}\right)=Q(t)$ for each $t \in R$.

Example 2.1. Let $\upsilon \left(t\right) = 2+\mathit{sin}\sqrt{5}t+\mathit{sin}t$ and $g\left(t\right)\in \mathbb{R}$ such that $g\left(t\right) = \mathit{cos}\frac{1}{\upsilon \left(t\right)}$. Then $g(t) \in AA(R, R)$, but $g(t)$ is not $AP(R, R)$. The graph of $g(t)$ on the analytical plane can be seen in Figure 1.

Definition 2.3. ^[2] $f \in C(R, R)$ is called PAA if it can be noted as $f = f_{1}+f_{2}$ with $f_{1} \in A A(R, R)$ and $f_{2} \in P A A_{0}(R, R)$ where space $P A A_{0}$ is defined by

Lemma 2.1. ^[3] $PAA(R, R)$ is a Banach space with norm ${\left\| {{\kern 1pt} \theta {\kern 1pt} } \right\|_\infty } = {\sup _{t \in R}}\left| {\theta (t)} \right|$.

It is clear that $AA(R, R) \subset$ $PAA(R, R)$ $\subset BC(R, R).$

Example 2.2. $f(t) = g(t) + {e^{ - 2t}}$ is PAA function but it is not $AA(R, R)$. The graph of $f(t)$ on the analytical plane can be seen in Figure 2.

Lemma 2.2. ^[1] If $\text { If } \quad l(t) \in A A(R, R)$ and $\Phi(t), \Upsilon(t) \in P A A(R, R)$ then $\gamma(t-k), \Phi(t) \times \Upsilon(t), \Phi(t)+\Upsilon(t), \Upsilon(t-l(t)) \in P A A(R, R)$

Assume the following conditions for our main results.

$({B_1})$ $n > 1$ and $\bar a = \min \left({\tilde c, \inf {k_1}(t)} \right)$,

$({B_2})$ ${\kern 1pt} {k_1}(t){\kern 1pt}, l(t)$ $\in {\kern 1pt} {\kern 1pt} AA(R, {R^ + }),$ and ${k_2}(t), {\kern 1pt} {\kern 1pt} {\kern 1pt} {k_3}(t)$, $e(t)$ $\in {\kern 1pt} {\kern 1pt} PAA(R, R)$ for all $t\in \mathbb{R}$,

$({B_3})$ $\gamma = \frac{{{{\sup }_{t \in R}}~~~\left| {e(t)} \right|}}{{k_1^ - }},$ $\upsilon = \frac{1}{{\bar a}}\max \left\{ {1, {{\sup }_{t \in R}}\left| {\tilde c{k_1}(t) - {{\tilde c}^2} + {k_2}(t)} \right| + {{\sup }_{t \in R}}\left| {{k_3}(t)} \right|} \right\}$, $\frac{\gamma }{{1 - \upsilon }} < 1,$ $\pi = \frac{1}{{\bar a}}\max \left\{ {1, \mathop {\sup }\limits_{t \in R} \left| {\left[{\left| {\tilde c{k_1}(t) - {{\tilde c}^2} + {k_2}(t)} \right| + n{{\left[{2\gamma /(1 - \upsilon)} \right]}^{n - 1}}\left| {{k_3}\left(t \right)} \right|} \right], 1} \right|} \right\} < 1,$

$({B_4})$ $\min \left\{ {\tilde c, k_1^ - + \sup \left({\left| {\tilde c{k_1}(t) - \tilde c + {k_2}(t)} \right| + n{{\left[{2\gamma /(1 - \upsilon)} \right]}^{n - 1}}\left| {{k_3}(t)} \right|} \right){\kern 1pt} } \right\} > 0.$

3.   PAA solutions

Theorem 3.1. Suppose that $\left(B_{1}\right)-\left(B_{3}\right)$ are satisfied. Define a nonlinear operator $G$ for each $\varphi = \left(\varphi_{1}, \varphi_{2}\right) \in P A A\left(R, R^{2}\right), \quad(G \varphi): = \varphi(t)$ where

where

Then $G\varphi \in PAA(R, {R}^{2})$.

Proof. Noting that $G\varphi \in PAA(R, {R}^{2}),$ then it follows from Definition 2.2 such that

Thus,

Firstly, we prove that $\left({{G_1}\varphi } \right) \in AA(R, {R^2})$.

Let $\left({{{a'}_n}} \right) \subset R$ be a sequence. We can extract a subsequence $\left({{a_n}} \right)$ of $\left({{{a'}_n}} \right)$ such that

Define

Then, we have

Using Lebesgue Dominated Convergence Theorem in ^[6], we get

Similarly, we can write

which implies that $\left({G}_{1}\varphi \right)\in AA(R, {R}^{2}).$

Secondly, we have

It can be clearly seen that

Similarly, we get

Then,

which implies that $\left({{G_2}\varphi } \right) \in A{A_0}(R, {R^2}).$ Thus, $G\varphi \in PAA(R, {R^2}).$

Theorem 3.2. Let conditions of $({B_1}) - ({B_3})$ hold. Then, system (1.5) has a unique PAA solution in the following region:

where

Proof. Since PAA functions are uniformly continuous on $\mathbb{R}$, one can get $\tilde C \subseteq PAA(R, {R^2})$.

Let ${\varphi _1} = {\left({{\varphi _{11}}, {\varphi _{12}}} \right)^T} \in \tilde C$. According to Lemma 2.2 and condition $({B_2})$, we have

Thus, from Lemma 1 and Lemma 2 in ^[2], we know that the auxiliary the system (1.5) has a unique PAA solution:

Define a mapping $\Sigma :\tilde C \to PAA(R, {R^2})$, by setting $\left({\Theta {\varphi _1}} \right) = {\left({{\varphi _{11}}, {\varphi _{12}}} \right)^T}.$

It is clear that

Also, ${\left\| {{\varphi _1}} \right\|_\infty } \leqslant {\left\| {{\varphi _0}} \right\|_\infty } + \left\| {{\varphi _1} - {\varphi _0}} \right\| \leqslant \gamma + \frac{{\gamma \nu }}{{1 - \nu }} = \frac{\gamma }{{1 - \nu }} < 1.$

Therefore, we get

where ${\gamma ^\eta }(\eta) = (\tilde c({k_1}(\eta) - \tilde c) + {k_2}(\eta)){\varphi _{11}}(\eta) - {k_3}(\eta)\varphi _{11}^n(\eta - \tau (\eta)).$ Hence,

Therefore, $\left({\Sigma {\varphi _1}} \right) \in \tilde C$. So $\Sigma :\tilde C \to \tilde C$ is a self-mapping. For all ${\varphi _1}, {\varphi _2} \in \tilde C$

where $0 < m\left(\eta \right) < 1.$ Then,

Since $\pi < 1$, $\Sigma$ is a contraction mapping. Therefore, $\Sigma$ has a fixed point $\tilde s(t) = (\tilde \varsigma (t), \tilde \rho (t))$ in the set $\tilde C$ such that $\Sigma {\kern 1pt} \tilde s = \tilde s$ which is the PAA solution.

4.   Globally exponential stability of PAA solutions

Theorem 4.1. Let ${\kern 1pt} \tilde s(t) = (\tilde \varsigma (t), \tilde \rho (t))$ be a unique PAA solution of (1.5) with initial function $({\tilde \varphi _1}(t), {\tilde \varphi _2}(t))$ and suppose conditions of Theorem 3.2. Then, $\tilde s(t)$ solution of (1.5) is globally exponential stability.

Proof. Due to the condition $({B_4})$, a constant $\zeta > 0$ can be found such that

Let ${\kern 1pt} s(t) = (\varsigma (t), \rho (t))$ be an arbitrary solution of (1.5) with initial function $({\varphi _1}(t), {\varphi _2}(t))$. Set ${A_1}(t) = \varsigma (t) - \tilde \varsigma (t)$, ${A_2}(t) = p(t) - \tilde p(t)$. Then,

Consider the following Lyapunov functions

Computing ${\left({{{U'}_i}(t)} \right)^ + }$ along the solution $({A_1}(t), {A_2}(t))$ of system (1.5) with the initial function $({\varphi _1}(t) - {\varphi _2}(t), {\tilde \varphi _1}(t) - {\tilde \varphi _2}(t))$, we get

Let $N>1$ is constant and set

It follows from (1.8) that

We claim that

Otherwise, the following two situations arise.

Case a. ${t}_{1}>0$ can be found as

Case b. ${t_2} > 0$ can be found as

If Case a holds, (1.7), (1.9), and (1.12) shows that

Thus, $\lambda \geqslant - \tilde c$ and this contrasts with (1.7).

If Case b holds, (1.7), (1.10), and (1.13) refers that

Hence, $\varsigma - k_1^ - + \sup \left({\left| {\tilde c{k_1}({t_2}) - {{\tilde c}^2} + {k_2}({t_2})} \right| + {{\left({2\gamma /(1 - \upsilon)} \right)}^{n - 1}}\left| {{k_3}({t_2})} \right|} \right) \geqslant 0$ and this contrasts with (1.7).

Thus, (1.11) holds. As a result, we get

5.   An application

Let $n = 2,$ $l(t) = \left| {\sin t} \right|$, $\tilde c = 25,$ ${k_1}(t) = 25 + cos\frac{1}{{2 + \omega (t)}}$, ${k_2}(t) = cos\frac{1}{{2 + \omega (t)}} + {e^{ - 2t}},$ ${k_3}(t) = \sin \frac{1}{{2 + \omega (t)}} + {e^{ - 2t}}$, $e(t) = cos\frac{1}{{2 + \omega (t)}} + {e^{ - 2t}}$, $\omega (t) = \sin t + \sin \sqrt 2 t$. If these notions are written in (1.4),

is obtained. Then, (1.17) has exactly one PAA solution.

Set $p(t) = \varsigma '(t) + 25\varsigma (t)$, then, (1.17) can be converted to the following system such that

Then, $\gamma = \frac{2}{{25}} < 1$, $\upsilon = \frac{4}{{25}} < 1$ and $\pi = \frac{{22}}{{175}} < 1.$

Consequently, whole conditions of Theorem 3.3 are satisfied; therefore, (1.18) has a unique globally exponential stable PAA solution in the following region:

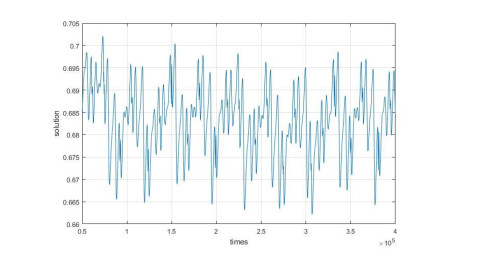
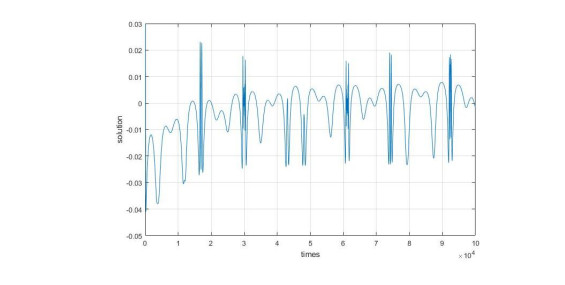
The numerical simulations for the state vectors of (1.18) are as follows in Figures 3 and 4:

6.   Conclusions

We remark that there is no phenomenon that is purely periodic in nature, and this gives the idea of the AP oscillation, the PAP oscillation, the WPAP oscillation, the automorphic oscillation, and the PAA oscillation. As seen in Example 2.2, the set of PAA functions is more extensive than the known sets of AP, PAP, and AA. Therefore, in this study, some important results are obtained regarding the PAA solutions of the discussed Duffing differential equation model. Some differential inequalities and the well-known Banach fixed point theorem are used for the existence and uniqueness of PAA solutions. Also, with the help of Lyapunov functions, sufficient conditions are obtained for globally exponential stability of PAA solutions. As a result, the main results of the study are new and complementary to the previous studies.

Conflict of interest

The author declares no conflicts of interest in this paper.