The role of physical activity in the physiological activation of the scholastic pre-requirements

1.   Introduction

Manipulators imitate human arms to grab and either carry objects or perform tasks in dangerous environments with fixed procedures (see References ^[1,2,3,4,5]). In terms of campus education and intelligent construction, it is important to deepen the application of manipulators in teaching management and research, skills training, campus safety and logistics distribution (see Reference ^[6]). The new generation of robot technology needs to adopt flexible and light materials and design them into slender structures. A flexible system is more susceptible to external disturbances and deformation, which undoubtedly increases the difficulty of control and reduces the accuracy of control. (see References ^{[7,8,9,10,11]}).

As we all know, a flexible manipulator (FM) has the characteristics of strong coupling and high nonlinearity (see References ^[12,13].) Therefore, the research on anti-disturbance control of FM has always been a hot issue in the field of control engineering (see References ^[14,15,16]). In Reference ^[17], an adaptive sliding mode fault tolerant control (ASMFTC) approach based on the Takagi-Sugeno (TS) fuzzy disturbance observer (TSFDO) is presented as an attitude control system (ACS) under environmental disturbance torque and elastic modal generated by flexible appendages. In recent decades, some scholars have designed many control methods for FM, such as Proportional-Integral-Derivative (PID) control (see References ^[18,19,20]), adaptive control (see References ^[21,22]), sliding mode control (see References ^[23,24]) and so on. In recent years, alongside the rapid development of artificial intelligence, some intelligent algorithms have also appeared in the design of system controllers. A fuzzy PID positioning controller based on particle swarm optimization (PSO) is designed to improve the robustness of manipulator control system. This method is very interesting and has a good engineering application value (see Reference ^[25]). Similar to Reference ^[25], in Reference ^[26], a PSO active disturbance rejection control (ADRC) algorithm is proposed to solve the problem that parameters depend on manual experience adjustment, thus further improving the robustness of underwater vehicle.

However, most of the documents mentioned above are based on ordinary differential equations (ODEs). Although the ODE model is relatively simple, there are some defects in describing the dynamic characteristics. Especially for distributed parameter systems (DPS) such as FM systems, the applicability of the ODE model is poor. The dynamic characteristics of FM systems are usually described by PDEs, so it is difficult for traditional rigid system control strategies to be directly applied to FM systems. FM is essentially a DPS. At present, there are three commonly used control methods for DPS (see References ^[27,28]), including, modal control (see Reference ^[29]), distributed control (see Reference ^[16]) and boundary control (see Reference ^[30]). However, distributed control requires a considerable number of actuators and sensors, which undoubtedly increases the difficulty of the controller design. Compared with distributed control, boundary control is a more effective control method.

There are essential differences between ODEs and PDEs. If the unknown function is a univariate function, it is called an ODE, and if the unknown function is a multivariate function, it is called a PDE. The asymptotic behavior of the partial states of coupled ODEs and PDEs is studied in ^[31]. In order to solve the vibration suppression control problem of an FM system modeled by PDEs, the boundary vibration deflection constraint problem is solved by using a barrier Lyapunov function (BLF). Under this control method, not only is the control efficiency is improved, but the system also has good robustness (see Reference ^[32]). In Reference ^[33], the complex satellite attitude system model is truncated based on ODEs, and then an adaptive fault-tolerant control method based on disturbance observer is proposed for the satellite attitude control system subject to elastic model and external disturbances. Different from the above documents, in ^[34], a boundary output feedback controller based on PDEs is designed for one-dimensional Euler-Bernoulli beams with general external disturbances. In Reference ^[35], according to the extended HP, the flexible hose for aerial refueling is modeled as a DPS described by PDEs. Then, based on the original PDEs, a scheme to adjust the vibration of the hose is proposed. Numerical simulations verify that effectiveness of the proposed boundary control method. In ^[36], based on the PDEs, the dynamic model of the flexible system is established, and a vibration observer which can estimate the infinite state is designed to increase the stability of closed-loop system. In ^[37], boundary compound controller based on output feedback is proposed to solve the control problems caused by the infinite dynamic model of FM. Similar to Reference ^[37], the dynamic model of a rigid-flexible manipulator with ODEs-PDEs parameter uncertainty is established by HP. Different from the traditional PD control method, a boundary control scheme based on adaptive iterative learning is proposed to deal with unmodeled dynamics and unknown external disturbances (see Reference ^[38]).

Inspired by the literatures above, one must realize the vibration suppression of FM under external disturbances. In this paper, the PDE dynamic model of an FM system is established by HP. Furthermore, a PDBC based on a PDE model can effectively realize the control of flexible system. The main contributions of this paper are as follows:

1) Different from the dynamic research of traditional rigid manipulators (see References ^{[39,40,41,42,43]}), FM belongs to DPS in essence. In this paper, the flexible manipulator is modeled based on PDEs, which can describe the dynamic characteristics of FM more accurately. Furthermore, the problem of overflow instability caused by modeling based on ODEs are avoided.

2) For the control of DPS, boundary control (see References ^{[44,45,46,47]}) can effectively realize the control of an FM system. Compared with the discrete distributed control, boundary control only needs a few actuators to achieve an improved control effect.

3) The boundary control is carried out at the end boundary of the FM, and the PDBC law is designed by designing Lyapunov function to meet the requirements of $y\left({x, t} \right) \to 0$ and $\dot y\left({x, t} \right) \to 0$. Using the boundary control based on Lyapunov direct method, the control performance of the system will be further improved.

The remainder of this paper is organized as follows. In Section II, the PDE model of the FM is established based on HP. In Section III, by designing a Lyapunov function, the boundary control law based on PD is designed to adjust the vibration of the FM. In Section IV, the numerical comparison simulation based on MATLAB/SIMULINK further verifies the robustness and anti-disturbance performance of the control method proposed in this paper. Finally, the paper is summarized in Section V.

2.   Modeling of flexible manipulator

2.1.   Mathematical description

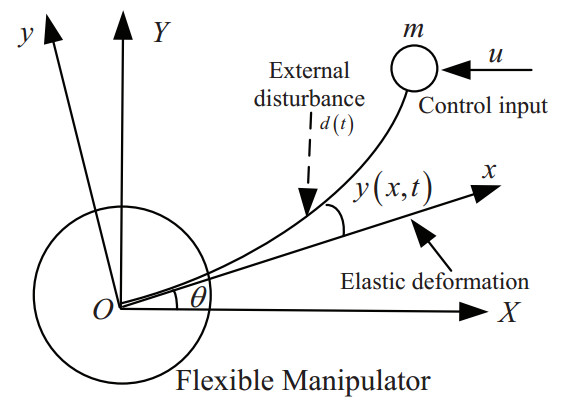
The control research of FM is mostly based on the ODE dynamic model. The advantage of HP is to avoid a complicated force analysis of the system, and not only the PDE equation of the system but also the corresponding boundary conditions of the system can be directly obtained by mathematical derivation. The research object is a single-link FM that moves horizontally, which is shown in Figure 1. From Figure 1, we can see that the end of the flexible mechanical arm has a boundary to control the input $u(t)$ and that the external disturbance $d(t)$. $y(x, t)$ represents the elastic deformation at the $x$ point.

Remark 1: For clarity, notations, the time $t$ symbol is omitted in the full-text function variable. For example, $\theta \left(t \right) = \theta$, ${\left(* \right)_x} = \frac{{\partial \left(* \right)}}{{\partial x}}$, ${\left(* \right)_t} = \frac{{\partial \left(* \right)}}{{\partial t}}$.

2.2.   Modeling based on PDE

$y\left({0, t} \right)$ is obtained by bending the origin flexibly to zero at any time. ${y_x}\left({0, t} \right)$ can be obtained from the zero change rate of the origin flexible bending along the $x$ axis at any time, and the boundary condition is expressed as

Any point $\left[ {\begin{array}{*{20}{c}} x & {y\left({x, t} \right)} \end{array}} \right]$ on the FM in the follow-up coordinate system, $xOy$ can be approximately expressed in the inertial coordinate system $XOY$ as

where $\eta\left(x \right)$ is the offset of the FM.

According to Eqs (2.1) and (2.2), it can be seen that

According to HP,

where, ${\delta {Q_k}}$, ${\delta {Q_p}}$ and ${\delta {Q_n}}$ represent the variation of kinetic energy (KE), potential energy (PE) and non-conservative force (NF), respectively. $\theta \left(t \right)$ is the joint rotation angle without considering elastic deformation and $y\left({x, t} \right)$ is the elastic deformation of the FM at point $x$.

The rotational KE of the flexible joint is $\frac{1}{2}{I_h}{\dot \theta ^2}$, and the kinetic energy and load kinetic energy of the FM are $\frac{1}{2}\int_0^L {\rho {{\dot \eta}^2}\left(x \right)} dx$ and $\frac{1}{2}m{\dot \eta^2}\left(L \right)$, respectively,

where $L$ is the length of the FM, ${I_h}$ is the central moment of inertia, $m$ is the terminal load mass of the FM and $\rho$ is the mass per unit length of the rod.

The total KE of the FM is

The PE of a FM can be expressed as

where $EI$ is the bending stiffness of the uniform beam. For the convenience of writing, we will abbreviate $EI$ as $\varpi$.

The NF work of the system is expressed as

where $\tau$ is the motor control input torque at the initial end point and $F$ is the motor control input torque of the end load.

The first item of Eq (2.6) is expanded

with

Then, we can obtain the following equation

where $\int_0^L \int_{t_1}^{t_2} \rho \ddot{\eta}(x) \delta \eta(x) \mathrm{d} t \mathrm{\; d} x = \int_{t_1}^{t_2} \int_0^L \rho \ddot{\eta}(x) \delta \eta(x) \mathrm{d} x \mathrm{\; d} t$.

Then,

According to ${\eta _{xx}}\left(x \right) = {y_{xx}}\left(x \right)$, and then expand the second item of Eq (2.6), the following is obtained:

Finally, the third item of Eq (2.6) is expanded to obtain

According to the above analysis, we can get

According to $\eta (0) = 0$, ${\eta _x}(0) = \theta$, ${\ddot \eta _x}(0) = \ddot \theta$ and $\frac{{{\partial ^n}\eta \left(x \right)}}{{\partial {x^n}}} = \frac{{{\partial ^n}y\left(x \right)}}{{\partial {x^n}}}$, we can subsequently obtain the following equation:

where

According to HP, have

Therefore, the PDE dynamic model is as follows:

where, $\ddot \eta \left(x \right) = x\ddot \theta + \ddot y\left(x \right)$, $\ddot \eta\left(L \right) = L\ddot \theta + \ddot y\left(L \right)$.

2.3.   Control objective

Considering that the dynamic characteristics of the flexible systems cannot be accurately described based on ODEs, this paper establishes a PDE dynamic model of complex flexible systems based on HP. The PDBC is designed to adjust the vibration of the FM and realize $y\left({x, t} \right) \to 0$, $\dot y\left({x, t} \right) \to 0$.

In order to better show the control logic, Figure 2 shows the control structure of this paper. The control structure diagram includes an attitude measurement sensor, an actuator, an FM based on PDE modeling and a boundary controller.

3.   Boundary controller design

The greater the elastic vibration of the FM, the more likely it will lead to the instability of the manipulator system. Therefore, understanding how to suppress the elastic vibration of the FM is challenging.

Lemama 1 ^[48]: Let ${\Im _1}\left({x, t} \right)$, ${\Im _2}\left({x, t} \right) \in R$, $\forall \left({x, t} \right) \in \left[ {\begin{array}{*{20}{c}} 0&L \end{array}} \right] \times \left[ {\begin{array}{*{20}{c}} 0 & \infty \end{array}} \right]$, the following inequalities hold:

where $\lambda > 0$.

Lemama 2: For $\aleph \left({x, t} \right) \in R$, $\forall \left({x, t} \right) \in \left[ {\begin{array}{*{20}{c}} 0&L \end{array}} \right] \times \left[ {\begin{array}{*{20}{c}} 0 & \infty \end{array}} \right]$. If $\aleph \left({0, t} \right) = 0, t \in \left[ {\begin{array}{*{20}{c}} 0 & \infty \end{array}} \right)$, then

Similarly, if ${\aleph _x}\left({0, t} \right) = 0, t \in \left[ {\begin{array}{*{20}{c}} 0 & \infty \end{array}} \right)$, then

Lemama 3: Let $\Xi :\left[ {\begin{array}{*{20}{c}} {\rm{0}} & \infty \end{array}} \right) \in R{\rm{ }}t \ge {t_0} \ge 0$, if $\dot \Xi \le - \varsigma \Xi + \wp$, then

where $\varsigma > 0$.

3.1.   The design of boundary control law

For the PDE model (Eqs (2.17)–(2.20)), in order to realize the vibration angle response of the manipulator and restrain the vibration deformation of FM, the boundary control law is selected as follows:

where ${k_p} > 0$, ${k_d} > 0$ and ${k} > 0$.

Then,

Let

where $\theta _d$ is an ideal angle and a constant value.

Theorem 1: With control laws Eqs (3.6) and (3.7), the closed-loop system is stable. For $t \to \infty$, $x \in \left[ {0, L} \right]$, have $\theta \to {\theta _d}, \dot \theta \to 0$, $y\left(x \right) \to 0, \dot y\left(x \right) \to 0$.

Proof: Select the Lyapunov function

where

where, ${\Phi _1}$ is the sum of the KE and PE of the FM, indicating the inhibition index for the bending deformation and bending change rate of the FM. The first two items in ${\Phi _2}$ represent the error index of control, and the third item is an auxiliary item. ${\Phi _3}$ is an auxiliary item. $\alpha$ is a very small positive real number and has

According to Lemma 1, we can get

According to Lemma 2, we can obtain

Then,

where

and

Select $0 < {\alpha _1} < 1$, it means that $0 < \max \left({2\alpha L, \frac{{4\alpha \rho {L^3}}}{{\varpi}}, \frac{{2\left({\alpha {I_{\rm{h}}} + 2\alpha \rho {L^2}} \right)}}{{{k_{\rm{p}}}}}, 2\alpha } \right) < 1$.

$\alpha$ can be designed as

Let $0 < 1 - {\alpha _1} = {\alpha _2} < 1$, $1 < 1 + {\alpha _1} = {\alpha _3} < 2$, then

From Eq (3.20), it can be written as

From the Eq (3.21), we can see that the Lyapunov function $V(t)$ is a positive definite function, then

where

where

The Eq (2.22) is brought into the Eq (3.23)

where ${\eta _{xx}}\left(L \right) = 0$, ${{\dot \eta }_x}\left(0 \right) = \dot \theta$.

Then,

and

According to Eqs (2.2)–(2.5), the following can be obtained:

Combining Eqs (3.33) and (3.24),

Substituting the control laws Eqs (3.6) and (3.7), then

Substituting Eq (2.22) into Eq (3.26), then

By integrating the above formula by parts and substituting the Eq (3.14), we can get

where

By using the partial integration method for Eqs (3.39) and (3.40), we can get

Through the above analysis, we can get

Then,

Substituting Eqs (3.1), (3.3) and (3.14) into Eq (3.44), we can get

Then,

According to the Eqs (3.14) and (3.27), the following can be obtained by a partial integral:

It can be obtained from Lemma 1, we have

From Eqs (3.45), (3.49) and (3.50)

Then,

By choosing the appropriate parameter $\alpha$, then we have $\varpi \left({1 - \alpha L} \right) > \frac{1}{2}\alpha \rho L$, and the following equation can be guaranteed to exist:

where ${\vartheta _0} > \max \left({{\vartheta _1}, \frac{{{\vartheta _1}{\vartheta _2}}}{{{\vartheta _2} - {\vartheta _1}}}} \right)$, $\vartheta _1$ and $\vartheta _2$ are appropriate parameters.

The solution of the above inequality Eq (3.52) is

If $V(0)$ is bounded, then when $t \to + \infty$, $V\left(t \right) \to 0$ and converges exponentially. According to Eq (3.21), we have ${\Phi _1} + {\Phi _2} \to 0$, then $e \to 0$, $\dot e \to 0$, $\theta \to {\theta _d}$ and $\dot \theta \to 0$. Furthermore, $\dot \eta\left(x \right) \to 0$ can be obtained, and then $\dot y\left(x \right) \to 0$ and $\eta \left(x \right) = x\theta + y\left(x \right)$.

4.   Simulation examples

4.1.   Two existing methods for comparison

In this paper, an exponentially convergent boundary controller (marked as "PDBC") is constructed in essence based on the PDBC method. In order to demonstrate the superiority of ECBC method, this section gives a distributed parameter boundary control method of flexible manipulator based on LaSalle (marked as "LSBC") for comparison. At the same time, the method based on the PDBC is divided into two forms: open loop-closed loop, which can further verify the simulation effect under the open loop state and the controller design proposed in this paper.

4.2.   PDBC method

In this section, MATLAB/SIMULINK is used for numerical simulation to verify the effectiveness of the exponentially convergent boundary controller (PDBC). The controller is used for boundary control at the end of the FM to adjust the vibration of the FM. The physical parameter of the machine is selected as $\varpi = 2.5 \; N \cdot {m^2}$, and the mechanical arm terminal load mass is $m = 0.25$ kg. The length of the mechanical arm and the mass per unit length of the rod are $L = 0.1$ m and $\rho = 0.3$ kg $\cdot {m^{ - 1}}$, respectively. The center moment of inertia of the mechanical arm is ${I_h} = 0.15$ kg $\cdot {m^2}$. In the simulation based on the PDBC method, in order to further prove the robustness and anti-disturbance performance of the proposed method, this part includes two cases (open loop and closed loop). We take $S = 2$ as the closed-loop test, and $S = 1$ as the open-loop test. At this time, the controllable input torque of the motor at the initial end point is $\tau = 0 \; N \cdot m$. At the end of the load, the control input torque is selected as $F = 0$.

4.3.   LSBC method

On the basis of the original FM based on the PDE model, we consider the simultaneous boundary control at the end of the manipulator. According to HP, if the viscous damping coefficient $\gamma_1$ and $\gamma_2$ caused by the speed signal is considered, then the dynamic model of the FM at this time includes the following three parts:

1) The distributed force balance can be considered

2) The force balance at the boundary point can be obtained

3) The boundary conditions are

Taking the error information of angle signal as follows,

Control objectives: $\theta(t) \rightarrow \theta_{\mathrm{d}}(t), \dot{\theta}(t) \rightarrow \dot{\theta}_{\mathrm{d}}(t), y(x, t) \rightarrow 0, \dot{y}(x, t) \rightarrow 0$, where $\theta_{\mathrm{d}}(t)$ is an ideal angle signal and $\theta_{\mathrm{d}}(t)$ is a constant value.

The design control law (LSBC) is

where $k_{\mathrm{p}} > 0, k_{\mathrm{d}} > 0, k > 0$, $F_1$ is the control input torque of the end point.

Discrete time is taken as $\Delta t = 5 \times 10^{-4} \mathrm{\; s}$, the discrete distance of the manipulator, the physical parameters of the flexible manipulator and the initial state are the same as those of the PDBC method. Select PDE dynamic model such as Eqs (2.22)–(2.25) and ignore the damping term ($\gamma_1 = \gamma_2 = 0$). The LSBC laws are Eqs (4.7)–(4.8). The controller parameters and ideal angles are selected as $\theta_{\mathrm{d}} = 0.50$, $k_{\mathrm{p}} = 50$, $k_{\mathrm{d}} = 30$, $k = 20$.

In this section, the robustness of the proposed control method is further verified by comparative simulation. First, the FM system is divided into open-loop and closed-loop situations. Figure 3 shows the joint angle and angular velocity response of the FM under open loop. When $M = 1$ is selected, it can be seen that there is a certain error between the joint angle and the ideal angle in the open loop. Besides, under open loop, the response diagram of deformation and deformation rate are shown in Figure 4. As can be seen from Figure 4, the FM deformation and deformation rate of the FM are large under open loop, and the plane is not smooth. Taking $S = 2$ as the closed-loop test, the model described in Eqs (2.22)–(2.25) are adopted. The boundary control input based on open loop are shown in Figure 5. Meanwhile, taking ${k_p} = 40$, ${k_d} = 30$, $k = 20$. The response diagram of joint angle tracking and angular velocity under two control methods (PDBC method and LSBC method) in Figure 6. It can be seen from the Figure 6 that although the given signal can be tracked under both control methods, the PCBC method proposed in this paper has faster response.

From Figures 7 and 8, we can see the response diagram of deformation and deformation rate under PDBC method and LSBC method. It can be seen from Figures 7 and 8 that the vibration effect of the flexible manipulator is similar under the two methods. However, it can be seen from Figure 9 that the boundary control input in the PDBC control mode is smoother and more stable.

5.   Conclusions

In this paper, a boundary-based control law is adopted to suppress the flexible vibration of FM. Different from the traditional modeling method based on ordinary differential equations, this paper establishes the PDE dynamic model of FM system by using HP. The boundary control method is used to add boundary control input to the end boundary of FM. By designing a Lyapunov function and the PD boundary control law, the vibration of flexible manipulator can be adjusted. Finally, the effectiveness and robustness of the control method proposed in this paper are further verified by comparative simulation.

Finally, this paper takes Wuxi Vocational College of Science and Technology as an example, relying on its own specialties such as intelligent product development and application, integrated circuits and industrial robots. Meanwhile, the management thought of top-level design is combined with engineering technology, and the FM is taken as the research object, which is devoted to serving and building a smart campus and made contributions to the high-level school construction and high-quality development of Wuxi Vocational College of Science and Technology.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

2021 General Project of Philosophy and Social Sciences Research in Jiangsu Colleges and Universities (Ideological and Political Special Project) "Research on Innovative Path of Integrating College Music Education into Curriculum Ideological and Political Education" (ProjectNo.: 2021SJB0438).

Conflict of interest

The authors declare there is no conflict of interest.