1. Introduction
1.1. Global status of biofuels
Transport sector is a vital part of global climate change mitigation strategies, as it accounts for about 28% of overall energy consumption and for 23% of world energy-related CO2 emissions [1]. The sector is growing more rapidly than most others, with emissions projected to double by 2050 [2]. With rapid economic development, India is experiencing substantial growth in vehicle population and motorised mobility [3,4], which has also led to a strong increase in greenhouse gas (GHG) emissions and air pollution caused by transport sector [5,6,7]. The Indian government has introduced several mitigation targets over the past few years to reduce emissions [8,9,10,11,12]. India's Intended Nationally Determined Contribution (INDC) includes a decarbonisation target of 33–35% of CO2 intensity of it's Gross Domestic Product (GDP) between 2005 and 2030 [12]. As stated in India's National Action Plan for Climate Change (NAPCC), transport emissions can be reduced by adopting low-carbon transport strategies, which includes measures such as increased public transport use, higher penetration of biofuels, enhanced vehicle efficiency [9]. This study looks at the status and technical and economic potential of lignocellulosic biofuels in India, identifies major challenges in achieving the country's biofuel targets, and analyses their role in India's long-term transport scenarios.
In 2015, the global biofuel production increased by just over 1% [13] and biofuels accounted for around 4% of total road transport fuel worldwide [14]. Global biofuel production falls primarily into three categories: ethanol, biodiesel, and hydrotreated vegetable oil (HVO)1. Of the 146 billion litres of biofuel produced globally in 2015 [15], 116 billion litres (79%) were ethanol. Ethanol is produced from fermenting the sugars or starches in feedstocks like corn, wheat, and sugarcane. Fuel ethanol production increased from 17 billion litres in 2000 [13] to 116 billion litres in 2015 globally [15], an average annual growth of approximately 13.6%. Biodiesel is the second largest category of global biofuel, accounting for 31 billion litres globally in 2015—21% of total biofuel production. Global production of biodiesel grew from 0.8 billion litres in 2000 [13] to 30.9 billion litres in 2015 [15].
1Hydrotreated Vegetable Oil (HVO), also called Hydroprocessed Esters and Fatty Acids (HEFA) is a renewable diesel fuel that can be produced from a wide array of vegetable oils and fats.
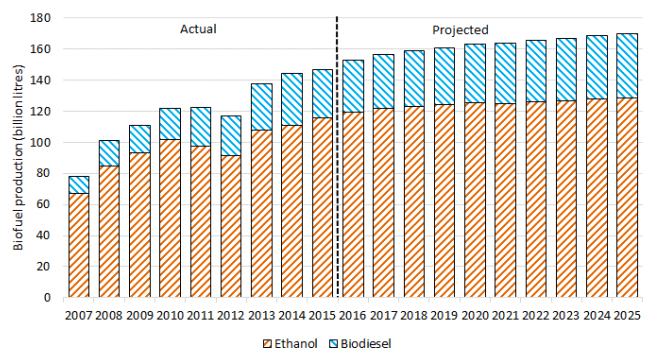
According to the OECD-FAO Agricultural Outlook 2016–2025, the global ethanol production is projected to increase moderately during the outlook period from about 115.6 billion litres in 2015 to nearly 128.4 billion litres by 2025 (Figure 1). More than half of this increase is expected to originate from Brazil mostly to fill domestic demand. In the United States, ethanol production will increase in 2016 and 2017 to meet the stronger demand induced by low crude oil prices and implied higher gasoline use and then decrease slightly because of lower transportation fuels demand. However, the country is expected to remain the major ethanol producer and exporter, followed by Brazil. The expansion of global biodiesel production will be driven by policies in place in the United States, Argentina, Brazil and Indonesia, and to a lesser extent the fulfilment of the Renewable Energy Directive (RED) target in the European Union [16]. It is expected to increase from 31 billion litres in 2015 to 41.4 billion litres by 2025 [15]. It may be noted that India's current biofuel production accounts for less than 1% of global biofuel production [17].
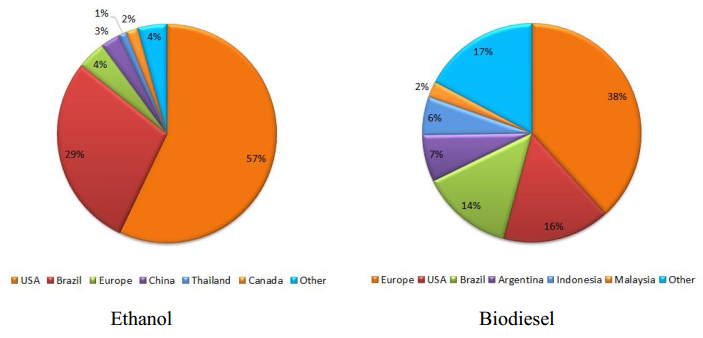
United States and Brazil are the two dominant producer and user of ethanol (Figure 2), accounting for approximately 86% of global ethanol production. The United States has allocated a significant portion of its highly productive agricultural areas to corn production in order to produce low-cost ethanol, whereas Brazil uses sugarcane as a raw material for ethanol production. Biodiesel production is more evenly distributed across different regions, with the top 10 countries accounting for less than 80% of total production as shown in Figure 2.
According to the International Energy Agency (IEA), biofuels have the potential to meet more than a quarter of world demand for transportation fuels by 2050 [18] if favourable policies and investments are in place. At present, biofuels are supported by governments in many different ways, including blending mandates or targets, subsidies, tax exemptions (exemptions from excise and pollution taxes, corporate tax breaks for biofuel producers) and credits, reduced import duties, support for research and development (R & D) and direct involvement in biofuel production, as well as other incentives to encourage local biofuel production and use. As of year-end 2015, biofuel mandates were in place in 66 countries at the national or state level [13].
1.2. National policy on biofuels and achievements
While the development of biofuel in India shows a slow progress as compared to other countries of the world, the Indian biofuel market is expected to increase dramatically with the steadily growing transport sector and the consequent increase in expenditure of petroleum products2 even with its small share (≈1%) in the global biofuel market. In 2016, India achieved its highest ever ethanol market penetration, a gasoline blend rate of 3.3% on average across the country. Ethanol demand was partly met by import (400 million litres of ethanol) in 2016 that is expected to rise approximately 600 million litres through 2018 [17]. Large-scale blending of biodiesel with conventional diesel has not yet begun in India. The market for biodiesel (B100) is nascent and will continue to grow if there is a strong commercially viable strategy for building a sustainable biodiesel industry. Notably, both the private and public sectors claim to be successful in developing and customizing technology for converting lignocellulosic materials into advanced biofuels [19]. Also, trials are still underway to process municipal solid waste [20], micro-algae [21,22] and photosynthetic organisms into advanced biofuels [23,24].
2India is the third-largest consumer of crude oil in the world. India's oil consumption averaged ~4.6 MMbpd (million barrels per day) in 2017, according to the EIA (U.S. Energy Information Administration). The EIA estimates that India's oil consumption could average ~4.8 MMbpd in 2018. The IEA (International Energy Agency) estimates that India's crude oil demand growth rate will be the highest by 2040 [17].
In India, biofuels are promoted with the main objective of achieving energy security [25]. In the initial stage, India's biofuel initiatives were mainly driven by policy actors at the central level [26]. Indian biofuel strategy focused on the use of ethanol and biodiesel, produced from non-food resources. Ethanol is produced from sugar molasses and biodiesel is produced from non-edible oils and oil waste. Ethanol is blended with gasoline while biodiesel is blended with diesel. The practice of blending ethanol under the Ethanol Blending Program (EBP) started in India in 2001. The Government of India (GoI) mandated blending of 5% ethanol with gasoline in 9 States and 4 Union Territories (UTs') in the year 2003 and subsequently mandated 5% blending of ethanol with gasoline on an all-India basis in November 2006 (in 20 States and 8 UTs' except a few North-eastern states and Jammu & Kashmir). GoI launched the National Biodiesel Mission (NBM) in April 2003 (Table 1) with the objectives to bring unutilized wasteland in to productive use by promotion of Jatropha and Pongamia plantation for 20% blending with diesel by 2012 and also generating a renewable source of biofuel, thereby reduce country's dependence on imported petroleum diesel [27]. Approximately 20 biodiesel plants produce 140 to 300 million litres of biodiesel annually [17]. NBM target was unmet due to a host of agronomical and economic constraints. To help fill the gap, several existing biodiesel units shifted operations to adopt multiple feedstock technology, which utilizes used cooking oils' (UCO), other unusable oil fractions, animal fats, and inedible oils; this achieved a minimal (0.001%) blend rate [17]. The growth in biodiesel market is encouraged by deregulated diesel prices, bulk sale of biodiesel (B100) by authorized dealers, and authorization of joint ventures of parastatal Oil Marketing Companies (OMCs) and private manufacturers to supply to bulk consumers only.
Table 1. India's biofuel policies and targets.
| Year |
Policy |
Targets |
| 2001 |
Ethanol blending program |
5% blending in gasoline |
| 2003 |
National biodiesel mission |
20% biodiesel blending by 2011–2012 |
|
Ethanol blending |
5% blending in gasoline-Coverage 9 States, 4 UTs |
| 2005 |
Biodiesel purchase policy by MoPNG |
Cost of biodiesel production higher (20–50%) than purchase price. No sale of biodiesel. |
| 2006 |
Ethanol blending |
5% blending in gasoline-Entire country except J & K and a few NE states |
| 2009 |
National policy on biofuels |
20% biofuels (ethanol and biodiesel) by 2017 |
| 2014 |
Biofuel pricing |
No targets but price bands that OMC can pay for ethanol based on distance from distillery |
| 2015 |
Marketing Rights |
No targets however private biodiesel producers allowed to sell directly if they meet standards |
The announcement of a biofuel purchase policy in 2006 was another important milestone that aimed at pushing the local biofuel industry. Like many other countries around the world, India has endured setbacks in its biofuel program due to supply shortages, sharp fluctuations in oil prices, and global concerns about food security [28]. The restrictive policies, availability of molasses, and cost hampered the fuel ethanol program whereas inconsistent policies, availability of land, choice of non-native crops, yield, and market price have been major impediments for biodiesel implementation [29].
To meet the increasing energy needs of the country and to provide energy Security, National Policy on Biofuels (NPB) was announced in December 2009. Key objectives of the policy are development and utilization of indigenous non-food feedstocks raised on degraded or waste lands, thrust on R & D on cultivation, processing and production of biofuels and a blending mandate of 20% ethanol and biodiesel by 2017 [30]. Although a target for blending fuels has been set, there are no provisions to make blending compulsory. Nevertheless, the NPB did not allow private biofuel manufacturers to market directly. The responsibility for biofuel storage, distribution and marketing is vested in OMCs. Biodiesel manufactures must send their biodiesel to OMC-approved collection centres where quality standards are verified. Price and minimum quality requirements are also laid out in the NPB.
Ethanol production in India has increased from 1.5 to 1.9 billion litres from 2002 to 2016 [31,32]. However, ethanol blends are only available in 13 states and the average blend is approximately 2% [33]. In order to improve the availability of ethanol and eliminate uncertainty regarding both pricing and supply GoI fixed price ranges from US$0.75–0.76/litre3 for ethanol in December 2014 [34]. Further, ethanol produced from cellulosic and lignocellulosic materials were allowed, provided they meet the specifications of the Bureau of Indian Standards (BIS). To increase energy security, GoI is considering a 10% ethanol blend (E10), which could reduce petroleum imports by up to US$3 billion a year [35]. To achieve the planned E10 mandate for 2016, sugar mills have so far signed contracts with OMC's to supply 1.04 billion liters of ethanol during 2015–2016, compared to just 780 million liters signed for 2014–2015 [36]. The volume contracted so far is enough to supply about a 4% blend. Policies remain a challenge for production and supply in certain states, hindering the ability to offer volumes for the mandate.
3Subject to the distance of the of sugar mill from the depot/installation of the OMCs.
In India, large-scale blending of biodiesel with conventional diesel has not yet started. Approximately, 20 biodiesel plants produce 140 to 300 million litres of biodiesel annually [36]. The NBM primarily focused on the expansion of Jatropha cultivation in two phases-demonstration phase and expansion phase, aiming to make the program self-sustainable by producing enough biodiesel to meet the 20% blending target. However, Jatropha-based biodiesel production projects have not been as promising as expected due to insufficient yield and revenue, despite state governments offering farmers a minimum purchase price [29,37,38]. Poor seed processing infrastructure is another bottleneck in the Jatropha value chain in India [39]. In June 2015, GoI made key cabinet decisions on biofuels, including granting marketing rights to private biodiesel manufacturers, provided they meet the quality standards of the Ministry of Petroleum and Natural Gas (MoPNG). An exemption was proposed for B100 biodiesel (pure, unblended biodiesel) to allow private manufacturing companies marketing rights for B100 biodiesel and authorises retailers to sell it directly to consumers. Further, the new policy will also determine the price of biodiesel. With the intention of further promoting biofuels, GoI is exploring the use of a 5% biodiesel blend by bulk users such as railways and defence establishments [40].
1.3. Aim of the paper
In India, ethanol is primarily produced using sugarcane molasses. However, current estimates indicate that sugarcane molasses alone will not be able to meet the mandated requirement of blending [41]. Further, the viability of the first-generation (1st) biofuels production is, however, questionable due to the conflict with the food supply, as well as questions about net GHG balance, net energy balance and water utilization. These discussions have led to growing interest in second-generation (2nd-generation) biofuels [42,43,44,45,46,47]. India's gross cropped area accounts for approximately 59% of the total Indian geographical area of 328 million hectares [48], producing massive amounts of crop residues that could be used for 2nd-generation biofuel production. Depending on the feedstock choice and the cultivation technique, 2nd-generation biofuel production can potentially lower GHG emissions since it is made from forest/agricultural residues, municipal and construction waste, that does not use land dedicated for energy crops. Additionally, sustainably produced, 2nd-generation biofuels can potentially promote rural development and improve economic conditions in developing regions.
This study aims to map the policy landscape for biofuels for India and provide a sound basis of setting blending targets, a key policy instrument in India, for biofuels. The paper analyses the technical and economic potentials for biofuels and the impacts the biofuel diffusion would have on environment and rural employment.
The paper is divided in seven sections. Section 2 presents the methodology used for the assessment of the technical and economic potential of 2nd-generation biofuels in India. Meanwhile India's biofuel policy has taken a top down approach of specifying blending targets therefore Section 3 of the paper analyses what these blending targets would mean for future biofuel demand. The technical potential of 2nd-generation biofuels are presented in Section 4. In section 5 costs and economic potential of biofuels are analysed using an energy system model. Section 6 presents the socio-economic impact of lignocellulosic biofuel industry in India. Finally, implications for policy are discussed in Section 7.
2. Methodology
The paper looks at three interrelated questions: ⅰ) what is the supply of biofuels that is needed for meeting the blending targets mandated by GoI, ⅱ) what would be the technical potential of supply based on the 2nd-generation pathways given that biofuel supply from 1st-generation pathway is inadequate, and ⅲ) what is the economic potential of the biofuels. The first research question is more related to the demand for gasoline and diesel and policy targets for biofuels. Nevertheless, both the technical and economic potential of biofuels require a detailed technical evaluation of surplus biomass feedstock availability for 2nd-generation biofuels and cost of biomass feedstock.
2.1. Surplus ethanol availability for biofuels
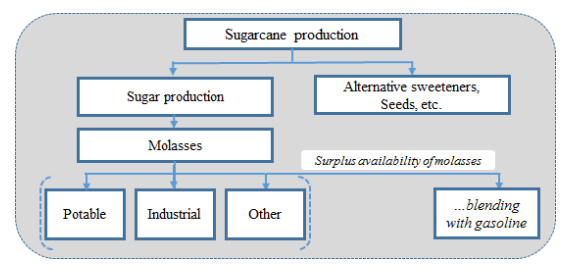
Ethanol is primarily produced by the fermentation of molasses produced during sugar production. Figure 3 presents flow diagram illustrating information required to assess surplus ethanol availability for blending after taking into account molasses used for potable, industrial, and other uses. The molasses production is related to sugar production and since sugarcane is a water intensive the increase in sugar production is taken in line with historical trend and expected to reach 418.8 million tonne (Mt) by 2030 [41]. Also, the demand for potable, industrial and other uses is expected to grow at historical rates and deducted from gross production of ethanol to get the surplus ethanol for blending.
2.2. Assessment of technical potential of 2nd-generation biofuels
The increasing importance of lignocellulosic biomass as a renewable energy (RE) source has led to an acute need for reliable and detailed information on its assessment, consumption and supply. In order to estimate the amount of agricultural residues that can be used for the production of liquid biofuels, we use the methodology developed by Tripathi et al. [49] that was further improved by Purohit et al. [50] after taking into account the crop and its residue production, environmental constraints and their competitive uses. The gross availability of agricultural residues (GARar) essentially depends upon the area under the crop, yield and residue to grain ratio for the crop. Therefore, the GARar can be estimated by using the following equation [50]:
|
GARar=∑m,ni=j=1(Ai,jYi,j)ζi
|
(1)
|
where Ai, j and Yi, j respectively, represent the area and yield of ith crop (I = 1, 2, 3, .... m crop) in the jth state (j = 1, 2, 3, .... n state) and ζi the residue to grain ratio for ith crop.
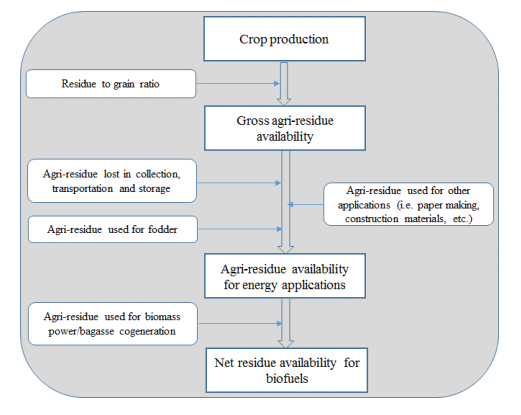
The surplus agricultural residue available for biofuel can be evaluated by introducing certain restrictions on the GARar potential of the crop residues. It has been revealed that the competing uses of a particular crop residue and the harvesting practices have a remarkable influence on the availability of crop residues [51,52]. Moreover, a certain amount of crop residues is also required for retaining the soil fertility [53,54]. Figure 4 presents a flow diagram illustrating information required for assessing biofuel potential through agricultural residue.
Therefore, the surplus agricultural residue availability (SARar) for biofuels can be estimated by using the following equation:
|
SARar=ξce(1−ξfodder)(1−ξoth)(1−ξbp)∑m,ni=j=1(Ai,jYi,j)ζi
|
(2)
|
Where ξce represents the collection efficiency of agricultural residues, ξfodder the fraction of agricultural residues used for fodder applications, ξoth the fraction of agricultural residues used for other applications (i.e., paper industry, construction materials etc.) and ξbp the fraction of agricultural residues used for biomass power/bagasse cogeneration.
Finally, the biofuel potential is estimated as a product of the surplus agricultural residue availability for biofuels (Mt) and biofuel conversion coefficient (litre/tonne).
2.3. Assessment of economic potential
The economic potential for biofuels takes agricultural residue availability for energy applications (Figure 4) as a starting point. The availability of residues is then combined with the cost of supplying the residues at different distances to create a supply curve for crop residues (biomass), explained further in section 5.2. It may be noted that this surplus biomass supply is for both biofuel production and biomass power generation. Apart from availability of biomass the penetration of biofuels would essentially depend on the rate of technological change, discount rates, capital cost of technologies, costs of primary energy (e.g., oil, gas, biomass) etc. [55]. In this study, we have used ANSWER MARKAL model to assess the economic potential of biofuels. ANSWER-MARKAL is a user friendly Windows interface with a gentle learning curve specifically developed for working with the MARKAL energy system model. It is an optimisation model suitable for analysing the national energy system [56]. ANSWER MARKAL model follows the bottom-up modelling paradigm enabling specification of technology details was initially setup for India by Loulou et al. [57] and significant model development in an integrated framework have been crafted. It has been used extensively for India for sectoral analysis [58,59,60,61] and analysis of RE policies [61]. The Indian MARKAL model has a rich characterisation of technologies on both supply and demand side of energy. The model database has a good coverage of transport sector and was used recently for a comprehensive analysis of transport scenarios for India [58].
For a particular demand, the model chooses a technology and fuel mix that minimises the overall system cost. Biofuels are an alternative fuel for transportation and therefore model structure is well suited for carrying the analysis. The demand for transportation is defined exogenously for each time period and for the current analysis the transportation demand is based on the business as usual (BAU) scenario for India from Dhar & Shukla [58]. The model framework and technology costs are described in Dhar & Shukla [58]. The model optimises the overall energy system costs and the results show the least cost solution. The model architecture is suited to assumptions of a perfect market however to model real world complexities constraints are applied e.g., in certain cases it is economical to retire capacities before their end of life however uncertainties in predicting demand, principal agent problem, information asymmetry, etc. can act as a barrier for investments. In such cases the model can be aligned with reality by putting constraints on how capacity can be retired.
In terms of technologies for the 2nd-generation biomass conversion, technologies based on the bio-chemical and thermo-chemical routes were used. Biomass was shown to be available for both the bio-refineries that produce 2nd-generation biofuels as well as those that generate power.
3. Current blending targets and future demand for biofuels in India
As mentioned in the previous section, India's biofuel policy is designed in a top down approach where national governments play a central role in setting targets, institutions, policies for ethanol/biodiesel blending; targets, as well as extends necessary incentives and support to the states and other local governments. The following sub-sections examine demand and supply aspects of India's biofuel policy and feasibility of achieving targets as stipulated by the NPB using the 1st-generation pathway.
3.1. Ethanol demand and supply in India
India is the second largest producer of sugarcane next to Brazil. India currently produces ethanol by the fermentation of sugar molasses (a by-product of sugar industry) and therefore ethanol production depends largely on availability of molasses. Since sugarcane production in India is cyclical, ethanol production also varies accordingly and therefore does not assure optimum supply levels needed to meet the demand at any given time. At times, lower availability of molasses and resultant higher molasses prices affect the cost of production of ethanol, thereby disrupting supply of ethanol for the blending program at pre-negotiated fixed ethanol prices. Approximately, 85–100 kg of sugar (8.5–10%) and 35–45 kg (3.5–4.5%) of molasses can be obtained from one tonne of sugarcane whereas the recovery of ethanol from molasses is 22–25%, as per Indian standards [62]. Theoretically, if the entire sugarcane crop (341.2 Mt in 2010) is used for sugar production, estimated molasses production is 15.4 Mt, and the associated estimated ethanol yield is 3.6 billion litres. Further details are available in the supplementary material (Section S.1). In reality, 70–80% of sugarcane produced in India is used for sugar production, and the remaining 20–30% is used for alternative sweeteners (jaggery and khandsari) and seeds [63]. Moreover, 61% of the available molasses is used in alcoholic beverages, industry and for other applications [41]. The surplus available alcohol is diverted for blending with transportation fuel (Figure 5).
In India, rising per capita income, urbanisation, and infrastructure development has led to increased vehicle density, and consequently, increased demand for gasoline [64]. During the five-year period from 2007 to 2012, demand for gasoline rose by 10.1% [65]. Similarly, the rate of growth in demand for ethanol increased by 3.0% for industrial and other uses and 3.3% for potable use [66]. As the GoI set an ambitious target of 20% ethanol and gasoline blends by 2017 [30], it is important to anticipate ethanol demand so that necessary measures can be taken to ensure sufficient supply.
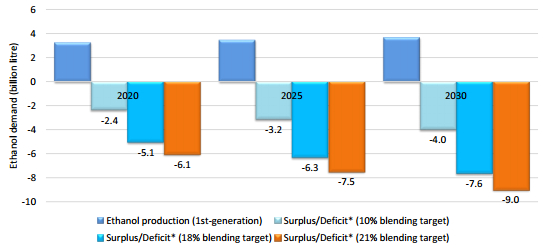
How much ethanol will be required for blending depends both on blending targets and gasoline demand. India's gasoline consumption will increase from 14.2 billion litres in 2010 to 45.6 billion litres in 2030 as per Dhar & Shukla [58], under the BAU scenario. In 2010, ethanol consumption was 1.8 billion litres, out of which only 50 million litres was used for blending [36]. If India is to achieve the 20% blending targets, the country will need to produce 9.1 and 12.3 billion litres of ethanol by 2020 and 2030, respectively. Over 7 billion litres of ethanol would be required by 2017 alone, to meet the NPB targets. Figure 5 presents the gross availability of ethanol derived through molasses route and overall ethanol demand (including potable, industry and other) with different blending targets.
Projections for area and production of sugarcane up to 2030 are derived from sugarcane production data from 1950–1951 to 2011–2012 [67] as shown in the supplement. The net ethanol availability is estimated at 3.2 and 3.6 billion litres in 2020 and 2030 respectively. In order to achieve the 20% blending target [30] without compromising industrial, potable and other needs, India must either increase its ethanol production by nearly three times the present level, or must opt for massive imports of ethanol. Increasing ethanol production to such levels would be extremely challenging, since the country's sugarcane yield has been stagnating at approximately 65–70 tonne/ha [67,68] for the past several years. It also would not be feasible to increase the area for cultivating sugarcane, due to land and water resource constraints. While only molasses is used in India to produce ethanol, its direct production from sugarcane juice would compete with sugar production for the food market.
3.2. Biodiesel demand and availability in India
In order to meet its goal of 20% biodiesel in high speed diesel (HSD) by 2012, the NBM set a target of dedicating 11.2 to 13.4 Mha of land to Jatropha cultivation by the end of its 11th Five-Year Plan [27]. The central and several state governments provided fiscal incentives to farmers for planting Jatropha and other non-edible oilseeds. However, the government's ambitious plan was not realized due to a lack of enough Jatropha seeds to produce the targeted amount of biodiesel. In fact contrary to expectations, yield of feedstock per ha was found to be half of what was postulated at the laboratory stage. Thus, NBM was behest from the beginning with chronic feedstock shortage for biodiesel. Further, the cost of biodiesel production turned out to be 20 to 50% more expensive than the set minimum purchase price (MPP). As a result, there were no biodiesel sales. Nearly, 20 Indian biodiesel plants annually produce 140 to 300 million litres of biodiesel [69], which is mostly utilised by the informal sector locally for irrigation, electricity etc., and by automotive companies for experimental projects. So far, only 0.5 Mha land has been planted with Jatropha, and the GoI has not initiated the purchase of biodiesel through the designated purchase centres, even though a MPP of $0.49 per litre was announced in 2006 [41]. In January 2015, the Union Cabinet amended the NPB to make it easier for customers to purchase biodiesel directly from private manufacturers, authorized dealers, and authorized OMC joint ventures. The price of biodiesel is presently market determined.
According to a study by Dhar & Shukla [58], under a BAU scenario, demand for diesel for transport is expected to grow from 46.9 billion litres in 2010 to 155.7 billion litres in 2030. Table 2 shows the estimated demand for biodiesel and the associated land requirements for Jatropha plantation according to various blending requirements. Biodiesel demand for 20% blending targets is expected to grow 19.8 and 31.1 billion litres in 2020 and 2030, respectively. The seed yield is assumed to be 2.5 t/ha and the biodiesel recovery rate is considered to be 30% [41]. Assuming that the yield and oil content of Jatropha would remain at the same level and that no new superior feedstocks would be introduced, some 25 Mha and 40 Mha of Jatropha cultivation would be necessary to meet the 20% blending target by 2020 and 2030, respectively (Table 2).
Table 2. Biodiesel demand and associated land requirement for Jatropha plantation.
| Year |
Diesel demand (BL) |
For 5% blending |
For 10% blending |
For 20% blending |
| Biodiesel demand (BL) |
Jatropha area (Mha) |
Biodiesel demand (BL) |
Jatropha area (Mha) |
Biodiesel demand (BL) |
Jatropha area (Mha) |
| 2010 |
46.9 |
2.3 |
3.0 |
4.7 |
6.0 |
9.4 |
11.9 |
| 2015 |
72.8 |
3.6 |
4.6 |
7.3 |
9.3 |
14.6 |
18.6 |
| 2020 |
98.8 |
4.9 |
6.3 |
9.9 |
12.6 |
19.8 |
25.2 |
| 2025 |
127.3 |
6.4 |
8.1 |
12.7 |
16.2 |
25.5 |
32.4 |
| 2030 |
155.7 |
7.8 |
9.9 |
15.6 |
19.8 |
31.1 |
39.7 |
3.3. Feasibility of achieving targets using the first-generation pathway
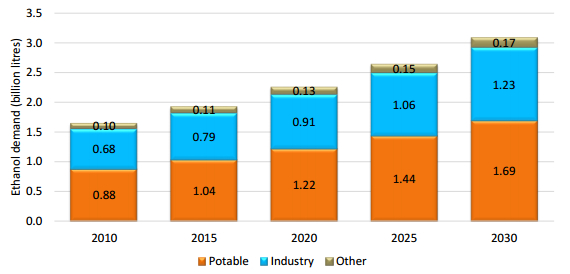
As discussed above, the current ethanol (from sugarcane) and biodiesel (from Jatropha) availability is not sufficient to meet the NPB's target of 20% blending by 2017. Figure 6 presents the surplus and deficit of ethanol according to different blending requirements, after taking into account the demand for ethanol in potable, industrial and other applications. The shares of molasses being used for potable, industrial, and other applications are 32.5%, 25%, and 3.5%, respectively [41]. The available surplus alcohol is being diverted for blending with transportation fuel. The rate of growth in demand for ethanol increased by 3% for industrial and other uses and 3.3% for potable use [66]. These growth rates are expected to continue over the next several years and are incorporated in the estimates presented in this study.
Figure 7 presents the ethanol demand for potable, industrial and other applications. To meet the 20% blending targets by 2020, an extra 5.74 billion litres of ethanol will be required from advanced or 2nd-generation processes. This does not seem realistic, as ethanol production through biomass has not yet started in India. In April 2016, the country has installed its first demonstration scale 2nd-generation biofuel production facility with annual ethanol capacity of 750,000 litre at Kashipur in Uttarakhand that processes 10 tonne of cellulosic feedstock per day using domestically developed technology. The project cost is $5.28 million. Another demonstration project with a capacity of 100 dry tonnes of biomass per day is under construction by Praj Industries in Pune, Maharashtra. Further, Shell India Markets Pvt Ltd (SIMPL) will proceed with the installation of a five tonne per day biofuel demonstration plant using IH2 technology4 in Bangalore, India.
4IH2 technology is a continuous catalytic thermo-chemical process which converts a broad range of forestry/agricultural residues and municipal wastes directly into renewable hydrocarbon transportation fuels and/or blend stocks.
As shown in Table 2 above, an estimated area of 25.2 Mha and 39.7 Mha would need to be planted with Jatropha by the year 2020 and 2030, respectively in order to meet the 20% blending targets (Table 2). The FAO/IIASA global agro-ecological zone modelling framework (GAEZ v3.0) assessed the spatial availability and suitability of culturable wastelands for Jatropha production in India [70]. Approximately, 11.1 Mha was assessed as very suitable and suitable (1.9 Mha), or as moderately suitable (8.2 Mha), with an estimated biodiesel production potential of 11.2 billion litre [41] that would be sufficient to meet only 10% of the blending targets by 2020. For the 20% blending target, approximately 20 billion litre of biodiesel will be required, which is not possible through tree-borne oilseeds (TBOs) due to land availability constraints. Though the GoI5 has deregulated the price of diesel in line with gasoline in August 2015, meeting a 5% biodiesel blending target by 2020 would require a dedicated plantation of energy crops or a probable switch to alternate sources of biodiesel from locally available TBOs, using multiple feedstock and imported biodiesel.
5GoI controlled OMCs have issued tenders to purchase up to 225 million gallons per year of biodiesel in August 2015 as an important step toward implementing a 5% biodiesel blend policy.
Although India, through its multi-pronged policy approach, has taken positive steps towards developing and promoting biofuels, the possibility of achieving the NPB's 20% blend target seems remote. Due to constraints such as the state of existing infrastructure and institutional set-up, production is currently limited to 1st-generation biofuels, namely molasses-derived ethanol and biodiesel from TBOs. Feedstock and 1st-generation biofuel production depends on well-established technologies, and the final product has been widely commercialized. However, concerns about food security and land use have raised questions about the viability of 1st-generation biofuels [71,72,73,74]. The direct benefits of biofuels are linked to indirect impacts that may adversely affect GHG emissions, ecosystems, and food and water security [75,76,77,78]. While both 1st- and 2nd-generation biofuel producers may compete with other industries for feedstock [79], currently competition is more pronounced with 1st-generation biofuels. The increasing questions about the sustainability of many 1st-generation biofuels has called attention to the potential of 2nd-generation (or "advanced") biofuels.
3.4. Second-generation pathway
Second-generation biofuels can be produced through biochemical conversion (i.e., fermentation and anaerobic digestion), thermochemical conversion (i.e., combustion, gasification, pyrolysis, liquefaction, hydrothermal upgrading), and extraction of vegetable oils. The biochemical process is based on enzymatic hydrolysis of the lignocellulosic material, using a variety of enzymes that break the cellulosic material into sugars. In the second step of the biochemical process, the sugars are fermented into alcohol, which is then distilled into ethanol. The thermochemical process uses high temperatures to transform feedstock into a synthesis gas. This gas is then transformed into different types of liquid or gaseous fuels, called "synthetic fuels" (such as BTL-diesel and bio-SNG). The future scenarios analyse two technology pathways for analysis i) cellulosic ethanol into ethanol and ii) BTL-diesel using the Fischer-Tropsch process [80], as there is information on these two technology pathways on their costs since there are demonstration projects for both these technologies [80,81].
Current global biofuel production of around 146 billion litres [15] is almost entirely 1st-generation, based mostly on sugarcane and corn, and to a lesser extent on canola, sunflower and other agricultural feedstocks. Lignocellulosic ethanol (based on bio-chemical process) and BTL-diesel (based on thermos-chemical process) are the most widely discussed 2nd-generation biofuel options as they can be used pure or blended with conventional gasoline and diesel [82]. Both the private and public sectors of the Indian biofuel industry claim to be successful in developing and customizing technology for converting lignocellulosic materials [36,83,84]. Trials are underway to process municipal solid waste, micro-algae, and photosynthetic organisms into advanced biofuels [85,86,87].
4. Technical potential of second-generation biofuels
Second-generation biofuels, produced using non-food agricultural residue and waste feedstocks, have undergone a notable scale-up in recent years. Seven new commercial-scale plants using biomass waste and agricultural residue feedstock were commissioned in 2014–2015 [15], bringing the total number of facilities worldwide to ten. In India, the concerns regarding the feedstock availability, economic viability and sustainability of molasses-based ethanol necessitated the search for alternate feedstocks. Sweet sorghum, for example, has advantages that make it a potential source of raw material for commercial ethanol production [88,89]. India ranks third in terms of area and sixth in terms of production of sorghum [90]. However, most farmers in India grow sorghum for food and fodder for their cattle. Thus, diverting sorghum for fuel may result in fodder-fuel conflict that may be unsustainable [91]. Among other cellulosic sources, agricultural residues are likely to be most readily available for 2nd-generation biofuels. In this section, we assess the biofuel potential of biomass resources in the form of residues and wastes in India.
Agriculture plays a vital role in employment generation in the Indian economy, with nearly half of the Indian population being dependent on agriculture and allied activities for livelihood. In 2015–2016, agriculture contributed 17.4% to India's GDP [92]. Area and production of different crops and their respective residue production in India is shown in section S.2 of the supplement and Table 3 [67]. The specific ratios of residue to grain production of different crops are taken from literature [62,93,94,95]. For the year 2010, the area and total crop production were 186 Mha and 656 Mt, respectively as shown in the supplement (Section S.2). The gross residue availability is estimated at 686 Mt for 2010. For the years 2020 and 2030, the area and productivity were projected based on the data from 1950–1951 to 2011–2012 as shown in the supplement (Section S.3).
Table 3. Gross residue availability by crop in India.
| Crop |
Economic produce |
Type of residue |
Residue to crop ratio |
Total residue production (air dry*)–Mt |
| 2010 |
2020 |
2030 |
| Foodgrains |
Rice |
Straw + husk |
1.80 |
172.8 |
197.9 |
221.8 |
| Wheat |
Straw |
1.60 |
139.2 |
173.1 |
193.7 |
| Jowar |
Stalk |
2.00 |
14.1 |
12.1 |
11.5 |
| Bajra |
Straw |
2.00 |
20.7 |
22.8 |
24.7 |
| Maize |
Stalk + cobs |
2.50 |
54.3 |
62.1 |
70.6 |
| Other cereals |
Stalk |
2.00 |
9.1 |
7.8 |
7.6 |
| Gram |
Waste |
1.60 |
13.2 |
13.5 |
13.8 |
| Tur (Arhar) |
Shell + waste |
2.90 |
8.3 |
8.9 |
9.6 |
| Lentil (Masur) |
Shell + waste |
2.90 |
2.7 |
3.6 |
4.1 |
| Other pulses |
Shell + waste |
2.90 |
18.0 |
18.4 |
19.8 |
| Oilseeds |
Groundnut |
Waste |
2.30 |
19.0 |
20.6 |
22.0 |
| Rapeseed & Mustard |
Waste |
2.00 |
16.4 |
19.3 |
22.1 |
| Other oilseeds |
Waste |
2.00 |
32.1 |
38.6 |
44.7 |
| Fibre |
Cotton |
Seeds + waste |
3.50 |
19.6 |
21.2 |
22.5 |
| Cotton gin trash |
0.08 |
0.4 |
0.5 |
0.5 |
| Jute and Mesta |
Waste |
1.60 |
3.1 |
3.6 |
3.9 |
| Coconut |
Fibre/husk |
0.42 |
4.9 |
7.4 |
8.4 |
| Shells |
0.12 |
1.4 |
2.1 |
2.4 |
| Sugar |
Sugarcane |
Bagasse + leaves |
0.40 |
137.0 |
162.6 |
183.7 |
| Total |
686.2 |
795.9 |
887.4 |
| *Moisture content (air day): 30% for bagasse and 10% for all other agricultural residues. |
Many of agricultural residues may have alternative uses or markets, and any decision to use them for biofuels must be made in the context of these alternatives. In our analysis, we have also considered alternative applications of crop residues in order to assess the surplus availability of agricultural residues for biofuels in the supplement (Section S.4). For the year 2010, the agricultural residues available for energy applications was estimated at 190 Mt, of which 133 Mt could be used to produce approximately 28 billion litres of ethanol annually (Table 4), assuming ethanol yields of 214 lge/ton dry matter (tDM) for cellulosic-ethanol or 217 lge/tDM for biomass-to-liquid (BTL) diesel [82]. For the estimates presented in Table 4, it is assumed that 20% agricultural residues is lost in collection, transportation and storage, etc. [95,96]. Ethanol yields per tDM will improve up to 250 litres per tDM in 2020,275 litres per tDM in 2025–2026 and to 300 litres per tDM in 2030 [97]. Other studies also showed the bio-conversion to cellulosic ethanol and BTL to be in a range that varies from 110–330 lge/tDM for biochemical enzymatic hydrolysis ethanol and 75–200 lge/tDM for Fischer-Tropsch BTL, respectively [45,98].
Table 4. Biofuel potential from net availability of agricultural residues.
| Crop residue |
Agricultural residue used for fodder, fuel and other purposes (%) [62] |
Net agricultural residue availability for biofuels6 (Mt) |
Net biofuel availability (equivalent ethanol billion litres) |
| Fodder |
Fuel |
Other |
2010 |
2020 |
2030 |
2010 |
2020 |
2030 |
| Rice straw and husk |
80.8 |
11.1 |
8.0 |
13.8 |
15.8 |
17.8 |
3.0 |
4.0 |
5.3 |
| Wheat straw |
86.4 |
0.0 |
13.6 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
| Jowar stalk |
100.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
| Bajra straw |
89.8 |
0.0 |
10.2 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
| Maize stalk and cobs |
81.0 |
19.0 |
0.0 |
7.4 |
8.5 |
9.7 |
1.6 |
2.1 |
2.9 |
| Other cereals stalk |
100.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
0.0 |
| Gram waste |
0.0 |
100.0 |
0.0 |
9.5 |
9.7 |
10.0 |
2.0 |
2.4 |
3.0 |
| Tur shell and waste |
3.5 |
48.5 |
48.0 |
2.9 |
3.1 |
3.3 |
0.6 |
0.8 |
1.0 |
| Lentil shell and waste |
3.5 |
48.5 |
48.0 |
1.0 |
1.3 |
1.4 |
0.2 |
0.3 |
0.4 |
| Other pulses shell/waste |
3.5 |
48.5 |
48.0 |
6.3 |
6.4 |
6.9 |
1.3 |
1.6 |
2.1 |
| Groundnut waste |
0.0 |
13.2 |
86.8 |
1.8 |
1.9 |
2.1 |
0.4 |
0.5 |
0.6 |
| Rape & Mustard waste |
0.0 |
100.0 |
0.0 |
11.8 |
13.9 |
15.9 |
2.5 |
3.5 |
4.8 |
| Other oilseeds waste |
0.0 |
100.0 |
0.0 |
23.1 |
27.8 |
32.2 |
4.9 |
7.0 |
9.7 |
| Cotton seeds and waste |
0.0 |
100.0 |
0.0 |
14.1 |
15.3 |
16.2 |
3.0 |
3.8 |
4.9 |
| Cotton gin trash |
0.0 |
100.0 |
0.0 |
0.3 |
0.3 |
0.4 |
0.1 |
0.1 |
0.1 |
| Jute and Mesta waste |
0.0 |
100.0 |
0.0 |
2.2 |
2.6 |
2.8 |
0.5 |
0.7 |
0.9 |
| Sugarcane bagasse/leaves |
11.8 |
41.0 |
47.2 |
35.9 |
42.6 |
48.2 |
7.7 |
10.7 |
14.5 |
| Coconut fibre/husk |
0.0 |
40.0 |
60.0 |
1.4 |
2.1 |
2.4 |
0.3 |
0.5 |
0.7 |
| Coconut shells |
0.0 |
100.0 |
0.0 |
1.0 |
1.5 |
1.7 |
0.2 |
0.4 |
0.5 |
| Total |
|
|
|
132.6 |
153.0 |
171.0 |
28.4 |
38.3 |
51.3 |
6Apart from fodder and other applications the net agricultural residue availability for biofuels also takes into account the residue used for biomass power/cogeneration projects.
As shown in Table 4, the net obtainable biofuel from agricultural residues is estimated at 38 and 51 billion litres in 2020 and 2030, respectively, which would be sufficient to meet the 20% blending target of NPB by 2030. The net ethanol/BTL production would increase by 23% (from 51 to 63 billion litres) in 2030 if an additional 10% of agricultural residues obtained from foodgrains (such as paddy straw, wheat and bajra straw, jowar stalks, etc.) could be diverted to the biofuel production route. Moreover, as per the Biomass Atlas of India [99], it is estimated that an additional 104 Mt of biomass is available in India in forest and wastelands, an amount that is not considered in this analysis.
5. Economic potential and cost of biofuels
5.1. Market price and cost of agricultural residues
The cost of supplying agricultural residue to biofuel production facilities is site specific and depends on type of crop residue, availability of the residues per square kilometre and type of transport infrastructure available. Crop residue prices in India show a wide variation for the same crop and across crops [88]. For example, molasses prices in India fluctuated from US$18–92 per tonne [41,100] whereas the price of rice straw varied from US$11 to US$13 per tonne [101].
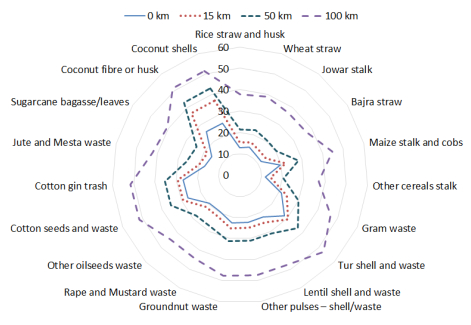
It is observed that after taking into account production, harvesting, collection, transport and storage costs, the cost of biomass feedstocks are substantial [41]. Figure 8 presents the cost of various agricultural residues at distances of 0 km, 15 km, 50 km and 100 km from farm gate using the methodology developed by Tripathi et al. [49] and updated by Purohit & Fisher [41]. The methodology considers that the total cost of agricultural resides is a sum of production cost, harvesting cost, collection cost, transportation cost and storage cost. A detailed description of how each of these costs are calculated is provided in Purohit & Fisher [41]. At a distance of 50 km from farm gate the cost of residues varies from a minimum of US$20 per tonne for bajra straw to a maximum of US$43 per tonne for coconut fibre/husk. These prices are quite close to the biomass/bagasse price estimates provided by the Central Electricity Regulatory Commission (CERC) as shown in the supplement (Section S.5). Transportation costs contribute significantly to the total price of the residues and beyond 50 km they become a dominant component of the cost.
5.2. Cost curve for biomass
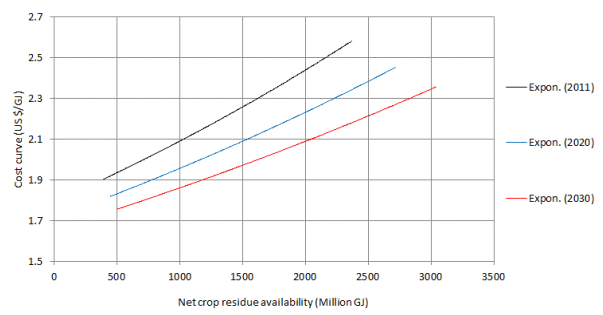
The costs of biomass as seen in previous section vary with type of biomass and transportation distances. However, bio-refineries based on the 2nd-generation pathway can use different types of biomass and this is helpful to overcome the seasonal variations in the availability of biomass. Therefore, instead of having supply curves for different types of biomass an aggregate supply curve is developed as shown in Figure 9. The different crop residues have different calorific values and therefore each crop residue is converted in terms of the energy content in GJ using calorific values shown in the supplement (Section S.6).
The supply curve reflects factory gate price for a bio-refinery and includes the production cost, harvesting cost, collection cost, storage and transportation cost. These costs for different kinds of biomass are taken from Table 24 [41]. A large part of cost is related to transportation costs [41] and this in turn is related to the size of a bio-refinery. A larger refinery can have economies of scale however at the same time can mean a larger catchment area and therefore a longer distance for transportation. The analysis has been made for a bio-refinery with a capacity of 500 million litres (150 million gallons) per year. Bio-refinery capacities range from 16 million gallons (61 million litres) per year for small-scale facilities, to 210 million gallons (795 million litres) per year for large-scale gasification facilities [102]. The 500 million litres size refinery therefore falls within range of small and large scale and is also close to an economical size for a bio-refinery [80]. For a 500 million litre sized refinery, based on 2nd-generation cellulosic ethanol production process, the agricultural residues required at refinery gate would be approximately 2.3 Mt7 to start with and decline to 1.6 Mt annually by 2030. Only top 12 states (Table S6.2 of the supplement) were able to supply biomass for such a refinery from within 100 km. Three states with a high cropping intensity, Haryana, Maharashtra and Uttar Pradesh, can meet this demand within a radius of 65 km from a bio-refinery.
7Assuming ethanol yield of 214 lge/ton dry matter (tDM) for cellulosic-ethanol and 217 lge/tDM for biomass to liquied (BTL) diesel in 2010 (IEA, 2010). Ethanol yield per tDM will improve up to 250 litres per tDM in 2020,275 litres per tDM in 2025 and to 300 litres per tDM in 2030.
5.3. Economic potential of biofuels
The approach of government policies has shifted lately from top down target setting towards getting the markets working for biofuels. For example, the policy of giving price supports for biofuel producers can reduce the price risks. Similarly, allowing biofuel producers to sell directly can deepen the biofuel markets by creating alternative supply chains and give more clout to biofuel producers in relation to OMCs. The success of these initiatives would however rest on the competiveness of biofuels with other fuels and technologies that drive vehicles. Biofuels using the 2nd-generation pathway would also need to compete for biomass leftover for energy since the same can also be used for power generation. This section covers the results from the economic assessment that was done using the ANSWER MARKAL model set up to analyse transport scenarios for India [58].
The economic assessment considers two future scenarios for biofuels. The first scenario which is labelled as the BAU scenario assumes that biofuel producers have access to markets through the OMCs and therefore make use of infrastructure for gasoline and diesel distribution, and so there are no additional distribution costs post bio-refinery gates. The scenario also assumes there would be a reduction in bio-refineries costs and the refining efficiencies would improve as lessons are learned from implementation. The scenario also assumes that biomass available for fuels is available for both biofuels and power generation and hence a competition between these two competing demands is considered.
The second scenario considers a strong climate regime that is in line with the Paris Agreement where the parties (national governments) agreed to the goal of limiting the temperature increase to well below 2 degrees Celsius (℃) and to limit the temperature increase to 1.5 ℃ [103]. The CO2 price trajectory is provided exogenously and starts from USD 13.9 per tCO2 in 2020 and then increases steadily to reach USD 200 per tCO2 in 2045 [104]. Biofuels produced using the second generation pathways have significantly lower CO2 emissions compared to gasoline and diesel [98] and therefore the social value of carbon can improve the competitiveness for biofuels.
The results are validated for future time periods so that they are consistent with the scenario narratives. For this first, a BAU scenario is set up and from this base year (2010) results are used to create an energy balance. This base year energy balance was validated against energy balance for 2010 available from IEA.
5.4. Economic potential of ethanol
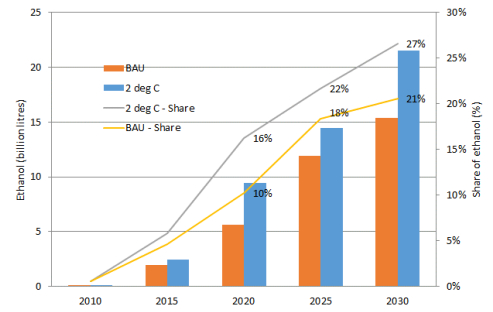
Ethanol production in India has so far been from sugarcane molasses and the surplus of this production has been used for blending in gasoline. The production from molasses route is sufficient to meet the demand for potable and industrial needs however only a small surplus is left over for blending with gasoline [97]. Therefore, ethanol produced from 2nd-generation route is essential to meet the blending target. The analysis shows that it would be possible to achieve the 20% blending target by 2030 whereas achieving the 20% blending target by 2020 would be difficult. A strong climate policy can however create stronger incentives for biofuels and even by 2020 a 16% share of ethanol in gasoline can be achieved (Figure 10). In both the scenarios, the share of ethanol exceeds 20% by 2030 since the vehicle technologies include vehicle that can run on 100% ethanol. The demand for ethanol increases rapidly in both the scenarios and by 2030 the demand in the 2 ℃ scenario is around 22 billion litres, which is more than the current demand for gasoline.
5.5. Economic potential for biodiesel
The Indian biodiesel program has so far relied on Jatropha however so far the achievement is negligible. An assessment of blending targets and their implications for Jatropha has been done in Section 3. The economic analysis using the model includes the 2nd-generation pathway (refer section 3.4 for different conversion technologies) for biodiesel production. The biofuel production is using crop wastes for which biodiesel competes with ethanol and power generation. Biodiesel emerges as the second priority relative to ethanol, and biodiesel production would be lower than ethanol in all the horizon years till 2030 and even by 2030 the blending would be only 3% of diesel demand (Figure 11). Biodiesel production however gets a boost in the 2 ℃ scenario and biodiesel demand would cross 10 billion litres by 2030 and the blending would be 6% of diesel demand (Figure 11).
6. Scenario analysis and socio-economic impact of lignocellulosic biofuel industry
6.1. Alternative biofuel scenarios
In Section 3 the blending targets proposed by the government were analysed and it was clear that to achieve these targets it will be necessary to go beyond 1st-generation solutions. The blending targets constitute the NPB scenario and this scenario ignores economic feasibility of the targets. The NPB scenario assumes that GoI will meet its 20% blending targets by 2020. The economic feasibility is however the basis of the BAU and 2 ℃ Scenario and the same were analysed in Section 5. Additionally, the economic potential is the basis of blending shares for the BAU scenario and 2 ℃ scenarios. Under the BAU scenario therefore, India will have a 10%, 18%, and 21% gasoline blending by 2020, 2025 and 2030, respectively whereas under the 2 ℃ scenario India will have a 16%, 22%, and 27% gasoline blending by 2020, 2025 and 2030, respectively (Figure 10). Similarly, since the economic potential for 2nd-generation biodiesel is low (Figure 11), share for biodiesel blending in BAU would be 1%, 2% and 3% by 2020, 2025 and 2030, respectively whereas under the 2 ℃ scenario biodiesel blending would be 1%, 4%, and 6% by 2020, 2025 and 2030, respectively. Figure 12 summarizes the ethanol and biodiesel demand up to 2030 in BAU, NPB and 2 ℃ scenarios after taking into account the biofuel availability from first generation route.
6.2. Investment and revenue through second generation biofuel industry
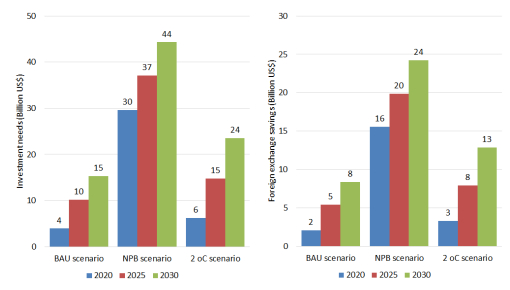
Ethanol and biodiesel production from lignocellulosic biomass will require sizable investments in 2nd-generation biofuels. Investment in biofuels in developed economies, at $2.1 billion, amounted to just over double that in developing nations in 2015 [105] with United States and Europe accounting for most of the funding for second-generation ethanol. These investments in 2nd-generation biofuel technologies will drive down the costs from an average cost of US$1.25 per litre of installed capacity to construct a separate 2nd-generation ethanol/BTL manufacturing facility [106] and therefore a decline in capital costs of 7% between 2010 and 2020 and 4% between 2020 and 2030 has been considered. Under the BAU scenario, a cumulative investment of US$4 billion and US$15 billion is needed by 2020 and 2030 respectively, whereas under the NPB and 2 ℃ scenarios a cumulative investment of US$44 billion and US$24 billion respectively required by 2030 (Figure 13). These investments would result in annual foreign exchange savings of US$8 billion, US$24 billion and US$13 billion in BAU, NPB and 2 ℃ respectively by 2030 (Figure 13), assuming that biofuels produced would substitute imported oil.
6.3. Biomass feedstock demand and biomass prices
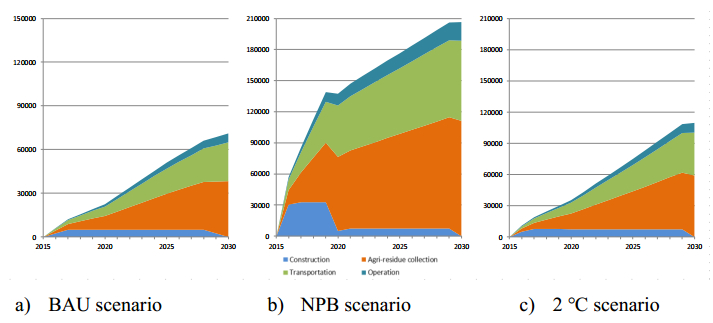
Biomass feedstock demand for biofuel production would increase in all the three scenarios (Figure 14) and the increase would be quite high relative to the demand in 2010. The increase in demand would however be higher in the NPB and 2 ℃ scenario. The demand for biomass in NPB scenario is much higher than what can be supplied within a distance of 100 km from farm gate and therefore is not economically feasible using crop residues from within India alone. It would necessitate import of biofuels or use of forestry residues (if available). In the MARKAL model since the aggregate supply curve was based on crop residue availability in top 12 states and demand in NPB scenario exceeds this therefore only BAU and the 2 ℃ scenario were analysed.
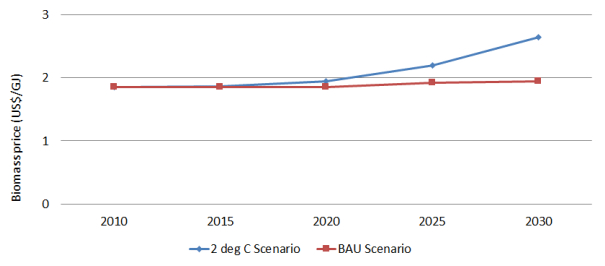
The demand for crop residues rises much faster in the 2 ℃ scenario and therefore it pushes up the prices for biomass (Figure 15). The increased demand for crop residues and increasing price at the same time would increase the revenues for the farmers. The value of crop residues sold would increase from around 230 million US$ in 2010 to around 3.3 and 10.2 billion US$ in 2030 under the BAU and 2 ℃ scenario, respectively.
6.4. Enabling jobs through second generation biofuel industry
Figure 16 presents the annual employment created by the 2nd-generation biofuel industry in BAU, NPB and 2 ℃ scenarios. Key assumptions and input parameters used to assess the skilled and unskilled jobs through 2nd-generation biofuel industry are presented in the supplement (Section S.7). In the construction of bio-refineries approximately 64,000,100,000 and 200,000 jobs will be needed between 2017 and 2030 in the BAU, 2 ℃ and NPB scenario respectively. The NPB scenario requires most of the jobs between 2015 and 2020 and this could be a challenge. The number of permanent jobs created at farms for agricultural residue collection (bailing and hauling) would witness a rapid increase across all the scenarios and by 2030 over 38,000,111,000 and 60,000 low-skilled labourers annually would be required under BAU, NPB and 2 ℃ scenarios respectively. Transportation would also provide a sizable employment and by 2030 almost 27,000 people could be employed in BAU and much more in the NPB and 2 ℃ scenarios. The operation of bio-refineries would by 2030, need approximately 6,000, 18,000 and 9,000 skilled jobs annually in the BAU, NPB and 2 ℃ scenarios respectively. The total number of jobs estimated in the above-mentioned methodologies is based on the studies on European market's where there is considerable mechanization of work. The potential number of jobs created in India could be more, since many jobs will be performed manually.
6.5. Environmental benefits through second generation biofuel industry
Second-generation ethanol has about 80% to 90% lower CO2 emissions as compared to gasoline and diesel if we discount for emissions due to indirect land use change (Directive 2009/28/EC)8. CO2 reductions from biofuels are therefore estimated considering an 80% reduction on a conservative side. The reduction that will happen due to substitution of gasoline by biofuels show a similar trend across scenarios (Figure 17) however the highest reduction happen in 2 ℃ scenario where annual reduction in 2030 is around 40 Mt CO2. The reduction due to substitution of diesel are very high in the NPB scenario however since NPB targets for diesel are not economically feasible the CO2 reductions might happen at a high cost. The overall reductions in the 2 ℃ in 2030 due to biofuels are around 60 Mt CO2 which is much higher than around 40 Mt CO2 in the BAU. Therefore, a social value of carbon for India in a low carbon scenario can create a strong incentive for biofuels and help in decarbonization of transportation sector. Besides reduction in CO2 emissions from fossil fuel substitution fostering a 2nd-generation biofuels industry in India can help in productive use of crop residues. Farmers have traditionally burned excess residues as a means of quick disposal. The burning of agricultural residues emitted 141.2 Mt of CO2, 8.57 Mt of CO, 0.04 Mt of SOx, 0.23 Mt of NOx, 1.21 Mt of particulate matter for the year 2008–2009 [107]. Using these residues in useful activities like ethanol/BTL conversion could potentially reduce both air pollution and GHG emissions.
8As per the Annex-V of the EU's Renewable Energy Directive on - Rules for calculating the greenhouse gas impact of biofuels, bioliquids and their fossil fuel comparators (Available at: http://eur-lex.europa.eu/legal-content/EN/TXT/PDF/?uri=CELEX:32009L0028&from=DE).
7. Conclusions
In this study, we provide an overview of the demand and supply of biofuels in India, identify key challenges in achieving the country's biofuel targets, and analyse their role in India's long-term transport scenarios. The results indicate that the current ethanol (from sugarcane) and biodiesel (from Jatropha) availability through the 1st-generation biofuel route is not sufficient to meet the target of 20% blending by 2017 set in the national policy on biofuels. It has become apparent that ethanol production based solely on sugarcane molasses is neither economically viable, nor sufficient and sustainable in the long run. However, there is sufficient technical potential from lignocellulosic biofuels in India using agricultural residues to meet the NPB's 20% blending targets by 2030. The estimates presented in the paper indicate that agricultural residues can produce 38 and 51 billion litres of lignocellulosic ethanol/BTL in 2020 and 2030, respectively. The economic potentials measured in terms of blending shares for lignocellulosic biofuels would however increase slowly with time under a business-as-usual scenario. In case of lignocellulosic ethanol, blending shares would increase from 10% in 2020 to 21% in 2030 whereas for biodiesel the shares would increase from 1% in 2020 to 3% in 2030. The achievement is therefore much lower than NPB's targets and the technical potentials. A strong climate policy (2 ℃ scenario) however improves the economic potential for biofuels. In case of lignocellulosic ethanol, these would increase to 27% in 2030 whereas for biodiesel these would increase to 6% in 2030.
Biofuels produced using 2nd-generation pathway offer many co-benefits. In place of the funds spent on importing oil, 2nd-generation biofuels could save US$16–24 billion in foreign exchange if India develops a 2nd-generation biofuel industry and maximizes the potential supply of 2nd-generation biofuels. It would result in job creation especially in rural areas. In the BAU scenario over 64,000 construction jobs would be generated between 2017 and 2030. In addition, biofuel industry can create approximately 6,000 operational jobs annually, over 38,000 low-skilled jobs annually (for baling and hauling of agricultural residues) whereas more than 26,000 persons could potentially be employed in the transportation sub-sector by 2030 on annual basis.
Nevertheless, large-scale penetration of 2nd-generation biofuels in India would need investments, a stable policy framework, extensive research and development (R & D) efforts, availability of biomass, and adjustments within the distribution supply chains. The cumulative investment needed for example from 2017 to 2030 would be US$15 billion by 2030 under the BAU scenario. This kind of investment would happen only when there is a stable policy environment for biofuel sector since investment in supply chain for biomass and bio-refineries are highly capital-intensive and involve large risks. A first step for India's policy-makers therefore should be to introduce blending shares for biofuels that are reasonable to achieve and then ensure that they implemented strictly. The BAU scenario provides a good starting point for shares that could be enforced in the short term. The biofuel policies also need to provide financial or fiscal incentives for infrastructure needed for the collection of biomass feedstock and for this fund could be used from existing programs e.g., the Mahatma Gandhi National Rural Employment Guarantee Scheme that provides at least 100 days of wage employment in a year. A large part of the investment in this sector would happen from the private sector. Therefore, private investors should be encouraged to invest in biofuel programs, and government policies should support their participation. In fact, the private sector should work in collaboration with public sector. The public sector could for example take a lead in R & D. A substantial research in 2nd-generation feedstocks is needed on proving the industrial reliability and technical performance and operability of the conversion pathways and some of the research can be in partnership with the private sector.
In spite of having high technical potential for supplying several different types of biomass feedstock, India has been unable in the past to meet the growing domestic bioenergy demand [108]. Preliminary estimates based on our analysis indicate that India has the surplus agricultural residue available to produce approximately 51 billion litres of biofuel from 2nd-generation route by 2030 that will be sufficient to meet the nation-wide blending targets. At present, there is no policy mechanism incentivising farmers to collect and deliver biomass residues to a next-generation ethanol plant. Therefore, it is important to establish a reliable supply chain for biomass covering collection, transportation and handling of biomass feedstock. If the policy makers seek to foster an industry longer term, they could create a mechanism whereby cooperatives or farming communities could be involved in the process of collection, storage and delivery of residues. To reduce supply chain uncertainties and high market risk associated with 2nd-generation biofuel industry a supply chain should be designed and operated to maximize economical potential and social benefit, and minimize environmental impact. In doing this India can use its demographic advantage and if there are any skill mismatches they should be addressed through training and integrated within larger national programs e.g., Skill India9.
9Skill India is a campaign launched by GoI on 15 July 2015 with an aim to train over 400 million people in India in different skills by 2022. It includes various initiatives of the Indian government like National Skill Development Mission, National Policy for Skill Development and Entrepreneurship 2015, Pradhan Mantri Kaushal Vikas Yojana (PMKVY) and the Skill Loan scheme [109].
Biofuel policies have mainly envisaged blending of biofuels in fossil fuels i.e., gasoline and diesel. Due to this the biofuels face two issues-first is integration of ethanol or biodiesel within existing petroleum infrastructure and second is limitation of vehicles to take biofuels beyond a certain percentage (10–15% in case of ethanol blending in gasoline). As of now the transportation of biofuels requires separate infrastructure. These separate infrastructure for biofuels are however either limited in capacity (e.g., rail) or unavailable (e.g., dedicated pipelines). The integration of biofuels would require a scale of biofuels and retail outlets need to be incentivized to sell blended fuels to accelerate the adoption of biofuel as a transport fuel. Biofuels can grow faster and reach an economical scale if the vehicle limitations in using biofuels can be addressed. As has been successfully demonstrated in Brazil and Sweden the introduction of flex-fuel vehicles (FFV) and high-level ethanol blends are good ways of avoiding ethanol infrastructure incompatibility issues. Policy measures may be required, such as obligations for retailers to provide high-level biofuel blends (e.g., E85) or tax incentives for FFVs.
Acknowledgments
The research for this paper was supported from UNEP Project "Promoting Low Carbon Transport in India" which was funded by the German Federal Ministry for the Environment, Nature Conservation and Nuclear Safety (BMU) under the International Climate Initiative project no. 10_I_129_IND_M_Low Carbon Transport. We thank three anonymous reviewers for their valuable comments and suggestions in shaping up this paper.
Conflict of interest
The authors declare no conflict of interest in this paper.









 DownLoad:
DownLoad: