We prove global existence of weak solutions to regularized versions of balance equations representing the dynamics over a torus of complex fluids, with microstructure described by a vector field taking values in the unit ball. Regularization is offered by the presence of second-neighbor microstructural interactions and our choice of filtering the balance of macroscopic momentum by inverse Helmholtz operator with unit length scale.
1.
Introduction
With a desire to generalize a single-layer auto-associative Hebbian correlator to a two-layer pattern-matched hetero-associative circuits, Kosko designed the celebrated bi-directional associative memory neural networks (BAMNs); see [1,2,3,4,5,6]. In last several decades, BAMNs have been applied successfully in classification, associative memory, parallel computation, combinatorial optimization, signal processing, pattern recognization, image processing, etc.; see [5,6,7]. Successful application of BAMNs in such wide areas relies essentially on their stability or synchronizability. And therefore, extensive attensions have been paid to the study of stability, synchronizability and other dynamics of various BAMNs; see [6,7,8,9,10,11,12,13,14] and the vast references therein. In this paper, we shall investigate further the synchronization problem associated to BAMNs.
Since it would cost time to communicate information between neurons, time delays are inevitable in neural network models originated from real world applications. As pointed in [14,15], delays could change the stability of dynamical systems, render dynamical systems to produce periodic oscillations or chaotic phenomenon, and so on. This makes it more challenging and interesting to study stabilization/synchronization problem for BAMNs with delays. Cao and Wan [11] exploited the so-called matrix measure technique to obtain a synchronization criterion for an inertial BAMN with time delays. Inspired partially by results in [11], Li and Li [12] obtained some new results concerning the synchronization problem for a time-delayed BAMN which is not inertial. Sader, Abdurahman and Jiang [13] designed a nonlinear feedback control for a special class of BAMNs, and proved that these controlled BAMNs are synchronizable at a general decay rate. For more inspiring results concerning stability of time-delayed BAMNs, the interested readers could consult [8,16,17,18,19,20] as well as the references therein.
In the real world, uncertainty is unavoidable in the transmission of information through neural nodes. By reading [21] and the related references therein, we can conclude that fuzzy logic could play important roles in dealing with uncertainty. Zhang and Wu [21] investigated the finite time synchronization problem for a class of Takagi-Sugeno fuzzy complex networks. Except for Takagi-Sugeno logic, there is another fuzzy logic which is widely used in constructing neural network models, namely, the fuzzy "AND" (∧) and "OR" (∨) operation reasoning. Under certain conditions, experts proved that fuzzy neural networks could approximate a large collection of nonlinear functions to any desired degree of accuracy; see [22]. In the last two decades, fuzzy BAMNs have also been well studied for their synchronizability, and a large number of papers on synchronization problem for fuzzy BAMNs have been published in recent years. Among the vast references in this respect, we would like to share [23], in which a class of BAMNs including fuzzy logic was investigated and interesting synchronization results on the concerning BAMNs were obtained via using the LMI (linear matrix inequalities) approach.
As indicated in [15,24], the synaptic transmission in nervous systems can be considered as a noisy process brought on by random fluctuations from the release of neurotransmitters or other probabilistic factors. In other words, here is, aside from fuzzy uncertainty, some other uncertainty occurring in the transmission of information through neural nodes that can be modeled by special stochastic process, such as (time homogeneous/inhomogeneous) Markovian chain, Wiener process (Brownian motion), Lévy process, and so forth. Compared with other frequently used stochastic process, Markovian chain has, in a certain sense, the simplest structure. And therefore, many interesting synchronization criterion have been presented for Markovian switched neural networks (including BAMNs) in recent years; see [21,25,26,27] and the references therein.
Thanks to the wide applicability, it is a hot topic to design control to synchronize neural networks in finite time in recent years; see [20,21,25,27,28,29,30,31] and the references therein. Jia et al. [29] designed adaptive sliding mode control for a class of uncertain fractional-order delayed memristive neural networks, and proved that the obtained controlled networks are synchronizable in finite time. Cheng et al. [30] proved that delayed memristive neural networks can be finite-time synchronized via adaptive aperiodically intermittent adjustment strategy. By reviewing the afore-mentioned references, we are inspired by the results to be interested in designing control to synchronize, in finite time, fuzzy BAMNs with Markovian jumping and several types of time delays. To improve the applicability of our theoretical results and inspired by [25,32], we seek to design appropriate intermittent quantized control for our concerned network. As indicated in [25], it would certainly cut down the control cost and communication resources by using intermittent quantized control to synchronize neural networks in finite time. The idea to realize our goal in this paper is enlightened by the the afore-mentioned references, besides, [33,34,35,36,37,38] and the references therein help us a lot to find the appropriate way to prove rigrously the suggested control is indeed effective in synchronizing our concerned network in finite time. Zhai et al. [33] shared intermittent control which can synchronize a class of stochastic complex networks with delays. Zhou et al. [34] and Liu et al. [35] provided two types of self-triggered intermittent control to synchronize complex network and hybrid delayed multi-links systems, respectively. In [36,37], the author group developed quantized control to synchronize a variety of inertial neural networks. Our contributions in this paper are summarized as follows:
(i) Intermittent quantized control is first designed successfully and proved to synchronize effectively in finite time fuzzy BAMNs with Markovian jumping, discrete-time delay in leakage terms, continuous-time and infinitely distributed delays in transmission terms. In comparison with [11,12,13,17,18,20,28], our concerned network includes simultaneously fuzzy uncertainty, random uncertainty and a variety of time delays of different nature and thus has wider potential applicability. The idea of applying intermittent quantized control would contribute towards cutting down control cost and communication resources in the real world.
(ii) Several novel criteria are established to guarantee the finite-time synchronizability of our concerned fuzzy network, and the convergence settling time is computed explicitly. Additionally, an illustrative example is solved numerically to justify the effectiveness of the suggested synchronization control and the correctness of the criteria established to guarantee the finite-time synchronizability. The main tool used in proving our main results is a Lyapunov-Krasovskii functional, which differs dramatically from the ones utilized in [25,32]. As in [25], the 1st moment of trajectories of the error network system associated to our concerned network is chosen in proving the correctness of the criteria established to guarantee the finite-time synchronizability. As alluded in [25], this could reduce in a certain degree the conservatism of our finite-time synchronization criteria.
Notational conventions: Throughout this paper, R, R+ and R− denotes the totality of real numbers, the interval [0,+∞) and the interval (−∞,0], respectively; D+f denotes the right upper Dini derivative of the given function f with respect to the independent variable t; (R,L,dt) denotes the usual Lebesgue measure space; (Ω,F,F,P) (or (Ω,F,F,dP)) denotes a complete filtered probability space, in which the filtration F={Ft;t∈R+} is assumed to satisfy the usual condition: F0 contains all P-null sets in F; and F is right-continuous in the sense that ∩s>tFs=Ft, t∈R+; "P almost surely" is abbreviated as P-a.s.; EX denotes the mathematical expectation of X, where X is an arbitrarily given random variable on Ω; (Ω×R,L⊗F,dP×dt) denotes the product measure space of (R,L,dt) and (Ω,F,dP); For every pair A, B∈F, P(B|A) designates the conditional probability of the event B given the event A; {γt}t∈R+ denotes an F-adapted time homogeneous Markovian chain whose state space Ξ is finite and whose infinitesimal generator is denoted by Π=(πξ˜ξ), that is, for every pair ξ, ˜ξ in Ξ, it holds that
where δξ˜ξ is the celebrated Kronecker delta symbol, more precisely, δξ˜ξ=1 if ξ coincides with ˜ξ, and δξ˜ξ=0, otherwise. By definition, Π=(πξ˜ξ) is required to satisfy πξ˜ξ⩾0 whenever ξ≠˜ξ, and
2.
Formulation of the problem and the main results
We are concerned with in this paper the following class of fuzzy BAMNs with several types of time delays in both leakage terms and transmission terms:
where the stochastic processes {Xi(t)} and {Yj(t)}, required to be F-adapted, are given by
In (2.1) and (2.2), l1i>0, l2j>0, τ1i>0 and τ2j>0 are constants; xi(t) and yj(t), required to be F-adapted and P almost surely continuous in t, are called state trajectories of BAMNs (2.1); the connection coefficients (or connection weights) aγt11ij, aγt12ij, aγt13ij, aγt14ij, aγt21ji, aγt22ji, aγt23ji and aγt24ji are real constants; the activation functions f11j(u), f12j(u), f13j(u), f14j(u), f21i(u), f22i(u), f23i(u) and f24i(u) are Lipschitz continuous real-valued functions on R; the delay kernels K11j(t), K12j(t), K21i(t) and K22i(t) are nonnegative-valued functions which are locally Lebesgue integrable in R+; Xi(t) and Yj(t) could be viewed as disturbances; i=1,…,m, j=1,…,n.
The response system controlled by Ui(t) and Vj(t) reads:
Proposition 2.1. Let xi0,yj0:Ω×(−∞,0]→R be F⊗L measurable, and suppose that xi0(t) and yj0(t) are F0 measurable for all t∈(−∞,0] and assume
i=1,…,m, j=1,…,n. Then, (2.1) admits a unique state trajectory
satisfying the initial condition
Remark 2.1. By Proposition 2.1, for the given initial data ˜xi0(t) and ˜yj0(t) (i=1,…,m, j=1,…,n), the response network system (2.3) subsequent by
admits a unique state trajectory
where the initial data ˜xi0(t) and ˜yj0(t) satisfy the same conditions as that obeyed by xi0(t) and yj0(t) in Proposition 2.1, i=1,…,m, j=1,…,n.
Definition 2.1. The drive network system (2.1) and the response network system (2.3) are said to be synchronized in finite time provided that there exists a T>0 such that
Now we are in a position to introduce the intermittent quantized controls that would be used to synchronize the drive-response network system (2.1)–(2.3). Let {tk}∞k=0 be a strictly increasing sequence in R+ such that t0=0 and that
The controls Ui(t) and Vj(t) are required to satisfy: For every k∈N0,
in which q is the so-called logarithmic quantizer, that is, an odd function mapping R into Λ obeying the rule q(v)=ℵk=ℵ0ρk if v∈(ℵk1+θ,ℵk1−θ] for a certain k∈Z, where θ=1−ρ1+ρ, ℵ0 is a sufficiently large positive number to be specified later, and
Υ>0, ˆkγt1i>0, ˆkγt2j>0, ˇk1i(t)∈[−θ,θ), ˇk2j(t)∈[−θ,θ);
i=1,…,m, j=1,…,n.
To summarize, for every k∈N0,
We are now ready to record some results which is necessary in the proof of our main results.
Definition 2.2. Let N be a positive integer. Given V(x):RN→R. V(x) is said to be a C-regular function provided that (i) V(x) is regular in RN; (ii) V(x) is positive definite in RN: V(0)=0, V(x)>0 for all x∈RN∖{0}; and (iii) V(x) is coercive in the sense that
Lemma 2.1. (See [25]) Let V(x):RN→R be a C-regular function, and x(t):I→RN be absolutely continuous where I is an interval (bounded or unbounded). Then V(x(t)) is absolutely continuous in I and it holds that: For every selection η(t) in ∂V(x(t)), the Clarke generalized gradient of V(x(t)) at x(t), it holds that
Remark 2.2. It is ready to verify that the well-known absolute value function V(x)=|x|, x∈R, is C-regular, and to check that in this situation
In the sequel, we denote C(x)=∂V(x), x∈R, in which V(x)=|x|.
Lemma 2.2. (See [25]) Let Ψ:R+→R+ be a continuous function. Suppose that Ψ(0)>0 and that either
holds if t∈[t2k,t2k+1) for some k∈N0, or
holds if t∈[t2k+1,t2k+2) for some k∈N0, where α, β and η are all given positive constants. If χk>1 holds for all k∈N0, then
and
where
Assumption 2.1. 0⩽L11j, L12j, L13j, L14j, L21i, L22i, L23i, L24i<+∞ with
i=1,…,m, j=1,…,n.
Assumption 2.2. σ1j(t) and σ2i(t) are absolutely continuous, and 0⩽σ1j(t), σ2i(t)<t, 0⩽ˉσ1j, ˉσ2i<+∞, 0⩽ˆσ1j, ˆσ2i<1 with
i=1,…,m, j=1,…,n.
Assumption 2.3. There exists a β∗>0 such that for every β∈(−∞,β∗), it holds that 0⩽ˉˇKβ11j, ˉˇKβ12j, ˉˇKβ21j, ˉˇKβ22j<+∞ where
with
t∈R+, i=1,…,m, j=1,…,n.
Theorem 2.1. Suppose that Assumptions 2.1–2.3 hold true. If there exists a β∈(0,β∗) (see 2.3), a ρ∈(0,1), a Υ and some pξ1i along with pξ2j, such that
then the drive-response network system (2.1)–(2.3) is synchronizable in finite time. More precisely, for every state trajectory (x1(t),…,xm(t);y1(t),…,yn(t)) of the drive network system (2.1) and every state trajectory (˜x1(t),…,˜xm(t);˜y1(t),…,˜yn(t)) of the response network system (2.3), the assertion (2.6) in Definition 2.1 holds with
i=1,…,m, j=1,…,n, ξ∈Ξ.
3.
Proof of the main results
Lemma 3.1. Let N be a given positive integer and let (μ1,μ2,…,μN)⊤∈RN. Then for every pair (x1,x2,…,xN)⊤ and (y1,y2,…,yN)⊤ of vectors in RN, it holds that
Proof of Theorem 2.1. It is readily to see that the synchronizability of the drive-response network system (2.1)–(2.3) is equivalent to the stability of the error network system
in which
i=1,…,m, j=1,…,n.
Let us write
t∈R+, P-a.s., ξ∈Ξ. As in [25,32], we introduce the weak infinitesimal operator L for every stochastic process X(t) (having actually certain regularity in time variable t) defined in an interval I:
In light of (3.8), we have
Thanks to Lemma 2.1, Remark 2.2, and the definition (3.10) of Vξ1(t), we have
in which ηui(t) is an arbitrarily given selection in C(ui(t)), and ηvj(t) is an arbitrarily given selection in C(vj(t)), i=1,…,m, j=1,…,n; see Remark 2.2 for the precise definition of the multi-valued function C(⋅); and Πξ11(t), Πξ12(t), Πξ21(t) as well as Πξ22(t) is
By the definition of the logarithmic quantizer q, we have
By the definition of C(⋅) (see Remark 2.2 for the details), we have
and
This, together (3.19) and (3.20) implies
whenever ui(t)vj(t)≠0, i=1,…,m, j=1,…,n.
Thanks to the definition of C(⋅) (see Remark 2.2), we have by using direct computation
Mimick the steps in (3.23), to obtain
Thanks to the definition of C(⋅) (see Remark 2.2), (3.4), and Assumption 2.1 (especially (2.14)), we have by
Owing to (3.6) and Assumption 2.1 (especially (2.15)), take similar steps as in (3.25), to obtain
Utilize the definition of C(⋅) (see Remark 2.2), Assumption 2.1 (especially (2.14)), Assumption 2.2 (especially (2.16) and (2.18)), and some routine but tedious calculations, to arrive at
In view of Assumption 2.1 (especially (2.15)) and Assumption 2.2 (especially (2.17) and (2.19)), we have immediately by mimicking steps in (3.27)
By Lemma 3.1, we have directly
where
which, together with Assumption 2.1 (especially (2.14)), implies
This, together with Assumption 2.3 and some tedious computations, implies
This, together with (3.29), implies
t∈R+, P-a.s., ξ∈Ξ. By analogy with (3.30), we can prove also
t∈R+, P-a.s., ξ∈Ξ. By using Lemma 3.1, combining Assumption 2.1 (especially (2.15)) along with Assumption 2.3, and mimicking the steps in proving (3.30) and (3.31), we can prove
t∈R+, P-a.s., ξ∈Ξ. Taking similar steps in proving (3.32), we can prove
t∈R+, P-a.s., ξ∈Ξ. Plug (3.23)–(3.28) and (3.30)–(3.33) into (3.14), to yield
or equivalently, to yield
where α, Mξ1i, Mξ2j, Vξ2(t), Vξ3(t) and Vξ4(t) are given by (2.23), (2.24), (2.25), (3.11), (3.12) and (3.13), respectively; i=1,…,m, j=1,…,n, ξ∈Ξ. Thanks to (2.20) and (2.21), in view of (3.9), we deduce from (3.34) that
This, together with (3.8), implies immediately
Taking similar steps as in deriving (3.34), we could get
which, together with some tedious calculations, implies
where η, ˜Mξ1i and ˜Mξ2j are given as in (2.29), (2.30) and (2.31), respectively;
i=1,…,m, j=1,…,n, ξ∈Ξ. This, together with (3.8), implies
In light of (3.35), (3.36) and (2.22), we deduce by applying Lemma 2.2 that
and
where T is given by (2.26) alongside with (2.27) and (2.28). But in light of (3.2), (3.3) and (3.8)–(3.13), we have
This, together with minξ∈Ξ, 1⩽i⩽mpξ1i>0 and minξ∈Ξ, 1⩽j⩽npξ2j>0 which follow from the related assumption, implies immediately that the proof is complete.
4.
An illustrative example
In this section, we shall conduct numerical simulations to show the validity of the synchronization criteria (see Theorem 2.1) of this paper. We consider here the following BAMN:
in which
We assume here that the Markovian chain γt takes Ξ={1,2,3} as its state space, and takes the following Metzler matrix as its infinitesimal generator:
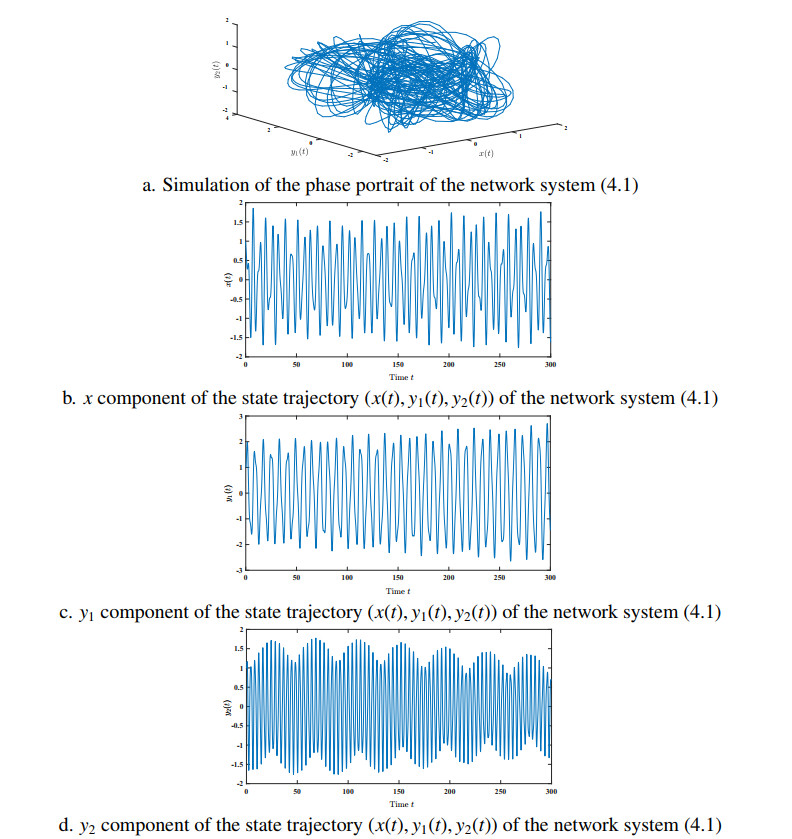
By utilizaing MATLAB, we can simulate numerically the trajectory, denoted by (x(t),y1(t),y2(t)) henceforth, of network system (4.1) supplemented by
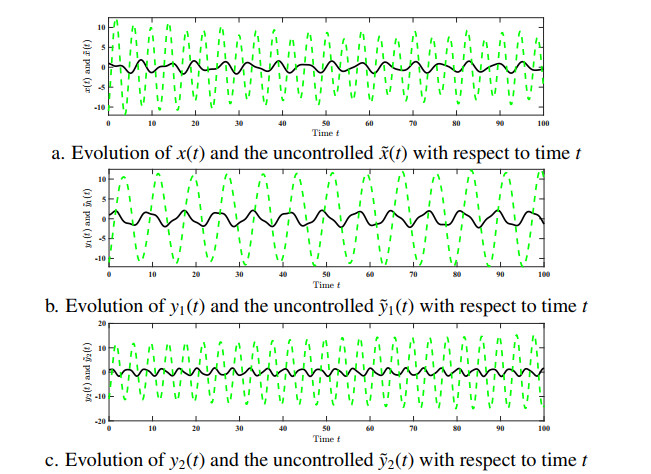
As the simulation result (see Figure 1) indicates, the network system (4.1) itself lacks stable equilibrium points, periodic trajectories and general trajectories (see especially y1(t) and the phase portrait).
This means that: For two different trajectories (a(t),b1(t),b2(t)) and (˜a(t),˜b1(t),˜b2(t)), there exists no 0<T⩽+∞ such that
Actually, we conduct numerically, by using MATLAB, comparison between the trajectory (x(t),y1(t),y2(t)) and the trajectory (˜x(t),˜y1(t),˜y2(t)) of the network system (4.1) supplemented by
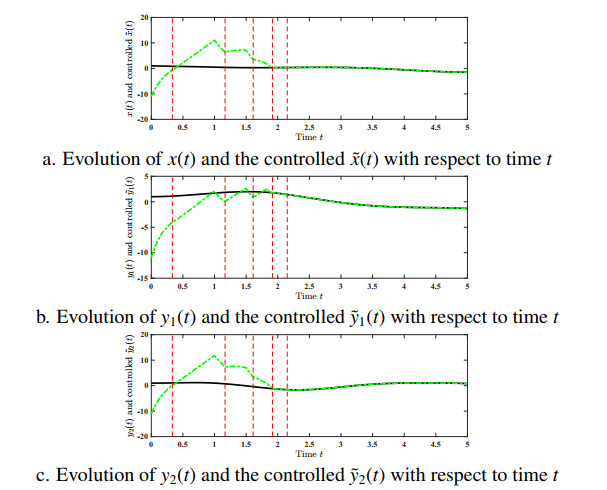
The simulation result (see Figure 2) reveals that: The trajectory (˜x(t),˜y1(t),˜y2(t)) does not approach the trajectory (x(t),y1(t),y2(t)) as time t tends to a finite/infinite time instant.
Illuminated by the results in Theorem 2.1, to design control to synchronize the network system (4.1), we introduce an infinite sequence {tn}∞n=0 in R+ by
where [x] denotes, here and hereafter, the greatest integer which does not exceed x, x∈R. After careful analysis, we can conclude that: The sequence {tn}∞n=0 is strictly increasing, and satisfies the following properties:
Enlightened by Theorem 2.1, we choose the control (2.12) and (2.13) as the candidate synchronization control for the network system (4.1). Numerical simulation based on MATLAB yields: The the control (2.12) and (2.13), with ˆkξ11=ˆkξ21=ˆkξ22=17.3914 (ξ=1,2,3) and Υ=25.9463, can render the trajectory (˜x(t),˜y1(t),˜y2(t)) to "arrive at" the trajectory (x(t),y1(t),y2(t)) before the time instant T=1.9167, and to coincide with (x(t),y1(t),y2(t)) thereupon; see Figure 3.
5.
Conclusions
We addressed the synchronization problem for a class of fuzzy BAMNs with Markovian switching in this paper. In comparision with the studies in the existing references, the concerned BAMNs in our paper include simultaneously discrete-time delay in leakage (in other words, forgetting) terms, continuous-time and infinitely distributed delays, fuzzy logic, as well as Markovian jumping in transmission terms (see (2.1) for the detailed information). This certainly provides more realistic models in applications, but brings us more difficulties in designing control to synchronize the concerned network system (2.1) in finite time. For the network system (2.1), we designed an intermittent quantized control. By coming up with a clever Lyapunov-Krasovskii functional, we proved under certain conditions that the controlled network system is stochastically synchronizable in finite time, more precisely, the 1st moments of trajectories of the error network system (3.1) of the drive network system (2.1) and the response network system (2.3) approach zero at finite time and remain to be zero thereupon. The main ingredient in proving our main results is a novel Lyapunov-Krasovskii functional, which can be adapted to deal with finite-time synchronization problem for BAMNs with time-varying leakage coefficients and transmission coefficients which generalize slightly our concerned network system (2.1).
Acknowledgments
C. Wang was supported partially by of Startup Foundation for Newly Recruited Employees, Xichu Talents Foundation of Suqian University (#2022XRC033) and NSFC (#11701050). X. Zhao was supported partially by Natural Science Foundation of Zhejiang Province (#LY18A010024, #LQ16A010003) and NSFC (#11505154, #11605156).
Conflict of interest
The authors declare that they have no conflicts of interest.









 DownLoad:
DownLoad: