1.
Introduction
For the last two decades, the utilization of electronic devices has been an inseparable aspect of everyday life. One of the most important components for electronic devices is the battery, which provides energy storage and is the main supplier for all electrical energy required for the device's components. Currently, battery commercialization is still limited to the lithium-ion battery (LIB) due to its high-operating voltage and general efficiency. However, the natural supply of lithium is very limited. Even with the discovery of a new lithium deposit in Oregon last month, lithium-shortage is still considered to be the main issue for the development of LIB [1,2,3,4]. Based on this, many studies have been conducted to find the alternatives of lithium, in which sodium is one of the most appropriate alternatives due to its large supply in nature and similar characteristics with an LIB when formed into a sodium-ion battery (SIB) [5,6,7,8,9,10,11].
In practice, an SIB generates energy by using sodium ions as its charge carrier. However, the main problem for the development of an SIB is its lower cycling capability and weaker structural stability for its cathode material. Aside from those, SIB have similar electrochemical properties to their lithium counterparts. The working voltage of an LIB is 3.4 V, while the working voltage of an SIB is 2.7 V [8]. This difference is not so significant and can be overcome by the addition of dopants [12,13,14,15].
NaFePO4 can be classified into two specific structural types (polymorphs): triphylite and maricite. While both structural types have the same anionic framework, the specific crystallographic sites for Na+ and Fe2+ are reversed [16,17,18]. In 2017, Heubner et al. showed that olivine NaFePO4 can be obtained from the LiFePO4 as a precursor through the delithiation-sodiation method [19]. The cathode material produced through this method showed a specific capacity that was close to the theoretical value of triphylite-type of NaFePO4 (154 mAh g−1) followed by poor cycling ability. The poor cycle characteristic of this sample originated from the different size of Li and Na ion radii, which is aggravated during the Li-Na exchange process to get NaFePO4 from LiFePO4 [20]. Due to the cycle instability and difficulties in the formation of triphylite structure of NaFePO4, the maricite structure of NaFePO4 is the preferred type for cathode material purposes [21,22].
In this study, we report the synthesis of maricite NaFePO4 by employing sol-get method followed by calcination at various air temperatures. The sol-gel method is preferred due to its capability to produce low-impurity maricite NaFePO4. Furthermore, we also investigate the effect of two different sodium sources (NaCl and Na2CO3) on the general characteristics of the synthesized NaFePO4. We chose NaCl as the other source of sodium because Indonesia has great potential in sea salt development and production. Aside from the structural analysis of the synthesized sample, an impedance analysis will also be performed to explore the parameters related to the electrochemical properties.
2.
Materials and methods
Two NaFePO4 samples were prepared by mixing Na2CO3/NaCl, FeCl2·4H2O, and NH4H2PO4. The stoichiometric calculation for each material was conducted to produce the exact composition ratio of NaFePO4. For the sol-gel method, the stoichiometric calculations are also conducted for the solvent (HCl/distilled water). For FeCl2·4H2O, NH4H2PO4, and NaCl, the chosen solvent is distilled water, while for Na2CO3 the chosen solvent is HCl. The chemical reaction of NaFePO4 formation based on NaCl can be explained based on Eqs 1–4:
2.1. Thermogravimetric analysis
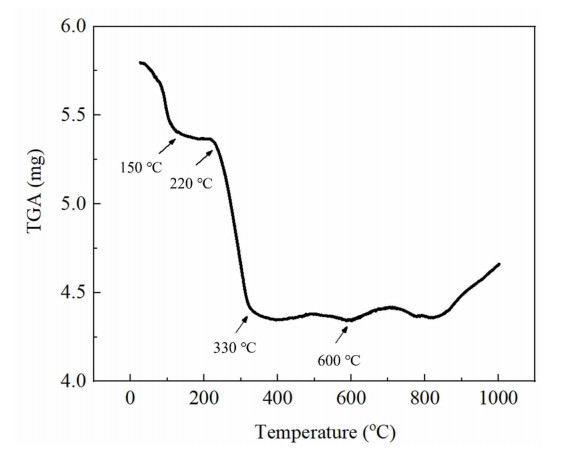
Thermogravimetric analysis (TGA) was performed by heating the as-synthesized NaFePO4 sample from 27 to 1000 ℃. It can be seen from Figure 1 that the first stage of weight loss (27–150 ℃) of the sample can be attributed to the removal of adsorbed water. The second stage of weight loss (220–330 ℃) is due to the evaporation of CO2, H2, and NH3 that is contained in the precursor materials of NaFePO4. After that, the TGA curve tends to be stable between 400 and 800 ℃. Since the TGA curve shows no indication of further mass reduction up to 840 ℃, the sample was then calcined at 550,600, and 650 ℃ for 10 h in the air atmosphere.
2.2. Structural and electrochemical analysis
All synthesized samples were characterized by X-ray diffractometer (XRD) and scanning electron microscopy that is combined with an energy dispersive X-ray analyzer (SEM-EDAX) to observe the microstructural properties of the sample. The XRD measurement is applied by small angle between 2θ = 5 and 60o. The XRD data are obtained by using Cu-Kα radiation λ = 1.54056 Å with the step data value of 0.04o. To obtain the electrochemical properties of the sample, measurements using electrochemical impedance spectroscopy (EIS) were carried out to determine the ionic and charge-transfer conductivities as well as ionic diffusivity. In order to determine the electronic conductivity (σe), ionic conductivity (σi), and the Na+ diffusion coefficient (DNa+), we form the half-coin cell from the synthesized sample with NaCl (1M) as the electrolyte component, and all of the aforementioned parameters are analyzed by a Gamry instrument, and the following equations are utilized:
where in Eqs 5 and 6, t is thickness (cm) of the sample, Rct and Rs are charge-transfer and electrolyte resistances (Ω), A is the surface area of the electrode (cm2). In Eq 7, DNa+is the diffusion coefficient (cm2 s−1) of Na+ ion, R is the ideal gas constant (8.314 J mol−1K−1), T is the absolute temperature (298.15 K), n is the number of ions per molecule that move during intercalation process, F is the Faraday constant (96496 C mol−1), C is the concentration of sodium ions in the bulk form, and σ represents the slope.
3.
Results and discussion
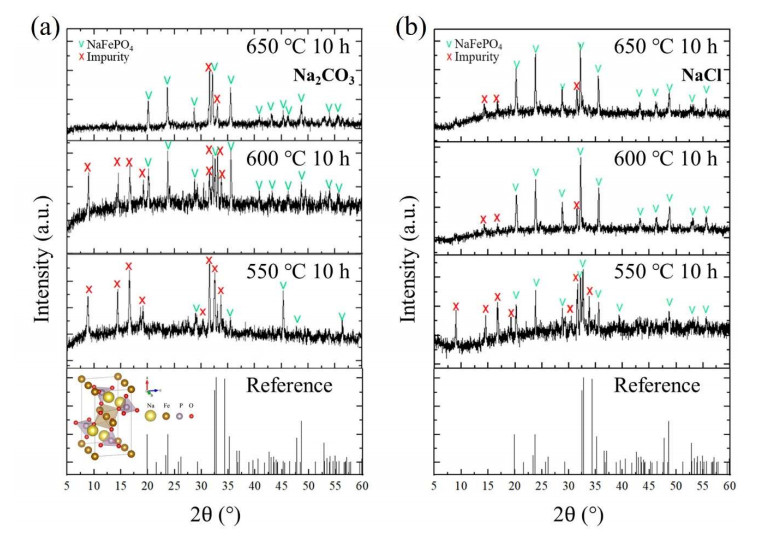
Parts a and b of Figure 2 show the XRD pattern of the representative samples heat treated in air atmosphere for 10 h using Na2CO3 and NaCl, respectively, as sodium sources. Bragg's law (i.e., 2dsinθ = nλ) can be applied to investigate the relation between the XRD patterns and the crystal materials, where d, θ, and λ are the spacing in between crystal planes, the incident angle, and the wavelength of the incident X-ray beam, respectively. The qualitative analysis was carried out to identify the maricite phase formation. According to the data base (PDF-98-005-6292), the diffraction peaks of maricite-type NaFePO4 phase are generally observed on all spectra, growing from samples calcined at the lowest to the highest temperatures. Besides, the presence of peaks concerning the impurity phases were also observed. These impurity peaks gradually disappear in the sample that is calcined at higher temperatures. This impurity can be identified as Na-Fe-P-O but has a different stoichiometry than the maricite type (Na3Fe2(PO4)3) with nasicon structure, which is considered an alternative cathode material in sodium-ion batteries that have lower performance and a specific capacity value of 105 mAh g−1, smaller than that of NaFePO4 [23,24]. The presence of Na3Fe2(PO4)3 as an impurity phase in NaFePO4 was also reported by Sun et al. [25]. NaFePO4 is a sample that can easily oxidize, forming the reaction as explained in Eq 8:
The purity of NaFePO4 maricite phase using Na2CO3 as a sodium source increases along with the calcination temperature. A similar pattern is also observed for XRD data in samples using NaCl as a source of sodium. The sample with the calcination temperature of 650 ℃ has the lowest impurity. Quantitative calculations of NaFePO4 maricite phase purity based on XRD data for all prepared samples are summarized in Figure 3. In general, the sol-gel process for oxide compounds followed by calcination in air will inhibit phase formation due to the excess oxygen content.
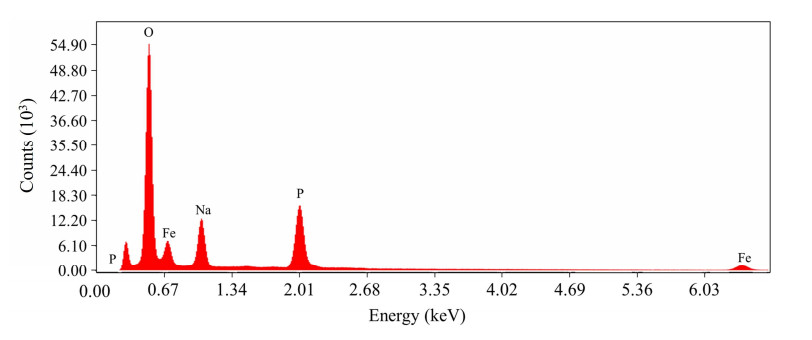
Scanning electron microscope (SEM) investigation was conducted to provide detailed high-resolution images of the sample by rastering a focused electron beam across the surface and detecting secondary or backscattered electron signals. Through the signal, the information of morphology of the sample can be collected. SEM are also equipped with other detectors that have different analytical capabilities, namely energy-dispersive X-ray spectroscopy (EDX). EDX is a measurement that is used to detect elemental identification and quantitative compositional information. Presented in Figure 4 are the representative micro-structure of the samples calcined in air atmosphere at various temperatures using Na2CO3 and NaCl as a source of sodium. It appears that the microstructure develops from initially unclear crystal grains at lower temperature (550 ℃) and evolves to form oval-shaped crystal grains at 650 ℃. There is no abrupt change of morphology of the sample even though the raw material is different. The maricite particles are long-oval shaped, fairly homogeneous, and enlarge upon calcination at higher temperatures. The morphology and averaged grain size of the sample have corroborated the previous synthesis of the same maricite NaFePO4 by solid state reaction [26]. Moreover, the molar ratio of atoms (Na, Fe, P and O) can be estimated from the EDX characterization. The best molar ratio of the constituent atoms Na:Fe:P:O = 1:0.8:0.9:3.7 (closest to 1:1:1:4) is achieved by the sample with the highest NaFePO4 content. The result of EDX shows that there is no unwanted element, implying that there was no contamination during the synthesis process. As shown in Figure 5, Na, Fe, P, and O peaks are presented in NaFePO4 sample heated at 650 ℃ for 10 h.
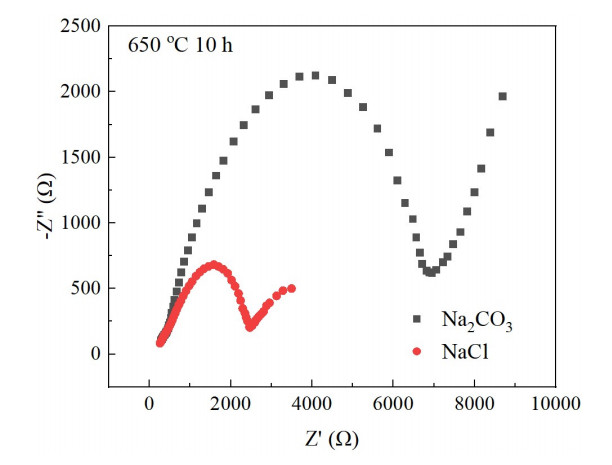
As suggested by Yu et al., the lower crystallinity of the sample will reduce the storage performance of battery system. Thus, we only consider the sample that is calcined at 650 ℃ for 10 h for electrochemical analysis [27]. As given in Figure 6, the Nyquist plot figures the x- and y-axes, standing for the real component (Z') and the imaginary component (Z'') from EIS measurement respectively. The impedance spectrum of a cell in a Nyquist plot consists of two parts: a semicircle (from the diameter, it represents the charge transfer resistance) and a linear tail (from the slope, it reflects the diffusion of Li+ and Na+). The semicircle curve in this high frequency region can be determined by the value of Rs indicated by the smallest Z' real value and Rct which is indicated by the intersection of the semicircle pattern with a straight line. The Rct value is related to the charge-transfer resistance, correlating to the electrochemical reaction between the electrode/electrolyte interface. The value of the electrolyte resistance is related to the magnitude of the flow of electron charges in the grain boundary region between particles. The value of Rs is related to the electrolyte resistance associated with the movement of Na+ ions. Rct is then used to determine the σe, and Rs determines the σi. The straight line in the low frequency region is also known as the Warburg impedance (Zw). The Warburg impedance shows the diffusion of sodium ions at the electrode. In the low frequency region, the Warburg coefficient (σw) gives the value of the Na+ diffusion coefficient (DNa+) [28,29].
The Na+ diffusion coefficient (DNa+), σi, and σe are the parameters which can be analyzed from the Nyquist plot. By comparing the semicircle size of Nyquist plot, it is displayed that sample using Na2CO3 has higher conductivity rather than NaCl. The ion diffusion of sample using Na2CO3 and NaCl are 4.6×10−13 and 7.1×10−14 cm2 s−1, respectively. Massaro et al. reported that the sodium ion diffusion value has a scale of ~10−9 cm2 s−1 calculated by the means of molecular dynamic simulation using a plane polarized force field (Drude model) [30]. Moreover, a study conducted by Liu et al. reported that one of the drawbacks for lithium-ion batteries (LiFePO4) is the sluggish diffusion of lithium ions [31]. They carried out a study related to increasing the diffusion coefficient of Li+ ions by increasing the concentration of added carbon. In LiFePO4, Li+ ion diffusion has a scale of ~10−16 cm2 s−1 without carbon coating and ~10−13 cm2 s−1 with carbon coating. Therefore, NaFePO4 has an ion diffusion coefficient similar to LiFePO4.
The ion diffusion can be studied further using muon spin relaxation (µSR) spectroscopy. Not only for magnetic and superconducting materials [32,33], µSR spectroscopy is also powerful to measure ion diffusion parameters in energy materials [34,35,36]. In EIS, resistance to ion diffusion through grain boundaries contributes to the total resistance of the sample, increasing the activation energy required for ion conduction, while µSR acts as a local probe, mostly sensing intragrain diffusion for ions [35]. One example of µSR measurement in sodium-ion batteries has been done in a Na1.5La1.5TeO6 system [36]. Using µSR, the sodium-ion diffusion coefficients from the fluctuation rate of muon at different temperatures are expressed using Eq 9:
where Ni is the number of accessible sodium sites, Nv, i is the vacancy fraction of each destination site, Si is the distance jumped between sodium ion sites, and v is the hopping rate obtained from µSR data at each temperature. In Na1.5La1.5TeO6 system, sodium ion diffusion coefficient at 300 K of 4.2×10−12 cm2 s−1 was achieved by µSR. The diffusion coefficient at room temperature is similar to that reported for the Na0.7CoO2 and Na0.5CoO2 layered cathode materials, which have values of 3.99×10−11 and 5×10−12 cm2 s−1 respectively [37]. NaMn2O4 has been reported as a sodium-ion cathode possessing a diffusion coefficient at room temperature of 1.1×10−11 cm2 s−1 [38]. The ion diffusion of Na1.5La1.5TeO6 is also comparable with other types of Na+ ionic conductors, for instance NaxWO2Cl2 and Na3PS4 with a diffusion coefficient of 10−13 and of 10−12 cm2 s−1 respectively [39,40]. The ion diffusion of samples in this report are 4.6×10−13 and 7.1×10−14 cm2 s−1, which has one order lower than other types of cathodes in sodium-ion batteries mentioned above and several orders lower compared to the latest experimental result of NaFePO4/C [41]. This could be due to the presence of impurities coming from the Na3Fe2(PO4)3 phase that has lower performance than that of the NaFePO4 phase, since ion diffusion is one of the reasons for the outstanding rate capability. Several studies on diffusion coefficient probing by µSR have been carried out in lithium-ion batteries. The lithium-ion diffusion coefficient in LiFePO4 and LiNi1/3Co1/3Mn1/3O2 at 300 K were reported by µSR to be 3.6×10−10 and 3.5×10−12 cm2 s−1 respectively [37,42].
Based on the comparison of ion diffusion in lithium-ion and sodium ion batteries, sodium-ions will be a strong candidate for the next generation of battery materials. We show that Na2CO3 and NaCl, which have wide availability on Earth, are able to be used as raw materials to obtain NaFePO4 phase that can be employed as cathode battery materials through sol-gel synthesis.
4.
Conclusions
The NaFePO4 sample with maricite phase has been successfully synthesized via the sol-gel method, resulting in a phase purity of 80% using either Na2CO3 or NaCl as a sodium source. The purity of the synthesized NaFePO4 maricite phase can be achieved even though the sintering process is conducted in ambient atmosphere. Based on SEM characterization, the maricite particles are long-oval shaped and fairly homogeneous, which will be enlarged with increasing calcination temperature. The sodium ion diffusion coefficient of two different sources of sodium have comparable values, indicating a great potential for NaCl to be utilized as an electrode in sodium-ion batteries.
Use of AI tools declaration
The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The authors would like to acknowledge the funding support from PDUPT research grant No. 923/PKS/ITS/2021 and Penelitian Keilmuan Dana ITS research grant No. 1724/PKS/ITS/2023.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: