1.
Introduction
Infectious diseases are the public enemy of human population and seriously threatening the health and life. In many infectious disease research methods, epidemic models are widely used for analyzing the spread and control of infectious diseases. Here, the researchers construct an idea of compartmental model to study the dynamics of infectious diseases, initially proposed and investigated by Kermack and McKendrick. That is, SIS epidemic model [1]. Now, Most of models are descended from the classical SIS model. Some authors [2,3,4] analyzed the dynamic behavior of the SIS epidemic model based on variable population, delay, vaccination, pulse, etc. Papers [5,6] have investigated the influence of age structure and stage structure on the dynamic behavior of the SIS epidemic model, and studied the extinction and persistence of the disease. In addition, many results on the SIS epidemic models with network have been reported [7,8,9].
However, In the natural world, epidemic systems are inevitably infected by some stochastic environmental noise. Hence, many authors introduce stochastic interference into deterministic models to reveal the effect of environmental variability on the dynamic of infectious disease. In general the environmental fluctuations can be modeled by Gaussian white noises. The authors [10,11,12] have qualitatively analyzed stochastic SIS epidemic model with vaccination and vertical transmission. In [13], Zhang et al. investigated stochastic SIS epidemic model with vaccination under regime switching, and sufficient conditions for the existence of a unique ergodic stationary distribution has been established. The authors [14,15] studied stochastic models of different diseases, and completed dynamic analysis and optimal control research. But Gaussian white noise can only describe a class of continuous stationary random disturbances. Otherwise, the population may suffer from sudden environmental shocks, namely, some jump type stochastic perturbation, such as, earthquakes, hurricanes, floods, tsunami, epidemic diseases (SARS, avian influenza), commercial harvesting, and so on. These phenomena are discontinuous and jumping at random time ti,i∈N, and the waiting time of jumps is independent. Therefore, these phenomena cannot be described by Gaussian white noise alone. For this kind of sudden, The discontinuous random disturbance of Lˊevy jumps can more truly reflect the changing law of such sudden things. So, it's necessary to introduce Lˊevy jumps process into the dynamics of infectious disease. Bao et al. [16] considered stochastic Lotka-Volterra population systems with jumps for the first time. Zhang et al. [17] studied a stochastic SIQS epidemic model with Lˊevy jumps. Recently, T. Caraballo et al. [18] considered a stochastic SIS model with Lˊevy noise perturbation. M. Naim et al. [19] discussed a stochastic SIS epidemic model with vertical transmission, specific functional response and Lˊevy jumps. About the knowledge of jumps, the readers can further refer to [20,21].
For example, Zhou et al [22] proposed a stochastic SIS model with Lˊevy jumps. as follows:
where dBi(t),i=1,2 are independent Brownian motion defined on the complete probability space (Ω,F,P) with a filtration {Ft}t≥0. And it is increasing and right continuous with F0 containing all P-null sets, σ2i,i=1,2 are the intensities of Bi(t). Λ stands for recruitment rate of the population; μ is the natural death rate; β reprents the disease transmission rate; α is the mortality rate from disease; λ is the recovery rate. S(t−) and I(t−) are the left limit of S(t) and I(t). γi(δ)>−1,i=1,2. Y is a measurable subset of (0,+∞), ν is a Lˊevy measure on Y with ν(δ)<+∞, such that ˜N(dt,dδ)=N(dt,dδ)−ν(dδ)dt. Therein, N is an independent Poisson counting measure with characteristic measure ν.
In (1.1), they obtain a unique positive and global solution of the system. Moreover, the sufficient conditions for extinction and persistence of the disease are established.
Furthermore, many disease are not only disturbed by various environmental noises, but also affected by time and seasonal variation. such as, rubella, measles, chickenpox etc. So, it is more realistic to assume that all of the coefficients in the models are positive T-periodic continuous functions [23,24,25]. In [26], Qi et al. investigated the dynamics of a nonautonomous stochastic SIS epidemic model with nonlinear incidence rate and double epidemic hypothesis. In [27], Zhang et al. formulated a new stochastic nonautonomous SIRI epidemic model, and the thresholds of the stochastic SIRI epidemic model have been obtained. To the best of our knowledge, there are few papers to deal with nonautonomous epidemic model with Lˊevy jumps. Motivated by the above analysis, we assume the following nonautonomous stochastic SIS model:
The rest of this paper is organized as follows: In Section 2, by constructing Lyapunov function, we show the existence and uniqueness of the positive solution of the system (1.2); The conditions for the extinction of the system (1.2) is given In Section 3; In Section 4, we discuss the conditions for the existence of at least one nontrivial positive T-periodic solution of the system (1.2); In Section 5, we obtain the sufficient conditions of the system (1.2) for the persistence of the epidemic disease; The conclusion and numerical experiments of our theoretical results are given in Section 6.
2.
Existence and uniqueness of the positive solution
In this section, based on the theorem of Mao [28], the coefficients of system (1.2) are locally Lipschitz continuous [29]. In what follows, we shall prove that the solution of system (1.2) is positive and global.(i.e. the solution of system (1.2) will not explode in a finite time with probability one). For a bounded integrable function f(t) on [0,∞), we define fl=inft∈[0,∞)f(t), fu=supt∈[0,∞)f(t) and ⟨f⟩t=1t∫t0f(t)dt.
Theorem 2.1. There is a unique and positive solution (S(t),I(t))∈R2+ of system (1.2) with initial condition (S(0),I(0))∈R2+ with probability one, assumptions A1 and A2 hold.
We suppose the jump diffusion coefficient that for each h>0 there exists Lh>0. i.e.
(A1) ∫Y|Fi(x,δ)−Fi(y,δ)|2ν(dδ)⩽, where F_{i}\left(x, \delta\right) = \gamma_{i}\left(\delta\right)x\left(t-\right) with \lvert x \rvert \vee \lvert y \rvert \leqslant h .
\left(A_2\right) \int_Y\left[\gamma_{i}\left(\delta\right)-\ln\left(1+\gamma_{i}\left(\delta\right)\right)\right]\nu \left(d\delta\right)\leqslant \kappa_i, i = 1, 2 , where \kappa_i is positive constant, which biologically interpret intensities of L \acute {e} vy jumps are not too large.
Proof. According to the theorem of Mao [28] and \left(A_{1}\right) , the system \left(1.2\right) exist a unique local solution \left(S\left(0\right), I\left(0\right)\right) on t\in [0, \tau_e) , where \tau_e is the explosion time. To prove that this solution is global, i.e. \tau_e = +\infty . Let the solution defined on the \left[\frac{1}{h_0}, h_0\right] , where h_0 is a sufficiently large positive number.
Define the stop-time
where all h\geqslant h_0 .
We denote \emptyset is the empty set. Let \emptyset = \infty . It is clear that \tau_h is increasing as h\to \infty . Set \tau_\infty = \lim\limits_{h\rightarrow\infty} \tau_h . Then \tau_\infty \leqslant \tau_h . If \tau_\infty = \infty is true, then \tau_h = \infty . Otherwise, there exist constants T > 0 and \varepsilon \in \left(0, 1\right) , such that P\left(\tau_\infty \leqslant T\right)\geqslant \varepsilon . Hence, there exists an integer h_1 \geqslant h_0 such that P\left(\tau_h \leqslant T\right)\geqslant \varepsilon , h \geqslant h_1 .
Define the function V\left(S\left(t\right), I\left(t\right)\right) for \left(S\left(t\right), I\left(t\right)\right)\in R^{2}_+ as follows
where a\left(t\right) is a positive function on [0, 1] .
Using the It \hat{o} 's formula, we have
where LV\left(S, I\right):R^2_+ \to R_+ by
Denote a\left(t\right) = \frac{\beta\left(t\right)}{\mu\left(t\right)+\alpha\left(t\right)} such that \frac {\beta\left(t\right)}{a\left(t\right)}-\mu\left(t\right)-\alpha\left(t\right) = 0 . By using of inequality x-\ln \left(1+x\right)\geqslant 0 for x > -1 and \left(A_2\right) , we conclude
where d = \max\left\{\int_{Y}\left[\gamma_{1}\left(\delta\right)-\ln\left(1+\frac{\gamma_{1}\left(\delta\right)}{1+a^u}\right)\right] \nu\left(d\delta\right), \int_{Y}\left[\gamma_{2}\left(\delta\right)-\ln\left(1+\gamma_{2}\left(\delta\right)\right)\right] \nu\left(d\delta\right)\right\} and D is a positive constant which is independent of S, I and t . we can get that
Integrating \left(2.4\right) from 0 to \tau_h\wedge T .
Taking the expectations of the above inequality leads to
So
Let \Omega_h = \left\{\tau_h\leqslant T\right\} for h \geqslant h_1 . Then P\left(\Omega_h\right)\geqslant \varepsilon . In addition, for any \omega \in \Omega_h , there is at least one of S\left(\tau_h, \omega\right) and I\left(\tau_h, \omega\right) that equals either h or \frac1{h} . We have
where I_{\Omega_h}\left(\omega\right) is the indicator function of \Omega_h\left(\omega\right) .
Let h \to \infty , we have
Therefore, we obtain \tau_\infty = \infty . The proof is completed.
3.
Extinction
In this section, we investigate the conditions for the extinction of disease I\left(t\right) .
Lemma 3.1 . Let \left(S\left(t\right), I\left(t\right)\right) be a solution of system \left(1.2\right) with initial value \left(S\left(0\right), I\left(0\right)\right)\in R^2_+ . Then \lim\limits_{t \to \infty} {\sup \left(S\left(t\right)+I\left(t\right)\right) < \infty} , a.s.
and
Proof. From system \left(1.2\right) , we obtain that
Solving above equation, we have
in which
Obviously, F\left(t\right) and G\left(t\right) are continuous local martingale with F\left(0\right) = 0 and G\left(0\right) = 0 .
Define
where H\left(0\right) = S\left(0\right)+I\left(0\right) , W\left(t\right) = \frac{\Lambda^u}{\mu^u}\left(1-e^{-\mu^ut}\right) and Y\left(t\right) = \left(S\left(0\right)+I\left(0\right)\right)\left(1-e^{-\mu^ut}\right) .
Note that S\left(t\right)+I\left(t\right)\leqslant H\left(t\right) for all t\geqslant 0 . W\left(t\right) and Y\left(t\right) are continuous adapted increasing process on t\geqslant 0 with W\left(0\right) = Y\left(0\right) = 0 . By Theorem 3.9 in [28], we have \lim\limits_{t \to \infty} {H\left(t\right)} < \infty a.s., then for almost all t\geqslant 0
Denote
Then, their quadratic variations
By the Theorem 3.4 in [28] and \left(3.1\right) , one has
which together with the positivity of the solution imply
The proof is therefore completed.
Theorem 3.1 . Let \left(S\left(t\right), I\left(t\right)\right) be a solution of system \left(1.2\right) with initial value \left(S\left(0\right), I\left(0\right)\right)\in R^2_+ . If
\left(i\right)
or
\left(ii\right)
where
Then
a.s.
Proof. By the system \left(1.2\right) , one has
Intergrating \left(3.2\right) , we obtain
Note that
where
Clearly, we have
where
From Lemma 3.1 , it follows that
Applying It \hat{o} 's formula to system \left(1.2\right) leads to
Intergrating \left(3.6\right) , we obtain
Substituting \left(3.4\right) into \left(3.7\right) , we get
According to the large number of theorem [28], we have
Then
Define
Clearly, if R_0 < 1 , then
Moreover, Deriving from \left(3.4\right) and \left(3.5\right) that
This completes the proof.
Remark 3.1 . Theorem 3.1 shows that the disease will die out under the influence of random perturbation. Note that even if \sigma_2\left(t\right) = 0 only L \acute {e} vy process is large, the disease also will die out. That is, L \acute {e} vy process can suppress the spread of the disease.
4.
Existence of Nontrivial T-periodic Solution
In this section, we discuss the existence of the nontrivial positive T-periodic solution.
Lemma 4.1 [30]. Assume that
\left(A_1\right) System has a unique global solution;
\left(A_2\right) There is a function V\left(t, x\right)\in C^2 which is T-periodic in t , and satisfies the following conditions:
and LV\leqslant-1 outside some compact set, where the operator L is given by
Then the system has a T-periodic solution.
Theorem 4.1 . Assume that
R_1 = \frac{3\langle \beta\left(t\right)b\left(t\right)\rangle_T} {\langle \mu\left(t\right)+\frac{\sigma^2_1\left(t\right)}{2}+\int_{Y}\left(\gamma_{1}\left(\delta\right)-\ln\left(1+\gamma_{1}\left(\delta\right)\right)\right) \nu\left(d\delta\right)\rangle_T \langle\lambda\left(t\right)+\mu\left(t\right)+\alpha\left(t\right)+\frac{\sigma^2_2\left(t\right)}{2}+\int_{Y}\left(\gamma_{2}\left(\delta\right) -\ln\left(1+\gamma_{2}\left(\delta\right)\right)\right)\nu\left(d\delta\right)\rangle_T} > 1.
then there exists a nontrivial positive T-periodic solution of system \left(1.2\right) .
Proof. Define
where V_1 = -C\ln S\left(t\right)-\ln I\left(t\right)-I\left(t\right) and C is a positive constant. By the It \hat{o} 's formula, we have
where b\left(t\right) = \Lambda\left(t\right)\bigwedge\lambda\left(t\right) , we get
Define the T-periodic function \omega\left(t\right) satisfying
So
Choose
Then
in which R_1 = \frac{3\langle \beta\left(t\right)b\left(t\right)\rangle_T} {\langle \mu\left(t\right)+\frac{\sigma^2_1\left(t\right)}{2}+\int_{Y}\left(\gamma_{1}\left(\delta\right)-\ln\left(1+\gamma_{1}\left(\delta\right)\right)\right) \nu\left(d\delta\right)\rangle_T \langle\lambda\left(t\right)+\mu\left(t\right)+\alpha\left(t\right)+\frac{\sigma^2_2\left(t\right)}{2}+\int_{Y}\left(\gamma_{2}\left(\delta\right) -\ln\left(1+\gamma_{2}\left(\delta\right)\right)\right)\nu\left(d\delta\right)\rangle_T},
Define Z\left(t\right) = S\left(t\right)+I\left(t\right) and V_2 = Z^{\theta}\left(t\right), \theta > 1 , by It \hat{o} 's formula, we obtain
where
and
Then,
Now, define a compact subset
where \varepsilon is a sufficiently small positive number. Set A^C such that A\cup A^C = R^2_+ and A\cap A^C = \phi .
Then
with
In the set A^C , we choose appropriate positive constant M and a sufficiently small positive constant \varepsilon satisfying the following inequalities
where
Next, we prove LV\leq -1 for any \left(S, I\right)\in A^C .
Case 1. If \left(S, I\right)\in A_1^C , \left(4.1\right) and \left(4.2\right) imply that
Case 2. If \left(S, I\right)\in A_2^C , \left(4.1\right) and \left(4.3\right) imply that
Case 3. If \left(S, I\right)\in A_3^C , we have from \left(4.1\right) and \left(4.4\right)
Case 4. If \left(S, I\right)\in A_4^C , from \left(4.1\right) and \left(4.5\right) it follows that
From the above discussion, we can conclude LV\leq -1 on A^C . By Lemma 4.1 , we show that there exists a T-periodic solution system \left(1.2\right) . This completes the proof of Theorem 4.1 .
5.
Permanence
In this section, we verify the conditions for the permanence of disease I\left(t\right) .
Theorem 5.1 . If R_1 > 1 and \lim\limits_{t \to \infty} {\inf\langle I \rangle_t\geqslant\langle Q\left(t\right)\rangle_T\left(R_1-1\right)} , then the disease of system \left(1.2\right) is permanent in mean.
Proof. Define
Then integrating both side of \left(5.1\right) , we have
where
By Lemma 3.1 and the large number of theorem [28], one can get that
Then
Remark 5.1 . Theorem 5.1 shows that if the random perturbation is not large and R_1 > 1 , the disease is persistent.
6.
Numerical simulations and conclusion
Now, we will introduce some numerical simulation to support our main theoretical results.
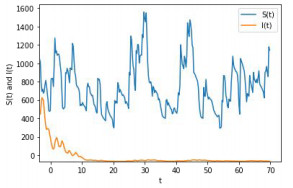
In Fig 1, we choose the parameter in system \left(1.2\right) as follows
\Lambda\left(t\right) = 0.5+0.1\sin\pi t , \beta\left(t\right) = 0.0011+0.001\sin\pi t , \mu\left(t\right) = 0.005+0.005\sin\pi t ,
\lambda\left(t\right) = 0.9+0.1\sin\pi t , \alpha\left(t\right) = 0.03+0.03\sin\pi t , \sigma_{1}\left(t\right) = 0.08+0.08\sin\pi t ,
\sigma_{2}\left(t\right) = 0.03+0.03\sin\pi t , \gamma_{1} = 0.03 , \gamma_{2} = 0.02 .
Then R_1 = \frac{3\langle \beta\left(t\right)b\left(t\right)\rangle_T} {\langle \mu\left(t\right)+\frac{\sigma^2_1\left(t\right)}{2}+\int_{Y}\left(\gamma_{1}\left(\delta\right)-\ln\left(1+\gamma_{1}\left(\delta\right)\right)\right) \nu\left(d\delta\right)\rangle_T \langle\lambda\left(t\right)+\mu\left(t\right)+\alpha\left(t\right)+\frac{\sigma^2_2\left(t\right)}{2}+\int_{Y}\left(\gamma_{2}\left(\delta\right) -\ln\left(1+\gamma_{2}\left(\delta\right)\right)\right)\nu\left(d\delta\right)\rangle_T} .
Note that R_1 > 1 and \lim\limits_{t \to \infty} {\inf\langle I \rangle_t\geqslant\langle Q\left(t\right)\rangle_T\left(R_1-1\right)} , That is, I\left(t\right) will lead the disease to permanent. From Fig 1, the disease will go persistent when the noises are sufficiently small.
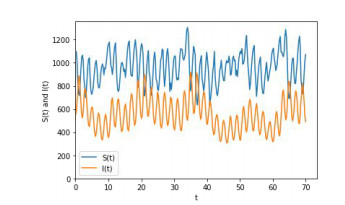
In Fig 2, we take the parameter values in system \left(1.2\right) as
\Lambda\left(t\right) = 0.8+0.1\sin\pi t , \beta\left(t\right) = 0.001+0.001\sin\pi t , \mu\left(t\right) = 0.2+0.1\sin\pi t ,
\lambda\left(t\right) = 0.9+0.1\sin\pi t , \alpha\left(t\right) = 0.1+0.1\sin\pi t , \sigma_{1}\left(t\right) = 0.1+0.1\sin\pi t ,
\sigma_{2}\left(t\right) = 0.3+0.1\sin\pi t , \gamma_{1} = 0.3 , \gamma_{2} = 0.1 .
Note that \eta > \frac{\beta^u \Lambda^u}{\mu^l} , so, condition \left(i\right) of Theorem 3.1 holds. From Fig 2, the disease will go to extinction when the noise is large.
In addition, The difference between conditions of Fig 3 is that the values of \lambda\left(t\right) = 0.8+0.1\sin\pi t , \sigma_{2}\left(t\right) = 0.1+0.1\sin\pi t , \gamma_{1} = 0.4 . Note that \eta\leqslant\frac{\beta^u \Lambda^u}{\mu^l} and R_0 < 1 . Therefore, conditions \left(ii\right) of Theorem 3.1 holds. Then the disease will also go extinct when the noise is large. Thus, it is necessary to consider the effect of the noise in model process.
From the above conclusion, we know that the effect of the noise cannot be ignored in model process. In addition, considering the sudden environmental shocks and the influence of seasonal changes, nonautonomous stochastic model with jumps is better than stochastic model be described by Gaussian white noise alone.
In this paper, Firstly, By constructing suitable stochastic Lyapunov function, we show that the system \left(1.2\right) has a unique positive solution.
\left(1\right) When \eta > \frac{\beta^u \Lambda^u}{\mu^l} , where \eta = \frac{\sigma _{2}^{2l}}2+\int_{Y}\left[\gamma_{2}\left(\delta\right)-\ln \left(1+\gamma_{2}\left(\delta\right)\right)\right]\nu \left(d\delta\right) , we know \eta increase as \sigma _{2}^{2l} and \gamma_{2}\left(\delta\right) increase, the disease will go extinct as long as the noises are large in mean. In addition, If \eta\leqslant\frac{\beta^u \Lambda^u}{\mu^l} and R_0 < 1 , where R_0 = \frac{\beta^u \Lambda^u}{\mu^l\left(\lambda^l+\mu^l+\alpha^l\right)}-\frac{\eta}{\lambda^l+\mu^l+\alpha^l} . The disease can also die out, when the noises are large in mean.
\left(2\right) If R_1 > 1 and \lim\limits_{t \to \infty} {\inf\langle I \rangle_t\geqslant\langle Q\left(t\right)\rangle_T\left(R_1-1\right)} ,
where R_1 = \frac{3\langle \beta\left(t\right)b\left(t\right)\rangle_T} {\langle \mu\left(t\right)+\frac{\sigma^2_1\left(t\right)}{2}+\int_{Y}\left(\gamma_{1}\left(\delta\right)-\ln\left(1+\gamma_{1}\left(\delta\right)\right)\right) \nu\left(d\delta\right)\rangle_T \langle\lambda\left(t\right)+\mu\left(t\right)+\alpha\left(t\right)+\frac{\sigma^2_2\left(t\right)}{2}+\int_{Y}\left(\gamma_{2}\left(\delta\right) -\ln\left(1+\gamma_{2}\left(\delta\right)\right)\right)\nu\left(d\delta\right)\rangle_T} , the disease can be permanent in mean, when the noises are small enough.
\left(3\right) If R_1 > 1 , there is at least one positive T-periodic solution of system \left(1.2\right) . This means the occurrence of disease may be periodic in the ecosystem.
On the basis of this article, some issues deserve further investigation. For instance, We can consider the effects of impulsive or delay perturbations in system \left(1.2\right) . Furthermore, we can also explore some complex nonautonoumous stochastic epidemic models, such as SIR and SEIR model with non-Gaussian white noise. We leave these investigations for the future work.
Conflict of interest
The authors have no conflict of interest.









 DownLoad:
DownLoad: