The semi-tensor product method for special least squares solutions of the complex generalized Sylvester matrix equation

1.   Introduction

In this paper, for the convenience of expression, we first introduce some notations. $\mathbb{R}$, $\mathbb{R}^m$ and $\mathbb{R}^{m\times n}$ stand for the sets of all real numbers, $m$-dimensional real column vectors and $m\times n$ real matrices, respectively. $\mathbb{C}$ and $\mathbb{C}^{m\times n}$ stand for the sets of all complex numbers and $m\times n$ complex matrices, respectively. $\mathbb{HC}^{m\times m}, \mathbb{AHC}^{m\times m}$ stand for the sets of all $m\times m$ complex Hermitian and anti-Hermitian matrices, respectively. $\mathbb{SR}^{m\times m}$ and $\mathbb{ASR}^{m\times m}$ stand for the sets of all $m\times m$ real symmetric and anti-symmetric matrices, respectively. $I_{m}$ is the $m\times m$ identity matrix, and $\delta_m^k$ is the $k$-th column of $I_m$, $k = 1, 2, \cdots, m$. $B^{\dagger }$ and $B^{T}$ represent the Moore-Penrose inverse and the transpose of the matrix $B$, respectively. For the matrix $B = (\beta_1, \beta_2, \cdots, \beta_n)\in \mathbb{R}^{m\times n}$, $vec(B)$ represents the $mn$-dimensional column vector $(\beta_1^T, \beta_2^T, \cdots, \beta_n^T)^T$. $B\otimes C$ represents the Kronecker product of matrices $B$ and $C$. $\|\cdot\|$ represents the 2 norm of a vector or the Frobenius norm of a matrix. For a matrix $C$, $row_i(C), col_j(C)$ represent the $i$-th row and the $j$-th column of $C$, respectively. $rand(m, n)$ is a function in MATLAB.

Linear matrix equations are widely used in applied mathematics, computational mathematics, computer science, control theory, signal and color image processing and other fields, which has aroused the interest of many scholars and achieved some valuable results ^{[1,2,3,4,5,6,7,8]}. Direct method ^{[9,10,11,12,13,14]} and iterative method ^{[15,16,17,18,19,20]} are two common methods to solve linear matrix equations.

In this paper, we are interested in the following generalized Sylvester matrix equation

in which $C, D, E, F, G$ are known matrices, and $X$ is unknown matrix. This matrix equation (1.1) over different number fields has been widely studied in recent years. Many scholars have proposed iterative methods for different solutions of the matrix equation (1.1) ^{[21,22,23,24,25,26]}. For the direct method, some meaningful conclusions are also obtained for the matrix equation (1.1). For example, Yuan et al. ^[27] gave the expression of the minimal norm of least squares Hermitian solution for the complex matrix equation (1.1) by a product for matrices and vectors. Zhang et al. ^[28] studied the least squares Hermitian solutions for the complex matrix equation (1.1) by the real representations of complex matrices. Yuan ^[29] proposed the expressions of the least squares pure imaginary solutions and real solutions for the quaternion matrix equation (1.1) by using the complex representations of quaternion matrices and the Moore-Penrose inverse. Wang et al. ^[30] gave some necessary and sufficient conditions for the complex constraint generalized Sylvester matrix equations to have a common solution by the rank method, and obtained the expression of the general common solution. Yuan ^[31] solved the mixed complex Sylvester matrix equations by the generalized singular-value decomposition, and gave the explicit expression of the general solution. Yuan et al. ^[32] studied the Hermitian solution of the split quaternion matrix equation (1.1). Kyrchei ^[33] represented the solution of the quaternion matrix equation (1.1) by quaternion row-column determinants.

In the process of solving the linearization problem of nonlinear systems, Cheng et al. ^[34] proposed the theory of the semi-tensor product of matrices in recent years. The semi-tensor product of matrices breaks through the limitation of dimension and realizes quasi commutativity, which is a generalization of the traditional matrix multiplication. Now, the semi-tensor product of matrices has been applied to Boolean networks ^[35], graph theory ^[36], game theory ^[37], logical systems ^[38] and so on.

To the best of our knowledge, there is no reference on the study of the complex matrix equation (1.1) by using the semi-tensor product method. Therefore, in this paper, we will use the real vector representations of complex matrices and the semi-tensor product of matrices to study the following two problems.

Problem 1. Suppose $C, E\in \mathbb{C}^{m\times n}, D, F\in \mathbb{C}^{n\times p}, G\in \mathbb{C}^{m\times p}$ and

Find out $\mathbb{X}_{HC}\!\in \!\mathbf{L}_H$ satisfying $\|\mathbb{X}_{HC}\|\! = \!\min\limits_{X\in \mathbf{L}_H}\|X\|$.

Problem 2. Suppose $C, E\in \mathbb{C}^{m\times n}, D, F\in \mathbb{C}^{n\times p}, G\in \mathbb{C}^{m\times p}$ and

Find out $\mathbb{X}_{AHC}\!\in \!\mathbf{L}_{AH}$ satisfying $\|\mathbb{X}_{AHC}\|\! = \!\min\limits_{X\in \mathbf{L}_{AH}}\|X\|$.

$\mathbb{X}_{HC}$, $\mathbb{X}_{AHC}$ are called the minimal norm of least squares Hermitian solution and the minimal norm of least squares anti-Hermitian solution for the complex matrix equation (1.1), respectively.

The rest of this paper is arranged as below. In Section 2, some preliminary results are presented for solving Problems 1 and 2. In Section 3, the solutions of Problems 1 and 2 are obtained by the real vector representations of complex matrices and the semi-tensor product of matrices. In Section 4, two numerical algorithms of solving Problems 1 and 2 are proposed, and two numerical examples are given to show the effectiveness of purposed algorithms. In Section 5, this paper is summarized briefly.

2.   Preliminaries

In this section, we review and present some preliminary results of solving Problems 1 and 2.

Definition 2.1. (^[39]) Let $B\in \mathbb{R}^{m\times n}, C\in \mathbb{R}^{s\times t}$. The semi-tensor product of $B, C$ is defined as

where $q$ is the least common multiple of $n, s$.

In Definition 2.1, when $n = s$, the semi-tensor product of $A, B$ is essentially the traditional matrix product. The semi-tensor product has the following properties.

Lemma 2.2. (^[39]) Suppose $A, B, C, D, E$ are real matrices of appropriate orders. There are the following conclusions:

${\rm (1)}$ $(A\ltimes B)\ltimes C = A\ltimes (B \ltimes C).$

${\rm (2)}$ $A\ltimes(D+E) = A\ltimes D+ A\ltimes E, \quad (D+E)\ltimes A = D\ltimes A+ E\ltimes A.$

Lemma 2.3. (^[39]) Let $\alpha\in \mathbb{R}^m$, $B\in \mathbb{R}^{p\times q}$, and then $\alpha\ltimes B = (I_m\otimes B)\ltimes \alpha.$

Lemma 2.3 reflects the quasi commutativity of vector and matrix. In order to realize the commutativity between vectors, the following swap matrix is important.

Definition 2.4. (^[39]) The following square matrix

is called the $(m, n)$-dimension swap matrix.

Lemma 2.5. (^[39]) Let $\alpha\in \mathbb{R}^m, \beta\in \mathbb{R}^n$, then

where $\mathbf{S}_{[m, n]}$ is as same as that in Lemma 2.4.

To study Problems 1 and 2, we define the real vector representations of complex number, complex vector and complex matrix, and give their properties.

Let a complex number $a = a_1+a_2\mathbf{i}$, where $a_1, a_2\in \mathbb{R}$, and then the following column vector

is defined as the real vector representation of the complex number $a$.

Lemma 2.6. (^[40]) Let $a, b\in \mathbb{C}$, then $\overrightarrow{ab} = \mathbf{W}\ltimes \overrightarrow{a}\ltimes \overrightarrow{b}$, where

Suppose $\alpha = (x_1, x_2, \cdots, x_n), \ \beta = (y_1, y_2, \cdots, y_m)^T$ are two complex vectors. The following column vectors

are defined as the real vector representations of the complex vectors $\alpha, \beta$, respectively. And we can easily get the following properties of the real vector representations of complex vectors:

in which $i = 1, 2, \cdots, n, j = 1, 2, \cdots, m.$

Lemma 2.7. (^[40]) Suppose $\alpha = (x_1, x_2, \cdots, x_n), \beta = (y_1, y_2, \cdots, y_n)^T$ are two complex vectors. $\mathbf{W}$ is as same as that in Lemma 2.7. Then

in which $\mathbf{F}_n = \mathbf{W}\ltimes \sum\limits_{i = 1}^n[(\delta_n^i)^T\ltimes(I_{2n}\otimes (\delta_n^i)^T)]$.

Let $A\in\mathbb{C}^{m\times n}$, and then the following column vectors

are defined as the real column and row vector representations of $A$, respectively. And they satisfy

in which $i = 1, 2, \cdots, n, \ j = 1, 2, \cdots, m.$

Further, the following properties also hold.

Lemma 2.8. Let $A, B\in\mathbb{C}^{m\times n}$, and then

${\rm (1)}$$\overrightarrow{(A\pm B)_c} = \overrightarrow{A_c}\pm\overrightarrow{B_c}, \quad \overrightarrow{(A\pm B)_r} = \overrightarrow{A_r}\pm\overrightarrow{B_r};$

${\rm (2)}$$\|A\| = \|\overrightarrow{A_c}\| = \|\overrightarrow{A_r}\|.$

Proof. (1) Notice that

Thus, we obtain

which show that (1) holds.

(2) Because $\|row_i(A)\|^2 = \|\overrightarrow{row_i(A)}\|^2$, $\|col_j(A)\|^2 = \|\overrightarrow{col_j(A)}\|^2$, we get

Thus (2) holds.

Lemma 2.9. Let $A\in\mathbb{C}^{m\times n}, B\in\mathbb{C}^{n\times p}$. $\mathbf{F}_n$ is as same as that in Lemma 2.8. Then

in which

Proof. Block the matrices $A, B$ into the following forms:

By using of Lemma 2.3 and Lemma 2.8, we obatin

Thus, there is

So Lemma 2.10 holds.

Lemma 2.10. (^[40]) Let $A\in\mathbb{C}^{m\times n}, B\in\mathbb{C}^{n\times p}$. $\mathbf{F}_n$ is as same as that in Lemma 2.8. Then

in which

In the last part of this section, we propose the necessary and sufficient conditions for a complex matrix to be Hermitian and anti-Hermitian.

Lemma 2.11. Let $X = X_1+X_2\mathbf{i}\in \mathbb{C}^{n\times n}$, then

where $\mathbf{M} = (\delta^1_{2n^2}, \delta^3_{2n^2}, \cdots, \delta^{2n^2-1}_{2n^2}, \delta^2_{2n^2}, \delta^4_{2n^2}, \cdots, \delta^{2n^2}_{2n^2}).$

Proof. Let $X_1 = (x_{ij}^{(1)})_{n\times n}, X_2 = (x_{ij}^{(2)})_{n\times n}$, and then

Notice that

so we have

So Lemma 2.12 holds.

For a real matrix $X = (x_{ij})\in \mathbb{R}^{n\times n}$, we denote

$vec_{S}(X), vec_{A}(X)$ stand for the following vectors

Lemma 2.12. (^[7]) Let $X\in \mathbb{R}^{n\times n}$, and then the following conclusions hold.

${\rm (1)}$ $X\in \mathbb{SR}^{n\times n}\Longleftrightarrow vec(X) = P_{n}vec_{S}(X),$ where

${\rm (2)}$ $X\in \mathbb{ASR}^{n\times n}\Longleftrightarrow vec(X) = Q_{n}vec_{A}(X),$ where

We can get the following results by Lemmas 2.12 and 2.13.

Lemma 2.13. Suppose $X = X_1+X_2\mathbf{i}\in \mathbb{C}^{n\times n}$.

${\rm (1)}$ If $X\in \mathbb{HC}^{n\times n}$, then $\overrightarrow{X_c } = \mathbf{H} \left(\!\!\begin{array}{c} vec_S(X_1)\\ vec_A(X_2)\\ \end{array} \!\!\right),$ where $\mathbf{H} = \mathbf{M} \left(\!\! \begin{array}{cc} P_n & 0 \\ 0 & Q_n \\ \end{array} \!\!\right).$

${\rm (2)}$ If $X\in \mathbb{AHC}^{n\times n}$, then $\overrightarrow{X_c } = \mathbf{\widetilde{H}} \left(\!\!\begin{array}{c} vec_A(X_1)\\ vec_S(X_2)\\ \end{array} \!\!\right),$ where $\mathbf{\widetilde{H}} = \mathbf{M} \left(\!\! \begin{array}{cc} Q_n & 0 \\ 0 & P_n \\ \end{array} \!\!\right).$

Proof. For $X\in \mathbb{HC}^{n\times n}$, we have $X_1\in \mathbb{SR}^{n\times n}, X_2\in \mathbb{ASR}^{n\times n}$. By Lemma 2.13, we obtain

According to Lemma 2.12, we get

which shows that (1) holds. Similarly, we can prove that (2) holds.

3.   The solutions of Problems 1 and 2

In this section, by using the real vector representations of complex matrices and the semi-tensor product of matrices, we first transform the least squares problems of Problems 1 and 2 into the corresponding real least squares problems with free variables, and then obtain the expressions of the solutions of Problems 1 and 2. Further, we give the necessary and sufficient conditions for the complex matrix equation (1.1) to have Hermitian and anti-Hermitian solutions.

Theorem 3.1. Let $C, E\in \mathbb{C}^{m\times n}, D, F\in \mathbb{C}^{n\times p}, G\in \mathbb{C}^{m\times p}$. $\mathbf{S}_{[2np, 2n^2]}, \mathbf{K}_{mnp}, \widetilde{\mathbf{K}}_{mnn}, \mathbf{H}$ are as same as those in Definition 2.4, Lemmas 2.10, 2.11 and Lemma 2.14, respectively. Denote

Then the set $\mathbf{L}_H$ in Problem 1 can be expressed as

and the unique Hermitian solution $\mathbb{X}_{HC}\in \mathbf{L}_{H}$ of Problem 1 satisfies

Proof. By Lemma 2.5, Lemmas 2.9–2.11, we obtain

For the Hermitian matrix $X = X_1+X_2\mathbf{i}$, by applying Lemma 2.14, we have

So we get

Then the complex least squares problem of Problem 1 is converted into the real least squares problem with free variables

The general solution of the above least squares problem is

Then applying Lemma 2.14, we obtain that (3.1) holds. Therefore, the unique Hermitian solution $\mathbb{X}_{CH}\!\in\!\mathbf{L}_{H}$ of Problem 1 satisfies (3.2).

Now, we propose a necessary and sufficient condition that the complex matrix equation (1.1) has a Hermitian solution, and the expression of general Hermitian solutions.

Corollary 3.2. Let $C, E\in \mathbb{C}^{m\times n}, D, F\in \mathbb{C}^{n\times p}, G\in \mathbb{C}^{m\times p}$. $\mathbf{H}$, $\mathbf{U}$ are as same as those in Lemma 2.14 and Theorem 3.1, respectively. Then the necessary and sufficient condition that the complex matrix equation (1.1) has a Hermitian solution is

If (3.3) holds, the Hermitian solution set of the complex matrix equation (1.1) is

Proof. The necessary and sufficient condition that the complex matrix equation (1.1) has a Hermitian solution is for any $X\in \mathbb{HC}^{n\times n}$,

According to Theorem 3.1, we have

Therefore, the necessary and sufficient condition that the complex matrix equation (1.1) has a Hermitian solution is

which illustrates that (3.3) holds. For $X\in {\mathbb{HC}}^{n\times n}$, we get

Therefore, when the complex matrix equation (1.1) has a Hermitian solution, the Hermitian solutions of (1.1) satisfy the following equation

By Theorem 3.1, (3.4) is established.

Theorem 3.3. Let $C, E\in \mathbb{C}^{m\times n}, D, F\in \mathbb{C}^{n\times p}, G\in \mathbb{C}^{m\times p}$. $\widetilde{\mathbf{H}}, \mathbf{U}$ are as same as those in Lemma 2.14 and Theorem 3.1, respectively. Then the set $\mathbf{L}_{AH}$ of Problem 2 can be expressed as

and the unique anti-Hermitian solution $\mathbb{X}_{AHC}\in \mathbf{L}_{AH}$ of Problem 2 satisfies

Proof. By Theorem 3.1, we obtain

For $X = X_1+X_2\mathbf{i}\in\mathbb{AHC}^{n\times n}$, we have $\overrightarrow{X_c} = \widetilde{\mathbf{H}}\left(\! \begin{array}{c} vec_A (X_1) \\ vec_S (X_2) \\ \end{array} \!\right).$ Thus we get

$\min\left\|\mathbf{U}\widetilde{\mathbf{H}}\left(\! \begin{array}{c} vec_A (X_1) \\ vec_S (X_2) \\ \end{array} \! \right)\! -\!\overrightarrow{G_c} \right\|$ has the following general solution:

By Lemma 2.14, (3.5) holds. Further, the unique anti-Hermitian solution $\mathbb{X}_{ACH}\!\in\!\mathbf{L}_{AH}$ of Problem 2 satisfies (3.6).

Now, we give the necessary and sufficient condition that the complex matrix equation (1.1) to have an anti-Hermitian solution and the expression of general anti-Hermitian solution. Because the research method is similar to Corollary 3.2, we only give these conclusions and omit the specific derivation process.

Corollary 3.4. Let $C, E\in \mathbb{C}^{m\times n}, D, F\in \mathbb{C}^{n\times p}, G\in \mathbb{C}^{m\times p}$. $\widetilde{\mathbf{H}}, \mathbf{U}$ are as same as those in Lemma 2.14 and Theorem 3.1, respectively. Then the necessary and sufficient condition that the complex matrix equation (1.1) has an anti-Hermitian solution is

If (3.7) holds, the anti-Hermitian solution set of the complex matrix equation (1.1) is

Remark 1. The semi-tensor product of matrices provides a new method for solving linear matrix equations. The feature of this method is to first convert complex matrices into the corresponding real vectors, and then use the quasi commutativity of the vectors to transform the complex matrix equation into the real linear system with the form $Ax = b$, so as to obtain the solution of the complex matrix equation. This method only involves real matrices and real vectors, so it is more convenient in numerical calculation. The weakness of this method is that it leads to the expansion of the dimension when the complex matrix is straightened into a real vector, and therefore it is not convenient to calculate the complex matrix equation of higher order.

Remark 2. In ^[27], the authors propose a method of solving the solution of Problem 1 by a product for matrices and vectors. This method involves a lot of calculations of complex matrix, the Moore-Penrose inverse and matrix inner product, which reduces the accuracy of the result to some degree. The numerical example in Section 5 will illustrate this.

4.   Numerical algorithms and examples

In this section, two numerical algorithms are first proposed to solve Problems 1 and 2, and then two numerical examples are given to show the effectiveness of purposed algorithms. In the first example, we give the errors of the solutions of Problems 1 and 2. In the second example, we compare the accuracy of the solution of Problem 1 calculated by Algorithm 4.1 and the method in ^[27]. All calculations are implemented on an Intel Core i7-2600@3.40GHz/8GB computer by using MATLAB R2021b.

Algorithm 4.1. (The solution of Problem 1)

$\rm (1)$ Input matrices $C, E\in \mathbb{C}^{m\times n}$, $D, F\in \mathbb{C}^{n\times p}$, $G\in \mathbb{C}^{m\times p}$, $\mathbf{W}$, $\mathbf{S}_{[2np, 2n^2]}$, $P_n, Q_n$, $\mathbf{M}$.

$\rm (2)$ Generate $\overrightarrow{C_r}, \overrightarrow{E_r}, \overrightarrow{D_c}, \overrightarrow{F_c}, \overrightarrow{G_c}$, $\mathbf{F}_n$, and then calculate $\mathbf{K}_{mnp}, \mathbf{\widetilde{K}}_{mnn}$, $\mathbf{U}$ and $\mathbf{H}$.

$\rm (3)$ Calculate the unique Hermitian solution $\mathbb{X}_{CH}$ of Problem 1 by (3.2).

Algorithm 4.2. (The solution of Problem 2)

$\rm (1)$ Input matrices $C, E\in \mathbb{C}^{m\times n}$, $D, F\in \mathbb{C}^{n\times p}$, $G\in \mathbb{C}^{m\times p}$, $\mathbf{W}$, $\mathbf{S}_{[2np, 2n^2]}$, $P_n, Q_n$, $\mathbf{M}$.

$\rm (2)$ Generate $\overrightarrow{C_r}, \overrightarrow{E_r}, \overrightarrow{D_c}, \overrightarrow{F_c}, \overrightarrow{G_c}$, $\mathbf{F}_n$, and then calculate $\mathbf{K}_{mnp}, \mathbf{\widetilde{K}}_{mnn}$, $\mathbf{U}$ and $\widetilde{\mathbf{H}}$.

$\rm (3)$ Calculate the unique anti-Hermitian solution $\mathbb{X}_{AHC}$ of Problem 2 by (3.4).

Example 4.1. Suppose $C, E\in \mathbb{C}^{m\times n}$, $D, F\in \mathbb{C}^{n\times p}$ are random matrices generated by MATLAB. Let $M_1\! = \!rand(n, n)$, $M_2\! = \!rand(n, n)$.

${\rm (1)}$ According to $M_1, M_2$, we generate the following matrix

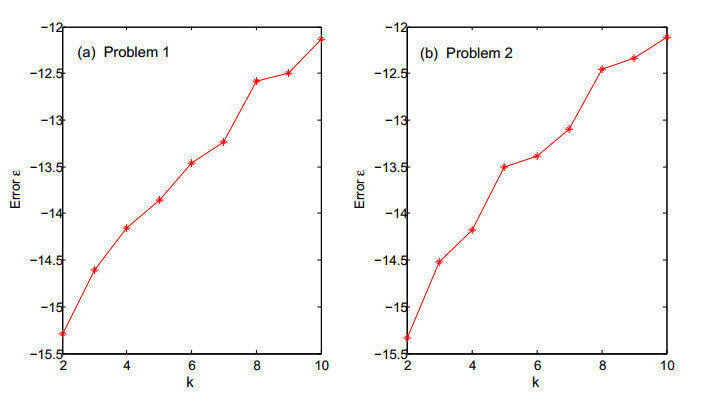
and then $X\in\mathbb{HC}^{n\times n}$. Let $G = CXD+EXF$, and $m\! = \!n\! = \!p\! = \!k$. Here, all matrices are square, and the probability that random matrices are nonsingular is 1. Therefore, (1.1) has a unique Hermitian solution $X$, which is also the unique solution of Problem 1. We calculate the solution $\mathbb{X}_{HC}$ of Problem 1 by Algorithm 4.1. Let $k = 2:10$ and the error $\varepsilon = log_{10}(\|\mathbb{X}_{HC}\!-\!X\|)$. The relation between the error $\varepsilon$ and $k$ is shown in Figure 1 (a).

${\rm (2)}$Generate the following matrix

and then $X\in\mathbb{AHC}^{n\times n}$. Let $G = CXD+EXF$, and $m\! = \!n\! = \!p\! = \!k$. Then, (1.1) has a unique anti-Hermitian solution $X$, which is also the unique solution of Problem 2. We calculate the solution $\mathbb{X}_{AHC}$ of Problem 2 by Algorithm 4.2. Let $k = 2:10$ and the error $\varepsilon = log_{10}(\|\mathbb{X}_{AHC}\!-\!X\|)$. The relation between the error $\varepsilon$ and $k$ is shown in Figure 1 (b).

From Figure 1, we see that $\varepsilon < -12$, and thus the errors of solving Problems 1 and 2 are all no more than $10^{-12}$. This shows that the effectiveness of Algorithms 4.1 and 4.2. In addition, (a) and (b) of Figure 1 are little difference, which reflects that the calculation amount of the solution of Problem 1 is almost the same as that of Problem 2. These are consistent with theoretical reality, and therefore Figure 1 is reasonable.

Example 4.2. Suppose

$X$ is same as that in Example 4.1. Let $G = CXD+EXF$. When $m = n = p = k$, $X$ is the unique solution of Problem 1. $X_1$, $X_2$ represent the solutions of Problem 1 computed by Algorithm 4.1 and the method in ^[27], respectively. Let $\epsilon_1 = \|X_1-X\|, \epsilon_2 = \|X_2-X\|$. Table 1 shows the errors $\epsilon_1, \epsilon_2$ of matrices of different orders.

Table 1 illustrates that the errors obtained by Algorithm 4.1 are smaller than those obtained by the method in ^[27]. This is because, in the process of solving Problem 1, ^[27] involves a lot of calculations of complex matrix, the Moore-Penrose inverse and matrix inner product, which leads to the reduction of calculation accuracy. Thus Algorithm 4.1 is more accurate and efficient.

5.   Conclusions

In this paper, we obtain the minimal norm of least squares Hermitian solution and the minimal norm of least squares anti-Hermitian solution for the complex matrix equation $CXD+EXF = G$ by the semi-tensor product of matrices. The numerical examples show that our purposed method is more effective and accurate. The semi-tensor product of matrices provides a new idea for the study of matrix equations. This method can also be applied to the study of solutions of many types of linear matrix equations.

Acknowledgments

The study is supported by the Scientifc Research Foundation of Liaocheng University (No. 318011921), Discipline with Strong Characteristics of Liaocheng University – Intelligent Science and Technology (No. 319462208), and the Natural Science Foundation of Shandong Province of China (Nos. ZR2020MA053 and ZR2022MA030).

Conflict of interest

The authors declare that there is no conflict of interest.