1.
Introduction
With more attention to integro-differential equations, the numerical calculation of fractional integro-differential equations has been also paid more attention by many scholars. Fractional integro-differential equations have a profound physical background and a rich connotation. For various phenomena in damping laws, diffusion process[31], earthquake model[13], fluid-dynamic traffic model[14], mathematical physics and engineering[28,37], chemistry, acoustics, fluid and continuum mechanics[8], psychology[1,35] and other fields, fractional integro-differential equations are suitable models.
In recent years, several numerical methods were proposed to solve the fractional integro-differential equation. The most common methods are the Adomian decomposition method [25], collocation method [33] and fractional differential transform method [2]. In [16], Huang proposed a method for solving linear fractional integro-differential equations by Taylor's expansion, including Fredholm type and Volterra type. Yang et al. [34] used the Laplace decomposition method to solve the fractional integro-differential equation. M. Jani et al. [17] proposed a numerical method for solving fractional integro-differential equations with nonlocal boundary conditions by using Bernstein polynomials.
The fractional differential operators are nonlocal and have weakly singular kernels. The fractional differential equations are more complicated than the integer-order counterparts. In recent years, many numerical methods have been extended to fractional integro-differential equations. Most of the analyses have some unreasonable limitations on solutions in order to achieve high accuracy. When these equations are transformed into the equivalent Volterra integral equations of the second kind with a weakly singular kernel, even if the input function is smooth, the solution of the equation usually exhibits a weak singularity at z=0. Which leads to a non-smooth solution and a lower order of convergence.
So far, in order to solve the fractional differential equations and fractional integro-differential equations with non-smooth solutions, several methods have been proposed. One method is to approximate the fractional derivative operators in the governing differential equation directly and then the corresponding collocation schemes are derived [3,18]. Another method is to rewrite the governing differential equation in an equivalent integral equation, solved by the corresponding collocation method [22,36,21]. The integral collocation method is more stable than the differential collocation method. The reason is that numerical differentiation is sensitive to small perturbations in the input. But numerical integration is essentially stable [10]. Therefore, when using the differential collocation method to solve differential equations, we need to employ efficient integration preprocessing to overcome the ill-conditioning problem. It is necessary with increasing of the number of collocation nodes [15]. We would like to note that Hao proposed an efficient finite difference algorithm to solve fractional boundary value problems with non-smooth solutions in [12].
For many types of equations with non-smooth solutions, the idea of introducing suitable transformations has been considered. It would eliminate the singularity in the transformed equation, and lead to a high convergence order. For the second kind of Volterra integral equation, Chen and Tang [9] proposed a variable transformation to eliminate the singularity of the solution. With a strict error analysis, the method is shown to have a spectral convergence. Pedas [19] made a proper transformation, and used the piecewise polynomial collocation method to solve the resulting equation on a mildly graded grid or a uniform grid. Baratella and Orsi [7] used a variable transformation to turn the solution of the linear Volterra integral equation of the second kind smooth, and solved it by the standard product integration method. Tang [20] used a variable transformation and the Jacobi spectral collocation method to solve the Abel-Volterra integral equation of the second kind. For the linear Fredholm integral equation of the second kind, Monegato and Scuderi [26] proposed a non-linear transformation to eliminate the singularity of the equation. Ghoreishi [11] used a variable transformation and a spectral method to solve the multi-order fractional differential equation. Pedas et al. [27] regularized the solution of fractional initial and boundary value problems by a suitable smoothing transformation. They solved the transformed equation by a piecewise polynomial collocation method on a mildly graded grid and on a uniform grid. Zaky [38] used a smoothing transformation and the Jacobi spectral collocation method to solve the rational-order fractional terminal value problems with non-smooth solutions.
Based on the above works, we apply the smoothing transformation and the Jacobi spectral collocation method with high accuracy and global characteristics to the following fractional integro-differential equations.
where ˜g(z) is the source function, and ˜K(z,τ) is the kernel function. The given function ˜g(z) and ˜K(z,τ) are continuous on their respective domains 0≤τ≤z≤T and ΔT:={(z,τ)∈R2:0≤τ≤z≤T}, λ∈R. Dαz is the Caputo fractional derivative of rational-order α, 0<α<1.
Let Γ(⋅) denote the Gamma function. For any positive integer n with n−1<α<n, the Caputo derivative is defined as follows:
In addition, the Riemann-Liouville fractional integral Iαz of order α is defined by
We note that,
The layout of this paper as follows: In Section 2, we introduce the basic properties of Jacobi polynomials and Jacobi-Gauss interpolation. The fractional integral differential equation is transformed into an equivalent integral equation, in Section 3. A smoothing transformation of variable is defined for the new equation so that the solution is smooth, in Section 3. The Jacobi spectral-collocation method is defined in Section 4. The convergence analysis of the collocation method is derived in Section 5. In Section 6, we give several numerical examples verifying the accuracy of the theoretical estimation and the feasibility and effectiveness of the method. Finally, some concluding remarks are drawn in Section 7.
2.
Some properties of Jacobi polynomials
In this section, we introduce some basic properties about Jacobi polynomials and Jacobi-Gauss interpolation that are related to spectral-collocation methods [30].
The Jacobi polynomials, denoted by Pα,βn(x), are orthogonal with the Jacobi weight function ωα,β(x)=(1−x)α(1+x)β over Λ=(−1,1), namely,
where δi,j is the Kronecker function and
For a given positive integer N≥0, let PN denote the space of all polynomials of degree not exceeding N. We denote by {xα,βi,ϖα,βi}Ni=0 the set of quadrature nodes and weights of the Jacobi-Gauss integration. The Jacobi-Gauss integration formula has the form
The above quadrature formula (5) is exact for any φ(x)∈P2N+1. Hence, by (3),
For any μ∈C(Λ), the Jacobi-Gauss interpolation operator Iα,βx,N:C(Λ)→PN is determined uniquely by
The interpolation condition (7) implies that Iα,βx,Nμ=μ for all μ∈PN. On the other hand, since Iα,βx,Nμ∈PN, we can write
In particular, for β=0, the set of Jacobi polynomials is reduced to Jαi(x). Therefore, we can also write xαj=xα,0j,ϖαj=ϖα,0j and Iαx,N=Iα,0x,N.
3.
Setting the problem
In this section, we use the definition and related properties of Riemann-Liouville fractional integral and Caputo fractional derivative to transform the original fractional equation with initial conditions into the second kind of Volterra integral equation with weak singular kernel. We show the equation has a non-smooth solution. Then we apply the smoothing transformation to eliminate the singularity of the solution at the left endpoint.
First, we use (2) to transform the original equation (1) into an equivalent Volterra integral equation with weak singular kernel.
Using Dirichlet's formula
we derive
The equation (9) is transformed into the following Volterra integral equation of the second kind by the linear transformation w=τ−sz−s, w∈[0,1],
where
Lemma 3.1. The kernel function K(w(z−s)+s,s) in (10) is continuous and bounded.
Proof. We have
For z∈[0,T] and s∈[0,z], z−s∈[−z,T]. It is known that ˜K(w(z−s)+s,s) is bounded and continuous on ΔT. Let ˜K(w(z−s)+s,s) have the maximum and minimum values on ΔT, Qmax and Qmin, respectively. There is
where B(⋅,⋅) is the β function: B(ξ,η)=∫10sξ−1(1−s)η−1dξ. The lemma is proved.
We give some lemmas on smoothness of solution of the general Volterra integral equation of the second kind.
Lemma 3.2. [23] Consider the following general Volterra integral equation of the second kind:
where γ>−1, f:I→Rn is a continuous bounded function, K:S×Rn→Rn is a continuous bounded function, and {S=(z,s):0≤s≤z≤T}.
1. If f(z) and K(z,s,y(s)) are differentiable, the integral equation has a unique solution y(z) that is also differentiable on (0,T];
2. If f(z)=F(z,z1+γ), F(z1,z2) and K(z,s,y(s)) are differentiable, the integral equation has a unique solution y(z) that satisfies
where Y(z1,z2) is differentiable at (0,0).
We note that the solution of equation (14) is not smooth at z=0 in general.
It can be obtained from the above lemma that the Eq.(10) has a unique solution y(z), which is differentiable on z∈(0,T] and is not necessarily smooth at z=0. For 0<1−α<1, the equation has a singular term (z−s)α−1. By the literature [5], for any positive integer m, if K(τ(w(z−s)),s) and f(z) are continuous differentiable functions of order m in the corresponding area, there exists a function Y=Y(z,v) possessing continuous derivatives of order m, such that the solution of the Eq.(10) can be written as y(z)=Y(z,zα). This indicates that when z→0, y(m)(z)∼zα−m, and thus y(z)∉Cm[0,T].
Lemma 3.3. [4] Let 0<α<1. We assume that f∈Cm(I), K∈Cm(D) for some m≥0.
1. If m=0, the Volterra integral equation,
possesses a unique solution y(z)∈C(I). This solution has the representation
where the resolvent kernel Rμ(z,s) of the kernel (z−s)−μK(z,s) has the form
Here, Qμ(z,s) is continuous on D.
2. If m≥1, every nontrivial solution has the property that y(z)∉C1(I): as z→0+ the solution behaves like
In earlier work, when 0<1−α<1, the general form of the exact solution of the Eq.(10) has been derived, in next lemma.
Lemma 3.4. [5] Assume that f∈Cm(I) with m∈N+, and K(w(z−s)+s,s)∈Cm(I×I) with K(w(z−s)+s,w(z−s)+s)≠0 on I=[0,T]. Then, the regularity of the unique solution of the weakly singular Volterra integral equation (10) can be described by
where (j,k)μ:={(j,k):j,k∈N+∪0,j+k(1−μ)<m},γj,k(μ) are some constants and Ym(⋅;μ)∈Cm(I).
Lemma 3.5. [6] Suppose that f∈Cm(I), K(w(z−s)+s,s)∈Cm(I×I) on I=[0,T], and K(w(z−s)+s,s)≠0 with m≥0. Let μ=1−α, 0<μ<1. The Volterra integral equation (10) has a unique solution y(z)∈C[0,T]⋂Cm(0,T]. Further, y(z) has the following form
where ψk∈Cm(I),k≥1, and the series is absolutely uniformly convergent on I.
If μ=pq is rational (i.e.,p,q∈N, reduced to lowest terms), then the solution of the Eq.(10) can be expressed in the form
where νs∈Cm(I)(0≤s≤q−1).
From the above lemma, y(z)∉Cm[0,T]. For the Eq.(13), Chen and Tang [9] proposed the function transformation ˜y(t)=tu+m−1[y(t)−y(0)] to remove a single term singularity like t1−u−m. They used the spectral-collocation method and achieved excellent results. Our work has been inspired by their excellent results in [9,32]. We apply the similar transformation mentioned above to (14), and obtain the following lemma. This result will be the starting point to the construction of the numerical method presented later.
Lemma 3.6. Using the following transformation for (14),
it is deduced that
1. if σ=q or a multiple of q, then Y(t)∈Cm(I′);
2. if σ≥qq−p, then Y(t) is at least a first-order differentiable function.
Proof. Taking the above transformation (15) to (14), we obtain
Thus we deduce the two conclusions in the lemma.
By the Lemma 3.5, it follows that the solution of the Eq.(10) can be written in the form of ˜y(z)=y1(z)+y2(z) where, for a fixed m, y1(z)∈Cm(I) and y2(z) is the non-smooth part of the solution.
Our first step is to replace ˜y(z) by ˜y(z):=y(z)+λ, where y(0)=0. Hence, the Eq.(1) can be expressed in terms of y as
An equivalent integral form of the above equation is
We apply the smoothing transformation
reducing the problem (17) to the following integral equation whose solution does not involve anymore singularities in the first derivative.
Moreover, using the linear transformation
the Eq.(18) becomes
Furthermore, in order to transform the integral interval (0,T(ξ+12)σ) into (−1,ξ), we use the linear transformation τ=T(η+12)σ,η∈Λ, we obtain
We use the following linear transformation to convert the integration interval (−1,ξ) to (−1,1).
Equation (20) can be written as
Using the formula
(21) becomes
where
Finally, by the change of variable
and setting
(22) is reduced to
where
4.
The Jacobi spectral collocation method
In this section, we propose the Jacobi spectral-collocation method to (23). Solving Equation (23) by the Jacobi spectral-collocation method is to find YN(x)∈PN, such that
where
In order to implement the above basic algorithm more effectively, we set
Employing (25) and (3), we can directly calculate the result that
Using (26), (5) and (8) it yields
where
Similarly, by Eqs. (3) and (25), we conclude that
Using (28), (5) and (8), we obtain
where
In summary, by (25)-(29), we deduce that
Finally, using (3) it yields
The numerical solution can be obtained by solving the equations.
5.
Error analysis
In this section, we estimate the error of the numerical solution. We bound the error in the L∞ and L2ωα,β norms. In order to give the subsequent lemmas conveniently, we first introduce some spaces.
Let the region Λ⊂Rn be a non-empty Lebesgue measurable set, u(x) is a real value Lebesgue measurable function defined on Λ. Lp(Λ) is defined as
equipped with the norm,
For a non-negative integer l, and 1≤p≤∞, the Sobolev space is defined as:
If p=2, we record Hl,2(Λ) as Hl(Λ), which is a separable Hilbert Space. If l=0, Hl,p(Λ) is Lp(Λ) space.
Then we introduce the weighted L2ωα,β(Λ) space. Assume that the weight function ωα,β(x)=(1−x)α(1+x)β with α,β>−1, then
endowed with the norm and inner product
The weighted Hilbert space is defined as follows
equipped with the norm, semi-norm and inner product
For a non-negative integer l, we introduce the non-uniformly Jacobi-weighted Sobolev space
endowed with the norm, semi-norm and inner product
where ‖u‖ωα,β is the norm of L2ωα,β(Λ). Especially, L2(Λ)=B0ω0,0, ‖⋅‖=‖⋅‖L2(Λ) and ‖⋅‖∞=‖⋅‖L∞(Λ). The non-uniform Jacobi-weighted Sobolev space distinguishes itself from the usual weighted Sobolev space Hlωα,β by involving different weight functions for derivatives of different orders. It is clear that Hlωα,β is a subspace of Blωα,β, that is ‖u‖Blωα,β≤c‖u‖Hlωα,β.
The space L∞(Λ) is the Banach space of the measurable functions u that are bounded outside a set of measure zero, equipped the norm
We denote by Cm(Λ) the space of m-times continuously differentiable functions on the interval Λ.
Lemma 5.1. [24] Let Fj(x)Nj=0 be the Nth Lagrange interpolation polynomials associated with the N+1 Gauss points of the Jacobi polynomials. Then
Lemma 5.2. [29] For α,β>−1, and any u∈Blωα,β with l≥1, and integers 0≤m≤l≤N+1,
Moreover, for any u∈Hlω−1/2,−1/2 with 1≤l≤N+1,
where c is a positive constant independent of l,N and u.
Lemma 5.3. [38] Let vα−1i be the Jacobi-Gauss nodes in Λ and ξα−1i=ξ(x,vα−1i). The mapped Jacobi-Guass interpolation operator xIα−1ξ,N:C(−1,x)→PN(−1,x) is defined by
Hence
and
From the above formula, we can derive the following results
Moreover, if we denote I as the identity operator, for any 1≤l≤N+1, we have that
Now, we are ready to prove the following convergence results.
5.1. Error analysis in L2ωα−1,0(Λ)
Theorem 5.4. Let Y be the exact solution of the original equation (16). Let YN be the numerical solution obtained by the discrete scheme (25) combined with the approximate Eq. (24). Let eN=Y−YN.
where
Here
Proof. It follows from (22) that
where xIα−1ξ,N is defined in Lemma 5.3, and
By (34), we obtain
where
The theorem is proved.
Theorem 5.5. Let Y be the exact solution of the original equation (16). Let YN is the numerical solution obtained by the discrete scheme (25) combined with the approximate equation (24). Let α∈(0,1). Assume that Y∈Blωα−1,0(Λ) with 1≤l≤N+1. We conclude the error estimate:
where
Proof. By Lemma 5.2,
Using (5), we get
Apply the Cauchy-Schwarz inequality,
where
and Lemma 5.3, we derive
where
For any xi∈(−1,1), and α∈(0,1),
Hence
In the same way, by the Cauchy-Schwarz inequality, we deduce that
Using Lemma 5.3 yields
Similarly, by (5), we obtain that
By the Cauchy-Schwarz inequality, we further get
where
Applying Lemma 5.3 leads to
By (41),
Similarly, using the Cauchy-Schwarz inequality, we further get
From the above formula, we can get
Also using Lemma 5.3 and (41), we obtain
Drawn by the above formula,
In summary,
5.2. Error analysis in L∞(Λ)
Theorem 5.6. Let Y be the exact solution of the original equation (16). YN is the numerical solution obtained by the discrete scheme (25) combined with the approximate equation (24). Let α∈(0,1). Suppose that Y∈Hlωα−1,0(Λ)⋂Hlw−1/2,−1/2(Λ) with 1≤l≤N+1, we conclude the following error estimate:
where
Proof. According to (32),
By (5.1) and (5.2), we deduce that
The Cauchy-Schwarz inequality, along with Lemmas 5.1 and 5.3, lead to
where
Similarly, by Lemma 5.1 and the Cauchy-Schwarz inequality, we obtain
Using the Lemma 5.3 yields
By Lemma 5.1,
We obtain from the Cauchy-Schwarz inequality that
where
By Lemma 5.1 and 5.3,
We further get from the Cauchy-Schwarz inequality that
It follows from Lemma 5.3 that
In summary, we get
The theorem is proved.
6.
Numerical tests
In this section, we give numerical examples to illustrate the feasibility and efficiency of the method.
6.1. Example 1
We consider the following fractional integro-differential equation:
The exact solution is t23.
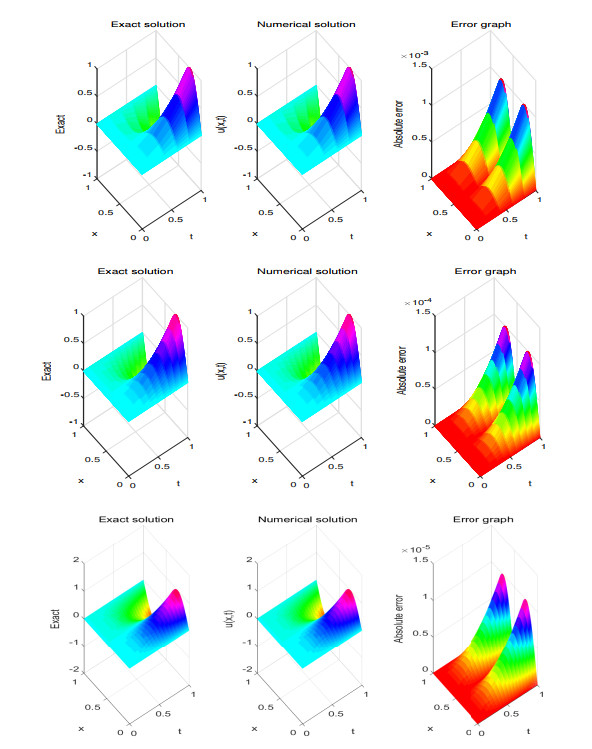
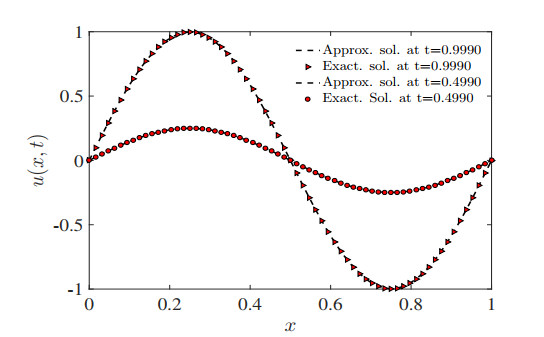
In the table 1, we list L∞- and L2ωα−1,0-error with N=10 and σ takes values of 1-9. As can be seen from the table, for σ takes values of 3, 6 and 9, which are multiples of 3, the error decreases faster and the convergence is better. Through a lot of numerical experiments, we conclude that not for all σ taking multiple value of 3, the convergence is better. One cannot predict that the greater the value of σ is, the better the convergence is. There is a critical point, which is the value of 27. For σ takes values of 2 to 27, the error convergence is better than that without smoothing transformation. To be more intuitive, we give a comparison of the exact solution and numerical solution with N=10 and σ=1, 3, 6, and 9 in Figures 1 and 2. And in Figure 3, we give L∞- and L2ωα−1,0-error between the exact solution and the numerical solution with N=10 and σ=1 to 9 and 28. Obviously, the error deceases faster when the value of 3, 6 and 9, which is the multiple of 3. Moreover, at the value of 28, the error convergence effect does not work well without smoothing transformation.
6.2. Example 2
Consider
where f(t)=√π2BesselJ[0,√t]−t(−2(−6+t)√tcos√t+6(−2+t)sin√t).
The exact solution is sin√t.
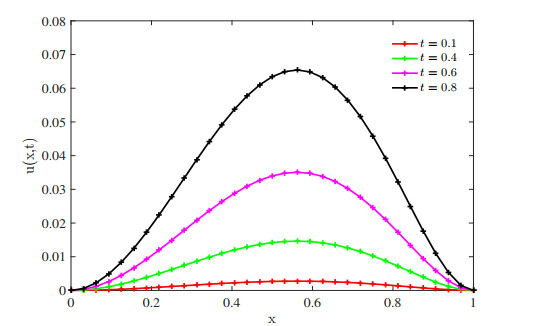
We list the L∞- and L2ωα−1,0-error with N=10 and σ takes values of 1-6 in Table 2. From the table, we can also see that when σ takes values of 2 and 4, which is the multiple of 2, the error decreases faster. And through a large number of numerical experiments, we get a critical point of σ, which is the value of 14. When σ takes values of 2-13, the error convergence is better than that without smoothing transformation. For the sake of intuition, we give a comparison when N=10 and σ takes values of 1, 2 and 4 in Figure 4. In Figures 5, the L∞- and L2ωα−1,0-error between the exact solution and the numerical solution are given when N=10 and σ takes values 1-6 and 14. It clear that when σ takes values of 2 and 4, which are multiples of 2, the error is smaller. While σ takes the value of 14, the error is worse than that without smoothing transformation.
6.3. Example 3
Consider the following equation
where f(t)=t4(2et(3−2t)√t−3√πErfi[√t]+3√πt14Γ(94)).
The exact solution is y(t)=t√t.
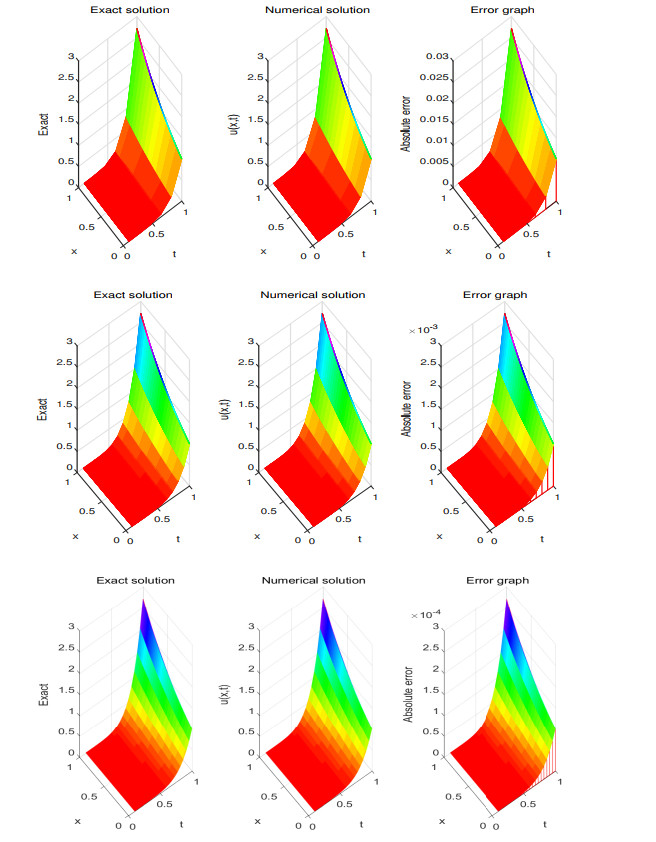
In Table 3, We list the L∞- and L2ωα−1,0-error when N=10 and σ takes values of 1-6. From the table, when σ takes the value of 4, which is the multiple of 4, the error decreases faster and the convergence is better. Through a lot of numerical experiments, we derive a critical point of the σ, which is the value of 8. When σ takes values of 2-7, the error is better than the situation that the smoothing transformation is not performed. We give a comparison when N=10 and σ takes values of 1, 2 and 4 in Figures 6. In Figure 7, the L∞- and L2ωα−1,0-error are given when N=10 and σ takes values of 1-6 and 8. Obviously, when σ takes multiples of 4, the error decreases faster. However, when σ takes the value of 8, the error is not good without smoothing transformation.
7.
Conclusion
In this paper, we first study in detail the reason why the fractional integro-differential equation has non-smooth solution. We eliminate the singularity of the solution by introducing a smooth transformation. Then we use the Jacobi spectral-collocation method with global and high precision characteristics to solve the transformed equation. Particularly, we have proved that the convergence rate for non-smooth solutions can be enhanced by using a suitable smoothing transformation, which allows us to adjust a parameter in the solution in view of a priori known regularity of the given data. The proposed scheme has many advantages, including (i) ease of implementation, (ii) lower computational cost, and (iii) exponential accuracy. In addition, we give a theoretical proof of the convergence of collocation method, in both L∞-norm and L2ωα,β-norm. Finally, we give some specific numerical examples. The numerical results confirm the validity of scheme and the correctness of the conclusions for solving the fractional integro-differential equation. This indicates that the proposed scheme possesses a good prospect in solving fractional integro-differential equations with non-smooth solutions. Next, we will apply the methods in this paper to solve the nonlinear fractional integro-differential equations.
Acknowledgments
This work was supported by National Natural Science Foundation of China Project (11671342, 11771369, and 11931003), the Project of Scientic Research Fund of the Hunan Provincial Science and Technology Department (2020JJ2027, 2018WK4006, and 2019YZ3003), and the Key Project of Hunan Provincial Department of Education (17A210).









 DownLoad:
DownLoad: