Natural convection flow and heat transfer of generalized Maxwell fluid with distributed order time fractional derivatives embedded in the porous medium

Jinhu Zhao; Jinhu Zhao

doi:10.3934/nhm.2024034

Networks and Heterogeneous Media

2024, Volume 19, Issue 2: 753-770. doi: 10.3934/nhm.2024034

Previous Article Next Article

Research article Special Issues

Natural convection flow and heat transfer of generalized Maxwell fluid with distributed order time fractional derivatives embedded in the porous medium

Jinhu Zhao ^,

School of Mathematics and Statistics, Fuyang Normal University, Fuyang 236037, Anhui, China

Received: 25 May 2024 Revised: 11 July 2024 Accepted: 18 July 2024 Published: 25 July 2024

Numerical simulation was performed for unsteady natural convection flow and heat transfer in a porous medium using the generalized Maxwell model and fractional Darcy's law with distributed order time fractional derivatives. The finite volume method combined with the fractional L1 scheme was used to solve strongly coupled governing equations with nonlinear fractional convection terms. Numerical solutions were validated via grid independence tests and comparisons with special exact solutions. The effects of porosity, Darcy number, and relaxation time parameters on transport fields are presented. The results illustrate that porosity and permeability have opposite influences on temperature and velocity profiles. Moreover, the relaxation time parameters have remarkable effects on velocity profiles, and the variations possess significant differences.

Keywords:

generalized Maxwell fluid,
distributed order time fractional derivatives,
porous medium,
finite volume method

Citation: Jinhu Zhao. Natural convection flow and heat transfer of generalized Maxwell fluid with distributed order time fractional derivatives embedded in the porous medium[J]. Networks and Heterogeneous Media, 2024, 19(2): 753-770. doi: 10.3934/nhm.2024034

Related Papers:

[1]	María Anguiano, Renata Bunoiu . Homogenization of Bingham flow in thin porous media. Networks and Heterogeneous Media, 2020, 15(1): 87-110. doi: 10.3934/nhm.2020004
[2]	Leda Bucciantini, Angiolo Farina, Antonio Fasano . Flows in porous media with erosion of the solid matrix. Networks and Heterogeneous Media, 2010, 5(1): 63-95. doi: 10.3934/nhm.2010.5.63
[3]	Verónica Anaya, Mostafa Bendahmane, David Mora, Ricardo Ruiz Baier . On a vorticity-based formulation for reaction-diffusion-Brinkman systems. Networks and Heterogeneous Media, 2018, 13(1): 69-94. doi: 10.3934/nhm.2018004
[4]	Edoardo Mainini . On the signed porous medium flow. Networks and Heterogeneous Media, 2012, 7(3): 525-541. doi: 10.3934/nhm.2012.7.525
[5]	Steinar Evje, Kenneth H. Karlsen . Hyperbolic-elliptic models for well-reservoir flow. Networks and Heterogeneous Media, 2006, 1(4): 639-673. doi: 10.3934/nhm.2006.1.639
[6]	Andro Mikelić, Giovanna Guidoboni, Sunčica Čanić . Fluid-structure interaction in a pre-stressed tube with thick elastic walls I: the stationary Stokes problem. Networks and Heterogeneous Media, 2007, 2(3): 397-423. doi: 10.3934/nhm.2007.2.397
[7]	Laura Cattaneo, Paolo Zunino . Computational models for fluid exchange between microcirculation and tissue interstitium. Networks and Heterogeneous Media, 2014, 9(1): 135-159. doi: 10.3934/nhm.2014.9.135
[8]	Michael Eden, Michael Böhm . Homogenization of a poro-elasticity model coupled with diffusive transport and a first order reaction for concrete. Networks and Heterogeneous Media, 2014, 9(4): 599-615. doi: 10.3934/nhm.2014.9.599
[9]	Catherine Choquet, Ali Sili . Homogenization of a model of displacement with unbounded viscosity. Networks and Heterogeneous Media, 2009, 4(4): 649-666. doi: 10.3934/nhm.2009.4.649
[10]	Jean-Marc Hérard, Olivier Hurisse . Some attempts to couple distinct fluid models. Networks and Heterogeneous Media, 2010, 5(3): 649-660. doi: 10.3934/nhm.2010.5.649

Abstract

1. Introduction

Transport phenomena in porous media have widespread applications throughout manifold aspects of nature and industrial engineering, such as groundwater utilization, oil and gas extraction, chemical industry, building materials, biomedical development, etc. ^[1,2]. The relevant studies on heat transfer theory also permeate many scientific and technical fields ^[3]. On account of the structural complexity inside porous media and the diversity of fluids, convective flow and heat transfer in porous media have strongly nonlinear characteristics, and the classical linear Darcy's law is not applicable. Moreover, the coupled effects of velocity and temperature fields have important influences on the heat transfer process. Because of this, a novel constitutive equation and heat transfer model should be derived to characterize and simulate the internal physical mechanism inside the porous medium.

For the last few years, constitutive relationship equations with fractional derivatives have been frequently adopted in the description of complex dynamics ^[4,5,6,7], and simultaneously relevant research on fractional porous media has also been carried out widely. Wei et al. ^[8] proposed two fractional derivative models to describe non-Darcy flow in porous media. Yang et al. ^[9] discussed anomalous imbibition of non-Newtonian fluid in a porous medium by a spatiotemporal fractional model. Ahmed ^[10] used Caputo fractional derivatives to govern the mixed convective flow of hybrid nanofluids together with the Darcy model. Ai et al. ^[11] presented a viscoelastic model with fractional derivatives to simulate multilayered cross-anisotropic porous media. Jiang et al. ^[12] solved the governing equations of fractional second-grade fluid through a porous medium. Jiang et al. ^[13] developed a fractional Burgers model to simulate non-Newtonian fluid in porous media with complex physical-chemical conditions.

Nevertheless, the restrictions of the mentioned research are fixed fractional derivative parameters that manifest a rather limited class of memory characteristics and nonlocal properties ^[14]. Recent developments in various fields, such as thermal management, manufacturing, blood flow, etc., emphasize the need to resolve the limitations of conventional models. The convective flow and heat transfer problems of viscoelastic fluids involve complex molecule internal structures and multi-scale effects. Therefore, the distributed order fractional derivative that was proposed by Caputo to develop the fractional derivative models ^[15] has been frequently applied to boundary layer flow and heat transfer in view of the advantage of integrating over a given range within a continuum. Liu et al. ^[16] utilized distributed order fractional derivatives to investigate boundary layer flow and heat transfer through a moving plate. Qiao et al. ^[17] solved the pipe flow governing equations of viscoelastic Maxwell fluids with distributed order time fractional derivatives. Long et al. ^[18] developed the generalized Maxwell and Cattaneo model with distributed order time fractional derivative to study unsteady Marangoni convective boundary layer flow and heat transfer. Yang et al. ^[19] discussed boundary layer flow and heat transfer by way of the space distributed-order constitution relationship. Feng et al. ^[20] investigated anomalous transport in a binary medium on finite domains and analyzed the properties of the distributed-order space fractional derivatives with nonlocal operators. Chen et al. ^[21] presented a study on start-up pipe flow with double time-distributed order Maxwell model. Niu et al. ^[22] proposed a fast numerical algorithm for the fractional Sobolev model, with non-smooth solutions for the nonlinear distributed-order equations in porous media. Liu et al. ^[23] constructed a novel distributed order time fractional model to solve heat conduction, anomalous diffusion, and viscoelastic flow problems. Hu et al. ^[24] applied a distributed order time fractional Maxwell constitutive fluid model to simulate viscoelastic blood flowing in a narrowing blood vessel with a uniform magnetic field. Zhang et al. ^[25] introduced the distributed-order time fractional derivative to establish the generalized fractional Bloch-Torrey equation in heterogeneous biological tissues.

On the other hand, there are very few studies on natural convective flow and heat transfer in porous media with distributed order time fractional derivatives. This is because the momentum and energy governing equations are strongly coupled, and the analytical solutions are difficult to obtain for the nonlinear fractional convection terms. Given this, the paper aims to further discuss the internal transport mechanism in natural convective flow and heat transfer of porous media within distributed order time fractional derivative models. The following Section 2 presents unsteady natural convective flow and heat transfer governing equations using the generalized Maxwell model and Darcy's law with initial and boundary conditions. Section 3 presents the fractional finite volume method together with the fractional L1 scheme to solve the nonlinear governing equations. A mid-point quadrature rule is employed to discretize the distributed order time fractional derivatives. Section 4 validates the acceptable accuracy of the numerical solutions after the grid independence test and comparisons with exact solutions. Section 5 analyzes and discusses the effects of active physical parameters on velocity and temperature distributions in detail. Section 6 summarizes the main conclusions of this paper.

2. Mathematical formulation

Consider unsteady convective flow and heat transfer of viscoelastic fluid over a vertical plate embedded in a porous medium. The wall temperature is denoted as T_w and is always maintained as a constant. The ambient temperature far away from the plate is T_∞. Particularly, the direction of the vertical plate is selected as the x-axis, while the y-axis is horizontal accordingly. It is assumed that the fluid is incompressible, and the Boussinesq approximation can apply to the external body force. The porous medium is also assumed to be isotropic and homogeneous. Under these assumptions, the momentum conservation equation in the porous medium follows

$\rho \left( {\frac{{\partial u}}{{\partial t}} + u\frac{{\partial u}}{{\partial x}} + v\frac{{\partial u}}{{\partial y}}} \right) = \frac{{\partial {\sigma _{xy}}}}{{\partial y}} + g{\beta _T}\left( {T - {T_\infty }} \right) + R ,$

(2.1)

where ρ is the fluid constant density, u and v are the corresponding velocity components, σ_xy is the shear stress tensor component, g is the acceleration due to gravity, β_T is the thermal expansion coefficient, T is the temperature, and R is the Darcy resistance.

The microscopic structure of the porous medium and associated environments introduce complexity via porosity and permeability. The distributed order fractional derivative provides more flexibility for simulating anomalous diffusion behaviors with multi-scale characteristics ^[26]. Thus, the generalized distributed order Maxwell model and Darcy's law are developed by integrating the order of the time-fractional derivative, respectively:

${\sigma _{xy}} + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }{\sigma _{xy}}}}{{\partial {t^\alpha }}}d\alpha = \mu \frac{{\partial u}}{{\partial y}} ,$

(2.2)

$R + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }R}}{{\partial {t^\alpha }}}d\alpha = - \frac{{\mu \varepsilon }}{K}u ,$

(2.3)

where α is the velocity fractional derivative parameter that characterizes the frequency-dependent complex modulus, λ₁ is the velocity relaxation time, ω₁(α) is the weight coefficient that follows ω₁(α) ≥ 0 and $\int_0^1 {{\omega _1}(\alpha)d\alpha = 1}$ ^[27], μ is the dynamic viscosity of the fluid, Κ and ε are the permeability and porosity of the porous medium, respectively, and $\frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}$ denotes the Caputo fractional derivative operator defined as in (2.4) ^[28].

$\frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}f(x, y, t) = \frac{1}{{\Gamma \left( {m - \alpha } \right)}}\int_0^t {{{\left( {t - \eta } \right)}^{m - \alpha - 1}}} \frac{{\partial {f^m}(x, y, \eta )}}{{\partial {\eta ^m}}}d\eta , m - 1 < \alpha < m ,$

(2.4)

where m∈ℕ⁺, Γ(∙) denotes the gamma function, and f(x, y, t) is simplified as f(t). Combining Eqs (2.1)-(2.3) to eliminate the terms σ_xy and R, the nonlinear coupled momentum equation with distributed order fractional derivative is derived as follows:

$\begin{array}{l} \frac{{\partial u}}{{\partial t}} + \frac{\partial }{{\partial x}}\left( {uu} \right) + \frac{\partial }{{\partial y}}\left( {vu} \right) + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^{\alpha + 1}}u}}{{\partial {t^{\alpha + 1}}}}d\alpha + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left( {\frac{\partial }{{\partial x}}\left( {uu} \right)} \right)d\alpha \hfill \\ + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left( {\frac{\partial }{{\partial y}}\left( {vu} \right)} \right)d\alpha = - \frac{{{\nu _f}\varepsilon }}{K}u + {\nu _f}\frac{{{\partial ^2}u}}{{\partial {y^2}}} + g{\beta _T}\left( {T - {T_\infty }} \right) \hfill \\ + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left[ {g{\beta _T}\left( {T - {T_\infty }} \right)} \right]d\alpha . \hfill \\ \end{array}$

(2.5)

Similarly, the homologous energy equation with distributed order fractional derivative is obtained in the following:

$\begin{array}{l} \frac{{\partial T}}{{\partial t}} + \frac{\partial }{{\partial x}}\left( {uT} \right) + \frac{\partial }{{\partial y}}\left( {vT} \right) + \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^{\beta + 1}}T}}{{\partial {t^{\beta + 1}}}}d\beta + \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial x}}\left( {uT} \right)} \right)d\beta \hfill \\ + \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial y}}\left( {vT} \right)} \right)d\beta = {\alpha _f}\frac{{{\partial ^2}T}}{{\partial {y^2}}}, \hfill \\ \end{array}$

(2.6)

where ω₂(β) denotes the weight coefficient that is suitable for certain conditions as ω₂(β) ≥ 0 and $\int_0^1 {{\omega _2}(\beta)d\beta = 1}$ , β is the temperature fractional derivative parameter that improves the efficiency of the thermoelectric material figure-of-merit, λ₂ is the temperature relaxation time, and α_f is the thermal diffusion coefficient.

The initial conditions and boundary conditions of the natural convective flow in the porous medium are assumed as follows:

$u\left( {x, y, 0} \right) = 0 , \frac{{\partial u\left( {x, y, 0} \right)}}{{\partial t}} = 0 , v\left( {x, y, 0} \right) = 0 , T\left( {x, y, 0} \right) = \left\{ \begin{array}{l} {T_w}, y = 0 \hfill \\ {T_\infty }, y \ne 0 \hfill \\ \end{array} \right. , \frac{{\partial T\left( {x, y, 0} \right)}}{{\partial t}} = 0 , \\ u\left( {x, 0, t} \right) = 0 , v\left( {x, 0, t} \right) = 0 , T\left( {x, 0, t} \right) = {T_w} , u\left( {0, y, t} \right) = 0 , T\left( {0, y, t} \right) = {T_\infty } , u\left( {x, \infty , t} \right) = 0 , T\left( {x, \infty , t} \right) = {T_\infty } .$

We employ non-dimensional quantities as follows:

${x^ * } = \frac{x}{L} , {y^ * } = \frac{y}{L}G{r^{1/4}} , {u^ * } = \frac{{uL}}{{{\nu _f}}}G{r^{ - 1/2}} , {v^ * } = \frac{{vL}}{{{\nu _f}}}G{r^{ - 1/4}} , {t^ * } = \frac{{{\nu _f}t}}{{{L^2}}}G{r^{1/2}} , \lambda _1^* = \frac{{{\lambda _1}{\nu _f}}}{{{L^2}}}G{r^{1/2}} , \\ \lambda _2^* = \frac{{{\lambda _2}{\nu _f}}}{{{L^2}}}G{r^{1/2}} , Da = \frac{K}{{{L^2}}} , Gr = \frac{{g{\beta _T}\left( {{T_w} - {T_\infty }} \right){L^3}}}{{\nu _f^2}} , \theta = \frac{{T - {T_\infty }}}{{{T_w} - {T_\infty }}} , Pr = \frac{{{\nu _f}}}{{{\alpha _f}}} ,$

where L is the total length of the plate, Da is the Darcy number, Gr is the Grashof number, and Pr is the Prandtl number.

Non-dimensional distributed order governing equations for the porous medium are derived with time-fractional derivatives after omitting the dimensionless sign "*":

$\frac{{\partial u}}{{\partial x}} + \frac{{\partial v}}{{\partial y}} = 0,$

(2.7)

$\begin{array}{l} \frac{{\partial u}}{{\partial t}} + \frac{\partial }{{\partial x}}\left( {uu} \right) + \frac{\partial }{{\partial y}}\left( {vu} \right) + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^{\alpha + 1}}u}}{{\partial {t^{\alpha + 1}}}}d\alpha + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left( {\frac{\partial }{{\partial x}}\left( {uu} \right)} \right)d\alpha \hfill \\ + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left( {\frac{\partial }{{\partial y}}\left( {vu} \right)} \right)d\alpha = - \frac{{\varepsilon u}}{{DaG{r^{1/2}}}} + \frac{{{\partial ^2}u}}{{\partial {y^2}}} + \theta + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }\theta }}{{\partial {t^\alpha }}}d\alpha , \hfill \\ \end{array}$

(2.8)

$\begin{array}{l} \frac{{\partial \theta }}{{\partial t}} + \frac{\partial }{{\partial x}}\left( {u\theta } \right) + \frac{\partial }{{\partial y}}\left( {v\theta } \right) + \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^{\beta + 1}}\theta }}{{\partial {t^{\beta + 1}}}}d\beta + \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} \right)d\beta \hfill \\ + \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} \right)d\beta = \frac{1}{{Pr}}\frac{{{\partial ^2}\theta }}{{\partial {y^2}}}. \hfill \\ \end{array}$

(2.9)

The non-dimensional initial conditions and boundary conditions are subject to

$u\left( {x, y, 0} \right) = 0 , \frac{{\partial u\left( {x, y, 0} \right)}}{{\partial t}} = 0 , v\left( {x, y, 0} \right) = 0 , \theta \left( {x, y, 0} \right) = \left\{ \begin{array}{l} 1, y = 0 \hfill \\ 0, y \ne 0 \hfill \\ \end{array} \right. , \frac{{\partial \theta \left( {x, y, 0} \right)}}{{\partial t}} = 0 , \\ u\left( {x, 0, t} \right) = 0 , v\left( {x, 0, t} \right) = 0 , \theta \left( {x, 0, t} \right) = 1 , u\left( {0, y, t} \right) = 0 , \theta \left( {0, y, t} \right) = 0 , u\left( {x, \infty , t} \right) = 0 , \theta \left( {x, \infty , t} \right) = 0 .$

3. Numerical technique

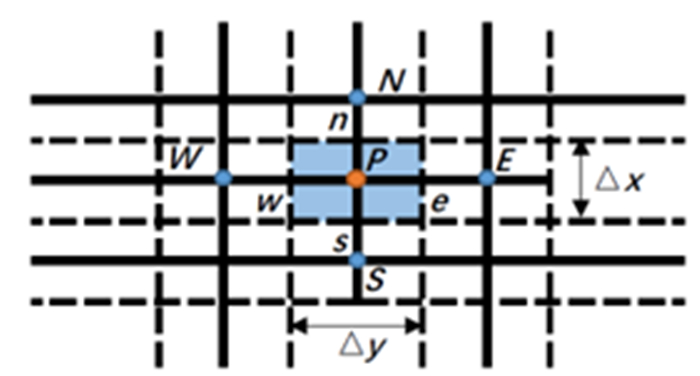
Figure 1 shows the collocated grid system of the physical problem, where the computational domain is divided into discrete control volumes, and the general central nodal point is denoted as ΡP. The grid distances between the inner nodes in the x and y directions are denoted as Δx and Δy, respectively. The time step is denoted as Δt.

Figure 1. The grid system of the control volume.

DownLoad: Full-Size Img PowerPoint

First, the following integral equation is derived by the integration of Eq (2.9):

$\begin{array}{l} \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{{\partial \theta }}{{\partial t}}} } dVdt + \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} } dVdt + \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} } dVdt \hfill \\ + \int_t^{t + \Delta t} {\int_{\Delta V} {\int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^{\beta + 1}}\theta }}{{\partial {t^{\beta + 1}}}}d\beta } } dVdt + \int_t^{t + \Delta t} {\int_{\Delta V} {\int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} \right)d\beta } } dVdt \hfill \\ + \int_t^{t + \Delta t} {\int_{\Delta V} {\int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} \right)d\beta } } dVdt = \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{1}{{Pr}}\frac{{{\partial ^2}\theta }}{{\partial {y^2}}}} } dVdt, \hfill \\ \end{array}$

(3.1)

where ΔV is the control volume that equals Δx·Δy.

The backward difference scheme is implemented to discretize the first item of the time term in Eq (3.1) as follows:

$\int_t^{t + \Delta t} {\int_{\Delta V} {\frac{{\partial \theta }}{{\partial t}}} } dVdt = \left( {\theta _P^k - \theta _P^{k - 1}} \right) \cdot \Delta V + O(\Delta t) .$

(3.2)

The volume integrals inside the convective terms and diffusive term are substituted by surface integrals, which are carried out by the first-order upwind difference scheme. In addition, the gradient at the interface is approximated by the central difference format. After these methods of processing, the following discretized equations of integer derivatives are formulated:

$\begin{array}{l} \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} } dVdt = \left[ {\left( {uA\theta } \right)_n^k - \left( {uA\theta } \right)_s^k} \right] \cdot \Delta t \hfill \\ = \left( {u_P^{k - 1}{A_n}\theta _P^k - u_S^{k - 1}{A_s}\theta _S^k} \right) \cdot \Delta t + O(\Delta x), \hfill \\ \end{array}$

(3.3)

$\begin{array}{l} \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} } dVdt = \left[ {\left( {vA\theta } \right)_e^k - \left( {vA\theta } \right)_w^k} \right] \cdot \Delta t \hfill \\ = \left( {v_E^{k - 1}{A_e}\theta _E^k - v_P^{k - 1}{A_w}\theta _P^k} \right) \cdot \Delta t + O(\Delta y), \hfill \\ \end{array}$

(3.4)

$\begin{array}{l} \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{{{\partial ^2}\theta }}{{\partial {y^2}}}} } dVdt = \left[ {\left( {A\frac{{\partial \theta }}{{\partial y}}} \right)_e^k - \left( {A\frac{{\partial \theta }}{{\partial y}}} \right)_w^k} \right] \cdot \Delta t \hfill \\ = \left( {{A_e}\frac{{\theta _E^k - \theta _P^k}}{{\Delta y}} - {A_w}\frac{{\theta _P^k - \theta _W^k}}{{\Delta y}}} \right) \cdot \Delta t + O(\Delta {y^2}), \hfill \\ \end{array}$

(3.5)

where A represents the face area of the control volume, and A_w = A_e = Δx, A_n = A_s = Δy.

Second, the mid-point quadrature rule is adopted to approximate the distributed order time fractional derivatives. Distributed-order fractional partial differential equations (PDEs) can be regarded as the limiting case of multi-term fractional PDEs ^[29]. Diethelm and Ford ^[30] have observed that small changes in the order of a fractional PDE lead to only slight changes in the final solution, which gives initial support to the employed numerical integration method. Thus, the summations of multi-fractional terms are acquired ^[31]:

$\int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^{\beta + 1}}\theta }}{{\partial {t^{\beta + 1}}}}d\beta \approx {h_\beta }\sum\limits_{s = 1}^K {{\omega _2}({\beta _s})\lambda _2^{{\beta _s}}} \frac{{{\partial ^{{\beta _s} + 1}}\theta }}{{\partial {t^{{\beta _s} + 1}}}} ,$

(3.6)

$\int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} \right)d\beta \approx {h_\beta }\sum\limits_{s = 1}^K {{\omega _2}({\beta _s})} \lambda _2^{{\beta _s}}\frac{{{\partial ^{{\beta _s}}}}}{{\partial {t^{{\beta _s}}}}}\left( {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} \right) ,$

(3.7)

$\int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} \right)d\beta \approx {h_\beta }\sum\limits_{s = 1}^K {{\omega _2}({\beta _s})\lambda _2^{{\beta _s}}} \frac{{{\partial ^{{\beta _s}}}}}{{\partial {t^{{\beta _s}}}}}\left( {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} \right) ,$

(3.8)

where ${h_\beta } = \frac{1}{K}$ denotes the fractional parameter step, and ${\beta _s} = \frac{{\left({s - 1} \right){h_\beta } + s{h_\beta }}}{2}$ .

The fractional L1 scheme is adopted to estimate the time-fractional derivative of order β_s, as 0 < β_s < 1 ^[32,33] under the assumption that f (t_k) ∈ C²[0, t]:

$\frac{{{\partial ^{{\beta _s}}}}}{{\partial {t^{{\beta _s}}}}}f({t_k}) = \frac{{\Delta {t^{ - {\beta _s}}}}}{{\Gamma \left( {2 - {\beta _s}} \right)}}\left[ {f({t_k}) - \beta _{k - 1}^sf\left( {{t_0}} \right) - \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)f({t_{k - j}})} } \right] + O\left( {\Delta {t^{2 - {\beta _s}}}} \right) ,$

(3.9)

where $\beta _j^s = {\left({j + 1} \right)^{1 - {\beta _s}}} - {j^{1 - {\beta _s}}}$ . For the order of β_s+1, the Caputo time fractional derivative has the following property ^[28]:

$\frac{{{\partial ^{{\beta _s} + 1}}}}{{\partial {t^{{\beta _s} + 1}}}}f({t_k}) = \frac{{{\partial ^{{\beta _s}}}}}{{\partial {t^{{\beta _s}}}}}f'({t_k}) ,$

(3.10)

where $f'({t_k}) = \left[{f({t_k})- f({t_{k- 1}})} \right]/\Delta t$ .

Utilizing Eqs (3.9) and (3.10), Eqs (3.6)-(3.8) can be transformed into the discretized forms

$\begin{array}{l} \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^{\beta + 1}}\theta }}{{\partial {t^{\beta + 1}}}}d\beta \hfill \\ = {h_\beta }\Delta V\sum\limits_{s = 1}^K {{\omega _2}({\beta _s})\frac{{\lambda _2^{{\beta _s}} \cdot \Delta {t^{ - {\beta _s}}}}}{{\Gamma \left( {2 - {\beta _s}} \right)}}} \left[ {\theta _P^k - \theta _P^{k - 1} - \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)\left( {\theta _P^{k - j} - \theta _P^{k - j - 1}} \right)} } \right] + {R_1}, \hfill \\ \end{array}$

(3.11)

$\begin{array}{l} \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial x}}\left( {u\theta } \right)} \right)d\beta = {h_\beta }\sum\limits_{s = 1}^K {{\omega _2}({\beta _s})} \frac{{\lambda _2^{{\beta _s}} \cdot \Delta {t^{ - {\beta _s} + 1}}}}{{\Gamma \left( {2 - {\beta _s}} \right)}} \cdot \hfill \\ \left[ {u_P^{k - 1}{A_n}\theta _P^k - u_S^{k - 1}{A_s}\theta _S^k - \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)\left( {u_P^{k - j - 1}{A_n}\theta _P^{k - j} - u_S^{k - j - 1}{A_s}\theta _S^{k - j}} \right)} } \right] + {R_2}, \hfill \\ \end{array}$

(3.12)

$\begin{array}{l} \int_0^1 {{\omega _2}(\beta )\lambda _2^\beta } \frac{{{\partial ^\beta }}}{{\partial {t^\beta }}}\left( {\frac{\partial }{{\partial y}}\left( {v\theta } \right)} \right)d\beta = {h_\beta }\sum\limits_{s = 1}^K {{\omega _2}({\beta _s})} \frac{{\lambda _2^{{\beta _s}} \cdot \Delta {t^{ - {\beta _s} + 1}}}}{{\Gamma \left( {2 - {\beta _s}} \right)}} \cdot \hfill \\ \left[ {v_E^{k - 1}{A_e}\theta _E^k - v_P^{k - 1}{A_w}\theta _P^k - \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)\left( {v_E^{k - j - 1}{A_e}\theta _E^{k - j} - v_P^{k - j - 1}{A_w}\theta _P^{k - j}} \right)} } \right] + {R_3}, \hfill \\ \end{array}$

(3.14)

where the truncation errors are ${R_1} \leqslant C(\Delta t)$ , ${R_2} \leqslant C(\Delta t + \Delta x)$ , ${R_3} \leqslant C(\Delta t + \Delta y)$ .

Finally, the iterative difference equation of Eq (2.9) is established:

${a_P}\theta _P^k = {a_S}\theta _S^k + {a_W}\theta _W^k + {a_E}\theta _E^k + {S_P} ,$

(3.15)

${a_P} = \left( {1 + \sum\limits_{s = 1}^K {{r_s}} } \right) \cdot \left( {\frac{{\Delta V}}{{\Delta t}} + \Delta y \cdot u_P^{k - 1} - \Delta x \cdot v_P^{k - 1}} \right) + \frac{{2\Delta x}}{{Pr \cdot \Delta y}} ,$

${a_S} = \left( {1 + \sum\limits_{s = 1}^K {{r_s}} } \right) \cdot \Delta y \cdot u_S^{k - 1} \text{, } {a_w} = \frac{{\Delta x}}{{Pr \cdot \Delta y}} \text{, } {a_E} = \frac{{\Delta x}}{{Pr \cdot \Delta y}} - \left( {1 + \sum\limits_{s = 1}^K {{r_s}} } \right) \cdot \Delta x \cdot v_E^{k - 1} \text{, }$

$\begin{array}{l} {S_P} = \left( {1 + \sum\limits_{s = 1}^K {{r_s}} } \right) \cdot \frac{{\Delta V}}{{\Delta t}} \cdot \theta _P^{k - 1} + \frac{{\Delta V}}{{\Delta t}} \cdot \sum\limits_{s = 1}^K {{r_s}} \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)\left( {\theta _P^{k - j} - \theta _P^{k - j - 1}} \right)} \hfill \\ + \Delta y \cdot \sum\limits_{s = 1}^K {{r_s}} \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)\left( {u_P^{k - j - 1}\theta _P^{k - j} - u_S^{k - j - 1}\theta _S^{k - j}} \right)} \hfill \\ + \Delta x \cdot \sum\limits_{s = 1}^K {{r_s}} \sum\limits_{j = 1}^{k - 1} {\left( {\beta _{j - 1}^s - \beta _j^s} \right)\left( {v_E^{k - j - 1}\theta _E^{k - j} - v_P^{k - j - 1}\theta _P^{k - j}} \right), } \hfill \\ \end{array}$

where ${r_s} = {h_\beta }{\omega _2}\left({{\beta _s}} \right)\frac{{\lambda _2^{{\beta _s}} \cdot \Delta {t^{ - {\beta _s}}}}}{{\Gamma \left({2 - {\beta _s}} \right)}}$ .

The velocity terms of Eq (2.8) are carried out to deduce the discretized forms similarly. The semi-implicit scheme is utilized for the integration of the single velocity and temperature terms:

$\int_t^{t + \Delta t} {\int_{\Delta V} u } dVdt = \frac{{\Delta t \cdot \Delta V}}{2}\left( {u_P^k + u_P^{k - 1}} \right) + O(\Delta t) ,$

(3.16)

$\int_t^{t + \Delta t} {\int_{\Delta V} \theta } dVdt = \frac{{\Delta t \cdot \Delta V}}{2}\left( {\theta _P^k + \theta _P^{k - 1}} \right) + O(\Delta t) ,$

(3.17)

$\begin{array}{l} \int_t^{t + \Delta t} {\int_{\Delta V} {\int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }\theta }}{{\partial {t^\alpha }}}d\alpha } } dVdt \approx \frac{{{h_\alpha }\Delta V}}{2}\sum\limits_{s = 1}^K {{\omega _1}({\alpha _s})} \frac{{\lambda _1^{{\alpha _s}} \cdot \Delta {t^{ - {\alpha _s} + 1}}}}{{\Gamma \left( {2 - {\alpha _s}} \right)}} \cdot \hfill \\ \left[ {\theta _P^k + \theta _P^{k - 1} - \sum\limits_{j = 1}^{k - 1} {\left( {\alpha _{j - 1}^s - \alpha _j^s} \right)\left( {\theta _P^{k - j} + \theta _P^{k - j - 1}} \right)} } \right] + O(\Delta t), \hfill \\ \end{array}$

(3.18)

where ${h_\alpha } = \frac{1}{K}$ , ${\alpha _s} = \frac{{\left({s - 1} \right){h_\alpha } + s{h_\alpha }}}{2}$ , $\alpha _j^s = {\left({j + 1} \right)^{1 - {\alpha _s}}} - {j^{1 - {\alpha _s}}}$ .

Meanwhile, Eq (2.7) is integrated to obtain the velocity component in the y direction as follows:

$\begin{array}{l} 0 = \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{{\partial u}}{{\partial x}}{\text{ }}} } dVdt + \int_t^{t + \Delta t} {\int_{\Delta V} {\frac{{\partial v}}{{\partial y}}} } {\text{ }}dVdt \hfill \\ = \frac{{\Delta t}}{2}\left[ {\left( {uA} \right)_n^k - \left( {uA} \right)_s^k + \left( {uA} \right)_n^{k - 1} - \left( {uA} \right)_s^{k - 1}} \right] + \frac{{\Delta t}}{2}\left[ {\left( {vA} \right)_e^k - \left( {vA} \right)_w^k + \left( {vA} \right)_e^{k - 1} - \left( {vA} \right)_w^{k - 1}} \right], \hfill \\ \end{array}$

(3.19)

$v_P^k = - v_P^{k - 1} + v_W^k + v_W^{k - 1} - \frac{{\Delta y}}{{\Delta x}}\left( {u_P^k - u_S^k + u_P^{k - 1} - u_S^{k - 1}} \right) + O\left( {\Delta x + \Delta y} \right).$

(3.20)

4. Validation of the numerical results

4.1. Solvability of the iterative difference equation

Theorem 1. The iterative difference equation (3.14) is uniquely solvable.

Proof. The values of θ at the kth time level are acquired from the iterative difference equation (3.14). We summarize the difference scheme on a particular i-level in matrix form:

${G_i}\theta _i^k = {H_i}\theta _i^{k - 1} + S_i^k ,$

(4.1)

$\theta _i^k = {\left[ {\theta _{i, 2}^k, \theta _{i, 3}^k, \cdots , \theta _{i, j}^k, \cdots , \theta _{i, N - 1}^k} \right]^T} , \theta _i^{k - 1} = {\left[ {\theta _{i, 2}^{k - 1}, \theta _{i, 3}^{k - 1}, \cdots , \theta _{i, j}^{k - 1}, \cdots , \theta _{i, N - 1}^{k - 1}} \right]^T} ,$

${G_i} = \left( {\begin{array}{*{20}{c}} {{a_P}\left( {i, 2} \right)} \\ 0 \\ 0 \end{array}{\text{ }}\begin{array}{*{20}{c}} { - {a_E}\left( {i, 3} \right)} \\ \cdots \\ \ldots \end{array}{\text{ }}\begin{array}{*{20}{c}} \ldots \\ { - {a_w}\left( {i, j - 1} \right)} \\ \ldots \end{array}{\text{ }}\begin{array}{*{20}{c}} \ldots \\ {{a_P}\left( {i, j} \right)} \\ \ldots \end{array}{\text{ }}\begin{array}{*{20}{c}} \ldots \\ { - {a_E}\left( {i, j + 1} \right)} \\ \ldots \end{array}{\text{ }}\begin{array}{*{20}{c}} \ldots \\ \cdots \\ { - {a_W}\left( {i, N - 2} \right)} \end{array}{\text{ }}\begin{array}{*{20}{c}} 0 \\ 0 \\ {{a_P}\left( {i, N - 1} \right)} \end{array}} \right) ,$

${H_i} = {\left[ {{a_s}\left( {i, 2} \right), {a_s}\left( {i, 3} \right), \cdots , {a_s}\left( {i, j} \right), \cdots , {a_s}\left( {i, N - 1} \right)} \right]^T} ,$

$S_i^k = {\left[ {{S_P}\left( {i, 2} \right) + {a_W}\left( {i, 2} \right)\theta _{i, 1}^k, {S_P}\left( {i, 3} \right), \cdots , {S_P}\left( {i, j} \right), \cdots , {S_P}\left( {i, N - 1} \right) + {a_E}\left( {i, N} \right)\theta _{i, N}^k} \right]^T} ,$

where G_i is a tri-diagonal and full-rank matrix.

Therefore, the difference scheme (3.20) is uniquely solvable. The numerical solutions of $u_i^k$ and $v_i^k$ are similarly proved. □

4.2. Grid independence verification

The computational domain is regarded as a rectangle with sides X_max = 1 and Y_max = 12, respectively, where Y_max represents y→∞ approximately. The coordinate mesh sizes are fixed as Δx = 0.05 and Δy = 0.1, respectively, and the time step is selected as Δt = 0.1. To compromise between numerical precision and computation time, the absolute differences between two consecutive time steps are set as smaller than 10⁻³ within the whole domain. To measure the grid independence, Figure 2 presents the numerical solutions through different groups of grid systems. The velocity curves are in good coincidence, which demonstrates that the selected mesh sizes are appropriate for the calculations.

Figure 2. Grid independence test.

DownLoad: Full-Size Img PowerPoint

4.3. Numerical test with source terms

To verify the numerical error accuracy of the presented fractional finite volume method, comparisons are implemented between numerical solutions and exact solutions with two source terms:

$\begin{array}{l} \frac{{\partial u}}{{\partial t}} + \frac{\partial }{{\partial x}}\left( {uu} \right) + \frac{\partial }{{\partial y}}\left( {vu} \right) + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^{\alpha + 1}}u}}{{\partial {t^{\alpha + 1}}}}d\alpha + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left( {\frac{\partial }{{\partial x}}\left( {uu} \right)} \right)d\alpha \hfill \\ + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }}}{{\partial {t^\alpha }}}\left( {\frac{\partial }{{\partial y}}\left( {vu} \right)} \right)d\alpha = - \frac{{\varepsilon u}}{{DaG{r^{1/2}}}} + \frac{{{\partial ^2}u}}{{\partial {y^2}}} + \theta + \int_0^1 {{\omega _1}(\alpha )\lambda _1^\alpha } \frac{{{\partial ^\alpha }\theta }}{{\partial {t^\alpha }}}d\alpha + {f_1}\left( {x, y, t} \right), \hfill \\ \end{array}$

(4.12)

(4.13)

The homogeneous initial conditions and boundary conditions are given as follows:

$u\left( {x, y, 0} \right) = 0 , \frac{{\partial u\left( {x, y, 0} \right)}}{{\partial t}} = 0 , v\left( {x, y, 0} \right) = 0 , \theta \left( {x, y, 0} \right) = 0 , \frac{{\partial \theta \left( {x, y, 0} \right)}}{{\partial t}} = 0 , u\left( {x, 0, t} \right) = 0 , v\left( {x, 0, t} \right) = 0 , \theta \left( {x, 0, t} \right) = 0 , u\left( {0, y, t} \right) = 0 , \theta \left( {0, y, t} \right) = 0 , u\left( {x, 1, t} \right) = 0 , \theta \left( {x, 1, t} \right) = 0 .$

The eligible exact solutions are chosen specially as

$u\left( {x, y, t} \right) = {x^2}{\left( {1 - x} \right)^2}{y^2}{\left( {1 - y} \right)^2}{t^2} ,$

$v\left( {x, y, t} \right) = - 2x\left( {1 - x} \right)\left( {1 - 2x} \right)\left( {\frac{1}{3}{y^3} - \frac{1}{2}{y^4} + \frac{1}{5}{y^5}} \right){t^2} ,$

$\theta \left( {x, y, t} \right) = {x^2}{\left( {1 - x} \right)^2}{y^2}{\left( {1 - y} \right)^2}{t^2} .$

The two source terms are constructed by reverse derivation:

$\begin{array}{l} {f_1}\left( {x, y, t} \right) = \hfill \\ \left[ {\int_0^1 {{\omega _1}} (\alpha )\frac{{2\lambda _1^\alpha {t^{1 - \alpha }}}}{{\Gamma \left( {2 - \alpha } \right)}}d\alpha + 2t - \int_0^1 {{\omega _1}} (\alpha )\frac{{2\lambda _1^\alpha {t^{2 - \alpha }}}}{{\Gamma \left( {3 - \alpha } \right)}}d\alpha - {t^2}} \right]{x^2}{\left( {1 - x} \right)^2}{y^2}{\left( {1 - y} \right)^2} \hfill \\ + \left( {\int_0^1 {{\omega _1}} (\alpha )\frac{{24\lambda _1^\alpha {t^{4 - \alpha }}}}{{\Gamma \left( {5 - \alpha } \right)}}d\alpha + {t^4}} \right)\left[ {{{\left( {1 - x} \right)}^3}\left( {2{x^3} - 4{x^4}} \right)\left( {{y^4} - {y^5}} \right)\left( {\frac{1}{3} - \frac{2}{3}y + \frac{3}{5}{y^2} - \frac{1}{5}{y^3}} \right)} \right] \hfill \\ - 2{x^2}{\left( {1 - x} \right)^2}\left( {1 - 6y + 6{y^2}} \right){t^2} + \frac{{\varepsilon {x^2}{{\left( {1 - x} \right)}^2}{y^2}{{\left( {1 - y} \right)}^2}{t^2}}}{{DaG{r^{1/2}}}}, \hfill \\ \end{array}$

$\begin{array}{l} {f_2}\left( {x, y, t} \right) = \hfill \\ \left[ {\int_0^1 {{\omega _2}} (\beta )\frac{{\lambda _2^\beta {t^{1 - \beta }}}}{{\Gamma \left( {2 - \beta } \right)}}d\beta + t} \right]2{x^2}{\left( {1 - x} \right)^2}{y^2}{\left( {1 - y} \right)^2} - \frac{2}{{\Pr }}{x^2}{\left( {1 - x} \right)^2}\left( {1 - 6y + 6{y^2}} \right){t^2} \hfill \\ + \left( {\int_0^1 {{\omega _2}} (\beta )\frac{{24\lambda _2^\beta {t^{4 - \beta }}}}{{\Gamma \left( {5 - \beta } \right)}}d\beta + {t^4}} \right)\left[ {{{\left( {1 - x} \right)}^3}\left( {2{x^3} - 4{x^4}} \right)\left( {{y^4} - {y^5}} \right)\left( {\frac{1}{3} - \frac{2}{3}y + \frac{3}{5}{y^2} - \frac{1}{5}{y^3}} \right)} \right]. \hfill \\ \end{array}$

Figure 3. Comparison between numerical solutions and exact solutions.

DownLoad: Full-Size Img PowerPoint

Figure 3 illustrates the comparisons between the exact solutions and numerical solutions of u and θ. The velocity and temperature distributions are both in fine consistency, which verifies that the presented fractional finite volume method has reliable convergence accuracy.

5. Results and discussion

In this section, the effects of the active physical parameters on velocity and temperature distributions are illustrated graphically: namely, the porosity, Darcy number, and the relaxation time parameters. The distributed order fractional derivatives are integrated over a given range [0, 1], and the summations of multi-fractional terms are acquired by the mid-point quadrature rule in Eqs (3.6)-(3.8). For this reason, the effects of the fractional derivative parameters are not discussed in this paper. The Prandtl number and Grashof number are both fixed as constants for brevity.

Figure 4. Temperature profiles with different ε.

DownLoad: Full-Size Img PowerPoint

Figure 5. Velocity profiles with different ε.

DownLoad: Full-Size Img PowerPoint

Figure 4 shows temperature profiles with different values of porosity. The temperature distribution is monotone decreasing for a single value of porosity. With the increase of ε, the temperature distribution increases, and the thickness of the thermal boundary layer gets bigger. The velocity profiles with different ε are depicted in Figure 5. Each velocity profile is not monotone and possesses a maximum value. As the porosity increases, the velocity distribution declines, and the velocity boundary layer becomes thinner. In addition, the point location of the maximum value gets closer to the vertical plate. These results demonstrate that larger porosity imposes negative restrictions on momentum transfer.

The effects of Darcy number on temperature and velocity profiles are illustrated in Figures 6 and 7. It is found in Figure 6 that the temperature distribution shifts down with the increase of Da, but the thickness of the temperature boundary layer declines slightly. In Figure 7, the velocity distribution rises with the increase of Da, but the increasing magnitude lessens distinctly. The result proves that the permeability accelerates the development of the velocity. It can be concluded that the porosity and permeability have opposite influences on temperature and velocity fields.

Figure 6. Temperature profiles with different Da.

DownLoad: Full-Size Img PowerPoint

Figure 7. Velocity profiles with different Da.

DownLoad: Full-Size Img PowerPoint

Figure 8. Temperature profiles with different λ₂.

DownLoad: Full-Size Img PowerPoint

Figure 9. Velocity profiles with different λ₁.

DownLoad: Full-Size Img PowerPoint

Figures 8-10 present the effects of the relaxation time λ₁ and λ₂ on velocity and temperature. It can be seen from Figure 8 that the temperature distribution decreases apparently with the increase of λ₂. In Figure 9, the velocity distribution rises as the relaxation time λ₁ increases, where the Newtonian fluid that responds to λ₁ = 0 has the smallest velocity values. The result verifies that the velocity relaxation time promotes the momentum transfer. In Figure 10, the velocity distribution descends with the augment of λ₂, which has inverse effects compared with the relaxation time λ₁. The velocity profiles stay close to each other near the plate and separate evidently at a certain distance from the plate. Meanwhile, the velocity boundary layer thickness reduces slightly with the increase of λ₂.

Figure 10. Velocity profiles with different λ₂.

DownLoad: Full-Size Img PowerPoint

6. Conclusions

This paper discussed the unsteady natural convective flow and heat transfer of generalized Maxwell viscoelastic fluids with distributed order time fractional derivatives embedded in a porous medium. The finite volume method combined with the fractional L1 scheme rule was applied to solve the nonlinear governing equations. Numerical solutions of velocity and temperature were discussed after the grid independence test and comparisons with exact solutions. Results are concluded as follows: (i). The temperature distribution rises with the increase of ε but declines as Da and λ₂ become larger. (ii). The velocity distribution ascends with the augment of Da and λ₁ but reduces with the increase of ε and λ₂.

Acknowledgments

The work is supported by the Outstanding Youth Research Project of Anhui Universities (2023AH030075), the National Natural Science Foundations of China (No. 12102093), the Natural Science Research Project of Anhui Universities (2022AH051336), the Talent Project of Fuyang Normal University (rcxm202105).

Use of AI tools declaration

The authors declare that they have not used artificial intelligence (AI) tools in the creation of this article.

Conflict of interest

The author declares that he has no conflict of interest.

References

[1]	B. Khuzhayorov, J. L. Auriault, P. Royer, Derivation of macroscopic filtration law for transient linear viscoelastic fluid flow in porous media, Int. J. Eng. Sci., 38 (2000), 487–504. https://doi.org/10.1016/S0020-7225(99)00048-8 doi: 10.1016/S0020-7225(99)00048-8
[2]	M. S. Malashetty, I. S. Shivakumara, S. Kulkarni, M. Swamy, Convective instability of Oldroyd-B fluid saturated porous layer heated from below using a thermal non-equilibrium model, Transp Porous Med, 64 (2006), 123–139. https://doi.org/10.1007/s11242-005-1893-0 doi: 10.1007/s11242-005-1893-0
[3]	I. S. Shivakumara, M. Dhananjaya, C. O Ng, Thermal convective instability in an Oldroyd-B nanofluid saturated porous layer, Int J Heat Mass Tran, 84 (2015), 167–177. https://doi.org/10.1016/j.ijheatmasstransfer.2015.01.010 doi: 10.1016/j.ijheatmasstransfer.2015.01.010
[4]	H. Xu, X. Jiang, Creep constitutive models for viscoelastic materials based on fractional derivatives, Comput. Math. Appl., 73 (2017), 1377–1384. https://doi.org/10.1016/j.camwa.2016.05.002 doi: 10.1016/j.camwa.2016.05.002
[5]	D. Yao, A fractional dashpot for nonlinear viscoelastic fluids, J. Rheol., 62 (2018), 619–629. https://doi.org/10.1122/1.5012504 doi: 10.1122/1.5012504
[6]	M. Shen, L. Chen, M. Zhang, F. Liu, A renovated Buongiorno's model for unsteady Sisko nanofluid with fractional Cattaneo heat flux, Int J Heat Mass Tran., 126 (2018), 277–286. https://doi.org/10.1016/j.ijheatmasstransfer.2018.05.131 doi: 10.1016/j.ijheatmasstransfer.2018.05.131
[7]	X. Chen, W. Yang, X. Zhang, F. Liu, Unsteady boundary layer flow of viscoelastic MHD fluid with a double fractional Maxwell model, Appl. Math. Lett., 95 (2019), 143–149. https://doi.org/10.1016/j.aml.2019.03.036 doi: 10.1016/j.aml.2019.03.036
[8]	Q. Wei, H. W. Zhou, S. Yang, Non-Darcy flow models in porous media via Atangana-Baleanu derivative, Chaos Soliton Fract, 141 (2020), 110335. https://doi.org/10.1016/j.chaos.2020.110335 doi: 10.1016/j.chaos.2020.110335
[9]	X. Yang, Y. J. Liang, W. Chen, Anomalous imbibition of non-Newtonian fluids in porous media, Chem. Eng. Sci., 211 (2020), 115265. https://doi.org/10.1016/j.ces.2019.115265 doi: 10.1016/j.ces.2019.115265
[10]	S. E. Ahmed, Caputo fractional convective flow in an inclined wavy vented cavity filled with a porous medium using Al₂O₃-Cu hybrid nanofluids, Int. Commun. Heat Mass Transf., 116 (2020), 104690. https://doi.org/10.1016/j.icheatmasstransfer.2020.104690 doi: 10.1016/j.icheatmasstransfer.2020.104690
[11]	Z. Y. Ai, Y. Z. Zhao, W. J. Liu, Fractional derivative modeling for axisymmetric consolidation of multilayered cross-anisotropic viscoelastic porous media, Comput. Math. Appl., 79 (2020), 1321–1334. https://doi.org/10.1016/j.camwa.2019.08.033 doi: 10.1016/j.camwa.2019.08.033
[12]	X. Y. Jiang, H. Zhang, S. W. Wang, Unsteady magnetohydrodynamic flow of generalized second grade fluid through porous medium with Hall effects on heat and mass transfer, Phys. Fluids., 32 (2020), 113105. https://doi.org/10.1063/5.0032821 doi: 10.1063/5.0032821
[13]	Y. H. Jiang, H. G. Sun, Y. Bai, Y. Zhang, MHD flow, radiation heat and mass transfer of fractional Burgers' fluid in porous medium with chemical reaction, Comput. Math. Appl., 115 (2022), 68–79. https://doi.org/10.1016/j.camwa.2022.01.014 doi: 10.1016/j.camwa.2022.01.014
[14]	A. V. Chechkin, R. Gorenflo, I. M. Sokolov, Retarding subdiffusion and accelerating superdiffusion governed by distributed order fractional diffusion equations, Phys. Rev. E, 66 (2002), 046129. https://doi.org/10.1103/PhysRevE.66.046129 doi: 10.1103/PhysRevE.66.046129
[15]	M. Caputo, Elasticità e dissipazione, Bologna: Zanichelli, 1969.
[16]	L. Liu, L. B. Feng, Q. Xu, L. C. Zheng, F. W. Liu, Flow and heat transfer of generalized Maxwell fluid over a moving plate with distributed order time fractional constitutive models, Int. Commun. Heat Mass Transf., 116 (2020), 104679. https://doi.org/10.1016/j.icheatmasstransfer.2020.104679 doi: 10.1016/j.icheatmasstransfer.2020.104679
[17]	Y. L. Qiao, X. P. Wang, H. Y. Xu, H. T. Qi, Numerical analysis for viscoelastic fluid flow with distributed/variable order time fractional Maxwell constitutive models, Appl. Math. Mech.-Engl. Ed., 42 (2021), 1771–1786. https://doi.org/10.1007/s10483-021-2796-8 doi: 10.1007/s10483-021-2796-8
[18]	Z. F. Long, L. Liu, S. Yang, L. B. Feng, L. C. Zheng, Analysis of Marangoni boundary layer flow and heat transfer with novel constitution relationships, Int. Commun. Heat Mass Transf., 127 (2021), 105523. https://doi.org/10.1016/j.icheatmasstransfer.2021.105523 doi: 10.1016/j.icheatmasstransfer.2021.105523
[19]	W. D. Yang, X. H. Chen, X. R. Zhang, L. C. Zheng, F. W. Liu, Flow and heat transfer of viscoelastic fluid with a novel space distributed-order constitution relationship, Comput. Math. Appl., 94 (2021), 94–103. https://doi.org/10.1016/j.camwa.2021.04.023 doi: 10.1016/j.camwa.2021.04.023
[20]	L. B. Feng, I. Turner, T. Moroney, F. W. Liu, An investigation of space distributed-order models for simulating anomalous transport in a binary medium, Appl. Math. Comput., 434 (2022), 127423. https://doi.org/10.1016/j.amc.2022.127423 doi: 10.1016/j.amc.2022.127423
[21]	X. H. Chen, H. B. Xie, W. D. Yang, M. W. Chen, L. C. Zheng, Start-up flow in a pipe of a double distributed-order Maxwell fluid, Appl. Math. Lett., 134 (2022), 108302. https://doi.org/10.1016/j.aml.2022.108302 doi: 10.1016/j.aml.2022.108302
[22]	Y. X. Niu, Y. Liu, H. Li, F. W. Liu, Fast high-order compact difference scheme for the nonlinear distributed-order fractional Sobolev model appearing in porous media, Math Comput Simul, 203 (2023), 387–407. https://doi.org/10.1016/j.matcom.2022.07.001 doi: 10.1016/j.matcom.2022.07.001
[23]	L. Liu, S. Y. Chen, L. B. Feng, J. Zhu, J. S. Zhang, L. C. Zheng, et al., A novel distributed order time fractional model for heat conduction, anomalous diffusion, and viscoelastic flow problems, Comput. Fluid., 265 (2023), 105991. https://doi.org/10.1016/j.compfluid.2023.105991 doi: 10.1016/j.compfluid.2023.105991
[24]	Y. J. Hu, B. T. Li, C. G. Cao, On viscoelastic blood in a locally narrow artery with magnetic field: application of distributed-order time fractional Maxwell model, Phys Scr, 99 (2024), 055018. https://doi.org/10.1088/1402-4896/ad3686 doi: 10.1088/1402-4896/ad3686
[25]	M. C. Zhang, F. W. Liu, I. W. Turner, V. V. Anh, Numerical simulation of the distributed-order time-space fractional Bloch-Torrey equation with variable coefficients, Appl. Math. Model., 129 (2024), 169–190. https://doi.org/10.1016/j.apm.2024.01.050 doi: 10.1016/j.apm.2024.01.050
[26]	W. Ding, S. Patnaik, S. Sidhardh, F. Semperlotti, Applications of distributed-order fractional operators: a review, Entropy, 23 (2021), 110. https://doi.org /10.3390 /e23010110
[27]	L. Liu, L. B. Feng, Q. Xu, Y. P. Chen, Anomalous diffusion in comb model subject to a novel distributed order time fractional Cattaneo–Christov flux, Appl. Math. Lett., 102 (2020), 106116. https://doi.org/10.1016/j.aml.2019.106116 doi: 10.1016/j.aml.2019.106116
[28]	I. Podlubny, Fractional Differential Equations, San Diego: Academic Press, 1999, 78–85.
[29]	K. Diethelm, N. J. Ford, Numerical analysis for distributed-order differential equations, J. Comput. Appl. Math., 225 (2009), 96–104. https://doi.org/10.1016/j.cam.2008.07.018 doi: 10.1016/j.cam.2008.07.018
[30]	K. Diethelm, N. J. Ford, Analysis of Fractional Differential Equations, J. Math. Anal. Appl., 265 (2002), 229–248. https://doi.org/10.1006/jmaa.2000.7194 doi: 10.1006/jmaa.2000.7194
[31]	L. Liu, S. Yang, L. B. Feng, Q. Xu, L. C. Zheng, F. W. Liu, Memory dependent anomalous diffusion in comb structure under distributed order time fractional dual-phase-lag model, Int. J. Biomath., 14 (2021), 2150048. https://doi.org/10.1142/S1793524521500480 doi: 10.1142/S1793524521500480
[32]	F. Liu, P. Zhuang, V. Anh, I. Turner, K. Burrage, Stability and convergence of the difference methods for the space time fractional advection diffusion equation, Appl. Math. Comput., 191 (2007), 12–20. https://doi.org/10.1016/j.amc.2006.08.162 doi: 10.1016/j.amc.2006.08.162
[33]	Z. Sun, X. Wu, A fully discrete difference scheme for a diffusion-wave system, Appl. Numer. Math., 56 (2006), 193–209. https://doi.org/10.1016/j.apnum.2005.03.003 doi: 10.1016/j.apnum.2005.03.003

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

Networks and Heterogeneous Media

1.2 1.8

Metrics

Article views(1112) PDF downloads(60) Cited by(0)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(10)

Networks and Heterogeneous Media

Natural convection flow and heat transfer of generalized Maxwell fluid with distributed order time fractional derivatives embedded in the porous medium

Related Papers:

Abstract

1. Introduction

2. Mathematical formulation

3. Numerical technique

4. Validation of the numerical results

4.1. Solvability of the iterative difference equation

4.2. Grid independence verification

4.3. Numerical test with source terms

5. Results and discussion

6. Conclusions

Acknowledgments

Use of AI tools declaration

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

Networks and Heterogeneous Media

Natural convection flow and heat transfer of generalized Maxwell fluid with distributed order time fractional derivatives embedded in the porous medium

Related Papers:

Abstract

1. Introduction

2. Mathematical formulation

3. Numerical technique

4. Validation of the numerical results

4.1. Solvability of the iterative difference equation

4.2. Grid independence verification

4.3. Numerical test with source terms

5. Results and discussion

6. Conclusions

Acknowledgments

Use of AI tools declaration

Conflict of interest

References

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog