Exploring variable-sensitive $q$-difference equations for $q$-SINE Euler polynomials and $q$-COSINE-Euler polynomials

1.   Introduction

This section briefly outlines the essential definitions and theorems required for understanding this study. For $q \in \mathbb{R}- \{1\}$, the $q$-number is defined as:

In the definition of the $q$-number, it noted that $\lim_{q \to 1}[n]_{q} = n$; see ^[8,9,23]. Moreover, for $k \in \mathbb{Z}$, $[k]_{q}$ is referred to as a $q$-integer.

The $q$-numbers introduced by Jackson (^[8,9]) have led to expanded theories that intersect with established fields; see, ^{[1,6,12,13,18,21,22]}. The $q$-Gaussian binomial coefficients (^[10,23]) are defined as

Here, $m$ and $r$ denote nonnegative integers. Note that $[{\omega}]_{q}! = [{\omega}]_{q}[{\omega}-1]_{q} \cdots [2]_{q}[1]_{q}$ and $[0]_{q}! = 1$.

The $q$-binomial theorem (^[10,14]) can be expressed as:

Definition 1.1. Let ${\alpha}$ be any complex numbers with $|{\alpha}| < 1$. Then, two forms of $q$-exponential functions (^[7,20,23]) can be expressed as

It is noted that $\lim_{q \to 1} e_{q}({\alpha}) = e^{{\alpha}}$ and $e_{q}({\alpha})E_{q}(-{\alpha}) = 1$.

Definition 1.2. The $q$-derivative of a function $f$ with respect to ${\alpha}$ is defined by

and $D_{q}f(0) = f'(0)$; see ^[2,5,8,23].

We can prove that $f$ is differentiable at zero, and it is clear that $D_{q}{\alpha}^{{\omega}} = [{\omega}]_{q}{\alpha}^{{\omega}-1}$. Because the polynomials covered in this study deal with multiple variables, we use the derivative with respect to ${\alpha}, {\beta}$, and $t$, which are expressed as $D_{q, {\alpha}}$, $D_{q, {\beta}}$, and $D_{q, t}$, respectively.

Theorem 1.3. Definition 1.2 gives us the following properties:

Based on the above, research on $q$-differential equations and $q$-difference equations has been conducted by many mathematicians. Bernoulli's differential equation, specific forms of differential equations, has been explored in conjunction with $q$-numbers, and studies on this topic have also been undertaken by researchers. In ^[20], differential equations manifest in the form of Bernoulli's differential equation as follows:

This equation has $q$-Euler polynomials as solutions.

Definition 1.4. The generating function for the $q$-Euler numbers and polynomials (^[3,4,17]) are

In this definition, when $q$ goes to $1$, give standard notation for the Euler numbers and polynomials; see ^[11,12]. Let $q \to 1$ in Definition 1.4. Then, we can find the Euler numbers and polynomials as

In ^[15], the authors introduced new Euler polynomials (sine Euler polynomials and cosine Euler polynomials) by replacing ${\alpha}$ with complex numbers and studied several properties thereof. Furthermore, ^[19] combines the polynomials discussed in ^[15] with $q$-numbers to construct a Euler polynomial that incorporates $q$-trigonometric functions. The study also reveals associated properties and symmetrical structures. Specifically, the authors pinpoint approximate roots that fluctuate based on the value of $q$ and present a visual representation of these roots.

Definition 1.5. The generating function for the $q$-SINE Euler (QSE) and $q$-COSINE Euler (QCE) polynomials ^[19] are

respectively.

In Definition 1.5, when $q$ goes to $1$, parametrically Euler or Bernoulli polynomials are obtained. In ^[16], $C_{{\omega}, q}({\alpha}, {\beta})$ and $S_{{\omega}, q}({\alpha}, {\beta})$ are defined as follows:

An important motivation for this study is to identify $q$-Bernoulli differential equations whose solutions are QSE and QCE polynomials. Given that QSE and QCE polynomials include $q$-trigonometric functions and two variables, $q$-Bernoulli's differential equations are expected to manifest in various forms.

The organization of this study is as follows: Section 2 outlines the essential elements required to achieve the key findings of this paper. In this section, we examine the relationships between polynomials and difference equations, which vary based on the variables involved. Section 3 elaborates on the $q$-difference equations associated with the QSE polynomial, drawing upon the lemmas established in the preceding section. We identify multiple $q$-difference equations that vary both by the type of polynomial and the variables. Section 4 employs computational methods to analyze the structure of the approximate roots of higher-order polynomials, aiming to uncover the QSE polynomial characteristics that emerge as a solution in Section 3. Understanding the form of these approximate roots enables further verification of the polynomial's properties.

2.   Several lemmas related to the main results

The requisite lemmas are obtained to derive the difference equations related to QCE and QSE polynomials. In this context, here, you can see that the relationships between the $q$-derivatives and QCE and QSE polynomials vary based on the variables ${\alpha}$ and ${\beta}$.

Lemma 2.1. Let $k$ be a nonnegative integer. Then, the following relations can be formulated:

Proof. After calculating the $q$-derivative of $e_{q}(t {\alpha})$ directly, we obtain

(ⅰ) Using the $q$-derivative and Eq (2.1) in $S_{{\omega}, q} ({\alpha}, {\beta})$ with respect to ${\alpha}$, we can express the relation:

Applying the coefficient comparison method to Eq (2.2) yields:

By repeating the same process as in Eq (2.2), we obtain the following:

The relationship between $D_{q, {\alpha}}^{(k)} S_{{\omega}, q} ({\alpha}, {\beta})$ and $S_{{\omega}-k, q} ({\alpha}, {\beta})$ that manifests at the $k$-th instance is captured in Lemma 2.1 (ⅰ) that has been obtained.

(ⅱ) Using a method similar to (ⅰ) in $C_{{\omega}, q} ({\alpha}, {\beta})$, we can find Lemma 2.1 (ⅱ); hence, the proof of (ⅱ) is omitted. □

Lemma 2.2. Let $k$ be a nonnegative integer. Then, the following is valid:

Proof. The $q$-derivative for the $q$-cosine function and $q$-sine function can be verified as follows:

see, ^[5,19,23].

(ⅰ) Upon applying Eq (2.3) in $S_{{\omega}, q} ({\alpha}, {\beta})$ with respect to ${\beta}$, the following is obtained:

Continuation of the process based on Eq (2.4) yields:

At the $k$-th instance, the desired result is obtained.

(ⅱ) Using the processes outlined in Eqs (2.3) and (2.4) similarly for $S_{{\omega}, q} ({\alpha}, {\beta})$, we can find Lemma 2.2; (ⅱ) hence, the related proof process can be omitted. □

Corollary 2.3. If $q \to 1$ in Lemma 2.2, the following result holds:

Lemma 2.4. For $k \in$ nonnegative integer, we have the following relations with ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ and ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$:

Proof. (ⅰ) Using the $q$-derivative in ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ about ${\alpha}$, we get:

After comparing the coefficients of ${\theta}^{{\omega}}$ in Eq (2.5) and continuing to use the same method as in Eq (2.5), we can formulate:

Via induction, we obtain lemma 2.4 (ⅰ).

(ⅱ) If we apply the proof of (ⅰ) of the lemma 2.4 similarly to ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$, we can derive (ⅱ) of the lemma; hence, the proof process is omitted. □

Lemma 2.5. Let $k$ be a nonnegative integer. Then, the following hold:

Proof. (ⅰ) Applying the $q$-derivative in ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ with respect to ${\beta}$, we obtain

Using the coefficient comparison method and induction, we can write:

to derive the desired result.

(ⅱ) If we apply the proof process of (ⅰ) of Lemma 2.5 similarly to ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$, we can derive (ⅱ) of the lemma; hence, the proof process is omitted. □

3.   Multiple $q$-difference equations for QSE and QCE polynomials

In this section, we use the lemmas of the previous section to verify the $q$-difference equations associated with QSE and QCE polynomials. The $q$-difference equations that vary based on the variables are shown to have QSE and QCE polynomials as solutions.

Theorem 3.1. The $q$-difference equation of the form

has ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as a solution.

Proof. Using the generating function of QSE polynomials, we find a relation for ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$, ${\mathfrak{E}}_{{\omega}, q}$ and $S_{{\omega}, q} ({\alpha}, {\beta})$ as

Comparing both sides of Eq (3.1) for ${\theta}^{{\omega}}$ yields

If we replace Eq (3.2) with Lemma 2.1. (ⅰ), we can write

We obtain the desired result by expanding the series in Eq (3.3). □

Corollary 3.2. For $q \to 1$ in Theorem 3.1, the following holds:

Theorem 3.3. The polynomial ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ is a solution of

Proof. Using a procedure similar to Eq (3.1) for the QCE polynomial, we can write:

Using Lemma 2.1.(ⅱ), Eq (3.4) becomes Eq (3.5):

From Eq (3.5), we can derive Theorem 3.3. □

Theorem 3.4. Let ${\omega}$ be a nonnegative integer. Then, the $q$-difference equation below, for variable ${\beta}$, has ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as the solution.

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Proof. In Lemma 2.2, we can formulate

Applying Eq (3.6) in Eq (3.2), we can complete the proof of Theorem 3.4. □

Corollary 3.5. Setting $q \to 1$ in Theorem 3.4, the following holds:

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Theorem 3.6. For variable ${\beta}$, ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ is one of the following solutions of the $q$-difference equations:

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Proof. In Lemma 2.2, it can be observed that

Considering Eq (3.7) in Eq (3.4), we obtain the result of Theorem 3.6. □

Theorem 3.7. For $e_{q}(t) \ne -1$, the QSE polynomial is one of the solutions of the following ${\omega}$-th order difference equation:

Proof. If $e_{q}({\theta}) \ne -1$ in the generating function of QSE polynomials, the following derivation is obtained:

After comparing the series on both sides in Eq (3.8), we can write:

If we substitute (ⅰ) of Lemma 2.4 into the righthand side of Eq (3.9), we can formulate

By expanding the finite series on the left-hand side of Eq (3.10), we obtain the desired result. □

Theorem 3.8. The $q$-difference equation

has ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as the solution.

Proof. Similar to the procedure used for finding Eq (3.9) in Theorem 3.7, the relationship between ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ and $C_{{\omega}, q}({\alpha}, {\beta})$ is:

Substituting (ⅱ) of Lemma 2.4 into the righthand side of Eq (3.9), we obtain:

Using Eq (3.12), we can finish the proof of Theorem 3.8. □

Corollary 3.9. For $q \to 1$ in Theorems 3.7 and 3.8, the following holds:

Theorem 3.10. Under the following conditions, the $q$-difference equation for ${\beta}$ has ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as the solution.

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Proof. By transforming Lemma 2.5, we can express:

The calculated result after applying Eq (3.13) to Eq (3.8) yields Theorem 3.10. □

Corollary 3.11. Consider $q \to 1$ in Theorem 3.10. Then, we can formulate:

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Theorem 3.12. The $q$-difference equation for ${\beta}$ has ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as the solution assuming the following conditions:

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Proof. Using Lemma 2.5, ${}_{C}{\mathfrak{E}}_{{\omega}-k, q} ({\alpha}, {\beta})$ is expressed as:

Applying Eq (3.14) to Eq (3.11) yields Theorem 3.12. □

Theorem 3.13. The $q$-difference equation below with the Euler polynomials ${\mathfrak{E}}_{{\omega}, q}({\alpha})$ has ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as a solution.

Proof. The $q$-difference formula for the product of functions can be verified as follows:

To find the $q$-difference equation, we can obtain Eq (3.16) by differentiating ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ with respect to $t$:

In addition, if we use Eq (3.15) to differentiate the generating function of ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ with respect to ${\theta}$, the following holds:

After replacing Euler polynomials ${\mathfrak{E}}_{{\omega}, q}({\alpha})$ and QSE polynomials ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ in Eq (3.17), we obtain:

Plugging Eq (3.16) into the left-hand side of Eq (3.18), we have

By using Lemma 2.4 (ⅰ), Eq (3.19) can lead to Eq (3.20):

Equation (3.20) represents the desired result. □

Corollary 3.14. By setting $q \to 1$ in Theorem 3.13, the following condition is satisfied :

Theorem 3.15. The $q$-difference equation below, which involves the Euler polynomials ${\mathfrak{E}}_{{\omega}, q}({\alpha})$, features ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as a solution:

Proof. Following a similar calculation process as used for Eq (3.19) with ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$, the result is as follows.

By applying Lemma 2.7 (ⅱ) to Eq (3.21), we find the result of Theorem 3.15. □

Theorem 3.16. The following $q$-difference equations, which vary based on the conditions of ${\omega}$, are:

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

The above equations have ${}_{S}{\mathfrak{E}}_{{\omega}, q}({\alpha}, {\beta})$ as their solution.

Proof. Application of Eq (3.13) to Eq (3.18) yields the desired result. □

Corollary 3.17. Based on Theorem 3.16, the following holds:

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Theorem 3.18. ${}_{C}{\mathfrak{E}}_{{\omega}, q}({\alpha}, {\beta})$ is a solution of the $q$-difference equations below that vary depending on the conditions of ${\omega}$.

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Proof. We obtain the required result after applying Eq (3.14) to Eq (3.21). □

Theorem 3.19. The $q$-difference equation

features ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as a solution.

Proof. Using $e_{q}(q {\theta} {\alpha}) E_{q}(- q {\theta} {\alpha}) = 1$ and considering the definition of $(1 \oplus {\alpha})_{q}^{{\omega}}$ for Eq (3.17), we can derive the following:

After plugging Eq (3.16) into the left-hand of Eq (3.22) and comparing the coefficients of both sides, we can formulate the following:

If we use the relation between $D_{q, {\alpha}}^{(m)} {}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ and ${}_{S}{\mathfrak{E}}_{{\omega}-m, q} ({\alpha}, {\beta})$ in Eq (3.23), then we obtain:

Equation (3.24) produces exactly the result we are looking for. □

Theorem 3.20. The $q$-difference equation

has ${}_{C}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as a solution.

Proof. As the proof can be established similarly to that of Theorem 3.19, it is omitted. □

Theorem 3.21. The $q$-difference equation, which varies based on the condition of the highest-order term, has ${}_{S}{\mathfrak{E}}_{{\omega}, q} ({\alpha}, {\beta})$ as its solution.

(ⅰ) If ${\omega}$ is an even number, then

(ⅱ) If ${\omega}$ is an odd number, then

Proof. After substituting Eq (3.13) in Eq (3.23), we obtain the result of Theorem 3.21. □

4.   Properties of approximate roots of QSE polynomials

In this section, we focus on the QSE polynomial, which is the solution to the $q$-difference equation obtained earlier. Using Wolfram Mathematica version 11.2, we fix the value of ${\beta}$ within a QSE polynomial and show the forms of specific polynomials and the structures of their approximate roots. Additionally, we understand the structure of the roots of the QSE polynomial in $3$-D and consider conjectures related to it. These characteristics depend on the value of $q$.

The polynomials that emerge, as defined by the QSE polynomial, are as follows:

To understand the structure and characteristics of the approximate roots of the QSE polynomial, we conducted several experiments by using specific values for the $q$ and ${\beta}$ variables.

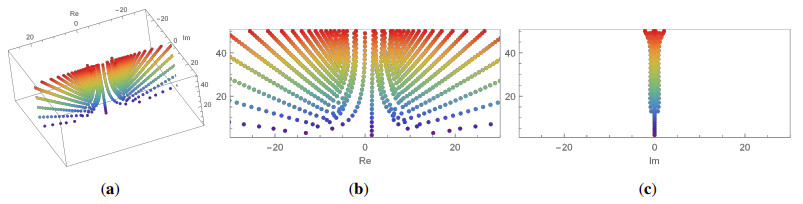
In Figure 1, ${\beta} = 10$ is fixed, and $q = 0.999$ is given. Figure 1(a) shows the stacking pattern of the approximate roots of the QSE polynomial for $0 \leq {\omega} \leq 50$. In (a), the approximate roots increase almost linearly along the line $\text{Im}({\alpha}) = 0$, with a noticeable deviation near $\text{Re}({\alpha}) = 0$. Figure 1(b) presents a perspective of Figure 1(a) with a focus on the axes of $\text{Re}({\alpha})$ and ${\omega}$. Figure 1(b) indicates that as the value of ${\omega}$ increases, the locations of the approximate roots expand to the left and right. Figure 1(c), viewed from the left side of (a), displays the approximate roots congregated near the line $\text{Im}({\alpha}) = 0$. Figure 1(c) indicates that the positions of the approximated roots change near $\text{Im}({\alpha}) = 0$.

Figure 2 is obtained by changing the range and value of $q$ based on this idea. After setting $q = 0.555$ and considering the range, $-2 \leq \text{Re}({\alpha}) \leq 2$ and $-2 \leq \text{Im}({\alpha}) \leq 2$ for the QSE polynomials, we obtain the numerical results shown in Figure 2. Figure 2(a) shows that the approximated roots form a pattern resembling a circle at ${\omega} = 50$, and it also verifies that these approximated roots are consistently aligned at $\text{Im}({\alpha}) = 0$. Figure 2(b) shows a top-down view of the arrangement in Figure 2(a). The red dots denote ${\omega} = 50$ and the blue dots indicate when the value of $n$ is small.

Figure 3 shows approximated roots resembling a circle based on Figure 2. In Figure 2, the approximate roots that deviate significantly from the circular pattern have been omitted. Hence, we can only present the approximated roots resembling a circle - see, Figure 3. In Figure 3(a), (b), and (c), the value of ${\omega}$ changed, and the blue line represents the circle closest to the approximated roots. The blue dot in the middle represents the center.

Figure 3 examines the discrepancy between the center of the approximated circle and the approximate roots. The observed error margins are detailed in Table 1 below.

From Figures 1, 2, and 3 and Table 1, we can infer the following:

Conjecture 4.1. As the value of ${\omega}$ increases, the approximated roots of ${}_{S}{\mathfrak{E}}_{{\omega}, 0.555}({\alpha}, 10)$ appear as an approximated circle, excluding the real roots.

5.   Conclusion

This study has identified several higher-order difference equations in the form of $q$-Bernoulli differential equations related to QSE polynomials. Differential equations expressed with $\alpha$ as a variable appear in the form of various numbers as coefficients, and differential equations expressed with $\beta$ as a variable show characteristics including the periodicity of $q$-trigonometric functions. Furthermore, the configuration and characteristics of the approximate roots of the QSE polynomial, which solve the previously mentioned difference equation, have been validated. If we select a high-order polynomial among the QSE polynomials and check the dynamic system of the roots, except for a few roots, the remaining roots maintain their circular form. We think this characteristic is an interesting feature that appears in polynomials containing $q$-numbers. Additionally, the table included in the paper strongly supports this idea and is a useful data for research related to the dynamic systems of roots.

Author contributions

Jung Yoog Kang: Software, Writing-original draft and Writing-review & editing, Conceptualization, Methodology, Cheon Seoung Ryoo: Supervision and Validation, Data curation, Software and Writing-review & editing. All authors equally contributed to this manuscript and approved the final version.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence tools in the creation of this article.

Conflict of interest

The authors declare no conflict of interest.