The discrete convolution for fractional cosine-sine series and its application in convolution equations

Rongbo Wang; Qiang Feng; Jinyi Ji; Rongbo Wang; Qiang Feng; Jinyi Ji

doi:10.3934/math.2024130

AIMS Mathematics

2024, Volume 9, Issue 2: 2641-2656. doi: 10.3934/math.2024130

Previous Article Next Article

Research article

The discrete convolution for fractional cosine-sine series and its application in convolution equations

School of Mathematics and Computer Science, Yan'an University, Yan'an 716000, China

Received: 01 November 2023 Revised: 13 December 2023 Accepted: 18 December 2023 Published: 27 December 2023
MSC : 44A35, 45E10, 47A30

The fractional sine series (FRSS) and the fractional cosine series (FRCS) were defined. Three types of discrete convolution operations for FRCS and FRSS were introduced, along with a detailed investigation into their corresponding convolution theorems. The interrelationship between these convolution operations was also discussed. Additionally, as an application of the presented results, two forms of discrete convolution equations based on the proposed convolution theorems were examined, resulting in explicit solutions for these equations. Furthermore, numerical simulations were provided to demonstrate that our proposed solution can be easily implemented with low computational complexity.

Keywords:

Citation: Rongbo Wang, Qiang Feng, Jinyi Ji. The discrete convolution for fractional cosine-sine series and its application in convolution equations[J]. AIMS Mathematics, 2024, 9(2): 2641-2656. doi: 10.3934/math.2024130

Related Papers:

[1]	Snježana Maksimović, Sanja Atanasova, Zoran D. Mitrović, Salma Haque, Nabil Mlaiki . Abelian and Tauberian results for the fractional Fourier cosine (sine) transform. AIMS Mathematics, 2024, 9(5): 12225-12238. doi: 10.3934/math.2024597
[2]	Ahmed Salem, Kholoud N. Alharbi . Fractional infinite time-delay evolution equations with non-instantaneous impulsive. AIMS Mathematics, 2023, 8(6): 12943-12963. doi: 10.3934/math.2023652
[3]	Ahu Ercan . Comparative analysis for fractional nonlinear Sturm-Liouville equations with singular and non-singular kernels. AIMS Mathematics, 2022, 7(7): 13325-13343. doi: 10.3934/math.2022736
[4]	Yue Liang . Study on the controllability of delayed evolution inclusions involving fractional derivatives. AIMS Mathematics, 2024, 9(7): 17984-17996. doi: 10.3934/math.2024876
[5]	Amir Ali, Abid Ullah Khan, Obaid Algahtani, Sayed Saifullah . Semi-analytical and numerical computation of fractal-fractional sine-Gordon equation with non-singular kernels. AIMS Mathematics, 2022, 7(8): 14975-14990. doi: 10.3934/math.2022820
[6]	Altyeb Taha . A novel deep learning-based hybrid Harris hawks with sine cosine approach for credit card fraud detection. AIMS Mathematics, 2023, 8(10): 23200-23217. doi: 10.3934/math.20231180
[7]	Yong-Ki Ma, Marimuthu Mohan Raja, Kottakkaran Sooppy Nisar, Anurag Shukla, Velusamy Vijayakumar . Results on controllability for Sobolev type fractional differential equations of order $ 1 < r < 2 $ with finite delay. AIMS Mathematics, 2022, 7(6): 10215-10233. doi: 10.3934/math.2022568
[8]	Ali Khalouta, Abdelouahab Kadem . A new computational for approximate analytical solutions of nonlinear time-fractional wave-like equations with variable coefficients. AIMS Mathematics, 2020, 5(1): 1-14. doi: 10.3934/math.2020001
[9]	Ali Khalouta, Abdelouahab Kadem . A new numerical technique for solving Caputo time-fractional biological population equation. AIMS Mathematics, 2019, 4(5): 1307-1319. doi: 10.3934/math.2019.5.1307
[10]	Erdal Bas, Ramazan Ozarslan, Resat Yilmazer . Spectral structure and solution of fractional hydrogen atom difference equations. AIMS Mathematics, 2020, 5(2): 1359-1371. doi: 10.3934/math.2020093

Abstract

1. Introduction

Convolution plays an important role in various fields, including integral evaluation, numerical analysis, solving differential and integral equations, mathematical physics ^{[1,2,3,4,5,6,7,8,9]} and filter design for signal processing ^{[10,11,12,13,14]}. The importance of different convolutions lies in their diverse properties and applications that they are able to exhibit ^{[15,16,17,18,19,20,21,22,23,24]}. Therefore, it is worthwhile and interesting to continually investigate convolution and associated theorems due to their high potential for revealing new properties and concrete applications

The discrete Fourier transforms ^[25] and the discrete fractional transform ^[26] have emerged as significant tools in digital signal processing, given that practical data is predominantly processed at discrete samples. Extensive research has been conducted on the discrete Fourier cosine convolution and the discrete Fourier sine convolution associated with the Fourier cosine and sine series, resulting in numerous valuable theoretical achievements including Titchmarsh's type theorem, discrete Young's type theorem and Watson's type theorem ^{[27,28,29,30]}, as well as the application of discrete fractional calculus ^[31,32]. However, while the theory of discrete convolution for fractional cosine and sine series is still in its early stages, it provides a driving force toward formulating a discrete fractional cosine and sine series. Additionally, compared to the Fourier transform, which lacks free parameters, the fractional transform offers enhanced flexibility and processing capabilities. Therefore, exploring the define fractional cosine series (FRCS) and fractional sine series (FRSS), along with their corresponding convolution theorems and applications, is both theoretically intriguing and practically beneficial.

The convolution equation has found significant applications in diverse fields such as engineering mechanics, energy transfer and membrane or axis oscillation, particularly when addressing problems related to the electrostatic field and magnetic field synthesis as well as the digital signal processing (see ^{[3,4,7,15,16,17,18,19,20,21,22,23,24,32,33]}). Obtaining solutions for these convolution equations is a meaningful issue in equation theory that has been extensively studied by many researchers.

Taking this opportunity, our primary objective is to introduce the concept of FRCS and FRSS while exploring their fundamental properties and applications in convolution equations. This paper makes a threefold contribution: First, we extend the Fourier sine series (FSS) and the Fourier cosine series (FCS) into the fractional domain by providing definitions for the FRSS and the FRCS. Second, we propose three types of convolution operations for FRSS and FRCS, derive corresponding convolution theorems and discuss the relationship between two discrete convolution operations. The classical Fourier series results can be viewed as a special case of the derived results in this paper. Third, the application of the discrete fractional cosine-sine series convolution is discussed, and solutions for two types of convolution equations are analyzed with numerical simulations demonstrating that our proposed solution is easily implementable with low computational complexity.

The structure of this paper is outlined as follows: Section 2 reviews the convolution operations for Fourier sine and cosine series, along with their associated convolution theorems. In Section 3, three distinct convolution operations for FRSS and FRCS are presented in detail together with derived convolution theorems. Section 4 investigates the relationship between two discrete convolution operations, while Section 5 discusses two types of convolution equations. Finally, conclusions are summarized in Section 6.

2. Preliminaries

In this section, we mainly review the Fourier cosine and sine series, discrete convolution and the corresponding convolution theorem relevant to this study.

The discrete Fourier cosine convolution $(x\underset{F_{cDT}}{\ast}y)(n)$ ^[29] and the discrete Fourier sine convolution $(x\underset{F_{sDT}}{\ast}y)(n)$ ^[30] of two series $x(n)$ and $y(n)$ on $\mathbb{N}$ are defined as follows:

$\begin{equation} \begin{aligned} (x\underset{F_{cDT}}{\ast}y)(n) = \sum\limits_{m = 1}^{\infty}x(m)[y(n+m)+y(|n-m|)]+x(0)y(n), \ \; n\geq0 \end{aligned} \end{equation}$

(2.1)

and

$\begin{equation} \begin{aligned} (x\underset{F_{sDT}}{\ast}y)(n) = \sum\limits_{m = 1}^{\infty}x(m)[y(|n-m|)-y(n+m)], \ \; n\geq0, \end{aligned} \end{equation}$

(2.2)

respectively. The following two convolution theorems fulfill

$\begin{equation} \begin{aligned} F_{cDT}\{(x\underset{F_{cDT}}{\ast}y)(n)\}(w) = 2F_{cDT}\{x(n)\}(w)F_{cDT}\{y(n)\}(w) \end{aligned} \end{equation}$

(2.3)

and

$\begin{equation} \begin{aligned} F_{sDT}\{(x\underset{F_{sDT}}{\ast} y)(n)\}(w) = 2F_{sDT}\{x(n)\}(w)F_{cDT}\{y(n)\}(w), \end{aligned} \end{equation}$

(2.4)

respectively, where $F_{cDT}$ denotes the FCS

$\begin{equation} \begin{aligned} F_{cDT}{x(n)}(\omega) = \frac{x(0)}{2}+\sum\limits_{n = 1}^{\infty}x(n)\cos(n\omega), \ \ \omega\in[0, \pi], \end{aligned} \end{equation}$

(2.5)

and $F_{sDT}$ denotes the FSS

$\begin{equation} \begin{aligned} F_{sDT}{x(n)}(\omega) = \sum\limits_{n = 0}^{\infty}x(n)\sin(n\omega), \ \ \omega\in[0, \pi]. \end{aligned} \end{equation}$

(2.6)

Let $\ell_{p}(\mathbb{N})$ , $1\leq p < \infty$ , denote the space of sequences $x = \{x(n)\}$ equipped with a norm

$\begin{equation} \begin{aligned} \|x\|_{p} = \left(\sum\limits_{n = 1}^{\infty}|x(n)|^{p}\right)^{\frac{1}{p}} < \infty, \ \ 1\leq p < \infty \end{aligned} \end{equation}$

(2.7)

and

$\begin{equation} \|x\|_{\infty} = \sup\limits_{n\geq0}|x_{n}| < \infty, \end{equation}$

(2.8)

where $\ell_{1}(\mathbb{N})$ is equipped with a norm

$\|x\| = (\sum\limits_{n = 1}^{\infty}|x(n)|),$

and $\ell_{p}^{o}(\mathbb{N})$ is its subspace with $x(0) = 0$ .

3. The convolution for FRCS and FRSS

In this section, we present the definitions of FRSS and FRCS, followed by three novel types of convolution operations for FRSS and FRCS. We also explore the relationships among these defined convolution operations and derive corresponding convolution theorems for FRCS and FRSS

Definition 3.1. Let $\alpha\in \mathbb{R}$ , a sequence $\{x(n)\}\in \ell_{1}(\mathbb{N})$ and the FRCS and the FRSS be defined as

$\begin{equation} \begin{aligned} S_{c}^{\alpha}{x(n)}(w) = 2\sum\limits_{n = 0}^{\infty}x(n)K^{\varphi}(n, w)\cos(\csc\varphi \cdot nw), \ \ w\in[0, \pi] \end{aligned} \end{equation}$

(3.1)

and

$\begin{equation} \begin{aligned} S_{s}^{\alpha}{x(n)}(w) = \sum\limits_{n = 0}^{\infty}x(n)K^{\varphi}(n, w)\sin(\csc\varphi \cdot nw), \ \ w\in[0, \pi], \end{aligned} \end{equation}$

(3.2)

respectively, where $K^{\varphi}(n, w)$ is given by

$K^{\varphi}(n, \omega) = \left\{\begin{array}{ll} A_{\varphi}e^{j\frac{n^{2}+w^{2}}{2} \cot\varphi}, &\; \varphi\neq k \pi, \\ \delta(n-w), &\; \varphi = 2k\pi, \\ \delta(n+w), &\; \varphi = (2k-1)\pi, \end{array}\right.$

and

$A_{\varphi} = \sqrt{\frac{1-j \cot\varphi}{2\pi}}, \ \ \varphi = \frac{\pi}{2}\alpha.$

Remark 3.1. When $\alpha = 2k-1$ , $k\in\mathbb{Z}$ , the FCS in (3.1) and the FSS in (3.2) reduce to the classical FCS in (2.5) and the FSS in (2.6), except for certain individual terms.

Definition 3.2. Let $x(n), y(n)\in \ell_{1}(\mathbb{N})$ and the convolution operation $\underset{c, \alpha}{\ast}$ for FRCS is defined as follows

$\begin{equation} \begin{aligned} (x\underset{c, \alpha}{\ast}y)(n) = T_{n, \varphi}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n-m|)+\widetilde{y}(n+m)], \end{aligned} \end{equation}$

(3.3)

where

$\begin{align*} A_{\varphi}& = \sqrt{\frac{1-j\cot\varphi}{2\pi}}, \ \ \widetilde{x}(n) = x(n)e^{M_{n, \varphi}}, \ \ \widetilde{y}(n) = y(n)e^{M_{n, \varphi}}, \\ T_{n, \varphi}& = A_{\varphi}e^{-M_{n, \varphi}}, \qquad\ \ M_{n, \varphi} = j\frac{n^{2}}{2}\cot\varphi, \quad\ \ \varphi = \frac{\pi}{2}\alpha, \ \alpha\in \mathbb{R}. \end{align*}$

Definition 3.3. Let $x(n), y(n)\in \ell_{1}(\mathbb{N})$ and the convolution operation $\underset{s, s, c, \alpha}{\ast}$ for FRSS is defined as follows

$\begin{equation} \begin{aligned} (x\underset{s, s, c, \alpha}{\ast}y)(n) = T_{n, \varphi}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n-m|)-\widetilde{y}(n+m)]. \end{aligned} \end{equation}$

(3.4)

Definition 3.4. Let $x(n), y(n)\in \ell_{1}(\mathbb{N})$ and the convolution operation $\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}$ with the weight function

$\omega = \sin(\csc\varphi\cdot w)$

for FRCS is defined as follows

$\begin{equation} \begin{aligned}(x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n) = &\frac{T_{n, \varphi}}{4}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n+m-1|)+\widetilde{y}(|n-m+1|)\\ &-\widetilde{y}(n+m+1)-\widetilde{y}(|n-m-1|)]. \end{aligned} \end{equation}$

(3.5)

Remark 3.2. When $\alpha = 2k-1$ , $k\in\mathbb{Z}$ , the convolution operations defined in Definitions 3.2 and 3.3 can be simplified to the convolution operations presented in (2.1) and (2.2), respectively. Additionally, the convolution operation described in Definition 3.4 can be reduced to the Fourier weighted convolution operation, except for certain individual terms.

The following convolution theorems for FRCS and FRSS are then derived in detail. Furthermore, the relationship between $\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}$ and ${\underset{s, s, c, \alpha}{\ast}}$ is provided.

Theorem 3.1. Let $x(n), y(n)\in \ell_{1}(\mathbb{N})$ , then

$(x\underset{c, \alpha}{\ast}y)(n)\in \ell_{1}(\mathbb{N}).$

$S_{c}^{\alpha}x$ and $S_{c}^{\alpha}y$ denote the FRCS for $x(n)$ and $y(n)$ . The convolution theorem for FRCS is obtained as follows

$\begin{equation} S_{c}^{\alpha }\left[(x\underset{c, \alpha}{\ast}y)(n)\right](w) = (S_{c}^{\alpha }x)(w)(S_{c}^{\alpha }\overline{y})(w), \ \ w\in[0, \pi], \end{equation}$

(3.6)

where

$(S_{c}^{\alpha }\overline{y})(w) = (S_{c}^{\alpha }y)(w)e^{-j\frac{w^{2}}{2}\cot\varphi}.$

Proof. We first prove the existence of the convolution operation $(x\underset{c, \alpha}{\ast}y)(n)$

$\begin{equation} \begin{aligned} \sum\limits_{n = 0}^{\infty}|(x\underset{c, \alpha}{\ast}y)(n)| \leq&|T_{n, \varphi}|\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}\left|\widetilde{x}(m)[\widetilde{y}(|n-m|)+\widetilde{y}(n+m)]\right|\\ \leq& 2|T_{n, \varphi}|\sum\limits_{m = 0}^{\infty}|\widetilde{x}(m)|\sum\limits_{n = 0}^{\infty}|\widetilde{y}(n)|\\ = &2|T_{n, \varphi}|\|x\|_{1}\cdot\|y\|_{1}; \end{aligned} \end{equation}$

(3.7)

therefore,

$(x\underset{c, \alpha}{\ast}y)(n)\in \ell_{1}(\mathbb{N}).$

Next, we prove the convolution Theorem 3.1 and from the Definition 3.1, we have

$\begin{equation} \begin{aligned} (S_{c}^{\alpha }x)(w)(S_{c}^{\alpha }y)(w) = &4\sum\limits_{a = 0}^{\infty}x(a)K^{\varphi}(a, w)\cos(\csc\varphi\cdot wa)\cdot \sum\limits_{b = 0}^{\infty}y(b)K^{\varphi}(b, w)\cos(\csc\varphi\cdot wb)\\ = &4N_{w, \varphi}A_{\varphi}^{2}\sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \cos(\csc\varphi\cdot w a)\cos(\csc\varphi\cdot w b) \\ = &2N_{w, \varphi}A_{\varphi}^{2}\sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \left[\cos( \csc\varphi\cdot w(a+b))+\cos(\csc\varphi\cdot w(a-b)) \right]\\ = &2N_{w, \varphi}A_{\varphi}^{2}\left[\sum\limits_{n = 0}^{\infty}\sum\limits_{m = n}^{\infty}e^{j\frac{n^{2}+(m-n)^{2}}{2}\cot\varphi}\right.\cdot x(n)y(m-n)\cos(\csc\varphi\cdot wm)\\ &+\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m+n)^{2}}{2}\cot\varphi}\cdot x(n)y(m+n)\cos(\csc\varphi\cdot wm)\\ &+\left.\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{n}e^{j\frac{n^{2}+(m-n)^{2}}{2}\cot\varphi}\cdot x(n)y(n-m)\cos(\csc\varphi\cdot wm)\right], \end{aligned} \end{equation}$

(3.8)

where

$N_{w, \varphi} = e^{jw^{2}\cot \varphi}.$

One finds

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}x)(w)(S_{c}^{\alpha}y)(w) = &2N_{w, \varphi}A_{\varphi}^{2}\sum\limits_{n = 0}^{\infty}x(n)\cdot \left[\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m-n)^{2}}{2}\cot\varphi}y(|m-n|)\cos(\csc\varphi\cdot wm)\right.\\ &\left.+\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m+n)^{2}}{2}\cot\varphi}y(m+n)\cos(\csc\varphi\cdot wm)\right]\\ = &2N_{w, \varphi}A_{\varphi}^{2}\sum\limits_{m = 0}^{\infty}\cos(\csc\varphi\cdot wm)\cdot \left\{\sum\limits_{n = 0}^{\infty}x(n)\left[e^{j\frac{n^{2}+(m-n)^{2}}{2}\cot\varphi}y(|m-n|)\right.\right.\\ &\left.\left.+e^{j\frac{n^{2}+(m+n)^{2}}{2}\cot\varphi}y(m+n)\right]\right\}. \end{aligned} \end{equation}$

(3.9)

From the Definition 3.2, we have

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}x)(w)(S_{c}^{\alpha}y)(w) = &2e^{j\frac{w^{2}}{2}\cot \varphi}\cdot \sum\limits_{m = 0}^{\infty}[(x\underset{c, \alpha}{\ast}y)(m)]K^{\varphi}(m, w)\cos(\csc\varphi\cdot wm)\\ = &e^{j\frac{k^{2}}{2}\cot\varphi}S_{c}^{\alpha }[(x\underset{c, {\alpha}}{\ast}y)(n)](w). \end{aligned} \end{equation}$

(3.10)

The proof is completed. □

Theorem 3.2. Let $x(n), 5y(n)\in \ell_{1}(\mathbb{N})$ , then

$(x\underset{s, s, c, \alpha}{\ast}y)(n)\in \ell_{1}(\mathbb{N}).$

$S_{s}^{\alpha}x$ , $S_{c}^{\alpha}y$ denote the FRSS for $x(n)$ and FRCS for $y(n)$ , respectively. The convolution theorem for FRSS is satisfied

$\begin{equation} \begin{aligned} S_{s}^{\alpha }[(x\underset{s, s, c, \alpha}{\ast}y)(n)](w) = (S_{s}^{\alpha }x)(w)(S_{c}^{\alpha }\overline{y})(w), \ \ w\in[0, \pi], \end{aligned} \end{equation}$

(3.11)

where

$(S_{c}^{\alpha }\overline{y})(w) = (S_{c}^{\alpha }y)(w)e^{-j\frac{w^{2}}{2}\cot\varphi}.$

Proof. The proof of Theorem 3.2 is similar to that of Theorem 3.1 and it is omitted. □

Remark 3.3. When $\alpha = 2k-1$ , $k\in\mathbb{Z}$ , it is easy to verify that the convolution theorem for the FCS and the FSS in (2.3) and (2.4) are both special cases of Theorems 3.1 and 3.2 in (3.6) and (3.11), respectively.

Theorem 3.3. Let $x(n), y(n)\in \ell_{1}(\mathbb{N})$ , then

$(x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n)\in \ell_{1}(\mathbb{N}).$

$S_{s}^{\alpha}x$ , $S_{c}^{\alpha}y$ denote the FRSS for $x(n)$ and the FRCS for $y(n)$ , respectively, then the convolution theorem with a weight function

$\omega = \sin(\csc\varphi\cdot w)$

for FRCS is obtained

$\begin{equation} \begin{aligned} S_{c}^{\alpha }[(x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n)](w) = \sin(\csc\varphi\cdot w)(S_{s}^{\alpha }x)(w)(S_{c}^{\alpha }\overline{y})(w), \ \ w\in[0, \pi], \end{aligned} \end{equation}$

(3.12)

where

$(S_{c}^{\alpha }\overline{y})(w) = (S_{c}^{\alpha }y)(w)e^{-j\frac{w^{2}}{2}\cot\varphi}.$

Proof. The existence of the convolution operation $(x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n)$ is similar to that of Theorem 3.1. Next, we will prove the convolution Theorem 3.3 and based on Definitions 3.1 and 3.4, we have

$\begin{equation} \begin{aligned} &\sin(\csc\varphi\cdot k)(S_{s}^{\alpha}x)(w)(S_{c}^{\alpha}y)(w)\\ & = 2\sin(\csc\varphi\cdot w)\sum\limits_{a = 0}^{\infty}x(a)K^{\varphi}(a, w)\sin(\csc\varphi\cdot wa)\cdot \sum\limits_{b = 0}^{\infty}y(b)K^{\varphi}(b, w)\cos(\csc\varphi\cdot wb)\\ & = \frac{N_{w, \varphi}A_{\varphi}^{2}}{2}\sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \left\{\cos[w( \csc\varphi\cdot (a+b-1))]-\cos[w( \csc\varphi\cdot (a+b+1))]\right.\\ &\quad+\left.\cos[w( \csc\varphi\cdot (a-b-1))]-\cos[w( \csc\varphi\cdot (a-b+1))]\right\} \\ & = -\frac{N_{w, \varphi}A_{\varphi}^{2}}{2}(F_{1}+F_{2}-F_{3}-F_{4}), \end{aligned} \end{equation}$

(3.13)

where

$\begin{equation} \begin{aligned} F_{1} = \sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \cos[w( \csc\varphi\cdot (a+b+1))], \\ F_{2} = \sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \cos[w( \csc\varphi\cdot (a-b+1))], \\ F_{3} = \sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \cos[w( \csc\varphi\cdot (a-b-1))], \\ F_{4} = \sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cdot \cos[w( \csc\varphi\cdot (a+b-1))]. \end{aligned} \end{equation}$

(3.14)

Let $a = n$ and $a+b+1 = m$ and we obtain

$\begin{equation} \begin{aligned} F_{1} = &\sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cos[w( \csc\varphi\cdot (a+b+1))]\\ = &\sum\limits_{n = 0}^{\infty}\sum\limits_{m = n+1}^{\infty}e^{j\frac{n^{2}+(m-n-1)^{2}}{2}\cot\varphi}x(n)y(m-n-1)\cdot \cos(\csc\varphi\cdot wm). \end{aligned} \end{equation}$

(3.15)

Similar to (3.15), we obtain

$\begin{equation} \begin{aligned} F_{2} = &\sum\limits_{a = 0}^{\infty}\sum\limits_{b = 0}^{\infty}e^{j\frac{a^{2}+b^{2}}{2}\cot\varphi}x(a)y(b)\cos[w( \csc\varphi\cdot (a-b+1))]\\ = &\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m+n+1)^{2}}{2}\cot\varphi}x(n)y(m+n+1)\cdot \cos(\csc\varphi \cdot wm)\\ &+\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{n+1}e^{j\frac{n^{2}+(n-m+1)^{2}}{2}\cot\varphi}x(n)y(n-m+1)\cdot \cos(\csc\varphi \cdot wm), \end{aligned} \end{equation}$

(3.16)

and from (3.15) and (3.16), we can derive

$\begin{equation} \begin{aligned} F_{1}+F_{2} = &\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m+n+1)^{2}}{2}\cot\varphi}x(n)y(m+n+1)\cdot \cos(\csc\varphi \cdot wm)\\ &+\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m-n-1)^{2}}{2}\cot\varphi}\cdot x(n)y(|m-n-1|)\cos(\csc\varphi \cdot wm). \end{aligned} \end{equation}$

(3.17)

In a manner similar to (3.15)–(3.17), we have

$\begin{equation} \begin{aligned} F_{3}+F_{4} = &\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m+n-1)^{2}}{2}\cot\varphi}x(n)y(|m+n-1|)\cdot \cos(\csc\varphi \cdot wm)\\ &+\sum\limits_{n = 0}^{\infty}\sum\limits_{m = 0}^{\infty}e^{j\frac{n^{2}+(m-n+1)^{2}}{2}\cot\varphi}\cdot x(n)y(|m-n+1|)\cos(\csc\varphi \cdot wm), \end{aligned} \end{equation}$

(3.18)

and by (3.17) and (3.18), we can achieve

$\begin{equation} \begin{aligned} \sin(\csc\varphi\cdot w)(S_{s}^{\alpha }x)(w)(S_{c}^{\alpha }y)(w) = &\frac{N_{w, \varphi}A_{\varphi}^{2}}{2}(F_{3}+F_{4}-F_{1}-F_{2})\\ = &2e^{j\frac{w^{2}}{2}\cot\varphi}{\sum\limits_{m = 0}^{\infty}(x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(m)K^{\varphi}(m, w)\cos(\csc\varphi \cdot wm)}\\ = &e^{j\frac{w^{2}}{2}\cot\varphi} S_{c}^{\alpha }[(x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(m)](w). \end{aligned} \end{equation}$

(3.19)

The proof is completed. □

4. Convolution relation of the fractional cosine and sine series

In this subsection, the convolution relation of $\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}$ and ${\underset{s, s, c, \alpha}{\ast}}$ will be presented as follows.

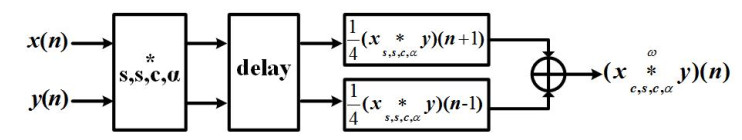
Theorem 4.1. Let $x(n), y(n)\in \ell_{1}(\mathbb{N})$ , and the convolution relation between $\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}$ and ${\underset{s, s, c, \alpha}{\ast}}$ satisfies the following equation

$\begin{equation} \begin{aligned} (x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n) = \frac{1}{4}(x{\underset{s, s, c, \alpha}{\ast}}y)(n+1)-\frac{1}{4} (x{\underset{s, s, c, \alpha}{\ast}}y)(n-1). \end{aligned} \end{equation}$

(4.1)

Proof. From Definition 3.4, we have

$\begin{equation} \begin{aligned} (x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n) = &\frac{A_{\varphi}e^{M_{n, \varphi}}}{4}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n-m+1|)-\widetilde{y}(n+m+1)]\\ &+\frac{A_{\varphi}e^{M_{n, \varphi}}}{4}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n+m-1|)-\widetilde{y}(|n-m-1|)], \end{aligned} \end{equation}$

(4.2)

and from Definition 3.3 and (4.2), we obtain

$\begin{equation} \begin{aligned} \frac{A_{\varphi}e^{M_{n, \varphi}}}{4}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n-m+1|)-\widetilde{y}(n+m+1)] & = \frac{1}{4} (x{\underset{s, s, c, \alpha}{\ast}}y)(n+1). \end{aligned} \end{equation}$

(4.3)

Similarly to (4.3), we can achieve the following result

$\begin{equation} \begin{aligned} &\frac{A_{\varphi}e^{M_{n, \varphi}}}{4}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n+m-1|)-\widetilde{y}(|n-m-1|)]\\ & = -\frac{A_{\varphi}e^{M_{n, \varphi}}}{4}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)[\widetilde{y}(|n-1-m|)-\widetilde{y}(n-1+m)]\\ & = -\frac{1}{4}(x{\underset{s, s, c, \alpha}{\ast}}y)(n-1). \end{aligned} \end{equation}$

(4.4)

From (4.3) and (4.4), we can obtain

$\begin{equation} \begin{aligned} (x\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}y)(n) = \frac{1}{4} (x{\underset{s, s, c, \alpha}{\ast}}y)(n+1)-\frac{1}{4}(x{\underset{s, s, c, \alpha}{\ast}}y)(n-1). \end{aligned} \end{equation}$

(4.5)

The proof is completed. □

Based on , it can be observed that the weighted convolution operation $\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}$ defined in Definition 3.4 can be equivalently represented by the convolution operation ${\underset{s, s, c, \alpha}{\ast}}$ introduced in Definition 3.3.

Figure 1. Convolution relationship between fractional cosine series and fractional sine series.

DownLoad: Full-Size Img PowerPoint

5. Application of the convolution theorems for FRCS and FRSS

The convolution equation is a crucial tool in various applications, including radiation energy transfer, film or shaft vibration problems, as well as the resolution of electrostatic field and magnetic field synthesis along with digital signal processing. The system of convolution equations represented by (5.1) and (5.2) also arises when addressing challenges in engineering mechanics and digital signal processing; thus, making it an active area of research interest. In this section, we will discuss two specific types of convolution equations

$\begin{eqnarray} \left\{\begin{array}{ll} x(n)+\mu_{1}\sum\limits_{m = 0}^{\infty}\widetilde{\eta}(m)y_{11}(n, m) = p(n), \\ y(n)+\mu_{2}\sum\limits_{m = 0}^{\infty}\widetilde{x}(m)\psi_{21}(n, m) = q(n) \end{array}\right. \end{eqnarray}$

(5.1)

and

$\begin{equation} \begin{aligned} z(n)+\mu\left[\sum\limits_{m = 0}^{\infty}\widetilde{\eta}(m)z_{12}(n, m)+\sum\limits_{m = 0}^{\infty}\widetilde{\psi}(m)z_{21}(n, m)\right] = p(n), \end{aligned} \end{equation}$

(5.2)

where

$\begin{equation} \begin{aligned} &y_{11}(n, m) = A_{\varphi}e^{j\frac{n^{2}}{2}\cot\varphi}\biggl[y(|m-n|)-y(m+n)\biggr], \\ &z_{12}(n, m) = A_{\varphi}e^{j\frac{n^{2}}{2}\cot\varphi}\biggl[z(|m-n|)+z(m+n)\biggr], \\ &z_{21}(n, m) = A_{\varphi}e^{j\frac{n^{2}}{2}\cot\varphi}\biggl[z(|m-n+1|) +z(|m+n-1|)-z(|m-n-1|)-z(m+n+1)\biggr], \\ &\psi_{21}(n, m) = A_{\varphi}e^{j\frac{n^{2}}{2}\cot\varphi}\biggl[\psi(|m-n+1|) +\psi(|m+n-1|)-\psi(|m-n-1|)-\psi(m+n+1)\biggr] \end{aligned} \end{equation}$

(5.3)

and

$\begin{equation} \begin{aligned} &\eta (m) = (\eta_{1}{\underset{c, \alpha}{\ast}}\eta_{2})(m), \ \ \psi (m) = (\psi_{1}{\underset{s, s, c, \alpha}{\ast}}\psi_{2})(m), \\ &\widetilde{x}(m) = A_{\varphi}e^{j\frac{m^{2}}{2}\cot\varphi}x(m), \ \ \widetilde{\eta}(m) = A_{\varphi}e^{j\frac{m^{2}}{2}\cot\varphi}\eta(m), \ \ \widetilde{\psi}(m) = A_{\varphi}e^{j\frac{m^{2}}{2}\cot\varphi}\psi(m). \end{aligned} \end{equation}$

(5.4)

where $\mu$ , $\mu_{1}$ , $\mu_{2} \in \mathbb{C}$ , $\widetilde{\eta}$ , $\eta$ , $\eta_{1}$ , $\eta_{2}$ , $\widetilde{\psi}$ , $\psi$ , $\psi_{1}$ , $\psi_{2}$ , $p, q \in \ell_{1}(\mathbb{N})$ , $x$ , $y$ and $z$ are unknown sequences in $\ell_{1}(\mathbb{N})$ , $m, n \in \mathbb{N}$ .

Next, we will utilize the convolution operation along with the corresponding convolution theorem proposed in this article to investigate the solution of the convolution equation. First, we will examine a system of convolution Eq (5.1).

Theorem 5.1. Let $x, y\in \ell_{1}(\mathbb{N})$ , such that

$1-\mu_{1}\mu_{2}e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(\eta\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)\neq0,$

then the system of convolutions Eq (5.1) has a unique solution

$\begin{equation} \begin{aligned} x(n) = &p(n)+(p\underset{s, s, c, \alpha}{\ast}u)(n)-\mu_{1}(\eta{\underset{s, s, c, \alpha}{\ast}}q)(n) -\mu_{1}(\eta{\underset{s, s, c, \alpha}{\ast}}q){\underset{s, s, c, \alpha}{\ast}}u)(n) \end{aligned} \end{equation}$

(5.5)

and

$\begin{equation} \begin{aligned} y(n) = q(n)+(q\underset{c, \alpha}{\ast}u)(n)-\mu_{2}(p\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(n) -\mu_{2}((p\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi){\underset{c, \alpha}{\ast}}u)(n), \end{aligned} \end{equation}$

(5.6)

where $u \in \ell_{1}(\mathbb{N})$ , and the following equality holds

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}u)(w) = \frac{\mu_{1}\mu_{2}S_{c}^{\alpha}(\eta\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)}{1-\mu_{1}\mu_{2}e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(\eta\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)}. \end{aligned} \end{equation}$

(5.7)

Proof. By applying the FRSS and FRCS to both sides of Eq (5.1) and simultaneously utilizing (5.3), we can obtain

$\begin{eqnarray} \left\{\begin{array}{ll} (S_{s}^{\alpha}x)(w)+\mu_{1}e^{-j\frac{w^{2}}{2}\cot\varphi}(S_{s}^{\alpha}\eta)(w)(S_{c}^{\alpha}y)(w) = (S_{s}^{\alpha}p)(w), \\ \mu_{2}e^{-j\frac{w^{2}}{2}\cot\varphi}\sin (\csc \varphi\cdot w)(S_{s}^{\alpha}x)(w)(S_{c}^{\alpha}\psi)(w) +(S_{c}^{\alpha}y)(w) = (S_{c}^{\alpha}q)(w) \end{array}\right. \end{eqnarray}$

(5.8)

from

$1-\mu_{1}\mu_{2}e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(\eta\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)\neq0.$

By applying the Wiener-Levi's theorem ^[34] and (5.7), we can deduce

$\begin{equation} \begin{aligned} (S_{s}^{\alpha}x)(w) & = \frac{(S_{s}^{\alpha}p)(w)-\mu_{1}S_{s}^{\alpha}(\eta{\underset{s, s, c, \alpha}{\ast}}q)(w)} {1-\mu_{1}\mu_{2}e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(\eta\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)}\\ & = ((S_{s}^{\alpha}p)(w)-\mu_{1}S_{s}^{\alpha}(\eta{\underset{s, s, c, \alpha}{\ast}}q)(w))\cdot (1+e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(u)(w))\\ & = (S_{s}^{\alpha}p)(w)+S_{s}^{\alpha}(p{\underset{s, s, c, \alpha}{\ast}}u)(w)-\mu_{1}S_{s}^{\alpha}(\eta{\underset{s, s, c, \alpha}{\ast}}q)(w) -\mu_{1}S_{s}^{\alpha}((\eta{\underset{s, s, c, \alpha}{\ast}}q){\underset{s, s, c, \alpha}{\ast}}u)(w). \end{aligned} \end{equation}$

(5.9)

Hence, we have

$\begin{equation} \begin{aligned} x(n) = p(n)+(p\underset{s, s, c, \alpha}{\ast}u)(n)-\mu_{1}(\eta{\underset{s, s, c, \alpha}{\ast}}q)(n) -\mu_{1}((\eta{\underset{s, s, c, \alpha}{\ast}}q){\underset{s, s, c, \alpha}{\ast}}u)(n). \end{aligned} \end{equation}$

(5.10)

Similarly, we have

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}y)(w) = &\frac{(S_{c}^{\alpha}q)(w)-\mu_{2}S_{c}^{\alpha}(p\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)} {1-\mu_{1}\mu_{2}e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(\eta\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w)}\\ = &((S_{c}^{\alpha}q)(w)-\mu_{2}S_{c}^{\alpha}(p\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w))\cdot (1+e^{-j\frac{w^{2}}{2}\cot\varphi}S_{c}^{\alpha}(u)(w))\\ = &(S_{c}^{\alpha}q)(w)+S_{c}^{\alpha}(q{\underset{c, \alpha}{\ast}}u)(w)-\mu_{2}S_{c}^{\alpha}(p\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(w) -\mu_{2}S_{c}^{\alpha}((p \overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi){\underset{c, \alpha}{\ast}}u)(w); \end{aligned} \end{equation}$

(5.11)

therefore, we can achieve

$\begin{equation} \begin{aligned} y(n) = q(n)+(q\underset{c, \alpha}{\ast}u)(n)-\mu_{2}(p\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi)(n) -\mu_{2}((p \overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi){\underset{c, \alpha}{\ast}}u)(n). \end{aligned} \end{equation}$

(5.12)

The proof is completed. □ The solutions of Eq (5.1) are illustrated in , where the fractional order is $\alpha = 1.14$ and $\mu_1 = \mu_2 = 0.5$ . The functions used in this paper are $p(n) = \sin n$ , $q(n) = n$ , $\eta(n) = \cos n$ and $\psi(n) = n^2$ .

Figure 2. The solution of Eq (5.1). (a)–(d): the input function

$p(n)$ ,

$q(n)$ ,

$\eta(n)$ and

$u(n)$ , respectively; (e): real part of solution

$x(n)$ ; (f): real part of solution

$y(n)$ ; (g): image part of solution

$x(n)$ ; (h): image part of solution

$y(n)$ .

DownLoad: Full-Size Img PowerPoint

The solution to Eq (5.2) will be discussed below.

Theorem 5.2. Let $y\in \ell_{1}(\mathbb{N})$ , such that

$1+\mu e^{-j\frac{w^{2}}{2}\cot\varphi}(S_{c}^{\alpha}(\eta_{1}{\underset{c, \alpha}{\ast}}\eta_{2})(w) +(S_{c}^{\alpha}(\psi_{1}\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi_{2})(w))\neq0,$

then Eq (5.2) has a unique solution

$\begin{equation} \begin{aligned} z(n) = p(n)-(p{\underset{c, \alpha}{\ast}}v)(n) \end{aligned} \end{equation}$

(5.13)

and the following equality holds

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}v)(w) = &\frac{\mu(S_{c}^{\alpha}(\eta_{1}{\underset{c, \alpha}{\ast}}\eta_{2})(w)+S_{c}^{\alpha}(\psi_{1}\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi_{2})(w))}{1+\mu e^{-j\frac{w^{2}}{2}\cot\varphi}(S_{c}^{\alpha}(\eta_{1}{\underset{c, \alpha}{\ast}}\eta_{2})(w)+S_{c}^{\alpha}(\psi_{1}\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi_{2})(w))}. \end{aligned} \end{equation}$

(5.14)

Proof. Applying the FRCS to both sides of Eq (5.2), we have

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}z)(w)(1+\mu e^{-j\frac{w^{2}}{2}\cot\varphi}(S_{c}^{\alpha}(\eta_{1}{\underset{c, \alpha}{\ast}}\eta_{2})(w) +S_{c}^{\alpha}(\psi_{1}\overset{\omega}{\underset{c, s, c, \alpha}{\ast}}\psi_{2})(w))) = (S_{c}^{\alpha}p)(w). \end{aligned} \end{equation}$

(5.15)

Owing to the Wiener-Levi's theorem ^[34], from (5.4) and (5.14), we can derive

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}z)(w) = (S_{c}^{\alpha}p)(w)\cdot(1-e^{-j\frac{w^{2}}{2}\cot\varphi}(S_{c}^{\alpha}v)(w)), \end{aligned} \end{equation}$

(5.16)

hence, we have

$\begin{equation} \begin{aligned} (S_{c}^{\alpha}z)(w) = (S_{c}^{\alpha}p)(w)-S_{c}^{\alpha}(p{\underset{c, \alpha}{\ast}}v)(w). \end{aligned} \end{equation}$

(5.17)

The proof of Theorem 5.2 is completed. □

The solution of Eq (5.2) is also depicted in , where the fractional order is $\alpha = 1.26$ , $p(n) = \sin n$ and $v(n) = n^3$ .

Figure 3. The solution of Eq (5.2). (a)-(b): represent the input function

$p(n)$ and

$v(n)$ , respectively; (c): real part of solution

$z(n)$ ; (d): image part of solution

$z(n)$ .

DownLoad: Full-Size Img PowerPoint

Next, let us analyze the computational complexity of the solution achieved in convolution integral Eqs (5.1) and (5.2) in detail. From (5.5), using the fast Fourier transform (FFT), $(p\underset{s, s, c, \alpha}{\ast}u)(n)$ , $(\eta{\underset{s, s, c, \alpha}{\ast}}q)(n)$ and $(\eta{\underset{s, s, c, \alpha}{\ast}}q){\underset{s, s, c, \alpha}{\ast}}u)(n)$ require $\frac{3}{2}Nlog_2^N$ , $\frac{3}{2}Nlog_2^N$ and $2Nlog_2^N$ real number multiplications, respectively, for a discrete signal of size N. Therefore, the computational complexity of a solution $x(n)$ to Eq (5.1) can be obtained as $O(\frac{15}{2}Nlog_2^N)$ , which is much lower than that of the computational complexity in fractional domain. Similarly, we can obtain the computational complexity of a solution $y(n)$ to Eq (5.1) and a solution $z(n)$ to Eq (5.2) as $O(\frac{15}{2}Nlog_2^N)$ and $O(\frac{5}{2}Nlog_2^N)$ , respectively.

6. Conclusions

The FRCS and the FRSS, which are valuable tools in mathematics and signal processing, were introduced in this paper. Convolution operations for FRCS and FRSS were proposed, along with a detailed derivation of their corresponding convolution theorems. Furthermore, it was demonstrated that our derived results encompassed all classical findings regarding Fourier cosine and sine series as special cases. As an application, explicit solutions for two classes of convolution equations in the FRCS and FRSS domains were explored. Additionally, the computational complexity of solving these equations was also analyzed in detail.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This research was supported by the National Natural Science Foundation of China (62261055 and 61861044); The Natural Science Foundation of Shanxi Province (2023-JC-YB-085 and 2022JM-400).

Conflict of interest

The authors declare there are no conflicts of interest.

References

[1]	I. E. Lager, M. Štumpf, G. A. E. Vandenbosch, G. Antonini, Evaluation of convolution integrals at late-times revisited, IEEE Trans. Antennas Propag., 70 (2022), 9953–9958. https://doi.org/10.1109/TAP.2022.3168347 doi: 10.1109/TAP.2022.3168347
[2]	L. Liu, J. J. Ma, Collocation boundary value methods for auto-convolution Volterra integral equations, Appl. Numer. Math., 177 (2022), 1–17. https://doi.org/10.1016/j.apnum.2022.03.004 doi: 10.1016/j.apnum.2022.03.004
[3]	Y. Xiang, S. Yuan, Q. Feng, Fractional Fourier cosine and sine Laplace weighted convolution and its application, IET Signal Process., 17 (2023), 12170. https://doi.org/10.1049/sil2.12170 doi: 10.1049/sil2.12170
[4]	M. L. Maslakov, New approach to the iterative method for numerical solution of a convolution type equation determined for a certain class of problems, Comput. Math. Math. Phys., 61 (2021), 1260–1268. https://doi.org/10.1134/S0965542521080054 doi: 10.1134/S0965542521080054
[5]	M. S. Gao, J. Yu, Z. F. Yang, J. B. Zhao, Physics embedded graph convolution neural network for power flow calculation considering uncertain injections and topology, IEEE Trans. Neural Networks Learn. Syst., 2023. https://doi.org/10.1109/TNNLS.2023.3287028
[6]	Ö. Civalek, Numerical analysis of free vibrations of laminated composite conical and cylindrical shells: discrete singular convolution (DSC) approach, J. Comput. Appl. Math., 205 (2007), 251–271. https://doi.org/10.1016/j.cam.2006.05.001 doi: 10.1016/j.cam.2006.05.001
[7]	J. González-Camus, C. Lizama, P. J. Miana, Fundamental solutions for semidiscrete evolution equations via Banach algebras, Adv. Differ, Equations, 2021 (2021), 378–410. https://doi.org/10.1186/s13662-020-03206-7 doi: 10.1186/s13662-020-03206-7
[8]	Y. Y. Mei, Q. Feng, X. X. Gao, Y. B. Zhao, Convolution theorem associated with the QWFRFT, Chin. J. Electron., 32 (2023), 485–492. https://doi.org/10.23919/cje.2021.00.225 doi: 10.23919/cje.2021.00.225
[9]	S. B. Yakubovich, Y. F. Luchko, The hypergeometric approach to integral transforms and convolutions, Springer Science & Business Media, 1994. https://doi.org/10.1007/978-94-011-1196-6
[10]	M. Kumar, T. K. Rawat, Design of fractional order differentiator using type-III and type-IV discrete cosine transform, Eng. Sci. Technol., 20 (2017), 51–58. https://doi.org/10.1016/j.jestch.2016.07.002 doi: 10.1016/j.jestch.2016.07.002
[11]	Q. Feng, B. Z. Li, Convolution theorem for fractional cosine-sine transform and its application, Math. Meth. Appl. Sci., 40 (2017), 3651–3665. https://doi.org/10.1002/mma.4251 doi: 10.1002/mma.4251
[12]	V. Guleria, S. Sabir, D. C. Mishra, Security of multiple RGB images by RSA cryptosystem combined with FrDCT and Arnold transform, J. Inf. Secur. Appl., 54 (2020), 102524. https://doi.org/10.1016/j.jisa.2020.102524 doi: 10.1016/j.jisa.2020.102524
[13]	Y. Ding, Z. L. Zhang, X. F. Zhao, D. Hong, W. Li, W. Cai, et al., AF2GNN: graph convolution with adaptive filters and aggregator fusion for hyperspectral image classification, Inf. Sci., 602 (2022), 201–219. https://doi.org/10.1016/j.ins.2022.04.006 doi: 10.1016/j.ins.2022.04.006
[14]	Q. Feng, R. B. Wang, Fractional convolution, correlation theorem and its application in filter design, Signal Image Video Process., 14 (2020), 351–358. https://doi.org/10.1007/s11760-019-01563-9 doi: 10.1007/s11760-019-01563-9
[15]	V. F. García, J. M. Delgado, C. Rodríguez, Exact analytical solution of the convolution integral equation for a general profile fitting function and Gaussian detector kernel, Phys. Med. Biol., 45 (2000), 645–650. https://doi.org/10.1088/0031-9155/45/3/306 doi: 10.1088/0031-9155/45/3/306
[16]	K. Trimeche, Hypoelliptic Dunkl convolution equations in the space of distributions on $\mathbb{R}^d$ , J. Fourier Anal. Appl., 12 (2006), 517–542. https://doi.org/10.1007/s00041-005-5073-y doi: 10.1007/s00041-005-5073-y
[17]	X. X. Gao, Q. Feng, Y. Y. Mei, Discrete convolution associated with fractional cosine and sine series, J. Beijing Inst. Technol., 30 (2021), 305–310. https://doi.org/10.15918/j.jbit1004-0579.2021.040 doi: 10.15918/j.jbit1004-0579.2021.040
[18]	Q. Feng, R. B. Wang, Fractional convolution associated with a class of integral equations, IET Signal Process., 14 (2020), 15–23. https://doi.org/10.1049/iet-spr.2019.0140 doi: 10.1049/iet-spr.2019.0140
[19]	Z. W. Li, W. B. Gao, B. Z. Li, The solvability of a class of convolution equations associated with 2D FRFT, Mathematics, 8 (2020), 1928. https://doi.org/10.3390/math8111928 doi: 10.3390/math8111928
[20]	D. Oliveira e Silva, R. Quilodran, Smoothness of solutions of a convolution equation of restricted-type on the sphere, Forum Math. Sigma, 9 (2021), e12. https://doi.org/10.1017/fms.2021.7 doi: 10.1017/fms.2021.7
[21]	P. R. Li, Existence of solutions for dual singular integral equations with convolution kernels in case of non-normal type, J. Appl. Anal. Comput., 10 (2020), 2756–2766. https://doi.org/10.11948/20200192 doi: 10.11948/20200192
[22]	L. P. Castro, R. C. Guerra, N. M. Tuan, New convolutions and their applicability to integral equations of Wiener-Hopf plus Hankel type, Math. Methods Appl. Sci., 43 (2020), 4835–4846. https://doi.org/10.1002/mma.6236 doi: 10.1002/mma.6236
[23]	Q. Feng, S. Yuan, The explicit solutions for a class of fractional Fourier-Laplace convolution equations, Integral Transforms Spec. Funct., 34 (2023), 128–144. https://doi.org/10.1080/10652469.2022.2093870 doi: 10.1080/10652469.2022.2093870
[24]	P. R. Li, G. B. Ren, Solvability of singular integro-differential equations via Riemann-Hilbert problem, J. Differ. Equations, 265 (2018), 5455–5471. https://doi.org/10.1016/j.jde.2018.07.056 doi: 10.1016/j.jde.2018.07.056
[25]	Z. R. Wang, B. Shiri, D. Baleanu, Discrete fractional watermark technique, Front. Inf. Technol. Electron. Eng., 21 (2020), 880–883. https://doi.org/10.1631/FITEE.2000133 doi: 10.1631/FITEE.2000133
[26]	H. M. Srivastava, W. Z. Lone, F. A. Shah, A. I. Zayed, Discrete quadratic-phase Fourier transform: theory and convolution structures, Entropy, 24 (2022), 1340. https://doi.org/10.3390/e24101340 doi: 10.3390/e24101340
[27]	G. Monegato, On a time discrete convolution-space collocation BEM for the numerical solution of two-dimensional wave propagation problems in unbounded domains, IMA J. Numer. Anal., 43 (2023), 3766–3795. https://doi.org/10.1093/imanum/drac068 doi: 10.1093/imanum/drac068
[28]	H. Tung, N. X. Thao, V. K. Tuan, The $h$ -Fourier sine-Laplace discrete generalized convolution on time scale, Integral Transforms Spec. Funct., 34 (2022), 444–456. https://doi.org/10.1080/10652469.2022.2142788 doi: 10.1080/10652469.2022.2142788
[29]	N. X. Thao, V. K. Tuan, N. A. Dai, Discrete-time Fourier cosine convolution, Integral Transforms Spec. Funct., 29 (2018), 866–874. https://doi.org/10.1080/10652469.2018.1510924 doi: 10.1080/10652469.2018.1510924
[30]	N. X. Thao, V. K. Tuan, N. A. Dai, A discrete convolution involving Fourier sine and cosine series and its applications, Integral Transforms Spec. Funct., 31 (2020), 243–252. https://doi.org/10.1080/10652469.2019.1687467 doi: 10.1080/10652469.2019.1687467
[31]	T. Chen, Fractional-order accumulative generation with discrete convolution transformation, Fractal Fract., 7 (2023), 402. https://doi.org/10.3390/fractalfract7050402 doi: 10.3390/fractalfract7050402
[32]	C. Y. Ma, B. Shiria, G. C. Wu, D. Baleanuc, New fractional signal smoothing equations with short memory and variable order, Optik, 218 (2020), 164507. https://doi.org/10.1016/j.ijleo.2020.164507 doi: 10.1016/j.ijleo.2020.164507
[33]	J. A. D. Appleby, E. Lawless, Solution space characterisation of perturbed linear Volterra integrodifferential convolution equations: the LP case, Appl. Math. Lett., 146 (2023), 108825. https://doi.org/10.1016/j.aml.2023.108825 doi: 10.1016/j.aml.2023.108825
[34]	H. Hochstadt, Integral equations, John Wiley & Sons, Inc., 1973. Avaible from: https://onlinelibrary.wiley.com/doi/pdf/10.1002/9781118165942.fmatter.

This article has been cited by:

1.	Chaoyang Zhu, Mawaheb Al-Dossari, S. Rezapour, B. Gunay, On the exact soliton solutions and different wave structures to the (2+1) dimensional Chaffee–Infante equation, 2024, 57, 22113797, 107431, 10.1016/j.rinp.2024.107431
2.	B. Reddappa, R. Geetha, B. Rushi Kumar, Mehdi Akermi, M. Ijaz Khan, Ilyas Khan, Nonlinear coupled ODEs for nanofluid flow and heat transfer with varying thickness: A comparative study, 2024, 1040-7790, 1, 10.1080/10407790.2024.2333013
3.	Handenur Esen, Aydin Secer, Mir Sajjad Hashemi, Muslum Ozisik, Mustafa Bayram, Examining the (1 + 1)-dimensional Schrödinger–Hirota equation with Kerr effect under inter-modal dispersion using the invariance theory, 2024, 56, 1572-817X, 10.1007/s11082-024-06813-w
4.	Rongbo Wang, Qiang Feng, Weighted Convolution for Quaternion Linear Canonical Cosine Transform and Its Application, 2024, 13, 2075-1680, 402, 10.3390/axioms13060402
5.	Saima Noor, Wedad Albalawi, Rasool Shah, Ahmad Shafee, Sherif M. E. Ismaeel, S. A. El-Tantawy, A comparative analytical investigation for some linear and nonlinear time-fractional partial differential equations in the framework of the Aboodh transformation, 2024, 12, 2296-424X, 10.3389/fphy.2024.1374049
6.	Saima Noor, Wedad Albalawi, Rasool Shah, M. Mossa Al-Sawalha, Sherif M. E. Ismaeel, S. A. El-Tantawy, On the approximations to fractional nonlinear damped Burger’s-type equations that arise in fluids and plasmas using Aboodh residual power series and Aboodh transform iteration methods, 2024, 12, 2296-424X, 10.3389/fphy.2024.1374481
7.	Yingnan Qi, Jalil Manafian, Kamal Mahir Sulieman, Anjan Kumar, Neha Kumari, Rohit Sharma, Mehdi Fazli, Tarek Awad, Multi wave solutions and wave propagation in plasma physics over the Gilson-Pickering equation by modified extended tanh-function method, 2024, 56, 1572-817X, 10.1007/s11082-024-06928-0
8.	Shanyuan Qin, Jidong Yang, Ning Qin, Jianping Huang, Kun Tian, Quasi-P-Wave Reverse Time Migration in TTI Media with a Generalized Fractional Convolution Stencil, 2024, 8, 2504-3110, 174, 10.3390/fractalfract8030174
9.	Jianwen Cheng, Jalil Manafian, Gurpreet Singh, Anupam Yadav, Neha Kumari, Rohit Sharma, Baharak Eslami, Naief Alabed Alkader, Interaction between soliton and periodic solutions and the stability analysis to the Gilson–Pickering equation by bilinear method and $$\exp (-\theta (\alpha ))$$-function approach arising plasma physics, 2024, 56, 1572-817X, 10.1007/s11082-024-06805-w
10.	Saima Noor, Wedad Albalawi, Rasool Shah, M. Mossa Al-Sawalha, Sherif M. E. Ismaeel, Mathematical frameworks for investigating fractional nonlinear coupled Korteweg-de Vries and Burger’s equations, 2024, 12, 2296-424X, 10.3389/fphy.2024.1374452
11.	Lan Lan, Jalil Manafian, Baharak Eslami, A. H. A. Hussein, K. H. Mahmoud, A. S. A. Alsubaie, Anmar Ghanim Taki, Afandiyeva Hajar, Homoclinic and N-soliton solutions to variable-coefficient KP equation arising two-temperature ions in dusty plasma, 2024, 56, 1572-817X, 10.1007/s11082-024-07041-y
12.	A. Kilina, P. Panteleeva, N. Stankevich, Multi-dimensional chaos initiated by short pulses in non-autonomous radio-physical generator, 2024, 135, 10075704, 108041, 10.1016/j.cnsns.2024.108041
13.	Ahmed M. Elsherbeny, Nivan M. Elsonbaty, Niveen M. Badra, Hamdy M. Ahmed, Mohammad Mirzazadeh, M. Eslami, M. S. Hashemi, Mustafa Bayram, Optical solitons of higher order mathematical model with refractive index using Kudryashov method, 2024, 56, 1572-817X, 10.1007/s11082-024-06846-1
14.	M.S. Hashemi, A variable coefficient third degree generalized Abel equation method for solving stochastic Schrödinger–Hirota model, 2024, 180, 09600779, 114606, 10.1016/j.chaos.2024.114606
15.	Naveed Iqbal, Shah Hussain, Muhammad Nazim Tufail, Wael W Mohammed, Solving the fractional Fornberg-Whitham equation within Caputo framework using the optimal auxiliary function method, 2024, 99, 0031-8949, 055234, 10.1088/1402-4896/ad3488
16.	Wafaa B. Rabie, Hamdy M. Ahmed, Mir Sajjad Hashemi, Mohammad Mirzazadeh, Mustafa Bayram, Generating optical solitons in the extended (3 + 1)-dimensional nonlinear Kudryashov’s equation using the extended F-expansion method, 2024, 56, 1572-817X, 10.1007/s11082-024-06787-9
17.	Shanwen Ke, S. Shateyi, Salman A. AlQahtani, Nouf F. AlQahtani, Discovering diverse soliton solutions in the modified Schrödinger’s equation via innovative approaches, 2024, 57, 22113797, 107420, 10.1016/j.rinp.2024.107420
18.	Nan Jiang, Qiang Feng, Xi Yang, Jin-Rong He, Bing-Zhao Li, The octonion linear canonical transform: Properties and applications, 2025, 192, 09600779, 116039, 10.1016/j.chaos.2025.116039

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1396) PDF downloads(119) Cited by(18)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(3)

AIMS Mathematics

The discrete convolution for fractional cosine-sine series and its application in convolution equations

Related Papers:

Abstract

1. Introduction

2. Preliminaries

3. The convolution for FRCS and FRSS

4. Convolution relation of the fractional cosine and sine series

5. Application of the convolution theorems for FRCS and FRSS

6. Conclusions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

The discrete convolution for fractional cosine-sine series and its application in convolution equations

Related Papers:

Abstract

1. Introduction

2. Preliminaries

3. The convolution for FRCS and FRSS

4. Convolution relation of the fractional cosine and sine series

5. Application of the convolution theorems for FRCS and FRSS

6. Conclusions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog