A study of coupled nonlinear generalized fractional differential equations with coupled nonlocal multipoint Riemann-Stieltjes and generalized fractional integral boundary conditions

1.   Introduction

Recent years have witnessed the development of distributed discrete fractional operators based on singular and nonsingular kernels with the aim of solving a large variety of discrete problems arising in different application fields such as biology, physics, robotics, economic sciences and engineering (see for example ^{[1,2,3,4,5,6,7,8,9]}). These operators depend on their corresponding kernels overcoming some limits of the order of discrete operators, for example the most popular operators are Riemann-Liouville and Caputo with standard kernels, Caputo-Fabrizio with exponential kernels, Attangana-Baleanu with Mittag-Leffler kernels (see for example ^{[10,11,12,13]}). We also refer the reader to ^{[14,15,16,17,18]} for discrete fractional operators. Modeling and positivity simulations have been developed or adapted for discrete fractional operators, ranging from continuous fractional models to discrete fractional frameworks; see for example ^[1,19,20]). For other results on positivity and monotonicity we refer the reader to ^{[21,22,23,24,25]} and for discrete fractional models with monotonicity and positivity which is important in the context of discrete fractional calculus we refer the reader to ^{[26,27,28,29]}.

In this work, we are interested in finding positivity and monotonicity results for the following single and composition of delta fractional difference equations:

and

where we will assume that $\mathtt{G}$ is defined on ${\mathbb{N}}_{a}:=\{a, a+1, \ldots\}$, and $\nu$ and $\mu$ are two different positive orders.

The paper is structured as follows. The mathematical backgrounds and preliminaries needed are given in Section 2. Section 3 presents the problem statement and the main results. Conclusions are provided in Section 4.

2.   Mathematical backgrounds and preliminaries

Let us start this section by recalling the notions of discrete delta Caputo-Fabrizio fractional operators that we will need.

Definition 2.1 (see ^[30,31]). Let $\bigl(\Delta \mathtt{G}\bigr)(\mathfrak{t}) = \mathtt{G}(\mathfrak{t}+1)-\mathtt{G}(\mathfrak{t})$ be the forward difference operator. Then for any function $\mathtt{G}$ defined on ${\mathbb{N}}_{a}$ with $a\in {\mathbb{R}}$, the discrete delta Caputo-Fabrizio fractional difference in the Caputo sense and Caputo-Fabrizio fractional difference in the Riemann sense are defined by

and

respectively, where $\lambda = -\frac{\alpha}{1-\alpha}$ for $\alpha\in[0, 1)$, and $\mathsf{B}(\alpha)$ is a normalizing positive constant.

Moreover, for the higher order case when $q < \alpha < q+1$ with $q\geqslant 0$, we have

Remark 2.1. It should be noted that

if $\alpha\in\left(0, \frac{1}{2}\right)$, where (as above) $\lambda = -\frac{\alpha}{1-\alpha}$.

Definition 2.2 (see ^[29,32]). Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ and $\alpha\in[1,2]$. Then $\mathtt{G}$ is $\alpha- {\rm{convex}} \; {\rm{iff}} \; (\Delta \mathtt{G})$ is $(\alpha-1)-$monotone increasing. That is,

3.   Convexity and positivity results

This section deals with convexity and positivity of the Caputo-Fabrizio operator in the Riemann sense (2.2). We first present some necessary lemmas.

Lemma 3.1. Let $\mathtt{G}: {\mathbb{N}}_{a}\to {\mathbb{R}}$ be a function satisfying

and

for $\alpha \in\left(0, \frac{1}{2}\right)$ and $\mathfrak{t}$ in ${\mathbb{N}}_{a+2}$. Then $\bigl(\Delta \mathtt{G}\bigr)(\mathfrak{t})\geqslant 0$, for every $\mathfrak{t}$ in ${\mathbb{N}}_{a+1}$.

Proof. From Definition 2.1, we have for each $\mathfrak{t}\in {\mathbb{N}}_{a+2}$:

Since $\frac{\mathsf{B}(\alpha)}{1-2\alpha} > 0, \, 1+\lambda > 0$ and $\left(_{\ \ \ \ \ a}^{CFC}\Delta^{\alpha}\, \Delta\mathtt{G}\right)(\mathfrak{t})\geqslant 0$ for all $\mathfrak{t}\in {\mathbb{N}}_{a+2}$, then (3.1) gives us

We will now show that $\left(\Delta\mathtt{G}\right)(a+i+1)\geqslant0$ if we assume that $\left(\Delta\mathtt{G}\right)(a+i)\geqslant 0$ for some $i\in {\mathbb{N}}_{1}$. Note from our assumption we have that $\left(\Delta\mathtt{G}\right)(a)\geqslant0$. But then from the lower bound for $(\Delta \mathtt{G})(a+i+1)$ in (3.2) and our assumption we have

where we used $\frac{\lambda}{1+\lambda} < 0$. Thus, the result follows by induction.

Lemma 3.2. Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ and

for $\alpha\in\left(1, \frac{3}{2}\right)$ and $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then $\mathtt{G}$ is monotone increasing, positive and $\left(\frac{1}{2-\alpha}\right)-$convex on ${\mathbb{N}}_{a}$.

Proof. From the definition with $q = 1$ we have

Since $\bigl(\Delta\mathtt{G}\bigr)(a)\geqslant 0$ is given we have

by Lemma 3.1. This implies that $\mathtt{G}$ is a monotone increasing function. Therefore,

and hence $\mathtt{G}$ is positive.

From the idea in Lemma 3.1 we have (here $\lambda = -\frac{\alpha-1}{2-\alpha}$ for $\alpha\in\left(1, \frac{3}{2}\right)$),

Consequently we have that $\mathtt{G}$ is $\left(\frac{1}{2-\alpha}\right)-$convex on the set ${\mathbb{N}}_{a}$.

Lemma 3.3. Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ and

for $\alpha\in\left(2, \frac{5}{2}\right)$ and $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then, Then $\bigl(\Delta^{2}\mathtt{G}\bigr)(\mathfrak{t})\geqslant 0$, for all $\mathfrak{t}\in {\mathbb{N}}_{a}$. Furthermore, one has $\mathtt{G}$ convex on the set ${\mathbb{N}}_{a}$.

Proof. Let $\left(_{\ \ \ \ \ a}^{CFC}\Delta^{\alpha}\, \mathtt{G}\right)(\mathfrak{t}):= \mathtt{F}(\mathfrak{t})$ for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Since $\alpha\in\left(2, \frac{5}{2}\right)$, we have:

for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$, and by assumption we have

Then, using Lemma 3.2, we get

for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Hence, $\mathtt{G}$ is convex on ${\mathbb{N}}_{a}$.

Lemma 3.4. Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ and

and

for $\alpha\in\left(0, \frac{1}{2}\right)$ and $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then $\bigl(\Delta^{2}\mathtt{G}\bigr)(\mathfrak{t})\geqslant 0$, for each $\mathfrak{t}\in {\mathbb{N}}_{a}$.

Proof. For $\mathfrak{t}\in {\mathbb{N}}_{a+1}$, we have

where $\lambda = -\frac{\alpha}{1-\alpha}$. It follows from (3.3) that,

Due to the nonnegativity of $\frac{(1+\lambda)\mathsf{B}(\alpha)}{1-2\alpha}$, from (3.4) we deduce

By substituting $\mathfrak{t} = a+1$ into (3.5), we get

Also, if we substitute $\mathfrak{t} = a+2$ into (3.5), we obtain

By continuing this process, we obtain that $\bigl(\Delta^{2}\mathtt{G}\bigr)(\mathfrak{t})\geqslant 0$ for each $\mathfrak{t}\in {\mathbb{N}}_{a}$ as desired.

Now, we are in a position to state the first result on convexity. Furthermore, three representative results associated to different subregions in the space of $(\mu, \nu)$-parameter will be provided.

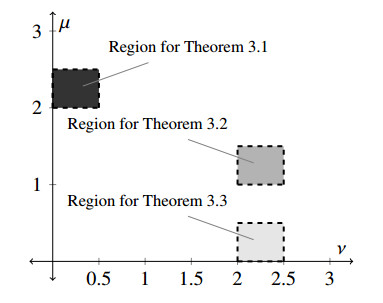
Theorem 3.1. Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ with $\nu\in\left(0, \frac{1}{2}\right)$ and $\mu\in\left(2, \frac{5}{2}\right)$, and

and

for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then $\mathtt{G}$ is convex on the set ${\mathbb{N}}_{a}$.

Proof. Let $\left(_{\ \ \ \ \ a}^{CFC}\Delta^{\mu}\, \mathtt{G}\right)(\mathfrak{t}):= \mathtt{F}(\mathfrak{t})$ for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then, by assumption we have

for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. From the definition with $q = 2$ we have

where $\lambda_{\mu} = -\frac{\mu-2}{3-\mu}$. Since $\bigl(\Delta^{2}\mathtt{G}\bigr)(a)\geqslant 0$, we find that $\bigl(\Delta^{2} \mathtt{G}\bigr)(\mathfrak{t})\geqslant 0$ for each $\mathfrak{t}\in {\mathbb{N}}_{a}$. Furthermore, we see that $\mathtt{G}$ is convex on ${\mathbb{N}}_{a}$ from Lemma 3.3.

Theorem 3.2. Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ with $\nu\in\left(1, \frac{3}{2}\right)$ and $\mu\in\left(2, \frac{5}{2}\right)$, and

and

for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then $\mathtt{G}$ is convex on ${\mathbb{N}}_{a}$.

Proof. Let $\mathtt{F}(\mathfrak{t}):= \left(_{\ \ \ \ \ a}^{CFC}\Delta^{\mu}\, \mathtt{G}\right)(\mathfrak{t})$. Note that:

for $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then we have

and

where $\lambda_{\mu} = -\frac{\mu-2}{5-2\mu}$. On the other hand, one has

Then, from Eqs (3.6)–(3.8), we see that $\mathtt{F}(a+2)\geqslant \mathtt{F}(a+1)\geqslant 0$. Therefore, Lemma 3.2 gives

for all $\mathfrak{t}$ in ${\mathbb{N}}_{a+1}$. Moreover, by considering $\bigl(\Delta^{2}\mathtt{G}\bigr)(a)\geqslant 0$ in Lemma 3.3, we can deduce that $\mathtt{G}$ is convex on the set ${\mathbb{N}}_{a}$.

Theorem 3.3. Let $\mathtt{G}$ be defined on ${\mathbb{N}}_{a}$ with $\nu\in\left(2, \frac{5}{2}\right)$ and $\mu\in\left(0, \frac{1}{2}\right)$, and

and

for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then we have that $\mathtt{G}$ is convex on ${\mathbb{N}}_{a}$.

Proof. Again, we write $\mathtt{F}(\mathfrak{t}):=\left(_{\ \ \ \ \ a}^{CFC}\Delta^{\mu}\, \mathtt{G}\right)(\mathfrak{t})$, and therefore, $\left(_{a+1}^{CFC}\Delta^{\nu}\, \mathtt{F}\right)(\mathfrak{t}) \geqslant 0$ by assumption, for each $\mathfrak{t}\in {\mathbb{N}}_{a+1}$. Then, we see that

where $\lambda_{\mu} = -\frac{\mu}{1-\mu}$. It follows that,

for each $\mathfrak{t}\in {\mathbb{N}}_{a}$ by Lemma 3.3. Considering, $\bigl(\Delta^{2}\mathtt{G}\bigr)(a)\geqslant 0$, we can deduce that $\mathtt{G}$ is convex on ${\mathbb{N}}_{a}$ by Lemma 3.4.

In Figure 1, we demonstrate the regions of the $(\mu, \nu)$-parameter space in which the above three Theorems 3.1–3.3 are applied.

4.   Conclusions

In this study, we present some new positivity results for discrete fractional operators with exponential kernels in the sense of Caputo. In particular new positivity, $\alpha-$convexity and $\alpha-$monotonicity were presented. We now refer the reader to observations for discrete generalized fractional operators in ^[33] which combined with this paper may motivate future work.

Author's contributions

Conceptualization, P.O.M., D.O., A.B.B. and D.B.; methodology, P.O.M., D.O.; software, D.O., D.B., K.M.A., A.B.B.; validation, P.O.M., D.O., D.B. and A.B.B.; formal analysis, K.M.A.; investigation, P.O.M., D.O., K.M.A.; resources, A.B.B.; writing-original draft preparation, P.O.M., D.O., D.B., K.M.A., A.B.B.; writing-review and editing, D.O., D.B. and A.B.B.; funding acquisition, D.B. and K.M.A. All authors read and approved the final manuscript.

Acknowledgements

This Research was supported by Taif University Researchers Supporting Project Number (TURSP-2020/217), Taif University, Taif, Saudi Arabia.

Conflict of interest

The authors declare that they have no conflicts of interest.