1.
Introduction
At present, the fractional calculus field is extensively developed to support and describe a wide variety of complex real-world phenomena. This filed, which is dated back to the 17th century, is a standard extension of the well-known formal calculus in which it can convert the integer-order integrals and derivatives into their fractional-order cases to gain additional degrees of freedom [1,2]. At the end of the 19th century, many theoretical and applied contributions were included to improve the knowledge and the perceptions of such field (see e.g.: [3,4,5,6]). In particular, the theory of fractional calculus was mostly established through several research papers conducted by Marchaud [7,8], Grünwald [9], Letnikov [10,11], Riemann [12], and Liouville [13]. In the past few decades, the fractional calculus has confirmed its significant role and its contribution in applied sciences such as cosmic rays, plasma dynamics, quantum mechanics, electrodynamics, thermodynamics, porous media, continuum mechanics, and rheology [14,15].
The Fractional-order Differential Equations (FoDEs) are undoubtedly considered the core of the fractional calculus. From this standpoint, numerous widely known approaches for offering approximate solutions to the traditional differential equations are numerically developed to meet solving the FoDEs. Adomian decomposition method [16], perturbation method [17], extrapolation method [18], homotopy analysis method [19], homotopy Sumudu transform method [20], and variational iteration method [21] are some of these numerical approaches. Different fractional-order derivative/integral operators are employed for expressing the FoDEs such as the Riemann-Liouville operators [12], the Atangana-Baleanu operators [1,22], the Caputo operators [23], the Caputo-Fabrizio operators [24], the Hadamard operators [25], the Caputo-Hadamard operators [26], the generalized Caputo type non-classical derivative [27], and many others. In fact, the most notable definitions among these operators are those given by Reimann-Liouville, Caputo, and recently those given by Caputo-Fabrizo and Atangana-Baleanu type fractional-order operators. Such operators have their characteristics in using. Since in the past two decades, Caputo operator has adopted mostly to handle several real-life models [27,28]. Therefore by using fractional-order differential operator, we state that in the fields of continuous-time modeling, various researchers have pointed out that fractional-order derivative operators are better tools in describing acoustics, rheology, polymeric chemistry, linear viscoelasticity, and many more such type of sciences [27,28]. Thus, establishing an appropriate numerical formula to introduce an accurate approximation of any of these operators is deemed necessary to solve these FoDEs. Given this pioneering idea, the discretization of the fractional-order integral operators and/or the fractional-order derivative operators will undoubtedly yield a series of quadrature formulae, as can be seen in the Grünwald-Letnikov approach. It has been recently reported in [15] that once the coefficients and nodes of these formulae are varied, different accuracies will be gained. For instance, several numerical approaches that depend on the order of differentiation and integration can be established in view of the so-called polynomial interpolation. Besides, the convolution quadratures can be also considered as other numerical approaches used for approximating the operators at hand [15]. In references [29,30,31], some (3−α)-order formulae were established for approximating the Caputo fractional-order operator through implementing a higher-order interpolant instead of the linear interpolant. This operator with order (3−α) was approximated by using a three-point L1 approach in [32], and also by using a high-order algorithm with convergence order (4−α) in [33]. In [6], a numerical algorithm for approximating the Caputo operator of a function was proposed and verified by taking some applications. Recently, Mokhtari and Mostajeran in [34] have introduced a new different (4−α)-order technique for approximating the Caputo operator. They have also implemented it on some schemes in order to solve some FoDEs numerically [34]. In view of all these pioneer works, we can report that if a continuous function can be expressed using an explicit formula, the Caputo fractional-order derivative operator will be computed. Therefore, throughout this work, we will attempt to propose new explicit formulae for approximating the Caputo fractional-order derivative operator with the help of the Weighted Mean Value Theorem (WMVT).
The remainder of this paper is structured as follows: Section 2 provides some basic definitions. Section 3 presents a robust power series formula with its error term for approximating the Caputo fractional-order operator. Section 4 illustrates some applications to verify the proposed formula. Section 5 summarizes the key conclusions of this paper.
2.
Basic definitions and preliminaries
Non-integer calculus is based on the concept of non-integer derivative and even non-integer integral. The Caputo differential operator, as one of the most significant operators of the fractional derivatives, meets all mathematical basics that lie under umbrella of fractional calculus. In the following content, some definitions and properties related to the operator at hand will be demonstrated for completeness. Let us, firstly, assume that [a,b] is a finite interval, where a,b∈R and −∞<a<b<∞. In this regard, when a function belongs to the class of absolutely continuous functions, then we define the left-sided Caputo fractional-order derivative operator of order α∈R+ as [35]:
On the other hand, the right-sided Caputo fractional-order derivative operator of order α∈R+ is defined as:
Although the formulas (2.1) and (2.2) are critically significant in fractional calculus, only (2.1) is commonly employed in calculus. Actually, it is for this reason that we have narrowed our focus to the formula (2.1). So, from now on we will only adopt this formula in the rest of this work, and we will assume a=0 to simplify the symbol Dαa(⋅) to be as Dα(⋅). Besides, in connection with the operator at hand, it should be mentioned its inverse operator, the Riemann-Liouville fractional-order integral operator, which has the form [35]:
where α∈R+, m∈N. Next, certain significant properties related to the Caputo differential operator are stated for more clarification.
Lemma 2.1. [35] Let m−1<α<m, m∈N, α∈R+ and f(t) be such that Dαf(t) exists, then we have:
Lemma 2.2. [35] Let m−1<α<m, m∈N, α∈R+ and f(t) be such that Dαf(t) exists, then we have:
● limα→mDαf(t)=f(m)(t),
● limα→m−1Dαf(t)=f(m−1)(t)−f(m−1)(0).
Lemma 2.3. [35] Let m−1<α<m, m∈N and α,λ∈C. Let the two functions f(t) and g(t) be such that Dαf(t) and Dαg(t) exist. Then the Caputo fractional-order derivative is a linear operator. That is,
Lemma 2.4. [35] Let m−1<α<m, m,n∈N, α,λ∈R+ and the function f(t) be such that Dαf(t) exists. Then we have:
The variable-order fractional derivative is one of the operators of interest; for additional information, see [36,37]. The variable-order fractional derivative, in the sense of Caputo, is defined as
and
3.
Theoretical study
This part intends to introduce a power series formula to approximate the Caputo operator when its fractional-order is α∈(m−1, m), where m∈N, see [38].
Theorem 3.1. Let y∈Cn+m[a,b], m−1<α<m; where m,n∈N, and a≥0, then for every t∈(a,b], there exist ξ∈(a,b) such that the Caputo fractional-order operator can be written in terms of a fractional-order series and its reminder term as follows:
Proof. By applying the integration by parts to (2.1), we obtain:
If one applies the integration by parts n-times to the second part of (3.2), then the following assertion will be obtained:
That is,
Again, since y∈Cm+n[a,b] and due to the term (t−x)m−α+n−1 does not change its sign on [a,t], and also by the WMVT, we conclude that there exist ξ∈(a,t) such that:
This leads us, consequently, to the desired result.
Corollary 3.2. Let y∈Cn+m[a,b], m−1<α(t)<m; where m,n∈N, and a≥0, then for every t∈(a,b], there exist ξ∈(a,b) such that the variable-order fractional operator given in (2.7) can be written in terms of a fractional variable-order series and its reminder term as follows:
Remark 3.3. In view of Theorem 3.1, if y∈C∞[a,b] and if |y(n)(t)| is bounded for all n∈N, then the power series:
is convergent for any t∈[a, b] and m−1<α<m, where m∈N.
Moreover,
The next corollary is a special case of Theorem 3.1 when m = 1.
Corollary 3.4. Let y∈Cn+1[a,b], 0<α<1, and a≥0, then for every t∈(a,b] there exist ξ∈(a,b) such that the Caputo fractional-order operator Dαay(t) can be written in terms of a fractional-order series and its reminder term as follows:
4.
Illustrative numerical applications
In this section, some numerical examples are taken into account to validate the proposed power series formula. The first example is formulated to offer an accurate approximation for the Caputo fractional-order derivative of a certain function, while the remain examples address some linear and nonlinear FoDEs.
Example 4.1. Given the function y(t)=5t3−2t+4cos(2t+3)+e2t+1. Through using Mathematica software package, we obtain results presented in Table 1 that illustrates different approximations for Dαy(t) according to various values of α using formula (3.6). For example, if a=0, α=1.4, m=2, and n=15, the approximated fractional derivative function is
Based on the above results, one may observe that the error terms can be reduced by increasing the value of n or by reducing the error in the round of error that appears in the calculations.
From now on, we intend to address some linear and nonlinear FoDEs. In particular, we attempt to offer accurate approximations for FoDEs using (3.6) to estimate the Caputo operator. For this purpose, we first suppose:
Then, we set Dαay(t) as:
Consequently, we substitute the above two relations (4.2) and (4.3) into the required FoDE to generate a system of linear or nonlinear equations, which can be solved by many methods. However, this manner can be further generalized to generate a proper power series formula for handling linear and nonlinear FoDEs having several variables with high accuracy results (see [39]).
Example 4.2. Consider the following linear FoDE [40]:
Note that the exact solution of (4.4) when a=0 is: y(t)=t2−t. To solve (4.4), D1/2y(t) can be replaced by the formula (3.6), we have
For α=1/2 and by equating the coefficients of tj in (4.5) yields: y′(0)=−1,y″(0)=2, and y(n)(0)=0, for n=3,4,....
Hence, the solution can be written in term of the power series y(t)=∑∞k=0y(k)tkk!=−t+t2, which coincides with the exact solution.
Example 4.3. Consider the following nonlinear FoDE:
In order to solve this nonlinear problem, we first use the formula (3.6) in the left hand side of (4.6) and taking the Taylor series for the right hand side about t=0 where y(0)=1, yields:
Equating the coefficient of t1−α in (4.7) yields:
Again, equating the coefficient of t2−α in (4.7) then substituting the previous value of y′(0) yields:
In the same manner, we can find y‴(0), y(4)(0), .... Consequently, the general solution can be written as:
and
Hence, the general solution for (4.6) in the form of power series would be in the following form:
Moreover, the power series solution would be consequently as:
In particular, the solution of (4.6) when α=1 using formula (4.8) in the power series form would be:
which coincides with the exact solution of (4.6) when α=1.
Example 4.4. Consider the following nonlinear Riccati FoDE:
The exact solution of (4.10) when α=1, a=0, and b=0 is:
In order to solve (4.10), we first use formula (3.6) in the left hand side of (4.10) and taking the Taylor series for the right hand side about t=a. That is,
Equating the coefficient of t1−α in (4.11) yields:
By equating the coefficient of t2−α in (4.11) and substituting the previous value of y′(a) we get:
In the same manner, we can find y‴(a), y(4)(a), .... Consequently, the general solution can be written as:
and
Hence, the general solution of (4.10) in the form of power series would be in the following form:
In addition, the solution when y(a)=0 would be:
In particular, the solution of (4.10) when a=0, b=0, and α=1 using formula (4.12) in the power series form would be as follows:
which coincides with the exact solution of (4.10) when a=0, b=0, and α=1.
Example 4.5. Consider the following nonlinear FoDE:
In order to solve (4.14), we first suppose that y(t)=∑ni=0c(i)ti, then we use formula (3.6) into the left hand side of (4.14) to generate a new equation with the unknowns ci,i=1,2,...,n:
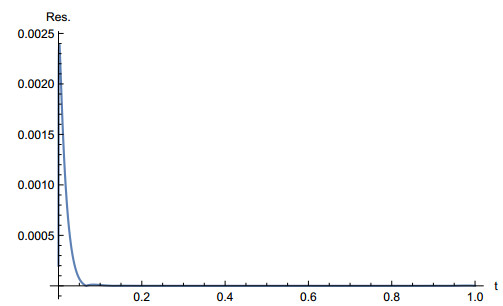
then substitute different values of t=0.1,0.2,...,1 into the resulting equation to generate a nonlinear system that can be solved numerically. The solution and the residual errors obtained are shown in Table 2, and in Figures 1 and 2 where the residual error is given by:
Example 4.6. Consider the nonlinear Riccati differential equation:
The exact solution for α=1 is given by
To solve (4.15), suppose that
then set Dαy(t) to be as:
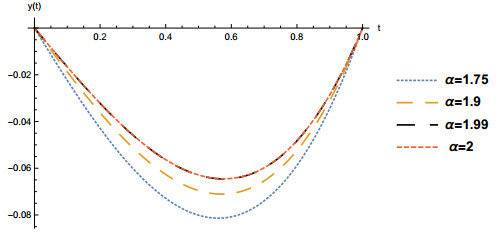
By substituting (4.16) and (4.17) into the Ricatii equation (4.15) for different values of t, we generate a system of nonlinear equations which solved numerically. However, the numerical solutions and the residual error for different values of α are given in Table 3. The result shows that our numerical solution converges to the exact solution as α approaches 1. Table 4 shows a comparison results for different values of α and as α approaches 1 with the results given in [41].
5.
Conclusions
In this paper, an efficient power series formula with its error term for approximating the Caputo fractional-order derivative operator has been introduced with the help of the weighted mean value theorem. It has been shown that this formula can be used to solve many linear and nonlinear problems in fractional calculus as exhibited in several examples taken into account.
Acknowledgments
We are grateful to the anonymous referee for his or her useful remarks that have improved the manuscript's quality.
Conflict of interest
The authors declare that they have no competing interests.









 DownLoad:
DownLoad: